Abstract
Fano resonance which describes a quantum interference between continuum and discrete states, provides a unique method for studying strongly interacting physics. Here, we report a Fano resonance between dark excitons and zone-edged acoustic phonons in few-layer WS2 by using the resonant Raman technique. The discrete phonons with large momentum at the M-point of the Brillouin zone and the continuum dark exciton states related to the optically forbidden transition at K and Q valleys are coupled by the exciton-phonon interactions. We observe rich Fano resonance behaviors across layers and modes defined by an asymmetry-parameter q: including constructive interference with two mirrored asymmetry Fano peaks (weak coupling, q > 1 and q < − 1), and destructive interference with Fano dip (strong coupling, ∣q∣ < < 1). Our results provide new insight into the exciton-phonon quantum interference in two-dimensional semiconductors, where such interferences play a key role in their transport, optical, and thermodynamic properties.
Subject terms: Two-dimensional materials, Two-dimensional materials, Raman spectroscopy
Here, the authors investigate the Raman spectra of few-layered WS2 when the excitation energy is in resonance with the dark exciton, and observe a Fano resonance between dark excitonsand zone-edge acoustic phonons.
Introduction
Resonance quantum interference is a general phenomenon, which strongly affects the electronic transport, optical, and vibronic properties of materials1–4. As one of the most representative phenomena of quantum interferences, Fano resonance describes interference between continuum states and discrete states, making it an ideal platform for studying the strongly interacting physics1,2,5, such as the magnetization and electronic polarization6–8, resonant electromagnetic effects9, and exciton-phonon interactions (EPIs)10–16. In particular, Fano resonance Raman scattering induced by EPIs provides a powerful tool to reveal underlying physics in solid materials6,12,13.
Recently, layered transition metal dichalcogenides (TMDs) and their heterostructures have received much attention due to their novel properties17–19. In these TMDs semiconductors, electronic energy band splitting induced by the spin-orbit coupling and multiple valleys (energy extrema) at different positions of the Brillouin zone form an abundance of exciton states17, which provides an ideal playground for studying the resonance quantum interferences between excitons, photons and other quasiparticles such as phonons. Moreover, these valley features associated with the band splitting support optically-forbidden excitons, i.e., spin- and momentum-forbidden excitons, the so-called dark excitons20–22, which play essential roles in their optoelectronic properties. The spin-forbidden dark excitons can be observed by measuring the photoluminescence spectrum with a giant in-plane magnetic field23–27, in-plane detection28. The momentum-forbidden dark excitons can be detected by time-resolved exciton diffusion29, or by directly imaging it in the momentum space by time-resolved angle-resolved photoemission spectroscopy (ARPES)30. These experiments show the dominant role of phonon-exciton scattering29,30. However, the response of phonons under such dark exciton-phonon interactions in these semiconductors remains largely unexplored. Moreover, the quantum interference between acoustic phonons and excitons in few-layer TMDs shows significant effects on their electrical transport properties31 and their optical properties, such as the exciton/valley dynamics32–36. Therefore, it is important to study the dark excitons-acoustic phonons interactions/interferences in few-layer TMDs semiconductors.
In this work, we experimentally observed the dark exciton in bilayer WS2 and studied the quantum interference between zone-edged acoustic phonon modes (the acoustic branches extending to the M-point, i.e., out-of-plane acoustic (ZA(M)), transverse acoustic (TA(M)), and longitudinal acoustic (LA(M)) modes) and dark excitons in a few-layer WS2 semiconductor. We found that the coupling strength of these zone-edged acoustic phonons varies from weak coupling (constructive interference with a Fano peak) to strong coupling (destructive interference with a Fano dip) across layers and modes. We further revealed the symmetry roles on the quantum interference processes between dark excitons and phonons.
Results
Quantum interference and dark excitons
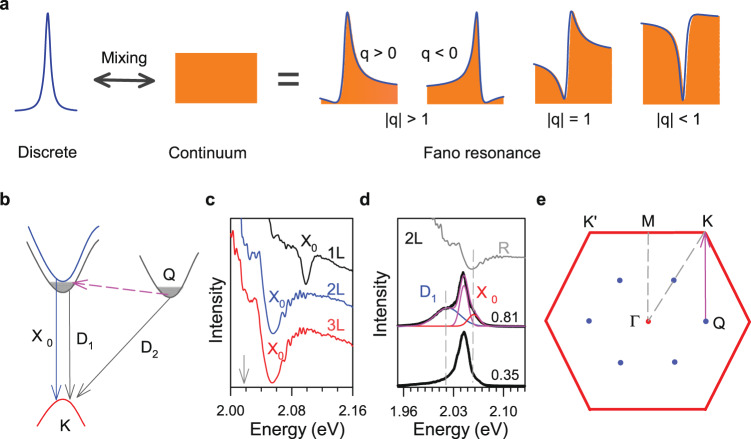
Figure 1 a shows the schematic diagram of the quantum interference between a discrete state and a continuum state, resulting in an asymmetry profile. This asymmetry profile can be described by the coupling term between these two states5,6,37:
| 1 |
where I0, γ, and ω0 are the intensity, the linewidth, and the frequency of the uncoupled discrete state respectively. Γ is the linewidth parameter. V is the matrix element for the interaction between discrete and continuum states, and ρ(E) is the density of continuum states. Tp and Te are the scattering amplitude of the electronic continuum and decoupled discrete state, R(E)/π term is the Hilbert transform of ρ(E). q is an asymmetry parameter, which gives the coupling strength and electronic information in varied materials7,11,38. The positive and negative q correspond to different phase shifts (or relative energy shifts) of the two states, resulting in two mirrored asymmetry lineshape (as shown in Fig. 1a). Generally, ∣q∣ = 1 means dispersion with comparable phonon and electron contribution; ∣q∣ > > 1 and ∣q∣ < < 1 demonstrate a constructive and destructive dominated quantum interference process between discrete and continuum states6, corresponding to the weak and strong coupling, respectively. Thus, a symmetric Lorentzian peak represents a negligible coupling case (∣q∣ ∝ ∞), while a Fano dip represents a strong coupling case (∣q∣ < < 1). Figure 1b shows a schematic diagram of the bright A exciton (X0), the dark exciton (D1), and the dark Q valley excitons (D2) transition. We should note that the K and Q valleys of few-layer WS2 are close to each other in energy39. Figure 1c shows the reflection spectra of 1-3L WS2 at 4K and Fig. 1d shows the PL spectrum of a hexagonal boron nitride (hBN) encapsulated bilayer WS2 excited/collected by two objectives with different numerical apertures (NA = 0.81 and 0.35) respectively. The high NA objective enables the detection of dark exciton (D1) transition even at the normal incidence28, as shown in Fig. 1d. We find that the dark state (~2.02 eV) lies below the bright state around 37 ± 2 meV, consistent with previously reported results in the monolayer case23,27,28.
Fig. 1. Fano-typed quantum interference and dark excitons.
a Schematic diagram of Fano interferences between a discrete state and a continuum state. b A transition schematic diagram of the bright exciton (X0), spin-forbidden dark exciton (D1), and momentum-forbidden dark exciton (D2). c Reflection spectra of 1-3L WS2 (S1) at 4 K. d PL spectra of hexagonal boron nitride encapsulated bilayer WS2 (S2) under a high numerical aperture (NA = 0.81) and a low numerical aperture (NA = 0.35) objective respectively. The bright exciton energy position is normalized to the exciton absorption energy of S1 (the gray line) to eliminate the small difference between two samples. The data are offset for clarity. e The first Brillouin zone of few-layer WS2. The wave vector of ΓM is equal to QK.
The long lifetime of dark exciton both at K and Q valley30,40, promotes the accumulation of these Bose particles to form a bounded quasi-continuum state upon photoexcitation when considering the time scale of exciton-phonon interactions41,42. Therefore, this is a favorable situation to evidence the quantum interference between these dark exciton continuum states and phonons. Considering the momentum conservation, the transition of dark Q valley excitons needs the assistance of phonons. Meanwhile, under the non-resonant excitation case, only the phonon modes at the Γ point can be detected due to the same reason. The phonon scattering process mediated by defects or bounded exciton states can overcome such a momentum mismatch, making it possible to observe the first-order phonon modes at non-Γ point by Raman scattering14,43–45. We note that the wave vector of ΓM is equal to QK in the first Brillouin zone of WS2, see Fig. 1e. It suggests that the phonon modes at the M point can be directly observed with the mediation of scattering K valley excitons to Q valley. Considering the splitting of the valence band, a similar picture applies to B excitons as well, see Supplementary Fig. S1 in Supplementary Information (SI).
Observation of quantum interference in WS2
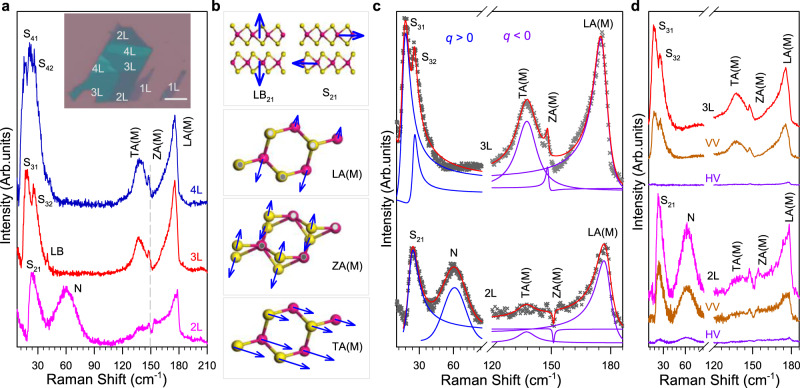
The few-layer WS2 samples are exfoliated mechanically from their bulk crystals onto SiO2/Si substrate, as shown in the inset in Fig. 2a. Their number of layers is determined by using the optical contrast and ultralow frequency Raman spectroscopy46, as shown in Supplementary Fig. S2. The measurements presented in Fig. 1d have been obtained with a WS2 bilayer encapsulated in hBN, which is prepared by using a standard dry transfer method. This yields a significant reduction of the inhomogeneous broadening47. As a consequence, the dark exciton D1 transition lying close to the bright one can be evidenced. Because the photoluminescence background signal of monolayer WS2 is too strong to obtain an available Raman signal when the excitation energies are close to the A exciton states, we focus our attention on bilayer (2L) to quadra-layer (4L) WS2. Figure 2a shows the low-frequency (10 cm−1 to 210 cm−1) Raman spectra of 2-4L WS2 with 612 nm (2.026 eV) excitation at 4 K. Figure 2b shows the vibration way of shear and layer breathing modes of 2L-WS2, and TA(M), ZA(M), and LA(M) modes (see more detailed vibration schematic of these modes in SI). Figure 2c shows the fittings of the major peaks of 2-3L WS2. We note that the excitation energy (2.026 eV) is close to the dark A exciton (D1). Under this condition, the shear modes in 2-4L WS2 are greatly enhanced and featured with an asymmetry Fano profile, whereas the layer breathing modes are weak and featured with a Lorentzian profile. The observed Fano profile of shear modes is due to the quantum interference between shear phonons and dark A excitons14,16.
Fig. 2. Observation of quantum interference in few-layer WS2.
a Raman spectra of 2-4L WS2 at 4 K. Shear and layer breathing modes are labeled as Smi and LBmi, where m and i represent layer numbers and the ith modes, respectively46. N denotes a new peak. The inset shows the optical microscopy image of the few-layer WS2 sample (S1). The scale bar is 10 μm. b Illustration of the vibration of the layer breathing (LB21), shear (S21) modes of 2L-WS2 and TA(M), ZA(M), and LA(M) modes (see more detailed schematics in SI). c The fitting results of shear and zone-edged acoustic modes in 2L- and 3L-WS2. (d) Polarized Raman spectra of 2L- and 3L-WS2 at 4 K. The excitation wavelength is 612 nm (2.026 eV). V (H) denotes the vertical (horizontal) polarization. VV and HV indicate parallel and cross-polarization configuration, respectively. The data are offset for clarity.
Besides these optical phonon modes at Γ point, three zone-edged acoustic phonon modes at M point, i.e., TA(M) (B1g symmetry), ZA(M) (B3g symmetry), and LA(M) (Ag symmetry) modes48, are also observed in 2-4L WS2. Remarkably, ZA(M) and LA(M) modes also show a Fano profile, which is mirrored with that for shear modes. In particular, the Fano coupling strength of ZA(M) and LA(M) modes varies across layers and modes, which is different from that for shear modes. Specifically, the ZA(M) mode in the 2L case behaves as a Fano dip (∣q∣ < 1), unlike a Fano peak for other modes and layers (see Fig. 2a).This Fano dip can be understood by considering the electromagnetically induced transparency (EIT), which is a result of destructive Fano interference among different transition pathways49–51 and occurs at ωlight - ωphonon = ωexciton. Specifically, in our case, the valence band together with the conduction band in the K valley and Q valley form an equivalent three-level system here, eliminating the absorption due to the quantum interference between ZA(M) phonon and continuum dark states. As a result, when near the condition ωlight − ωphonon = ωexciton, the destructive interference gives a narrow transparency window, i.e., the Fano dip. In 3L(4L)-WS2, the condition above is broken may be attributed to the slight Q valley shift induced by the additional layers52,53 and thus, giving peaks (instead of dips).
As discussed above, the observed three zone-edged acoustic phonons: TA(M), ZA(M), and LA(M) modes, can be explained by considering the bounded dark Q excitons mediated phonon scattering process (Fig. 1b, e). These bounded dark Q valley excitons (D2) form a quasi-continuum state, similarly to the dark excitons (D1) in the K valley. As a result, the quantum interference between dark K/Q excitons continuum and zone-edged acoustic phonon modes leads to the observation of the Fano profile (see more detailed discussions and schematic of phonon-involved scattering process in Supplementary Fig. S3). The mirrored Fano profiles between shear phonon at Γ point (q > 0) and zone-edged acoustic modes at M point (q < 0), suggest the different phase shift (or relative energy shift) of two interference states. Specifically, the shear phonons (at Γ point) are mainly involved in intravalley dark exciton scattering processes, whereas the zone-edged acoustic phonons are mainly involved in intervalley exciton scattering processes (higher level K excitons and lower level Q excitons), as shown in Fig. S3. Meanwhile, the phase of phonons at Γ point changes by π relative to that at M point also can lead to this mirrored feature.
Additionally, we find a new peak (N) at ~60 cm−1 only in 2L WS2 at 4 K. This N mode can be observed in all 2L WS2 regions, and thus, the defect-induced phonon modes and localized excitons can be excluded. To further confirm the origin of this peak, more studies are required in the future. Figure 2d shows the polarized-Raman spectra of 2-3L WS2 at 4 K. We find that all Raman modes almost vanish under cross-polarization configuration (HV) (although these in-plane shear modes should survive under the normal case, see more discussion in SI). This result can be understood due to the breakdown of Raman selection rules by the Fröhlich interaction between dark excitons and phonons15.
Excitation energy dependence of quantum interference
When the excitation energies are away from the D1, the intensity of zone-edged acoustic phonons is much weaker than that with 612 nm excitation, as shown in Fig. 3 and Supplementary Figs. S4–S7). For example, when the excitation energy (2.086 eV, 594 nm) is slightly higher than the energy of X0, the Fano resonance vanishes. Figure 3c shows the resonant profile of shear and LA(M) mode of 2L WS2. We find that the resonant peak is close to the dark exciton D1, different from that for A1g (see Fig. S4 in SI). These results can be explained by considering that, when the excitation energies are higher than X0, i.e., above optical bandgap excitation, instead of scattering K excitons to Q valley through zone-edged acoustic phonons, there are more relaxation channels for excitons/electrons to Q valley without the assistance of zone-edged acoustic phonon30. Similarly, when the laser energy (1.96 eV, 633 nm) is slightly below dark exciton D1, the Fano resonance also disappears due to the off-resonance. For these excitation energy far away from the exciton energies, e.g., 2.331 eV, the intensity of these shear and zone-edged acoustic modes is weak, as shown in Fig. 3. These results prove that the continuum states in the quantum interference are indeed from dark excitons. Meanwhile, the power-dependent measurements suggest these processes correspond to first-order Raman scattering, as shown in Supplementary Fig. S8 (see more discussions in SI).
Fig. 3. Excitation energy dependence of quantum interference.
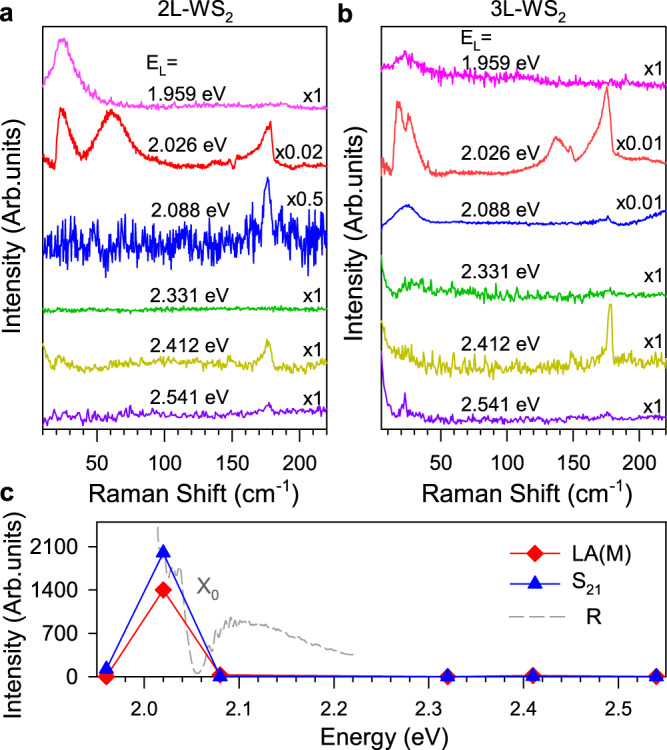
a, b Raman spectra of 2L- and 3L WS2 under different excitation energies (EL) at 4 K, respectively. The intensities are normalized to the Raman modes of silicon substrate (524 cm−1 at 4 K). The data are offset for clarity. c The intensity of shear and LA(M) modes respect to the excitation energies. The dotted gray line is the reflection spectrum of 2L-WS2.
Temperature dependence of quantum interference
Figure 4 shows the temperature-dependent Raman spectra of 2-3L WS2 with 612 nm excitation. The Fano dip of ZA(M) mode in 2L WS2 nearly vanishes at around 100 Kelvin (K), and the Fano peak of ZA(M) and LA(M) modes almost disappeared at around 160 K. These results can be well explained by considering that the WS2 exciton energy decreases when the temperature increases (see Supplementary Fig. S9). Therefore, at higher temperatures, the off-resonance with dark excitons will destroy such Fano resonance processes. On the other side, although X0 is getting close to the excitation energy (612 nm) up to 160 K (see Supplementary Fig. S9), the Fano resonance still becomes weaker, unlike other modes, e.g., E(M)-LA(M). The above results further prove that the Fano resonance results from the interference between acoustic phonons and dark excitons.
Fig. 4. Temperature dependence of quantum interference.
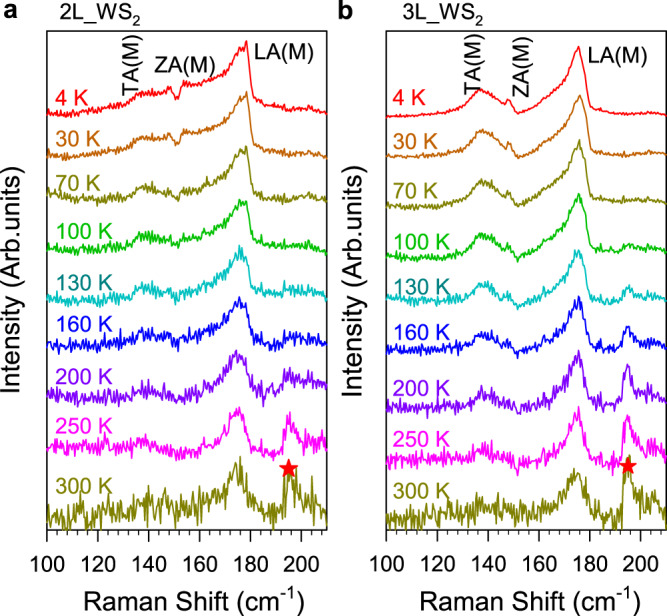
a, b Temperature-dependent Raman spectra of TA(M) and ZA(M) and LA(M) phonon modes in 2L and 3L WS2 with 612 nm excitation. The intensities are normalized to the LA(M) mode. The star symbol indicates the second-order phonon mode: E(M) - LA(M). The data are offset for clarity.
Discussions
Generally, the Fano-type quantum interference will appear when the continuum states and discrete states have the same symmetry (e.g., propagation/polarization direction) and meet the energy matching10. Therefore, the symmetry-determined coupling between zone-edged acoustic (or shear) phonons and dark excitons is the key factor in understanding why only these modes appeared with the Fano profile under the resonant excitation20. First, we note that the shear and layer breathing phonons are the relative vibrations between layers, different from those between atoms within a single layer, i.e., high-frequency optical phonons. If we only consider the vibration way of a single layer, the shear and layer breathing modes can be treated as “quasi-acoustic" phonons. It implies that the quantum interference occurs mainly between dark excitons and acoustic phonons.
The EPI strength is given by2
| 2 |
the coupling matrix , where un,k is the periodic part of Bloch wavefunction and the integral is over the whole unit cell. is the electron annihilation (creation) operator, while denotes the phonon’s with momentum q, mode ν and frequency ωqν. The strength of EPI is determined by how strong the lattice vibration affects the wavefunction of the excitons2,54. Here the wavefunctions of the conduction and valence bands near the K point are mainly contributed from d orbitals of the W atoms, which are greatly confined within a single layer. In particular, the propagation (polarization) of dark A exciton (Γ4 symmetry) is along the in-plane (Z) direction24,25,28, a strong interference with the in-plane vibrational modes is expected. By contrast, the variation of out-of-plane vibration shows a slight effect on the dark exciton at K point, and thus, the coupling between them is weak, see more discussions in SI. Consequently, the shear phonons, instead of layer breathing phonons, show Fano profiles. For Fano resonance of zone-edged acoustic phonons, both dark excitons in K and Q valley are involved in this interference process. Since the electronic states in Q valley are made from both W and S atoms orbitals, the strong interference/coupling occurring only in-plane is removed.
Finally, we analyze the coupling strength difference for three zone-edged acoustic phonons and dark excitons. It can be understood by considering the effective coupling between their different vibration ways (see Supplementary Figs. S10, S11) and momentum direction. Specifically, when neglecting the “umklapp” (folding) processes, only the first-order term of the coupling matrix gmnν(k, q) needs to be considered2:
| 3 |
where eqν is the polarization of the acoustic wave with wave vector q, and mode ν and g0 are the parameters depending on the materials. Considering the symmetry of these zone-edged acoustic phonon modes, the electron-phonon coupling strength of longitudinal modes is stronger than that for the transverse mode (q ⋅ eqν) in WS2. Consequently, the TA mode holds a weaker interference effect and appears with a nearly symmetric profile in spectra.
In summary, we found that the dark state is ~37 meV below the bright state in bilayer WS2. We further revealed that the first-order zone-edged acoustic phonon modes in few-layer WS2 can be directly observed thanks to the momentum match between ΓM (for acoustic phonons) and QK (for scattering K excitons to Q valley). The quantum interference strength between dark excitons and these phonons varies from constructive to destructive across layers and modes, which is strongly determined by the vibration way of phonons and the symmetry of dark excitons. Since the electronic energy band structure of few-layer WSe2 and MoS2 is similar to that of few-layer WS223,26,39, such quantum interferences are expected to be observed with proper excitation energies. Furthermore, the twisted bilayer or multilayer TMDs-based heterostructures and homostructures will also provide an additional platform to study and tune such quantum interferences between excitons and phonons, as well as the interference effects on their physical properties. Our results can give deep insight into the dark excitons-phonons interferences in layered semiconductors and pave the way for designing novel devices based on such excitons-phonons quantum interferences.
Methods
Sample preparation
The few-layer WS2 samples are exfoliated mechanically from their bulk crystals onto SiO2/Si substrate. For WS2 bilayer encapsulated in hBN, which is prepared by using a standard dry transfer method.
Optical measurements
Raman measurements were undertaken in backscattering geometry with a Jobin-Yvon HR800 system equipped with a liquid-nitrogen-cooled charge-coupled detector. The spectra were collected with a 50 × long-working-distance objective lens (NA = 0.5) at low temperature measurements. The ultralow-frequency Raman spectra were obtained down to ±5 cm−1 by combining three volume Bragg grating filters into the Raman system to efficiently suppress the Rayleigh signal. The Montana cryostat system was employed to cool the samples down to 4 K under a vacuum of 0.1 mTorr. The reflectance contrast ΔR/R were undertaken with a 50 × objective lens (NA = 0.5) and an 300 lines mm−1 grating with white light sources. The PL spectra were undertaken with objective lens with different numerical apertures (NA = 0.81 and 0.35).
Supplementary information
Acknowledgements
We thank Maciej Molas for helpful discussions. J. Z. acknowledges support of the National Key Research and Development Program of China (Grant No. 2017YFA0303401), Beijing Natural Science Foundation (Grant No. JQ18014), National Natural Science Foundation of China (Grant No. 12074371), CAS Interdisciplinary Innovation Team, Strategic Priority Research Program of Chinese Academy of Sciences (grant NO. XDB28000000). P. H. Tan acknowledges support of the National Natural Science Foundation of China (Grant nos. 11874350) and CAS Key Research Program of Frontier Sciences (Grant no. ZDBS-LY-SLH004 and XDPB22). W. B. Gao thanks the support of the Singapore NRF through its CRP Program (CRP Award Nos. NRF-CRP21-2018-0007, NRF-CRP22-2019-0004).
Author contributions
J.Z. and P.T. supervised the project; J.Z., P.T., and Q.T. conceived the ideas; Q.T. and J.L. prepared the samples. P.T. designed Raman experiments; Q.T., J.L., Y.S., Z.Z., and F.L. performed experiments; Q.T., Y.L., C.R., X.M., W.G., P.T. and Z.J. analyzed the data; Q.T., Y.L. and Z.J. wrote the manuscript with input from all authors.
Peer review
Peer review information
Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Data availability
The data that support the findings of this study are available from the corresponding authors on reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
These authors contributed equally: Qing-Hai Tan, Yun-Mei Li.
Contributor Information
Ping-Heng Tan, Email: phtan@semi.ac.cn.
Jun Zhang, Email: zhangjwill@semi.ac.cn.
Supplementary information
The online version contains supplementary material available at 10.1038/s41467-022-35714-3.
References
- 1.Devereaux TP, Hackl R. Inelastic light scattering from correlated electrons. Rev. Mod. Phys. 2007;79:175–233. [Google Scholar]
- 2.Giustino F. Electron-phonon interactions from first principles. Rev. Mod. Phys. 2017;89:015003. [Google Scholar]
- 3.Lin K-Q, Bange S, Lupton JM. Quantum interference in second-harmonic generation from monolayer WSe2. Nat. Phys. 2019;15:242–246. [Google Scholar]
- 4.Zhang S, et al. Quantum interference directed chiral raman scattering in two-dimensional enantiomers. Nat. Commun. 2022;13:1–7. doi: 10.1038/s41467-022-28877-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 5.Fano U. Effects of configuration interaction on intensities and phase shifts. Phys. Rev. 1961;124:1866–1878. [Google Scholar]
- 6.Miroshnichenko AE, Flach S, Kivshar YS. Fano resonances in nanoscale structures. Rev. Mod. Phys. 2010;82:2257–2298. [Google Scholar]
- 7.Hadjiev VG, et al. Strong superconductivity-induced phonon self-energy effects in HgBa2Ca3Cu4O10+δ. Phys. Rev. B. 1998;58:1043–1050. [Google Scholar]
- 8.Luk’yanchuk B, et al. The fano resonance in plasmonic nanostructures and metamaterials. Nat. Mater. 2010;9:707–715. doi: 10.1038/nmat2810. [DOI] [PubMed] [Google Scholar]
- 9.Limonov MF, Rybin MV, Poddubny AN, Kivshar YS. Fano resonances in photonics. Nat. Photonics. 2017;11:543–554. [Google Scholar]
- 10.Rousseau DL, Porto SPS. Auger-like resonant interference in raman scattering from one- and two-phonon states of batio3. Phys. Rev. Lett. 1968;20:1354–1357. [Google Scholar]
- 11.Cerdeira F, Fjeldly TA, Cardona M. Interaction between electronic and vibronic raman scattering in heavily doped silicon. Solid State Commun. 1973;13:325–328. [Google Scholar]
- 12.Tang T-T, et al. A tunable phonon-exciton fano system in bilayer graphene. Nat. Nanotechnol. 2010;5:32–36. doi: 10.1038/nnano.2009.334. [DOI] [PubMed] [Google Scholar]
- 13.Tan PH, et al. The shear mode of multilayer graphene. Nat. Mater. 2012;11:294–300. doi: 10.1038/nmat3245. [DOI] [PubMed] [Google Scholar]
- 14.Tan QH, et al. Observation of forbidden phonons, fano resonance and dark excitons by resonance raman scattering in few-layer WS2. 2D Mater. 2017;4:031007. [Google Scholar]
- 15.Tan Q-H, et al. Breakdown of raman selection rules by Fröhlich interaction in few-layer WS2. Nano Res. 2021;14:239–244. [Google Scholar]
- 16.Yang J, Lee J-U, Cheong H. Excitation energy dependence of raman spectra of few-layer WS2. FlatChem. 2017;3:64–70. [Google Scholar]
- 17.Wang G, et al. Colloquium: Excitons in atomically thin transition metal dichalcogenides. Rev. Mod. Phys. 2018;90:021001. [Google Scholar]
- 18.Liu Y, et al. Van der Waals heterostructures and devices. Nat. Rev. Mater. 2016;1:1–17. [Google Scholar]
- 19.Kennes DM, et al. Moiré heterostructures as a condensed-matter quantum simulator. Nat. Phys. 2021;17:155–163. [Google Scholar]
- 20.Dery H, Song Y. Polarization analysis of excitons in monolayer and bilayer transition-metal dichalcogenides. Phys. Rev. B. 2015;92:125431. [Google Scholar]
- 21.Song Y, Dery H. Transport theory of monolayer transition-metal dichalcogenides through symmetry. Phys. Rev. Lett. 2013;111:026601. doi: 10.1103/PhysRevLett.111.026601. [DOI] [PubMed] [Google Scholar]
- 22.Echeverry JP, Urbaszek B, Amand T, Marie X, Gerber IC. Splitting between bright and dark excitons in transition metal dichalcogenide monolayers. Phys. Rev. B. 2016;93:121107. [Google Scholar]
- 23.Molas MR, et al. Brightening of dark excitons in monolayers of semiconducting transition metal dichalcogenides. 2D Mater. 2017;4:021003. [Google Scholar]
- 24.Zhou Y, et al. Probing dark excitons in atomically thin semiconductors via near-field coupling to surface plasmon polaritons. Nat. Nanotechnol. 2017;12:856. doi: 10.1038/nnano.2017.106. [DOI] [PubMed] [Google Scholar]
- 25.Zhang X-X, et al. Magnetic brightening and control of dark excitons in monolayer WSe2. Nat. Nanotechnol. 2017;12:883. doi: 10.1038/nnano.2017.105. [DOI] [PubMed] [Google Scholar]
- 26.Robert C, et al. Measurement of the spin-forbidden dark excitons in MoS2 and MoSe2 monolayers. Nat. Commun. 2020;11:1–8. doi: 10.1038/s41467-020-17608-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27.Zinkiewicz M, et al. Neutral and charged dark excitons in monolayer WS2. Nanoscale. 2020;12:18153–18159. doi: 10.1039/d0nr04243a. [DOI] [PubMed] [Google Scholar]
- 28.Wang G, et al. In-plane propagation of light in transition metal dichalcogenide monolayers: Optical selection rules. Phys. Rev. Lett. 2017;119:047401. doi: 10.1103/PhysRevLett.119.047401. [DOI] [PubMed] [Google Scholar]
- 29.Wagner K, et al. Nonclassical exciton diffusion in monolayer WSe2. Phys. Rev. Lett. 2021;127:076801. doi: 10.1103/PhysRevLett.127.076801. [DOI] [PubMed] [Google Scholar]
- 30.Madéo J, et al. Directly visualizing the momentum-forbidden dark excitons and their dynamics in atomically thin semiconductors. Science. 2020;370:1199–1204. doi: 10.1126/science.aba1029. [DOI] [PubMed] [Google Scholar]
- 31.Glazov MM. Quantum interference effect on exciton transport in monolayer semiconductors. Phys. Rev. Lett. 2020;124:166802. doi: 10.1103/PhysRevLett.124.166802. [DOI] [PubMed] [Google Scholar]
- 32.Mai C, et al. Exciton valley relaxation in a single layer of WS2 measured by ultrafast spectroscopy. Phys. Rev. B. 2014;90:041414. [Google Scholar]
- 33.Jiang C, et al. Microsecond dark-exciton valley polarization memory in two-dimensional heterostructures. Nat. Commun. 2018;9:753. doi: 10.1038/s41467-018-03174-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Carvalho BR, et al. Intervalley scattering by acoustic phonons in two-dimensional MoS2 revealed by double-resonance Raman spectroscopy. Nat. Commun. 2017;8:14670. doi: 10.1038/ncomms14670. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Tan Q, et al. Layer-engineered interlayer excitons. Sci. Adv. 2021;7:eabh0863. doi: 10.1126/sciadv.abh0863. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Helmrich S, et al. Phonon-assisted intervalley scattering determines ultrafast exciton dynamics in MoSe2 bilayers. Phys. Rev. Lett. 2021;127:157403. doi: 10.1103/PhysRevLett.127.157403. [DOI] [PubMed] [Google Scholar]
- 37.Klein, M. V. Electronic raman scattering. 147-204 (Springer, 1983).
- 38.Tristant D, et al. Optical signatures of bulk and solutions of KC8 and KC24. J. Appl. Phys. 2015;118:044304. [Google Scholar]
- 39.Bussolotti F, et al. Roadmap on finding chiral valleys: screening 2D materials for valleytronics. Nano Futures. 2018;2:032001. [Google Scholar]
- 40.Robert C, et al. Fine structure and lifetime of dark excitons in transition metal dichalcogenide monolayers. Phys. Rev. B. 2017;96:155423. [Google Scholar]
- 41.Jiang X, et al. Real-time GW-BSE investigations on spin-valley exciton dynamics in monolayer transition metal dichalcogenide. Sci. Adv. 2021;7:eabf3759. doi: 10.1126/sciadv.abf3759. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 42.Wang Z, et al. Intravalley spin–flip relaxation dynamics in single-layer WS2. Nano Lett. 2018;18:6882–6891. doi: 10.1021/acs.nanolett.8b02774. [DOI] [PubMed] [Google Scholar]
- 43.Ferrari AC, et al. Raman spectrum of graphene and graphene layers. Phys. Rev. Lett. 2006;97:187401. doi: 10.1103/PhysRevLett.97.187401. [DOI] [PubMed] [Google Scholar]
- 44.Ferrari AC. Raman spectroscopy of graphene and graphite: Disorder, electron–phonon coupling, doping and nonadiabatic effects. Solid State Commun. 2007;143:47–57. [Google Scholar]
- 45.Shi W, et al. Raman and photoluminescence spectra of two-dimensional nanocrystallites of monolayer WS2 and WSe2. 2D Mater. 2016;3:025016. [Google Scholar]
- 46.Liang L, et al. Low-Frequency Shear and Layer-Breathing Modes in Raman Scattering of Two-Dimensional Materials. ACS Nano. 2017;11:11777–11802. doi: 10.1021/acsnano.7b06551. [DOI] [PubMed] [Google Scholar]
- 47.Cadiz F, et al. Excitonic linewidth approaching the homogeneous limit in mos2-based van der waals heterostructures. Phys. Rev. X. 2017;7:021026. [Google Scholar]
- 48.Livneh T, Spanier JE. A comprehensive multiphonon spectral analysis in MoS2. 2D Mater. 2015;2:035003. [Google Scholar]
- 49.Fleischhauer M, Imamoglu A, Marangos JP. Electromagnetically induced transparency: Optics in coherent media. Rev. Mod. Phys. 2005;77:633–673. [Google Scholar]
- 50.Arimondo E. Dark resonances in quantum optics. Acta Phys. Pol. A. 2007;112:723. [Google Scholar]
- 51.Peng B, Özdemir ŞK, Chen W, Nori F, Yang L. What is and what is not electromagnetically induced transparency in whispering-gallery microcavities. Nat. Commun. 2014;5:1–9. doi: 10.1038/ncomms6082. [DOI] [PubMed] [Google Scholar]
- 52.Mak KF, Lee C, Hone J, Shan J, Heinz TF. Atomically thin MoS2: A new direct-gap semiconductor. Phys. Rev. Lett. 2010;105:136805. doi: 10.1103/PhysRevLett.105.136805. [DOI] [PubMed] [Google Scholar]
- 53.Splendiani A, et al. Emerging photoluminescence in monolayer MoS2. Nano Lett. 2010;10:1271–1275. doi: 10.1021/nl903868w. [DOI] [PubMed] [Google Scholar]
- 54.Yu, P. Y. & Cardona, M.Optical Properties II: Fundamentals of Semiconductors: Physics and Materials Properties, 345-426 (Springer Berlin Heidelberg, Berlin, Heidelberg, 2010).
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The data that support the findings of this study are available from the corresponding authors on reasonable request.