Abstract
Vanadium dioxide (VO2) exhibits an insulator-to-metal transition accompanied by a structural transition near room temperature. This transition can be triggered by an ultrafast laser pulse. Exotic transient states, such as a metallic state without structural transition, were also proposed. These unique characteristics let VO2 have great potential in thermal switchable devices and photonic applications. Although great efforts have been made, the atomic pathway during the photoinduced phase transition is still not clear. Here, we synthesize freestanding quasi-single-crystal VO2 films and examine their photoinduced structural phase transition with mega-electron-volt ultrafast electron diffraction. Leveraging the high signal-to-noise ratio and high temporal resolution, we observe that the disappearance of vanadium dimers and zigzag chains does not coincide with the transformation of crystal symmetry. After photoexcitation, the initial structure is strongly modified within 200 femtoseconds, resulting in a transient monoclinic structure without vanadium dimers and zigzag chains. Then, it continues to evolve to the final tetragonal structure in approximately 5 picoseconds. In addition, only one laser fluence threshold instead of two thresholds suggested in polycrystalline samples is observed in our quasi-single-crystal samples. Our findings provide essential information for a comprehensive understanding of the photoinduced ultrafast phase transition in VO2.
Subject terms: Structure of solids and liquids, Phase transitions and critical phenomena, Chemical physics
The atomic pathway in the photoinduced ultrafast structural phase transition of VO2 has been a controversial problem for a long time. Here the authors, using MeV ultrafast electron diffraction, show that the melting of V-V dimers and the transformation of crystal symmetry are two processes with different timescales.
Introduction
Ultrafast photoexcitation by femtosecond (fs) lasers can help understand and manipulate the interaction among electronic, magnetic, and structural degrees of freedom1–5. Various quantum materials, including charge-density-wave materials6–11, superconductors12–15, ferromagnets16, and phase transition materials17–21, have been explored via ultrafast photoexcitation techniques. Of these, the strongly correlated oxide VO2 has attracted extensive interests. VO2 exhibits a first-order insulator-to-metal transition accompanied by a monoclinic-to-rutile structural phase transition (SPT) upon heating22,23. The transition occurs near the room temperature with a dramatic change in resistance as well as in optical properties. Such a transition can also be induced by ultrafast photoexcitation17–20,24–37. Thus, VO2 has great potential in fast/smart thermal switchable devices and photonic applications.
In the monoclinic insulating phase (called the M1 phase), V atoms dimerize and form zigzag chains (called the superstructure in this work). In the rutile (tetragonal) metallic phase (called the R phase), V–V dimers and related zigzag chains disappear. The unit cell in the M1 phase is nearly twice larger than that in the R phase, resulting in more X-ray or electron diffraction peaks (called superstructure peaks) in the M1 phase. To understand the phase transition, VO2 was studied by various experimental methods, such as terahertz (THz) spectroscopy26–28,30,31, optical spectroscopy24,25, X-ray absorption spectroscopy (XAS)36, ultrafast electron diffraction (UED)17–19,35,37,38, and ultrafast X-ray diffraction (UXRD)20. However, the true nature of the phase transition is still not yet fully understood. For instance, while the SPT involves both the loss of the V–V dimerization and the lattice transformation39,40, the related atomic pathway has not been fully revealed17,37.
The very first UED (in a reflected electron diffraction mode) experiment with femtosecond resolution17 on single crystalline VO2 proposed that V–V dimers first dilate to form an intermediate phase without dimers but with V zigzag chains. Then V–V bonds begin tilting to eliminate the zigzag chain, leading to the R-phase17. UED experiment19 on polycrystalline VO2 proposed that V atoms might first reach an intermediate structure, which is akin to the M2 phase (a phase with a special configuration of V zigzag chains41). UXRD experiment have found that the disordered movement of V atoms played an important role in the light-induced ultrafast phase transition20. Recent UED experiment on single-crystal VO2 indicated that V–V dimers dilate and tilt simultaneously in a coherent way in the very early stage of the photoinduced SPT (PSPT)37.
SPT from the M1 to R phase will not only change the fractional coordinates of V atoms within a unit cell, but also cause a change in the lattice symmetry39. VO2 has the monoclinic symmetry in the M1 phase, and turns into the tetragonal symmetry in the R phase. So far, the question whether the change of the fractional position of V atoms coincides with the change of the lattice symmetry in PSPT remains unanswered. An ultrafast diffraction conoscopy experiment showed that VO2 films grown on an Al2O3 substrate could sustain an intermediate biaxial structure that is different from the initial M1 phase (the M1 phase is also a biaxial phase) for several hundreds of femtoseconds, which provides some indirect information that the disappearance of V–V dimers and zigzag chains may not coincide with the change of the crystal symmetry in PSPT34.
Furthermore, previous UED experiments on polycrystalline VO2 samples suggested a photoexcited long-lived monoclinic metallic phase (called the mM phase) lasting for hundreds of picoseconds18. This phase is isostructural to the M1 phase18,35,36,38. The key evidence in this UED experiment was that the laser fluence threshold for non-superstructure peaks is smaller than that for superstructure peaks18. However, the experimental uncertainty is considerably large due to the low signal-to-noise ratio in polycrystalline samples. Recently, only one threshold was observed in time-resolved XAS36. UED on a single crystalline VO2 sample thinned by focused ion beam from a bulk single-crystal also gave some indication of one threshold37.
In this work, we succeed in fabricating high quality quasi-single crystalline VO2 freestanding films and revisit the PSPT using mega-electron-volt (MeV) UED with 50 fs resolution42. Due to the high signal-to-noise ratio and the domain structures in quasi-single crystalline samples, we reveal the comprehensive atomic pathway, including the melting of V–V dimers and the transformation from monoclinic symmetry to tetragonal symmetry. We present direct evidence that the disappearance of superstructure does not coincide with the change of the crystal symmetry. We find that V–V dimers melt and V atoms reach the same fractional coordinates as that in the R phase within 200 fs, resulting in a transient monoclinic structure without V–V dimers and zigzag chains. Then, it continues to evolve from this transient monoclinic structure to the final tetragonal structure within 5 picoseconds. In addition, the laser fluence thresholds are accurately measured benefited from the well separation of the superstructure peaks from other diffraction peaks. A single threshold is obtained within the experimental uncertainty.
Results
Preparation of quasi-single crystalline freestanding VO2 films
The state-of-the-art UED technique uses transmission mode, so freestanding films are required. Recently, Sr3Al2O6 (SAO) has been used as a water-soluble sacrificial layer to obtain freestanding single crystalline films prepared by pulsed laser deposition (PLD)43. Directly growing ultrathin freestanding single-crystal films on SAO has been demonstrated for materials with perovskite structures such as SrTiO3 (STO)43, La0.7Sr0.3MnO343, BaTiO344, BiFeO345, and LaMnO346 that have lattice parameters close to SAO. However, this method does not apply to VO2 due to the large lattice mismatch. Our solution for obtaining freestanding VO2 films by PLD is to add an ultrathin STO(111) buffer layer between the VO2 and SAO. By limiting the thickness of STO buffer layer to 2 nm, we can minimize the effect from the buffer layer on our diffraction measurements. We grew 20 nm SAO on a bulk STO(111) substrate followed by a 2 nm single-crystal STO(111) film as the buffer layer for the VO2 growth. Then we grew ~40 nm VO2 on the STO(111) buffer layer. The layout of the sample is shown in Fig. 1a.
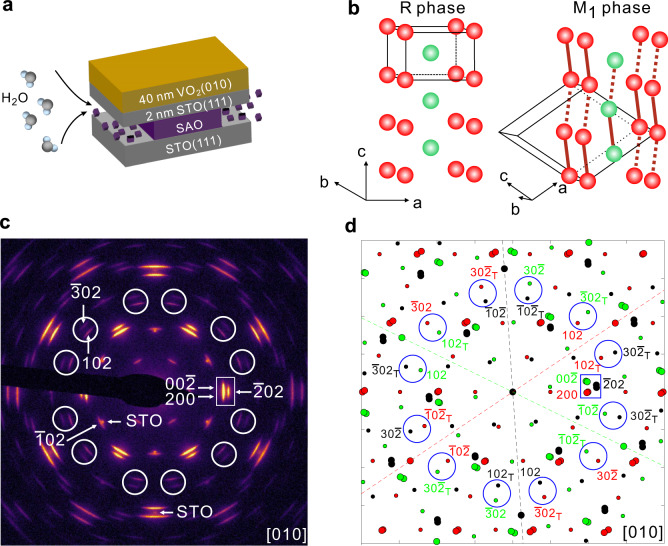
Fig. 1. Static electron diffractions of VO2 by TEM.
a Schematic layout of the VO2 films for UED experiments. STO represents SrTiO3(111) which is used as substrate and buffer layer. SAO represents Sr3Al2O6 which is used as sacrificial layer. SAO can be etched by water indicated by black arrows. b Crystal structures of VO2 in the R phase and M1 phase. Only V atoms are presented. Red solid lines in the M1 phase represent V–V dimers. Hexahedrons with black lines are the unit cells. c Static electron diffraction pattern measured at 300 K by TEM. The incident electron beam is along [010] direction of VO2. d Simulated TEM diffraction pattern in the M1 phase with three domains and twinning. Red/green/black colors represent the contributions from three 120° domains, respectively. For convenience, we use smaller dots to present the superstructure spots. The subscript “T” means the contribution from a twin domain.
The epitaxial growth of VO2 films on the STO(111) surface was systematically studied in the previous report47. The normal direction of the film is [010], which nicely matches the UED experiments because V–V dimers exist in the (010) plane. By the strict definition, VO2/STO(111) film is a (010) textured film. Unlike usual textured films with lots of in-plane orientations, VO2/STO(111) film only has three in-plane orientations (called three 120° domains, for convenience) in the R phase. In the M1 phase, each domain will further form twins. Details about the crystal structure are presented in Supplementary Note 1 in the Supplementary Materials (SM). To be distinct from usual textured films, we call VO2/STO(111) films quasi-single crystalline films. After removing SAO by etching in water, we obtained relatively large flakes of freestanding 40-nm VO2/2-nm STO films. The lateral size of the flakes varies around 0.5 0.5 mm. See more details about the samples in Supplementary Fig. 1. The freestanding films are transferred to a transmission electron microscope (TEM) grid with ultrathin amorphous carbon film for MeV UED measurements. The amorphous carbon film effectively reduces heat accumulation from the optical pump with a repetition rate of 100 Hz.
Static electron diffractions of quasi-single-crystal VO2
The static electron diffraction pattern at 300 K (M1 phase) measured by 200 keV TEM is shown in Fig. 1c with Miller indices shown for several representative diffraction spots. All Miller indices use the M1-phase’s representation. Diffraction spots from the 2-nm STO buffer layer were also observed, and are indicated by “STO”. Figure 1d shows the simulated ideal TEM diffraction pattern in the M1 phase considering three 120° domains and the twinning in each domain. The twinning in the M1 phase results from the difference in the lattice constants ( = 5.356 Å and = 5.383 Å39,48) (see more details in Supplementary Figs. 1 and 2). Red/green/black colors in Fig. 1d represent the contributions from three 120° domains, respectively. The simulation is in good agreement with the measurements. The elongated shape along the tangential direction in Fig. 1c is related to two effects. First, three sets of diffraction spots from 120° in-plane domains distribute in the tangential direction, seen from Fig. 1d. They partially overlapped in experiments, which makes the spots broaden along the tangential direction. Second, the shape of crystal grains is anisotropic (see details in Supplementary Fig. 1), which also makes the spots broader in the tangential direction than in the radial direction.
In Fig. 1c, we marked 12 groups of spots that are well separated from STO spots by white circles. There are two spots in each group. Indexed in Fig. 1d, the inner 12 spots are . Each 120o domain contributes four spots when twinning is considered. The subscript “T” means the contribution from a twin domain. and are equivalent because of the same index despite that they are from different domains. Since VO2 has the inversion symmetry, and are equivalent. So, , , and are indeed equivalent spots. The outer 12 spots are 3. They are also equivalent spots. Therefore, such 12 groups are equivalent. Another important group of spots is marked by a white rectangle in Fig. 1c. According to Fig. 1d, this group consists of six spots, i.e., and . Due to the limited space, we omitted indices of, and for simplicity. Each 120° domain contributes two spots when twinning is considered.
Static electron diffractions of quasi-single-crystal VO2 by UED
Figure 2a presents the static diffraction pattern measured by the 3-MeV electrons in the MeV UED instrument below and above the phase transition temperature. It should be noted that three 120° domains equally contribute to the diffraction intensities because the electron beam size (∼150 µm in diameter) in UED is much bigger than the grain size in the film. The momentum resolution in Fig. 2a is not as good as that in Fig. 1c. There are two reasons. First, unlike the TEM, the electron beam is not focused in UED to achieve a high temporal resolution. Second, the higher kinetic energy in the MeV UED also slightly lowers the momentum resolution. As a result, the diffraction spots close to each other in Fig. 1c merge together in Fig. 2a. For instance, 102 and become one spot. For simplicity, we use 102 to index this big spot. 102 and spots are only existed in the M1 phase related to the V–V dimers. They are superstructure spots. The structure factor of 102 and are zero in the R phase, so they both disappear during the SPT from the M1 phase to R phase. Similarly, , , and spots are also overlapped. We use 200 as the simple index. In the previous diffraction experiments in a single crystalline sample37, the intensities of , , and spots all enhance during the SPT from the M1 phase to R phase. Therefore, it is reasonable to trace the intensities of the big 102 and 200 spots to study the dynamics of the SPT.
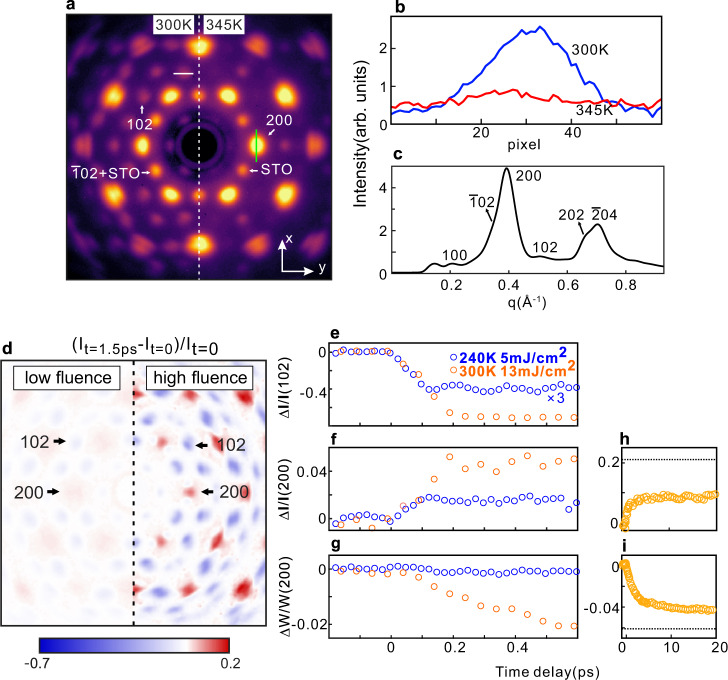
Fig. 2. Ultrafast evolution of the diffraction spots in VO2.
a Static electron diffraction patterns measured at 300 K (left) and 345 K (right) with the 3-MeV electrons in UED. Green line labels the direction where we trace the peak width. b Line profile along the white line in a at 300 K and 345 K, respectively. c Azimuthally averaged intensity of the diffraction pattern at 300 K. d Image plot of the intensity difference between the diffraction patterns at t = 1.5 ps after photoexcitation and t = 0 (before photoexcitation) under a low fluence (laser fluence is 5 mJ/cm2 at 240 K) and a high fluence (laser fluence is 13 mJ/cm2 at 300 K). Time evolution of the intensity for (e) 102 peaks, (f) 200 peaks and (g) width of 200 peaks. Data up to 20 ps for (h) intensity of 200 peaks and (i) width of 200 peaks under a high fluence. Black dashed line marks the level of relative change when 100% M1-R transition occurs based on the thermal transition data.
As expected, the superstructure spots disappear above the transition temperature (Fig. 2a right). Figure 2b presents the line profiles of a superstructure peak along the direction indicated by the white line in Fig. 2a. At 300 K, the diffraction peak is well-defined. At 345 K, the diffraction peak disappears and the background is slightly enhanced due to the thermal effect. To simulate the polycrystalline sample to some extent, we plot the azimuthally averaged diffraction intensity in Fig. 2c. The superstructure peaks (100, and 102) are influenced by the neighboring non-superstructure peak (200) with very high intensity, which renders accurate determination of the threshold difficult in polycrystalline samples18. Following the temperature dependence of the intensity of 102 peak, a clear SPT with a temperature hysteresis loop was observed (see details in Supplementary Fig. 3).
Ultrafast evolution of the diffraction spots in VO2
The VO2 sample shows an ultrafast response to the laser pump. Figure 2d presents the normalized intensity differences between the diffraction pattern at t = 1.5 ps after photoexcitation and that at t = 0 (without photoexcitaion) under the low (~5 mJ/cm2 at 240 K) and high (~13 mJ/cm2 at 300 K) laser fluence, respectively. Upon photoexcitation, the intensity of all the superstructure spots decreases, while non-superstructure spots enhance.
Figure 2e shows the transient intensity change of the 102 peak. Under a low fluence, the intensity only drops by about 13% and oscillations of 6 THz related to a coherent phonon of the zigzag motion of V atoms20,25,29,49, are clearly observed. There is no SPT under low fluence (see data with 1.5 ps in Supplementary Fig. 4). A similar coherent phonon was detected in the UXRD experiment20, but had not been observed in previous UED experiments primarily due to the insufficient temporal resolution. In contrast, under a high fluence, the intensity drops by more than 70% within 200 fs, and no oscillation was detected. The absence of oscillation implies a fast structural transition, in agreement with the UXRD experiments20. Qualitatively consistent with previous UED experiments18, the intensity of the non-superstructure 200 peak increases after photoexcitation, as shown in Fig. 2f. However, the timescale dichotomy in the intensity evolution of the superstructure and non-superstructure diffraction peaks observed in polycrystalline samples18,35,38 is not observed in our measurements. Rather, the intensities of all the peaks changed with nearly the same femtosecond timescale, also consistent with UXRD on single-crystal samples20.
Interestingly, we can trace the ultrafast structure evolution by following not only the peak intensity but also the peak width due to the high signal-to-noise ratio and the domain structure in our quasi-single crystalline samples. Presented in Fig. 2g, the peak width of 200 (W200) along the x-direction (indicated by a green line in Fig. 2a) decreased with a picosecond timescale under a high fluence, while it remained constant under a low fluence. To do the analysis on the peak width along x-direction, we superposed the intensities of 200 spot along the y-direction. Since no SPT occurs under a low fluence, the decrease of W200 under a high fluence is very likely related to the SPT. In fact, the decrease of W200 can be understood based on the difference in the lattice constants and , as well as the angle between and , in the monoclinic structure. As we show in Fig. 1, there are three 120° domains plus twin domains in our samples, which has two implications for the peak width. First, the separation between the diffraction spots from different 120° domains is correlated to . Second, the twin domains originated from the difference in and cause the additional peak splitting. With the gradual suppression of twin domains and increase in , six diffraction spots (Fig. 1d) inside a big 200 spot get closer and closer (see more details in Supplementary Fig. 5). As a result, the peak width becomes narrower. Figure 2i indicates that the peak width decreases in a picosecond timescale, implying that a monoclinic structure persists for several picoseconds even after the complete disappearance of the superstructure in VO2.
Transient monoclinic structure without V–V dimers
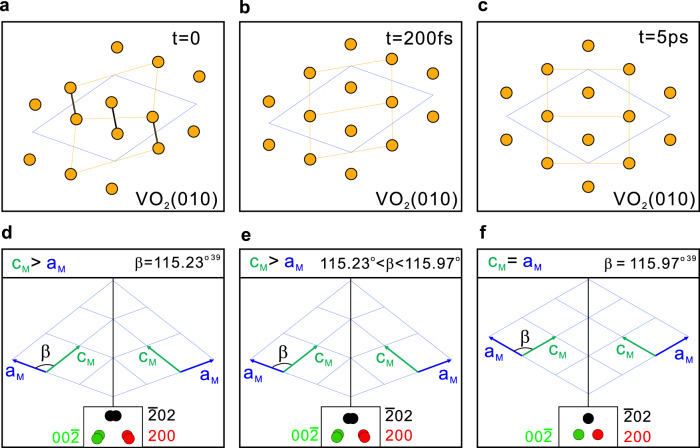
Based on our experimental results, we propose that there are two processes with different timescales during the transition from the M1 Phase to R phase in the PSPT of VO2. Figure 3a–c sketches the projected positions of V atoms on the (010) plane at three different time. The corresponding sketches of twin domains are shown in Fig. 3d–f. The insets present six diffraction spots inside the big 200 spot observed in Fig. 2a. In the initial M1 phase (Fig. 3a, d), the sample consists of the superstructure and twin domains. In the first process, the V–V dimers dilate and tilt simultaneously, which is characterized by the very fast disappearance of the superstructure peaks (Fig. 2e). This fast process has been observed in previous scattering studies and was thought to end up in the R phase in a femtosecond timescale18,20. It should be noted that in diffraction experiments, the intensity of diffraction peaks is mainly affected by the fractional coordinates of atoms in the unit cell. Therefore, the disappearance of the superstructure peaks does not necessarily mean that VO2 is in the R phase, but rather it means that V atoms are in the same fractional coordinates as in the R phase.
Fig. 3. Transient monoclinic structure without V–V dimers in VO2.
Schematic of Monoclinic M1 phase with V–V dimers and zigzag chains at t = 0 (a), transient monoclinic structure without V–V dimers and zigzag chains at t = 200 fs (b) and R phase at t = 5 ps (c). Orange dots represent the projected positions of V atoms on the (010) plane. Black lines present the V–V dimers. Orange lines are a guide for the eyes. Blue quadrilateral indicates the unit cell using M1-phase’s representation. The sketches of twin domains at t = 0 (d), t = 200 fs (e), t = 5 ps (f) after photoexcitation. Red\green\black dots indicate the diffraction spots from three 120° domains and the twin domains respectively. And the separation between the two spots is exaggerated for clarity.
The evolution of the width of 200 peak in Fig. 2g indicates that the fast process does not lead to the R phase, but to an intermediate monoclinic structure without V–V dimers and/or zigzag chains. Figure 3b, e sketches the final stage of this fast process at t = 200 fs. The V–V dimers and/or zigzag chains no longer exist, and V atoms are located at the same fractional coordinates as in the R phase. Correspondingly, there is no superstructure spot, but aM and cM remains different and does not reach that in the R phase. Hence, the twin domains still exist after the first process. The second process is the transition from the monoclinic symmetry to the tetragonal symmetry through the lattice expansion, mainly along the aM direction. This process is characterized by the continual decrease of W200 (Fig. 2g). It is a slow process and nearly finishes within 5 ps, ending up in the R phase sketched in Fig. 3c, f with aM = cM and no twinning. The second process is not distinguishable from the first process within the first 200 fs because the movement of V atoms in the first process expands aM simultaneously. The second process suggests that a transient monoclinic structure without V–V dimers and/or zigzag chains exists after the first process and lasts for several picoseconds.
Fluence thresholds measurements
To determine the fluence thresholds for the PSPT, we measured the diffraction intensity at a time delay of 10 ps when the electron and lattice largely reaches a quasi-equilibrium condition. Figure 4a shows the fluence dependence of 102 peak measured at 300 K. A distinct kink is observed at ~3.1 mJ/cm2. The intensity decreases slowly below this value, while a fast decrease is observed above this value. Because the 102 peak is directly associated with the V–V dimerization, the presence of a kink indicates a fluence threshold for SPT, e.g., a critical energy is needed to melt the V–V dimers for the transition to the R phase. Therefore, at 300 K, we have the threshold (Fc1) of ~3.1 mJ/cm2. For the 200 peak, a kink is also observed at ~3.1 mJ/cm2, as shown in Fig. 4b. Thus, we obtained the threshold (Fc2) of ~3.1 mJ/cm2. This is in stark contrast to previous work in polycrystalline samples where Fc1 is much larger than Fc218. We repeated the measurements at several temperatures (280, 260, 240, and 220 K) and the threshold for the 102 and 200 peaks are consistently very similar (see details in Supplementary Fig. 6). We also measured the fluence threshold for the change of W200 as shown in Fig. 4c. Again, it has a similar threshold (Fc3) of ~3.1 mJ/cm2.
Fig. 4. Measurements of the laser fluence.
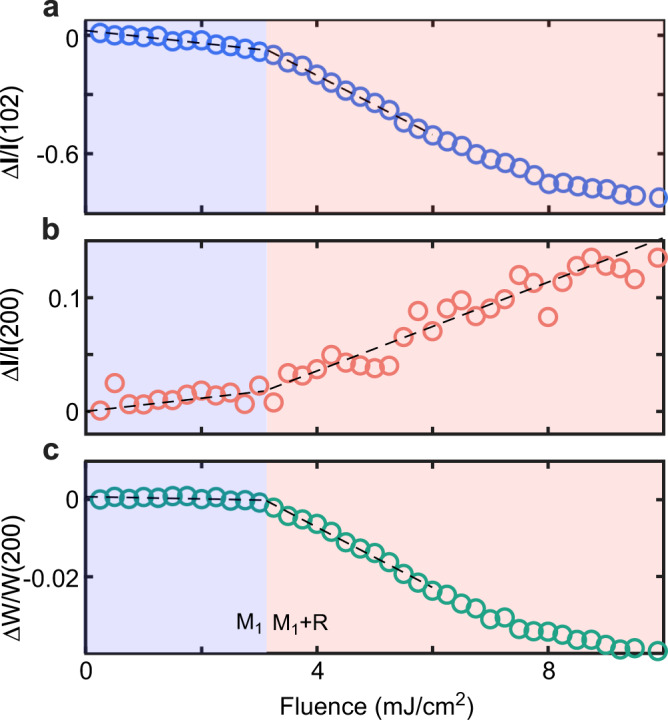
Fluence dependence of the changes measured at t = 10 ps after photoexcitation for 102 peak intensity (a), 200 peak intensity (b), and 200 peak width (c). Dashed lines are a guide to the eye.
It should be noted that the diffraction measurements do not yield the direct information of free-electron density that confirm metallicity. Ref. 18 suggested an interesting photoinduced mM phase with a very long lifetime (sever hundreds of picoseconds). Besides the optical measurements, one of the key evidences is the existence of two thresholds in UED. Since only one threshold is detected in our experiments, the proposed long lifetime mM phase in ref. 18 very likely does not exist in our quasi-single crystalline samples.
Discussion
The pathway with two processes we proposed during the PSPT in VO2 differs from the proposed two steps model17. In the previous model, the first step is the dilation of the V–V dimers, followed by the tilting of the V–V bonds. Therefore, two timescales were expected for the decay of the superstructure peaks. However, only one timescale following the superstructure peak was observed by the UXRD20 and UED37 experiments in the single crystalline samples. While two timescales were observed by UED in the polycrystalline VO2 samples, they were extracted from superstructure and non-superstructure peaks18. Some optical measurements observed two processes with two timescales of <10 ps and >45 ps50 which is not directly related to the ultrafast phase transition. In our experiments, only one timescale was obtained based on the superstructure peak, which is in agreement with the UXRD and the UED experiments in the single-crystal samples. A single timescale in the superstructure peak indicates a direct movement of V atoms; in other word, the V–V dimers dilate and tilt at the same time to the fractional coordinates the same as that of the R phase, which is the first process in our proposal. The second process revealed in our experiments is the transformation from a transient monoclinic structure to the tetragonal R phase. Recent ultrafast X-Ray hyperspectral imaging experiments40 observed two time constants of 132 fs and 4.75 ps, and they are not related to dilation and tilting17. Considering the fact that these time constants are close to the timescales we observed, it is possible that they might be related to the two processes revealed in our experiments, which would be interesting to explore in the future.
The second process can hardly be explored in polycrystalline samples by UED. Although we found this new process in quasi-single crystalline samples, we still cannot tell the exact route how V atoms move as a function of time because of the in-plane domains. High quality freestanding single crystals are needed to completely solve this problem. Preliminary results have been obtained in single-crystal samples37. However, much more efforts are needed to have well controlled freestanding single crystalline samples. In principle, by tracing the intensity of various diffraction spots in single crystals, we can determine the position of V atoms through the structure factor.
In summary, we studied the PSPT in the quasi-single crystalline VO2 samples with the MeV UED. The highly improved signal-to-noise ratio and temporal resolution not only allowed us to observe the coherent phonon related to the zigzag motion of V atoms connecting the M1 and R phases under a low laser fluence, but also revealed two processes with different timescales when SPT occurs: the fast destruction of V–V dimers and zigzag chains within ~200 fs and the relatively slow transformation from the monoclinic symmetry to the tetragonal symmetry within ~5 ps. Only a single fluence threshold was observed for different diffraction peaks. Our work promotes the comprehensive understanding of the ultrafast PSPT in VO2 and will stimulate more theoretical and experimental efforts in the future.
Methods
Sample preparation
Quasi-single-crystal VO2 films were epitaxially grown on SrTiO3 (111) (STO) substrates by pulsed laser deposition with a KrF excimer laser (λ = 248 nm, Coherent) using a V2O5 target. The base pressure of our PLD system is 8 × 10−10 Torr. The STO(111) substrate was first annealed at 1000 °C for 1 h in oxygen at 1 × 10−6 Torr to obtain a clean and flat surface. Then, a 20-nm SAO (111) sacrificial layer followed by a 2-nm STO (111) buffer layer was deposited at 880 °C under 5 × 10−6 Torr oxygen pressure. The thickness of SAO and STO was monitored by the reflection high energy electron diffraction (RHEED) oscillations. The laser repetition rate is 5 Hz, and the fluence is 1.5 J/cm2. After that, 40 nm quasi-single-crystal VO2(010) films was deposited on the STO (111) buffer layer at 450 °C in 3 mTorr oxygen pressure. The laser repetition rate is 10 Hz, and the fluence was 1 J/cm2. We used deionized water to remove the SAO layer and transferred the 40 nm VO2(010)/2 nm STO (111) freestanding film to a TEM grid with ultrathin (~5 nm) amorphous carbon film for 200 keV TEM and 3 MeV UED measurements. Thickness of VO2 film were checked by AFM.
Transmission electron microscopy
The TEM experiments were conducted using a Talos F200X STEM. The accelerating voltage was 200 kV. The selected area aperture is 200 μm in diameter and the diffracting region is 4 μm in diameter. The electron beam was incident on the sample along the [010] direction of VO2.
MeV ultrafast electron diffraction
The electron beam in this MeV UED system has a kinetic energy of about 3 MeV and is compressed with a double-bend achromatic magnet system. An electron-multiplying CCD camera and a phosphor screen were used to measure the diffraction pattern. A 800-nm Ti:Sapphire laser with a pulse width of ~30 fs was used as the pump laser. The radius spot size of the pump laser was 1.5 mm. The pump laser was near-normal incidence. More details of the MeV UED setup can be found in the literature42. A repetition rate of 100 Hz or 50 Hz was used to mitigate the heat accumulation. The temporal resolution was about 50 fs (FWHM) for the measurements of the ultrafast dynamic. The temporal resolution was 100 fs to increase the signal-to-noise ratio for the measurements of the thresholds.
Supplementary information
Acknowledgements
This work was supported by the National Key R&D Program of China (No. 2022YFA1402400, No. 2021YFA1400202, No. 2021YFA1400100), the National Natural Science Foundation of China (Grants No. 11521404, No. 11925505), the office of Science and Technology, Shanghai Municipal Government (No. 16DZ2260200), and the additional support from a Shanghai talent program.
Author contributions
D.Q. and D.X. conceived the experiments, C.X. conducted the experiments with the help of C.J., Z.C., Q.L., Y.C. B.Z., F.F.Q., J.C., X.Y., G.H.W., C.X., D.Q. and D.X. analyzed the results. All authors reviewed the manuscript.
Peer review
Peer review information
Nature Communications thanks Richard Haglund and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Data availability
All of the data supporting the conclusions are available within the article and the Supplementary Information. Because the raw data include tens of thousands of images, they are available from the corresponding authors upon request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Contributor Information
Dao Xiang, Email: dxiang@sjtu.edu.cn.
Dong Qian, Email: dqian@sjtu.edu.cn.
Supplementary information
The online version contains supplementary material available at 10.1038/s41467-023-37000-2.
References
- 1.Rini M, et al. Control of the electronic phase of a manganite by mode-selective vibrational excitation. Nature. 2007;449:72–74. doi: 10.1038/nature06119. [DOI] [PubMed] [Google Scholar]
- 2.Ichikawa H, et al. Transient photoinduced ‘hidden’ phase in a manganite. Nat. Mater. 2011;10:101–105. doi: 10.1038/nmat2929. [DOI] [PubMed] [Google Scholar]
- 3.Sie EJ, et al. An ultrafast symmetry switch in a Weyl semimetal. Nature. 2019;565:61–66. doi: 10.1038/s41586-018-0809-4. [DOI] [PubMed] [Google Scholar]
- 4.Nova TF, Disa AS, Fechner M, Cavalleri A. Metastable ferroelectricity in optically strained SrTiO3. Science. 2019;364:1075–1079. doi: 10.1126/science.aaw4911. [DOI] [PubMed] [Google Scholar]
- 5.Li X, et al. Terahertz field-induced ferroelectricity in quantum paraelectric SrTiO3. Science. 2019;364:1079–1082. doi: 10.1126/science.aaw4913. [DOI] [PubMed] [Google Scholar]
- 6.Eichberger M, et al. Snapshots of cooperative atomic motions in the optical suppression of charge density waves. Nature. 2010;468:799–802. doi: 10.1038/nature09539. [DOI] [PubMed] [Google Scholar]
- 7.Zong A, et al. Evidence for topological defects in a photoinduced phase transition. Nat. Phys. 2019;15:27–31. doi: 10.1038/s41567-018-0311-9. [DOI] [Google Scholar]
- 8.Kogar A, et al. Light-induced charge density wave in LaTe3. Nat. Phys. 2020;16:159–163. doi: 10.1038/s41567-019-0705-3. [DOI] [Google Scholar]
- 9.Zhou F, et al. Nonequilibrium dynamics of spontaneous symmetry breaking into a hidden state of charge-density wave. Nat. Commun. 2021;12:566. doi: 10.1038/s41467-020-20834-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 10.Duan S, et al. Optical manipulation of electronic dimensionality in a quantum material. Nature. 2021;595:239–244. doi: 10.1038/s41586-021-03643-8. [DOI] [PubMed] [Google Scholar]
- 11.Cheng Y, et al. Light-induced dimension crossover dictated by excitonic correlations. Nat. Commun. 2022;13:963. doi: 10.1038/s41467-022-28309-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Fausti D, et al. Light-induced superconductivity in a stripe-ordered cuprate. Science. 2011;331:189–191. doi: 10.1126/science.1197294. [DOI] [PubMed] [Google Scholar]
- 13.Stojchevska L, et al. Ultrafast switching to a stable hidden quantum state in an electronic crystal. Science. 2014;344:177–180. doi: 10.1126/science.1241591. [DOI] [PubMed] [Google Scholar]
- 14.Mitrano M, et al. Possible light-induced superconductivity in K3C60 at high temperature. Nature. 2016;530:461–464. doi: 10.1038/nature16522. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Gerber S, et al. Femtosecond electron-phonon lock-in by photoemission and x-ray free-electron laser. Science. 2017;357:71–75. doi: 10.1126/science.aak9946. [DOI] [PubMed] [Google Scholar]
- 16.Dornes C, et al. The ultrafast Einstein-de Haas effect. Nature. 2019;565:209–212. doi: 10.1038/s41586-018-0822-7. [DOI] [PubMed] [Google Scholar]
- 17.Baum P, Yang D-S, Zewail AH. 4D visualization of transitional structures in phase transformations by electron diffraction. Science. 2007;318:788–792. doi: 10.1126/science.1147724. [DOI] [PubMed] [Google Scholar]
- 18.Morrison VR, et al. A photoinduced metal-like phase of monoclinic VO2 revealed by ultrafast electron diffraction. Science. 2014;346:445–448. doi: 10.1126/science.1253779. [DOI] [PubMed] [Google Scholar]
- 19.Tao Z, et al. The nature of photoinduced phase transition and metastable states in vanadium dioxide. Sci. Rep. 2016;6:1–10. doi: 10.1038/srep38514. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Wall S, et al. Ultrafast disordering of vanadium dimers in photoexcited VO2. Science. 2018;362:572–576. doi: 10.1126/science.aau3873. [DOI] [PubMed] [Google Scholar]
- 21.Horstmann JG, et al. Coherent control of a surface structural phase transition. Nature. 2020;583:232–236. doi: 10.1038/s41586-020-2440-4. [DOI] [PubMed] [Google Scholar]
- 22.Morin FJ. Oxides which show a metal-to-insulator transition at the Neel temperature. Phys. Rev. Lett. 1959;3:34–36. doi: 10.1103/PhysRevLett.3.34. [DOI] [Google Scholar]
- 23.Park JH, et al. Measurement of a solid-state triple point at the metal-insulator transition in VO2. Nature. 2013;500:431–434. doi: 10.1038/nature12425. [DOI] [PubMed] [Google Scholar]
- 24.Cavalleri A, et al. Femtosecond structural dynamics in VO2 during an ultrafast solid-solid phase transition. Phys. Rev. Lett. 2001;87:237401. doi: 10.1103/PhysRevLett.87.237401. [DOI] [PubMed] [Google Scholar]
- 25.Cavalleri A, Dekorsy T, Chong HHW, Kieffer JC, Schoenlein RW. Evidence for a structurally-driven insulator-to-metal transition in VO2: a view from the ultrafast timescale. Phys. Rev. B. 2004;70:161102. doi: 10.1103/PhysRevB.70.161102. [DOI] [Google Scholar]
- 26.Hilton DJ, et al. Enhanced photosusceptibility near Tc for the light-induced insulator-to-metal phase transition in vanadium dioxide. Phys. Rev. Lett. 2007;99:226401. doi: 10.1103/PhysRevLett.99.226401. [DOI] [PubMed] [Google Scholar]
- 27.Kübler C, et al. Coherent structural dynamics and electronic correlations during an ultrafast insulator-to-metal phase transition in VO2. Phys. Rev. Lett. 2007;99:116401. doi: 10.1103/PhysRevLett.99.116401. [DOI] [PubMed] [Google Scholar]
- 28.Pashkin A, et al. Ultrafast insulator-metal phase transition in VO2 studied by multiterahertz spectroscopy. Phys. Rev. B. 2011;83:195120. doi: 10.1103/PhysRevB.83.195120. [DOI] [Google Scholar]
- 29.Wall S, et al. Ultrafast changes in lattice symmetry probed by coherent phonons. Nat. Commun. 2012;3:721. doi: 10.1038/ncomms1719. [DOI] [PubMed] [Google Scholar]
- 30.Liu M, et al. Terahertz-field-induced insulator-to-metal transition in vanadium dioxide metamaterial. Nature. 2012;487:345–348. doi: 10.1038/nature11231. [DOI] [PubMed] [Google Scholar]
- 31.Cocker TL, et al. Phase diagram of the ultrafast photoinduced insulator-metal transition in vanadium dioxide. Phys. Rev. B. 2012;85:155120. doi: 10.1103/PhysRevB.85.155120. [DOI] [Google Scholar]
- 32.Wegkamp D, et al. Instantaneous band gap collapse in photoexcited monoclinic VO2 due to photocarrier doping. Phys. Rev. Lett. 2014;113:216401. doi: 10.1103/PhysRevLett.113.216401. [DOI] [PubMed] [Google Scholar]
- 33.Jager MF, et al. Tracking the insulator-to-metal phase transition in VO2 with few-femtosecond extreme UV transient absorption spectroscopy. Proc. Natl Acad. Sci. USA. 2017;114:9558–9563. doi: 10.1073/pnas.1707602114. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34.Kumar N, Rúa A, Fernández FE, Lysenko S. Ultrafast diffraction conoscopy of the structural phase transition in VO2: evidence of two lattice distortions. Phys. Rev. B. 2017;95:235157. doi: 10.1103/PhysRevB.95.235157. [DOI] [Google Scholar]
- 35.Otto MR, et al. How optical excitation controls the structure and properties of vanadium dioxide. Proc. Natl Acad. Sci. USA. 2019;116:450–455. doi: 10.1073/pnas.1808414115. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Vidas L, et al. Does VO2 host a transient monoclinic metallic phase? Phys. Rev. X. 2020;10:031047. [Google Scholar]
- 37.Li J, et al. Direct detection of V-V atom dimerization and rotation dynamic pathways upon ultrafast photoexcitation in VO2. Phys. Rev. X. 2022;12:021032. [Google Scholar]
- 38.Sood A, et al. Universal phase dynamics in VO2 switches revealed by ultrafast operando diffraction. Science. 2021;373:352–355. doi: 10.1126/science.abc0652. [DOI] [PubMed] [Google Scholar]
- 39.Kucharczyk D, Niklewski T. Accurate X-ray determination of the lattice parameters and the thermal expansion coefficients of VO2 near the transition temperature. J. Appl. Crystallogr. 1979;12:370–373. doi: 10.1107/S0021889879012711. [DOI] [Google Scholar]
- 40.Johnson, A. S. et al. Ultrafast X-ray imaging of the light-induced phase transition in VO2. Nat. Phys. 10.1038/s41567-022-01848-w (2022).
- 41.Yuan X, Zhang Y, Abtew TA, Zhang P, Zhang W. VO2: orbital competition, magnetism, and phase stability. Phys. Rev. B. 2012;86:235103. doi: 10.1103/PhysRevB.86.235103. [DOI] [Google Scholar]
- 42.Qi F, et al. Breaking 50 femtosecond resolution barrier in MeV ultrafast electron diffraction with a double bend achromat compressor. Phys. Rev. Lett. 2020;124:134803. doi: 10.1103/PhysRevLett.124.134803. [DOI] [PubMed] [Google Scholar]
- 43.Lu D, et al. Synthesis of freestanding single-crystal perovskite films and heterostructures by etching of sacrificial water-soluble layers. Nat. Mater. 2016;15:1255–1260. doi: 10.1038/nmat4749. [DOI] [PubMed] [Google Scholar]
- 44.Dong G, et al. Super-elastic ferroelectric single-crystal membrane with continuous electric dipole rotation. Science. 2019;366:475–479. doi: 10.1126/science.aay7221. [DOI] [PubMed] [Google Scholar]
- 45.Ji D, et al. Freestanding crystalline oxide perovskites down to the monolayer limit. Nature. 2019;570:87–90. doi: 10.1038/s41586-019-1255-7. [DOI] [PubMed] [Google Scholar]
- 46.Lu Q, et al. Photoinduced evolution of lattice orthorhombicity and conceivably enhanced ferromagnetism in LaMnO3 membranes. npj Quantum Mater. 2022;7:47. doi: 10.1038/s41535-022-00456-4. [DOI] [Google Scholar]
- 47.Lee S, Ivanov IN, Keum JK, Lee HN. Epitaxial stabilization and phase instability of VO2 polymorphs. Sci. Rep. 2016;6:19621. doi: 10.1038/srep19621. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 48.Longo JM, et al. A refinement of the structure of VO2. Acta Chem. Scand. 1970;24:420–426. doi: 10.3891/acta.chem.scand.24-0420. [DOI] [Google Scholar]
- 49.Yuan X, Zhang W, Zhang P. Hole-lattice coupling and photoinduced insulator-metal transition in VO2. Phys. Rev. B. 2013;88:035119. doi: 10.1103/PhysRevB.88.035119. [DOI] [Google Scholar]
- 50.Abreu E, et al. Ultrafast electron-lattice coupling dynamics in VO2 and V2O3 thin films. Phys. Rev. B. 2017;96:094309. doi: 10.1103/PhysRevB.96.094309. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
All of the data supporting the conclusions are available within the article and the Supplementary Information. Because the raw data include tens of thousands of images, they are available from the corresponding authors upon request.