Abstract
All leading long-term global population projections agree on continuing fertility decline, resulting in a rate of population size growth that will continue to decline toward zero and would eventually turn negative. However, scholarly and popular arguments have suggested that because fertility transmits intergenerationally (i.e., higher fertility parents tend to have higher fertility children) and is heterogeneous within a population, long-term population growth must eventually be positive, as high-fertility groups come to dominate the population. In this research note, we show that intergenerational transmission of fertility is not sufficient for positive long-term population growth, for empirical and theoretical reasons. First, because transmission is imperfect, the combination of transmission rates and fertility rates may be quantitatively insufficient for long-term population growth: higher fertility parents may nevertheless produce too few children who retain higher fertility preferences. Second, today even higher fertility subpopulations show declining fertility rates, which may eventually fall below replacement (and in some populations already are). Therefore, although different models of fertility transmission across generations reach different conclusions, depopulation is likely under any model if, in the future, even higher fertility subpopulations prefer and achieve below-replacement fertility. These results highlight the plausibility of long-term global depopulation and the importance of understanding the possible consequences of depopulation.
Keywords: Fertility, Population growth, Intergenerational transmission
Introduction
Global fertility is projected to fall below replacement levels, which will eventually cause negative population growth (KC and Lutz 2017; United Nations 2019; Vollset et al. 2020). Survey evidence confirms agreement among demographers that fertility will continue to fall (Gietel-Basten et al. 2014). However, some recent publications that apply models of intergenerational transmission from the mathematical biology literature proposed that low fertility is unlikely to endure and global population growth is unlikely to become negative (Burger and DeLong 2016; Collins and Page 2019; Ellis et al. 2017; Murphy and Wang 2003). These arguments also appear in popular-audience accounts of fertility (Ingraham 2015; Kaufmann 2010). Such authors reason that high fertility is intergenerationally transmissible, and so if there are higher and lower fertility patterns exhibited within subpopulations, eventually the composition of the population will converge toward the higher fertility pattern.1
Here we observe that—even granting the premise that higher fertility parents have higher fertility children with high probability—such intergenerational transmission is not sufficient for positive long-term population growth (LTPG).2 One reason is that researchers should not conflate higher fertility within a heterogeneous population with high or above-replacement fertility: it is an empirical question whether future higher fertility subpopulations will have above-replacement fertility. If not, then population growth will be negative. There is strong historical and global evidence that even higher fertility groups will trend to near or below replacement fertility. The second reason is that the existence of a subpopulation with above-replacement fertility is not sufficient for positive LTPG, even with intergenerational transmission. This is because an insufficient number of children of high-fertility parents may retain their parents’ behaviors—that is, even if fertility is correlated within a family across generations, the correlation may be less than 1.0.
There are other convergent arguments in the literature. Boyd and Richerson (1988:199–202), for example, described mechanisms by which cultural and biological transmission could together cause sustained fertility decline. Kolk et al. (2014), in a point allied to but separate from ours, highlighted that fertility heterogeneity and intergenerational transmission could be consistent with long-term population decline, but only if new low-fertility groups (“types”) are culturally invented again and again in the future. One of our contributions is to show that this is possible in a model with just two types.
Because we respond to a literature concerned with both genetic and social transmission and because we claim that intergenerational transmission is not sufficient for positive growth, we do not specify or limit our arguments to either genetic or social transmission of parental traits. Our arguments show that neither of these forms of transmission is sufficient for positive LTPG.
We formalize conditions under which intergenerational transmission does not cause positive LTPG: if enough children of high-fertility parents become low-fertility adults, long-term population growth can be negative even with both intergenerational transmission and an above-replacement-fertility subpopulation. Whether modeled population growth is positive or negative in the long run depends on model structure, parameters, and initial conditions, but we show that it is not guaranteed by the mere fact of intergenerational transmission.3 We also show that depopulation is likely under any model if, in the future, even higher fertility subpopulations prefer and achieve below-replacement fertility, as empirical facts suggest.
Evidence of Fertility Decline Among High-Fertility Groups
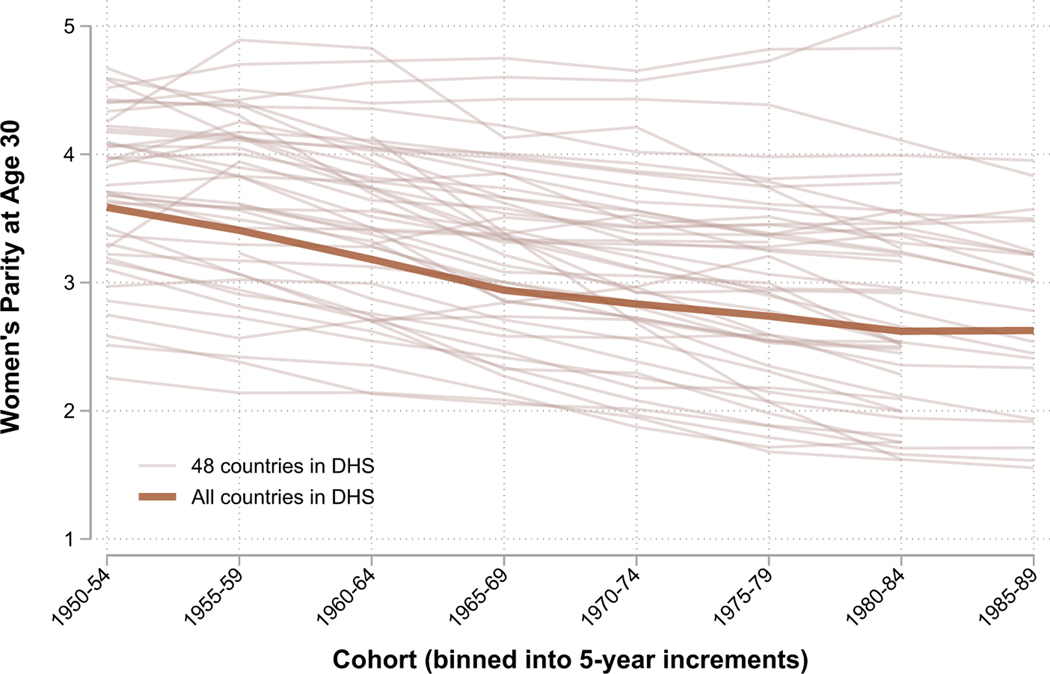
Figure 1 displays fertility trends in 48 countries using data from the Demographic and Health Survey (DHS).4 These 48 countries account for nearly 45% of the world’s population and 60% of births each year. They are primarily developing and emerging economies, which is useful for our purposes because these are, in general, the populations for which above-replacement fertility currently exists. The horizontal axis of the figure is cohort (year of birth) binned into five-year increments from 1950 to 1989; the vertical axis is the average parity at age 30 of women in that cohort bin.5 Each thin line represents a different country, and the thick line represents the average across countries. All but two of the 48 countries have decreasing fertility rates. The evidence offers no reason to conclude that the downward trend will stop above replacement levels. Indeed, many of even these emerging and developing economies are already below or near replacement level.
Fig. 1.
Women’s parity at age 30, by birth cohort, for 48 countries. The horizontal axis is cohort (year of birth) binned into five-year increments from 1950 to 1989; the vertical axis is the average parity at age 30 of women in that cohort bin. Each thin line represents a different country; the thick line represents the average across countries. Source: Demographic and Health Surveys.
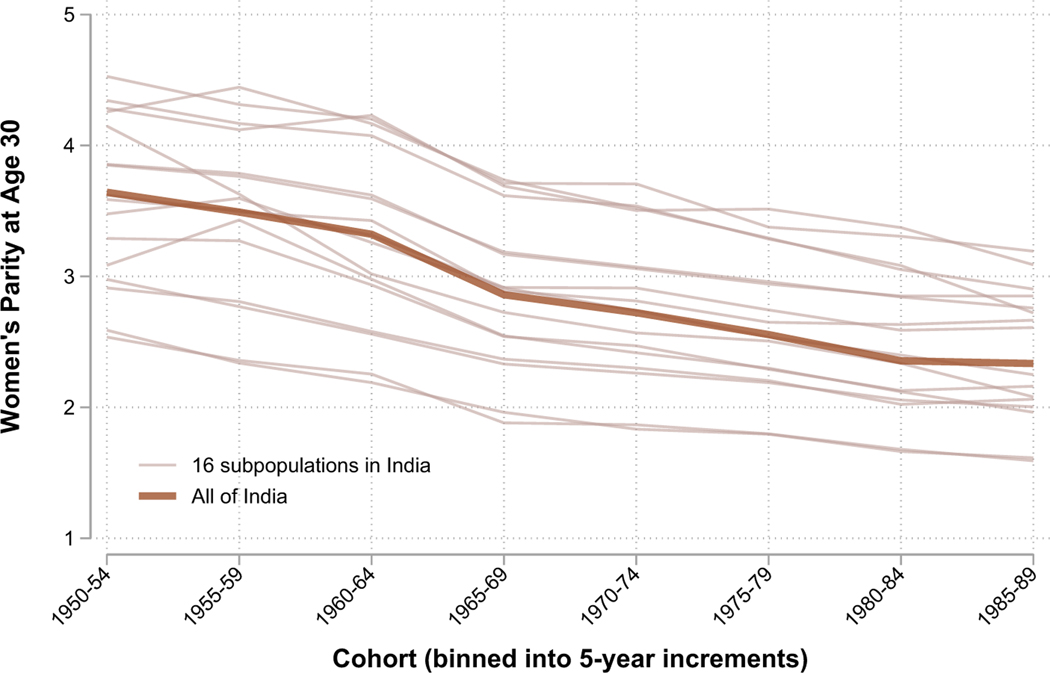
Figure 2 focuses on India, which accounts for one sixth of the world’s population and has historically been a focus of global population policy debates (Connelly 2010). India is home to diverse subpopulations, which differ, among other ways, in their average fertility levels. In this figure, we categorize women into 16 nonoverlapping groups by interacting indicators for north India/south India, rural/urban, Muslim/not Muslim, and no education/some education. For example, one of the thin lines represents south Indian, rural, Muslim women with some education. Each of the 16 groups demonstrates a clear downward slope. As with nearly all countries in the DHS, all major subpopulations within India—including those with the highest levels of fertility—have declining fertility.
Fig. 2.
Women’s parity at age 30, by birth cohort, for 16 different subpopulations in India. The 16 nonoverlapping groups are generated by interacting indicators for north India/south India, rural/urban, Muslim/not Muslim, and no education/some education. The horizontal axis is cohort (year of birth) binned into five-year increments from 1950 to 1989; the vertical axis is the average parity at age 30 of women in that cohort bin. Each thin line represents a different subpopulation; the thick line represents the average for India overall. Source: Demographic and Health Surveys.
These empirical facts cast doubt on a necessary condition for positive LTPG: that higher fertility subpopulations will sustain high (i.e., above replacement) levels of fertility. The necessity of this condition can be seen clearly by considering the mathematical biology model of Kolk et al. (2014). Their Model 1 describes the long-term evolution of the composition of a population with two fertility types: higher and lower. In their model, the composition of the population converges entirely to the higher type. But the quantitative fertility level of the higher fertility types is an unconstrained, exogenous parameter of the model.6 If higher fertility types have below-replacement fertility (as Figures 1 and 2 suggest may someday happen) then the model of Kolk et al. (2014) would project a depopulating world, even as the composition shifts to higher fertility types.
Figures 1 and 2 focus on developing countries, where fertility is generally highest today. But discussions of intergenerational transmission in fertility are often motivated by reference to higher fertility among religious subpopulations in developed countries, such as the United States (Ellis et al. 2017; Ingraham 2015; Kaufmann 2010). Although these observations are not essential to our argument, we note two important facts about demographic patterns in the United States. First, fertility rates among religious Americans, despite a consistently higher level than among nonreligious Americans, are falling approximately in parallel with fertility rates for the whole population (Perry and Schleifer 2019). For example, the National Survey of Family Growth (NSFG) shows that, between cohorts born in the 1940s and cohorts born in the 1970s, completed fertility for both religious and nonreligious women (operationalized as religious service attendance) fell by approximately 20%. Second, such group identities—whether defined by religion, educational attainment, rural/urban status, or cultural conservatism—are not transmitted perfectly across generations. The NSFG shows that the fraction of Americans who report being religious is falling over time, from 57% in 1988 to 43% in 2019. We take up the implications of such imperfect intergenerational transmission next.
A Stylized Two-Type Model of Population Dynamics
So far we have provided empirical evidence that many groups are trending toward below-replacement fertility. However, some authors have argued that if even one group remains above replacement, then this high-fertility group would eventually drive population fertility toward their high rate (e.g., Collins and Page 2019). In this section, we show analytically that the existence of such a subgroup is not a sufficient condition for positive LTPG. Instead, we demonstrate in a two-type model that long-run population decline can exist in a world with a subgroup that has both above-replacement fertility and (imperfect) vertical transmission of fertility from mother to daughter. The intuition is this: if high-fertility parents have children at above-replacement levels, but only some fraction of those children receive the high-fertility type, then the size of the high-fertility group (and the overall population) can nonetheless decline.
Denote high- and low-fertility types with reproductive rates , respectively, where we have simplified to a single-sex environment such that a reproductive rate 1 is replacement level.7 This two-type model with transmissible fertility from parents to offspring builds on the structure employed by Kolk et al. (2014).8 In our model, we emphasize that children’s received fertility type, which is a function solely of their parent’s type, is imperfectly transmitted. In particular, the offspring of type retain their parent’s fertility preferences with probability and switch types with probability .
For simplicity, we focus on the case in which the fertility rate and transition probabilities are constant for each type. Such a special case with fixed transmission probabilities is called a Markov model. Because we are not interested in deriving or characterizing constant (or other) equilibrium shares of the population,9 but instead are interested in the asymptotic total size of the population, we need not assume fixed Markov probabilities: it would be sufficient for our purposes if transition probabilities fluctuate, are linked to the proportion of the trait in the population, or otherwise evolve but are bounded by the inequality we derive below (Condition 2).10 Fixed probabilities would be incompatible with standard models of genetic inheritance in which genetic transmission is dependent on the proportion of a trait in a population.11 However, for simplicity we assume fixed Markov probabilities, knowing that our model can be immediately relaxed in this way.
In this setting, the evolution of types can be written as follows, where is the number of types in each period:
| (1) |
where
and the transition matrix specifies how types evolve. The number of high types at equals , which could be greater or less than the number of high types at , depending on model parameters. Because, by construction, the low types cannot sustain their numbers without inflow , it is straightforward to show that there is positive LTPG only if there is long-run growth in the subset of high types.12
To further simplify the initial exposition of high-type dynamics, assume that . That is, children of low types receive that type with certainty. This exact assumption is not necessary for the model’s main qualitative conclusions, and we relax it in the appendix, but it generates a simple and intuitive condition for long-term decline. Combining this one-way switching assumption with the general property of Markov processes that , it can be shown that the population of high types evolves according to . The high types decline—and therefore the long-run aggregate population size declines—if
| (2) |
Condition 2 is the essential, intuitive requirement for negative LTPG. Even if transition probabilities are not fixed in a Markov sense, LTPG is negative if there is a time after which Condition 2 is always true.
In the online appendix, we relax the assumption that . We prove, with fixed transmission probabilities, that positive LTPG will not occur if
| (3) |
which can be satisfied by a range of feasible and empirically plausible parameters. This condition simplifies to Condition 2 if or .
For example, consider a world in which the average high-fertility woman has 1.2 female children and the average low-fertility woman has 0.33 female children. Assume the higher fertility group is culturally attractive, so that a child of a high-fertility parent has an 80% chance of becoming a high-fertility adult (and a 20% chance of becoming a low-fertility adult), and a child of a low-fertility parent has only a 75% chance of becoming a low-fertility adult (and a 25% chance of becoming a high-fertility adult). In this world, the intergenerational correlation of parents’ and children’s fertility would be high, but Condition 3 is met, so the size of the population would decrease over time.13
Note that if this model were the true data-generating process in some population, then a regression of one’s parent’s fertility on own fertility in that population would return a positive coefficient. This is exactly the type of empirical correlation pointed to (incorrectly) as evidence that high-fertility patterns will come to dominate a population and lead to long-term population growth. Here, the correlation arises in a statistical process in which long-term fertility and population both asymptote to zero. This is the key error in this literature: the existence of (high, positive) mother–daughter fertility correlations and a higher fertility subgroup are not enough to infer whether population size will increase over time.
Finally, to return to the point of our empirical section, may itself decline below replacement, which would ensure that . In fact, although the model of Kolk et al. (2014) differs from ours in assuming that transmission probabilities depend on the composition of the population (we model them as fixed), their model and ours agree on this implication, in the empirical case where higher fertility becomes low. Especially as fertility rates become low, fertility outcomes are importantly shaped by fertility preferences, choices, and intentions (e.g., Gietel-Basten 2019; Goldstein et al. 2003; Pritchett 1994; Yeatman et al. 2020). One key way in which human population dynamics differ from the mathematical dynamics of nonhuman populations is the importance of fertility determinants such as culture, economics, preferences, and contraception (Kohler and Rodgers 2003).
Discussion
Negative population growth, if it occurs, may have many consequences for societies and economies (Jones 2020; Morgan 2003). Here we respond to a literature motivated by mathematical biology that intends to cast doubt on projections of depopulation. Human fertility is unlike the fertility of other animals because it is shaped by culture, economics, and intention. Building on the work of Kolk et al. (2014), we have shown here that intergenerational transmission of fertility is not sufficient to prevent long-run population decline.
To generate positive LTPG in the simple foregoing model, the number of children who retain the high-fertility preferences of their parents must exceed replacement; it is not sufficient merely that higher fertility types have above-replacement fertility, even with transmissibility. The condition for positive LTPG is met through some combination of both high fertility rates and low net outflow. Empirical evidence presented here and elsewhere suggests that even “higher fertility” types of the future may prefer and achieve fertility rates near (or even below) replacement. This would leave little (or no) room for positive LTPG in the presence of any intergenerational outflow under any theoretical model.
Positive LTPG depends on the facts: there may yet arise high-fertility groups with sufficiently low intergenerational outflow to meet the required conditions. Evidence broadly suggests that most socioeconomic properties show imperfect intergenerational correlation, including important examples such as religious practice, political affiliation, and income (Chetty et al. 2014; Vogl and Freese 2020). Fertility itself has been recently examined in post–demographic transition populations and has been shown to have positive but low intergenerational correlation, providing evidence that the transmission parameter above, , is likely low (Vogl 2020).
The twentieth century was characterized by uniquely rapid population growth (Lam 2011). Understanding the implications of a switch to population decline, or even merely stabilization, is of clear importance. Contrary to some arguments in the literature, empirical facts and models of intergenerational transmission do not provide reason to conclude that positive population growth is bound to continue.
Supplementary Material
Footnotes
ELECTRONIC SUPPLEMENTARY MATERIAL The online version of this article (https://doi.org/10.1215/00703370-10290429) contains supplementary material.
In fact, the model of Collins and Page (2019) implies the stronger claim that aggregate fertility rates are always increasing via this mechanism in post–demographic transition settings, counter to the experiences of developed countries over the previous half century.
Our note complements the empirical findings of Vogl (2020), who does not focus on long-term future population growth but quantifies with survey data that any effect of intergenerational transmission on aggregate fertility has historically been small.
Our purpose here is not to establish which is the correct model of intergenerational transmission of fertility heterogeneity.
The set of 48 countries is the subset of all DHS countries for which at least 500 women are interviewed in at least six of the eight cohort bins pictured.
In principle, such cohort fertility rates could be declining over time merely because women are delaying fertility to later ages; however, the declining trends documented in this section also appear for later-age cohort fertility (restricting attention to earlier cohorts).
In contrast, the post–demographic transition model of Collins and Page (2019) does not contain any parameter for average desired fertility levels. Instead, the fertility of the next generation is a function (representing what they call “heritability”) only of the fertility of the last generation. Because such a model does not admit cultural, social, or economic influences on desired and achieved fertility other than through this narrow intergenerational transmission channel, we interpret it as difficult to reconcile with the empirical facts of Figures 1 and 2, which reflect children of high-fertility parents transitioning to radically lower fertility behavior over the course of only a few generations. Therefore, we depart from their model by modeling the empirically relevant possibility that post–demographic transition fertility could, in part, reflect changes (such as in preferences) beyond or in addition to intergenerational transmission.
This single-sex model allows us to ignore assortative mating and is equivalent to assuming that only females’ types matter. The most literal reading of our model is that the probability that a daughter is high or low type is a function only of whether her mother is high or low type. Our explicit assumption of a single-sex model serves to clarify our departure from a model in which genetic transmission depends, through parental matching, on the proportion of a trait in the population—though see Model D in the online supplementary materials for an example of negative LTPG in a two-sex model with matching and transmission dependent on the proportion of the trait in the population.
Cavalli-Sforza and Feldman (1981) also used a simplified two-type model of vertical transmission.
Compare this with Preston and Campbell (1993), who used a Markov model of differential fertility to study the share of the population with intergenerationally transmissible traits. Lam (1993) observed in response that their argument for convergence to a steady-state composition of the population is driven by the setting of fixed Markov transition probabilities. Because we have a different theoretical goal, we need not assume a fixed Markov matrix, but we do so for ease of exposition.
For example, it could be the case that increases over the generations but decreases, so that Condition 2, introduced below, is always met.
For example, in population geneticists’ simple Hardy–Weinberg model of a randomly mating, stable population, the observed proportion of a genotype trait is a nonlinear function of its genetic prevalence because parents of a type must match. See details in Falconer (1960).
In the case where high types grow, temporary population decline is possible if the low types shrink sufficiently fast at the start; eventually, however, only high types are left and their population growth necessarily takes over.
See the online supplementary materials for the calculations corresponding to this numerical example (Model A). These materials also contain examples with transmission probabilities that vary exogenously over time (Model B) and transmission probabilities that are a function of the proportion of the trait in the population (Model C for a single-sex case and Model D for a two-sex case with matching dynamics). Models B, C, and D all generate negative LTPG despite the fact that and for all generations .
Contributor Information
Samuel Arenberg, National Bureau of Economic Research, Cambridge, MA, USA.
Kevin Kuruc, Population Wellbeing Initiative, The University of Texas at Austin, Austin, TX, USA.
Nathan Franz, Department of Economics, The University of Texas at Austin, Austin, TX, USA.
Sangita Vyas, Department of Economics, Hunter College, City University of New York, New York, NY, USA.
Nicholas Lawson, Département des Sciences Économiques, Université du Québec à Montréal, Montréal, Québec, Canada.
Melissa LoPalo, Department of Economics, Montana State University, Bozeman, MT, USA.
Mark Budolfson, Department of Philosophy and School of Public Health, Rutgers University, New Brunswick, NJ, USA.
Michael Geruso, Department of Economics, The University of Texas at Austin, Austin, TX, USA.
Dean Spears, Department of Economics, The University of Texas at Austin, Austin, TX, USA.
References
- Boyd R, & Richerson PJ (1988). Culture and the evolutionary process. Chicago, IL: University of Chicago Press. [Google Scholar]
- Burger O, & DeLong JP (2016). What if fertility decline is not permanent? The need for an evolutionarily informed approach to understanding low fertility. Philosophical Transactions of the Royal Society B: Biological Sciences, 371, 20150157. 10.1098/rstb.2015.0157 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Cavalli-Sforza LL, & Feldman MW (1981). Cultural transmission and evolution: A quantitative approach. Princeton, NJ: Princeton University Press. [PubMed] [Google Scholar]
- Chetty R, Hendren N, Kline P, & Saez. E. (2014). Where is the land of opportunity? The geography of intergenerational mobility in the United States. Quarterly Journal of Economics, 129, 1553–1623. [Google Scholar]
- Collins J, & Page L.(2019). The heritability of fertility makes world population stabilization unlikely in the foreseeable future. Evolution and Human Behavior, 40, 105–111. [Google Scholar]
- Connelly M.(2010). Fatal misconception: The struggle to control world population. Cambridge, MA: Harvard University Press. [Google Scholar]
- Ellis L, Hoskin AW, Dutton E, & Nyborg H.(2017). The future of secularism: A biologically informed theory supplemented with cross-cultural evidence. Evolutionary Psychological Science, 3, 224–242. [Google Scholar]
- Falconer DS (1960). Introduction to quantitative genetics. Edinburgh, Scotland: Oliver & Boyd. [Google Scholar]
- Gietel-Basten S.(2019). The “population problem” in Pacific Asia. New York, NY: Oxford University Press. [Google Scholar]
- Gietel-Basten S, Sobotka T, & Zeman K.(2014). Future fertility in low fertility countries. In Lutz W, Butz WP, & KC S.(Eds.), World population and human capital in the twenty-first century: An overview (pp. 39–146). Oxford, UK: Oxford University Press. [Google Scholar]
- Goldstein J, Lutz W, & Testa MR (2003). The emergence of sub-replacement family size ideals in Europe. Population Research and Policy Review, 22, 479–496. [Google Scholar]
- Ingraham C.(2015, May 12). Charted: The religions that make the most babies. The Washington Post. Retrieved from https://www.washingtonpost.com/news/wonk/wp/2015/05/12/charted-the-religions-that-make-the-most-babies/ [Google Scholar]
- Jones CI (2020). The end of economic growth? Unintended consequences of a declining population (NBER Working Paper 26651). Cambridge, MA: National Bureau of Economic Research. [Google Scholar]
- Kaufmann E.(2010). Shall the religious inherit the earth? Demography and politics in the twenty-first century. London, UK: Profile Books. [Google Scholar]
- KC S, & Lutz W.(2017). The human core of the shared socioeconomic pathways: Population scenarios by age, sex and level of education for all countries to 2100. Global Environmental Change, 42, 181–192. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kohler H-P, & Rodgers JL (2003). Education, fertility, and heritability: Explaining a paradox. In Wachter KW & Bulatao RA (Eds.), Offspring: Human fertility behavior in biodemographic perspective (pp. 46–90). Washington, DC: National Academies Press. [PubMed] [Google Scholar]
- Kolk M, Cownden D, & Enquist M.(2014). Correlations in fertility across generations: Can low fertility persist? Proceedings of the Royal Society B: Biological Sciences, 281, 20132561. 10.1098/rspb.2013.2561 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lam D. (1993). Comment on Preston and Campbell’s “Differential fertility and the distribution of traits.” American Journal of Sociology, 98, 1033–1039. [Google Scholar]
- Lam D.(2011). How the world survived the population bomb: Lessons from 50 years of extraordinary demographic history. Demography, 48, 1231–1262. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Morgan SP (2003). Is low fertility a twenty-first-century demographic crisis? Demography, 40, 589–603. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murphy M, & Wang D.(2003). The impact of intergenerationally-transmitted fertility and nuptiality on population dynamics in contemporary populations. In Rodgers JL & Kohler H-P (Eds.), The biodemography of human reproduction and fertility (pp. 209–228). New York, NY: Springer Science+Business Media. [Google Scholar]
- Perry SL, & Schleifer C.(2019). Are the faithful becoming less fruitful? The decline of conservative Protestant fertility and the growing importance of religious practice and belief in childbearing in the U.S. Social Science Research, 78, 137–155. [DOI] [PubMed] [Google Scholar]
- Preston SH, & Campbell C.(1993). Differential fertility and the distribution of traits: The case of IQ. American Journal of Sociology, 98, 997–1019. [Google Scholar]
- Pritchett LH (1994). Desired fertility and the impact of population policies. Population and Development Review, 20, 1–55. [Google Scholar]
- United Nations. (2019). World population prospects 2019: Highlights (Report). New York, NY: United Nations, Department of Economic and Social Affairs, Population Division. [Google Scholar]
- Vogl TS (2020). Intergenerational associations and the fertility transition. Journal of the European Economic Association, 18, 2972–3005. [Google Scholar]
- Vogl TS, & Freese J.(2020). Differential fertility makes society more conservative on family values. Proceedings of the National Academy of Sciences, 117, 7696–7701. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Vollset SE, Goren E, Yuan C-W, Cao J, Smith AE, Hsiao T, . . . Murray CJL (2020). Fertility, mortality, migration, and population scenarios for 195 countries and territories from 2017 to 2100: A forecasting analysis for the Global Burden of Disease Study. Lancet, 396, 1285–1306. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Yeatman S, Trinitapoli J, & Garver S.(2020). The enduring case for fertility desires. Demography, 57, 2047–2056. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.