Abstract
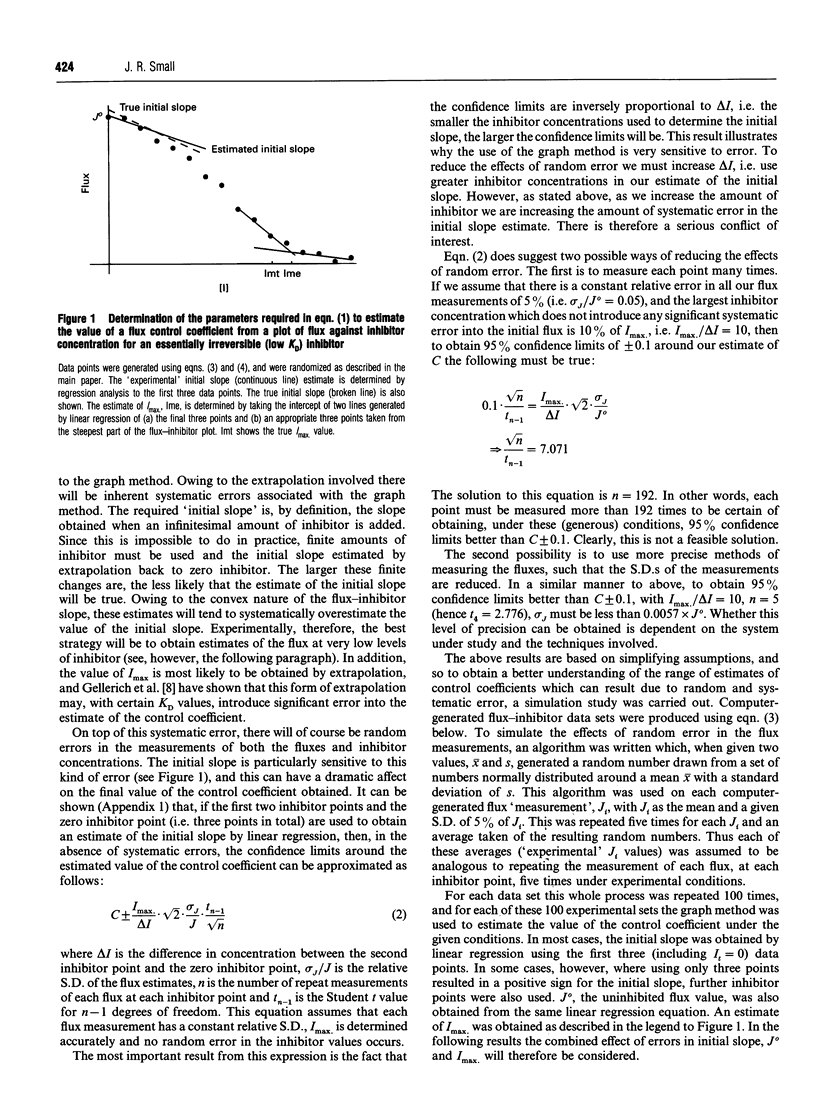
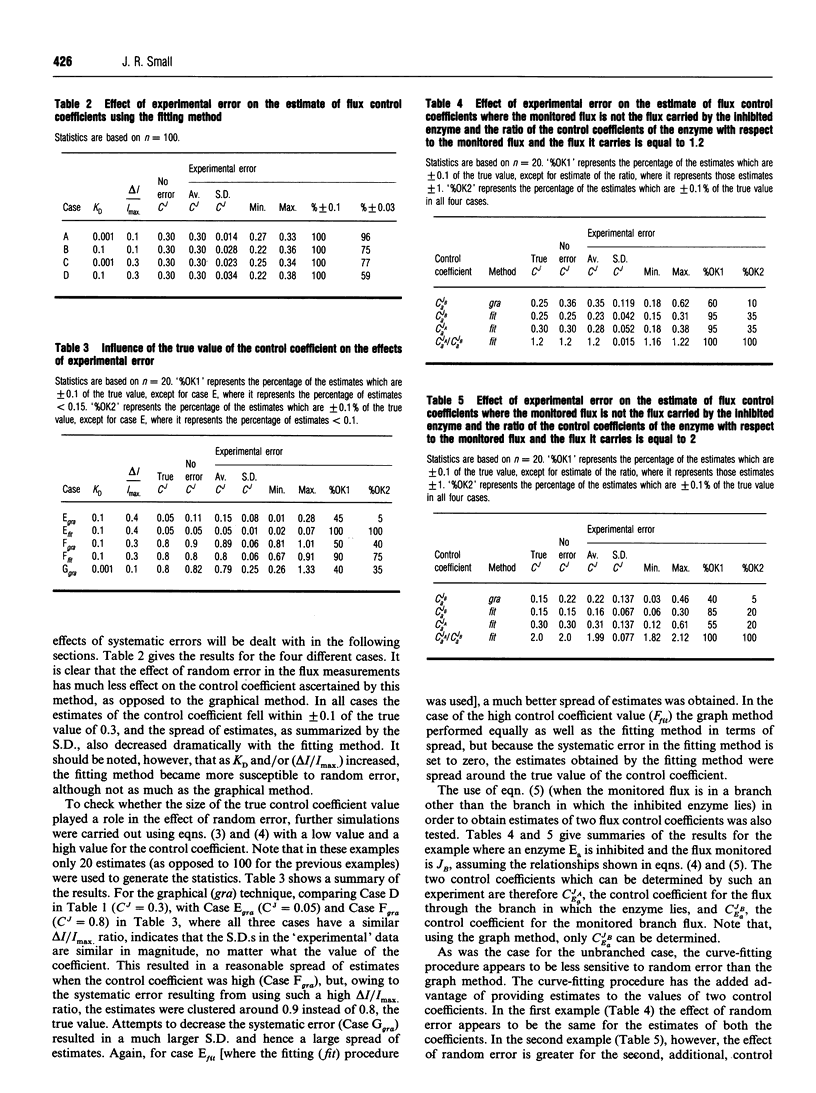
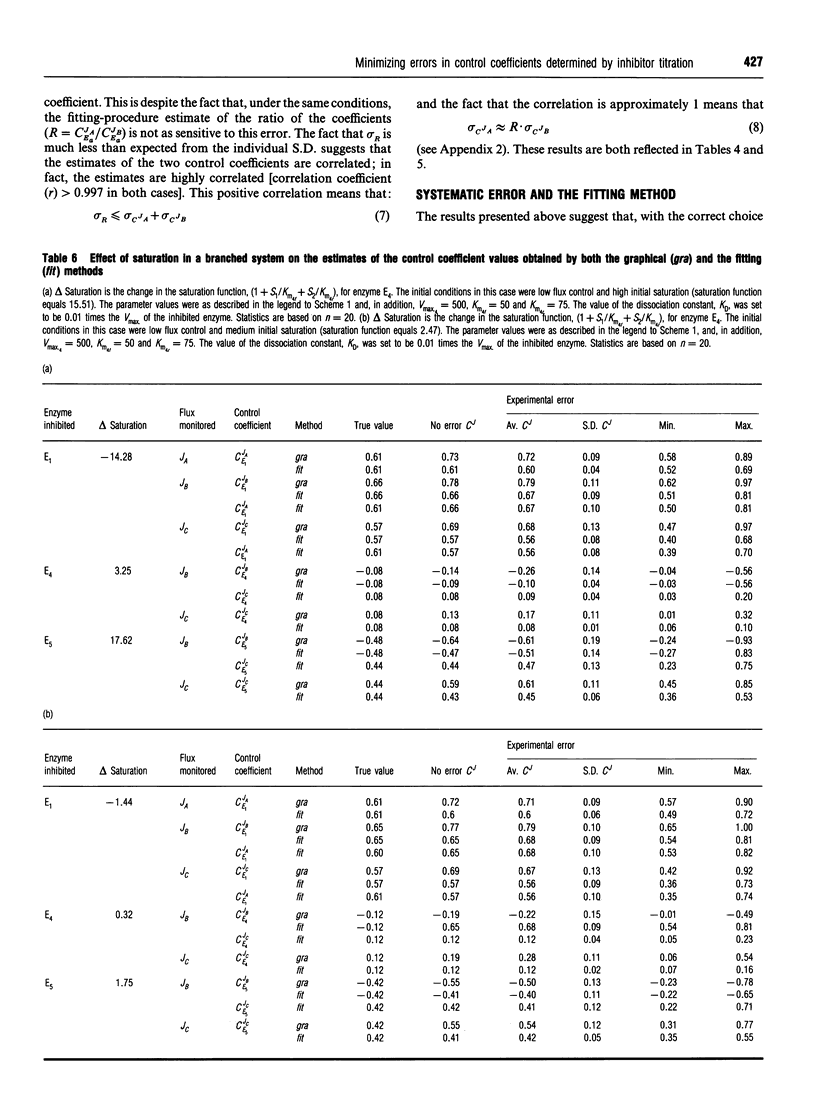
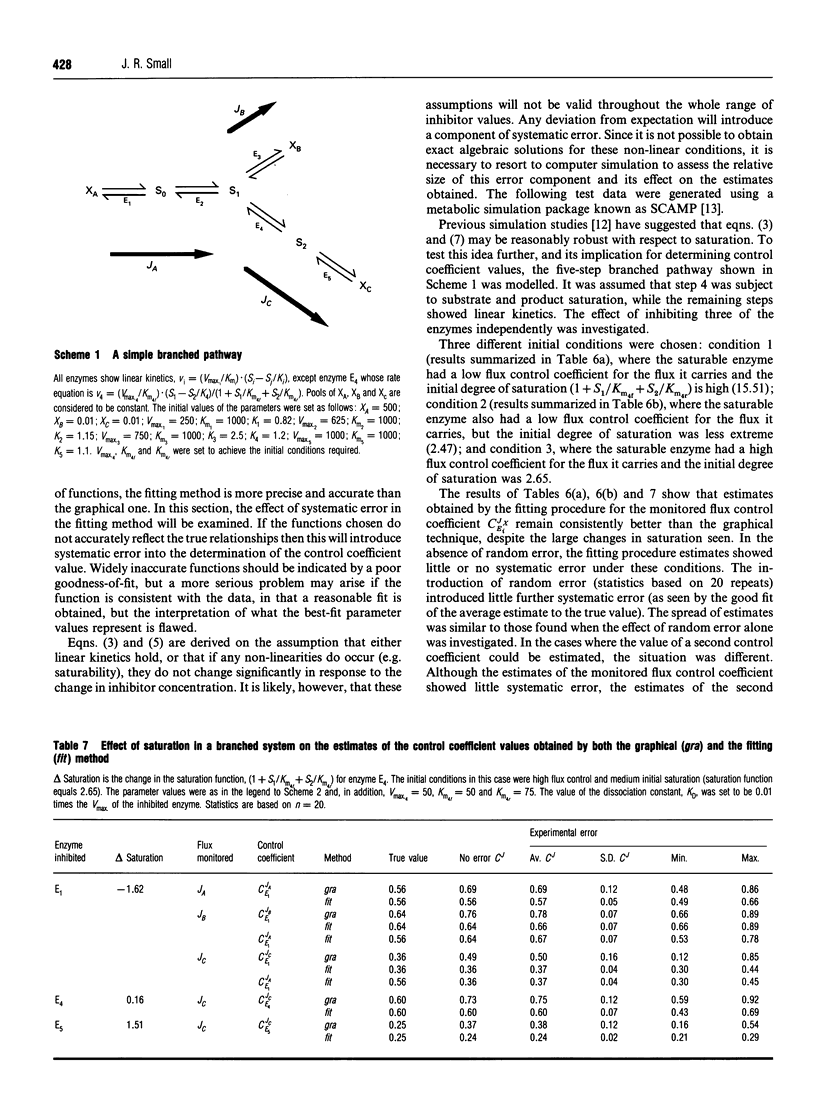
This paper is a study into the effects of experimental error on the estimated values of flux control coefficients obtained using specific inhibitors. Two possible techniques for analysing the experimental data are compared: a simple extrapolation method (the so-called graph method) and a non-linear function fitting method. For these techniques, the sources of systematic errors are identified and the effects of systematic and random errors are quantified, using both statistical analysis and numerical computation. It is shown that the graph method is very sensitive to random errors and, under all conditions studied, that the fitting method, even under conditions where the assumptions underlying the fitted function do not hold, outperformed the graph method. Possible ways of designing experiments to minimize the effects of experimental errors are analysed and discussed.
Full text
PDF





Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Brown G. C., Hafner R. P., Brand M. D. A 'top-down' approach to the determination of control coefficients in metabolic control theory. Eur J Biochem. 1990 Mar 10;188(2):321–325. doi: 10.1111/j.1432-1033.1990.tb15406.x. [DOI] [PubMed] [Google Scholar]
- Fell D. A. Metabolic control analysis: a survey of its theoretical and experimental development. Biochem J. 1992 Sep 1;286(Pt 2):313–330. doi: 10.1042/bj2860313. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gellerich F. N., Kunz W. S., Bohnensack R. Estimation of flux control coefficients from inhibitor titrations by non-linear regression. FEBS Lett. 1990 Nov 12;274(1-2):167–170. doi: 10.1016/0014-5793(90)81355-r. [DOI] [PubMed] [Google Scholar]
- Groen A. K., van Roermund C. W., Vervoorn R. C., Tager J. M. Control of gluconeogenesis in rat liver cells. Flux control coefficients of the enzymes in the gluconeogenic pathway in the absence and presence of glucagon. Biochem J. 1986 Jul 15;237(2):379–389. doi: 10.1042/bj2370379. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hafner R. P., Brown G. C., Brand M. D. Analysis of the control of respiration rate, phosphorylation rate, proton leak rate and protonmotive force in isolated mitochondria using the 'top-down' approach of metabolic control theory. Eur J Biochem. 1990 Mar 10;188(2):313–319. doi: 10.1111/j.1432-1033.1990.tb15405.x. [DOI] [PubMed] [Google Scholar]
- Heinrich R., Rapoport T. A. A linear steady-state treatment of enzymatic chains. General properties, control and effector strength. Eur J Biochem. 1974 Feb 15;42(1):89–95. doi: 10.1111/j.1432-1033.1974.tb03318.x. [DOI] [PubMed] [Google Scholar]
- Hofmeyr J. H., Cornish-Bowden A., Rohwer J. M. Taking enzyme kinetics out of control; putting control into regulation. Eur J Biochem. 1993 Mar 15;212(3):833–837. doi: 10.1111/j.1432-1033.1993.tb17725.x. [DOI] [PubMed] [Google Scholar]
- Kacser H., Burns J. A. The control of flux. Symp Soc Exp Biol. 1973;27:65–104. [PubMed] [Google Scholar]
- Letellier T., Malgat M., Mazat J. P. Control of oxidative phosphorylation in rat muscle mitochondria: implications for mitochondrial myopathies. Biochim Biophys Acta. 1993 Feb 8;1141(1):58–64. doi: 10.1016/0005-2728(93)90189-m. [DOI] [PubMed] [Google Scholar]
- Sauro H. M. SCAMP: a general-purpose simulator and metabolic control analysis program. Comput Appl Biosci. 1993 Aug;9(4):441–450. doi: 10.1093/bioinformatics/9.4.441. [DOI] [PubMed] [Google Scholar]
- Small J. R., Fell D. A. Metabolic control analysis. Sensitivity of control coefficients to elasticities. Eur J Biochem. 1990 Jul 31;191(2):413–420. doi: 10.1111/j.1432-1033.1990.tb19137.x. [DOI] [PubMed] [Google Scholar]
- Small J. R., Kacser H. Responses of metabolic systems to large changes in enzyme activities and effectors. 1. The linear treatment of unbranched chains. Eur J Biochem. 1993 Apr 1;213(1):613–624. doi: 10.1111/j.1432-1033.1993.tb17801.x. [DOI] [PubMed] [Google Scholar]
- Small J. R., Kacser H. Responses of metabolic systems to large changes in enzyme activities and effectors. 2. The linear treatment of branched pathways and metabolite concentrations. Assessment of the general non-linear case. Eur J Biochem. 1993 Apr 1;213(1):625–640. doi: 10.1111/j.1432-1033.1993.tb17802.x. [DOI] [PubMed] [Google Scholar]


