Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1997 Nov;73(5):2347–2358. doi: 10.1016/S0006-3495(97)78265-1

Effects of bath resistance on action potentials in the squid giant axon: myocardial implications.

J Wu 1, J P Wikswo Jr 1
PMCID: PMC1181138  PMID: 9370430

Abstract

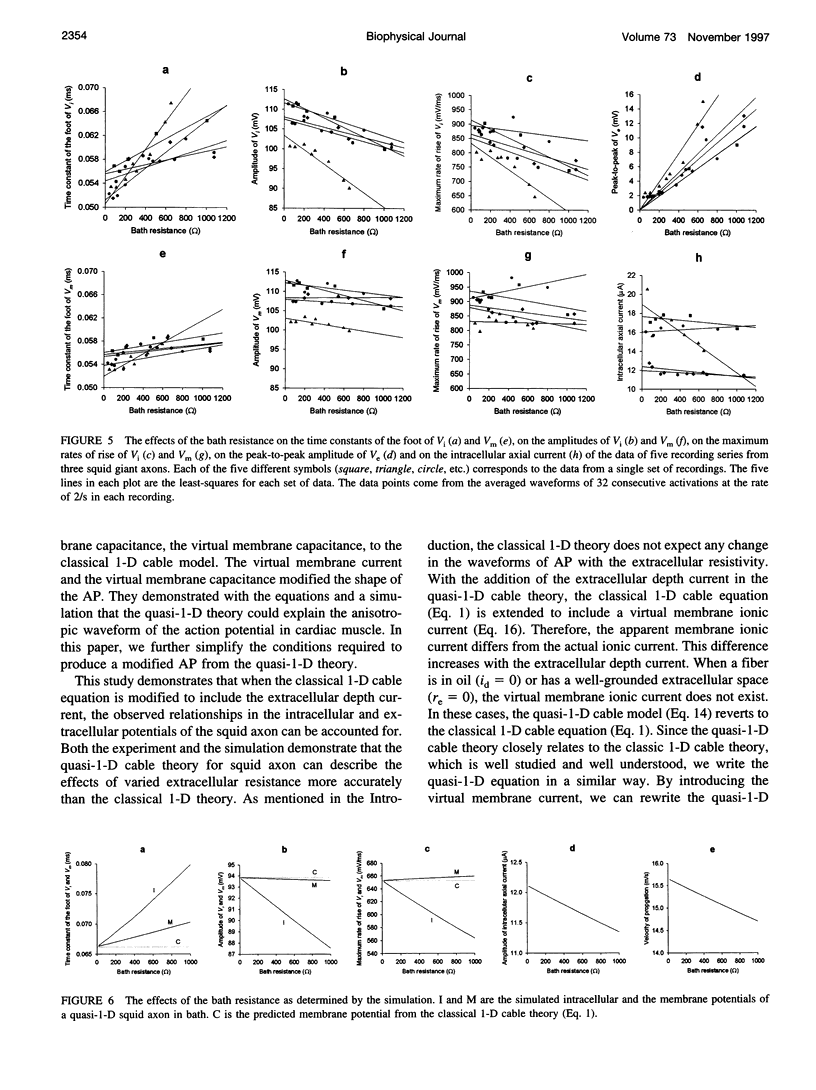
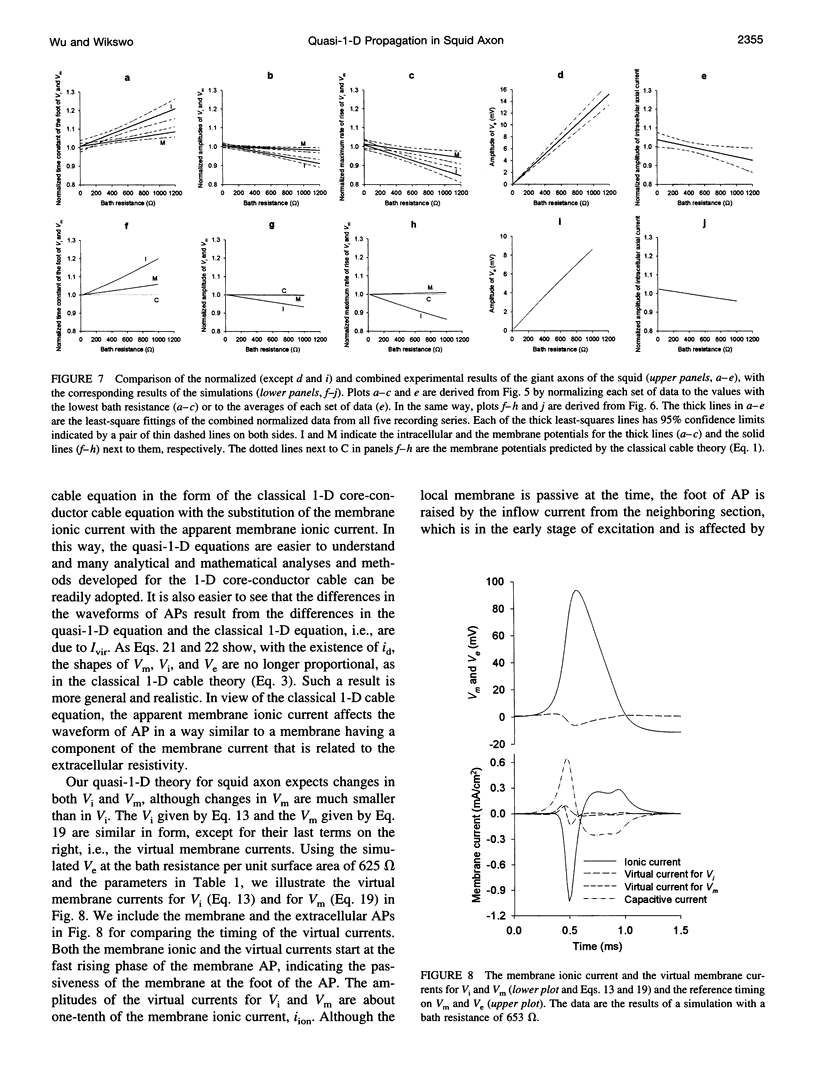
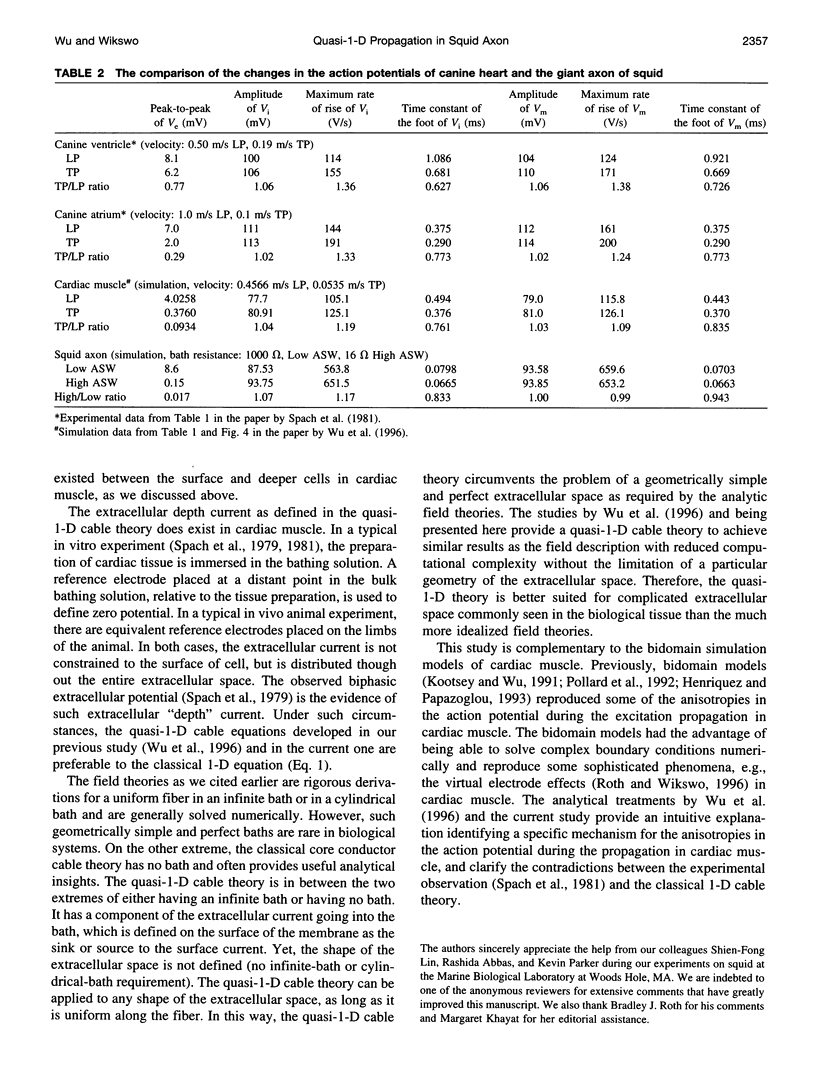
This study presents a simplified version of the quasi-one-dimensional theory (Wu, J., E. A. Johnson, and J. M. Kootsey. 1996. A quasi-one-dimensional theory for anisotropic propagation of excitation in cardiac muscle. Biophys. J. 71:2427-2439) with two components of the extracellular current, along and perpendicular to the axis, and a simulation and its experimental confirmation for the giant axon of the squid. By extending the one-dimensional core conductor cable equations, this theory predicts, as confirmed by the experiment, that the shapes of the intracellular and the extracellular action potentials are related to the resistance of the bath. Such a result was previously only expected by the field theories. The correlation between the shapes of the intracellular and the extracellular potentials of the giant axon of the squid resembles that observed during the anisotropic propagation of excitation in cardiac muscle. Therefore, this study not only develops a quasi-one-dimensional theory for a squid axon, but also provides one possible factor contributing to the anisotropic propagation of action potentials in cardiac muscle.

Full text

PDF
2356

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Andreassen S., Rosenfalck A. Relationship of intracellular and extracellular action potentials of skeletal muscle fibers. Crit Rev Bioeng. 1981 Nov;6(4):267–306. [PubMed] [Google Scholar]
  2. Clark J., Plonsey R. A mathematical evaluation of the core conductor model. Biophys J. 1966 Jan;6(1):95–112. doi: 10.1016/S0006-3495(66)86642-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Clark J., Plonsey R. The extracellular potential field of the single active nerve fiber in a volume conductor. Biophys J. 1968 Jul;8(7):842–864. doi: 10.1016/S0006-3495(68)86524-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Eisenberg R. S., Barcilon V., Mathias R. T. Electrical properties of spherical syncytia. Biophys J. 1979 Jan;25(1):151–180. doi: 10.1016/S0006-3495(79)85283-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. HODGKIN A. L. A note on conduction velocity. J Physiol. 1954 Jul 28;125(1):221–224. doi: 10.1113/jphysiol.1954.sp005152. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. HODGKIN A. L., HUXLEY A. F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J Physiol. 1952 Aug;117(4):500–544. doi: 10.1113/jphysiol.1952.sp004764. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. HODGKIN A. L., KATZ B. The effect of sodium ions on the electrical activity of giant axon of the squid. J Physiol. 1949 Mar 1;108(1):37–77. doi: 10.1113/jphysiol.1949.sp004310. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Henriquez C. S., Muzikant A. L., Smoak C. K. Anisotropy, fiber curvature, and bath loading effects on activation in thin and thick cardiac tissue preparations: simulations in a three-dimensional bidomain model. J Cardiovasc Electrophysiol. 1996 May;7(5):424–444. doi: 10.1111/j.1540-8167.1996.tb00548.x. [DOI] [PubMed] [Google Scholar]
  9. Henriquez C. S., Plonsey R. Simulation of propagation along a cylindrical bundle of cardiac tissue--I: Mathematical formulation. IEEE Trans Biomed Eng. 1990 Sep;37(9):850–860. doi: 10.1109/10.58596. [DOI] [PubMed] [Google Scholar]
  10. Henriquez C. S., Trayanova N., Plonsey R. Potential and current distributions in a cylindrical bundle of cardiac tissue. Biophys J. 1988 Jun;53(6):907–918. doi: 10.1016/S0006-3495(88)83172-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Leon L. J., Roberge F. A. A new cable model formulation based on Green's theorem. Ann Biomed Eng. 1990;18(1):1–17. doi: 10.1007/BF02368414. [DOI] [PubMed] [Google Scholar]
  12. Leon L. J., Roberge F. A. Structural complexity effects on transverse propagation in a two-dimensional model of myocardium. IEEE Trans Biomed Eng. 1991 Oct;38(10):997–1009. doi: 10.1109/10.88445. [DOI] [PubMed] [Google Scholar]
  13. Lieberman M., Sawanobori T., Kootsey J. M., Johnson E. A. A synthetic strand of cardiac muscle: its passive electrical properties. J Gen Physiol. 1975 Apr;65(4):527–550. doi: 10.1085/jgp.65.4.527. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Plonsey R. The active fiber in a volume conductor. IEEE Trans Biomed Eng. 1974 Sep;21(5):371–381. doi: 10.1109/TBME.1974.324406. [DOI] [PubMed] [Google Scholar]
  15. Pollard A. E., Hooke N., Henriquez C. S. Cardiac propagation simulation. Crit Rev Biomed Eng. 1992;20(3-4):171–210. [PubMed] [Google Scholar]
  16. Roth B. J. Effect of a perfusing bath on the rate of rise of an action potential propagating through a slab of cardiac tissue. Ann Biomed Eng. 1996 Nov-Dec;24(6):639–646. doi: 10.1007/BF02684177. [DOI] [PubMed] [Google Scholar]
  17. Rudy Y., Quan W. L. A model study of the effects of the discrete cellular structure on electrical propagation in cardiac tissue. Circ Res. 1987 Dec;61(6):815–823. doi: 10.1161/01.res.61.6.815. [DOI] [PubMed] [Google Scholar]
  18. Shiba H. Heaviside's "Bessel cable" as an electric model for flat simple epithelial cells with low resistive junctional membranes. J Theor Biol. 1971 Jan;30(1):59–68. doi: 10.1016/0022-5193(71)90036-1. [DOI] [PubMed] [Google Scholar]
  19. Shiba H., Kanno Y. Further study of the two-dimensional cable theory: an electric model for a flat thin association of cells with a directional intercellular communication. Biophysik. 1971;7(4):295–301. doi: 10.1007/BF01190241. [DOI] [PubMed] [Google Scholar]
  20. Spach M. S., Dolber P. C., Heidlage J. F., Kootsey J. M., Johnson E. A. Propagating depolarization in anisotropic human and canine cardiac muscle: apparent directional differences in membrane capacitance. A simplified model for selective directional effects of modifying the sodium conductance on Vmax, tau foot, and the propagation safety factor. Circ Res. 1987 Feb;60(2):206–219. doi: 10.1161/01.res.60.2.206. [DOI] [PubMed] [Google Scholar]
  21. Spach M. S., Heidlage J. F., Darken E. R., Hofer E., Raines K. H., Starmer C. F. Cellular Vmax reflects both membrane properties and the load presented by adjoining cells. Am J Physiol. 1992 Dec;263(6 Pt 2):H1855–H1863. doi: 10.1152/ajpheart.1992.263.6.H1855. [DOI] [PubMed] [Google Scholar]
  22. Spach M. S., Heidlage J. F. The stochastic nature of cardiac propagation at a microscopic level. Electrical description of myocardial architecture and its application to conduction. Circ Res. 1995 Mar;76(3):366–380. doi: 10.1161/01.res.76.3.366. [DOI] [PubMed] [Google Scholar]
  23. Spach M. S., Miller W. T., 3rd, Geselowitz D. B., Barr R. C., Kootsey J. M., Johnson E. A. The discontinuous nature of propagation in normal canine cardiac muscle. Evidence for recurrent discontinuities of intracellular resistance that affect the membrane currents. Circ Res. 1981 Jan;48(1):39–54. doi: 10.1161/01.res.48.1.39. [DOI] [PubMed] [Google Scholar]
  24. Spach M. S., Miller W. T., 3rd, Miller-Jones E., Warren R. B., Barr R. C. Extracellular potentials related to intracellular action potentials during impulse conduction in anisotropic canine cardiac muscle. Circ Res. 1979 Aug;45(2):188–204. doi: 10.1161/01.res.45.2.188. [DOI] [PubMed] [Google Scholar]
  25. Spach M. S. The discontinuous nature of electrical propagation in cardiac muscle. Consideration of a quantitative model incorporating the membrane ionic properties and structural complexities. The ALZA distinguished lecture. Ann Biomed Eng. 1983;11(3-4):209–261. doi: 10.1007/BF02363287. [DOI] [PubMed] [Google Scholar]
  26. Suenson M. Interaction between ventricular cells during the early part of excitation in the ferret heart. Acta Physiol Scand. 1985 Sep;125(1):81–90. doi: 10.1111/j.1748-1716.1985.tb07694.x. [DOI] [PubMed] [Google Scholar]
  27. Trayanova N. A., Henriquez C. S., Plonsey R. Limitations of approximate solutions for computing the extracellular potential of single fibers and bundle equivalents. IEEE Trans Biomed Eng. 1990 Jan;37(1):22–35. doi: 10.1109/10.43608. [DOI] [PubMed] [Google Scholar]
  28. Trayanova N., Henriquez C. S. Modification of a cylindrical bidomain model for cardiac tissue. Math Biosci. 1991 Apr;104(1):59–72. doi: 10.1016/0025-5564(91)90030-m. [DOI] [PubMed] [Google Scholar]
  29. Trayanova N., Henriquez C. S., Plonsey R. Extracellular potentials and currents of a single active fiber in a restricted volume conductor. Ann Biomed Eng. 1990;18(3):219–238. doi: 10.1007/BF02368439. [DOI] [PubMed] [Google Scholar]
  30. Wikswo J. P., Jr, Samson P. C., Giffard R. P. A low-noise low input impedance amplifier for magnetic measurements of nerve action currents. IEEE Trans Biomed Eng. 1983 Apr;30(4):215–221. doi: 10.1109/tbme.1983.325220. [DOI] [PubMed] [Google Scholar]
  31. Wikswo J. P., Jr, van Egeraat J. M. Cellular magnetic fields: fundamental and applied measurements on nerve axons, peripheral nerve bundles, and skeletal muscle. J Clin Neurophysiol. 1991 Apr;8(2):170–188. [PubMed] [Google Scholar]
  32. Wu J., Johnson E. A., Kootsey J. M. A quasi-one-dimensional theory for anisotropic propagation of excitation in cardiac muscle. Biophys J. 1996 Nov;71(5):2427–2439. doi: 10.1016/S0006-3495(96)79436-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  33. van Egeraat J. M., Wikswo J. P., Jr A model for axonal propagation incorporating both radial and axial ionic transport. Biophys J. 1993 Apr;64(4):1287–1298. doi: 10.1016/S0006-3495(93)81495-4. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES