Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1994 Oct;67(4):1669–1677. doi: 10.1016/S0006-3495(94)80639-3

A program for developing a comprehensive mathematical description of the crossbridge cycle of muscle.

M P Slawnych 1, C Y Seow 1, A F Huxley 1, L E Ford 1
PMCID: PMC1225528  PMID: 7819498

Abstract

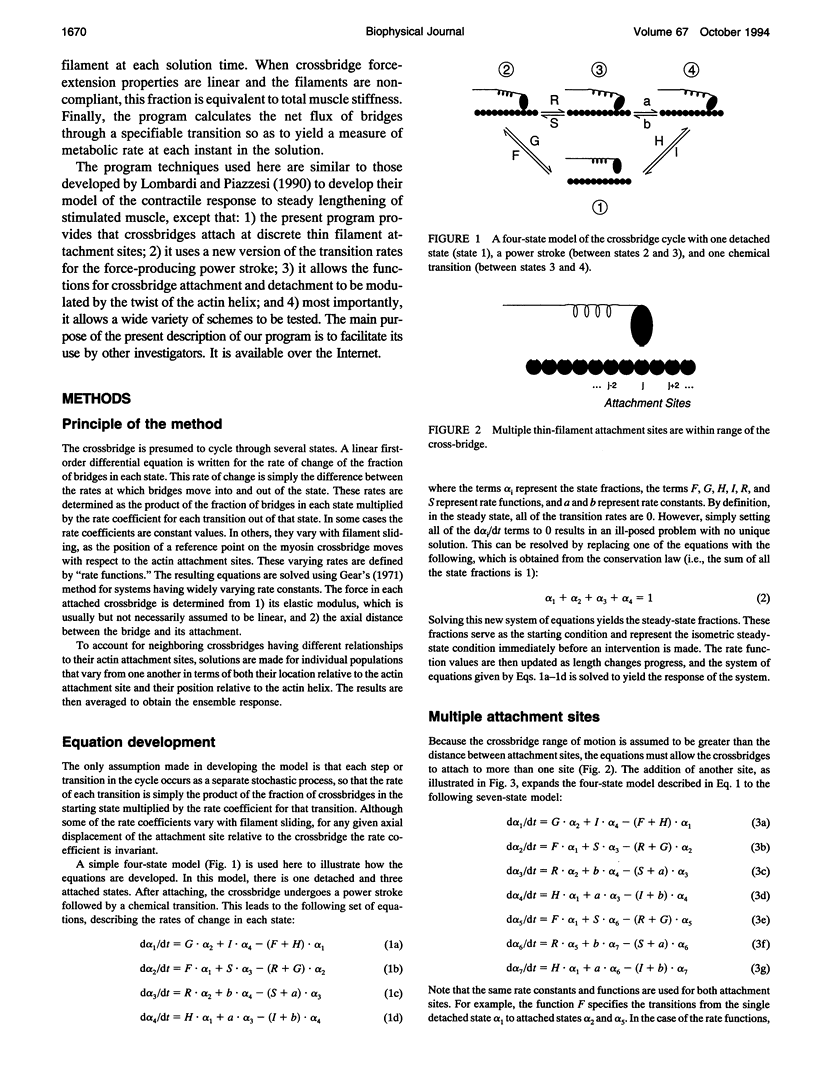
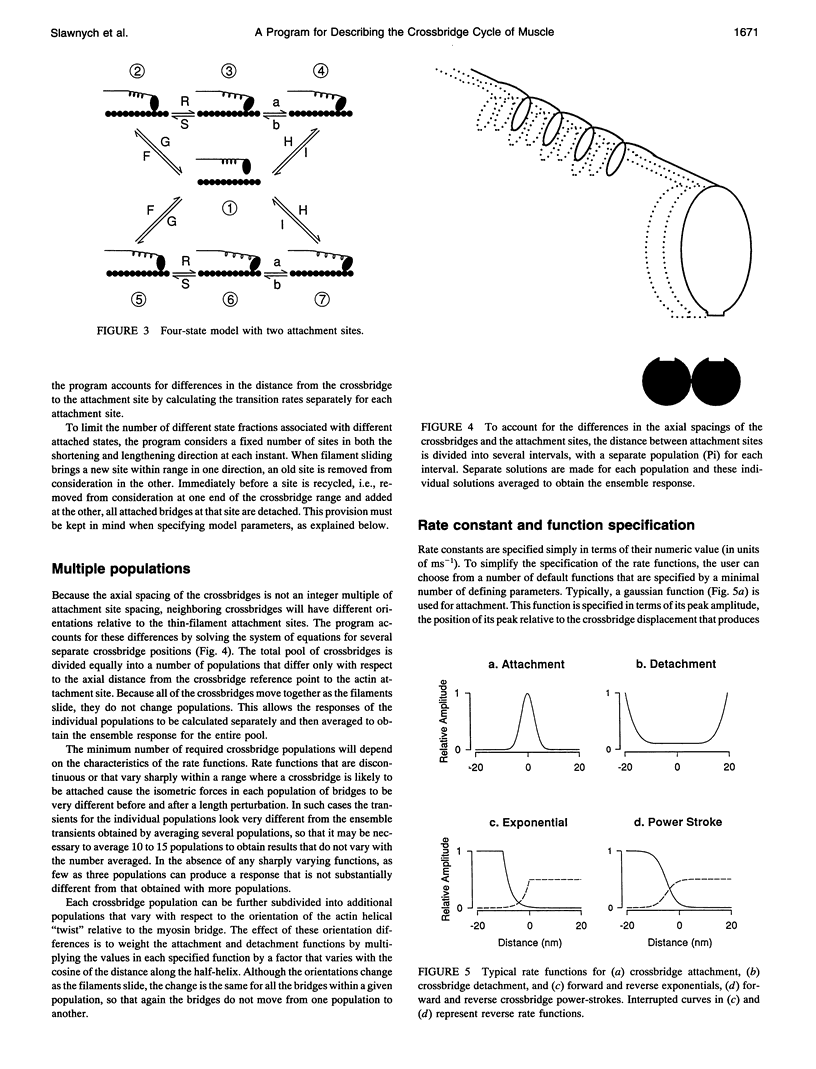
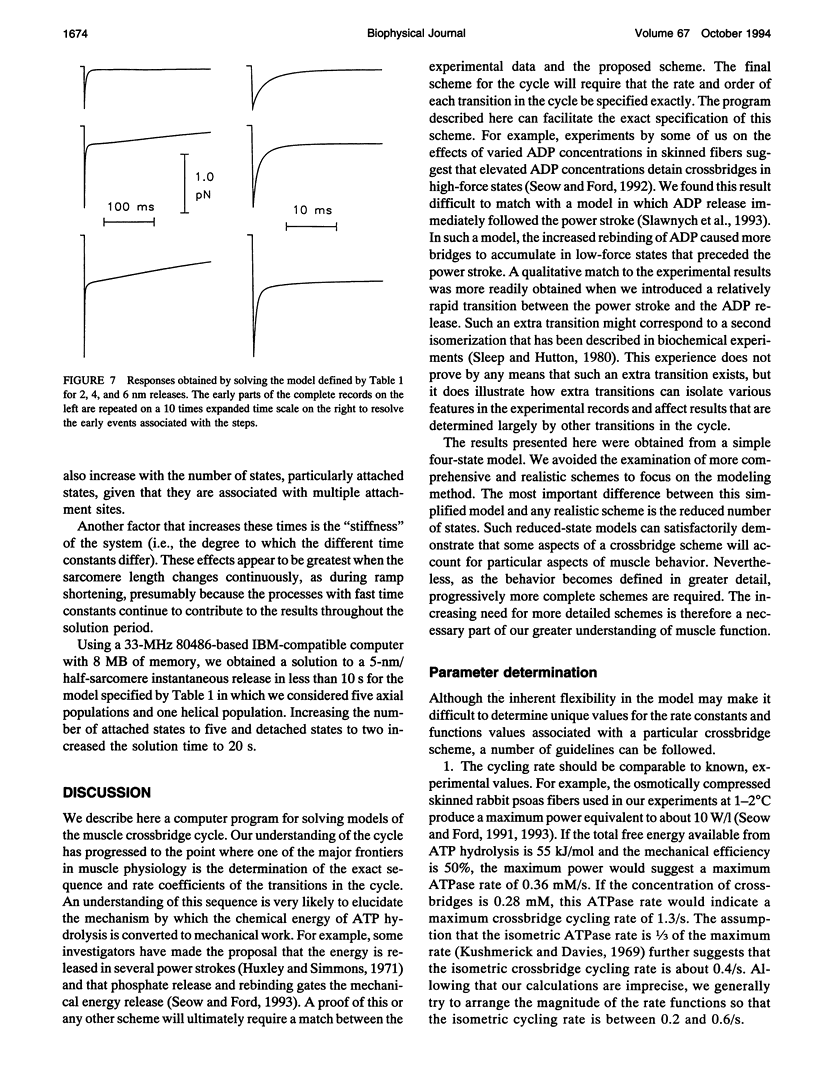
We describe a computer modeling system for determining the changes of force, fraction of attached crossbridges, and crossbridge flux rate through a specifiable transition in response to length changes imposed on a crossbridge model of muscle. The crossbridge cycle is divided into multiple attached and detached states. The rates of transition from one state to another are defined by rate coefficients that can either be constant or vary with the position of the crossbridge relative to the thin-filament attachment site. This scheme leads to a system of differential equations defining the rates of change for the fractions of bridges in each state. Solutions for this system of equations are obtained at specified times during and after a length change using a method for systems with widely varying time constants (C. W. Gear, 1971, Numerical Initial Value Problems in Ordinary Differential Equations, Prentice-Hall, Englewood Cliffs, NJ). Crossbridges are divided into discrete populations that differ both in their axial displacement with respect to thin filament attachment sites and with respect to the twist of the actin helix. Separate solutions are made for the individual populations and are then averaged to obtain the ensemble response. Force is determined as the sum of the product of the force associated with each state multiplied by the fraction of bridges in that state. A measure of metabolic rate is determined as the net flux through one of the crossbridge transitions. When the force-extension characteristics of the individual crossbridges are linear and the filaments are noncompliant the fraction of attached bridges is equivalent to sarcomere stiffness. To illustrate the operation of the program, we also describe here some results obtained with a simplified scheme.

Full text

PDF
1672

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Eisenberg E., Greene L. E. The relation of muscle biochemistry to muscle physiology. Annu Rev Physiol. 1980;42:293–309. doi: 10.1146/annurev.ph.42.030180.001453. [DOI] [PubMed] [Google Scholar]
  2. Eisenberg E., Hill T. L. A cross-bridge model of muscle contraction. Prog Biophys Mol Biol. 1978;33(1):55–82. doi: 10.1016/0079-6107(79)90025-7. [DOI] [PubMed] [Google Scholar]
  3. Eisenberg E., Hill T. L. Muscle contraction and free energy transduction in biological systems. Science. 1985 Mar 1;227(4690):999–1006. doi: 10.1126/science.3156404. [DOI] [PubMed] [Google Scholar]
  4. Ford L. E., Huxley A. F., Simmons R. M. Tension responses to sudden length change in stimulated frog muscle fibres near slack length. J Physiol. 1977 Jul;269(2):441–515. doi: 10.1113/jphysiol.1977.sp011911. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Geeves M. A., Goody R. S., Gutfreund H. Kinetics of acto-S1 interaction as a guide to a model for the crossbridge cycle. J Muscle Res Cell Motil. 1984 Aug;5(4):351–361. doi: 10.1007/BF00818255. [DOI] [PubMed] [Google Scholar]
  6. Geeves M. A. The dynamics of actin and myosin association and the crossbridge model of muscle contraction. Biochem J. 1991 Feb 15;274(Pt 1):1–14. doi: 10.1042/bj2740001. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. HUXLEY A. F. Muscle structure and theories of contraction. Prog Biophys Biophys Chem. 1957;7:255–318. [PubMed] [Google Scholar]
  8. Hill T. L. Theoretical formalism for the sliding filament model of contraction of striated muscle. Part I. Prog Biophys Mol Biol. 1974;28:267–340. doi: 10.1016/0079-6107(74)90020-0. [DOI] [PubMed] [Google Scholar]
  9. Hill T. L. Theoretical formalism for the sliding filament model of contraction of striated muscle. Part II. Prog Biophys Mol Biol. 1975;29(2):105–159. doi: 10.1016/0079-6107(76)90021-3. [DOI] [PubMed] [Google Scholar]
  10. Huxley A. F., Simmons R. M. Proposed mechanism of force generation in striated muscle. Nature. 1971 Oct 22;233(5321):533–538. doi: 10.1038/233533a0. [DOI] [PubMed] [Google Scholar]
  11. Julian F. J., Sollins K. R., Sollins M. R. A model for the transient and steady-state mechanical behavior of contracting muscle. Biophys J. 1974 Jul;14(7):546–562. doi: 10.1016/S0006-3495(74)85934-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Kawai M., Zhao Y. Cross-bridge scheme and force per cross-bridge state in skinned rabbit psoas muscle fibers. Biophys J. 1993 Aug;65(2):638–651. doi: 10.1016/S0006-3495(93)81109-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Kushmerick M. J., Davies R. E. The chemical energetics of muscle contraction. II. The chemistry, efficiency and power of maximally working sartorius muscles. Appendix. Free energy and enthalpy of atp hydrolysis in the sarcoplasm. Proc R Soc Lond B Biol Sci. 1969 Dec 23;174(1036):315–353. doi: 10.1098/rspb.1969.0096. [DOI] [PubMed] [Google Scholar]
  14. Lombardi V., Piazzesi G. The contractile response during steady lengthening of stimulated frog muscle fibres. J Physiol. 1990 Dec;431:141–171. doi: 10.1113/jphysiol.1990.sp018324. [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Lymn R. W., Taylor E. W. Mechanism of adenosine triphosphate hydrolysis by actomyosin. Biochemistry. 1971 Dec 7;10(25):4617–4624. doi: 10.1021/bi00801a004. [DOI] [PubMed] [Google Scholar]
  16. Podolsky R. J., Nolan A. C., Zaveler S. A. Cross-bridge properties derived from muscle isotonic velocity transients. Proc Natl Acad Sci U S A. 1969 Oct;64(2):504–511. doi: 10.1073/pnas.64.2.504. [DOI] [PMC free article] [PubMed] [Google Scholar]
  17. Schoenberg M. Equilibrium muscle cross-bridge behavior. Theoretical considerations. Biophys J. 1985 Sep;48(3):467–475. doi: 10.1016/S0006-3495(85)83802-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Sleep J. A., Hutton R. L. Exchange between inorganic phosphate and adenosine 5'-triphosphate in the medium by actomyosin subfragment 1. Biochemistry. 1980 Apr 1;19(7):1276–1283. doi: 10.1021/bi00548a002. [DOI] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES