Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1980 May;30(2):209–220. doi: 10.1016/S0006-3495(80)85090-9

Nonequilibrium linear behavior of biological systems. Existence of enzyme-mediated multidimensional inflection points.

K J Rothschild, S A Ellias, A Essig, H E Stanley
PMCID: PMC1328730  PMID: 7260273

Abstract

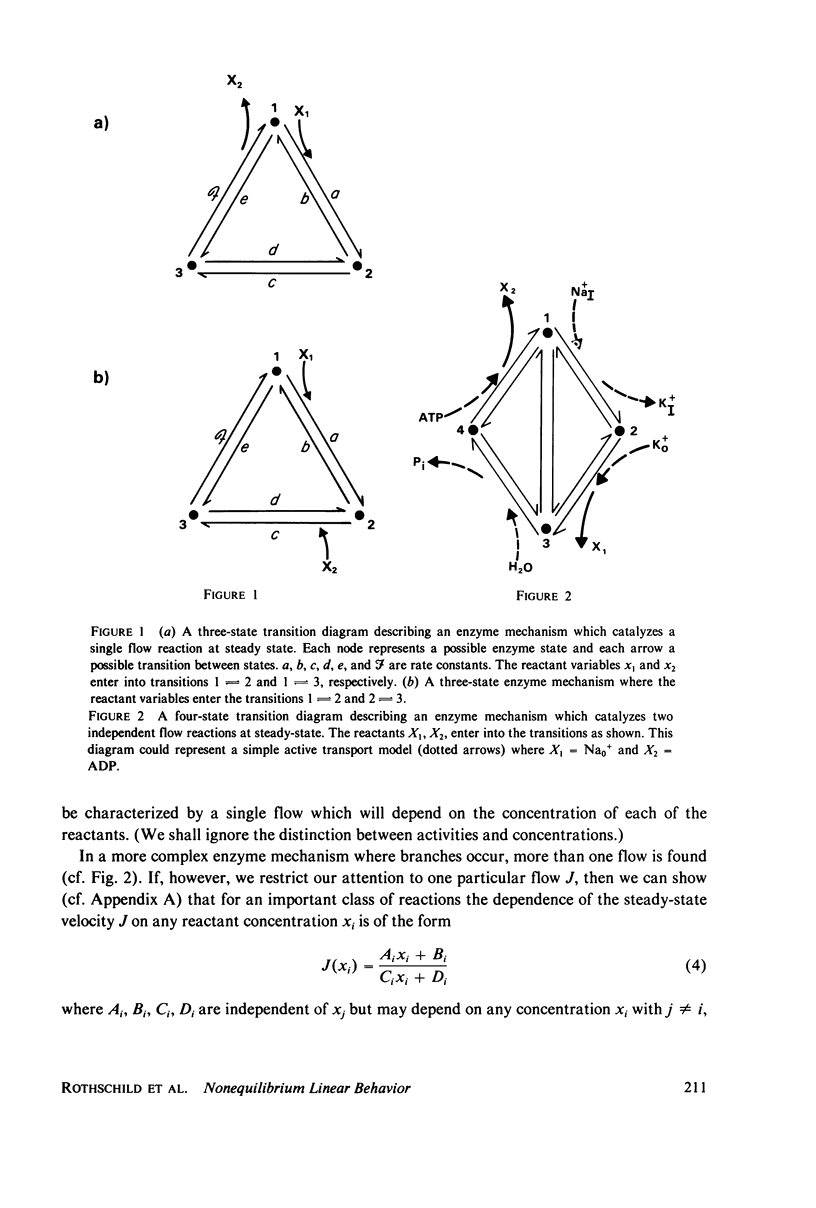
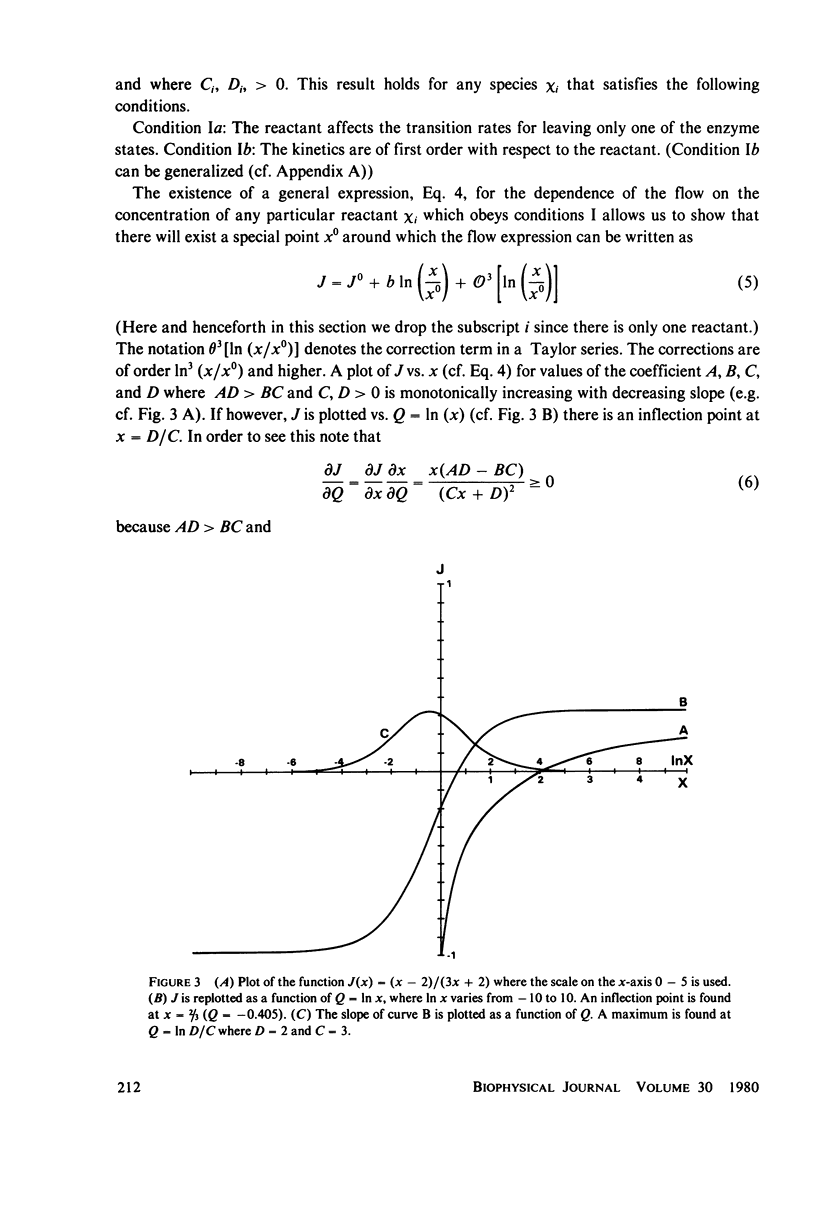
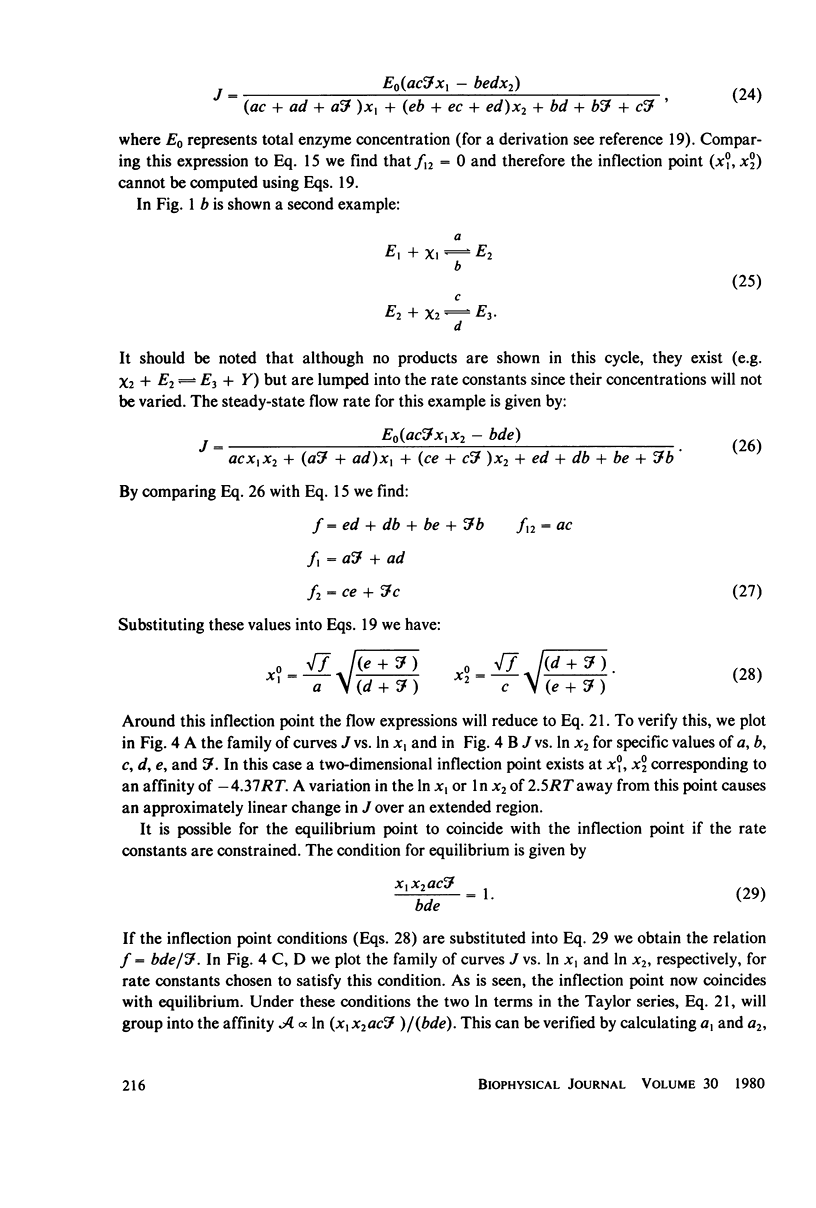
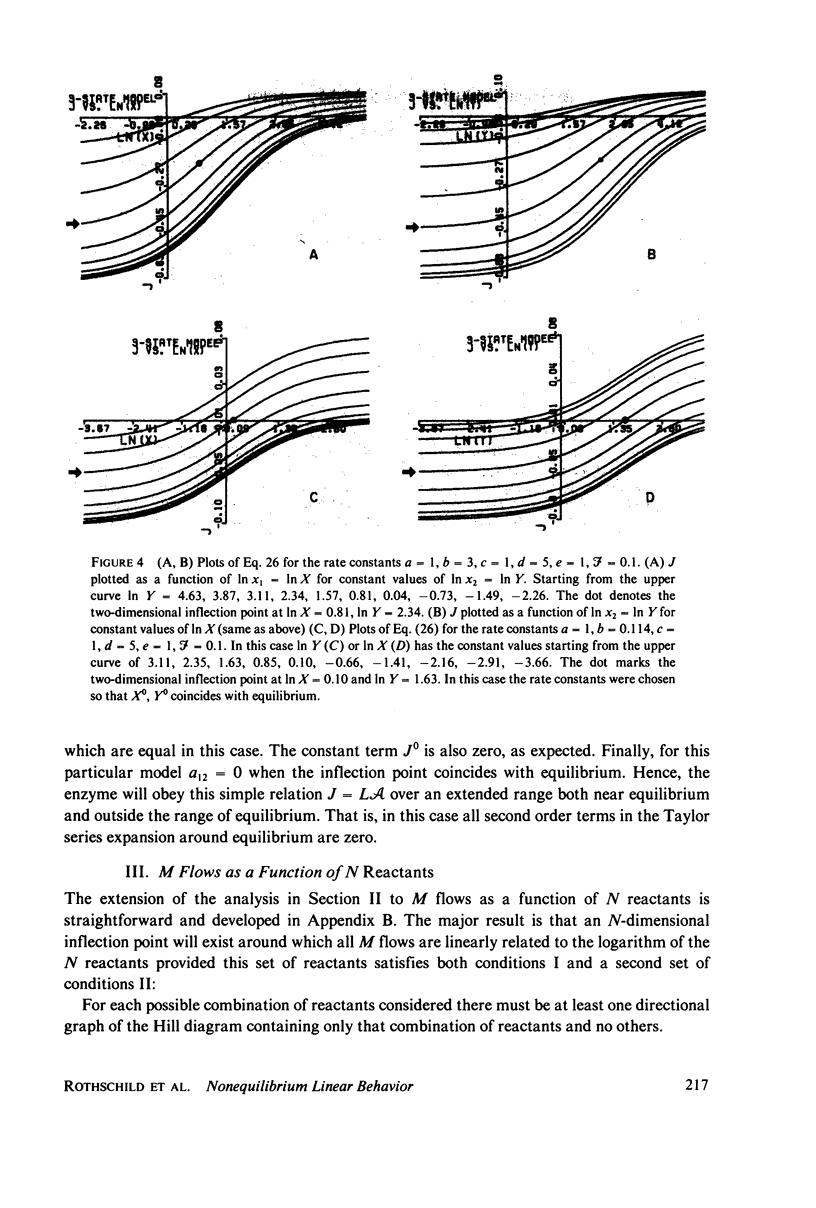
The linear phenomenological equations of nonequilibrium thermodynamics are limited theoretically to near equilibrium although a number of biological systems have been shown to exhibit a "linear" relationship between steady-state flows and conjugate thermodynamic forces outside the range of equilibrium. We have found a multidimensional inflection point which can exist well outside the range of equilibrium around with enzyme-catalyzed reactions exhibit "linear" behavior between the logarithm of reactant concentrations and enzyme catalyzed flows. A set of sufficient conditions has been derived which can be applied to any enzyme mechanism to determine whether a multidimensional inflection point exists. The conditions do not appear overly restrictive and may be satisfied by a large variety of coupled enzyme reactions. It is thus possible that the linearity observed in some biological systems may be explained in terms of enzyme operating near this multidimensional point.

Full text

PDF
212

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Beauwens R., Al-Awqati Q. Active H+ transport in the turtle urinary bladder. Coupling of transport to glucose oxidation. J Gen Physiol. 1976 Oct;68(4):421–439. doi: 10.1085/jgp.68.4.421. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Blumenthal R., Caplan S. R., Kedem O. The coupling of an enzymatic reaction to transmembrane flow of electric current in a synthetic "active transport" system. Biophys J. 2008 Dec 31;7(6):735–757. doi: 10.1016/S0006-3495(67)86620-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Bunow B. Chemical reactions and membranes: a macroscopic basis for facilitated transport, chemiosmosis and active transport. Part I: Linear analysis. J Theor Biol. 1978 Nov 7;75(1):51–78. doi: 10.1016/0022-5193(78)90202-3. [DOI] [PubMed] [Google Scholar]
  4. Caplan S. R., Essig A. Oxidative phosphorylation: thermodynamic criteria for the chemical and chemiosmotic hypotheses. Proc Natl Acad Sci U S A. 1969 Sep;64(1):211–218. doi: 10.1073/pnas.64.1.211. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Danisi G., Vieira F. L. Nonequilibrium thermodynamic analysis of the coupling between active sodium transport and oxygen consumption. J Gen Physiol. 1974 Sep;64(3):372–391. doi: 10.1085/jgp.64.3.372. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Essig A., Caplan S. R. Energetics of active transport processes. Biophys J. 1968 Dec;8(12):1434–1457. doi: 10.1016/S0006-3495(68)86565-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Essig A., Caplan S. R. Energetics of active transport processes. Biophys J. 1968 Dec;8(12):1434–1457. doi: 10.1016/S0006-3495(68)86565-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Green D. E., Ji S. The electromechanochemical model of mitochondrial structure and function. J Bioenerg. 1972 May;3(1):159–202. doi: 10.1007/BF01516006. [DOI] [PubMed] [Google Scholar]
  9. Hill T. L., Kedem O. Studies in irreversible thermodynamics. 3. Models for steady state and active transport across membranes. J Theor Biol. 1966 Apr;10(3):399–441. doi: 10.1016/0022-5193(66)90136-6. [DOI] [PubMed] [Google Scholar]
  10. Hill T. L. Studies in irreversible thermodynamics. IV. Diagrammatic representation of steady state fluxes for unimolecular systems. J Theor Biol. 1966 Apr;10(3):442–459. doi: 10.1016/0022-5193(66)90137-8. [DOI] [PubMed] [Google Scholar]
  11. Katchalsky A., Spangler R. Dynamics of membrane processes. Q Rev Biophys. 1968 Jun;1(2):127–175. doi: 10.1017/s0033583500000524. [DOI] [PubMed] [Google Scholar]
  12. Lang M. A., Caplan S. R., Essig A. Thermodynamic analysis of active sodium transport and oxidative metabolism in toad urinary bladder. J Membr Biol. 1977 Feb 24;31(1-2):19–29. doi: 10.1007/BF01869397. [DOI] [PubMed] [Google Scholar]
  13. MITCHELL P. Coupling of phosphorylation to electron and hydrogen transfer by a chemi-osmotic type of mechanism. Nature. 1961 Jul 8;191:144–148. doi: 10.1038/191144a0. [DOI] [PubMed] [Google Scholar]
  14. Mikulecky D. C. Global flow equations for membrane transport from local equations of motion: I. The general case for (n-1) nonelectrolyte solutes plus water. Bull Math Biol. 1978;40(6):791–805. doi: 10.1007/BF02460607. [DOI] [PubMed] [Google Scholar]
  15. Oster G. F., Perelson A. S., Katchalsky A. Network thermodynamics: dynamic modelling of biophysical systems. Q Rev Biophys. 1973 Feb;6(1):1–134. doi: 10.1017/s0033583500000081. [DOI] [PubMed] [Google Scholar]
  16. PRIGOGINE I., OUTER P., HERBO C. Affinity and reaction rate close to equilibrium. J Phys Colloid Chem. 1948 Feb;52(2):321–331. doi: 10.1021/j150458a004. [DOI] [PubMed] [Google Scholar]
  17. Rottenberg H., Gutman M. Control of the rate of reverse electron transport in submitochondrial particles by the free energy. Biochemistry. 1977 Jul 12;16(14):3220–3227. doi: 10.1021/bi00633a028. [DOI] [PubMed] [Google Scholar]
  18. Rottenberg H. The thermodynamic description of enzyme-catalyzed reactions. The linear relation between the reaction rate and the affinity. Biophys J. 1973 Jun;13(6):503–511. doi: 10.1016/S0006-3495(73)86004-7. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES