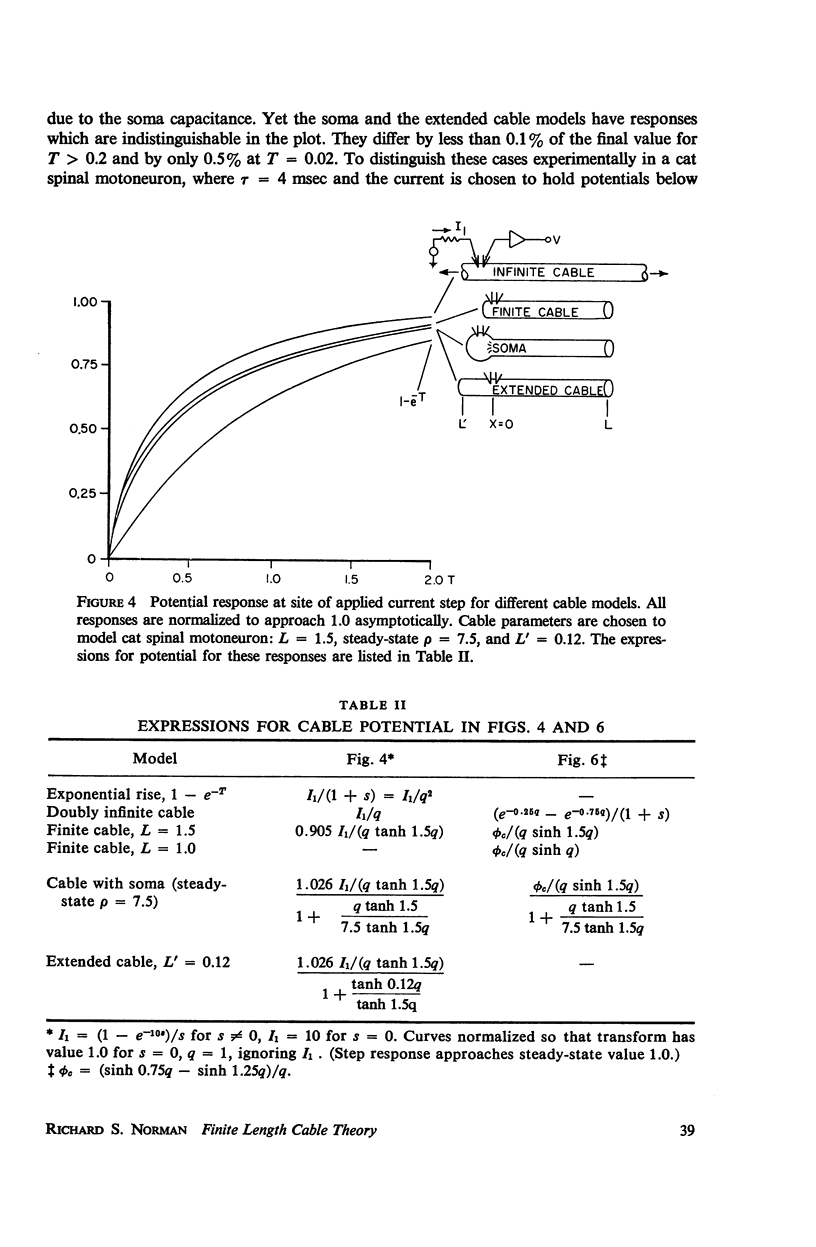
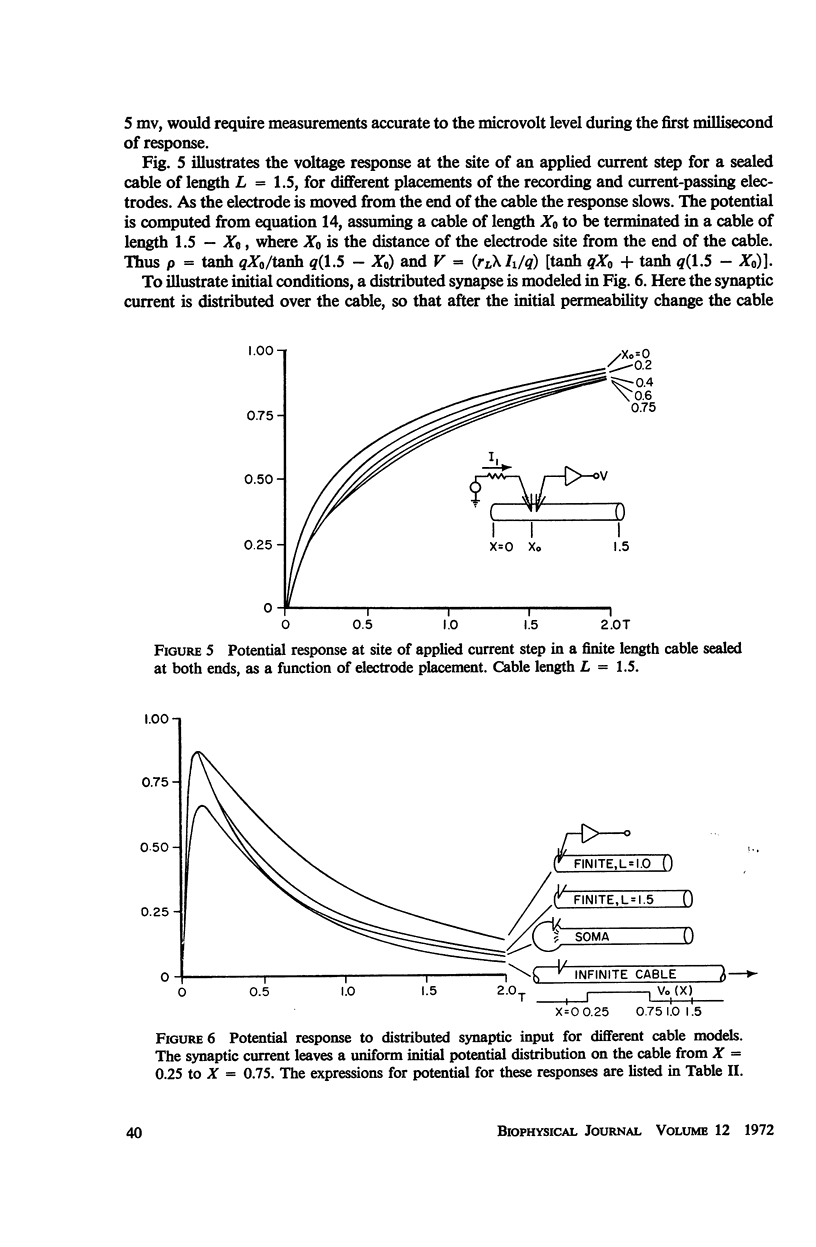
Abstract
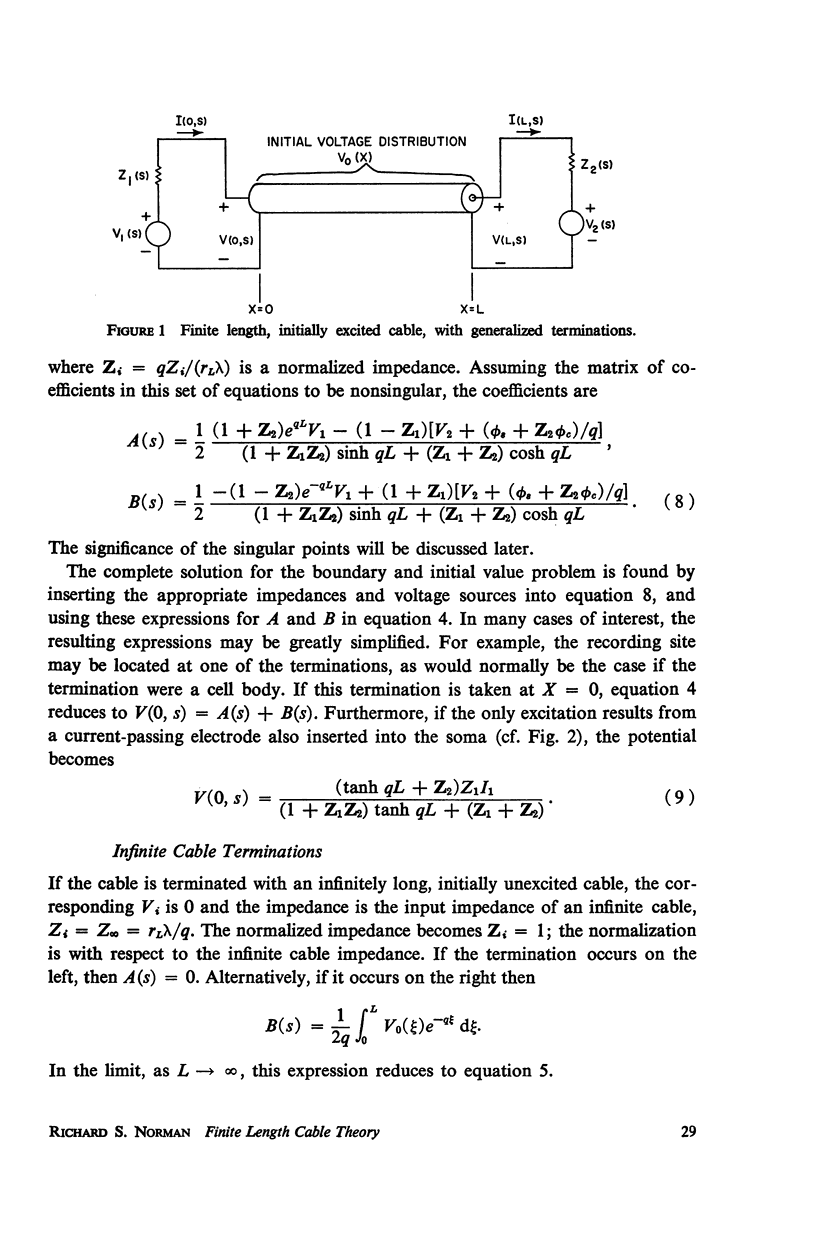
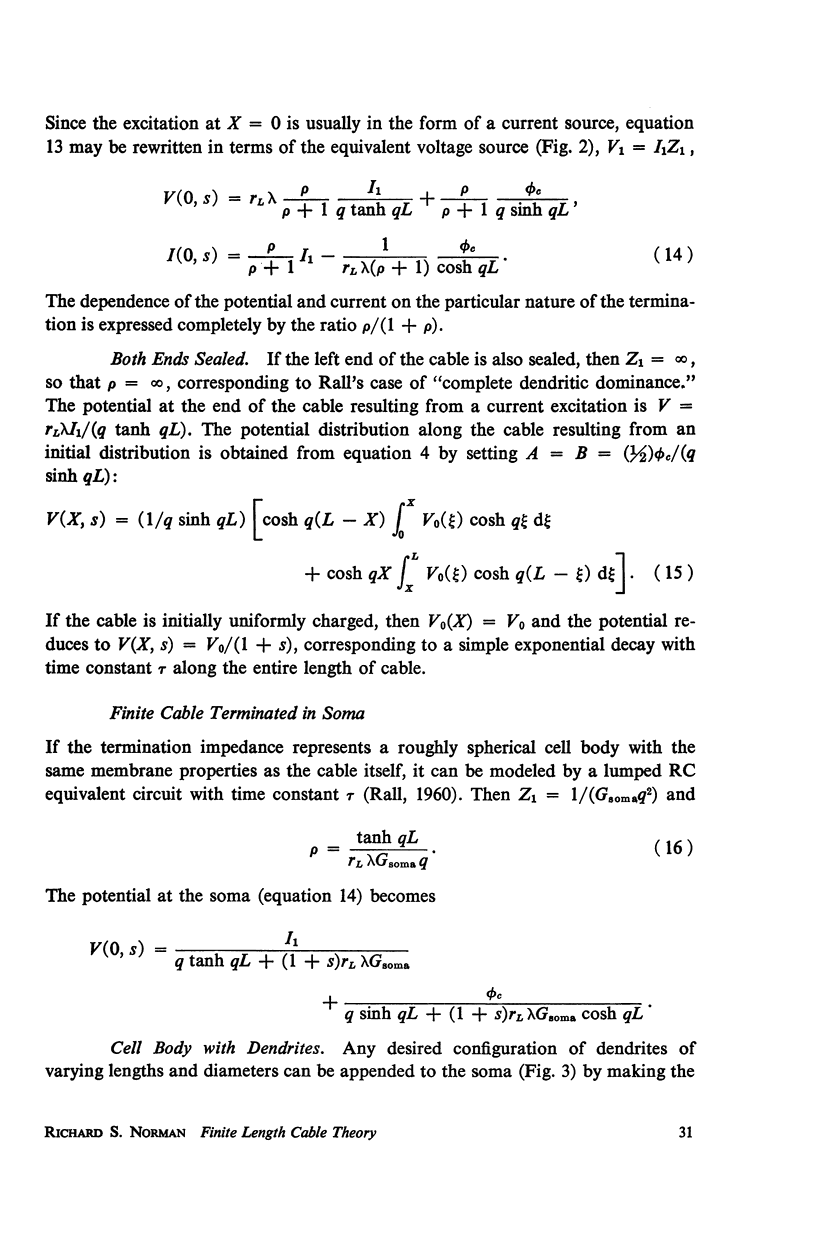
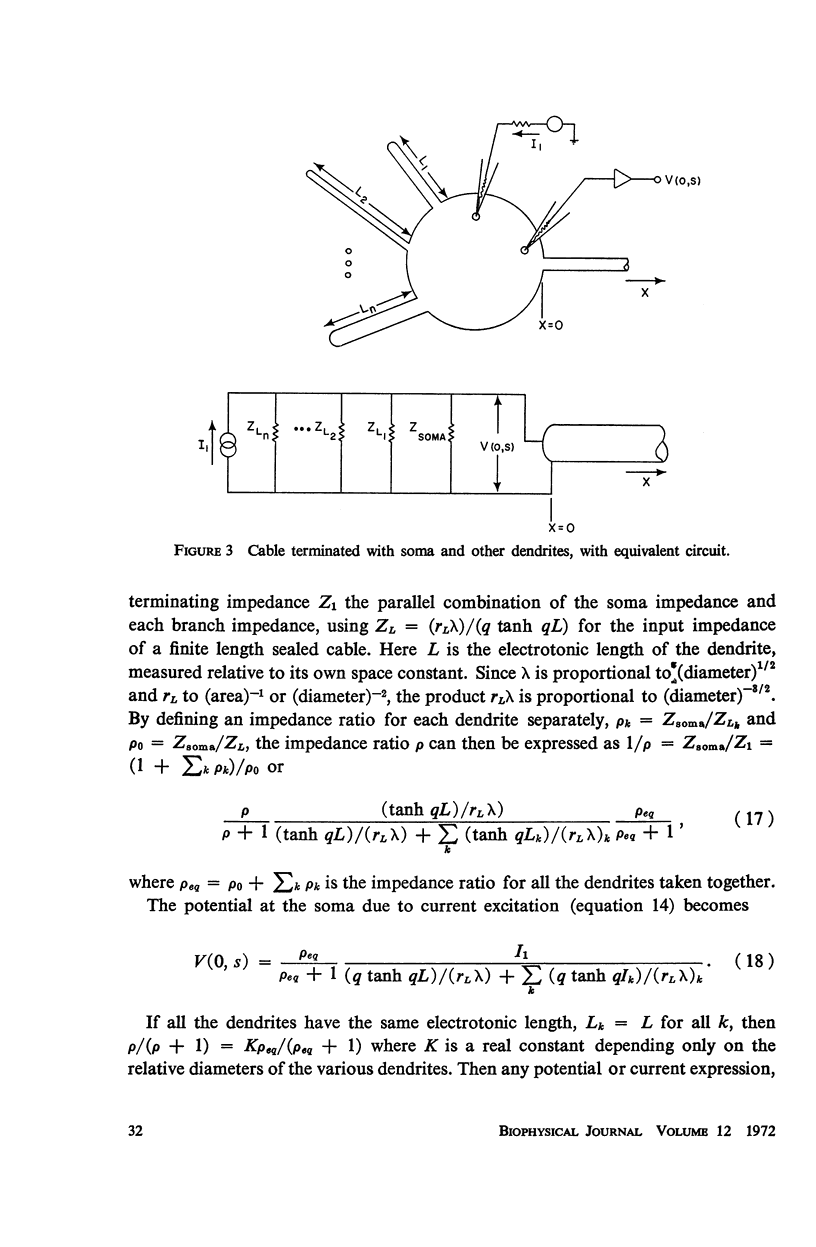
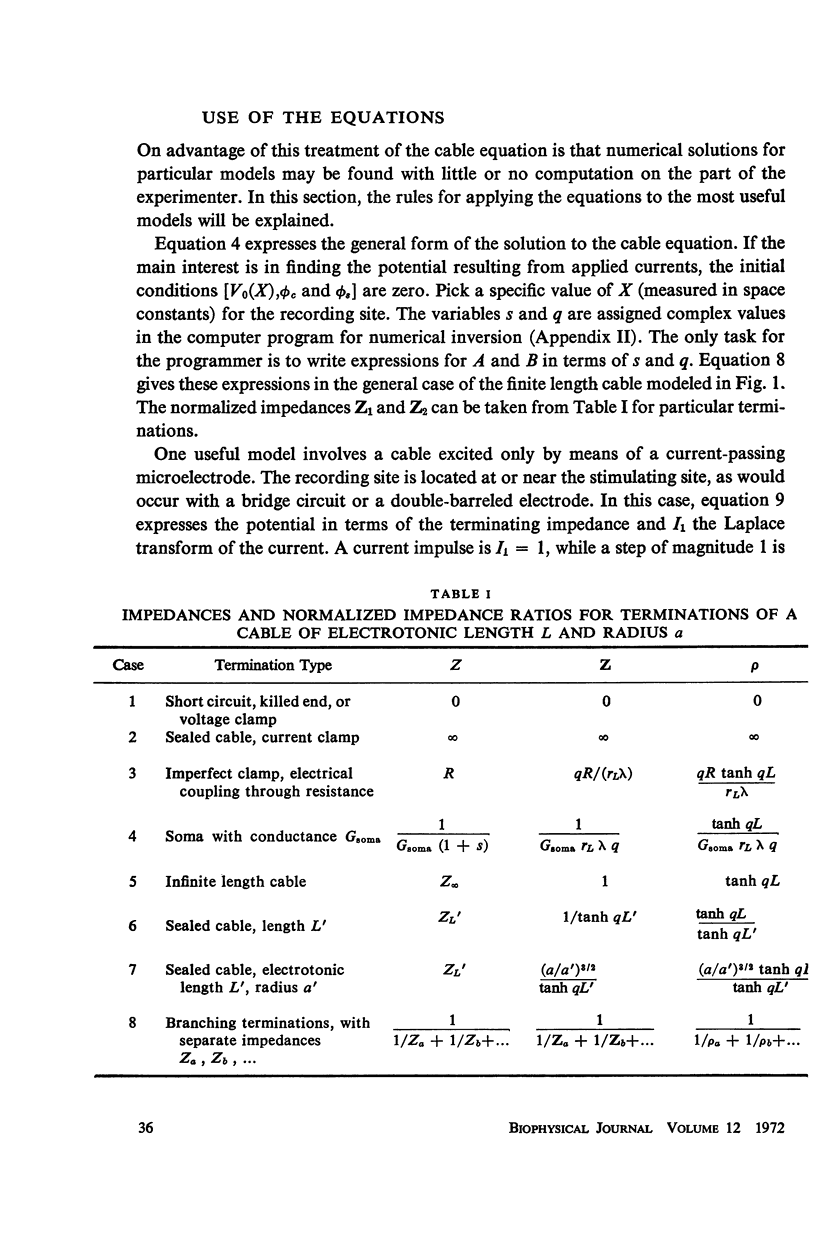
The cable equation is solved in the Laplace transform domain for arbitrary initial and boundary conditions. The cable potential is expressed directly in terms of the impedance of the terminations and the cable electrotonic length. A computer program is given to invert the transform. Numerical solutions may be obtained for any particular model by inserting expressions describing the terminations and parameter values into the program, without further computation by the modeler. For a finite length cable, sealed at one end, the solution is expressed in terms of the ratio of the termination impedance to the impedance of the finite length cable, a generalization of the steady-state conductance ratio. Analysis of a model of a soma with several primary dendrites shows that the dendrites may be lumped into one equivalent cylinder if they have the same electrotonic length, even though they may vary in diameter. Responses obtained under voltage clamp are conceptually predictable from measurements made under current clamp, and vice versa. The equalizing time constants of an infinite series expression of the solution are the negative reciprocals of the roots of the characteristic equation. Examination of computed solutions shows that solutions which differ theoretically may be indistinguishable experimentally.
Full text
PDF














Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Clark J., Plonsey R. A mathematical evaluation of the core conductor model. Biophys J. 1966 Jan;6(1):95–112. doi: 10.1016/S0006-3495(66)86642-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Nelson P. G., Lux H. D. Some electrical measurements of motoneuron parameters. Biophys J. 1970 Jan;10(1):55–73. doi: 10.1016/S0006-3495(70)86285-3. [DOI] [PMC free article] [PubMed] [Google Scholar]
- RALL W. Branching dendritic trees and motoneuron membrane resistivity. Exp Neurol. 1959 Nov;1:491–527. doi: 10.1016/0014-4886(59)90046-9. [DOI] [PubMed] [Google Scholar]
- RALL W. Membrane potential transients and membrane time constant of motoneurons. Exp Neurol. 1960 Oct;2:503–532. doi: 10.1016/0014-4886(60)90029-7. [DOI] [PubMed] [Google Scholar]
- RALL W. Theory of physiological properties of dendrites. Ann N Y Acad Sci. 1962 Mar 2;96:1071–1092. doi: 10.1111/j.1749-6632.1962.tb54120.x. [DOI] [PubMed] [Google Scholar]
- Rall W. Distributions of potential in cylindrical coordinates and time constants for a membrane cylinder. Biophys J. 1969 Dec;9(12):1509–1541. doi: 10.1016/S0006-3495(69)86468-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Volkov G. A., Platonova L. V. Elektricheskie svoistva kletok vodorosli Nitella flexilis. I. Kabel'naia teoriia v usloviiakh perekhodnogo i ustanovivshegosia rezhimov s uchetom konechnoi dliny kletki i soprotivleniia tortsov kletki. Biofizika. 1970 Jul-Aug;15(4):635–642. [PubMed] [Google Scholar]


