Exaggeration and suppression of iridescence: the evolution of two-dimensional butterfly structural colours

Abstract

Many butterfly species possess ‘structural’ colour, where colour is due to optical microstructures found in the wing scales. A number of such structures have been identified in butterfly scales, including three variations on a simple multi-layer structure. In this study, we optically characterize examples of all three types of multi-layer structure, as found in 10 species. The optical mechanism of the suppression and exaggeration of the angle-dependent optical properties (iridescence) of these structures is described. In addition, we consider the phylogeny of the butterflies, and are thus able to relate the optical properties of the structures to their evolutionary development. By applying two different types of analysis, the mechanism of adaptation is addressed. A simple parsimony analysis, in which all evolutionary changes are given an equal weighting, suggests convergent evolution of one structure. A Dollo parsimony analysis, in which the evolutionary ‘cost’ of losing a structure is less than that of gaining it, implies that ‘latent’ structures can be reused.

1. Introduction

Structural colour, which is produced by the interaction of light with transparent microstructures, has been identified in the scientific literature since 1665 (Hooke 1665; Newton 1730). It has been shown to occur both widely in current species as well as in the fossil record (Vukusic & Sambles 2003; Parker 2005). Rarely, however, does it exhibit the complexity and diversity found in butterflies (Lepidoptera), where the microstructures are found in the wing scales (Ghiradella 1989, 1984, 1985, 1991; Vukusic et al. 2000; figure 1a). In the past, these structures have often been approximated as thin-film reflectors or diffraction gratings. However, recent studies of the angle-dependence of their hue and intensity (iridescence) have revealed more complex optical behaviours due to the compound effects of interference, diffraction, absorption and scattering (Vukusic et al. 1999, 2002; Gralak et al. 2001; Kinoshita et al. 2002; Lawrence et al. 2002; Plattner 2004; Yoshioka & Kinoshita 2004).

Figure 1.

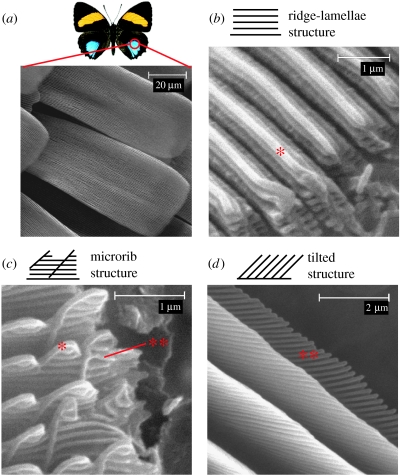
(a) Butterfly wing scales. A key feature of all scales is the ridges running along their length. Several modified types of ridge are found to produce structural colour, these include: (b) a structure in which overlapping flanges running along the ridge, ‘ridge-lamellae’ (Ghiradella 1989), are exaggerated; (c) one in which flanges running perpendicular to the ridge-lamellae, called ‘microribs’ (Ghiradella 1989), are exaggerated; and (d) a variant in which a tilted multi-layer is formed by the microribs, and the ridge-lamellae are absent. Asterisk, ridge-lamellae; double asterisk, microribs. (Image of Callicore aegina courtesy of The Insect Company (www.insectcompany.com).)

Different categories of structural colour in butterflies may be identified according to which of the basic scale structures are adapted to produce colour (Vukusic et al. 2000). One such group of structures are those in which a multi-layer structure is formed by the longitudinal ridges found on most butterfly scales (figure 1). Three variations of this structure have been identified (Vukusic et al. 2000). In the first type the multi-layer is formed by overlapping ‘ridge-lamellae’ that run along the length of each ridge (figure 1b). The second type consists of a multi-layer that is formed by ‘microribs’ that are perpendicular to the ridge-lamellae (figure 1c). The third subgroup is a form of tilted multi-layering, which may be formed by either the ridge-lamellae or the microribs (figure 1d).

Extensive previous work (Vukusic et al. 1999; Gralak et al. 2001; Kinoshita et al. 2002; Plattner 2004; Yoshioka & Kinoshita 2004) has focussed on the first type, and in particular the ridge-lamellae multi-layer found in the Morpho species. The angle-dependent absolute reflectivity and transmission of these wing scales indicates a much broader angle reflection than is typical of multi-layer systems (Vukusic et al. 1999). It is only possible to explain these spectral measurements by considering the finite extent of each ridge reflector (Gralak et al. 2001; Plattner 2004), irregularities in the structure (Kinoshita et al. 2002; Plattner 2004), and the presence of other highly diffractive wing scales (Vukusic et al. 1999; Kinoshita et al. 2002). The tilted multi-layer structures have also been studied in detail (Lawrence et al. 2002; Vukusic et al. 2002). The abrupt termination of the layers in this structure brings about diffraction and interference concurrently, producing reflection of broad wavelength range but restricted viewing angle. As yet no study has examined the microrib multi-layer structure in any detail, and this is done here for the first time. Also, no study has compared the properties of all three types, or quantified the diversity within each type. This study will address these questions by examining in detail the optical properties of 10 species of butterflies, of which only two (Morpho didius (Vukusic et al. 1999; Gralak et al. 2001; Kinoshita et al. 2002; Yoshioka & Kinoshita 2004) and Troides magellanus (Lawrence et al. 2002)) have been studied previously.

By considering the optical properties of a large number of structures and correlations with the known phylogeny of the species, some aspects of the evolution of the structures may also be inferred. An analogous study of the evolution of structural colour in some crustaceans found a direct relationship between the evolutionary development of species and the optical efficiency of their microstructures (Parker 1995; Parker et al. 1998). However, it should not be assumed that the evolution of structural colour in butterflies would necessarily follow this model, because the scale structures may not have evolved with light as their main stimulus. If this is not the case, a compromise may have been reached with other scale properties, such as thermodynamic, aerodynamic and mechanical properties, resulting in a structure that is not strictly optimal in an optical sense. Rather, it may be optimal in a biological sense. The evolution of structural colours found in birds and dragonflies has been shown to be quite different to that in crustaceans. In these examples, a number of species converge on the same solution (Prum & Torres 2003; Prum et al. 2004). However, in these studies only one type of optical structure is considered, a quasi-ordered array of spherical scatterers. In the case of butterflies, a much greater diversity of optical structures is observed, giving greater scope for an analysis of the relationships between different types of structure. By correlating the range and type of butterfly microstructures with their known phylogeny, we may begin to look for evidence of different mechanisms of adaptation. These may include: simple inheritance, convergent evolution, or a more complex pattern of evolution that may arise if a number of competing selection pressures are present. This will benefit our understanding of the mechanisms of adaptation that occur in this biological system.

Furthermore, this increased understanding of the structures will also benefit our ability to adapt them for technological applications. Indeed, several of the optical microstructures found in nature have already been adapted for use in novel biomimetic devices (Wilson & Huntley 1982; Stern et al. 1988), and it has been suggested that multi-layer structures have the potential for use in reflective display technologies (Micheron 2005). The ultimate benchmark in such systems is to produce a display that approaches the optical quality of more traditional ink-based printed media, with high contrast over a wide viewing angle (Granmar & Cho 2005). Thus, a clear understanding of the angle-dependent optical properties of multi-layer structures is key to their implementation in such technologies.

2. Material and methods

To quantify the diversity of butterfly structural colours, a large number of species were selected for detailed structural and optical characterization. These included Nymphalidae: Caligo martia, Callicore aegina, Diaethria neglecta, Eryphanis aesacus, Euploea midamus, M. didius, Perisama humboldtii, Paulogramma peristera, and Papilionidae: Trogonoptera brookiana and T. magellanus. Firstly, the wing scales of each species were examined using scanning electron microscopy (SEM). Small wing samples of each differently coloured area were mounted on aluminium stubs with conductive tape. All samples were then coated to a thickness of 20 nm with gold or platinum and viewed with a Phillips XL30 Scanning Electron Microscope at 15 kV, with the secondary electron detector. Once the type of structure was identified, the SEM images were used to make detailed measurements of the scale features.

The small size of butterfly wing-scales (approximately 200×75 μm), makes the complete characterization of their angular and spectral reflectance properties difficult. For this reason two experimental systems were chosen. Firstly, the diffuse reflection spectrum of an area of the wing is measured using a Cary 5E UV-Vis-IR spectrophotometer with a Labsphere integrating sphere. This system allows accurate absolute reflectivity measurements to be made from 200–1000 nm, over an area (approximately 10×5 mm) that covers many scales but is nevertheless small enough to isolate a single colour in most cases. The ‘speckled’ colouration of some species makes it difficult to isolate a single type of scale. In these species, sampling of the black ‘ground’ scales results in a relative rather than an absolute reflectance measurement. Samples were consistently mounted in the same orientation with respect to the incident beam. However, because there is some variation in the angle each scale makes with the wing membrane, a multi-scale measurement necessarily averages over a small range of incident angles. The integrating sphere in the system also collects light over the full range of reflected angles, so no information on the iridescence of the colour is obtained.

To complement this, angle-dependent spectral measurements were made using a Zeiss Micro-spectrophotometer. Essentially, this system contains a white light source of constant colour temperature (3200 K), a system of lenses and apertures to focus the light onto a small area of a single scale, a collection aperture to select a small region of the field of view, and a detector to measure the spectrum of this selected area. The sample is illuminated and the reflectance collected through the same optics. The numerical aperture of the system is set by the objective lens (NA=0.22). This value represents a compromise made between the requirement for high angular resolution and the requirement for a larger acceptance cone to ensure the relevant optical effect is detected. In order to minimize the detection of diffuse reflection from other areas of the wing scale the collection area was restricted to a square of dimensions 18×18 μm, and the spot size of the illumination was restricted to a radius of 39.4 μm. This was used in combination with a goniometer stage with a range of ±70° (±2.5°) to obtain angle-dependent spectral measurements along two perpendicular rotation axes. These axes correspond to the cross-sectional axis of the scale (X-tilt) and the longitudinal axis of the scale (L-tilt).

3. Results

From the structural analysis, four butterflies were observed to have the ridge-lamellae structure: C. aegina, M. didius, P. humboldtii and T. brookiana; two butterflies were found to have the microrib structure: C. martia and E. aesacus; and finally, four species were found to have the tilted multi-layer structure: D. neglecta, E. midamus, P. peristera and T. magellanus. The dimensions of each structure are given as insets to figures 2 and 3, SEM images of each species are given as electronic supplementary material.

Figure 2.

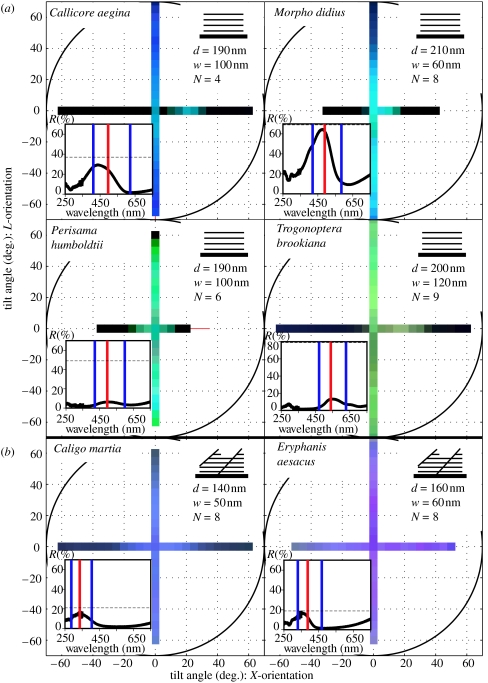
Angle-dependent spectral measurements of the (a), ridge-lamellae and (b), microrib butterflies. Structural characteristics from the SEM images: the periodicity (d), and width (w) of the layers and the average number of layers (N), are given in the insets on the top right of each set of results. The insets on the bottom left of each set of results show the integrated normal incidence spectrum from the Cary measurement. The predicted peak wavelength (red line), FWHM spectral bandwidth (blue lines) and peak reflectance (dotted line) from a simple thin film analysis with the refractive index of chitin (n=1.56) are also shown on the normal incidence plot. This predicted reflectance value is corrected by the percentage of the scale actually covered in the reflecting structure (the coverage, C). As this inset is a multi-scale measurement, the presence of scattered non-coloured scales in the sample area of P. humboldtii and T. brookiana results in peak reflectance measurements that are an underestimate of the true absolute value, therefore giving a disagreement with the predicted intensity.

Figure 3.

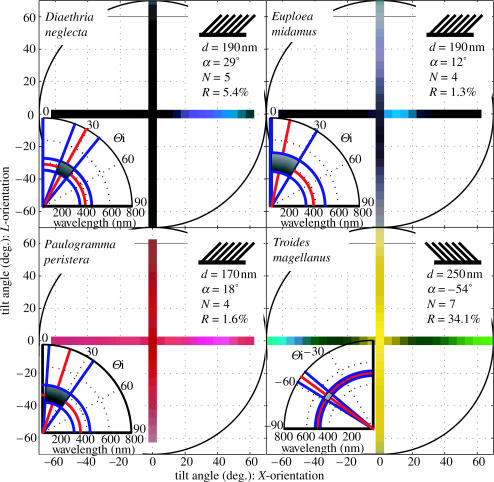
Angle-dependent spectral measurements of the four tilted multi-layer butterflies. The tristimulus values for each species are normalized to aid colour reproduction. The peak intensity of the reflection (R) and the periodicity (d), tilt angle (a) and number of layers (N) found in each structure are given in the insets on the top right of each set of results. The insets on the bottom left of each set of results show the theoretical prediction of the wavelength and X-tilt angle range of the strongest reflection as predicted by the bi-grating analysis.

A direct analysis of the angle-dependent spectral data for each of the 10 species revealed two patterns of angle dependence: either almost no change at all, or a distinct ‘flash’ effect where reflection is only strong at a particular angle. The precise relationship between the spectral data and the perceived colour of the scales will depend on the perceptual system of the viewer. Butterflies are known to have quite different colour sensitivities to our own (Brunton & Majerus 1995). However, the particular types of angle-dependence observed in the spectral data remain qualitatively independent of the viewer. Because this work focuses on angle-dependent effects rather than the specific colour perceived, an arbitrary choice of the human perceptual system may be made in order to visualize the spectral data. Thus, a convenient way to visualize the large amount of data collected is to use the CIE standard (IEC 61966-2-1 Official Specification), to convert each complete spectrum to a single RGB colour. The spectra of each butterfly are then plotted on an x–y plane, where the x-axis corresponds X-tilt, and the y-axis to L-tilt. Each measurement is represented by a coloured square, where the colour is generated from the entire visible spectrum at that point.

The angle-dependent spectra of the four ridge-lamellae butterflies (figure 2a) indicate very broad-angle reflectance in the direction of L-tilt, with very narrow-angle reflectance in the X-tilt direction. The angle-dependent spectra of the microrib species (figure 2b) show that their colouration is largely independent of angle. In contrast, the angle-dependent spectra for the four species with the tilted ridge-lamellae structure (figure 3) show strong reflectance only at a specific angle, corresponding to a bright flash of colour.

4. Discussion

4.1 Optical properties

Within the group of ridge-lamellae structures a diverse range of shorter wavelength colours is observed, while the angle-dependence remains consistent. That is, all species exhibit less iridescence than expected from a simple multi-layer along the L-tilt axis, but are still strongly iridescent along the X-tilt axis. These results agree with earlier work on M. didius (Vukusic et al. 1999; Gralak et al. 2001; Kinoshita et al. 2002; Yoshioka & Kinoshita 2004). In some measurements the spectra are slightly off-centre, and there is a discontinuity along the two axes at the point of zero tilt (e.g. C. aegina). However, from the SEM images the structure in this species is symmetric, and the zero point shift only occurs along one axis. Thus, the off-centre results in this species are more likely to be due to an error in the positioning of the scale. In contrast, both of the microrib structures are largely non-iridescent along both axes. The ridge-lamellae and microrib structures clearly differ in their iridescence along the X-tilt axis.

The application of a standard ‘thin-film’ multi-layer analysis (Born & Wolf 1999) to these non-tilted structures allows us to predict the peak reflectance, peak wavelength and full width at half maximum spectral bandwidth (FWHM) of the structure at normal incidence (insets figure 2). The integrated spectral measurements at normal incidence and these theoretical results agree, confirming the dominant mechanism of colour production in these structures is thin-film interference. In the case of C. martia and E. aesacus both ridge-lamellae and microribs are present. Given that the ridge-lamellae and the microribs have quite different periodicities, an independent one-dimensional analysis of each is an appropriate approximation. For both species the microrib analysis alone agrees well with the experimental results, and the periodicity (d=380 nm, 420 nm, respectively), number (N=1–2), and filling fraction (F=21, 12%, respectively) of the ridge-lamellae give only a small contribution to the reflection at optical frequencies. Thus, the ridge-lamellae play only a secondary role in the optical properties of these scales. Scattered regions of structurally coloured scales on T. brookiana and P. humboldtii lead to an experimental sample area that includes a significant number of black scales. This results in a measured reflectance that underestimates the true reflectance of the coloured scales, and explains the difference between the predicted and measured reflectance for the two species. Generally, this analysis demonstrates that the colours are produced by thin film interference. However, this simple model does not explain the angle-dependence of the spectra, or the differences between the ridge-lamellae and microrib structures.

In previous work, the broad-angle spectrum along the L-tilt axis of the Morpho butterflies is attributed to irregularities in the height of the adjacent ridges (Kinoshita et al. 2002), and in the exact geometry of each ridge reflector (Vukusic et al. 1999; Gralak et al. 2001; Kinoshita et al. 2002; Plattner 2004; Yoshioka & Kinoshita 2004). Re-examining the SEM image of the microrib structure (figure 1c), it is obvious that it incorporates more irregular elements than the ridge-lamellae structure (figure 1b). In particular, the height varies significantly along each ridge due to the presence of the nearly vertical ridge lamellae, and the height of adjacent ridges varies significantly. It is plausible then that the microrib butterflies achieve a similar spectral blurring to that seen in L-tilt spectra of the ridge-lamellae species. The difference is that in this case the degree of spatial correlation between reflecting elements is reduced along both axes of the scale.

The three butterfly species with the tilted multi-layer structure that have not been studied previously are D. neglecta, E. aesacus, and P. peristera. These species were all found to have a flash of colour in the blue–violet spectral region, at a similar wing orientation. The results for the butterfly that has been studied previously, T. magellanus, again agree with earlier work (Lawrence et al. 2002). However, because the multi-layer found in this species tilts in the opposite direction to that found in the previous three, the flash of colour occurs in the opposite hemisphere. In addition, the second green flash seen towards positive angles in T. magellanus corresponds to a second order scattering direction. This higher order peak is not detected in the other species because their intensity is much lower. The main degree of diversity seen in these structures is in the angle of the flash. There is also some difference in the overall effect due to the background colour of two of the species. The background yellow colour in T. magellanus is produced by a specialized fluorescent chemical chromophore known as papiliochrome (Lawrence et al. 2002). The fact that there are some scales on P. peristera that are only pink, with no structural blue flash, and others with only the blue flash and no background colour strongly indicates that this background colour is also due to the presence of pigments.

For these tilted structures the diffraction of light is a more significant effect in determining the reflected colour, so a more sophisticated model than the thin-film model is necessary. In this case the structures are analysed as bi-gratings (Lawrence et al. 2002; Vukusic et al. 2002). The geometry of a single ridge is approximated as a two-dimensional periodic lattice, which is finite in one dimension to represent the abrupt termination of the layers at the top of the ridge. Conservation of energy and crystal momentum within this lattice then allow us to predict the way light will interact with it, giving the range of reflected wavelengths as a function of angle (Kittel 1963). This analysis allows us to determine the angle at which the strongest reflection occurs (θ) and the peak wavelength (λ) at that angle. The finiteness of the lattice in the vertical direction is incorporated as an uncertainty in the periodicity of the corresponding reciprocal lattice. This uncertainty is defined as the FWHM of the scattering intensity function for a structure with N layers of periodicity d, as predicted by Fourier analysis. We may then calculate the wavelength as a function of angle for both the smallest and largest possible values. This leads to a prediction of the range of wavelengths reflected at the angle of strongest reflection (Δλ). To approximate the range of angles over which the curve is relatively flat, the difference of the angles at which the two curves representing the smallest and largest wavelengths reach a maximum is taken. This value then represents the range of angles over which the reflected wavelengths are approximately constant (Δθ). These values can then be visualized on a polar plot. The results for each species are given as the insets of figure 3. There is good agreement between these calculations and the experimental results, which confirms that both the colour and flash effect are a result of the tilted multi-layer.

4.2 Evolutionary relationships

Now that the physical and optical properties of these structures have been related using simple conceptual models, we can begin to consider any correlations with the phylogenetic distribution of the species as derived from the literature (figure 4). Rather than assuming progression from a simple solution to a more optically sophisticated one, we can then look for evidence to support such a trend, and identify other evolutionary trends. While sections of this tree have been verified by different morphological and molecular phylogenetic studies (Harvey 1991; Parsons 1996; Ackery et al. 1999; Morinaka et al. 1999; Brower 2000; Reed & Sperling 2001; Wahlberg et al. 2003; Freitas & Brown 2004; Hauser et al. 2005), no single study covers all the species. Thus, the tree represents a fairly conservative estimate of the phylogeny. Subsequent to sequence data becoming available for all the species involved, a more rigorous phylogenetic study will be necessary to determine the most probable tree and hence the most probable evolution of traits. However, it is possible that this would merely result in a more highly resolved tree, and hence an extension of these conclusions, rather than a complete reordering of the species.

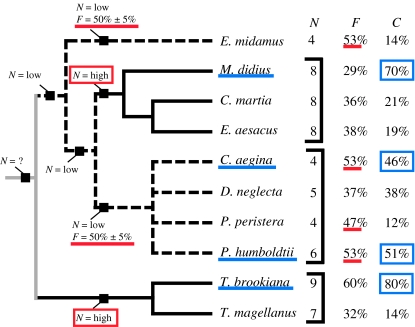
Figure 4.

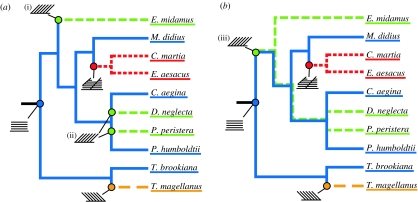
Phylogeny of the butterflies. Classification of Papilionidae (T. brookiana, T. magellanus) follows GloBIS (Hauser et al. 2005), Parsons (1996), Morinaka et al. (1999) and Reed & Sperling (2001), classification of Nymphalidae (C. aegina, M. didius, P. humboldtii, D. neglecta, E. midamus, P. peristera, C. martia, E. aesacus) follows Harvey (1991), Ackery et al. (1999), Brower (2000). Wahlberg et al. (2003) and Freitas & Brown (2004). Colour is used to indicate which type of multi-layer structure each species possesses (ridge-lamellae=blue, microrib=red, forward tilted multi-layer=green, backward tilted multi-layer=yellow). Ancestral structures are inferred using either (a) simple parsimony (Fitch 1971) or (b) Dollo parsimony (Farris 1977; Felsenstein 1989), and are shown in the same colours with a schematic of the type of structure used to indicate the node(s) at which is it predicted to have evolved. In both cases, the progressive evolution of more complex microstructures is observed.

Firstly, by considering the distribution of the three types of structure, it is possible to infer the ancestral structures present at different nodes in the tree. There are several methods used to infer such ancestral traits, the simplest being a parsimony analysis that minimizes the total number of state changes in the tree (Fitch 1971). The results of this analysis are given in figure 4a. The tilted multi-layer structures are considered as two groups, depending on whether the tilt is towards or away from the socket of the scale, where it joins the wing membrane. We consider it an overgeneralization to group these structures together because they have quite different visual effects, and hence presumably have different biological functions (Lawrence et al. 2002; Vukusic et al. 2002). As a result of this analysis, the ridge-lamellae structure is inferred to exist at the base of the tree, and may be considered a more ‘primitive’ structure. The microrib and tilted multi-layer structures occur much later in evolutionary history. A simple parsimony analysis of butterfly structural colours predicts multiple convergence events; as has been previously found in a phylogenetic analysis of the structural colour in dragonflies (Prum et al. 2004). That is, the forward tilted multi-layer structure is inferred to have developed independently at least twice in the evolution of the species (indicated at nodes (i) and (ii) in figure 4a).

However, this approach does not account for the fact that the structural colours found in butterflies are very complex. In such cases it is considered that the evolutionary ‘cost’ involved in losing a complex structure is much less than in gaining it in the first place, and an application of Dollo parsimony may be more appropriate (Farris 1977). Dollo parsimony allows up to one forward change (0→1), and as many reversions (1→0) as necessary, and minimizes the number of such reversions (Felsenstein 1989). The results of this analysis are shown in figure 4b. The high degree of phenotypic plasticity observed in butterfly structural colours (Ghiradella 1984, 1985, 1989, 1991) suggests this model may be more useful in analysing their evolution. Indeed, several species are known to possess two different types of structural colour in adjacent wing areas, including complex three-dimensional photonic crystals (Ghiradella 1989). The major difference between the results of the simple and Dollo parsimony analysis is the point at which the forward tilted multi-layer structure is predicted to have first evolved. Using Dollo parsimony, it is predicted to have evolved much earlier (node (iii) in figure 4b), and there is a group of species within which both the tilted multi-layer structure and the ridge-lamellae structure are inferred to exist. It is possible then that these ancestral species had the potential to express either one, or both, of the structures. The structure which is not expressed is hypothesized to remain as a ‘latent’ structure, which can be switched on should the need arise (H. Ghiradella 1989, personal communication, July 2004).

While it is not possible to clearly define which evolutionary model is more applicable to this case from the information currently available, it is still possible to draw conclusions from the areas in which the two analyses overlap. In both cases the ridge-lamellae structure occurs at the base of the tree, suggesting that this type of multi-layer structure is the primitive form. Both types of tilted multi-layer structure occur more recently than the ridge-lamellae structure, and thus may represent an adaptation of the primitive structure to produce a more angle-dependent colour. The large evolutionary separation of the two groups of tilted multi-layer structures suggests that they may have independently arrived at similar solutions for exaggerating multi-layer angle-dependence. Similarly, the more complex microrib structure, with optical properties that depend on the interaction between the regularly arranged microribs and irregularly spaced vertical ridge-lamellae, is found to occur much later in evolutionary history. Thus, it also represents an adaptation of the primitive ridge-lamellae structure. Furthermore, in the Dollo parsimony analysis (figure 4b), the microrib structure is inferred to have arisen after the forward tilted multi-layer structure, and in a branch of the tree in which it is thought that the tilted structure remains latent. Thus, it is also possible that this structure is a further adaptation of the tilted multi-layer structure. The morphology seen in the micrographs (figure 1c,d) would seem to support such a hypothesis. In either case, as we move across the tree from left to right, moving forward in evolutionary time, we observe the progressive evolution of more complex microstructures. That is, microstructures which move further away from the inherent iridescence of a simple multi-layer structure towards a solution which may be thought of as more ‘biologically optimal’.

As distinct from the biological fitness, the optical efficiency of these structures may be unambiguously calculated from simple physical models. Thus, to compare the optical efficiency of these structures we examine the structural features affecting the intensity of the reflection. Any correlations between these features and the phylogeny may then indicate the degree to which the structures are optimized to produce a strong reflection. The features considered are: the number of layers in the multi-layer (N), the ratio of the periodicity to the width of the reflecting elements or ‘filling fraction’ (F), and the percentage of each scale actually covered in the reflecting structure, referred to as the coverage (C). In this case, the simple parsimony method is used as the three traits above (N, F, C) are not as complex as the structures themselves, and there is no evidence to suggest that the likelihood of a change in one direction is greater than the reverse. The results of this analysis are given in figure 5.

Figure 5.

Phylogeny of the butterflies showing structural features relating to the intensity of the reflected colour, such as the number of layers (N), the filling fraction (F), and the percentage of the wing scale covered in reflecting elements (C). Some of these traits, such as N and F, follow the phylogenetic distribution of the species, and the ancestral traits predicted by a simple parsimony analysis (Fitch 1971) are shown. A black line indicates N=high, a broken line indicates N=low, and a grey line indicates the number of layers is ambiguous. The coverage is more closely correlated with the occurrence of the ridge-lamellae structure, underlined in blue.

Firstly, the number of layers (N) correlates strongly with the subfamily classification of the butterflies. That is, of the species examined, the Papilioninae and Morphinae were observed to have a high number of layers, and the Danainae and Biblidinae a low number of layers. If the ancestry of this feature is traced back through the tree using Fitch's algorithm (Fitch 1971), the most likely value at each node can be inferred. These points are indicated by the black squares on the tree. Thus the predicted primitive state for the Nymphalidae is a low number of layers (4–6), and that of the Papilionidae is a high number of layers (7–9). The number of layers found in a common ancestor of these two branches of the tree remains ambiguous, but it is clear that a higher number of layers, which corresponds to a more intense reflection, has independently evolved at two points on the tree (highlighted in red).

A similar pattern occurs in the distribution of the filling fraction (F). The optimal value for the filling fraction, in terms of producing the strongest reflection, is known to be 50% (Land 1972). The values within 5% of this optimal value occur predominantly in one branch of the tree, and in this case the primitive state for the whole tree is unambiguously a less optimal filling fraction. A more optimal value is then seen to have evolved independently at least twice (underlined in red). Interestingly, in both cases this occurs at a node corresponding to a low number of layers. It is possible then that these species were under the same pressure to evolve a higher intensity reflection as those that evolved a higher number of layers, but adapted differently in response to this pressure.

In contrast, the coverage (C) does not correlate directly with any taxonomic groups. However, there is a strong correlation between the highest coverage values and the occurrence of the primitive ridge-lamellae structure. The high coverage values found in these species suggest additional intensity based selection pressures may be placed on this structure. It also suggests that while this type of structure can be considered as primitive in terms of its iridescence, it has evolved to optimize other traits, such as the intensity of reflection.

5. Conclusions

By using structural colours rather than pigments, butterflies are able to adapt the hue, intensity and angle-dependence of a colour. In the ridge-lamellae and microrib structures the angle-dependence of the colour is suppressed, and in the tilted multi-layer structure it is exaggerated. The further adaptation of scale features relating to the intensity of the reflection is observed in the ridge-lamellae structure. Thus, although it can be considered more primitive in terms of angular effects, the ridge-lamellae structure has adapted towards more efficient reflection. Other groups have previously speculated that the suppressed iridescence of the ridge-lamellae structure is useful for ‘in-flight’ signalling (Vukusic et al. 1999), and the flash effect of the tilted structure for ‘at-rest’ signalling (Vukusic et al. 2002). If this were the case, then the biological function of the ridge-lamellae structure would be strongly linked to the strength of its reflection. The increased efficiency of this structure supports the hypothesized function. In addition to this, the fact that the microrib and tilted multi-layer species are not as optically efficient suggests that ‘optical efficiency’ may not always correspond to ‘biological fitness’. In these species a compromise might have been made between increased optical efficiency, and some other trait, such as mechanical or aerodynamic properties, highlighting the multi-objective nature of evolution. However, in all these structures, the selection pressures driving their evolution remain speculative, and an ecological study is required to fully resolve the biological function of each structure and the corresponding selection pressures.

While these optical trends are interesting, in some ways the evolutionary trends tell the more compelling story, because the phylogenetic distribution of the structures indicates that they may be evolving by more complex mechanisms than simple inheritance. When we applied simple and Dollo parsimony analyses, the former indicated convergent evolution of the tilted multi-layer microstructure, and the latter indicated that certain structures remain latent. At this stage it is an open question as to which proposition is correct, though the diversity of structures both within species groups, and indeed on a single butterfly wing, is suggestive that Dollo parsimony is more appropriate. We believe this question merits further work, encompassing a larger range of species and structures, and this is one of the ways in which we intend to expand the current study. In the longer term, we aim to resolve the mechanism of evolutionary adaptation in butterfly scales, and in particular, to understand the possible role of latent structures.

This idea of ‘latency’ in biological structures is potentially a very powerful one, because it allows previous structures to be rapidly redeployed and reused, perhaps modified, for a slightly different function, or in combination with other structures. The optical properties for example may not be the only ones experiencing selection pressures. The developmental cost of the structure, its thermal or mechanical properties, may also be important. The reuse of previous structures clearly allows functional change to be achieved much more quickly than is likely if the structure had evolved from scratch. It also highlights however, the essential conservatism of adaptation, in which previous solutions are intrinsically more likely than genuinely new, and perhaps more effective, ones.

Perhaps less obviously, the role of legacy structures and designs is also a characteristic of technological change, where issues of backward compatibility and use of existing infrastructure act as a brake on truly radical change. Thus, the study of biological adaptation as an approach to multi-objective optimization not only benefits our understanding of the biological systems involved, but it may also provide genuine technological insights.