Abstract
In the articles in this Discussion, a wide variety of topics are treated, including reorganization energy, initially introduced for electron transfers (‘environmentally assisted tunnelling’), nuclear tunnelling, H/D and kinetic isotope effects (KIEs), the effect of changes of distal and nearby amino acid residues using site-directed mutagenesis, and dynamics versus statistical effects. A coordinate-free form of semi-classical theory is used to examine topics on data such as tunnelling versus ‘over-the-barrier’ paths and temperature and pressure effects on KIEs. The multidimensional semi-classical theory includes classically allowed and classically forbidden transitions. More generally, we address the question of relating kinetic to thermodynamic factors, as in the electron transfer field, so learning about specific versus thermodynamic effects in enzyme catalysis and KIEs.
Keywords: enzyme catalysis, isotope effects, semi-classical theory, hydrogen tunnelling, enzyme kinetics, pressure effect
1. Introduction
The papers presented in this symposium offer a broad cross-section of research in catalytic H+, H• and H− transfer in enzymes. If a modern Rip van Winkle had gone into a scientific sleep some 20–25 years ago, awakened and attended this symposium, he would have been impressed and perhaps bewildered with the wide array of developments that had occurred during his long nap, though some of the questions would have had a familiar ring. It has been some 20–25 years since I have studied H-transfers and I can appreciate his apprehension in offering a summary.
In listening to the papers in this Discussion, Rip would have recognized that they are insightful and provide broad reviews and new studies of the exciting and multi-faceted research in the enzymatic field. They include experimental studies, applications of theories and fundamental theories. A common theme draws upon concepts used for electron transfer reactions, taking similarities and differences into account. Transition state theory (TST) and nuclear tunnelling play a major role in interpretation in many of the articles, and the question of dynamical versus statistical behaviour arises a number of times. In enzymes, the effect of mutants studied by site-directed mutagenesis of remote and nearby amino acid residues demonstrates the large effect that remote residues can have on the catalysis.
We are also reminded in several articles that for a multi-step reaction the H-transfer step need not be the rate limiting one, and so may cause an H/D kinetic isotope effect (KIE) on the rate to be closer to unity than in a single-step mechanism. A kinetic analysis is then needed to extract rate constants and the KIE for the H-transfer step.
In many papers in the Discussion, it is pointed out that environmental effects, in the form of the ‘reorganization energy’ concept initially introduced for electron transfers, influence the rate, as do factors controlling the distance of closest approach of the heavy atoms participating immediately in the H-transfer (sometimes called gating), the extent of nuclear tunnelling of the H and the effect of nearby and distal residues on the rate. The reorganization serves to bring the H-energy levels immediately before and after the H-transfer rate into resonance (same total energy). Any ‘tunnelling’ of H is, as noted by many of the authors, a two- or multidimensional problem rather than simply the old one-dimensional one.
The calculation of the reorganization now involves a detailed knowledge of the structure of the enzyme and a combination of quantum mechanical and molecular mechanics (QM/MM) computations to study the configurational changes in the protein that permit the substrate and the cofactor to form a transition state (TS).
We explore these features of the Discussion, and to that end first introduce in §2, a coordinate-free semi-classical view of nuclear tunnelling in multidimensional systems. Themes appearing in the Discussion are described in §3, some experiences drawn from electron transfers are considered in §4 and concluding remarks are given in §5.
2. Background and model
We first recall, before commenting in more detail on the papers, several studies that appeared before Rip's sleep (Marcus 1980; Klippenstein et al. 1986 and references therein). In the process, we ask what physical insight can basic semi-classical theory provide on the nature of the QM description of the H-transfer and on different procedures used for treating tunnelling? We use semi-classical in the strict sense of the term (Marcus 1970; Miller 1970) that includes both classically allowed and classically forbidden (tunnelling) transitions. We use, for simplicity, an approximate analysis based on a more rigorous expression given for H-transfer by Babamov & Marcus (1981).
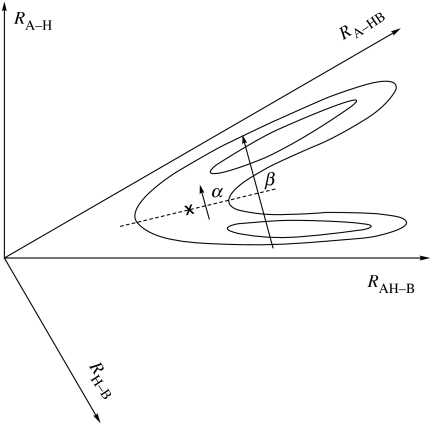
We consider a reaction AH+B→A+BH, where A and B are heavy atoms. However, in the context of this Discussion, each now denotes a heavy atom, such as O, N or C, bonded to other atoms in the protein, and H denotes an H+, H• or H−. For each pair of A–H and H–B distances, there is now also a huge number of remaining coordinates of the entire system—the protein and its surroundings. A projection of the multidimensional potential energy surface diagram of the system is shown schematically in figure 1, a projection onto the two-dimensional subspace spanned by the two distances, AH and HB. Prior to the H-transfer there is, in the present case, a ‘reorganization’ of all the other coordinates so as to facilitate the transfer, i.e. to make the total energy immediately before and after the H-transfer equal. This reorganization, which parallels that occurring in electron transfer reactions, is referred to in many of the talks in this symposium, and is depicted, e.g. in the articles of Sutcliffe and Klinman (Albery 1982). In referring below to individual papers of this Discussion, the name of the presenter is given rather than of the first author, and the year is omitted.
Figure 1.
Schematic potential energy surface for the reaction AH+B→A+HB, using mass-weighted coordinates. X denotes the saddle-point and the α and β paths are indicated.
In an H-transfer between two heavy atoms (figure 1), there is a continuum of reaction paths for proceeding from the well in the potential energy surface for the reactants (AH, B) to the well for the products (A, HB), paths such as α and β there. This figure is adapted from one that appeared earlier (Marcus 1979). The coordinates in the diagram are the usual mass-weighted coordinates in reaction rate theory (Glasstone et al. 1941). The low mass of the H is reflected in the rather acute nature of the angle in figure 1. Here, we see that the paths going from the well of the reactants, AH+B, to the well of the products, A+HB, range from those passing near the saddle-point X (paths α) to those occurring at longer AB distances (paths β). The β paths typically involve tunnelling of the H, and there is a gradation between α- and β-type paths.
This type of diagram was used (Marcus 1979) to explore a difference in the electrochemical literature some 30 years ago for H+-transfer from H3O+ to a metal electrode. The difference was between one view based on conventional (saddle-point) TST, in which most classical trajectories or QM wave packets proceed from the reactants' well to the products' well via or near the saddle-point, i.e. near the ‘minimum energy path’ (MEP; e.g. review by Appleby et al. 1973), and another view which introduced the analogy between H-transfer and electron transfer processes (e.g. Dogonadze et al. 1968). Roughly speaking, the two views correspond to the α- and β-type paths, respectively—only roughly since there is a reorganization of the remaining coordinates even for α paths.
In the case of an α-type path the deuterium/tritium KIE is attributed primarily to the difference of zero-point energies in the reactants and the TS; an A–H stretching vibration has disappeared in forming the TS AHB†, and bending vibrations have been modified. In this case, the maximum KIE without tunnelling is perhaps a factor of about 7 (Klinman) or 6 (Sutcliffe) at room temperature. The KIE is a maximum at (e.g. Bell 1973, 1980) and so the maximum KIE for an α-type path may be less than 7, depending on the value of . In a β-type path, unless β is close to α, the light particle (H) typically tunnels from the reactants' well to the products' well, and the KIE can considerably exceed 7.
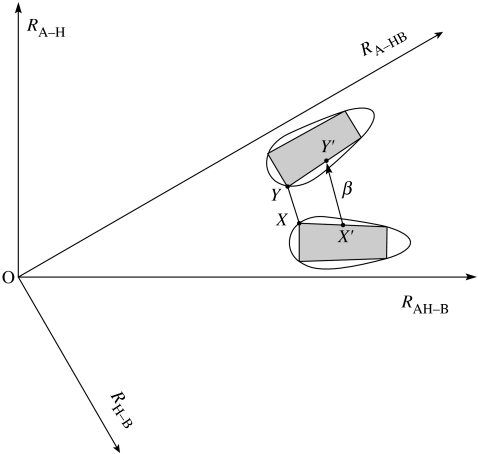
Instead, if the AH vibration were sufficiently excited or if the barrier is sufficiently low in a β region, as in some highly exothermic H-transfer reactions, the system can travel by a β path without a nuclear tunnelling of the H, e.g. at an energy greater than or equal to the energy where the points X and Y in figure 2 (see later) coincide and at energies above that value.
Figure 2.
Diagram showing the space swept out by a classical mechanical trajectory in the reactants' well and by one in the products' well and showing tunnelling on a β path from point X′ on the boundary of the reactants' distorted rectangle to point Y′ on the boundary of the products' distorted rectangle, including the nearest points X′=X and Y′=Y at the corners of the distorted rectangles.
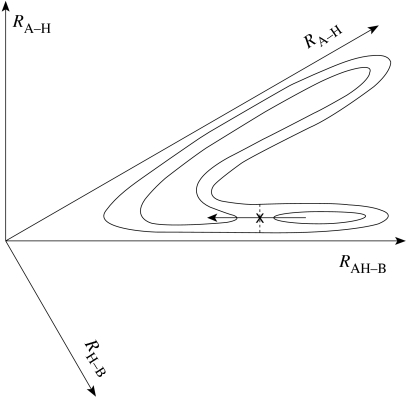
When there is a symmetry in the two wells, and there are only two coordinates, an accurate expression was obtained for the reaction probability (Babamov & Marcus 1981) and tested by comparing with accurate QM computations for the reaction probability as a function of energy. For the purpose of present Discussion, which is intended to focus on physical concepts, we simplify the analysis, using an approximate semi-classical form. We consider for simplicity the symmetric case and so introduce polar coordinates (R, θ) in figures 1 and 2 with the origin at O. The latter are the two-dimensional version of hyperspherical coordinates commonly used in accurate computations of rates for small systems (e.g. Kuppermann & Hipes 1986). For any given total energy E and H-vibration quantum state, the corresponding classical trajectory for a bound quantum state fills the distorted rectangle in figure 2 and has fixed classical action variables and for the H-motion and R-motion, respectively. The amplitude of the side of the ‘rectangle’ nearer to the origin is larger than that of the opposite side, because of the decreasing H-vibration frequency at fixed as the system moves closer to the TS region (decrease in R). In the semi-classical theory of bound states of Noid et al. (1981), the entire description can be made coordinate independent, but we have used R and θ for simplicity and concreteness. The action variables are the classical counterparts of the corresponding quantum numbers, with when is a vibration. No separation of variables is assumed in semi-classical theory, the classical invariants are quantized and more than two coordinates can be treated (Noid et al. 1980). In the more general asymmetric system, figures 1 and 2 become asymmetric as in figure 3 (e.g. Fernandez-Ramos et al. 2002).
Figure 3.
Diagram illustrating the scenario where the saddle-point X is in the reactants' well.
For the given H-quantum state and the given energy E, the wave function decays exponentially outside these distorted rectangles. In wave mechanical terms, the boundary of the distorted rectangle forms the ‘caustic’. The system tunnels in figure 2 from any point X or on the side of the distorted rectangle nearest to the products' well to a corresponding point on Y or on the nearest side of the distorted rectangle in the products' well. Excited H-vibrational states of the reactant and product can also be incorporated (Klippenstein et al. 1986). The tunnelling analysis is made coordinate independent by choosing the path from X′ to Y′ variationally, instead of along an arc of θ or along a chord. (There are subtleties here in choosing the Y′ to correspond to X′ and in selecting the path that we will discuss elsewhere.) Or, one can treat the problem quantum mechanically with many diabatic states to treat this highly asymmetrical system. A variational method for an approximate form (‘small curvature approximation’) was given by Marcus & Coltrin (1977) and further extended by Liu et al. (1993). A large curvature approximation was given by Ovchinnikova (1979) and Babamov & Marcus (1981) and developed further (e.g. Fernandez-Ramos & Truhlar 2001, 2005 and references therein). A recent review of H+-transfer has been published (Jaczewski & Hubbard 2003).
If P(E) is the probability of an H-transfer for any given initial H-quantum state and total energy E, then the unimolecular rate constant krate for this bound AH–B system is obtained by integration over all E (Appendix A),
| (2.1) |
where Q is the product of the partition functions for the R-vibration (QR) and for the H-motion () in the reactants' well. If we write QR approximately as a classical harmonic value, , and as when the H is in its lowest quantum state in the reactants, being the zero-point energy of the H-motion, then we have,
| (2.2) |
For our present purpose, aimed at discussing the physical aspects, can be written approximately as an integral over the time spent in multiplied by , the frequency of the H-motion at that R and, in the tunnelling region, by the probability of tunnelling at that R, , where is the phase integral for crossing from the reactants' to the products' well at any given R, namely from any to in figure 2. (In the classically forbidden region, is imaginary and its absolute value appears.) The time spent in is , where is the R-velocity at R for the given E and . For small tunnelling probabilities, the tunnelling can occur in both the forward and backward direction, and so a factor of 2 is then introduced into equation (2.3) below. The R corresponding to X is denoted by RX. We thus have, treating as ∞ because of the smallness of at large R,
| (2.3) |
| (2.4) |
Although vanishes at the R-turning point at in equation (2.3) and figure 2, the integral remains finite since varies as . When the integrand in equation (2.3) decreases exponentially from its value at , i.e. at and , as used tacitly in the so-called Marcus & Coltrin (1977) path, the integration is immediately performed (Babamov & Marcus 1981). A more rigorous expression is also tested and used in the latter article.
For any given , the higher the E, the more easily the system can reach smaller Rs, an effect that contributes to the activation energy. The tunnelling barrier for the H-motion at any R becomes smaller as R decreases and ultimately disappears when the two distorted rectangles in figure 2 touch at their nearest corners, typically before reaching the saddle-point. When for the given E and action variable the corners touch, and for higher Es they ‘overlap’, we have . A more accurate expression is given in Babamov & Marcus (1981). Other subtleties are commented on in Appendix A.
In addition to the integrand in equation (2.3), there is also, for a triatomic collinear system, an off-resonant factor, given in Klippenstein et al. (1986). This factor introduced into the rate a factor of approximately 0.6 in the systems studied. These off-resonance energies Δ are not very large, since protonic states in each well at any R are relatively closely spaced compared with the several electron volt spacing of electronic states. They are less significant when there are many other coordinates, as in a protein, since the coordinates can ‘reorganize’ so as to make Δ very small. Taking the reorganization effect into account by introducing the reorganization factor, taken from electron transfer theory as some papers in this symposium have done, one obtains from equation (2.2)
| (2.5) |
where
| (2.6) |
| (2.7) |
where is the standard free energy of reaction for the H-transfer step (hereinafter referred to as the internal ); λ(R) is the reorganization energy; contains the ‘gating’ term for the reactants plus any change (e.g. conformational change) in the protein–substrate complex that cannot be overcome by a favourable ; is the corresponding term for the products. The gating term in is the energy to bring reactants from their lowest energy level in the H-quantum state to the distance of closest approach. In equation (2.5), we have neglected the off-resonant factor, whose effect is reduced by the reorganization.
When the distorted rectangles touch or begin to overlap, is replaced approximately by for that E and distance of closest approach RX. When more than one H-state of the reactants and/or products contribute, one can sum over those states. As in electron transfers, one can also modify the in equation (2.7) by incorporating some of the quantized vibrations contributing to the reorganization that have high vibration frequencies.
In principle, for a sufficiently exothermic process, the saddle-point in the projected potential energy surface can also occur in or nearer the entrance well, as in figure 3 (‘early transition state’) and similarly for a sufficiently endothermic process, it can occur in the products' well (‘late transition state’). In each case, the motion across the saddle-point TS is now primarily that of an A–B motion rather than of an H, and so the should now be smaller for a ground state to ground state transition . This effect is known for reactions in solution (e.g. Bell 1973). For a sufficiently exothermic reaction, there can also be a and higher transitions, so making the actual H-transfer more thermoneutral. For some systems (Burton), the calculated kH/kD is less than unity, which the authors note appears to be unphysical and is attributed to the breakdown of the model for the H-transfer (small reaction path curvature model). As also indicated in several papers in the symposium, the ratio can also be closer to unity when the H-transfer is not the rate-determining step.
One reaction coordinate for the entire system is the energy difference of the energies of two electronic valence bond states, introduced initially for electron transfer (Marcus 1960; King & Warshel 1990) and is used as a reaction coordinate for H-transfers here (e.g. Hammes-Schiffer, Warshel). In Warshel's analysis, the non-separability is treated using a one-dimensional QM treatment for H and a classical treatment for the remaining coordinates. A different reaction coordinate, the difference of AH and HB bond distances, is used by Sutcliffe and has an extensive history in the literature (e.g. Fernandez-Ramos et al. 2002 and references therein).
The asymmetry in figure 3 is a limiting case. It illustrates a situation where of the two diabatic states (AH, B and A, HB) would not necessarily be the best reaction coordinate; the states just before and after the saddle-point in figure 3 are both dominantly in a largely (AH, B) electronic configuration rather than in the (AH, B) and (A, HB) ones. The reaction coordinate more closely related to the MEP, e.g. a difference of the AH and HB bond lengths or a difference of their ‘bond orders’, avoids this particular problem. Use of the reaction coordinate will result in some recrossings of the dividing surface (surface for which ), which can nevertheless be included and corrected for in the molecular dynamics calculations. In calculations (Hammes-Schiffer, Warshel) using as a reaction coordinate, the recrossings have not been extensive, and so is a useful reaction coordinate.
We have not considered time-scales for the H and other motions. An example of a use of classical dynamics in the TS region is seen in Nam et al. (2004).
In virtue of a Discussion in this symposium, it is useful to recall the basic assumptions of classical mechanical TST. In a remarkably insightful article, Wigner (1938) pointed out that a key assumption in classical TST is that there is a hypersurface in the full phase space across which there are no recrossings of classical mechanical trajectories. In some simple double well problems, there could be many recrossings of the H-motion, though they are much less likely in the tunnelling regime. Hammes-Schiffer finds in her study that recrossings were minor.
No ‘separation of variables’ is needed and none was assumed by Wigner in the classical mechanical form of TST. However, because of the extension of the relevant wave function of the TS outside the hypersurface (uncertainty principle) in the QM or semi-classical description, one needs to treat the dynamics of several coupled non-separable coordinates, as for example, in equations (2.3) and (2.4). Fortunately, as in these equations or in the Hammes-Schiffer article or in other articles in the Discussion, one can modify the TST so as to include this non-separable aspect. The non-separability issue is noted by Schwartz.
The extent of nuclear tunnelling in a reaction has actually been quantified for simple systems; a QM method using hyperspherical coordinates yields the reactive flux lines and the fraction of the flux that passes through the classically forbidden region using the method of Kuppermann, Adams and Truhlar (Kuppermann 1981). It has not been adapted to enzymatic reactions, to my knowledge, and the possibility of combining it with calculations has not been explored. Nevertheless, it provides a precise definition of the fraction of the reactive flux that proceeds via tunnelling.
Even a cursory study of the papers in this Discussion and in the present literature reveals how much has developed during the past 20–25 years. Detailed structural information and extensive (QM/MM) calculations are used for atoms near the reaction site and for the remaining atoms, tunnelling methods have been extended and combined with MM, and changes in electrostatic effects, in networks of H-bonds and in conformations have been invoked. With this background in mind, we turn to the present papers.
3. Themes in the discussion
Certain themes are common in the Discussion. For example, a reorganization of the environment (protein and immediate vicinity of the reaction site) serves to bring the H-energy level of the reactant and that of the product into an approximate equality, as in the electron transfer case (e.g. Allemann, Hammes-Schiffer, Klinman, Kohen, Sutcliffe, Warshel). The transfer then occurs via paths such as α or β. Also pointed out largely in the same articles, is the gating that can facilitate H-transfer or in the present terminology, decrease in the R-coordinate. The role of tunnelling is also stressed, with a three-coordinate quantum description and surface hopping of the H-transfer employed by Hammes-Schiffer. The three-coordinate analysis permits the inclusion of quantum aspects of a bending vibration of the A–H–B centre.
The presence of several steps in the overall transfer in some systems is frequently noted, e.g. Allemann, Banerjee, Burton and Kohen. When there are several steps, a full-kinetic analysis is needed to disentangle the KIE for the H-transfer from the other effects.
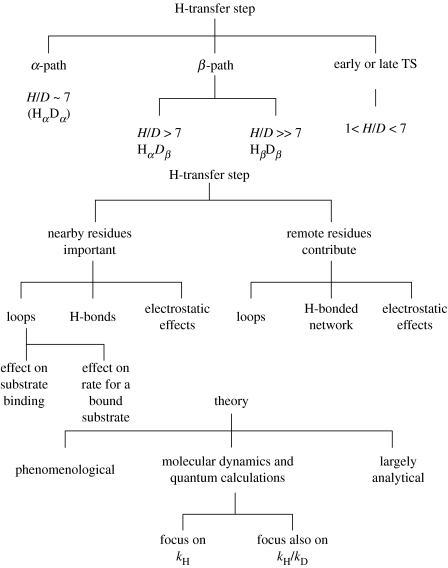
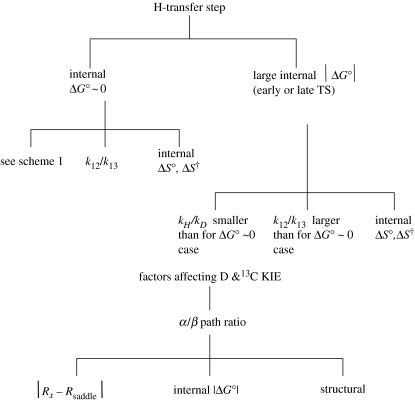
How may one classify the many results, theories and findings of the present Discussion? More than one property indeed appears to be needed. For example, in an enzymatic reaction, there is the KIE , which might be classified as in scheme 1. To incorporate asymmetric cases scheme 1 is extended to scheme 2.
Scheme 1.
Classification of H-transfer results.
Scheme 2.
Extension of scheme 1 to include asymmetric reactions.
In addition to the ratio, there is the ratio of the corresponding Arrhenius pre-exponential factors . At sufficiently low temperatures, the reaction occurs by tunnelling from the zero-point state of both H and D, and so and the activation energy difference, , is close to zero. At somewhat higher temperatures, the reaction for D will be over-the-barrier, but H still tunnels, and so and there is a large for . At still higher temperatures, and hence higher Es and smaller Rs both H and D systems are of the over-the-barrier type and so (also somewhat larger than 1) and any for is smaller, and is related to the zero-point energy differences. If we label this behaviour as , and , respectively (β for tunnelling via a β path and α for over-the-barrier via an α path), an behaviour is seen by Kohen for the wild-type, with the onset of for the mutants. The paths (for the wild-type) and (for the mutant) is seen by Klinman, and and by Allemann. Examples of , and perhaps are found in Sutcliffe.
But how does one define tunnelling in semi-classical terms? In a coordinate-free semi-classical description, there is tunnelling when the distorted rectangles in figure 2 do not touch or overlap. Clearly, there can be an energy region where the rectangles would overlap and then there is a division of the phase space; one trajectory starting in the reactants' well may stay localized in that well, another may start in and stay localized in the products' well and a trajectory in a third class will occupy parts of both wells. The first two are connected by nuclear tunnelling path, and the third provides a classically allowed path, an α path. Only when the third class is absent or of negligible importance is the process fully a tunnelling one.
We see that for any initial H-quantum state, there can be both classically forbidden and classically allowed transitions in the borderline energy region. Examining the classical trajectory or trajectories corresponding to an initial quantum state, this three atom or so cluster with environmental configurations that permit the energy of the products' state to equal that of the reactants' just before and after the H-transfer, can provide additional insight into tunnelling in several dimensional QM calculations. If one uses three coordinates in the quantum mechanics such as the three coordinates of the H-atom (Hammes-Schiffer) one uses three in the corresponding semi-classical analysis (Noid et al. 1980).
The behaviour shown in figure 2 is sometimes described in a different language; at any R, one has a two-potential well problem as a function of θ, with a diabatic protonic wavefunction defined for each well. When R is reduced, the two diabatic wave functions increasingly overlap and eventually, if continued to the saddle-point region, merge into one (e.g. Kiefer & Hynes 2002). The region of small overlap corresponds to spatially well-separated diabatic H-quantum states before and after the transfer.
Results obtained from site-directed mutagenesis in proteins, indicated in scheme 1, are also of particular interest. There is sometimes a large effect of mutants of remote residues, attributed to changes in hydrogen-bonded networks and in electrostatic effects caused by the mutations (Allemann, Hammes-Schiffer, Klinman, Kohen).
Various miscellaneous effects on enzymatic rates are also seen, e.g. the effect of pH on changing the rigidity of the protein structure and its effect on the rates (Allemann), and the effect of temperature and of the more proximal side chains on the protein flexibility (Klinman). A difference between a calculated and the observed temperature dependence of kH/kD is found by Warshel, the data being almost temperature-independent and the calculated results showing a marked decrease of the ratio with increasing temperature. It will be interesting to see if in subsequent calculations, the calculated values are of the type, while the experimental result is . The measured KIE of 70–95 (Knapp et al. 2002) is large, and . Both the features indicate significant tunnelling of H and D, as so are labelled . A large KIE is also obtained in calculations by Warshel. The error in the calculated temperature dependence is attributed (A. Warshel 2006, personal communication) to the shallowness of the potential, making it difficult to determine the important Rs for the tunnelling.
When kH and kD are calculated separately it is possible that kH may be reasonably accurate but that the calculated ratio kH/kD and its temperature dependence may be in substantial error; a small error in the two free energies of activation can cause a large error in the ratio. To reduce the error in kH/kD one presumably needs to focus in the calculation on the detailed nature of the H-transfer mechanics, as a number of papers in the Discussion have done and as in figure 2.
The effect of changing remote amino acid residues, explored by site-directed mutagenesis, the effect of binding of the cofactor and substrate on conformational changes near and far from the catalytic site, revealed by nuclear magnetic resonance relaxation, and the use of X-ray studies to trace the reaction pathway, were each noted by Hammes-Schiffer, and correlations are also discussed by Allemann and by Kohen. The correlated motions have been investigated in classical dynamics simulations by Hammes-Schiffer and others (e.g. Rod et al. 2003). Related computations linked to hydrogen-bonded networks and to catalysis were discussed (Allemann, Kohen, Hammes-Schiffer). The Swain–Schaad relationship for H/D/T KIE is another property studied in these systems and its deviations due to tunnelling are discussed by Klinman.
Yet another classification of the studies may be based on theoretical calculations, e.g. in scheme 1. As discussed in this symposium, detailed calculations of rate constants and of the KIEs are typically based on a combination of protein structural data, QM calculations of the cluster of atoms near the H-transfer reaction centre, MM for other coordinates and TST. Comparisons with this experiment are described in the articles of Banerjee, Burton, Hammes-Schiffer, Sutcliffe, Burton and Warshel. Such calculations at the molecular level permit an estimate in a fundamental way of the quantity related to the reorganization term in equation (2.6). To the extent that one is interested in minimizing QM tunnelling effects so that one can focus in computation on the structural factors, it is useful to compare experimental and computational s since they are less affected by H-quantum effects.
A comparison of reactions in solution and the corresponding enzymatic reactions is discussed by Warshel and Burton. In an example cited by Burton, the presence of water, by its bonding, inhibits the reaction, while Warshel notes examples where the calculated KIE is similar in the two media.
Using a time-dependent correlation function to calculate the rate, and a cumulant expansion, truncating as is frequently done to quadratic terms, Warshel obtains the microscopic equivalent of the in equation (2.4). Statistical mechanics and the truncated cumulant expression have been useful in a variety of other problems, including electron transfers, in relating the quadratic exponent to microscopic properties (e.g. Georgievski et al. 1999 and references therein). As many authors have recognized in discussing electron transfers in proteins, the protein serves to reduce the ‘reorganization energy’. The same effect occurs for H-transfers in proteins. The results are also related to other approximate formulations (Borgis & Hynes 1996; Antoniou & Schwartz 1997; Kusznetsov & Ulstrup 1999; Kiefer & Hynes 2004). The basic centroid path integral method (Gillan 1987; Voth et al. 1989) has been adapted to these larger systems by Warshel. The effect of a decrease in R is to decrease tunnelling and so to decrease the KIE, as pointed out by Warshel. In terms of figure 1, a decrease in R increases the ratio of α to β paths, and so decreases the amount of tunnelling and thereby decreases kH/kD.
A unique study is described by Northrop, the effect of pressure on the deuterium and KIEs, the only study for KIE (D. L. Northrop 2005, personal communication). We first recall that in the calculations of Hammes-Schiffer, the donor–acceptor A–B distance in various proteins is typically reduced to approximately 2.7 Å to reach the TS, at room temperature and atmospheric pressure. Since inter-atomic distances are compressed in order to reach the TS region, the effect of increased pressure is to increase the ratio of α to β paths in figure 1. In Isaacs' study of a non-enzymatic reaction, described by Northrop in this Discussion, the kH/kD decreases with increasing pressure from a value of 11 to a value of about 7.9. At atmospheric pressure in Northrop's enzymatic reaction, the kH/kD is about 4.9 and decreases with increasing pressure to about 2.7 and at normal pressure and decreases to about 1.01 at high pressures. Northrop also found that the decrease for k12/k13 and kH/kD occurs in the same pressure interval. Assuming a factorization of the KIEs into zero-point energy and tunnelling factors, he succeeded in fitting the data on the two KIEs, using a number of parameters. While the parametrization and the model do not explain why the two KIEs change over the same pressure interval, the fitting represents an interesting first attempt and a challenge to fundamental approaches.
To explain the trends and why the two KIEs change over the same pressure range, we first recall that all masses in AHB contribute to the effective mass for the tunnelling or for going over-the-barrier, not just H. A change in the mass of either A or B changes the contours in the mass-weighted diagram in figure 2, the distorted rectangles there, and the acute angle. Phrased slightly differently, it is not just the H that tunnels or goes over the barrier. Since the nearby atoms contribute to the ‘effective mass’ they do also, but their contribution is considerably smaller, a KIE of a few per cent instead of a factor of the order of 10 for deuterium! So, it is perhaps not surprising in hindsight that the and deuterium KIEs change in same pressure range.
An interpretation of the decrease in the KIEs with increasing pressure is that an increase of pressure favours a smaller R and so increases the ratio of α to β paths, thereby reducing the tunnelling and so decreasing kH/kD, and since all masses contribute to the effective mass, decreasing the k12/k13. In passing, we note that pressure can also affect each H-transfer k by affecting ΔG° for the H-transfer step (the internal ), as in equations (2.5) and (2.6). This can affect the KIEs when there is a differential effect for different isotopes. A determination of the ΔV° for the H-transfer step (the internal ΔV°) for each isotope would be of interest in understanding the pressure effect.
It would also be interesting to study the effect of pressure on an enzyme for which kH/kD is considerably greater than the value of 4.9 in the present study, namely a KIE comparable with the high values reported for some other enzymatic reactions, e.g. (Banerjee) or (Knapp et al. 2002; Hammes-Schiffer). If the relevant thermodynamic data are more available for non-enzymatic reactions than for enzymatic ones, the former group may be more desirable for initial tests of fundamental theories.
A result that initially seemed puzzling to me is the factor of 3 in , interpreted from the wave function as ‘tunnelling’ (Hammes-Schiffer). Frequently, there will be some tunnelling and in spite of that, perhaps because of asymmetry, kH/kD<7. It would be of interest for insight to specify in calculations some measure of the calculated asymmetry, i.e. the difference of the calculated internal from zero, where and λ are inferred from the calculations. To the extent that the flux method of Kuppermann, Adams and Truhlar (Kuppermann 1981) can be adapted to these systems, one can have a precise quantification of how much of the reactive flux is classically forbidden. The use of classical trajectories and figures such as figure 2 or a somewhat asymmetric version of that can also provide insight into the classically allowed and classically forbidden contributions to the reactive flux.
The Discussion is not without diversity of opinion, as for example the different views of Warshel and Hammes-Schiffer on the one hand, and of Schwartz on the other on the question of dynamical, effects. Many effects that may sometimes appear as dynamical, such as the effect of distant residues, can be consistent with a statistical behaviour, as in TST (Warshel, Hammes-Schiffer). In order to resolve the question of a statistical versus a dynamical effect, a definition is needed and can be made in terms of correlations, e.g. a correlation between a vibrational excitation at one point and reaction at another. If the correlation is substantially larger than what one would obtain from a statistical theory, subject to whatever constraints one might impose on the statistics, then the correlation can be termed dynamical.
Efforts to find such correlations in experiments on isolated dissociating small molecule gas-phase systems (say 10 atoms) have been negative, except in rare experiments where the time measurement is so short that there is no time for redistribution of energy among the various vibrations of the molecule before the dissociation. In the field of gas-phase unimolecular reactions, this non-statistical behaviour of an isolated molecule of a given energy and total angular momentum is termed non-Rice–Ramsperger–Kassel–Marcus (non-RRKM) and is absent for the relevant energies except in the extremely short-time experiments. In the case of proteins, one could try to search for such correlations in computations, but at present the computations are restricted to times in the order of 10 ns, whereas events of interest in enzymatic catalysis are on the order of milliseconds. Even in 10 ns, the correlations were mixed and a different explanation, based on kinetics between different groups of configurations of the hydrogen-bonded networks, was invoked (Rod et al. 2003).
Clearly, this point will be discussed in the future, together with a discussion of what specific experiments can distinguish the two views. We note that TST is a theory of rare events. For example, based on a typical vibration frequency of 1013 s−1, the probability of reaction for a first-order reaction that requires a millisecond is 103/1013, i.e. 1 in 1010. So the rarity of an event does not in itself require a dynamical origin.
A topic that played a major role in the interaction of theory and experiment in the field of electron transfers is the effect of on the rate constant (Marcus & Sutin 1985). While this aspect has not been discussed at this meeting, an example for enzymes is found in a recent study (Brinkley & Roth 2005). Another significant topic in electron transfers, even explaining an observed negative energy of activation, is the effect of on the entropy of activation ΔS† (e.g. Marcus & Sutin 1975, 1985). However, perhaps such information on may not be readily available for enzymes. While an equilibrium constant K is sometimes given for the H-transfer step, and used to adjust approximately an assumed potential energy surface in calculations, a value of d(kT ln K)/dT is needed to obtain the internal . The information has potential application for understanding the different temperature behaviour of different enzymes (e.g. Knapp et al. 2002; Liang et al. 2004). Although this topic has not been considered in this Discussion, I added it to scheme 2 because of its potential importance. One expects ΔS° for charge separation reactions to be more negative and ΔS° for charge recombination reactions to be more positive than that for charge shift reactions. A similar remark applies to the entropy of activation, but this is modified by the effect of nuclear tunnelling, which adds a negative component to it.
While the present summary has focused on H-transfers, there are stimulating papers on electron transfers by Dutton and by Onuchic, and Nocera on coupled electron–proton transfer, and an interesting paper by Limbach on a phenomenological approach to H-transfer. A large literature on electron transfers in proteins exists and is discussed in these articles. A variety of biological electron transfers, such as those involving electron transfer between the catalytic cofactor and the DNA thymine dimer involved in DNA repair, have now been studied in combination with MM (e.g. Antony et al. 2000).
It has been too early to include detailed papers on single molecular studies, although reference is made to the millisecond time-scale in proteins found in such studies (Hammes-Schiffer). Now, there are number of single molecular studies in the protein literature and we can expect to see more in the next symposium on enzymatic catalysis of H-transfers. In a recent paper on semi-conductor quantum dots, we have noted that single particle and ensemble experiments are complementary, rather than competitive in the information they provide (Tang & Marcus 2005). The long-time behaviour of single particles gets lost in the noise, whereas in ensemble studies, the behaviour has contributions from both short- and long-time events, and so provides information on the long-time behaviour.
4. Lessons learned from the electron transfer field?
The influence of concepts originating in electron transfers on the study of enzymatic catalysis is clear from the many papers in this Discussion. Are there also perhaps, some particular topics studied in electron transfers but not yet applied or only rarely applied to enzymatic catalysis and to KIEs, topics which may distinguish specific from thermodynamic effects in the catalysis?
Thermodynamic factors such as affect the rate constant, and affects the pre-exponential factor. They have played a key role in understanding particular aspects of electron transfers. Examples for proteins include the work of Mines et al. (1996) and Dutton, where is varied by varying one of the reactants. With such studies, information was obtained about a specific factor, in this case the electronic coupling between donor and acceptor.
In contrast to electron transfers, an H-transfer is typically a short-range effect, and so a question arises as to whether one can still vary by varying a substituent without causing other changes such as in H-bonded networks. At low s will the slope of a plot of versus have the value of 1/2 found in electron transfers and in equation (2.6)? One such study (Brinkley & Roth 2005) was referred to in the previous section. Ultimately, for H-transfers the limiting behaviour at extremely large would differ from that for electron transfers, namely a large ‘inverted effect’ at sufficiently high driving force, , should not occur in the adiabatic (i.e. no H-tunnelling) case (Marcus 1968). The barrier now occurs in the reactants channel, as in figure 3. The question of an inverted effect in the H-tunnelling case is still, I believe, an open question.
An aspect where played a major role in electron transfers was in understanding the negative activation energy of an electron transfer (Marcus & Sutin 1975) and was referred to earlier. However, the measurement of the internal in enzymes seems to be rare or non-existent—perhaps due to the challenges in obtaining the ‘internal’ equilibrium constants and their temperature derivative? For many electron transfers, these experimental data were often readily accessible. If one knows the internal , one can determine to what extent the high-negative entropy of activation of an enzyme (one which operates at low temperatures in the millisecond range) is due in part to a very negative . In this way, one can distinguish between thermodynamic (i.e. , ΔG°, ΔV°) and specific effects. The answer can also be used to test computational models. Do they also give a very negative and if so, why? The , and ΔV° provide constraints on these computations. We also referred earlier to an expected difference in charge shift, charge recombination and charge separation reactions.
Again, in looking at a KIE, to what extent are some of the smaller KIEs (closer to unity) due to the reaction not being at an internal ? This general effect of on KIEs in simple systems has been studied and there can be a lot of scatter (Bell 1973) reflecting specific structural effects, but are some of the smaller KIEs in the present Discussion small due to the internal being quite different from zero? While the range of s for natural enzymes appears to be small for the overall reaction, for them to operate in the millisecond regime, the variation in the internal s may be large. Broad issues such as the effect of thermodynamics (internal and ) versus specific factors influencing the KIEs can also be explored in computations, in addition to the important calculations that are made of rate constants, activation energies and KIEs. The study of enzymatic kinetics using a stopped-flow apparatus (Sutcliffe) can help unravel some of the reaction steps and so provide information on the thermodynamics properties of the H-transfer step.
In this context, there is an interesting study on the effect of a change in reaction asymmetry () on the KIEs in an enzymatic system. The asymmetry was increased by changing . For one nucleotide substrate, the deuterium KIE was 6.5 and the was 1.012 and for another substrate which formed a more asymmetrical system (larger ) the deuterium KIE decreased from 6.5 to 4 and the increased from 1.012 to 1.025 (Scharschmidt et al. 1984). Thus, on increasing the asymmetry (figure 3 is an extreme case of an asymmetrical system) the deuterium KIE decreases and the KIE, in contrast, increases. In an asymmetrical system, R becomes a more dominant contributor to the reaction coordinate and so the KIE increases while the deuterium KIE decreases, in agreement with the data.
From the present Discussion, we infer that three factors influencing the KIE and the deuterium KIE are (i) the asymmetry, i.e. |ΔG°|, (ii) the distance from the closest approach Rx to the saddle-point Rsaddle, and (iii) structural and other specific effects, as indicated in scheme 2. The first factor is prompted by results of Scharschmidt et al. (1984), the second by the pressure effect of Dexter and the third is tentatively inferred from the extreme isotope effects.
5. Concluding remarks
In summary, these notes are intended to give an idea of the wealth and diversity of new information and insights into the various mechanistic aspects of enzymatic catalysis and an indication of challenges that lie ahead. The organizers of this symposium are to be congratulated for setting up this timely Discussion and bringing so many interesting results and analyses together in one volume.
Acknowledgments
It is a pleasure to acknowledge the support of this research by the Office of Naval Research and the National Science Foundation. I am pleased to acknowledge too the helpful comments of Prof. M. E. Michel-Beyerle. I have benefitted also from many helpful suggestions, clarification, and comments from participants in this Discussion. It is a pleasure to express my appreciation to them. I thank my coworkers, Meher Prakash and Wei-Chen Chen, for the figures.
Appendix A
The derivation of equation (2.1) is standard; the probability of the system being near the TS in an element of phase space along a reaction coordinate q just prior to tunnelling or going over the barrier is and so the linear probability density along q is this quantity divided by dq. When multiplied by the velocity and by the probability of reaction and integrated over all p one obtains equation (2.1), since , μ being the reduced mass for this motion along q.
We note, in virtue of a remark in the Discussion, that the TS of is not simply the saddle-point, but instead is an N−1 dimensional space in a space of N coordinates, which may or may not contain the saddle-point. More generally, as Wigner (1938) pointed out, it is really a 2N−1 subspace in a 2N-dimensional phase space (coordinate–momentum space). In some cases, this distinction between coordinate space and phase space is important, but typically is not expected to be so for the present enzymatic systems, except when ‘viscous’ effects occur. In coordinate space, they lead in that case to many recrossings. An example of the importance of a TS, hypersurface in (q, p) space in a totally different field is the recombination of atoms in a gas to form a diatomic molecule in the presence of a third body; the third body removes the excess energy of the newly formed atom–atom bond, a necessary step for forming a stable diatomic molecule in a non-radiative process (Wigner 1939). Thereby the dividing surface (TS) cannot be defined solely in q space but was defined in (q, p) space.
Using a coordinate definition of the TS, Kramers (1940) described deviations arising from viscous effects. These effects may not be important for the enzymatic reactions and have not been invoked in the articles in this Discussion.
We have noted the small curvature and large curvature approximations in the text. Using a curvilinear coordinate system for the small curvature case, it was possible to formulate a QM treatment that in the tunnelling region led to a ‘corner cutting’ (negative centrifugal force; Marcus 1966). However, when the radius of curvature becomes small, there are complications, and one uses a different coordinate system (‘hyperspherical coordinates’) often used in accurate QM computations of chemical reactions. For two coordinates, the latter becomes polar coordinates (R, θ) used in Babamov & Marcus (1981). Extensive developments of small and large curvature methods and combination with other methods for treating complex systems are described in Fernandez-Ramos & Truhlar (2001, 2005). Because of the complications, there can be an advantage in analyses aimed at physical insight in using a semi-classical coordinate-free method for the analysis and comparison with selected QM computations.
Footnotes
One contribution of 16 to a Discussion Meeting Issue: ‘Quantum catalysis in enzymes—beyond the transition state theory paradigm’.
References
- Albery W.J. A Marcus model for concerted proton-transfer. Faraday Disc. Chem. Soc. 1982;74:245–256. doi:10.1039/dc9827400245 [Google Scholar]
- Antoniou D, Schwartz S.D. Large kinetic isotope effects in enzymatic proton transfer and the role of substrate oscillations. Proc. Natl Acad. Sci. USA. 1997;94:12 360–12 365. doi: 10.1073/pnas.94.23.12360. doi:10.1073/pnas.94.23.12360 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Antony J, Medvedev D.M, Stuchebrukhov A.A. Theoretical study of electron transfer between the photolyase catalytic cofactor FADH(−) and DNA thymine dimer. J. Am. Chem. Soc. 2000;122:1057–1065. doi:10.1021/ja993784t [Google Scholar]
- Appleby A.J, Bockris J.O'M, Sen R.K, Conway B.E. Quantum mechanical model for electronic charge transfer at interfaces. MTP Int. Rev. Sci. Phys. Chem. Ser. One. 1973;6:1–40. [Google Scholar]
- Babamov V.K, Marcus R.A. Dynamics of hydrogen atom and proton transfer reactions. Symmetric case. J. Chem. Phys. 1981;74:1790–1798. doi:10.1063/1.441267 [Google Scholar]
- Bell R.P. Cornell University Press; Ithaca, NY: 1973. The proton in chemistry; p. 265. [Google Scholar]
- Bell R.P. Chapman and Hall; London, UK: 1980. The tunnel effect in chemistry; p. 103. [Google Scholar]
- Borgis D, Hynes J.T. Curve crossing formulation for proton transfer reactions in solution. J. Phys. Chem. 1996;100:1118–1128. doi:10.1021/jp9522324 [Google Scholar]
- Brinkley D.W, Roth J.P. Determination of a large reorganization energy barrier for hydride abstraction by glucose oxidase. J. Am. Chem. Soc. 2005;127:15 720–15 721. doi: 10.1021/ja056025l. doi:10.1021/ja056025l [DOI] [PubMed] [Google Scholar]
- Dogonadze R.R, Kuznetsov A.M, Levich V.G. Theory of hydrogen ion discharge on metals—case of high irreversibility. Electrochim. Acta. 1968;13:1025–1044. doi:10.1016/0013-4686(68)80033-7 [Google Scholar]
- Fernandez-Ramos A, Truhlar D.G. Improved algorithm for corner-cutting tunneling calculations. J. Chem. Phys. 2001;114:1491–1496. doi:10.1063/1.1329893 [Google Scholar]
- Fernandez-Ramos A, Truhlar D.G. A new algorithm for efficient direct-dynamics calculations of large-curvature tunneling and its application to radical reactions with 9–15 atoms. J. Chem. Theory Comput. 2005;1:1063–1078. doi: 10.1021/ct050153i. doi:10.1021/ct050153i [DOI] [PubMed] [Google Scholar]
- Fernandez-Ramos A, Truhlar D.G, Corchado J.C, Espinosa-Garcia J. Interpolated algorithm for large-curvature tunneling calculations of transmission coefficients for variational transition state theory calculations of reaction rates. J. Phys. Chem. A. 2002;106:4957–4960. doi:10.1021/jp014204t [Google Scholar]
- Georgievskii Y, Hsu C.P, Marcus R.A. Linear response in theory of electron transfer reactions as an alternative to the molecular harmonic oscillator model. J. Chem. Phys. 1999;110:5307–5317. [Google Scholar]
- Gillan M.J. Quantum-classical crossover of the transition rate in the damped double well. J. Phys. C. Solid State Phys. 1987;20:3621–3641. doi:10.1088/0022-3719/20/24/005 [Google Scholar]
- Glasstone S, Laidler K.J, Eyring H. McGraw-Hill; New York, NY: 1941. The theory of rate processes; pp. 100–102. [Google Scholar]
- Jarczewski A, Hubbard C.D. A review of proton transfer reactions between various carbon-acids and amine bases in aprotic solvents. J. Mol. Struct. 2003;649:287–307. doi:10.1016/S0022-2860(03)00086-3 [Google Scholar]
- Kiefer P.M, Hynes J.T. Nonlinear free energy relations for adiabatic proton transfer reactions in a polar environment. I. Fixed proton donor–acceptor separation. J. Phys. Chem. A. 2002;106:1834–1849. [Google Scholar]
- Kiefer P.M, Hynes J.T. Kinetic isotope effects for nonadiabatic proton transfer reactions in a polar environment. 2. Comparison with an electronically diabatic description. J. Phys. Chem. A. 2004;108:11 809–11 818. doi:10.1021/jp040498h [Google Scholar]
- King G, Warshel A. Investigation of the free-energy functions for electron-transfer reactions. J. Chem. Phys. 1990;93:8682–8692. doi:10.1063/1.459255 [Google Scholar]
- Klippenstein S.J, Babamov V.K, Marcus R.A. A test of two approximate two-state treatments for the dynamics of H-atom transfers between two heavy particles. J. Chem. Phys. 1986;85:1924–1930. doi:10.1063/1.451835 [Google Scholar]
- Knapp M.J, Rickert K, Klinman J.P. Temperature-dependent isotope effects in soybean lipoxygenase-1: correlating hydrogen tunneling with protein dynamics. J. Am. Chem. Soc. 2002;124:3865–3874. doi: 10.1021/ja012205t. doi:10.1021/ja012205t [DOI] [PubMed] [Google Scholar]
- Kramers H.A. Brownian motion in a field of force and the diffusion model of chemical reactions. Physica. 1940;7:284–304. doi:10.1016/S0031-8914(40)90098-2 [Google Scholar]
- Kuppermann A. Accurate quantum calculations of reactive systems. Theoretical chemistry: advances and perspectives. vol. 6A. Academic Press; London, UK: 1981. pp. 79–164. [Google Scholar]
- Kuppermann A, Hipes P.G. Three-dimensional quantum mechanical reactive scattering using symmetrized hyperpherical coordinates. J. Chem. Phys. 1986;84:5962–5963. doi:10.1063/1.450781 [Google Scholar]
- Kuznetsov A.M, Ulstrup J. Proton and hydrogen atom tunneling in hydrolytic and redox enzyme catalysis. Can. J. Chem. 1999;77:1085–1096. doi:10.1139/cjc-77-5-6-1085 [Google Scholar]
- Liang Z.X, Lee T, Resing K.A, Ahn N.G, Klinman J.P. Thermal-activated protein mobility and its correlation with catalysis in thermophilic alcohol dehydrogenase. Proc. Natl Acad. Sci. USA. 2004;101:9556–9561. doi: 10.1073/pnas.0403337101. doi:10.1073/pnas.0403337101 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Liu Y.P, Lynch G.C, Truong T.N, Lu D.H, Garrett B.C. Molecular modeling of the kinetic isotope effect for the [1,5]-sigmatropic rearrangement of cis-1, 3-pentadiene. J. Am. Chem. Soc. 1993;115:2408–2415. doi:10.1021/ja00059a041 [Google Scholar]
- Marcus R.A. Theory of oxidation–reduction reactions involving electron transfer 4. A statistical–mechanical basis for treating contributions from solvent, ligands, and inert salt. Discuss. Faraday Soc. 1960;29:21–31. [Google Scholar]
- Marcus R.A. Theoretical relations among rate constants, barriers, and Brönsted slopes of chemical reactions. J. Phys. Chem. 1968;72:891–899. doi:10.1021/j100849a019 [Google Scholar]
- Marcus R.A. Extension of the WKB method to wave functions and transition probability amplitudes (S-matrix) for inelastic or reactive collisions. Chem. Phys. Lett. 1970;7:525–531. doi:10.1016/0009-2614(70)80164-6 [Google Scholar]
- Marcus R.A. Similarities and differences between electron and proton transfers at electrodes and in solution. Theory of a hydrogen evolution reaction. In: Bruckenstein S, McIntyre J.D.E, Miller B, Yeager E, editors. Proceedings of the third Symposium Electrode Processes, 1979. Electrochemical Society; Princeton, NJ: 1980. pp. 1–12. [Google Scholar]
- Marcus R.A, Coltrin M.E. A new tunneling path for reactions such as H+H2→H2+H. J. Chem. Phys. 1977;67:2609–2613. doi:10.1063/1.435172 [Google Scholar]
- Marcus R.A, Sutin N. Electron-transfer reactions with unusual activation parameters. A treatment of reactions accompanied by large entropy decreases. Inorg. Chem. 1975;14:213–216. doi:10.1021/ic50143a051 [Google Scholar]
- Marcus R.A, Sutin N. Electron transfers in chemistry and biology. Biochim. Biophys. Acta. 1985;811:265–322. [Google Scholar]
- Miller W.H. Semiclassical theory of atom–diatom collisions—path integrals and classical S-matrix. J. Chem. Phys. 1970;53:1949–1959. doi:10.1063/1.1674275 [Google Scholar]
- Mines G.A, Bjerrum M.J, Hill M.G, Casimiro D.R, Chang I.J, Winkler J.R, Gray H.B. Rates of heme oxidation and reduction in Ru(His33) cytochrome c at very high driving forces. J. Am. Chem. Soc. 1996;118:1961–1965. doi:10.1021/ja9519243 [Google Scholar]
- Nam K, Prat-Resina X, Garcia-Viloca M, Davi-Kesavan L.S, Gao J. Dynamics of an enzymatic substitution reaction in haloalkane dehalogenase. J. Am. Chem. Soc. 2004;126:1369–1376. doi: 10.1021/ja039093l. doi:10.1021/ja039093l [DOI] [PubMed] [Google Scholar]
- Noid D.W, Koszykowski M.L, Marcus R.A. Semiclassical calculation of eigenvalues for a three-dimensional system. J. Chem. Phys. 1980;73:391–395. doi:10.1063/1.439886 [Google Scholar]
- Noid D.W, Koszykowski M.L, Marcus R.A. Quasiperiodic and stochastic behavior in molecules. Ann. Rev. Phys. Chem. 1981;32:267–309. doi:10.1146/annurev.pc.32.100181.001411 [Google Scholar]
- Ovchinnikova M.Y. Tunneling dynamics of the low temperatureH- exchange-reactions. Chem. Phys. 1979;36:85–95. doi:10.1016/0301-0104(79)85106-X [Google Scholar]
- Rod T.H, Radkiewicz J.L, Brooks C.L. Correlated motion and the effect of distal mutations in dihydrofolate reductase. Proc. Natl Acad. Sci. USA. 2003;100:6980–6985. doi: 10.1073/pnas.1230801100. doi:10.1073/pnas.1230801100 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Scharschmidt M, Fisher M.A, Cleland W.W. Variation of transition-state structure as a function of the nucleotide in reactions catalyzed by dehydrogenases. 1. Liver alcohol dehydrogenase with benzyl alcohol and yeast aldehyde dehydrogenase with benzaldehyde. Biochemistry. 1984;23:5471–5478. doi: 10.1021/bi00318a015. doi:10.1021/bi00318a015 [DOI] [PubMed] [Google Scholar]
- Tang J, Marcus R.A. Single particle versus ensemble average. From power-law intermittency of a single quantum dot to quasi-stretched exponential fluorescence decay of an ensemble. J. Chem. Phys. 2005;123 doi: 10.1063/1.2128409. doi:10.1063/1.2128409 art. no. 204 511. [DOI] [PubMed] [Google Scholar]
- Voth G.A, Chandler D, Miller W.H. Rigorous formulation of quantum transition state theory and its dynamical corrections. J. Chem. Phys. 1989;91:7749–7760. doi:10.1063/1.457242 [Google Scholar]
- Wigner E. The transition state method. Trans. Faraday Soc. 1938;34:29–40. doi:10.1039/tf9383400029 [Google Scholar]
- Wigner E.P. Some remarks on the theory of reaction rates. J. Chem. Phys. 1939;7:646–650. doi:10.1063/1.1750508 [Google Scholar]