Exo-endocytosis at mossy fiber terminals: Toward capacitance measurements in cells with arbitrary geometry

Exocytosis and endocytosis are ubiquitous cellular phenomena necessary for diverse functions such as secretion, internal signaling, protein traffic, and motility. Many different techniques have been developed to assay exocytosis and endocytosis, but to date only electrical measurements of plasma membrane capacitance have had the time resolution necessary to capture both the fusion and reuptake of small clear-core vesicle membrane during fast neurotransmission. In this issue of PNAS, Hallermann et al. (1) present capacitance measurements from hippocampal mossy fiber nerve terminals during stimulated exocytosis. These are the first time-resolved membrane capacitance measurements from bona fide (albeit relatively large) bouton-type synaptic terminals, with diameters of ≈3 μm and a resting capacitance of ≈1 pF. The results obtained not only provide important data on basic synaptic properties of this nerve terminal, in particular on the vesicle pool size and the question of multivesicular release, but also provide an example of how to extend capacitance measurements from cells with simple and compact geometry to more general classes of neurons and nerve terminals.

The accessibility of exocytosis and endocytosis to voltage-clamp measurements stems from the fact that these processes, by their nature, change the surface area of the plasma membrane. Because the plasma membrane acts as an electrical capacitor, changes in surface area can be detected as changes in its total capacitance. Thus by electrical measurements, net changes in plasma membrane (i.e., exocytosis minus endocytosis) can be measured. Coupled with voltage protocols that open voltagegated calcium channels, this technique can be used to follow membrane changes during Ca²⁺-dependent secretion of neurotransmitters. If exocytosis and endocytosis are temporally distinct phenomena, the rate of exocytosis can be determined by measuring capacitance jumps triggered by step depolarizations of different durations (see refs. 2–5). In addition, synaptic vesicle membrane retrieval (or reinternalization via endocytosis) after fusion can be monitored in real time as a decrease in membrane capacitance back to baseline resting levels (6–9).

Most measurements obtained to date have relied on one of two general techniques to relate membrane current to capacitance (6, 9). Time-domain methods use the amplitude and time course of membrane current relaxations after step changes in electrical potential to determine cell membrane parameters.

These are the first membrane capacitance measurements from bona fide bouton-type synaptic terminals.

Frequency-domain methods use the phase shift between an applied sinusoidal membrane potential and the induced current to calculate the complex impedance of the circuit. In both techniques voltage-dependent conductances are avoided (pharmacologically and by careful choice of membrane potential) such that only passive (capacitive and leak) currents remain.

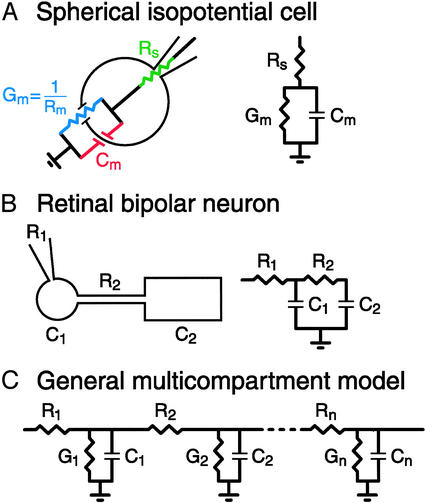
Regardless of the choice of technique, interpretation of the data depends on the use of an a priori electrical model of the preparation. The simplest model, appropriate for a compact isopotential cell, consists of the membrane capacitance in parallel with its conductance (6). The rest of the circuit (recording patch pipette, cytoplasm, and extracellular path) is combined into the series resistance term for a total of three passive parameters (C_m, G_m, and R_s; see Fig. 1). In this case, theoretical time-domain responses consist of single exponential relaxations from which the three cell parameters can be uniquely determined. Likewise, for a sinusoidal excitation, the components of current in phase and 90° out of phase with the voltage stimulus can be analyzed by using standard circuit theory to determine C_m, G_m, and R_s. To date, most capacitance measurements have been made on secretory cells for which the compact isopotential approximation seems, prima facie, to be justified, including adrenal chromaffin cells (10), mast cells (11), and neuroendocrine cells (12), which secrete via large dense-core vesicles. In addition, small clear-core synaptic vesicle fusion and membrane retrieval have been measured from retinal bipolar cell terminals (2, 3, 13), hair cells (4, 5), and photoreceptors (14). However, these sensory neurons contain nonconventional ribbon-type active zones (3, 13, 15).

Fig. 1.

Model circuits for different cell geometries. (A) Spherical isopotential cells. These cells can be described by three passive circuit parameters: membrane capacitance (C_m), membrane conductance (G_m), and series resistance (R_s, a composite parameter usually dominated by the resistance of the attached whole-cell mode recording patch pipette but also including the resistance of the cytoplasmic and extracellular paths). (B) Two-compartment model proposed by Mennerick et al. (18) to describe goldfish retinal bipolar neurons. This four-component model assumes negligibly low membrane conductance and axon capacitance. R₂ is the axon resistance, C₁ and C₂ are the synaptic terminal and cell soma capacitance, and R₁ is series resistance of a patch pipette recording from the synaptic terminal. (C) General n-compartment model described by 3n parameters.

Recently, attempts have been made to measure exocytosis in cells with complex geometry and multiple electrical compartments by using capacitance measurements. Hsu and Jackson (16) first showed that capacitance measurements are possible in pituitary slices from nerve varicosities (with attached axons) that secrete both via dense- and clear-core vesicles (17). Mennerick et al. (18) used the time-domain method to analyze the electrotonic structure of the intact goldfish retinal bipolar cell, consisting of a soma and terminal connected by a short axon. They showed that a two-compartment model adequately described their recordings and, under the assumption of high membrane resistance, allowed for unique determination of the resistance of the axon and the capacitance of the soma and terminal. A third example, the calyx of Held, is a giant nerve terminal in the brainstem ascending auditory pathway. This synapse, unlike the bipolar cell synapse, contains conventional active zones. Depending on the length of the attached axon, the calyx appears as one or more electrotonic compartments (19). Sun and Wu (20) used frequency-domain capacitance measurements and the compact isopotential cell approximation to measure exocytosis in calyces that behaved as a single electrical compartment (i.e., calyces that appeared to have a single exponential current relaxation in response to a step hyperpolarization). Using the same measurement technique, Taschenberger et al. (21) demonstrated empirically that capacitance jumps following exocytosis were similar across calyces, independent of whether the structure consisted of one or two electrical compartments. Thus it seems that capacitance measurements in the calyx of Held are not greatly affected by the attached axon. However, a rigorous modeling of such capacitance measurements would be useful to show the theoretical relationship of measured to true changes in membrane capacitance for different-length axons.

The approach adopted by Hallermann et al. (1) to calculate membrane capacitance from currents recorded from the mossy fiber terminal in slice started with an anatomical model obtained by reconstruction of a biocytin-filled terminal. Although they record directly from the bouton (where the active zones are located and thus the putative site of exocytotic capacitance changes), the presence of multiple large associated processes makes direct application of the spherical cell approximation inadequate. Indeed, the authors found that a three-compartment model was necessary to provide a fit to their data comparable to that provided by the full morphological model.

The authors then used their model cell to test several time- and frequency-domain capacitance measurements techniques to determine which most faithfully reported simulated changes in the electrical parameters of the bouton. Notwithstanding the complex structure, they found that time- or frequency-domain techniques with analysis based on single-compartment models were adequate to detect such changes. However, because this was only an approximation, the authors were careful to show the extent to which the calculations are valid and pointed out three artifacts that arose during their simulations. First, large simulated conductance decreases in the model terminal were associated with small phantom increases in calculated terminal capacitance. Second, a correction (<10%) was needed to relate the capacitance change reported by the calculations to the amplitude of the simulated capacitance jump. Finally, there was a correlated decrease in calculated series resistance with increasing simulated capacitance increases.

Having characterized the response of their model to simulated changes in membrane parameters, Hallermann et al. (1) went on to characterize tetanus toxin-sensitive capacitance jumps that occur during a step depolarization of the mossy fiber terminal, during which Ca²⁺ influx occurred. They found two components of capacitance increase in response to varying length depolarizations: a fast component with a time constant near 1 ms and a second, slower component with a time constant near 20 ms. They interpreted these components as distinct pools of synaptic vesicles with differential release kinetics. Recovery of membrane capacitance (i.e., putative endocytosis) after a maximally effective stimulation that depleted the releasable pool of vesicles occurred on a time scale of several seconds.

Perhaps the most provocative aspect of the article concerns the quantitative estimate of the rate of vesicle release per active zone and implications for multivesicular release (i.e., release of more than one vesicle per active zone per action potential) (22). The arguments stem from the observation that during a prolonged depolarizing voltage step, the calculated release corresponded, on average, to more than one vesicle per active zone per millisecond. If release were uniform in time during the depolarization, this would imply that lateral inhibition of release of vesicles from the same active zone, if present, was very short-lived (<1 ms, compare with action potential duration half-width of ≈0.6 ms). In fact, during the fast component of release, the rate per active zone may have been much higher. Assuming that all the increase in capacitance signal is due to exocytosis of synaptic vesicles that are docked at active zones, this measurement implies that multivesicular release occurs at this synapse. The list of synapses that experience multivesicular release has been growing recently and now includes both inhibitory and excitatory synapses in the cerebellum (23, 24), cultured hippocampal and cortical synapses (25, 26), and bouton-to-spine synapses in hippocampal slices (27). During early postnatal development, the immature calyx of Held also experiences multivesicular release, although this probably changes to univesicular release in more mature calyxes because release probability decreases with age (21).

The extension of capacitance measurements to hippocampal synaptic terminals is important also because these nerve terminals undergo presynaptic long-term potentiation (LTP) and long-term depression (LTD) mediated by cAMP and protein kinase A (PKA) (28, 29). LTP and LTD are ubiquitous among cortical synapses and are thought to be involved in learning and memory. Accordingly, LTP does not seem to occur in ribbon-type synapses and at the calyx of Held synapse, which probably function as highly specialized and faithful relay stations of presynaptic input under most circumstances in adult animals. Thus capacitance measurements, which have played an important role in studies of short-term synaptic plasticity at ribbon-type nerve terminals and neuroendocrine cells (30), now can be extended to long-term plasticity studies in more conventional nerve terminals. It will be very interesting, for example, to directly study presynaptic LTP via the dialysis of cAMP into mossy fiber terminals to determine whether presynaptic Ca currents and/or vesicle pool size are augmented and whether release machinery is affected via phosphorylation by PKA.

Capacitance measurements have proved to be a powerful tool in the study of secretion and synaptic physiology. To date, they have been applied mostly to certain model cells, chosen for their simple geometry and accessibility to patch-clamp electrophysiological techniques. As the field moves toward more general classes of cells and synaptic terminals, correct interpretation of electrophysiology data will depend on a careful combination of morphometry and modeling. The article by Hallermann et al. (1) shows the way forward.