Abstract
Four different conceptual models of metacommunities have been proposed, termed ‘‘patch dynamics,’’ ‘‘species sorting,’’ ‘‘mass effect,’’ and ‘‘neutral.’’ These models simplify thinking about metacommunities and improve our understanding of the role of spatial dynamics both in structuring communities and in determining local and regional diversity. We tested whether mosquito communities inhabiting water-filled tree holes in southeastern Florida, USA, displayed any of the characteristics and dynamics predicted by the four models. The densities of the five most common species in 3–8 tree holes were monitored every two weeks during 1978–2003. We tested relationships between habitat variables and species densities, spatial synchrony, the presence of life history trade-offs, and species turnover. Dynamics showed strong elements of species sorting, but with considerable turnover, as predicted by the patch dynamics model. Consistent with patch dynamics, there was substantial asynchrony in dynamics for different tree holes, substantial species turnover in space and time, and an occupancy/colonization trade-off. Substantial correlations of density and occupancy with tree hole volume were consistent with the species-sorting model, but unlike this model, species did not have permanent refuges. No evidence of mass effects was found, and correlations between habitat variables and dynamics were inconsistent with neutral models. Our results did not match a single model and therefore caution against overly simplifying metacommunity dynamics by using one dynamical characteristic to select a particular metacommunity perspective.
Keywords: Florida, USA, mass effect, metacommunity, mosquito, neutral model, patch dynamics, species sorting, synchrony, trade-off, tree hole, turnover
Introduction
Metacommunity ideas consider sets of local communities that are linked by dispersal at multiple spatial scales and attempt to provide an integrated explanation for regional species diversity (Leibold et al. 2004). By contrast, previous studies of spatial processes have focused only on the detailed dynamics of one or two species (metapopulation studies; e.g., Hanski and Gilpin 1997) or on patterns of biodiversity (MacArthur and Wilson 1967). Rarely have ecologists studied spatial community structure. This is despite the fact that mechanisms at a community level, such as multispecies interactions and trophic structure, may be necessary to explain and predict the manner in which biodiversity responds to anthropogenic disturbance and habitat change (e.g., habitat fragmentation). Moreover, examination of the role of spatial dynamics in community ecology has led ecologists to question the validity of some existing community theories. For instance, Roxburgh et al. (2004) showed that the intermediate disturbance hypothesis is an incomplete explanation for coexistence because it does not consider trade-offs in species traits that can generate regional coexistence.
Existing metacommunity studies have typically evaluated theoretical models consisting only of competitors (e.g., Amarasekare et al. 2004), empirically tested neutral community models (Bell 2001, Hubbell 2001, reviewed by Chave 2004), and empirically tested the role of competition and colonization trade-offs in coexistence (e.g., Levine and Rees 2002, Mouquet et al. 2004, Yu et al. 2004). Leibold et al. (2004) placed such tests in a broader context by describing four conceptual models, which we refer to as ‘‘patch dynamics,’’ ‘‘species sorting,’’ ‘‘mass effect,’’ and ‘‘neutral’’ (Table 1).
Table 1.
Characteristics of metacommunity models evaluated in this study (modified from Chase et al. [2005]) and their presence in tree hole mosquito communities.
| Characteristic | Patch dynamics | Species sorting | Mass effects | Hubbell’s neutral model |
|---|---|---|---|---|
| 1) Patch similarity | physical conditions similar | dissimilar in physical conditions | like species sorting | like patch dynamics |
| 2) Spatial synchrony | some asynchrony | not specified | not specified | some asynchrony |
| 3) Spatial and temporal variability in composition | variable locally and regionally | more or less constant locally and regionally | like species sorting | highly variable locally and regionally |
| 4) Effects of habitat on dynamics/composition | weak | required | not specified | similar in all species |
| 5) Life history trade-offs | necessary for regional coexistence | species differ in ability to perform in different conditions | like species sorting | assumed absent: identical competitive abilities and net fitness |
Notes: Characteristics in boldface type were supported by results presented here. Tree holes were located in a 4-ha woodland on the grounds of the University of Florida’s Florida Medical Entomology Laboratory in Vero Beach, Florida, USA.
In the patch dynamics model, populations in multiple identical patches are assumed to undergo both stochastic and deterministic extinctions that are counteracted by recolonization, as in predator–prey metapopulation models (Harrison and Taylor 1997). Because all patches are identical and permanent refuges are absent, local species composition and diversity vary both through time and in response to disturbance. Coexistence relies on an appropriate trade-off, such as between competitive and colonization abilities (or fecundity). Potential examples include the butterfly and parasitoid dynamics studied by van Nouhuys and Hanski (2005) and simplified microcosm systems in which patches are identical (Holyoak 2000, Gonzalez 2005).
The species-sorting model (Leibold et al. 2004) views local patches as heterogeneous in some factors, and the outcome of local species interactions depends on abiotic patch conditions. This approach focuses on differences among species that allow specialization on different patch types rather than on trade-offs between such traits and dispersal. Colonization is assumed to occur frequently enough that local assembly trajectories have reached their end states (Law and Morton 1993), but dispersal is not so frequent that species regularly occur in sinks (e.g., Pulliam 1988). Local species diversity and composition are expected to be relatively constant or bounded and to recover to previous states following disturbance. Species sorting appears to occur in a broad range of systems (Gilbert and Lechowicz 2004, Cottenie and De Meester 2005, Kolasa and Romanuk 2005, Miller and Kneitel 2005). The assumed absence of species from sink habitats is expected to break down as dispersal increases, promoting mass effects.
Mass effects can drive relationships between local conditions and community structure (Mouquet et al. 2005). Immigration can enhance densities of local populations beyond those expected in closed communities and can thereby rescue local populations from extinction. Mouquet et al. (2005) reviewed relevant modeling work and showed that coexistence requires a constraint of regional similarity between species. Such mass effects were found by Cottenie and De Meester (2005) and Miller and Kneitel (2005). Based on the evidence available, mass effects are not as frequent as species sorting in natural systems (Holyoak et al. 2005).
The three previous models assume that species differ from one another in their niches or abilities to disperse or avoid local extinction. In the absence of any such species differences, the dynamics of metacommunities are described by neutral community models (Hubbell 2001, Chave 2004). This view can be regarded as a null hypothesis for the other three views described above (Bell 2001), but it may also describe dynamics of some communities in which species are close to being equivalent or in which transient dynamics are very long. Species composition changes through a random process termed ecological drift (Hubbell 2001), and all but one species would go extinct unless there is some counteracting process (e.g., speciation). Under these conditions, Hubbell’s model shows slow random drift in species composition in space and time. Disturbances, however, could lead to abrupt changes in species composition. In a review, McGill et al. (in press) showed that stringent tests of neutral theory always selected alternative models (e.g., niche models) or null distributions (e.g., a logarithmic distribution) over neutral community models. Nonetheless, neutral models usefully highlight the role of species traits, dispersal limitation, stochasticity, and transient dynamics in generating community patterns.
Various differences between the conceptual models are either implied directly or follow from considering variation in factors included in the models (Table 1; Chase et al. 2005). For example, the models differ in their predictions about the constancy of local and regional species composition and degree of synchrony in local dynamics. Species composition is expected to vary more because of perturbations to density or local population extinctions in neutral and patch dynamics models, which contributes to asynchronous dynamics across local populations. In species-sorting and mass effect models, habitat characteristics should anchor species composition and generate more constant local and regional dynamics. While it is unlikely that natural communities will conform solely to any one of these models, they help to simplify thinking and they may provide insight into the degree to which spatial dynamics are important in structuring communities and diversity. To date, some empirical studies have used these models to guide studies of natural systems; however, most have typically only used snapshots of communities or a few generations of species composition and abundance data (though see Gonzalez 2005). Because communities are dynamic, tests spanning many generations are desirable.
Mosquito communities inhabiting water-filled tree holes present a rare opportunity to compare community dynamics to those described by the four metacommunity perspectives. Tree holes are discrete habitats for mosquito larvae, which facilitates definition of local community boundaries. Moreover, tree holes represent temporary distinct patches, suggesting that the mosquito communities may be structured by metacommunity processes (Leibold et al. 2004). Finally, the food web formed by mosquito species is well known (e.g., Bradshaw and Holzapfel 1983, Lounibos 1985, Teng and Apperson 2000, Griswold and Lounibos 2005).
We used differences between the four conceptual models of metacommunities to guide examination of mosquito communities inhabiting water-filled tree holes in southeastern Florida, USA. One of us (L. P. Lounibos) began in 1978 to census at fortnightly intervals the mosquito fauna of 3–8 tree holes in a woodland at Florida Medical Entomology Laboratory in Vero Beach, Florida. In addition to the long period of study, the system is unique because the component species and their interactions have been extensively studied (Table A2 [Appendix]). We evaluated the characteristics and dynamics of these natural communities and compared them to those predicted by each of the four metacommunity models (Table 1). First, we describe the study system and whether tree holes are expected to be similar in their physical conditions based on published information. Next, we present time series analyses to answer four questions: (1) Do habitat or extrinsic variables affect the dynamics of individual species, and do these effects differ between species (as in the species-sorting and mass effect models)? (2) Do species have synchronous dynamics in different patches? (3) Are species similar in their dynamics (as in the neutral model) or unequal? Or, is there evidence for trade-offs between colonization and competition ability (as in the patch dynamics model)? (4) Is species composition more variable across patches (as in the species-sorting and mass effect models) or through time (as in the neutral and patch dynamics models)? Finally, we used our results to illustrate the manner in which dynamics can show features of multiple metacommunity models and how these models inform us about the system being studied.
The Study System and Data
Bark-lined cavities or rot holes in hardwood trees collect water and provide habitat for communities of microorganisms, small invertebrates, and occasionally anurans (e.g., Kitching 1983, Yanoviak 2001). The fauna of tree holes varies regionally, but is dominated worldwide by similar groups of organisms (Röhnert 1950, Kitching 1971, 1983, Woodward et al. 1988, Barrera 1996, Yanoviak 2001). Much research on natural tree holes has focused on larval mosquitoes, partly because of their role as vectors of human disease. Although tree holes may persist for decades as aquatic habitats (Kitching 2000), studies spanning multiple years are rare (e.g., Kitching 1971, Lounibos 1981, Sota et al. 1994), and no published studies have examined 26 consecutive years of tree hole community dynamics.
Study site
Tree holes were located in a 4-ha woodland on the grounds of the University of Florida’s Florida Medical Entomology Laboratory (27°35′ N, 80°22′ W). The two dominant trees were the cabbage palm, Sabal palmetto, and the southern live oak, Quercus virginiana; the latter tree species contained all 15 censused holes. The area is relatively undisturbed and is adjacent to a biological reserve (Oslo Road Conservation Area) and 1 km from a brackish lagoon.
Time series data
Fortnightly sampling was uninterrupted from February 1978 through July 2003. Tree hole aquatic contents were removed by aspiration through a hose or turkey baster and brought to the laboratory. Volume was measured, mosquito immatures were sorted, counted, and identified to species, and samples were reconstituted and replaced. Fig. A1 (Appendix) shows the mean density of the five most common dipterous species over the 26-year period. During this period, the number of holes censused ranged from three to eight. While there were other water-holding tree holes in the woodland (~40 were found in one month in 1998; Raul Campos, personal observation), logistical constraints limited the number of tree holes that could be censused regularly. When the number of censused tree holes became low as a consequence of losses of water-holding capacity, alternative tree holes with water were found to replace them. Tree hole drying and the replacement of tree holes in some cases limited the kinds of statistics we could perform. For example we could not perform multivariate statistics (e.g., canonical correspondence analysis) that treated data from all tree holes as missing if a single tree hole had no water. Temperatures were recorded continuously from 1978 to 2003 in the woodland and from the water of one tree hole. Rainfall data were also collected. Census lengths, mean water volume, and mean hydroperiod for each tree hole monitored are described in Table A1 (Appendix).
Mosquito food web structure
The dipteran food web in the system described here is typically comprised of five species. Four species occurred regularly in censuses up to 1990: Toxorhynchites rutilus, Corethrella appendiculata, Ochlerotatus triseriatus, and Orthopodomyia signifera (Lounibos 1985). Although Corethrella appendiculata is now placed in the family Corethrellidae, it was formerly considered a mosquito (family Culicidae) by some authors (e.g., Belkin 1962) and has a similar life cycle, including blood-feeding adult females, similar ecological dynamics, and interacts in aquatic stages with the four mosquito species. Because it has similar ecological roles and interactions, we follow other studies in analyzing C. appendiculata with the true mosquitoes. In 1991, an invasive mosquito, Aedes albopictus, became established in the communities (Lounibos et al. 2001) and occurred regularly thereafter. Five other mosquito species occasionally occupied some tree holes. However, because these species rarely occurred, they are omitted from our analyses. Two of the five common species, T. rutilus and C. appendiculata, have predatory larvae (Table A2 [Appendix]). The remaining three species, Ochlerotatus triseriatus, A. albopictus, and Orthopodomyia signifera, are primarily filter-feeders and browsers. Pairwise interactions between most of the five common species have been documented, as summarized in Table A2 (Appendix).
Expectations Based on the Influence of Patch Conditions on Mosquitoes
Metacommunity models raise the question of whether heterogeneity among patches in physical conditions influences species’ demography and interactions within patches. Neutral and patch dynamics models assume that habitat does not cause differences in demography, whereas habitat-specific demography is implicit in species-sorting and mass effect models.
A variety of evidence from other study systems suggests that between-patch variation in conditions is likely to influence mosquito communities. Tree hole size, water volume, and drought susceptibility vary considerably on relatively small spatial scales (Bradshaw and Holzapfel 1988, Kitching 2000, Paradise 2004) and can influence mean biomass (e.g., Bradshaw and Holzapfel 1983), species richness (e.g., Paradise and Dunson 1998), and density (e.g., Bradshaw and Holzapfel 1988, Paradise and Dunson 1998, Paradise 2004) of mosquito larvae. Studies have also shown high variation in nutrient concentrations among tree holes (e.g., Walker et al. 1991, Kitching 2000), which can influence microbial abundance (Walker et al. 1991) and mosquito growth, development, survivorship, and richness (e.g., Walker et al. 1991, Paradise and Dunson 1998). Larger quantity and higher quality of leaf detritus may also increase mosquito growth and productivity (Carpenter 1983, Walker et al. 1991).
Beyond general differences in size, resources, and water quality, tree holes also differ in their ability to retain water. Tree hole drying, or drought, forces the system to undergo frequent extinction and recolonization of populations. In such systems, differences in the frequency of drought among tree holes and differences in the ability of species to colonize recently filled holes following drought can be important to community dynamics. Because Ochlerotatus triseriatus and A. albopictus have drought-resistant eggs (Lounibos et al. 2001), these species can hatch immediately upon addition of water following a drought. The other species common in this system must locate the tree hole and oviposit, resulting in a delay in their presence in the community. Consequently, Ochlerotatus triseriatus may occur more often in smaller, more ephemeral holes, while T. rutilus and Orthopodomyia signifera may occur more often in larger, more permanent holes (Bradshaw and Holzapfel 1988). In our system, such differences are borne out by the representative annual cycle shown in Fig. A2 (Appendix) for a representative year (2000) in tree hole 2. These patterns are explored quantitatively in the next section.
Metacommunity Characteristics
Relationships between tree hole volume and populations
The tree holes studied here varied in volume and the number of days they contained water before drying up (‘‘hydroperiod’’; Table A1 [Appendix]). We used these data to examine the relationship between density and volume, presence and volume, and differences in species’ colonization ability and occupancy in relation to the volume and hydroperiod of tree holes.
The extrinsic effects of habitat variables on community dynamics were examined using randomization tests to account for spatial and temporal autocorrelation and logistic regressions of presence vs. volume for each species. The randomization test consisted of linear regression of density (per milliliter) vs. volume (in milliliters) with significance and confidence limits calculated using 10 000 randomizations (Manly 1991) using Rundom Projects 2.0 LITE (P. Jadwiszczak, unpublished computer program). The ln-transformed inverse of density (ln(density)−1) was used to linearize the relationship and zeros were removed. For the logistic regressions of presence vs. volume, only sample dates when the tree holes contained water were used. Because of spatial and temporal correlation in the data we regard logistic regression as an approximate test, but the best available to us, and we interpret it cautiously.
Tree hole volume accounted for 1–39% of variance in density (R2= 0.01–0.39) and was able to predict species’ presence in 60–75% of cases (Table 2). All species showed a positive relationship between ln(density)−1 and volume. All species except A. albopictus showed a negative relationship between presence and volume. These results are consistent with species sorting, however, not to the extent that species had permanent refuges in particular tree holes.
Table 2.
Results of linear regression with randomization of ln (density)−1 vs. volume (in milliliters) with 10 000 randomizations/ bootstrap samples and of logistic regression of presence vs. volume (in milliliters).
| Presence vs. volume
|
|||||||||
|---|---|---|---|---|---|---|---|---|---|
| ln(density)−1 vs. volume
|
|||||||||
| Species | N | Slope | SE | R2 | P | Slope | SE | P | Percentage concordant with model |
| T. rut | 1122 | 0.00005 | 0.000002 | 0.39 | <0.0001 | −0.0013 | 0.00009 | <0.0001 | 71.4 |
| C. app | 1356 | 0.00017 | 0.00004 | 0.01 | <0.0001 | −0.001 | 0.00009 | <0.0001 | 65.6 |
| O. sig | 289 | 0.00005 | 0.000007 | 0.13 | <0.0001 | −0.0015 | 0.0001 | <0.0001 | 74.9 |
| O. tris | 1506 | 0.0002 | 0.00006 | 0.01 | 0.002 | −0.0005 | 0.00008 | <0.0001 | 60.5 |
| A. alb | 483 | 0.0005 | 0.0002 | 0.02 | 0.003 | 0.0014 | 0.0002 | <0.0001 | 60.9 |
Notes: Only samples in which a tree hole contained water were included. Because of spatial and temporal correlation, the P values and SE estimates from the logistic regressions may be biased and are presented only as a guide to the explanatory power of tree hole volume. Abbreviations are: T. rut, Toxorhynchites rutilus; C. app, Corethrella appendiculata; O. sig, Orthopodomyia signifera; O. tris, Ochlerotatus triseriatus; and A. alb, Aedes albopictus.
Spatial synchrony
Lag-zero cross-correlations of ln-transformed densities (per milliliter; cross-correlations performed in Statistica 6.1; StatSoft, Tulsa, Oklahoma, USA) examined whether a species’ population fluctuations were synchronous across tree hole pairs using only periods when both tree holes were occupied. Synchrony in occupancy (with zeros for absence and ones for presence) was also calculated, reflecting that changes in occupancy and population density may have different causes. Less-than-perfect synchrony would increase the potential for movement to alter local community dynamics, providing that tree holes are not so different that species cannot survive there. Since we have a lagzero cross-correlation value for each tree hole pair and multiple tree hole pairs, we calculated bootstrap means for each species (which allow for nonindependence of tree hole pairs). Randomized linear regressions with 10 000 randomizations using Rundom Projects 2.0 LITE (P. Jadwiszczak, unpublished computer program) were used to examine the relationship between degree of synchrony of densities and distance between tree holes. Regressions were performed for each species individually and for all species combined.
All five species had mean cross-correlations of density and occupancy that were significantly different from +1 (P < 0.0001 in all cases), indicating substantial independence among dynamics in different tree holes (Fig. 1). Mean cross-correlations were significantly different from zero for T. rutilus, A. albopictus, and Ochlerotatus triseriatus (P < 0.05 in all cases), but not for C. appendiculata and Orthopodomyia signifera (P > 0.06 for both). There was no significant relationship between degree of synchrony and interpatch distance (distance ranged from <1 m for two tree holes on the same tree to 278 m, mean = 130 m), neither for individual species nor for all species combined (P > 0.05 in all cases). This indicates that either heterogeneity among patches had strong effects on dynamics or that there was little synchronizing movement among patches. This result could be consistent with any of the four metacommunity models, whereas high synchrony would have ruled out some models.
Fig. 1.
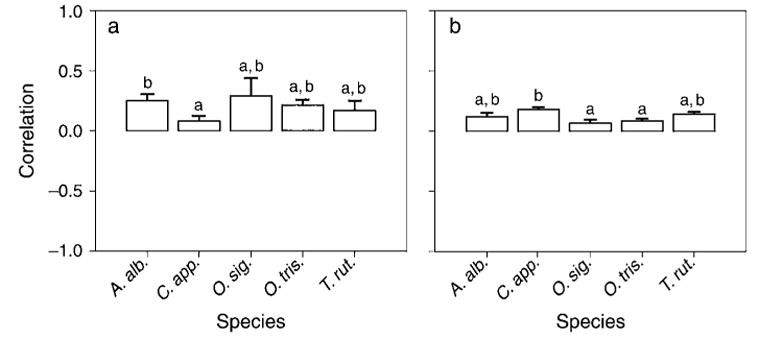
Mean lag-zero cross-correlations (presented as+1 bootstrapped standard error) of (a) ln-transformed density (no./mL) and (b) presence for a given species among tree holes and results of t tests that group species according to the differences among mean lag-zero cross-correlations. Species with the same letter do not differ at P < 0.05. Abbreviations are: A. alb, Aedes albopictus; C. app., Corethrella appendiculata; O. sig., Orthopodomyia signifera; O. tris., Ochlerotatus triseriatus; and T. rut., Toxorhynchites rutilus. Tree holes were located in a 4-ha woodland on the grounds of the University of Florida’s Florida Medical Entomology Laboratory in Vero Beach, Florida, USA.
Life history trade-offs: colonization and occupancy
As a surrogate for competitive or dispersal abilities, we examined occupancy and colonization. Occupancy was the proportion of water-filled tree holes occupied by a species on each census date (Fig. A3 [Appendix]), and colonization was the appearance of larvae after a dry tree hole became water-filled. Colonization and occupancy data for A. albopictus were calculated only after its invasion in 1991. Colonization could occur both from the immediate hatching of desiccation-resistant eggs remaining in the tree hole (for Ochlerotatus triseriatus and A. albopictus only) and by the deposition of new eggs into the tree hole (for all species). For each species, we calculated bootstrap means with 1000 resamplings for occupancy and colonization using S-PLUS 6.1 statistical software (Insightful Corporation, Seattle, Washington, USA). Colonization values were pooled across all tree holes. Randomized linear regression was used to test whether the ability of a species to occupy a tree hole was related to the volume or frequency of drought (using 10 000 randomizations in Rundom Projects 2.0 LITE; P. Jadwiszczak, unpublished computer program). We regressed occupancy (arcsine square-root transformed) for each species on mean volume and hydroperiod.
Ochlerotatus triseriatus and A. albopictus, which have drought-resistant eggs that can hatch immediately upon tree hole flooding, colonized tree holes faster than the other species (Fig. 2). However, A. albopictus had a much lower occupancy than Ochlerotatus triseriatus (Fig. 2). This may be because A. albopictus is a more recent invader and is thus present at lower densities or because it prefers more disturbed habitats than Ochlerotatus triseriatus (Lounibos et al. 2001). The proportion of tree holes occupied by Ochlerotatus triseriatus was negatively related to the mean hydroperiod of tree holes (Table 3), and A. albopictus occupancy was weakly negatively related to mean tree hole volume (P = 0.08; Table 3). These relationships suggest that A. albopictus and Ochlerotatus triseriatus tended to occupy a higher proportion of smaller, more ephemeral tree holes while the other species occupy larger, more stable tree holes. Consistent with this, T. rutilus occupancy was positively related to mean tree hole volume and hydroperiod (P < 0.05 for both; Table 3), and Orthopodomyia signifera occupancy was weakly positively related to mean volume (P = 0.1; Table 3). These results support the conclusions of Bradshaw and Holzapfel (1988) that Ochlerotatus triseriatus occurred more often in smaller, more ephemeral holes, while T. rutilus and Orthopodomyia signifera occurred more often in larger, more permanent holes. The occupancy/colonization trade-off (Fig. 2) is broadly similar to the competition/colonization trade-off and its occurrence is consistent with the preexisting evidence for strong competitive interactions in this system (see Table A2 [Appendix]). The presence and dynamical effects of this trade-off are inconsistent with neutral theory, they are a necessary part of dispersal-limited patch dynamics, and they are not specified by the species-sorting and mass effect models (Table 1).
Fig. 2.
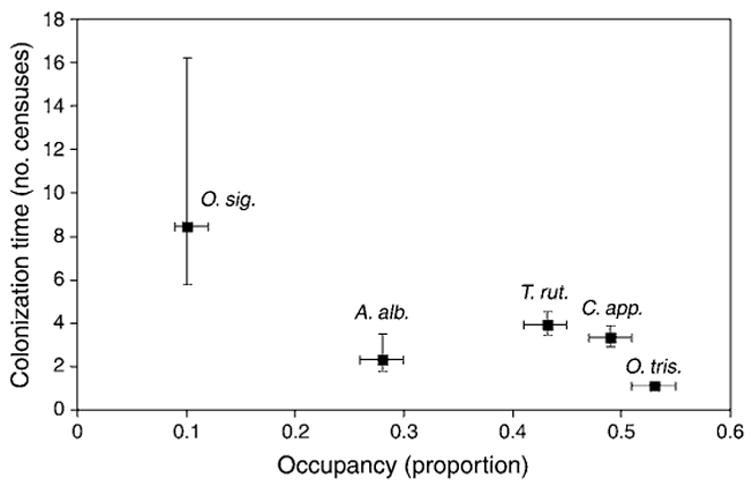
Mean colonization time (number of two-week censuses to colonization following drought) vs. mean occupancy (proportion of water-filled holes occupied during each census). Points are presented as means ± 1 SE in both x and y directions. The standard error for Ochlerotatus triseriatus along the y-axis is smaller than the width of the symbol. Standard errors come from parametric bootstrapping. Abbreviations are: A. alb, Aedes albopictus; C. app., Corethrella appendiculata; O. sig., Orthopodomyia signifera; O. tris., Ochlerotatus triseriatus; and T. rut., Toxorhynchites rutilus.
Table 3.
Results for the randomized regressions (10 000 randomizations) of the proportion of water-filled tree holes occupied (arcsine square-root transformed) vs. mean volume, and the proportion of water-filled tree holes occupied vs. mean hydroperiod. Species
| Volume
|
Hydroperiod
|
||||||||
|---|---|---|---|---|---|---|---|---|---|
| Species | Slope | SE | R2 | P | N | Slope | SE | R2 | P |
| T. rut | 0.0004 | 0.0002 | 0.4 | 0.03 | 12 | 0.007 | 0.003 | 0.38 | 0.03 |
| C. app | 0.0002 | 0.0002 | 0.07 | 0.42 | 12 | −0.0002 | 0.0041 | 0.0002 | 0.97 |
| O. sig | 0.0004 | 0.0002 | 0.24 | 0.1 | 12 | 0.003 | 0.004 | 0.05 | 0.49 |
| O. tris | −0.00002 | 0.0003 | 0.0004 | 0.94 | 12 | −0.013 | 0.005 | 0.42 | 0.02 |
| A. alb | −0.0005 | 0.0002 | 0.49 | 0.08 | 7 | −0.0014 | 0.0038 | 0.02 | 0.74 |
Notes: Hydroperiod was the number of sampling dates during which a tree hole held water continuously. Abbreviations are: T. rut, Toxorhynchites rutilus; C. app, Corethrella appendiculata; O. sig, Orthopodomyia signifera; O. tris, Ochlerotatus triseriatus; and A. alb, Aedes albopictus.
Species composition and abundance
Variation in community composition across space and time was compared using spatial and temporal turnover in composition and spatial and temporal components of variation in species density. We used sample dates that were four months apart to obtain separate generations in all except rare cases. Temporal turnover was calculated as (Cobs + Eobs)/(Si + Si+1) × 100, where Cobs is the number of species present at time i + 1 but not at time i, Eobs is the number of species absent at time i + 1 but present at time i, and Si is the number of species in a tree hole at time i (Davies et al. 2001). From the above, it follows that spatial turnover is (Aobs+Bobs)/Sa+Sb × 100, where Aobs is the number of species present in tree hole A but not tree hole B at a point in time, Bobs is the number of species present in tree hole B but not in tree hole A, and Sa is the number of species in tree hole A, and Sb is the number of species in tree hole B. Temporal turnover was calculated for as many values as there were consecutive pairs of data points through time for each tree hole. Spatial turnover was calculated for all tree hole pairs that contained water and at least one species at the same time. We then computed bootstrap means (1000 resamplings) of temporal turnover values for each tree hole and of spatial turnover values for each tree hole pair using S-PLUS 6.1 (Insightful Corporation). We then performed randomized regressions (10 000 randomizations) of temporal turnover vs. mean hydroperiod and mean spatial turnover vs. distance between tree hole pairs using Rundom Projects 2.0 LITE (P. Jadwiszczak, unpublished computer program). Spatial and temporal components of variation in species density (ln-transformed) were obtained using proc GLM in SAS 9.1 (SAS Institute, Cary, North Carolina, USA) using the same data used for the turnover analyses (sample dates separated by four months).
Mean temporal turnover in species composition was 62.1%/4 mo (range 35.6–92.6%). Mean spatial turnover was 75.2% (range 33.3–100%). Hence, both spatial and temporal turnover were substantial, which is consistent with neutral and patch dynamics models, but not species-sorting and mass effect models. There was no relationship between spatial turnover and distance (R2= 0.004, slope= 0.02, P value for slope= 0.70) or between temporal turnover and mean hydroperiod (R2 = 0.11, slope = −0.27, P value for slope = 0.29). Hence, like individual species dynamics, community dynamics did not appear to be explicitly spatially structured within the study area. If mass effects operate, they do so at a larger scale than that measured or their signal was overwhelmed by local environmental variation.
Most species also showed substantial spatial and temporal variation in density (Table 4). Overall 59–92% of variance in density was accounted for by space and time. Ochlerotatus signifera showed little spatial variation but a lot of temporal variation in density (84% of variance in density was accounted for by time). This is also consistent with the higher spatial synchrony in density for this species than other species (Fig. 1a). For T. rutilus, C. appendiculata, and Ochlerotatus triseriatus, temporal variation in density was greater than spatial variation. Aedes albopictus showed approximately equal spatial and temporal variation in density. The substantial spatiotemporal turnover in composition and density is more consistent with the patch dynamics and neutral models than with the species-sorting and mass effects models (Table 1).
Table 4.
Spatial and temporal variation in density.
| Species | No. observations | Space (%) | Time (%) | Sum (%) |
|---|---|---|---|---|
| T. rut | 124 | 27.80 | 36.90 | 64.70 |
| C. app | 149 | 21.20 | 44.90 | 66.10 |
| O. sig | 27 | 7.40 | 84.40 | 91.80 |
| O. tris | 177 | 19.30 | 39.90 | 59.30 |
| A. alb | 54 | 38.50 | 34.60 | 73.10 |
Notes: Percentage attributed to space and time were calculated as type III sums of squares for space (or time)/total sums of squares. Abbreviations are: T. rut, Toxorhynchites rutilus; C. app, Corethrella appendiculata; O. sig, Orthopodomyia signifera; O. tris, Ochlerotatus triseriatus; and A. alb, Aedes albopictus.
Conclusions
Dynamics did not fit the expectations of any single metacommunity perspective (Table 1). Correlations of density and occupancy with habitat variables (volume, drought) were substantial, which was consistent with the species-sorting model, but not to the extent that species had permanent refuges in particular tree holes. Expectations of the patch dynamics model, on the other hand, were supported by asynchrony in dynamics in different tree holes, substantial species turnover in space and time, and the presence of an occupancy/colonization trade-off. No evidence of dispersal (distance) altering dynamics was found within the area (spatial scale) studied. These results indicate that mosquito communities were structured by life history trade-offs, habitat (spatial niche) differences, and repeated colonization and extinction of at least those species that lack drought resistant life stages and that the volume of tree holes had important effects on species composition and abundance.
Comparison with existing studies of the relevance of the four models shows that there is much variation in the dynamics shown by different systems. Cottenie and de Meester (2005) and Urban (2004) found strong species sorting despite substantial interpatch dispersal. Cottenie (2005) surveyed species composition in 158 published community data sets and found that habitat variables (species sorting or mass effects) accounted for more variation than distance (dispersal) between communities. Some degree of species sorting was also found in five other studies (Holyoak et al. 2005), spanning pitcher plant inquilines, microinvertebrates on moss patches, plant–butterfly–parasitoid metacommunities, rock pool invertebrates, and terrestrial beetles. In Urban’s (2004) study, patch (pond) duration also played an important part in determining species composition, a feature shared with pitcher plant communities (e.g., Miller and Kneitel 2005) and the present study system. These empirical studies caution against using a single metacommunity characteristic (e.g., association with habitat variables, dispersal rates, patterns of synchrony) to identify the kinds of dynamics that are present in a community.
While metacommunity theories help to guide empirical investigations of spatially structured communities, the models currently lack specific predictions that can be tested in natural systems. For example, most metacommunity models do not specify the nature of species interactions or the manner in which they are influenced by dispersal or patch conditions. In addition, it is not clear how results depend on the scale of study or on the type of data available. For example, while no differences in synchrony were found at the spatial scale of our study, they may be present at larger spatial scales. This may result directly from the complex life history of the organism and the environments inhabited by different life stages or simply because different patterns emerge at different spatial scales. The long length of the time series used here could also lead to higher probability of picking up temporal-variance components compared to spatial-variance components (a temporal bias), which could influence conclusions about the types of dynamics the community experiences. Thus, before metacommunity theory can be empirically tested, much work is needed to determine the dynamical behavior and specific predictions of metacommunity models. While quantitative models are available for competitive metacommunities (reviewed by Amarasekare et al. 2004), such models are not available for spatial food webs.
Our results demonstrate that the long-term dynamics of natural systems can show features of multiple metacommunity models. This suggests that one model cannot fully capture the dynamics of natural systems. However, each model may have assumptions and properties that are relevant in natural systems. An important next step for metacommunity studies is to modify and synthesize these models so that they help identify under what conditions communities will exhibit the characteristics predicted by each model. Detailed studies of the dynamical behavior of the current models would represent great progress towards this end and would also provide specific predictions that can be tested in the field.
Supplementary Material
Tables and figures reporting tree-hole characteristics and sampling periods, pairwise inter-specific interactions, average densities of species over time, a typical annual cycle of tree-hole characteristics, and the proportion of water-filled tree holes occupied by each species over time (Ecological Archives E087-155-A1). ALICIA M. ELLIS ET AL. 2590 Ecology, Vol. 87, No. 10
Acknowledgments
M. Holyoak was supported by NSF DEB-0213026 and DEB-0414465, A. M. Ellis by NSF 02-09736 to Mark A. McPeek, and L. P. Lounibos by NIH R01 AI044793. We thank Richard Escher for many years of faithful tree hole censuses.
Literature Cited
- Amarasekare P, Hoopes MF, Mouquet N, Holyoak M. Mechanisms of coexistence in competitive metacommunities. American Naturalist. 2004;164:310–326. doi: 10.1086/422858. [DOI] [PubMed] [Google Scholar]
- Barrera R. Species concurrence and the structure of a community of aquatic insects in tree holes. Journal of Vector Ecology. 1996;21:66–80. [Google Scholar]
- Belkin JN. The mosquitoes of the South Pacific (Diptera: Culicidae) I. II. University of California Press; Berkeley, California, USA: 1962. [Google Scholar]
- Bell G. Neutral macroecology. Science (Washington DC) 2001;293:2413–2418. doi: 10.1126/science.293.5539.2413. [DOI] [PubMed] [Google Scholar]
- Bradshaw WE, Holzapfel CM. Predator mediated, non-equilibrium coexistence of tree-hole mosquitoes in southeastern North America. Oecologia. 1983;57:239–256. doi: 10.1007/BF00379586. [DOI] [PubMed] [Google Scholar]
- Bradshaw WE, Holzapfel CM. Drought and the organization of tree-hole mosquito communities. Oecologia. 1988;74:507–514. doi: 10.1007/BF00380047. [DOI] [PubMed] [Google Scholar]
- Carpenter SR. Resource limitation of larval tree hole mosquitoes subsisting on beech detritus. Ecology. 1983;64:219–223. [Google Scholar]
- Chase JM, Amarasekare P, Cottenie K, Gonzalez A, Holt RD, Holyoak M, Hoopes MF, Leibold MA, Loreau M, Mouquet N, Shurin JB, Tilman D. Competing theories for competitive metacommunities. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 335–354. [Google Scholar]
- Chave J. Neutral theory and community ecology. Ecology Letters. 2004;7:241–253. [Google Scholar]
- Cottenie K. Integrating environmental and spatial processes in ecological community dynamics. Ecology Letters. 2005;8:1175–1182. doi: 10.1111/j.1461-0248.2005.00820.x. [DOI] [PubMed] [Google Scholar]
- Cottenie K, De Meester L. Local interactions and local dispersal in a zooplankton metacommunity. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 89–211. [Google Scholar]
- Davies K, Melbourne B, Margules C. Effects of within- and between-patch processes on community dynamics in a fragmentation experiment. Ecology. 2001;82:1830–1846. [Google Scholar]
- Gilbert B, Lechowicz MJ. Neutrality, niches, and dispersal in a temperate forest understory. Proceedings of the National Academy of Sciences (USA) 2004;101:7651–7656. doi: 10.1073/pnas.0400814101. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Gonzalez A. Local and regional community dynamics in fragmented landscapes: insights from a bryophyte-based natural microcosm. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 146–179. [Google Scholar]
- Griswold MW, Lounibos LP. Competitive outcomes of aquatic container Diptera depend on predation and resource levels. Annals of the Entomological Society of America. 2005;98:673–681. doi: 10.1603/0013-8746(2005)098[0673:COOACD]2.0.CO;2. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Hanski IA, Gilpin ME. Metapopulation biology: ecology, genetics, and evolution. Academic Press; San Diego, California, USA: 1997. [Google Scholar]
- Harrison S, Taylor AD. Empirical evidence for metapopulation dynamics: a critical review. In: Hanski I, Gilpin ME, editors. Metapopulation dynamics: ecology, genetics, and evolution. Academic Press; San Diego, California, USA: 1997. pp. 27–42. [Google Scholar]
- Holyoak M. Habitat subdivision causes changes in food web structure. Ecology Letters. 2000;3:509–515. [Google Scholar]
- Holyoak M, Leibold MA, Holt RD. Coda. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 491–492. [Google Scholar]
- Hubbell SP. The unified neutral theory of biodiversity and biogeography. Princeton University Press; Princeton, New Jersey, USA: 2001. [Google Scholar]
- Kitching RL. An ecological study of water-filled treeholes and their position in the woodland ecosystem. Journal of Animal Ecology. 1971;40:281–302. [Google Scholar]
- Kitching RL. Community structure in water-filled treeholes in Europe and Australia—comparisons and speculations. In: Frank JH, Lounibos LP, editors. Phytotelmata: terrestrial plants as hosts for aquatic insect communities. Plexus, Medford; New Jersey, USA: 1983. pp. 205–222. [Google Scholar]
- Kitching RL. Food webs and container habitats: the natural history and ecology of phytotelmata. Cambridge University Press; Cambridge, UK: 2000. [Google Scholar]
- Kolasa J, Romanuk TN. Assembly of unequals in the unequal world of a rock pool metacommunity. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 212–232. [Google Scholar]
- Law R, Morton RD. Alternative permanent states of ecological communities. Ecology. 1993;74:1347–1361. [Google Scholar]
- Leibold MA, Holyoak M, Mouquet N, Amarasekare P, Chase JM, Hoopes MF, Holt RD, Shurin JB, Law R, Tilman D, Loreau M, Gonzalez A. The metacommunity concept: a framework for multi-scale community ecology. Ecology Letters. 2004;7:601–613. [Google Scholar]
- Levine JM, Rees M. Coexistence and relative abundance in annual plant assemblages: the roles of competition and colonization. American Naturalist. 2002;160:452–467. doi: 10.1086/342073. [DOI] [PubMed] [Google Scholar]
- Lounibos LP. Habitat segregation among African treehole mosquitoes. Ecological Entomology. 1981;6:129–154. [Google Scholar]
- Lounibos LP. Interactions influencing production of treehole mosquitoes in South Florida. In: Lounibos LP, Rey JR, Frank JH, editors. Ecology of mosquitoes: proceedings of a workshop. Florida Medical Entomology Laboratory; Vero Beach, Florida, USA: 1985. pp. 65–78. [Google Scholar]
- Lounibos LP, O’Meara GF, Escher RL, Nishimura N, Cutwa M, Nelson T, Campos RE, Juliano SA. Testing predictions of displacement of native Aedes by the invasive Asian tiger mosquito Aedes albopictus in Florida, USA. Biological Invasions. 2001;3:151–166. [Google Scholar]
- MacArthur RH, Wilson EO. The theory of island biogeography. Princeton University Press; Princeton, New Jersey, USA: 1967. [Google Scholar]
- Manly BF. Randomization and Monte Carlo methods in biology. Chapman and Hall; London, UK: 1991. [Google Scholar]
- McGill BJ, Maurer BA, Weiser MD. Empirical evaluation of the neutral theory. Ecology. 2006;87:1411–1423. doi: 10.1890/0012-9658(2006)87[1411:eeont]2.0.co;2. [DOI] [PubMed] [Google Scholar]
- Miller TE, Kneitel JM. Inquiline communities in pitcher plants as a prototypical metacommunity. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 122–145. [Google Scholar]
- Mouquet N, Hoopes MF, Amarasekare P. The world is patchy and heterogeneous! Trade-off and source sink dynamics in competitive metacommunities. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 237–262. [Google Scholar]
- Mouquet N, Ledley P, Meriget J, Loreau M. Immigration and local competition on herbaceous plant communities: a three-year seed-sowing experiment. Oikos. 2004;104:77–90. [Google Scholar]
- Paradise CJ. Relationship of water and leaf litter variability to insects inhabiting treeholes. Journal of the North American Benthological Society. 2004;23:793–805. [Google Scholar]
- Paradise CJ, Dunson WA. Relationship of atmospheric deposition to the water chemistry and biota of treehole habitats. Environmental Toxicology and Chemistry. 1998;17:362–368. [Google Scholar]
- Pulliam R. Sources, sinks and population regulation. American Naturalist. 1988;132:652–661. [Google Scholar]
- Röhnert U. Wassererfüllte Baumhöhlen und ihre Besiedlung. Ein Beitrag zur Fauna dendrolimnetica Archiv für Hydrobiologie. 1950;44:472–516. [Google Scholar]
- Roxburgh SH, Shea K, Wilson JB. The intermediate disturbance hypothesis: patch dynamics and mechanisms of species coexistence. Ecology. 2004;85:359–371. [Google Scholar]
- Sota T, Mogi M, Hayamizu E. Habitat stability and larval mosquito community in treeholes and other containers on a temperate island. Researches in Population Ecology. 1994;36:93–104. [Google Scholar]
- Teng HJ, Apperson CS. Development and survival of immature Aedes albopictus and Aedes triseriatus (Diptera : Culicidae) in the laboratory: effects of density, food, and competition on response to temperature. Journal of Medical Entomology. 2000;37:40–52. doi: 10.1603/0022-2585-37.1.40. [DOI] [PubMed] [Google Scholar]
- Urban MC. Disturbance heterogeneity determines freshwater metacommunity structure. Ecology. 2004;85:2971–2978. [Google Scholar]
- van Nouhuys S, Hanski I. Metacommunities of butterflies, their host plants, and their parasitoids. In: Holyoak M, Leibold MA, Holt RD, editors. Metacommunities: spatial dynamics and ecological communities. University of Chicago Press; Chicago, Illinois, USA: 2005. pp. 99–121. [Google Scholar]
- Walker ED, Lawson DL, Merritt RW, Morgan WT, Klug MJ. Nutrient dynamics, bacterial populations and mosquito productivity in tree hole ecosystems and microcosms. Ecology. 1991;72:1529–1546. [Google Scholar]
- Woodward DL, Cowell A, Anderson N. The aquatic insect communities of tree holes in northern California woodlands. Bulletin of the Society of Vector Ecology. 1988;13:221–234. [Google Scholar]
- Yanoviak SP. The macrofauna of water-filled tree holes on Barro Colorado Island, Panama. Biotropica. 2001;33:110–120. [Google Scholar]
- Yu DW, Wilson HB, Frederickson ME, Palomino W, De la Colina R, Edwards DP, Balareso AA. Experimental demonstration of species coexistence enabled by dispersal limitation. Journal of Animal Ecology. 2004;73:1102– 1114. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Tables and figures reporting tree-hole characteristics and sampling periods, pairwise inter-specific interactions, average densities of species over time, a typical annual cycle of tree-hole characteristics, and the proportion of water-filled tree holes occupied by each species over time (Ecological Archives E087-155-A1). ALICIA M. ELLIS ET AL. 2590 Ecology, Vol. 87, No. 10


