Structure and function of the feed-forward loop network motif

Abstract

Engineered systems are often built of recurring circuit modules that carry out key functions. Transcription networks that regulate the responses of living cells were recently found to obey similar principles: they contain several biochemical wiring patterns, termed network motifs, which recur throughout the network. One of these motifs is the feed-forward loop (FFL). The FFL, a three-gene pattern, is composed of two input transcription factors, one of which regulates the other, both jointly regulating a target gene. The FFL has eight possible structural types, because each of the three interactions in the FFL can be activating or repressing. Here, we theoretically analyze the functions of these eight structural types. We find that four of the FFL types, termed incoherent FFLs, act as sign-sensitive accelerators: they speed up the response time of the target gene expression following stimulus steps in one direction (e.g., off to on) but not in the other direction (on to off). The other four types, coherent FFLs, act as sign-sensitive delays. We find that some FFL types appear in transcription network databases much more frequently than others. In some cases, the rare FFL types have reduced functionality (responding to only one of their two input stimuli), which may partially explain why they are selected against. Additional features, such as pulse generation and cooperativity, are discussed. This study defines the function of one of the most significant recurring circuit elements in transcription networks.

Cells contain networks of biochemical transcription interactions. These networks have evolved to perform information-processing functions (1, 2). The inputs to the network, such as external nutrients and stresses, affect the activity of transcription factor proteins. The transcription factors bind regulatory regions of specific genes and activate or repress their transcription. As a result, cell processes are modulated to fit the environmental conditions. Transcription networks can be described as directed graphs, in which the nodes are genes (3–12). Directed edges represent transcription interactions, where a transcription factor encoded by one gene modulates the transcription rate of the second gene.

It is of interest to understand the dynamic behavior of transcription networks (2, 3, 5, 7–10). It was recently found that these networks contain significantly recurring wiring patterns termed “network motifs” (6, 11, 12). Network motifs are patterns that occur in the network far more often than in randomized networks with the same degree sequence (6, 11). The transcription networks of the bacterium Escherichia coli (6, 11) and the yeast Saccharomyces cerevisiae (11, 12) were found to contain the same small set of highly significant motifs. The significance of these structures raises the question of whether they have specific information-processing roles in the network. If they do, they might be used to understand the network dynamics in terms of elementary computational building blocks.

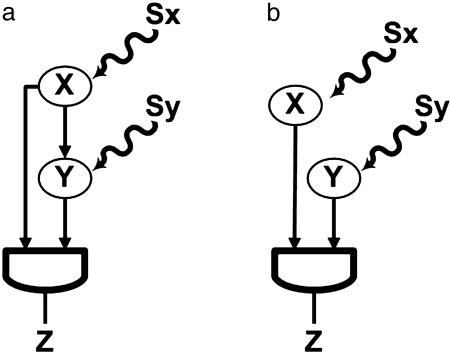
One of the most significant network motifs in both E. coli and yeast is the feed-forward loop (FFL) (6, 11). The FFL is composed of a transcription factor X, which regulates a second transcription factor Y (Fig 1a). X and Y both bind the regulatory region of target gene Z and jointly modulate its transcription rate. The FFL has two input signals, the inducers, Sx and Sy, which are small molecules, protein partners, or covalent modifications that activate or inhibit the transcriptional activity of X and Y (Fig. 1a). The FFL has three transcription interactions. Each of these can be either positive (activation) or negative (repression). There are therefore eight possible structural configurations of activator and repressor interactions (6) (Tables 1 and 2). Four of these configurations are termed “coherent” (Table 1): the sign of the direct regulation path (from X to Z) is the same as the overall sign of the indirect regulation path (from X through Y to Z) (6). The other four structures are termed “incoherent” (Table 2): the signs of the direct and indirect regulation paths are opposite.

Fig. 1.

(a) FFL. Transcription factor X regulates transcription factor Y, and both jointly regulate Z. Sx and Sy are the inducers of X and Y, respectively. The action of X and Y is integrated at the Z promoter with a cis-regulatory input function (7, 14), such as AND or OR logic. (b) Simple regulation of Z by X and Y.

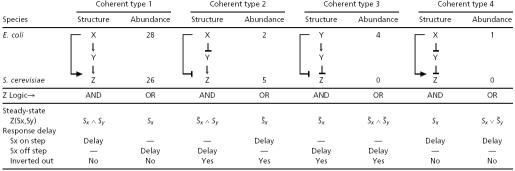
Table 1. Structure and function of the coherent FFL types, with AND- and OR-gates at the Z promoter.

Coherent FFL types and their abundance in transcription databases of E. coli and S. cerevisiae (6, 11). Z(Sx,Sy): Steady-state Z expression of coherent FFLs for the four combinations of Sx and Sy on and off levels (∧,∨,^- represent AND, OR, NOT). Response: Response delay of coherent FFLs to on and off S_x steps in the presence of Sy. —, not delayed. Inverted out means that Z goes off in response to Sx on step.

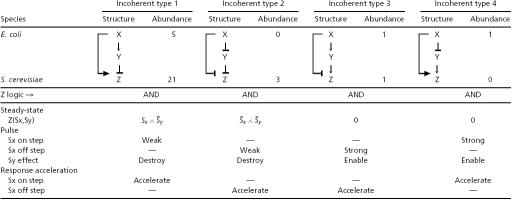
Table 2. Structure and function of the incoherent FFL types, with AND-gates at the Z promoter.

Incoherent FFL types and their abundance in transcription databases (6, 11). Z(Sx,Sy): Steady-state Z expression of incoherent FFL with no basal level of Y (∨, ^- represent AND, NOT). Pulse: Response to steps of Sx, in the presence of Sy, in FFLs with no basal activity, Sy effect on pulse: Enable, no pulse is created when Sy is off; Destroy, Z output is a low pulse when Sy is on, but is high and steady when Sy is off (Fig. 3). Response acceleration: Acceleration of response of and steady-state values of incoherent FFL with basal activity to on and off steps in the presence of Sy. —, not accelerated.

The effects of transcription factors X and Y are integrated at the promoter region of gene Z. The level of Z expression is modulated according to the concentrations of X and Y transcription factors bound to their inducers. This modulation is described by the cis-regulatory input function of gene Z (7, 13, 14). Common examples of cis-regulatory input functions include AND-like gates, in which both X and Y are needed to express Z, and OR-gate logic in which either X or Y is sufficient to express Z.

Here we use mathematical modeling to study the function of the eight FFL structural configurations, with AND- and OR-gate logic. This work extends our previous study that was limited to only one FFL type with three activators and AND logic (6). We find that incoherent FFLs can serve as a novel mechanism for accelerating the expression of the target genes. Both coherent and incoherent FFL behavior is sign sensitive: they accelerate or delay responses to stimulus steps, but only in one direction. The FFL functions are essentially the same with either AND- or OR-gates, but with reversed sign sensitivity. These results directly suggest experiments that can test the function of this network motif.

Materials and Methods

Equations for Gene Regulation Reactions. The active forms of X and Y are X* and Y*, respectively. For simplicity, we assume that Sx and Sy activate X and Y, and thus X* = X if Sx = 1, and X* = 0 if Sx = 0. Similarly, Y* = Y if Sy = 1, and Y* = 0 if Sy = 0. We assume constitutive production of X, X = 1. The concentrations of Y and Z are described by kinetic equations (6, 9, 15–19):

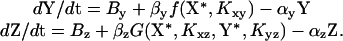The regulation function for an activator is f(u, K) = (u/K)^H/(1 + (u/K)^H), and for repressor f(u, K) = 1/(1 + (u/K)^H). The K_ij parameters are the activation or repression coefficient of gene j by transcription factor i. The gate function for an AND-gate is G_z = f(X*, K_xz)f(Y*, K_yz). For an OR-gate (with the two transcription factors competing for binding to the promoter region), G_z = fc(X*; K_xz, K_yz, Y*) + fc(Y*; K_yz, K_xz, X*), where for an activator fc(u; K_u, K_v, v) = (u/K)^H u/(1 + (u/K_u)^H + (v/K_v)^H), and for a repressor fc(u; K_u, K_v, v) = 1/(1 + (u/K_u)^H + (v/K_v)^H). (Other models for OR-gate, such as noncompetitive binding, showed the same qualitative results.) B_y and B_z are the basal transcription rates of Y and Z. α_z = α_deg + α_dil, where α_deg is the degradation rate and α_dil is the dilution rate of protein Z by cell division (17). If production stops at time t = 0, then Z decays as Z = Z(t = 0)exp(–α_zt), and protein Z reaches half of its initial concentration at time t = log (2)/α_z defined as the lifetime of protein Z. Similar definitions apply to the lifetime of Y. The simple regulation circuit is modeled by the same equation for Z, with Y constitutively expressed, Y = 1.

Here, we treat Sx and Sy as inducers. This treatment can be readily extended for the case where they are inhibitors, that is when Sx or Sy binding decreases the activity of the corresponding transcription factor. The conclusions are the same, with appropriate changes in the sign sensitivity of the FFL functions.

Parameters for Functional FFLs. Functional FFLs are those in which a saturating Sx signal causes a change in Y sufficient to cause significant change in Z. In nonfunctional FFLs, the regulation of Y by X does not significantly affect Z. Examples include cases where the basal level of Y, in the absence of Sx, is much larger than K_yz. Functional parameters, when Y is an activator, obey: f(Y_min, K_yz) ≪ f(Y_max, K_yz), where Y_min = B_y/α_y is the minimal Y level, and Y_max = (B_y + β_y)/α_y is the maximal Y level. Because these are inequalities, there is a broad range of parameters where FFLs are functional, for example, (α_yK_yz/β)^H ≪ 1, (α_yK_yz/B_y)^H ≫ 1. The qualitative results in this article apply for virtually all parameter choices within this functional domain. In this sense, the functions of the FFL are robust to variations in biochemical parameters (9, 19–22). In the figures, for FFL we use H = 2, β_y = β_z = 1, α_y = α_z = 1, B_y = B_z = 0; unless otherwise noted. For simple regulation we used Y = 1, H = 2, β_z = 1, α_z = 1, B_z = 0, and K_xz = 1. Steady-state logic was described as Boolean states (3) in Table 1 and 2, where 0 corresponds to basal level and 1 corresponds to high expression (within 2-fold of maximal expression). For incoherent FFLs, the steady-state logic with OR-gates has many intermediate levels and is not easily described as a Boolean output.

Analytical solutions can be obtained for step-like regulation (H ≫ 1). The delay of type 1 coherent FFL relative to simple regulation is 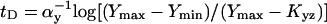. The response time for incoherent type 1 FFL in which Y maximally represses Z by a factor of F > 1 is 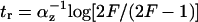, compared to 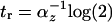for simple regulation.

Response Time. The response time is a measure of the time it takes a gene product to reach its physiologically determined steady-state level. We use the traditional definition of response time: the time to reach 50% of the steady-state level (9, 16, 17).

Transcription Network Databases. Literature-based databases of experimentally verified direct transcription interactions for E. coli (6) and S. cerevisiae (11) used were E. coli V1.1 and S. cerevisiae V1.3 available at www.weizmann.ac.il/mcb/UriAlon. FFLs were enumerated as described (6, 11). In E. coli, five FFLs have a “dual-regulation” transcription factor, which behaves as an activator in the presence of an inducer and as a repressor in the absence of an inducer. These were counted as activators in the present study. In the S. cerevisiae database, four apparent FFLs in which A regulates a complex BC, and both A and BC regulate B were removed.

Results

Some FFL Types Occur More Often than Others in E. coli and Yeast. We enumerated the appearances of each FFL type in databases of E. coli (6) and S. cerevisiae (11) transcription interactions (Tables 1 and 2). In both E. coli and yeast, we found that the type 1 coherent FFL, which has three activation interactions, is by far the most common coherent configuration. Incoherent FFLs are more common in yeast than in E. coli. In both organisms, the type 1 incoherent FFL, in which X activates a repressor of Z, is the most common incoherent configuration. The next most common configuration in yeast is type 2, which is somewhat more abundant than types 3 and 4.

The rest of the article is organized as follows. We analyze the behavior of the eight types of FFL by using kinetic equations for the protein levels (see Materials and Methods). For simplicity, we first assume that X and Y act in an AND-gate fashion to regulate Z and later discuss the OR-gate case. We begin with the four coherent FFL types and discuss their steady-state and kinetic behavior. Then we analyze the four incoherent FFLs. The results are summarized in Table 3.

Table 3. Summary of functions of the FFLs.

Function	Circuit class	Circuit types
Steady-state logic is sensitive to both Sx and Sy	Coherent and incoherent*	Types 1, 2 AND
		Types 3, 4 OR
Sign-sensitive delay upon Sx steps	Coherent	Types 1, 2, 3, 4
Sy-gated pulse generator upon Sx steps	Incoherent with no basal Y level	Types 3, 4 AND
		Types 1,2 OR
Sign-sensitive acceleration upon Sx steps	Incoherent with basal Y level	Types 1,2,3,4
Cooperativity enhancement for Sx input	Coherent	Type 1 AND

^*In incoherent FFL with basal level, Sy modulates Z between two nonzero levels.

Steady-State Behavior of Coherent FFL with AND-Gate: Only Types 1 and 2 Respond to Sy. Table 1 lists the steady-state behavior of the four coherent FFLs as a function of the two input stimuli, Sx and Sy. The steady-state of Z is evaluated for all four combinations of Sx = {1, 0} and Sy = {1, 0}, where 1 means saturating stimulus. We find that only types 1 and 2 coherent FFL (with AND-gate regulation) respond strongly to both Sx and Sy inputs (Table 1). Types 3 and 4 respond to Sx but not to Sy. To understand this, consider the type 4 coherent FFL. Here, X activates Z directly and also represses a repressor of Z. Z can be expressed only when Sx is present, because it requires active X. However, when Sx is present X acts to repress Y. As a result, the protein Y is not significantly expressed and therefore cannot interact with Sy to affect Z. When Sx is absent, Z cannot be expressed because the activator X is inactive, and even though protein Y is present, Sy has no effect on Z. Thus, Sy has no effect on Z in either the presence or absence of Sx. In contrast, in the type 1 coherent FFL X activates Y, and thus the protein Y is expressed when Sx is present and can interact with Sy to modulate Z expression.

Coherent FFL Kinetics: All Types Serve as Sign-Sensitive Delay Elements. We now consider the kinetic response of Z to step-like addition of the inducer Sx, in the presence of Sy. In the type 1 coherent FFL (with AND-gate regulation), for example, upon a step addition of Sx, Z expression begins only when the activator Y builds up to a sufficient concentration and crosses the activation threshold for Z (Fig. 2 Left). The speed of the response is characterized by the response time, the time that it takes Z to reach half of its steady-state level (17, 22). The response time after an on step of Sx is longer in the coherent FFL than for a corresponding simple regulation design (Fig. 1b) that has the same steady-state Z levels (Fig. 2, compare thick and medium curves to the thin curves). The magnitude of the delay can be tuned by the relationships between four biochemical parameters: lifetime of Y, lifetime of Z, the threshold K_yz, and the basal Y level (Fig. 2).

Fig. 2.

Kinetics of coherent type 1 (Left) and type 4 (Right) FFLs with AND regulatory logic, in response to on and off steps of Sx. Note that the delayed response to on steps of the FFLs (thick, medium lines) compared to a corresponding simple system (thin line). Note that FFLs can behave as simple regulation for nonfunctional parameter domains (see Materials and Methods). Simulation parameters: K_xz = K_xy = 0.1; for type 1, K_yz = {0.5, 5}; for type 4, K_yz = {0.6, 0.3}; all others are as stated in Materials and Methods.

The delay in the response is sign sensitive: the response to on steps is delayed, but the response to off steps of Sx is not delayed (Fig. 2). We term this behavior sign-sensitive delay. It is carried out by all four types of coherent FFLs. Type 2 and 3 coherent FFLs have reversed sign sensitivity: the response to off, but not on steps is delayed. The delay response is summarized in Table 1.

Steady-State Behavior of AND-Gate Incoherent FFL with No Basal Activity: Only Types 1 and 2 Respond to Sy. As in the case of AND-gate coherent FFLs, we find that only type 1 and 2 incoherent FFLs with AND-gate Z regulation are able to respond in steady state to both of their input stimuli, Sx and Sy. Types 3 and 4 have a constant steady state, which does not depend on either Sx or Sy values (Table 2).

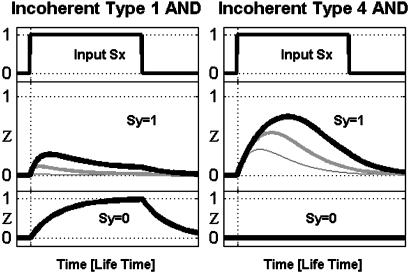
Kinetics of Incoherent FFL with No Basal Activity: Only Types 3 and 4 Are Good Pulsers. We now consider the kinetic response of the incoherent FFL to steps of Sx, in the extreme case where Y modulation by X leads to a strong effect on Z. In this case, the incoherent FFL functions as a pulser. For example, in the type 4 incoherent FFL, when Sx turns on, Z is first induced by the joint action of X and Y. Meanwhile, Y production is repressed by X and its levels drop, until Z production begins to decrease. Thus, in type 4 upon an on step of Sx, in the presence of Sy, Z levels first rise and then drop (Fig. 3 Right). A similar scenario holds for type 3. We find that type 1 and 2 incoherent FFLs (with AND gate Z regulation) are generally poor pulsers (Table 2 and Fig. 3a). The pulse amplitude is much smaller than the maximal level that can be reached by the circuit (the maximum level is reached in the absence of Sy, Fig. 3 Left Bottom). We find that type 1 and 2 incoherent FFLs are poor pulsers for all parameter values. In contrast, types 3 and 4 are good pulsers: For some biochemical parameters, the pulse reaches high amplitude relative to the maximal circuit response (Table 2 and Fig. 3 Right). The pulse occurs in the absence but not in the presence of Sy in the case of type 3 and 4 FFLs. Thus Sy is an enabling signal that can be used to allow or block the pulse (Fig. 3 Right).

Fig. 3.

Kinetics of incoherent type 1 (Left) and type 4 (Right) FFLs with AND regulatory logic and no basal activity of Y, in response to on and off steps of Sx. Note that type 4 FFLs can produce a strong pulse that is enabled by Sy. Type 1 can produce only a weak pulse when Sy = 1, and the pulse-like nature of the response is lost when Sy = 0. Simulation parameters: K_xz = K_xy = 0.1; for type 1, K_yz = {0.01, 0.1, 0.3}; for type 4, K_yz = {1, 0.3, 0.1} (thick, medium, thin lines); all others are as stated in Materials and Methods.

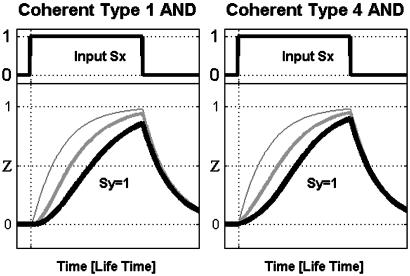
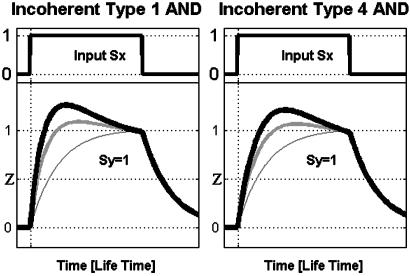
Kinetics of Incoherent FFL with Basal Y Activity: All Four Types Are Sign-Sensitive Accelerators. We now consider the incoherent FFL where the affect on Z by the indirect path through Y is not complete. In the type 1 incoherent FFL-AND, for example, upon a step addition of Sx, Z expression first rises, and then when Y levels build up, Z expression decreases to a nonzero level (Fig. 4 Left).

Fig. 4.

Kinetics of incoherent type 1 (Left) and type 4 (Right) FFLs with basal Y activity and AND regulatory logic, in response to on and off steps of Sx. Note that the response of the FFL to on steps (thick, medium lines) is faster than that of a corresponding simple system (thin line). Simulation parameters: for type 1, K_xz = 1, K_xy = 1, K_yz = 0.5, B_y = {0.5, 0.3}; for type 4, K_xz = 1, K_xy = 0.1, K_yz = 0.5, B_y = {0.45, 0.35}; all others are as stated in Materials and Methods.

We find that the response time of the incoherent FFL is smaller than the response time of a simple regulation system (Figs. 1b and 4). To make a mathematically controlled comparison (9), we compare a simple regulation system and an FFL that have the same steady-state Z expression upon addition of Sx (that is, with a Z promoter in the type 1 incoherent FFL that is stronger than in the corresponding simple regulation design, to compensate for the repressing effect of Y on the steady state). The simple regulation design has a response time of one lifetime (17) of protein Z (Fig. 4, thin line). The response time of the incoherent FFL is shorter (Fig. 4, thick and medium lines). The accelerated response occurs because Z initially rises quickly because of its relatively strong promoter and is then stopped by the repressor Y. Thus, in cases where speedy responses are needed, an incoherent FFL has an advantage over simple regulation with the same steady state.

The acceleration of the response is sign sensitive. We find that all four types of incoherent FFLs show sign-sensitive acceleration. In types 1 and 4, for example, the response is accelerated for on steps of Sx, but not for off steps (Fig. 4). Types 2 and 3 show sign-sensitive acceleration for off but not on steps of Sx (Table 1). The acceleration is tunable and controlled by the same parameters that control the delay in the coherent FFLs. For example, decreasing Y basal activity enhances the acceleration.

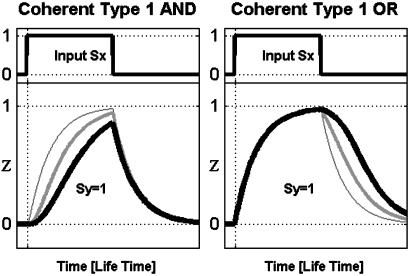
FFLs with OR-Gates Have the Same Functions but with Reversed Sign Sensitivity Relative to FFLs with AND-Gates. The discussion thus far considered FFLs in which X and Y act as an AND-gate to regulate gene Z. We now consider the effect of an OR-gate, where either X or Y is sufficient to express Z. We find that the FFLs with OR-gate regulation have the same sign-sensitive acceleration or delay functions, but with the sign sensitivity reversed relative to FFLs with AND gates. For example, the type 1 coherent FFL with an OR-gate shows a delayed response to off steps of Sx and a rapid response to on steps (Fig. 5 and Table 1).

Fig. 5.

Kinetics of coherent type 1 with AND (Left) and OR (Right) regulatory logic at Z promoter. Note that the AND FFL has delayed response to on steps, whereas OR FFL has delayed response to off steps. FFL: thick, medium lines; simple system: thin line. Simulation parameters: K_xz = 0.1, K_xy = 0.5; for AND, K_yz = {0.5,5}; for OR, K_yz = {0.7,0.3}; all others are as stated in Materials and Methods.

Incoherent FFLs that are poor pulsers with AND-gates, namely types 1 and 2, are better pulsers with OR-gates. Conversely, the good pulsers with AND-gates, types 3 and 4, are poor pulsers with OR-gates. The steady-state behavior of OR-gate FFLs is more intricate than that of AND-gate FFLs, because more intermediate states of expression are generally found.

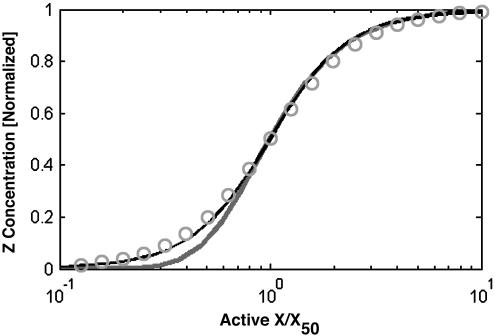
Only Coherent Type 1 AND-Gate FFL Shows Increased Apparent Cooperativity. We checked the effect of the FFL on the cooperativity of Z induction as a function of Sx (12), both analytically and by using simulations (Fig. 6). We found that only the type 1 AND-gate FFL shows a non-negligible increase of the apparent cooperativity. This effect occurs at low Sx levels, where the effective Hill coefficient for type 1 AND FFL is proportional to H_xz + H_yzH_xy, where H_ij is the Hill coefficient for gene j by transcription factor i. The other FFL types, including coherent type 1 OR-gate FFLs, showed no significant increase in cooperativity (some types even reduce apparent cooperativity). We note that simple transcription cascades are known to increase cooperativity (23).

Fig. 6.

Apparent cooperativity of steady-state Z response as a function of X activity. The graph shows the z(x) response curve for type 1 (thick line), type 4 (thin line) FFLs, and a simple regulation system (○), for H_zx = H_yx = H_zy = 2. Simulation parameters: α_i = 1, β_i = 1, K_ij = 1, B_i = 0. Type 1 coherent FFL (thick line) has an effective cooperativity of H_eff = H_xz + H_xy*H_yz, where H_ij is the Hill coefficient of the regulation reaction of protein j by protein i. Other coherent FFL types have H_eff = H_zx.

Discussion

We theoretically analyzed the functions of the eight FFL structural configurations. We find that the incoherent FFLs act as sign-sensitive accelerators: they provide a mechanism for speeding up the responses of the target genes. In addition, some incoherent FFL types can act as pulsers. Coherent FFLs act as sign-sensitive delays. These functions are carried out with either AND- or OR-gate regulation in the Z promoter, with reversed sign sensitivity. The results are summarized in Table 3.

Why Do Some FFL Configurations Occur More Often than Others in Transcription Networks? We find that in transcription databases of E. coli and yeast coherent type 1 FFLs occur far more often than the other three coherent types. Similarly, incoherent type 1 occurs much more often than the other incoherent types. Type 2 coherent and type 2 incoherent FFLs appear to be the next most selected configurations in yeast.

Can the difference in the abundance of the FFL types be simply explained by the relative numbers of repressor and activator interactions in the network? In the E. coli database, there are ≈2/3 activator and 1/3 repressor interactions (6). This finding would naively mean that there should be a total of ≈18 coherent FFLs of types 2–4, which is much more than observed. Similarly, in the yeast database, ≈80% of the interactions are acivators (11). Yet type 1 and types 3 and 4 incoherent FFLs occur in very different numbers, despite the fact that they have one repressor and two activator interactions. Thus, the difference in the frequencies of the FFL types is not simply explained by the relative abundances of repressor and activator interactions in the network.

Are all types of FFLs biologically feasible? In FFL types 3 and 4, the protein X has regulations of different signs for Y and Z (one repression and one activation), whereas in types 1 and 2 the regulation is of the same sign (both activation or both repression). It is well established that many transcription activators act to repress a subset of their downstream genes (24, 25). Hence, types 3 and 4 FFLs are, in principle, biologically feasible. What, then, might underlie their relative scarcity?

Our analysis suggests that AND-gate FFLs of types 3 and 4 have reduced functionality relative to types 1 and 2. Types 3 and 4 respond at most to one of their input stimuli (Sx) at steady state, whereas types 1 and 2 respond to both stimuli (Sx and Sy). This reduced functionality might be part of the reason that types 3 and 4 appear to be selected against during evolution of transcription networks. Furthermore, type 1 coherent FFL benefits from increased cooperativity. This reasoning does not apply to FFLs with OR-gate logic, and additional reasons may underlie the observed bias in FFL types.

Coherent FFLs as Persistence Detectors. An equivalent way to describe the sign-sensitive delay function of the coherent FFL is sign-sensitive persistence detection (6): it responds only to persistent Sx stimuli and rejects short Sx pulses. Short pulses are rejected, however, only in one direction. For example, type 1 AND-gate FFLs (Fig. 1a) reject short on pulses of Sx (in these simulations, the input Sy is on throughout). On the other hand, Z responds strongly even to short off pulses of Sx. Similar persistence detection can be performed by all four coherent FFL types.

Incoherent FFLs as a Mechanism for Speeding Response Times in Transcription Networks. The response time of transcription networks is generally slow (16, 17). Although it takes only a few minutes for the first protein products to appear, the response time (time to reach half-steady-state level) is governed by the lifetime of the protein product (9, 16, 17), which is often on the order of hours. One way to speed up the response time is to increase the degradation rate of the protein product (16, 17). This has the cost of requiring increased production to achieve a given steady-state level. An additional solution is to implement negative autoregulation, in which a transcription factor represses its own transcription. This negative feedback loop has been shown both theoretically (17, 26) and experimentally (17) to speed the response. Negative autoregulation, however, can only work for transcription factors (or genes on an operon that encodes a transcription factor). Genes that are not transcription factors cannot negatively autoregulate their own transcription.

The present study suggests a mechanism for speeding transcriptional responses. We find that the incoherent FFL can greatly reduce the response time relative to simple regulation designs with the same steady state (Fig. 4). The incoherent FFL mechanism can in principle apply to any gene, not only to transcription factors, because the acceleration is carried out by the two transcription factors upstream of the target gene.

In E. coli, several key global regulators play the role of X in coherent type 1 FFLs, including regulators that respond to glucose starvation (CRP), nitrogen limitation (rpoN), and noxious drugs (rob). Interestingly, nonhomologous yeast systems that respond to these key stimuli also display coherent FFLs, with X transcription factors such as MIG1, GLN3, and PDR1 that respond to glucose, nitrogen, and drugs, respectively. Incoherent type 1 FFLs in yeast include anaerobic metabolism (HAP1 as X) and nitrogen starvation (DAL80 or GLN3 as X) systems. One pair of transcription factors in E. coli that respond to anaerobic conditions (fnr as X and arcA as Y) show four different types of FFLs with different operons: coherent type 1 for Z = focA, coherent type 3 for Z = cyoABDCE, incoherent type 1 for Z = glpACB, and incoherent type 3 for Z = cydAB. This diversity suggests an intricate kinetic regulation of different anaerobic metabolism systems, possibly some with sign-sensitive delays, others with sign-sensitive acceleration and others with pulses, with respect to oxygen availability (27, 28). Often, several FFLs share the same X, e.g., 16 for CRP, a property that should not affect any of the present conclusions. Tables 4 and 5, which are published as supporting information on the PNAS web site, www.pnas.org, show all FFLs in the E. coli and S. cerevisiae databases, respectively.

The present study suggests a defined experimental program to test the functions of the FFL types. We found experimentally that the ara system in E. coli, which is a type 1 AND-gate coherent FFL, acts as a sign-sensitive delay with respect to cAMP signals (34). Similar experiments on other natural systems can help establish the function of the FFL in its various structural configurations. In addition, all of the FFL types may be synthetically built out of characterized components (16, 17, 29–33), allowing construction of pulsers and other useful circuit elements. This article focused on kinetic behavior and steady-state logic. The FFL may have been selected to perform additional functions, including functions associated with intermediate steady-state levels. Some of the characteristics of FFLs can be carried out by simpler circuits; for example, AND-like and OR-like steady-state logic can be carried out by simple regulation, and cooperativity increase can be carried out by cascades. However, FFLs have some unique features, such as acceleration and pulse generation that cannot be carried out by cascades or simple regulation. It would be interesting to map additional functions that can be performed by FFLs, in particular functions that cannot be carried out by simpler circuits.

New network motifs are likely to emerge as our knowledge of biological networks becomes more complete. It would be fascinating to study the function of additional network motifs to determine whether biological networks can be understood in terms of recurring circuit elements, each with a defined information-processing role.