Role of the β1 Subunit in Large-Conductance Ca²⁺-Activated K⁺ Channel Gating Energetics

Abstract

Over the past few years, it has become clear that an important mechanism by which large-conductance Ca²⁺-activated K⁺ channel (BK_Ca) activity is regulated is the tissue-specific expression of auxiliary β subunits. The first of these to be identified, β1, is expressed predominately in smooth muscle and causes dramatic effects, increasing the apparent affinity of the channel for Ca²⁺ 10-fold at 0 mV, and shifting the range of voltages over which the channel activates −80 mV at 9.1 μM Ca²⁺. With this study, we address the question: which aspects of BK_Ca gating are altered by β1 to bring about these effects: Ca²⁺ binding, voltage sensing, or the intrinsic energetics of channel opening? The approach we have taken is to express the β1 subunit together with the BK_Ca α subunit in Xenopus oocytes, and then to compare β1's steady state effects over a wide range of Ca²⁺ concentrations and membrane voltages to those predicted by allosteric models whose parameters have been altered to mimic changes in the aspects of gating listed above. The results of our analysis suggest that much of β1's steady state effects can be accounted for by a reduction in the intrinsic energy the channel must overcome to open and a decrease in its voltage sensitivity, with little change in the affinity of the channel for Ca²⁺ when it is either open or closed. Interestingly, however, the small changes in Ca²⁺ binding affinity suggested by our analysis (K_c 7.4 μM → 9.6 μM; K_o = 0.80 μM → 0.65 μM) do appear to be functionally important. We also show that β1 affects the mSlo conductance–voltage relation in the essential absence of Ca²⁺, shifting it +20 mV and reducing its apparent gating charge 38%, and we develop methods for distinguishing between alterations in Ca²⁺ binding and other aspects of BK_Ca channel gating that may be of general use.

INTRODUCTION

Several mechanisms have evolved to tune the functional properties of ion channel proteins and thereby the electrical activity of various cell types. Prominent among them is the expression of auxiliary subunits that alter channel gating (Isom et al. 1994; Gurnett and Campbell 1996; Trimmer 1998). How auxiliary subunits accomplish this task is in most cases not understood, but finding answers to this question is important both for gaining a better understanding of the relationship between the structure of ion channels and how they function, and for providing information that may be used to design pharmaceuticals that can modulate the many physiological processes in which ion channels play important roles.

One ion channel whose activity is markedly affected by the expression of an auxiliary subunit is the large conductance Ca²⁺-activated potassium (BK_Ca) channel. In certain tissues, it appears that this channel is composed simply of four identical α subunits surrounding a central pore (Shen et al. 1994; Rothberg and Magleby 1999). Indeed, cloning and heterologous expression studies have revealed that channels composed of only α subunits display the essential properties of native BK_Ca channels: Ca²⁺ sensitivity, voltage sensitivity, K⁺ selectivity, and a large single-channel conductance (Atkinson et al. 1991; Adelman et al. 1992; Butler et al. 1993; Pallanck and Ganetzky 1994; Tseng-Crank et al. 1994). When BK_Ca channels where purified from bovine smooth muscle, however, two different subunits where isolated: a pore-forming α subunit (1,197 amino acids) and a smaller auxiliary β subunit (191 amino acids) (Garcia-Calvo et al. 1994; Knaus et al. 1994b; Giangiacomo et al. 1995). Both α and β subunits were shown to be integral membrane proteins. Subsequent cloning and expression of these subunits revealed that while the α subunit forms a functional channel, coexpression of the β subunit increases the sensitivity of the channel to Ca²⁺ at many membrane voltages (McManus et al. 1995; Wallner et al. 1995; Tseng-Crank et al. 1996). The presence of the β subunit therefore appears to explain previous observations that indicated that smooth muscle BK_Ca channels are more sensitive to Ca²⁺ than are BK_Ca channels in many other tissues (McManus 1991; Tanaka et al. 1997).

Recently, additional BK_Ca β subunits have been discovered and characterized (Ramanathan et al. 1999; Wallner et al. 1999; Xia et al. 1999; Brenner et al. 2000; Meera et al. 2000; Weiger et al. 2000). Although structurally similar, these novel βs each alter BK_Ca channel gating in distinct ways, and they have different tissue distributions. They thus appear to have evolved to provide tissue-specific tuning of BK_Ca channel function, altering such properties as Ca²⁺ sensitivity, voltage–range-of-activation, and activation, deactivation, and inactivation kinetics. In this study, we have analyzed the functional effects of the original β subunit, now designated β1.

An intriguing property of BK_Ca channel gating even in the absence of β1 is that in addition to being sensitive to internal Ca²⁺, these channels are also sensitive to changes in membrane voltage, and there is a synergy between these stimuli. One manifestation of this synergy is a shift in the channel's conductance–voltage relation toward more negative voltages as internal Ca²⁺ is raised (Marty 1981; Barrett et al. 1982; Latorre et al. 1982; Methfessel and Boheim 1982; Wong et al. 1982; Moczydlowski and Latorre 1983). Another is an apparent increase in the channel's Ca²⁺ affinity as the membrane voltage is made more positive (Markwardt and Isenberg 1992; Cui et al. 1997). Recently, we have developed allosteric models that mimic this synergy (Cox et al. 1997a). They do so not by supposing that changes in voltage are directly affecting the binding of Ca²⁺ to the channel in a given conformation, but rather by supposing that the favorable decrease in free energy imparted to the channel's open conformation by one stimulus lessens the amount of work necessary for the other stimulus to activate the channel. The behavior of these models underscores the possibility that changes in the channel's apparent Ca²⁺ affinity can be brought about by means other than a direct effect on the channel's Ca²⁺ binding sites.

How then might the BK_Ca β1 subunit increase the apparent affinity of the channel for Ca²⁺? At the single channel level, Nimigean and Magleby 1999, Nimigean and Magleby 2000 have demonstrated that β1 increases the time the mSlo channel occupies a set of predominately open bursting states, and that simply an increase in all of the channel's forward Ca²⁺ binding rates cannot account for this behavior. Thus, perhaps something more than just a change in Ca²⁺ binding affinity is occurring. On the other hand, their experiments did not rule out β1-induced changes in Ca²⁺ unbinding rates. In fact, Meera et al. 1996 have found that substantial Ca²⁺ (∼300 nM) must be present for human β1 to shift the hSlo G-V relation toward more negative potentials. This would seem to argue for a direct effect of β1 on Ca²⁺ binding or unbinding because when Ca²⁺ is not present, and thus its binding properties are irrelevant, β1's steady state effects are apparently lost.

More generally, we may list at least three mechanisms by which the β1 subunit may plausibly increase the BK_Ca channel's apparent Ca²⁺ affinity. It may alter the voltage sensitivity of the channel and thereby indirectly alter the channel's apparent Ca²⁺ affinity. It may change the energetics of conformational changes that are separate from both voltage sensing and Ca²⁺ binding, or it may in fact change the affinities of Ca²⁺ binding sites. To distinguish between these possibilities, we have examined the gating properties of the mSlo channel (the BK_Ca channel cloned from mouse; Butler et al. 1993) in the presence and absence of the β1 subunit (bovine) over a wide range of conditions, and analyzed these changes in terms of allosteric models that represent our current understanding of BK_Ca channel gating (Cox et al. 1997a; Cui et al. 1997; Horrigan and Aldrich 1999; Horrigan et al. 1999; Rothberg and Magleby 1999). The results of this analysis suggest that β1 increases the Ca²⁺ sensitivity of the mSlo channel by both altering aspects of gating that do not involve Ca²⁺ binding, and by making subtle but important changes to the channel's Ca²⁺ binding affinity when it is either open or closed.

MATERIALS AND METHODS

Channel Expression

The BK_Ca α subunit clone (mbr5) was kindly provided by Dr. Larry Salkoff (Butler et al. 1993), and the β subunit clone (bovine β1) was kindly provided by Dr. Owen McManus (McManus et al. 1995). Both clones were propagated in the Escherichia coli strain Top 10. In vitro transcription was performed with the mMessage mMachine kit with T3 or T7 RNA polymerase (Ambion Inc.). To record macroscopic currents ∼0.05–0.5 ng of total cRNA was injected into Xenopus laevis oocytes 2–6 d before recording. β1 and α cRNA were mixed in a ratio of 6:1 (β/α) before injection. We found this ratio to be well above that necessary for saturation of β1's effects.

Electrophysiology

Electrophysiological recordings were performed essentially as described previously (Cox et al. 1997b). All recordings were done in the inside-out patch clamp configuration (Hamill et al. 1981). Patch pipettes were made of borosilicate glass (VWR Micropipettes). Their tips were coated with wax (Sticky Wax) and fire polished before use. Data were acquired using an Axopatch 200-A patch-clamp amplifier (Axon Instruments, Inc.) in the resistive feedback mode and a Macintosh-based computer system using “Pulse” acquisition software (HEKA Electronik) and the ITC-16 hardware interface (Instrutech). Records were digitized at 20-μs intervals (50,000 samples/s) and low pass filtered at 10 KHz with the Axopatch's four pole bessel filter. All experiments were carried out at 23°C. Under most conditions, before current records were analyzed and displayed (see, for example, Fig. 6), capacity and leak currents were subtracted using a P/5 leak subtraction protocol with a holding potential of −120 mV and voltage steps opposite in polarity to those in the experimental protocol. With the β1 subunit present, there was significant holding current at −120 mV with 39 or 74 μM [Ca²⁺]. Under these conditions, capacity and leak currents were measured by lowering [Ca²⁺] to 0.0005 μM and repeating the experimental protocol. Because of the limited voltage range, no channel activation was observed. The currents recorded with 0.0005 μM [Ca²⁺] were then used for capacity and leak current subtraction. As estimated from the amplitude and decay time constant of capacity currents, the series resistance (Rs) under our experimental conditions was 5–6 MΩ. Typically, 50–90% of Rs was compensated for using the Axopatch 200-A's Rs compensation circuitry. To increase the signal-to-noise ratio, typically three to six current series were taken consecutively under identical conditions and averaged before analysis and display. Maximum current amplitudes in each patch were typically 1–4 nA. In a series of 45 experiments, 23 without and 22 with the β1 subunit, the means and standard deviations of the current amplitudes recorded with 74 μM [Ca²⁺] at +150 mV were 2.38 ± 1.19 nA without and 2.44 ± 1.00 nA with the β1 subunit. These means are not statistically, significantly different (Students t test, P = 0.84); however, the 95% confidence interval calculated from these data for the difference between mean current amplitudes is 0.06 ± 0.66 nA.

Figure 6.

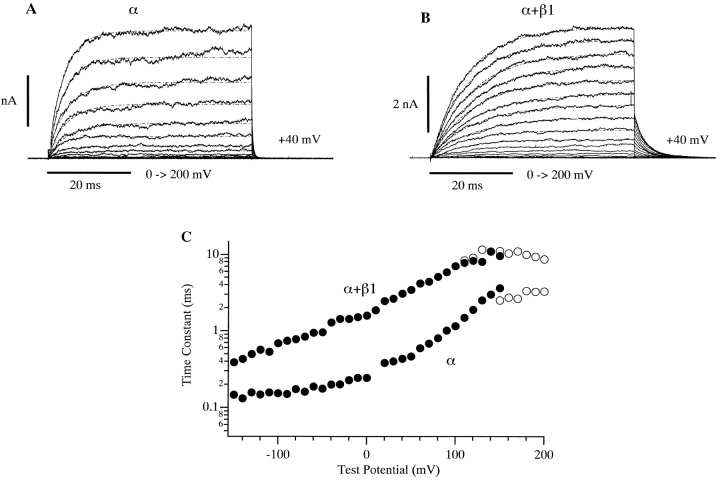
The β1 subunit has effects on mSlo macroscopic current kinetics at subnanomolar [Ca²⁺]. Shown are families of current traces recorded in the absence (A) and presence (B) of β1. For each trace, the membrane voltage was held at −50 mV and stepped to the indicated voltages. [Ca²⁺] equaled 0.5 nM. Superimposed on each trace are monoexponential fits. Time constants from these fits are plotted in C (○). Also plotted are deactivation time constants (•) determined from monoexponential fits to currents elicited with a protocol that activated the channels with a depolarizing step, and then deactivated with steps to the indicated potentials (traces not shown).

Solutions

Recording solutions were composed of the following (mM): pipette solution: 80 KMeSO₃, 60 N-methyl-glucamine-MeSO₃, 20 HEPES, 2 KCl, 2 MgCl₂, pH 7.20; internal solution: 80 KMeSO₃, 60 N-methyl-glucamine MeSO₃, 20 HEPES, 2 KCl, 1 HEDTA or 5 EGTA, and CaCl₂ sufficient to give the appropriate free Ca²⁺ concentration, pH 7.20. EGTA (Sigma-Aldrich) was used as the Ca²⁺ buffer for solutions intended to contain <1 μM free Ca²⁺. HEDTA (Sigma-Aldrich) was used as the Ca²⁺ buffer for solutions intended to contain between 1 and 50 μM free Ca²⁺, and no Ca²⁺ chelator was used in solutions intended to contain >50 μM free Ca²⁺. 50 μM (+)-18-crown-6-tetracarboxylic acid (18C6TA) was added to all internal solutions to prevent Ba²⁺ block at high voltages.

The appropriate amount of total Ca²⁺ (100 mM CaCl₂ standard solution; Orion Research Inc.) to add to the base internal solution containing 1 mM HEDTA or 5 mM EGTA to yield the desired free Ca²⁺ concentration was calculated using the program Max Chelator (Bers et al. 1994; available online at www.stanford.edu/~cpatton/maxc.html), and the proton and Ca²⁺ binding constants of Bers (supplied with the program) for pH 7.20, T = 23°C, and ionic strength = 0.15. The ability of 18C6TA to chelate Ca²⁺, as well as K⁺ and Ba²⁺, was also considered in these calculations using the following dissociation constants: Ca²⁺ 10⁻⁸ M (Dietrich 1985), K⁺ 3.3 × 10⁻⁶ M (Dietrich 1985), and Ba²⁺ 1.6 × 10⁻¹⁰ M (Diaz et al. 1996). For solutions intended to contain 0.5 μM free Ca²⁺ or more, free Ca²⁺ was measured with a Ca-sensitive electrode (Orion Research Inc.), and the measured value was reported. Free Ca²⁺ measurements were precise to within ∼8%. Standard Ca²⁺ solutions for calibration of the Ca-sensitive electrode were prepared by adding known amounts (1, 10, 100, 1,000, and 10,000 μM) of standard CaCl₂ solution to a solution identical to our base internal solution, except that no chelator was added. Endogenous Ca²⁺ in this solution was estimated from the deviation from linearity of the Ca-sensitive electrode's response at 10 μM added Ca²⁺, and was typically ∼10 μM. Endogenous Ca²⁺ was then compensated for when making Ca²⁺-buffered solutions. When preparing solutions intended to contain <1 μM free Ca²⁺, the base internal solution was passed through a chelex 100 column before addition of chelator and Ca²⁺. This lowered the endogenous free Ca²⁺ to ∼3 μM.

The concentration of Ca²⁺ in the solution bathing the cytoplasmic face of the patch was exchanged using a sewer pipe flow system (DAD 12) purchased from Adams & List Associates, Ltd. To minimize potential systematic variation, all comparisons between mSlo_α and mSlo_{α + β1} currents were done with the same experimental set up and the same solutions. The same batches of oocytes were also often but not always used.

Determination of G-V Curves and Fitting

At [Ca²⁺] ≥600 nM, G-V relations were determined from the amplitude of tail currents 200 μs after repolarization to a fixed membrane potential (−80 mV) after voltage steps to the indicated test voltages. Each G-V relation was fitted with a Boltzmann function G=G _max1+e ^{zFV₁₂−VRT}and normalized to the peak of the fit. At [Ca²⁺] < 600 nM, G-V relations were determined from tail current measurements 200 μs after repolarization to +40 mV. Since these curves did not reach saturation, the percentage of channels active at +200 mV in a given patch was estimated by comparison to current amplitudes recorded from the same patch with 9.1 μM [Ca²⁺], as described in the text (see Fig. 7). Boltzmann fits were performed with “Igor Pro” graphing and curve fitting software (WaveMetrics Inc.) using the Levenberg-Marquardt algorithm to perform nonlinear least squares fits.

Figure 7.

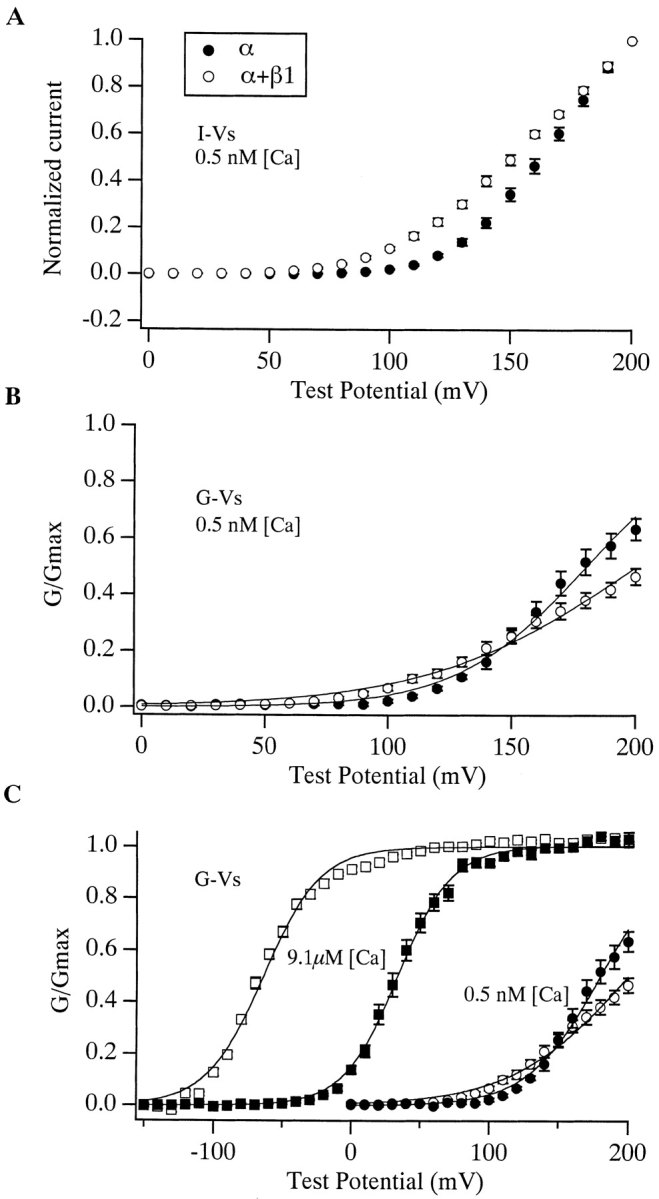
Effects of the β1 subunit at subnanomolar [Ca²⁺]. (A) Current–voltage curves for mSlo_α (•, n = 11) and mSlo_{α + β1} (○, n = 8) determined from patches superfused with a solution buffered to 0.5 nM [Ca²⁺]. Each current measurement was made at the end of a 50-ms voltage step to the indicated test voltage. Each curve has been normalized to their values at +200 mV. (B) G-V relations were determined as described in the text from the same data as used to generate the I-V plots in A. Smooth curves represent Boltzmann fits. Parameters of the fits are: mSlo_α V_1/2 = 180 mV, z = 0.98, mSlo_{α + β1} V_1/2 = 200 mV, z = 0.61. (C) In each experiment, data was acquired at both 9.1 μM (squares) and 0.5 nM [Ca²⁺] (circles). G-V plots for both concentrations are shown in C. In A–C, error bars indicate SEM.

Model fits to many G-V curves simultaneously were performed with Table-Curve 3D software, which employs an 80-bit Levenberg-Marquardt algorithm (Jandel Scientific) to minimize the sum of squares. When fitting to the 50-state model, several sets of initial parameters were tested. The resulting fits were usually similar, but not always identical. The best fit was then chosen as that which minimized the sum of squares.

Statistical Tests

Unpaired, two-tailed Student's t tests were used to test for significant differences between the mean V_1/2 and z values listed in Table and Table .

Table 1.

G-V Parameters

	G G max=11+e zFV12−VRT
	V1/2				z
[Ca]i	mSloα	n	mSloα + β1	n	mSloα	n	mSloα + β1	n
μM	mV		mV
0.99	117.0 ± 11.7‡	10	79.3 ± 8.8	10§	1.32 ± 0.129	10	1.03 ± 0.093	10§
1.9	102.0 ± 6.5	9	24.7 ± 13.0	6§	1.27 ± 0.120	9	1.29 ± 0.198	6
4.6	65.8 ± 7.6	14	−6.4 ± 12.3	11§	1.32 ± 0.130	14	1.15 ± 0.101	11§
9.1	51.8 ± 8.1	15	−28.1 ± 17.7	13§	1.24 ± 0.101	15	1.09 ± 0.184	13§
39	29.5 ± 8.0	12	−90.0 ± 8.0	9§	1.14 ± 0.090	12	1.08 ± 0.155	9
74	21.7 ± 8.5	12	−95.9 ± 9.0	9§	1.12 ± 0.129	12	0.92 ± 0.108	9§

Table 2.

G-V Parameters

	G G max=11+e zFV12−VRT
	V1/2				z
[Ca]i	mSloα	n	mSloα + β1	n	mSloα	n	mSloα + β1	n
μM	mV		mV
0.0005	181.0 ± 14.4‡	8	200.5 ± 14.4	8§	1.01 ± 0.106	8	0.62 ± 0.052	8§
0.002	175.5 ± 14.0	4	207.0 ± 26.8	5	0.91 ± 0.166	4	0.64 ± 0.037	5§
0.010	182.4 ± 13.0	5	179.1 ± 22.2	11	0.96 ± 0.137	5	0.65 ± 0.652	11§
0.050	174.1 ± 10.0	4	193.2 ± 24.6	8	0.96 ± 0.102	4	0.64 ± 0.050	8§
0.100	171.5 ± 11.5	4	176.3 ± 30.7	10	0.96 ± 0.070	4	0.61 ± 0.050	10§
0.66	110.6 ± 10.1	6	72.6 ± 21.0	11§	1.61 ± 0.250	6	1.22 ± 0.291	11§
0.99	105.3 ± 1.8	2	68.1 ± 4.6	2§	1.57 ± 0.003	2	1.00 ± 0.005	2§

We tested whether there was a constant shift between mSlo_α and mSlo_{α + β1} mean z*V_1/2 values over all [Ca²⁺] by fitting the data used to calculate the values plotted in Fig. 5 A (below) with two statistical models. Model 1 assumed that the β1 subunit had a constant affect on z*V_1/2 regardless of [Ca²⁺]. Model 2 allowed the effects of β1 on z*V_1/2 to vary with [Ca²⁺]. The two models were fitted to the data using a maximum likelihood criteria. The linear models employed were as follows.

Figure 5.

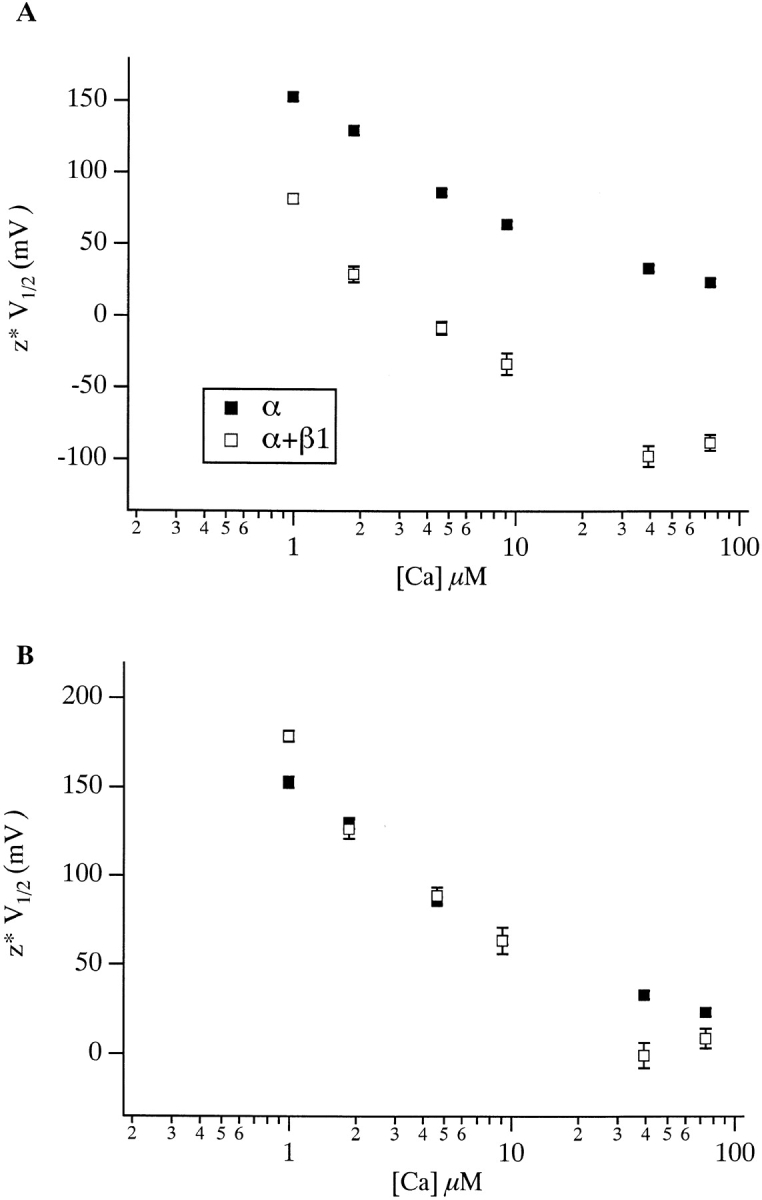
(A) z*V_1/2 vs. [Ca²⁺] plots for mSlo_α (▪) and mSlo_{α + β1} (□). Each point represents the product of z and V_1/2 values listed in Table . In B, the mSlo_{α + β1} curve has been shifted vertically so that the two curves overlap at 9.1 μM. Errors bars indicate SEM and are often smaller than the plot symbols.

Model 1:(means assumed parallel).

For this model, we define the following parameters. A0 represents the expected z*V_1/2 value in the absence of the β1 subunit at 0.99 μM Ca²⁺. A1 represents the effect of β1 on z*V_1/2, which in this model is assumed to be the same for all [Ca²⁺]. A2–A6 represent the additional effect of [Ca²⁺] over that observed at 0.99 μM for each measurement depending on the [Ca²⁺] at which a particular measurement was taken. Under this model, the expected value for a particular data point recorded, for example, with the β1 subunit present and 9.1 μM [Ca²⁺], would be A0 (the expected value at 0.99 μM) + A1 (the effect of the β1 subunit) + A4 (the additional effect of 9.1 μM [Ca²⁺] over that caused by 0.99 μM). Using statistical nomenclature, we may write the model then as: expected value at every point i = A0 + A1 I(ab_i = b) + A2 I(ca_i = 1.86) + A3 I(ca_i = 4.63) + A4 I(ca_i = 9.1) + A5 I(ca_i = 39) + A6 I(ca_i = 74).

Here, as follows from the definitions below, the terms not related to the conditions of a particular measurement evaluate to 0. The following definitions are applied. I() is the incremental function defined as [I(x = y) = 1, if x = y and I(x = y) = 0 if x is not equal to y]: ab_i = b if for the ith observation the β1 subunit was present, ab_i = a if for the ith observation the β1 subunit was absent, ca_i = 0.99 if for the ith observation [Ca²⁺] = 0.99, ca_i = 1.86 if for the ith observation [Ca²⁺] = 1.86, ca_i = 4.63 if for the ith observation [Ca²⁺] = 4.63, and so on.

Model 2: (means not assumed parallel).

Following the above discussion, Model 2 may be written as: expected value of p_i = A0 + A1 I(ab_i = b) + A2 I(ca_i = 1.86) + A3 I(ca_i = 4.63) + A4 I(ca_i = 9.1) + A5 I(ca_i = 39) + A6 I(ca_i = 74) + A7 I(ab_i = b) I(ca_i = 1.86) + A8 I(ab_i = b) I(ca_i = 4.63) + A9 I(ab_i = b) I(ca_i = 9.1) + A10 I(ab_i = b) I(ca_i = 39) + A11 I(ab_i = b) I(ca_i = 74), where A0–A11 are parameters of the model. Here the effect of the β1 subunit is not assumed to be the same at each [Ca²⁺], and so there is a separate parameter that represents the additional effect of the β1 subunit (A7–A11) at each [Ca²⁺] above 0.99 μM. Under this model, the expected value for a particular data point recorded, for example, with the β1 subunit present at 9.1 μM [Ca²⁺] would be A0 (the expected value at 0.99 μM) + A1 (the effect of the β1 subunit at 0.99 μM [Ca²⁺]) + A4 (the additional effect of 9.1 μM [Ca²⁺] over that caused by 0.99 μM) + A9 (the additional effect of the β1 subunit observed at 9.1 μM over that observed at 0.99 μM).

The likelihood of each individual observation was calculated from the expected value under the model at the appropriate condition and the estimated variance of the mean at each point. Model performance was compared using a likelihood ratio test that assumed normal errors, and allowed for heterogeneity of variance. The test was implemented with S-PLUS 2000 software (Mathsoft), and it gave a chi-square value of 38.236 on five degrees of freedom.

RESULTS

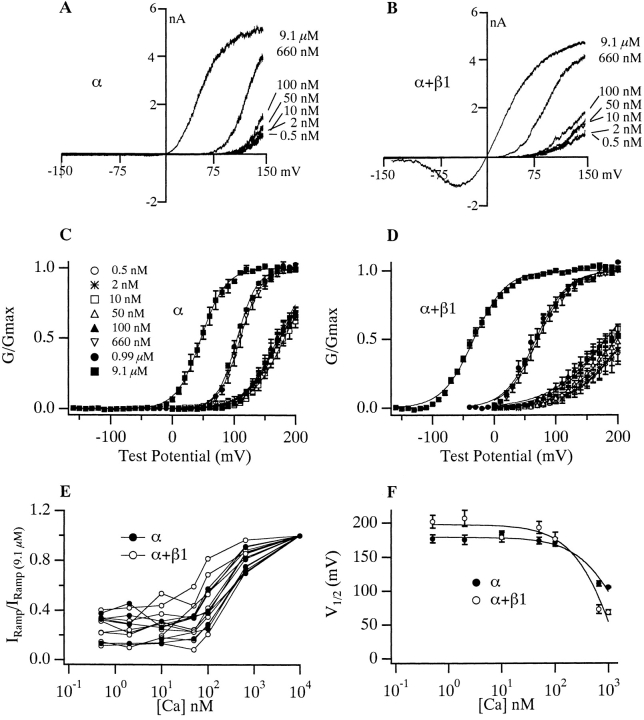
We will refer to channels composed of α subunits alone as mSlo_α and those composed of both α and β1 subunits as mSlo_{α + β1}. The influence that internal Ca²⁺ and membrane voltage have on the steady state open probability of mSlo_α is often displayed as a series of conductance-voltage curves determined at several internal Ca²⁺ concentrations ([Ca²⁺]) (Fig. 1 A). The curves in Fig. 1 A correspond to [Ca²⁺] ranging from ∼1 to ∼75 μM. As [Ca²⁺] is raised, the mSlo_α G-V curve moves leftward along the voltage axis. When the β1 subunit is coexpressed with α, a similar Ca²⁺-dependent shifting is observed (Fig. 1 B). The G-V curves determined with β1 present, however, differ from those determined with α alone in that each mSlo_{α + β1} G-V curve lies to the left of the corresponding mSlo_α curve. Over this [Ca²⁺] range, at every combination of [Ca²⁺] and voltage, the channels are more active when β1 is present. This effect was first demonstrated by McManus et al. 1995. Notice also that the mSlo_{α + β1} G-V curves are more widely spaced than are the mSlo_α curves. As shown in Fig. 1 C, when half-maximal-activation–voltage (V_1/2) is plotted as a function of [Ca²⁺], the wider spacing of the mSlo_{α + β1} curves creates a function with a steeper slope.

Figure 1.

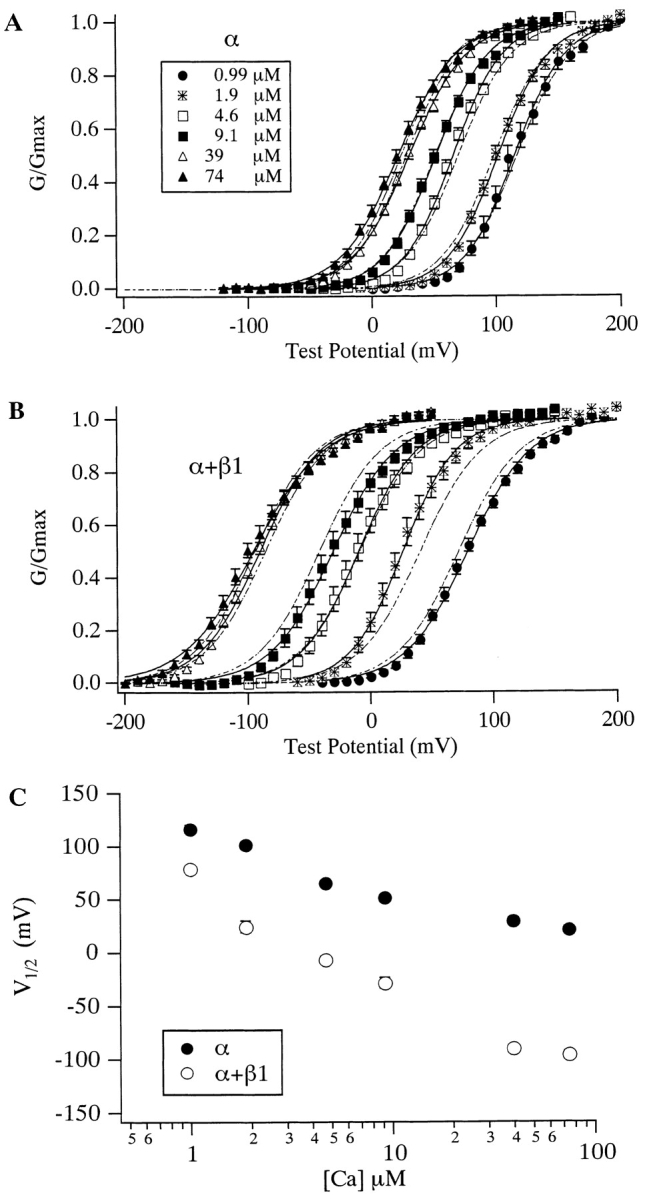
The effects of Ca²⁺ on the mSlo G-V relation with and without the β1 subunit. G-V relations determined without (A) and with (B) β1 coexpression. [Ca²⁺] are as indicated in A. Each curve represents the average of between 6 and 15 experiments, as indicated in Table . Error bars represent SEM. Solid curves represent fits to the Boltzmann function G G _max=11+e ^{zFV₁₂−VRT}.Parameters of the fits are as follows, in order of increasing [Ca²⁺]. mSlo_α V_1/2 (mV) = 115.0, 101.9, 65.7, 51.8, 30.4, and 21.2; z = 1.25, 1.25, 1.27, 1.20, 1.10, and 1.09. mSlo_{α + β 1} V_1/2 (mV) = 79.2, 26.4, −9.6, −30.6, −90.1, and −95.6; z = 1.01, 1.18, 1.08, 0.99, 1.04, and 0.90. Dashed curves represent fits to . Fit parameters are as follows: mSlo_α L(0) = 1,263, Q = 1.18, K _C = 6.00 μM, K _O = 1.24 μM; mSlo_{α + β1} L(0) 281, Q = 1.02, K _C = 9.82 μM, K _O = 0.82 μM. (C) Plots of V_1/2 vs. [Ca²⁺] for mSlo_α (•) and mSlo_{α + β1} (○) channels. Data are from Table . Error bars indicate SEM and are often smaller than the plot symbols.

Perhaps less apparent, the mSlo_{α + β1} G-V curves are also somewhat less steep than are the mSlo_α curves. In Fig. 1A and Fig. B, each G-V curve represents the average of many experiments (for n values, see Table ). These curves were fitted with Boltzmann functions (solid curves), and the parameters of these fits are listed in the legend. At each [Ca²⁺], the Boltzmann parameter z, which is a measure of the voltage sensitivity of the channel, is smaller for mSlo_{α + β1} than it is for mSlo_α, on average by 14%. When mean parameter values from each experiment fitted individually are examined (Table ), a similar decline in voltage sensitivity is observed at all [Ca²⁺] except 1.9 μM, although the small decline at 39 μM is not clearly statistically significant. Over all, however, looked at in this way, the average decline in z is 11%.

The effects of the β1 subunit can also be seen from the point of view of changing [Ca²⁺] at constant voltage (Fig. 2). Looked at in this way, at many voltages β1 increases the channel's apparent Ca²⁺ affinity. For example at +60 mV the apparent Ca²⁺ affinity of mSlo_α as judged by the concentration at which the channel's Ca²⁺ dose-response curve is half maximal ([Ca²⁺] _1/2), is 3.8 μM, while for mSlo_{α + β1} it is 1.2 μM. At 0 mV, [Ca²⁺] _1/2 for mSlo_α is 32.8 μM, while for mSlo_{α + β1} it is 3.4 μM. Since the G-V curves in Fig. 1 and the dose-response curves in Fig. 2 represent different ways of looking at the same steady-state gating behavior, the effects of the β1 subunit illustrated in these figures are manifestations of the same underlying process.

Figure 2.

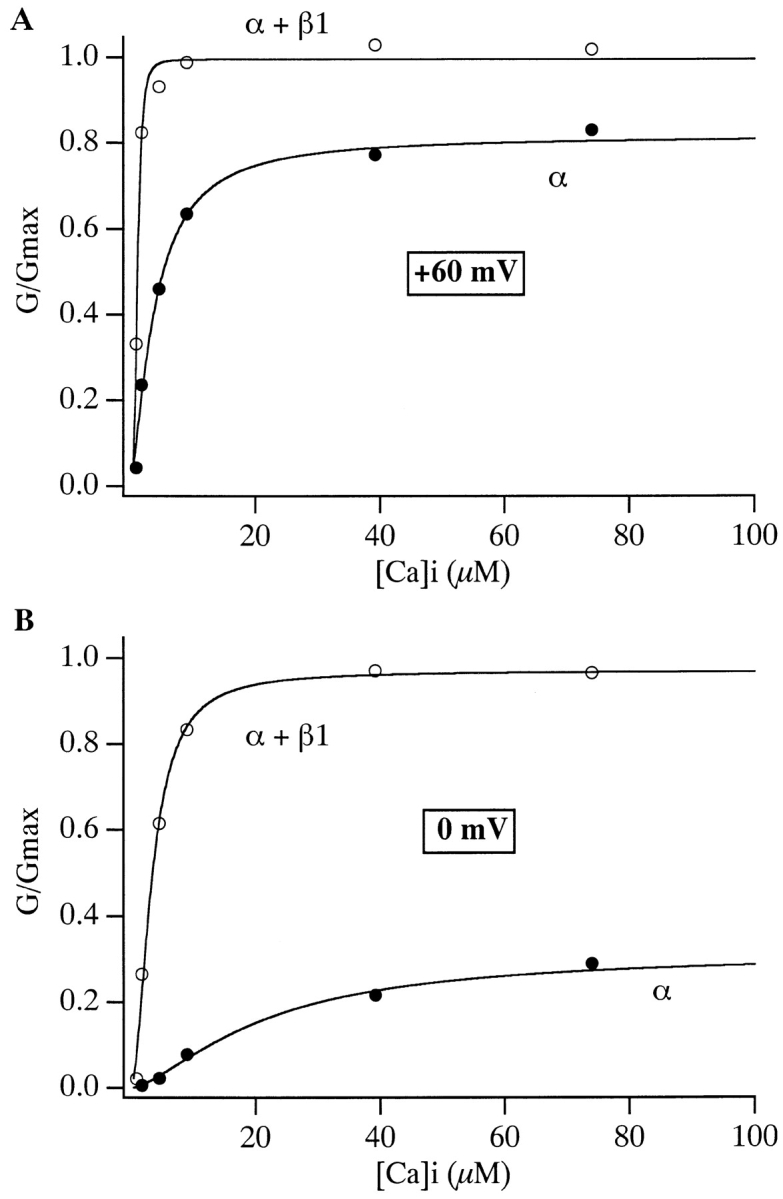
Ca²⁺ dose–response curves for mSlo_α (•) and mSlo_{α + β1} (○) at (A) +60 mV and (B) 0 mV. Data are from those shown in Fig. 1A and Fig. B. Smooth curves represent fits to the Hill equation G/G _max = Amplitude/{1 + (K _d/[Ca])ⁿ}. Fit parameters are as follows: +60 mV mSlo_α K _d = 3.84 μM, n = 1.5, Amplitude = 0.82; mSlo_{α + β1} K _d = 1.20 μM, n = 3.5, Amplitude = 1.00: 0 mV mSlo_α K _d = 32.8 μM, n = 1.3, Amplitude = 0.39; mSlo_{α + β1} K _d = 3.43 μM, n = 1.9, Amplitude = 0.97.

An Interpretation Using a Simple Model

What aspects of gating does the β1 subunit alter to bring about the effects described above? Because a primary effect of β1 is to alter the position of the mSlo G-V curve at many [Ca²⁺], to answer this question it seems important to understand what determines this curve's position. In general, the position of the G-V curve of any voltage-gated channel is determined by the free energy difference between open and closed states in the absence of an applied voltage and the degree to which an applied voltage alters this energy difference. This later factor is itself influenced by many factors including: the number of gating charges the channel has and how far each can move through the membrane's electric field, the degree to which these charges move in concert, and the degree to which the change in energy associated with each moving charge affects the energy difference between open and closed. Without some hypothesis about the physical situation, then, it is difficult to relate changes in the channel's G-V relation to changes in specific aspects of gating.

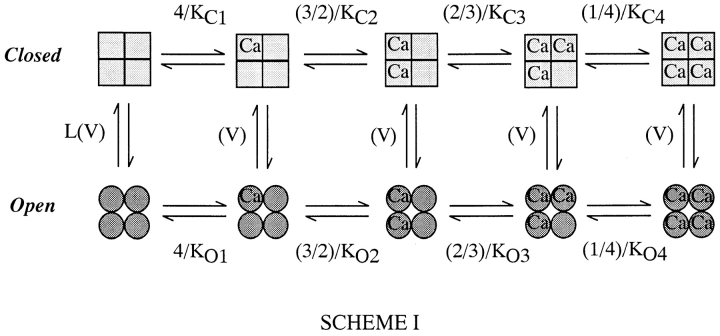
Recently, however, we have shown that models of the form of Scheme I (Fig. 3) can mimic many aspects of mSlo_α gating including: the leftward shifting of the mSlo_α G-V curve as [Ca²⁺] is raised, an apparent increase in Ca²⁺ affinity as the membrane voltage is made more positive, near maximal activation with strong depolarizations at very low [Ca²⁺], and many properties of the kinetics of mSlo_α macroscopic currents (Cui et al. 1997; Cox et al. 1997a). The dashed lines in Fig. 1 A represent a fit with a model of this form. While Scheme I cannot account for all mSlo_α gating properties (see below and Cox et al. 1997a; Stefani et al. 1997; Horrigan and Aldrich 1999; Horrigan et al. 1999; Rothberg and Magleby 1999), the success of this scheme in many respects suggests that it can be useful, at least initially, as a guide for interpreting the effects of the β1 subunit in terms of changes in specific aspects of mSlo gating. We have discussed the properties of models of the form of Scheme I previously (Cox et al. 1997a). We review them here in so far as they relate to the analysis that follows.

Figure 3.

Scheme I, two-tiered gating scheme. Those states in the top tier are designated closed. Those in the bottom tier are designated open. The central conformational change is voltage dependent with gating charge Q, and equilibrium constant L(0) = [closed]/[open]. K_C1, K_C2, K_C3, and K_C4 represent Ca²⁺ dissociation constants in the closed conformation. K_O1, K_O2, K_O3, and K_O4 represent Ca²⁺ dissociation constants in the open conformation. When K_C1 = K_C2 = K_C3 = K_C4 and K_O1 = K_O2 = K_O3 = K_O4, Scheme I represents a voltage-dependent version of the Monod-Wyman-Changeux model of allosteric proteins (Monod et al. 1965).

Scheme I supposes the following. The channel exists in two conformations, open and closed. The equilibrium between open and closed conformations is voltage dependent, with depolarization favoring opening. The channel is a homotetramer containing four Ca²⁺ binding sites, one on each subunit. Ca²⁺ can bind to both the open and closed conformations of the channel but on average binds better to the open conformation, and thereby promotes opening. The simplest form of Scheme I (wherein in a given conformation each binding site binds Ca²⁺ equivalently and independently) corresponds to a voltage-dependent version of the well known Monod-Wyman-Changeux (MWC) model of allosteric proteins (Monod et al. 1965). Its open probability as a function of [Ca²⁺] and voltage (V) is given by:

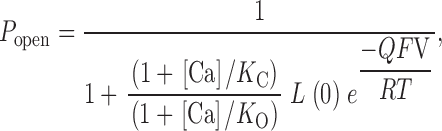1

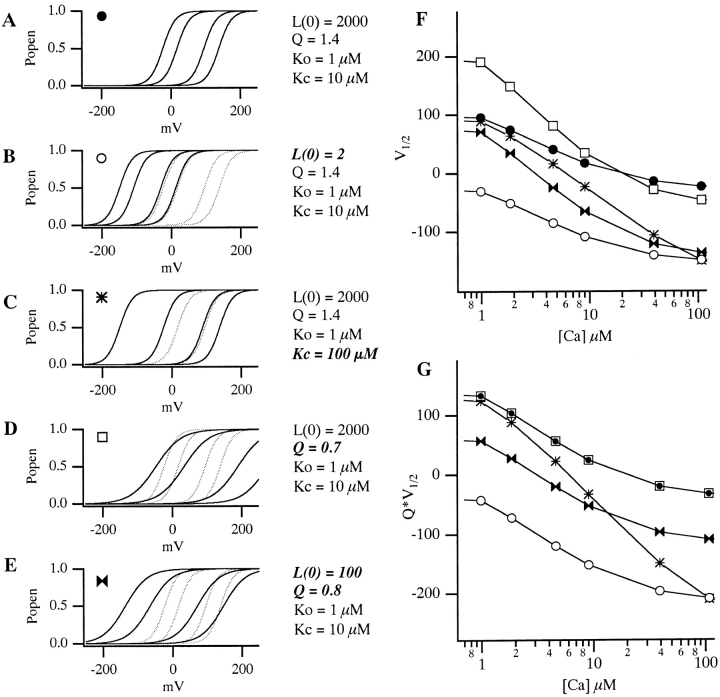
where L(0) represents the open-to-closed equilibrium constant in the absence of an applied voltage, a measure of the intrinsic energetics of channel opening. Q represents the equivalent gating charge associated with this equilibrium, K _C the closed-conformation Ca²⁺ dissociation constant, and K _O the open-conformation Ca²⁺ dissociation constant (F, R, and T have their usual meanings). Thus, four parameters determine the behavior of the model at equilibrium and, as illustrated in Fig. 4, changes in any of these parameters can affect leftward G-V shifts at many [Ca²⁺]. Of interest to us, however, is which of these changes mimic the effects of the BK_Ca β1 subunit.

Figure 4.

Effects of changes in various aspects of gating according to the voltage-dependent MWC model. (A) Control G-V simulations, with (from left to right) 0, 1, 10, and 100 μM [Ca²⁺]. Model parameters are as indicated. (B) Simulated effect of decreasing L(0). (C) Simulated effect of decreasing K _C. (D) Simulated effect of decreasing Q. (E) Simulated effect of decreasing both Q and L(0). In A–E, the control curves of A are shown in gray for comparison. (F) Simulated V_1/2 vs. [Ca²⁺] and (G) Q*V_1/2 vs. [Ca²⁺] plots for the various conditions shown in A–E. Symbols are as indicated in A–E. Notice in G that only when the actual affinity of the model for Ca²⁺ is altered does the Q*V_1/2 vs. [Ca²⁺] plot change shape.

The position of the voltage-dependent (VD) MWC model's G-V relation on the voltage axis, as indexed by its half-maximal activation voltage (V_1/2) is given by:

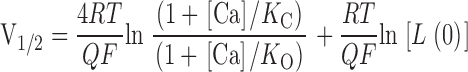2

and the derivative of with respect to [Ca²⁺] is:

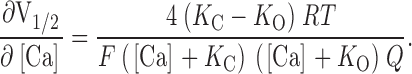3

Interestingly, this derivative is not a function of L(0). This means that according to the VD-MWC model alterations in the intrinsic energy difference between open and closed states will affect G-V position to the same extent at each [Ca²⁺], and if the β1 subunit were only affecting this parameter, there would be no change in the slope of a plot of V_1/2 vs. [Ca²⁺]. This is illustrated in Fig. 4 B. Here, a simulated decrease in L(0) causes equivalent leftward G-V shifts at each [Ca²⁺] (compare Fig. 4B with A), with the spacing between the curves maintained. On a plot of V_1/2 vs. [Ca²⁺], this appears as a downward translation along the V_1/2 axis with no change in slope (see Fig. 4 F). In contrast to this prediction, we see in Fig. 1 C that β1 not only shifts the mSlo channel's V_1/2 vs. [Ca²⁺] relation downward, but it also increases its steepness. That is, the magnitude of the β1-induced G-V shift increases with increasing [Ca²⁺]. This suggests that the effects of β1 are more complicated than just an alteration in the intrinsic energy difference between open and closed. The general downward movement of the channel's V_1/2 vs. [Ca²⁺] relation, however, does suggest that this energy difference may be decreased by β1 in combination with changes in other aspects of gating.

According to , an increase in the steepness of a plot of V_1/2 vs. [Ca²⁺] can come about either by changes in the channel's Ca²⁺ binding constants or by a decrease in its voltage sensitivity. An example of the former possibility is shown in Fig. 4 C. Here, raising K _C in our simulation produces leftward G-V shifts when [Ca²⁺] is present, with larger shifts at higher [Ca²⁺] (compare Fig. 4C with A). At many voltages, this would appear as an increase in apparent Ca²⁺ affinity. At 0 mV, for example, the model channel is only 28% activated at 10 μM [Ca²⁺] when K _C equals 10 μM, but 78% activated at the same [Ca²⁺] when K _C equals 100 μM. Thus, by decreasing the channel's true Ca²⁺ binding affinity in the closed conformation, we can, in fact, create an apparent increase in Ca²⁺ affinity. This underscores the sometimes counterintuitive nature of even simple allosteric systems and highlights the need for a mechanistic and mathematical framework for interpreting alterations in their behavior. In this case, the increase in the model's apparent Ca²⁺ affinity with increasing K _C arises because as K _C and K _O become farther apart, Ca²⁺ binding has a more powerful effect on the model's closed-to-open equilibrium. This can be seen from , where dV_1/2/d[Ca] depends always on the difference between K _C and K _O such that as these constants are separated, larger effects of Ca²⁺ are expected. Furthermore, because dV_1/2/d[Ca] is a function of both K _C and K _O, any change in either of these constants will affect the steepness of the model's V_1/2 vs. [Ca²⁺] relation.

also states that the steepness of the model's V_1/2 vs. [Ca²⁺] relation is inversely related to its equivalent gating charge Q. This relation is therefore expected to become steeper as Q decreases and the model's G-V curves become more shallow (Cox et al. 1997a; Cui, J., and R.W. Aldrich, manuscript in preparation). This is illustrated in Fig. 4D and Fig. F, where we have decreased Q in our simulation from 1.4 to 0.7 and observe an increase in G-V spacing (Fig. 4 D), and thus an increase in V_1/2 vs. [Ca²⁺] slope (Fig. 4 F). Thus, some of the increased steepness of the mSlo channel's V_1/2 vs. [Ca²⁺] relation may be due to the general decrease in voltage sensitivity evident upon β1 coexpression (see Fig. 1).

According to Scheme I (Fig. 3), however, we can eliminate changes in voltage sensitivity (and thus G-V steepness) from consideration if we plot Q*V_1/2 vs. [Ca²⁺] rather than V_1/2 vs. [Ca²⁺] (Cox et al. 1997a; Cui, J., and R.W. Aldrich, manuscript in preparation). This can be seen by rearranging to:

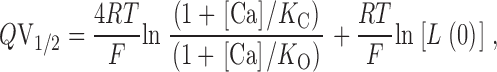4

and again taking the derivative with respect to [Ca²⁺]:

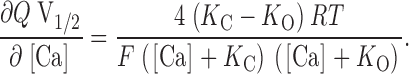5

This derivative is independent of both L(0) and Q and depends only on the model's Ca²⁺ binding constants. According to the VD-MWC model, then, a plot of Q*V_1/2 vs. [Ca²⁺] will only change shape upon β1 coexpression, if β1 is actually altering Ca²⁺ binding site affinities. This is illustrated in Fig. 4 G, where Q*V_1/2 vs. [Ca²⁺] is plotted for the simulations in Fig. 4A–E. Furthermore, although we have come to this conclusion based on the mathematics of the VD-MWC model ( ), it is possible to show that this conclusion holds for all two-tiered models similar in form to Scheme I (Cox et al. 1997a). It therefore does not depend on the simplifying, MWC assumption that in either channel conformation all Ca²⁺ binding steps are equivalent. Nor is it necessary to suppose that the channel has any particular number of Ca²⁺ binding sites. All that is required is a central voltage-dependent conformational change allosterically modulated by Ca²⁺ binding (Cox et al. 1997a).

To apply this test to the effects of the BK_Ca β1 subunit, we have multiplied each V_1/2 value in Table by its corresponding z value and plotted z*V_1/2 vs. [Ca²⁺] for both mSlo_α and mSlo_{α + β 1} in Fig. 5 A. Comparing the resulting relations to those in Fig. 1 C, we see that, in fact, a good deal of the difference in steepness between the mSlo_α V_1/2 vs. [Ca²⁺] relation and the corresponding mSlo_{α + β1} relation can be accounted for by β1-induced changes in voltage sensitivity. That is, the two relations in Fig. 5 A appear close to parallel. This result suggests that much of β1's steady state effects can be accounted for by alterations in aspects of gating separate from Ca²⁺ binding. In particular, it appears that β1 decreases the intrinsic energy difference between open and closed, thus shifting all G-V curves leftward and, in addition, β1 decreases the channel's equivalent gating charge, thus expanding their spacing.

To facilitate comparison of the relations plotted in Fig. 5 A, in B we have shifted the mSlo_{α + β1} relation upward along the z*V_1/2 axis, so that at 9.1 μM [Ca²⁺] the two relations have the same value. Comparing the two relations in this way we see their similar shape. It is also clear, however, that the mSlo_{α + β1} relation has a somewhat higher value than expected at 0.99 μM [Ca²⁺], if the β1 subunit where to have no effect on Ca²⁺ binding, and it has somewhat lower values than expected at 39 and 74 μM. Also, as the data are plotted in Fig. 5 B, the standard errors of the mean for the z*V_1/2 values at 0.99 and 39 μM [Ca²⁺] (indicated on the figure) are small compared with the differences between mSlo_α and mSlo_{α + β1} z*V_1/2 values at these [Ca²⁺]. Thus, the differences in shape between the two curves in Fig. 5 A may not be due to random variation in our experiments.

To examine this issue more rigorously, we performed a statistical test on the data used to calculate the values plotted in Fig. 5 A. We used a likelihood ratio test and two statistical models. One assumed that β1 has a constant effect on z*V_1/2 as a function of [Ca²⁺]; that is, that the two sets of data in Fig. 5 A are parallel. The other allowed for variation in the magnitude of β1's effect with changing [Ca²⁺]. Comparison of the performance of the two models indicates that the two curves in Fig. 5 A are not likely to be parallel (P < 0.0001), even when the variation about each mean is considered (for details of the statistical method used see materials and methods). Thus, in addition to its effects on the energetics and voltage dependence of channel opening, the β1 subunit may also have direct effects on Ca²⁺ binding.

To gain some quantitative estimate of how large these effects may be, we fit the mSlo_{α + β1} G-V data in Fig. 1 B with the VD-MWC model. The dashed lines in this figure represent the least squares best fit. Examining the parameters of this fit, and comparing them with those that best fit the G-V curves in Fig. 1 A, we see that to accommodate the changes produced by β1 the model, as expected, decreases L(0) (1,263 → 281.3) and Q (1.2 → 1.0). In addition, however, small changes in K _O (1.24 → 0.82 μM) and K _C (6.0 → 9.82 μM) are also observed, changes that together increase the ratio (K _C/K _O) and thereby also tend to expand G-V spacing. Interestingly, similar conclusions are also arrived at when these data are analyzed in terms of a more complex model of BK_Ca gating discussed below.

The Effects of the β1 Subunit at Subnanomolar [Ca²⁺]

The above analysis suggests that although the β1 subunit may alter Ca²⁺ binding-site affinities to a small degree (less than twofold in either conformation), much of its steady state effects are on aspects of gating that are separate from Ca²⁺ binding. If this is in fact the case, then some β1 effects should be apparent at subnanomolar [Ca²⁺], where channel gating is Ca²⁺ independent (Meera et al. 1996; Cui et al. 1997). Recently, however, Meera et al. 1996 have found that at subnanomolar [Ca²⁺] there is essentially no change in the hSlo G-V relation upon β1 coexpression. They concluded that at very low [Ca²⁺] there is “functional uncoupling” between α and β subunits. They called this functional uncoupling a “Ca²⁺ switch.” What this means in a physical sense is unclear. One possibility is that the two subunits become physically disassociated at low [Ca²⁺]. We know this is not the case, however, because the β1 subunit has effects on the kinetics of mSlo macroscopic currents that are still evident at subnanomolar [Ca²⁺]. This is illustrated in Fig. 6, and was also pointed out by Meera et al. 1996 (see their Figure 2 C). The other possibility seems to be that β1's steady state effects are solely on Ca²⁺ binding such that at very low [Ca²⁺], where these sites are irrelevant, no change in channel gating is observed. This explanation, however, contradicts the above analysis, which suggested that, at micromolar [Ca²⁺], a large part of the steady state effects of β1 were on aspects of gating separate from Ca²⁺ binding. Furthermore, a recent report indicates that the β1 subunit does have effects on mSlo gating at subnanomolar [Ca²⁺] when examined at the single channel level (Nimigean and Magleby 2000).

To investigate this issue further, we examined mSlo_α and mSlo_{α + β1} currents at subnanomolar [Ca²⁺]. In Fig. 7 A is plotted mean I-V relations determined from current families like those shown in Fig. 6 recorded with 0.5 nM [Ca²⁺]. Each current measurement was made at the end of a 50-ms voltage step to the indicated test voltage. Each I-V curve was then normalized to its value at +200 mV, and the resulting curves were averaged. Two properties of these curves are of interest. First, even in the presence of β1, it takes high voltages to activate the mSlo channel, at least +80 mV. And second, the two curves do not superimpose. The mSlo_{α + β1} I-V curve is more shallow than the mSlo_α curve. If β1 were having no effect on the steady state activation of mSlo, then we would expect the shapes of their I-V curves to be the same. This is true regardless of the absolute degree to which the channels are activated by +200 mV. Thus, there does appear to be an effect of the β1 subunit on mSlo steady state gating at subnanomolar Ca²⁺.

To examine this effect further, we determined mSlo_α and mSlo_{α + β1} G-V curves under these low [Ca²⁺] conditions. This was done by plotting tail current amplitude vs. test voltage for several current families like those shown in Fig. 6. Each plot was then normalized to its value at +200 mV and then multiplied by the following ratio: the current recorded with 0.5 nM [Ca²⁺] at the end of a step to +200 mV, divided by the current recorded with 9.1 μM [Ca²⁺] under the same conditions. This procedure estimates the percentage of channels that are active at +200 mV by comparing currents recorded from the same patch at 9.1 μM and 0.5 nM [Ca²⁺]. It was necessary because at ∼0.5 nM [Ca²⁺] neither G-V curve reached saturation by +200 mV, and higher voltages often caused the patches to break. Mean G-V curves determined in this way are shown in Fig. 7 B. Most evident is a β1-induced reduction in voltage sensitivity. Both curves have been fitted with Boltzmann functions with z values of 0.98 and 0.61 for mSlo_α and mSlo_{α + β1}, respectively. Similar to the observations of Meera et al. 1996, however, the V_1/2 values of these fits are roughly similar (+180 mV for mSlo_∝ and +200 mV for mSlo_{α + β1}), although, statistically, the observed 20-mV difference is significant (P < 0.02).

At first glance, the relatively small and in fact right-shifting effect of the β1 subunit on mSlo's half-maximal activation voltage at subnanomolar [Ca²⁺] seems to suggest that much of its ability to cause leftward G-V shifts at higher [Ca²⁺] is due to its effects on Ca²⁺ binding, and thus not evident in the essential absence of Ca²⁺. Upon reflection, however, it becomes clear that the reduction in apparent gating charge induced by β1 at subnanomolar [Ca²⁺] would, if left unchecked, shift the mSlo_{α + β1} V_1/2 far rightward. Consider, for example, a channel behaving according to Scheme I with a single voltage-dependent step between closed and open. In this case, in the absence of Ca²⁺, the system reduces to two states, and we may write:

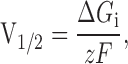6

where ΔG _i represents the intrinsic standard free energy difference between open and closed. Thus, according to , a 50% reduction in z would be expected to cause a twofold increase in V_1/2, and a reduction in z from 0.98 to 0.61, as is observed upon β1 coexpression, would be expected to shift V_1/2 from its mSlo_α value of +180 mV to +294 mV, a difference of 114 mV. We, however, see only a +20-mV rightward shift at subnanomolar [Ca²⁺] upon β1 coexpression. This suggests that some compensating effect must be present. In fact, it suggests that in addition to reducing the channel's voltage sensitivity, β1 decreases ΔG _i. This would act to oppose the right shift expected to accompany a decrease in voltage sensitivity, and thus it could maintain the mSlo_{α + β1} V_1/2 close to that of mSlo_α, while allowing G-V steepness to decrease. Interestingly, however, according to Scheme I, over a range of [Ca²⁺], a decrease in ΔG _i [which amounts to a decrease in the parameter L(0) in Scheme I] would be expected to cause equivalent leftward G-V shifts at each [Ca²⁺], and a decrease in voltage sensitivity would be expected to expand G-V spacing. Thus, together these effects could in fact produce little change in V_1/2 at subnanomolar [Ca²⁺], but large leftward G-V shifts at higher [Ca²⁺]. This is illustrated for the VD-MWC model in Fig. 4 E, and it is qualitatively similar to what is observed in the data (Fig. 1). Thus, our recordings at subnanomolar [Ca²⁺] demonstrate an effect of the β1 subunit on the mSlo G-V relation under this condition, and, in fact, they qualitatively support the analysis above based on recordings with higher [Ca²⁺].

The β1 Subunit Has Little Effect on the Affinity of the Channel for Ca²⁺ when It Is Open

Because Ca²⁺ binding promotes BK_Ca channel opening, thermodynamic principles dictate that the average affinity of the channel's binding sites for Ca²⁺ will be higher when it is open than when it is closed. This suggests that if we make the channel's open probability most energetically sensitive in the absence of Ca²⁺ (that is, bring it to V_1/2) and then see how much Ca²⁺ it takes to just begin to activate the channels further, this critical [Ca²⁺] ([Ca²⁺]_critical) will depend primarily on the affinity of the channel for Ca²⁺ when it is open (K _O in the VD-MWC model). In fact, the mathematics of the VD-MWC model support this conclusion. For the VD-MWC model, the relationship between V_1/2 and [Ca²⁺] is given by . Because K _C is necessarily significantly larger than K _O, when [Ca²⁺] << K_C, may be approximated by:

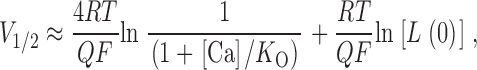7

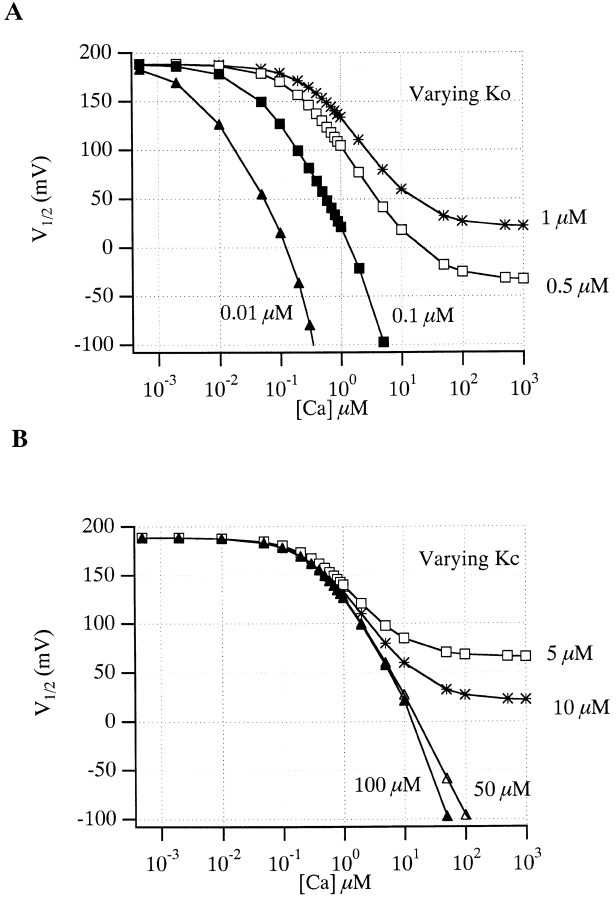
states that at [Ca²⁺] substantially less than K _O (less than ∼5% of K _O, depending on Q), small changes in [Ca²⁺] will not affect V_1/2. This is in fact what is observed experimentally (see below and Meera et al. 1996). As [Ca²⁺] is raised, however, at some point it will become appreciable relative to K _O and V_1/2 will start to move leftward. Furthermore, this [Ca²⁺]_critical will not depend on L(0), which adds a constant term to V_1/2, and it will depend only weakly on Q, which influences the magnitude of the shift but not the ratio of [Ca²⁺] to K _O. This is illustrated graphically in Fig. 8. Here we have calculated V_1/2 vs. [Ca²⁺] plots for the VD-MWC model using parameters similar to those used to fit the mSlo_α data in Fig. 1 A. In Fig. 8 A, the model's open-conformation Ca²⁺ dissociation constant (K _O) is varied and we see large changes in [Ca²⁺]_critical. In Fig. 8 B, however, when K _C is varied, we see very little effect on [Ca²⁺]_critical. Similarly, in Fig. 8 C we also see very little effect on [Ca²⁺]_critical when L(0) or Q are varied. Thus, the [Ca²⁺] at which the VD-MWC model channel's G-V relation just starts to move leftward is determined almost exclusively by its affinity for Ca²⁺ when it is open. As shown in Fig. 11 (below), we have also found this to be true for a more complex BK_Ca gating model.

Figure 8.

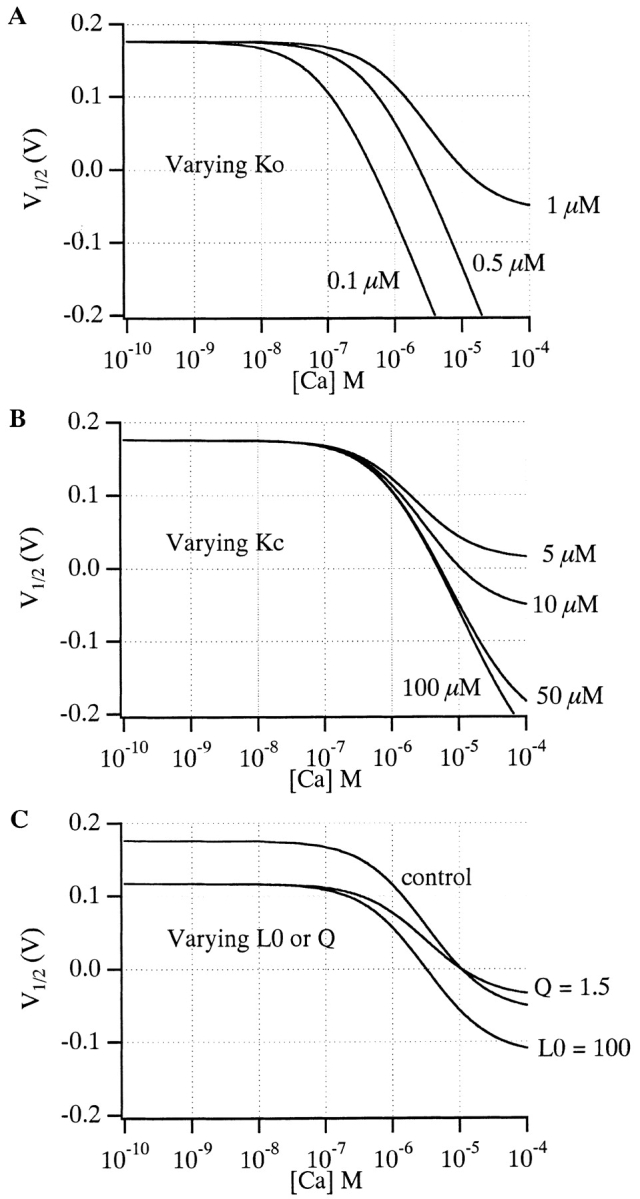
The [Ca²⁺] at which the voltage-dependent MWC model's V_1/2 begins to shift is determined primarily by its affinity in the open conformation. (A) V_1/2 vs. [Ca²⁺] plots calculated from with L(0) = 1,000, Q = 1.0, K _C = 10 μM, and K _O varying as indicated. (B) V_1/2 vs. [Ca²⁺] plots determined from with L(0) = 1,000, Q = 1.0, K _O = 1 μM, and K _C varying as indicated. (C) V_1/2 vs. [Ca²⁺] plots determined from with K _O = 1 μM and K _C = 10 μM, and L(0) or Q as above, or varying as indicated.

Figure 11.

Simulations that demonstrate that [Ca²⁺]_critical for Scheme III, as for Scheme I, depend primarily on the affinity of the model channel when it is open. (A) 50-state-model V_1/2 vs. [Ca²⁺] plots as a function of K _O. A series of G-V relations were simulated with at [Ca²⁺] ranging from 0 to 1,000 μM. V_1/2 for each curve was then plotted as a function of [Ca²⁺]. K _O was varied as indicated and the simulations were repeated. (B) Simulated V_1/2 vs. [Ca²⁺] plots were generated as in A, except K _C rather than K _O was varied. Except where indicated, model parameters were: L(0) = 5e⁵, Q = 0.40, K _C = 10 μM, K _O = 1 μM, Vh _C = 135.9, D = 16.7, Z = 0.51.

While it is difficult to prove this result for all classes of reasonable models, it seems intuitively clear that the concentration at which a ligand begins to allosterically affect a central conformational change will be determined primarily by the affinity of the system when it is most sensitive to ligand given that two requirements are met. (a) In the absence of ligand, the central equilibrium constant is close to 1. For mSlo, we can force this to be true by adjusting the voltage to V_1/2. And (b), there is a substantial difference in affinity between active and inactive forms. This requirement, however, is simply necessary for the ligand to have any appreciable effect at all. With the mSlo channel, therefore, if the β1 subunit is altering the affinity of the channel for Ca²⁺ when it is open, we would expect to see this as a change in [Ca²⁺]_critical.

We have taken two approaches to examining the effects of β1 on [Ca²⁺]_critical. To rapidly determine the effects of [Ca²⁺] on channel activity over a range of voltages, we applied voltage ramps from −150 to +150 mV at the following series of [Ca²⁺]: 0.5, 2, 10, 50, 100, and 660 nM, and 9.1 μM. Data from a patch expressing mSlo_α channels are shown in Fig. 9 A. As is evident, there is very little consistent effect of Ca²⁺ on channel activity at concentrations below 100 nM, a small effect at 100 nM, and then a large effect at 660 nM. The [Ca²⁺]_critical for mSlo_α therefore appears to be close to 100 nM. Interestingly, the same experiment produces a very similar pattern with mSlo_{α + β1} (Fig. 9 B), again no consistent effect below 100 nM, a small effect at 100 nM, and then a large effect at 660 nM. To quantify these results, we measured current amplitudes at +150 mV over the same [Ca²⁺] range for a series of mSlo_α and mSlo_{α + β1} patches, divided each patch's amplitudes by that recorded at 9.1 μM [Ca²⁺], and then plotted this ratio as a function of [Ca²⁺]. The results are shown in Fig. 9 E. For a large number of both mSlo_α and mSlo_{α + β1} patches, [Ca²⁺]_critical appears close to 100 nM, with a large effect of Ca²⁺ at 660 nM. (Fig. 9)

Figure 9.

[Ca²⁺]_critical changes very little with β1 coexpression. (A and B) Current traces recorded from macropatches in response to 400-ms ramps from −150 to +150 mV at the indicated [Ca²⁺] for mSlo_α (A) and mSlo_{α + β1} (B). All traces in A are from the same patch, as are all traces in B. In E, current amplitude at +150 mV recorded with each [Ca²⁺] relative to that recorded at 9.1 μM are plotted as a function of [Ca²⁺]. Plots from 6 mSlo_α (•) and 11 mSlo_{α + β1} (○) experiments are shown. (C and D) G-V relations determined as described with reference to Fig. 7 B for mSlo_α (C) and mSlo_{α + β1} (D). [Ca²⁺] are as indicated. Each curve represents the average of between 2 and 11 experiments as indicated in Table . Each curve has been fitted with a Boltzmann function with the following parameters, in order of increasing [Ca²⁺]. mSlo_α V_1/2 (mV) = 177, 176, 182, 174, 171, 110, 105, and 47; z = 0.90, 0.88, 0.93, 0.94, 0.93, 1.47, 1.57, and 1.28; mSlo_{α + β1} V_1/2 (mV) = 212, 211, 181, 197, 181, 71, 68, and −33; z = 0.62, 0.60, 0.61, 0.62, 0.55, 1.00, 1.00, and 0.98. In F are shown V_1/2 vs. [Ca²⁺] plots for mSlo_α (•) and mSlo_{α + β1} (○). Each point represents the mean of between 2 and 11 experiments, as indicated in Table . Error bars indicate SEM. Smooth curves represent fits to . For the fits, the Q parameter was constrained to the mean value from analysis of all of our 0.5 nM G-V data. Fit parameters were as follows: mSlo_α L(0) = 1,034, Q = 0.99, K _O = 0.84 μM; mSlo_{α + β1} L(0) = 141, Q = 0.64, K _O = 0.68 μM.

To examine more rigorously the effects of the β1 subunit on [Ca²⁺]_critical, we recorded current families at each [Ca²⁺] and determined G-V relations as described above with reference to Fig. 7. The results of Boltzmann fits to these data are listed in Table , and averaged G-V curves are shown in Fig. 9C and Fig. D. Again, as is consistent with published data (Meera et al. 1996), we see very little consistent effect of Ca²⁺ on both mSlo_α and mSlo_{α + β1} G-V relations at low nanomolar Ca²⁺, perhaps a small effect at 100 nM, and then a large effect at 660 nM [Ca²⁺]. Mean V_1/2 values from a series of experiments are plotted for both mSlo_α and mSlo_{α + β1} in Fig. 9 F. Although at 660 nM [Ca²⁺] mSlo_{α + β1} responds with a larger leftward shift than does mSlo_α (likely reflecting mSlo_{α + β1}'s weaker voltage dependence), clearly the Ca²⁺ concentrations at which the two channels begin to respond to Ca²⁺ are essentially the same. This suggests that, when open, the affinity of the channel for Ca²⁺ is minimally affected by β1, or perhaps not affected at all. To estimate K _O for the two channel types, we fit the plots in Fig. 9 F with . These fits yielded K _O estimates of 0.84 and 0.68 μM for mSlo_α and mSlo_{α + β1} channels, respectively. Thus, a small (19%) change in K _O is suggested.

Analysis in Terms of a More Complex Model

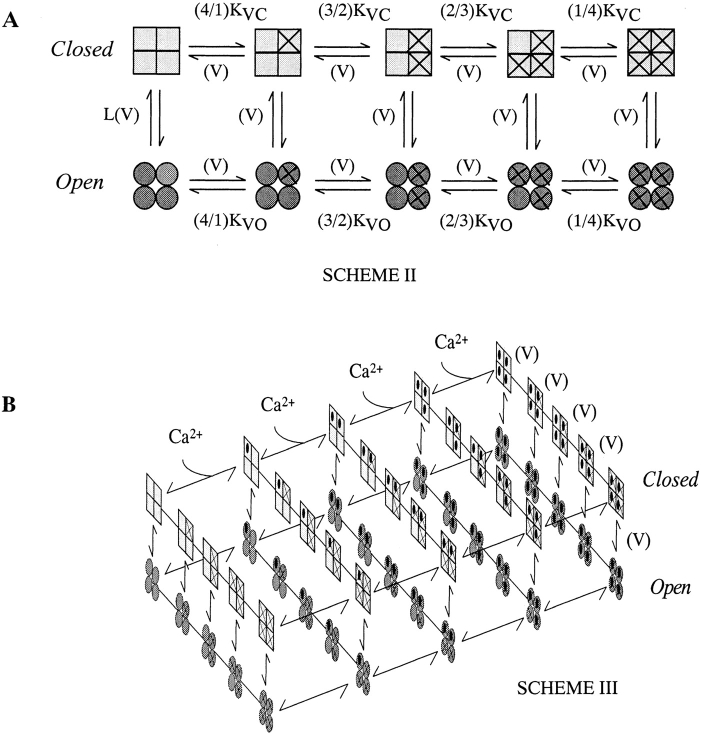
Although Scheme I–based models mimic many properties of BK_Ca channel gating, there are some properties that they do not reproduce. In particular, gating current measurements indicate that the majority of mSlo_α's gating charge moves before channel opening rather with the same time course as opening, as is predicted by Scheme I (Stefani et al. 1997; Horrigan and Aldrich 1999). Also, mSlo_α has a complex relationship between its deactivation time constant and membrane voltage that is inconsistent with this simple scheme (Cox et al. 1997a; Horrigan et al. 1999). Recently, Horrigan et al. 1999 performed a detailed analysis of the macroscopic gating behavior of BK_Ca channels in the essential absence of Ca²⁺. Based on their results, they proposed that, rather than there being just a single voltage-dependent step between opened and closed, voltage sensors in each subunit move rapidly in response to changes in membrane voltage regardless of whether the channel is open or closed, and that this movement, in an allosteric manor, favors but is not required for channel opening (Horrigan et al. 1999; Horrigan and Aldrich 1999). This idea, coupled with the assumption of a weak voltage dependence associated with the central opening conformational change, is represented by Scheme II (Fig. 10 A). Here, horizontal transitions represent voltage sensor movement with an X indicating a voltage sensor in its active conformation, and vertical transitions represent channel opening. Interestingly, Horrigan et al. 1999 showed that Scheme II can account well for the properties of mSlo_α macroscopic gating and ionic currents in the essential absence of Ca²⁺, and they proposed that this scheme, coupled with the allosteric mechanism by which Ca²⁺ affects channel gating in Scheme I, may also account for BK_Ca gating at higher [Ca²⁺]. This idea in its simplest form is represented by Scheme III (Fig. 10 B). In Scheme III, the subset of states represented by the 2 × 5 face at the front is equivalent to Scheme I, and the subset of states represented by the 2 × 5 face at the left edge is equivalent to Scheme II. Scheme III has also been recently proposed by Rothberg and Magleby 1999 to account for the gating of single skeletal muscle BK_Ca channels at high [Ca²⁺].

Figure 10.

(A) Scheme II, allosteric model of Horrigan et al. 1999. Horizontal transitions represent voltage sensor motion in each of four subunits with K _VC and K _VO representing the forward microscopic equilibrium constants for these transitions for the closed and open channel, respectively. Vertical transitions represent the conformation change by which the channel opens. All transitions are hypothesized to be voltage dependent. (B) Allosteric model to account for both the Ca²⁺- and voltage-dependent properties of mSlo gating. This model represents the simplest combination of Schemes I and II. Transitions along the long horizontal axis represent Ca²⁺ binding and unbinding. Transitions along the short horizontal axis represent voltage sensor movement. Transitions from top to bottom represent channel opening. Implicit in this scheme and are the assumptions that voltage sensors and Ca²⁺ binding sites in each subunit are identical and act independently, and that voltage-sensor movement does not directly influence Ca²⁺ binding, and vice versa.

Although Scheme III has many states (50), it is based on the straightforward idea that both Ca²⁺ and voltage allosterically influence a central closed-to-open conformational change. The large number of states arises naturally as a property of gating systems regulated by two stimuli. Despite its many states, the equilibrium behavior of Scheme III is governed by only seven parameters: L(0), the open-to-closed equilibrium constant when no voltage sensors are active and no Ca²⁺ binding sites are occupied; Q, the gating charge associated with this equilibrium; Vh _c, the voltage at which a single voltage sensor is half the time active when the channel is closed; Vh _o, the same for when the channel is open; Z, the equivalent gating charge associated with each voltage sensor's movement; and K _C and K _O, the open and closed Ca²⁺ dissociation constants, respectively. P _open for Scheme III is given by the following function of [Ca²⁺] and voltage:

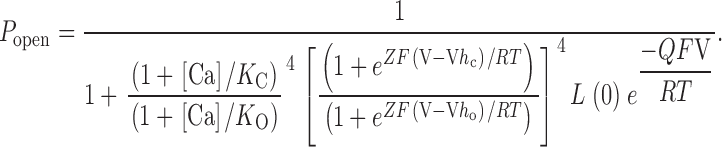8

In keeping with other work (Cox et al. 1997a; Horrigan and Aldrich 1999), we may also define the ratio of Ca²⁺ dissociation constants as C (C = K _C/K _O), and the ratio of voltage sensor equilibrium constants when no voltage is applied as D [D = e⁽ ZF ^V h ^c/ RT ⁾/e⁽ ZF ^V h ^o/ RT ⁾].

Interestingly, although it is not possible to write V_1/2 as an explicit function of [Ca²⁺] for Scheme III, simulations indicate that, as with the VD-MWC model, the concentration at which Scheme III starts to respond to Ca²⁺ is also determined primarily by the affinity of the channel for Ca²⁺ when it is open. This is illustrated in Fig. 11. Here we have constructed V_1/2 vs. [Ca²⁺] plots from G-V curves simulated with a model of the form of Scheme III. The parameters used in these simulations were similar to those used below to fit the mSlo_α data (see below). Varying K _O (Fig. 11 A) has a large effect on [Ca²⁺]_critical, while varying K _C (B) has very little effect. Thus, according to Schemes I and III, the fact that the mSlo channel's [Ca²⁺]_critical changes very little upon β1 coexpression suggests very little or no effect of β1 on the open channel's Ca²⁺ binding affinity.

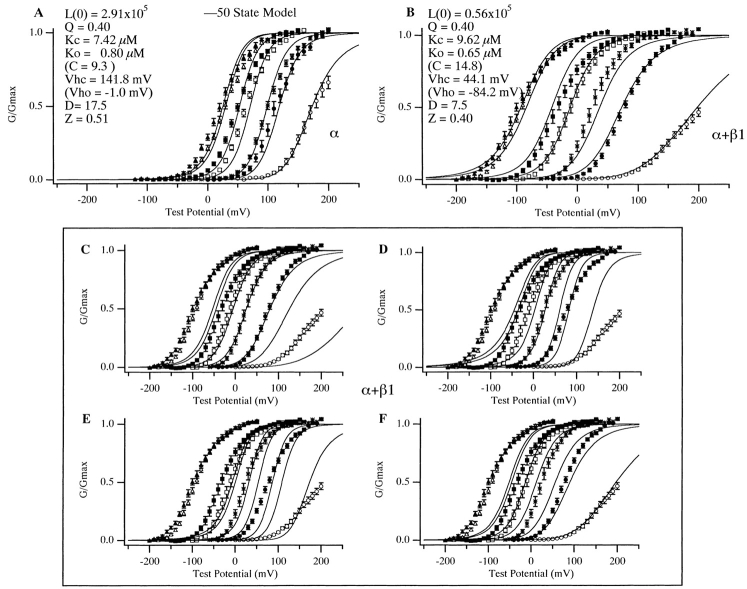
To further examine the effects of the β1 subunit in terms of Scheme III, we fitted mSlo_α and mSlo_{α + β1} G-V data with . For the mSlo_α data, we restricted L(0), Vh _c, D, Q, and Z to a small range of values determined by Horrigan et al. 1999 to reasonably fit mSlo_α gating and ionic current data in the absence of Ca²⁺. We also restricted K _O to be close (within 5%) to the value (0.84 μM) estimated for mSlo_α from the [Ca²⁺]_critical analysis described with reference to Fig. 9. As shown in Fig. 12 A, even with these restrictions, Scheme III can reproduce the shifting of the mSlo_α G-V relation as a function of [Ca²⁺] fairly well. From the parameters of the fit (listed in Fig. 12 A) K _C for mSlo_α is estimated to be 7.4 μM. When both Ca²⁺ dissociation constants were free to vary, a very similar best fit was found (not shown) with K _C equal to 7.3 μM and K _O equal to 0.72 μM.

Figure 12.

50-state model analysis. (A) mSlo_α and (B) mSlo_{α + β1} G-V relations over a series of [Ca²⁺] were fit with as described in the text. The resulting parameters are as indicated on the figure. Those values indicated in parentheses were not parameters of the fit, but rather calculated from the fit parameters. In A, the parameters other than those related to Ca²⁺ binding were constrained to be within the following ranges, which might reasonably fit the data of Horrigan et al. 1999 at subnanomolar [Ca²⁺] (Frank Horrigan, personal communication). L(0) 0.25–1 × 10⁶, Q 0.35–0.45, Vh _c 135–155, D = 10–20, Z = 0.51–0.59. K ₀ was constrained to be within 5% of the value estimated from [Ca²⁺]_critical analysis 0.8–0.88 μM and K _C was unconstrained. In B, K _O was constrained to be within 5% of the value estimated from [Ca²⁺]_critical analysis 0.65–0.72 μM, all other parameters were free to vary. In C–F, various parameters from the fit in B were restored to their values in A. In C, L(0) was restored. In D, the voltage-sensing parameters Q, Z, Vh _c, and D were restored. In E, L(0), Q, Z, Vh _c, and D were restored. In F, K _O and K _C were restored. In each panel, G-V relations at the following [Ca²⁺] are shown: from right to left, 0.0005, 0.99, 1.86, 4.63, 9.1, 39, and 74 μM. Error bars indicate SEM.

We next fit the G-V data acquired with the β1 subunit present. In doing so, we constrained only K _O, again keeping it close to (within 5%) the value (0.68 μM) estimated for mSlo_{α + β1} from [Ca²⁺]_critical analysis. As shown in Fig. 12 B, over the [Ca²⁺] range ∼1–75 μM, the 50-state model and the VD-MWC model fits are quite similar (compare with Fig. 1 B, dashed curves). This is perhaps not surprising as the mechanism by which Ca²⁺ binding affects channel opening is the same for the two schemes. The more complex voltage-dependent gating mechanism of the 50-state model, however, also allows it to account for the prominent change in G-V steepness evident in Fig. 12 B between 0.0005 and 0.99 μM [Ca²⁺]. This is something that is also evident without β1 coexpression (see Fig. 12 A and Cui et al. 1997), but is most pronounced when β1 is present. Scheme I–based models cannot account for changes in G-V steepness with changing [Ca²⁺] because they contain all of their gating charge in the central close-to-open conformational change.

Most interesting, however, is the mechanism by which the 50-state model accounts for the β1 subunit's steady state effects. As expected based on the preceding analysis, it decreases L(0), which reflects in part the intrinsic free energy difference between open and closed, and it decreases its voltage sensitivity (decreasing its gating charge z and altering Vh _o and Vh _c), while changing its Ca²⁺ dissociation constants very little (K _O moves from 0.8 to 0.65 μM, and K _C moves from 7.4 to 9.6 μM). Also, we found that it was not possible to achieve any sort of reasonable fit to the mSlo_{α + β1} G-V data if only K _O and K _C were allowed to vary freely while the parameters not related to Ca²⁺ binding were constrained to those used to fit the mSlo_α data. Thus, analysis of our data in terms of Scheme III, as with Scheme I, suggests that although the β1 subunit increases the mSlo channel's apparent affinity for Ca²⁺, much of this effect is via its action on aspects of gating that are separate from, but energetically coupled to, Ca²⁺ binding.

To examine the extent that changes in various aspects of gating account for β1's effects, we restored various 50-state model parameters from the mSlo_{α + β1} fit to their value in the mSlo_α fit and recalculated the model G-V curves. We did this without redoing the fitting. The effects of such changes are shown in Fig. 12C–F. Clearly, restoration of both the model's L(0) value (Fig. 12 C) and its voltage-sensing parameters (D), as well as both together (E), severely disturbed the original mSlo_{α + β1} fit. In fact, qualitatively, the effects of changing these aspects of gating are similar to what is predicted by Scheme I (Fig. 4). Increasing L(0) shifts all G-V curves rightward (Fig. 12 C), while increasing the model's voltage sensitivity increases G-V steepness and collapses their spacing (D). Thus, in these respects, Schemes I and III behave similarly.

We also restored just the model's Ca²⁺ dissociation constants to their values in the mSlo_α fit. Interestingly, this also substantially disturbed the model's fit to the mSlo_{α + β1} G-V curves (Fig. 12 F). Not at very low [Ca²⁺], but as [Ca²⁺] is increased, the model's G-V spacing becomes too narrow and the fit becomes very poor. Also, when we did redo the fitting, but restricted K _C and K _O to the values used in the mSlo_α fit, a good fit to the mSlo_{α + β1} G-V data was not found. Thus, while both Schemes I and III suggest only small changes in the channel's Ca²⁺ dissociation constants with β1 coexpression, the small changes that do occur appear to be important.

It is not clear, however, whether both K _C and K _O need to change to account for β1's effects, as we were able to find reasonable fits to the mSlo_{α + β1} G-V data when either one, but not both, of these parameters were held at their mSlo_α G-V fit values. What appeared necessary to fit the data, however, was an increase in the ratio K _C/K _O (Fig. 12 C) from ∼9.3 for mSlo_α to ∼14.8 for mSlo_{α + β1}. Also, the standard errors of the fit parameter K _C for the fits shown in Fig. 12A and Fig. B, (0.5 μM for the mSlo_α fit and 0.6 μM for the mSlo_{α + β1} fit, as determined by the curve fitting software) are small, relative to the K _C estimates: <10%. This also suggests that the change in Fig. 12 C that Scheme III uses to account for β1's effects is required. Interestingly, this change corresponds to a change in the standard free energy difference between open and closed at saturating ligand of only 4.6 kJ/mol.

DISCUSSION

BK_Ca channels are found in a wide variety of tissues where they are most often thought to provide feedback control over processes that involve membrane depolarization and a rise in intracellular Ca²⁺ (Latorre et al. 1989). One place they do this is at nerve terminals. Here, Ca²⁺ entry through nearby Ca²⁺ channels, in combination with membrane depolarization, results in rapid activation of BK_Ca currents that then contribute to the repolarization of the action potential (Robitaille and Charlton 1992; Robitaille et al. 1993a,Robitaille et al. 1993b). Thus, neuronal BK_Ca channels are likely optimized for speed rather than sensitivity as Ca²⁺ concentrations in close proximity to Ca²⁺ channels are thought to rise as high as 100 μM (Roberts 1994), a concentration that according to Fig. 1 is sufficient to achieve a P _open of ∼0.5 at the peak of the action potential (approximately +20 mV). BK_Ca channels also play a central role in the control of smooth muscle contractility. In vascular smooth muscle, brief puffs or “sparks” of Ca²⁺ are released periodically from the sarcoplasmic reticulum. These sparks, which are thought to achieve Ca²⁺ concentrations near the plasma membrane in the tens of micromolar (Perez et al. 1999), activate nearby BK_Ca channels on the plasma membrane, and the ensuing hyperpolarization leads to muscle relaxation (Nelson et al. 1995; Nelson and Quayle 1995; Perez et al. 1999). In this tissue, however, the membrane voltage seldom exceeds −20 mV and typically rests near −40 mV (Nelson and Quayle 1995). Thus, for vascular smooth muscle BK_Ca channels to be similarly activated, they must be sensitive to Ca²⁺ at lower voltages than their neuronal counterparts.

To achieve this increase in sensitivity, nature apparently has evolved an auxiliary BK_Ca β subunit (β1) that shifts the BK_Ca channel's G-V relation −70 to −80 mV, into the physiological voltage range at concentrations of 5 or 10 μM. This shift increases the apparent Ca²⁺ affinity of these channels at many voltages. It has been the goal of this study to elucidate in a biophysical sense the mechanism by which the β1 subunit exerts this powerful effect, and in particular to determine whether the apparent increase in Ca²⁺ affinity evident upon β1 coexpression is due to a real increase in Ca²⁺ binding site affinity.

Perhaps the most straightforward approach to this question would be to measure the binding of Ca²⁺ to the channel. The high nonspecific binding associated with attempting to measure ⁴⁵Ca²⁺ binding to a low affinity integral membrane protein, however, renders this approach, at present, technically unfeasible. We have therefore relied on electrophysiological measurements. These measurements, however, are necessarily indirect as they measure channel opening rather than Ca²⁺ binding, and therefore require interpretation. Binding measurements, if achieved, however, would also require some mechanistic framework for their interpretation, as such measurements may also be influenced by aspects of gating that are separate from, but linked to, Ca²⁺ binding (Wyman and Gill 1990).

To aid us in the interpretation of our electrophysiological data, we have relied on two models of BK_Ca channel gating that have been developed after extensive examination of the macroscopic gating behavior of the mSlo_α channel. The first is Scheme I and the special case of Scheme I that corresponds to the voltage-dependent MWC model. While, as we have discussed (Cox et al. 1997a; Horrigan and Aldrich 1999; Horrigan et al. 1999), this system is too simple to account for some of the voltage-dependent properties of BK_Ca channel gating, it can recreate the synergistic relationship between Ca²⁺ and voltage as they act together to activate the BK_Ca channel over a wide range of [Ca²⁺] and voltage. Furthermore, the VD-MWC model is sufficiently simple that it allows one to derive general principles about the ligand-dependent allosteric regulation of voltage-sensitive equilibria. The first of these principles is that an alteration in the intrinsic energy difference between open and closed (ΔG_i) can indeed cause large G-V shifts. This is perhaps intuitively clear, and readily understandable as simply due to a change in the energy the voltage-sensing mechanism needs to apply to the central conformational change to affect opening. An important characteristic of these shifts reflected by the VD-MWC model, however, is that as long as the channel's voltage sensitivity remains constant with [Ca²⁺](as is necessarily the case for Scheme I) the magnitude of the shift will not vary with Ca²⁺. That is, the spacing of the G-V curves will be maintained. Thus, on a V_1/2 vs. [Ca²⁺] plot, a simple V_1/2 offset will be observed. We, however, see a change in V_1/2 vs. [Ca²⁺] slope as well as position in Fig. 1 upon β1 coexpression. This suggests that the β1 subunit is doing more than just changing the equilibrium constant between open and closed.

The second general principle we may derive from the VD-MWC model is that the spacing of a BK_Ca channel's G-V relations determined over a series of [Ca²⁺] is likely to be inversely proportional to the voltage sensitivity of the channel (Cox et al. 1997a; Cui, J., and R.W. Aldrich, manuscript in preparation). The more shallow the channel's voltage dependence, all other things being equal, the wider the spacing. We saw this in Fig. 4 for Scheme I and in Fig. 12 for Scheme III. This phenomenon is readily understandable as being due to the fact that as the channel's voltage sensitivity decreases, it takes a larger change in the transmembrane electrical field to apply the same amount of energy to the closed-to-open conformational change. Thus, an increase in the steepness of a channel's V_1/2 vs. [Ca²⁺] plot is not necessarily indicative of an increase in Ca²⁺ binding affinity. For this reason, in our analysis we have multiplied the channel's effective gating valence at each [Ca²⁺] by its V_1/2 and plotted this value as a function of [Ca²⁺]. As indicated by , this results in a plot whose shape, for models of the form of Scheme I, is only sensitive to changes in Ca²⁺ binding. Applying this test to the effects of the β1 subunit, we have found that the z*V_1/2 vs. [Ca²⁺] plots for mSlo_α and mSlo_{α + β1} are more nearly parallel than are the V_1/2 vs. [Ca²⁺] plots, thus suggesting that some of mSlo_{α + β1}'s expanded G-V spacing is due to its general decrease in voltage sensitivity. Analysis of the two curves in Fig. 5, however, indicates that there are differences in shape between the mSlo_α and mSlo_{α + β1} z*V_1/2 vs. [Ca²⁺] plots, and thus some of the increased spacing of the mSlo_{α + β1} G-V curves is likely due to effects of β1 on Ca²⁺ binding.

A third principle we may derive from the VD-MWC model is that the spacing of the BK_Ca channel's G-V relation as a function of [Ca²⁺] will likely change if the model's Ca²⁺ dissociation constants in the open or closed configuration are altered. This is evident from , which shows that δV_1/2/δ[Ca²⁺] depends in a complex way on both K _C and K _O, and this derivative is an even more complex function of K _C and K _O for Scheme III. In either case, however, the complexity of this relationship makes it difficult to make general predictions about how G-V spacing is likely to change in response to changes in Ca²⁺ binding constants without specifying the exact nature of the change. One important general principle of such ligand-gated voltage-sensitive systems, however, is that the power of a ligand to shift the channel's G-V relation along the voltage axis [that is, the maximum change in V_1/2 that a ligand can produce (ΔV_1/2max)], is dependent on the change in affinity that occurs upon channel opening, rather than the absolute affinities of the closed or open channel. In fact, for the VD-MWC model in the limit of saturating [Ca²⁺], we may write:

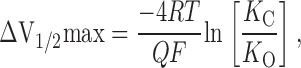9

which states that ΔV_1/2max varies as the logarithm of the ratio K _C/K _O. An important result of this fact is that large leftward G-V shifts at high [Ca²⁺] can occur if either K _O is reduced or K _C is increased. In the later case, the channel would in fact bind Ca²⁺ with lower affinity in the closed conformation and yet affect leftward G-V shifts. Furthermore, such shifts will increase the channel's Ca²⁺ sensitivity, particularly under conditions where multiple Ca²⁺ ions normally need to bind before the channel is appreciably open. Thus, an increase in a channel's apparent Ca²⁺ binding affinity can be the result of a true decrease in binding affinity. In fact, analysis of our data suggests that, among other things, the BK_Ca channel's K _C/K _O ratio must change from ∼9.3 to ∼14.9 to account for β1's effects, and that simply a small increase in K _C may well be responsible. Interestingly, this change in K _C/K _O corresponds to a change in the standard free energy difference between open and closed at saturating ligand of only 4.6 kJ/mol, less than the energy of a single hydrogen bond. For a weakly voltage-dependent channel such as mSlo (Q ∼ 1.2), however, this small energy change converts to a 40-mV change in ΔV_1/2max. Thus, even quite small changes in the K _C/K _O ratio can have substantial effects on the power of Ca²⁺ to shift the channel's G-V relation leftward and thus affect an apparent increase in Ca²⁺ affinity.

The general principles just described illustrate the complexity of systems containing binding coupled to conformational changes, and they underscore the futility of interpreting results of structural, modulatory, or pharmacological alterations in such cases without the aide of an appropriate and well-tested mechanistic model.

We have also examined the effects of the β1 subunit on the mSlo G-V relation in the essential absence of Ca²⁺. A previous report suggested that there is a functional uncoupling between the hSlo channel and the human β1 subunit under these conditions such that β1 has no effect on the channel's G-V relation (Meera et al. 1996). Similar to this observation, we have found that the ability of β1 to shift the mSlo G-V relation is greatly reduced at subnanomolar [Ca²⁺]. Inconsistent with this report, however, we have observed a 20-mV rightward G-V shift induced by the β1 subunit at subnanomolar [Ca²⁺] and a 38% reduction in apparent gating charge. Furthermore, we show that these results can be accounted for if we suppose that coupled with the decrease in voltage sensitivity is a decrease in ΔGº_i (see Fig. 4 E). Since this explanation is consistent with our overall analysis, we greatly favor it over an uncoupling mechanism, particularly as the kinetic effects of β1 remain quite evident at subnanomolar [Ca²⁺], and effects of β1 on mSlo gating have been observed under this condition at the single channel level (Nimigean and Magleby 2000). While species differences may account for the differences between our results and those of Meera et al. 1996, it may also be that the limited voltage range used in their study obscured the effects we have observed. The kinetic effects of β1 at subnanomolar [Ca²⁺], however, were clearly evident in both studies.

One advantage in studying the regulation of BK_Ca channel gating over other ligand-gated channels is the additional sensitivity of the BK_Ca channel to voltage. This allows the experimenter to manipulate the equilibrium between open and closed to a point where it is most sensitive to ligand binding, its V_1/2, and then determine how much ligand it takes to begin to activate the channels further. We have argued that this critical [Ca²⁺] is reflective of the affinity of the channel when it is open. To examine [Ca²⁺]_critical, we have looked for changes in BK_Ca currents at high voltages as we increased [Ca²⁺], and have found that, consistent with published data (Meera et al. 1996), both mSlo_α and mSlo_{α + β1} channels begin to respond to [Ca²⁺] at the same concentration, close to 100 nM. Thus, the β1 subunit does not appear to substantially alter the mSlo channel's Ca²⁺ binding affinity when it is open.

In a similar experiment, we have also looked at the point where the mSlo_α and mSlo_{α + β1} channels' G-V relations just start to shift with [Ca²⁺]. We show, mathematically () for the VD-MWC model and graphically for the VD-MWC and the 50-state models (see Fig. 8 and Fig. 11), that this Ca²⁺ concentration depends almost exclusively on the affinity of the channel for Ca²⁺ when it is open. To be strictly correct, however, it should be stated that the channel's voltage sensitivity can also affect this critical concentration. This is because the size of the shift observed once the channel does start to respond to Ca²⁺ will be inversely related to voltage sensitivity, and this may influence the ability of the experimenter to detect the shift. We have accounted for changes in voltage sensitivity in our analysis, however, by restricting the value of z in our fits to the channel's V_1/2 vs. [Ca²⁺] plot around [Ca²⁺]_crtical (Fig. 9 F) to the value that best fit the G-V at subnanomolar [Ca²⁺] (the data displayed in Fig. 7 B). An alternative approach would be to look at the point where the channel's z*V_1/2 vs. [Ca²⁺] just starts to respond to [Ca²⁺]. For the VD-MWC model, this point is independent of Q. Clearly, this type of analysis could also be used to determine whether alterations in BK_Ca channel function brought about by mutation, phosphorylation, or chemical modulation alter the affinity of the BK_Ca channel for Ca²⁺ when it is open.

To confirm and extend our analysis based on Scheme I, we have also fit our data with Scheme III. This scheme is identical in general form to that which Rothberg and Magleby 1999 proposed to best describe the Ca²⁺-dependent properties of single skeletal muscle BK_Ca channels at +30 mV. It is also a straightforward extension of Scheme II, which, after extensive investigation, Horrigan et al. 1999 found could account well for the gating and ionic current properties of mSlo_α at subnanolmolar [Ca²⁺]. They proposed that combining Scheme II with the mechanism by which Ca²⁺ affects channel gating in the MWC model to produce Scheme III might account also for mSlo_α's Ca²⁺-dependent behavior. Remarkably, we have found that we could reproduce the Ca²⁺-dependent shifting of the mSlo_α G-V relation over a wide range of [Ca²⁺], and as well a change in G-V slope from very low to moderate [Ca²⁺], while maintaining the voltage-sensing parameters (Q, Z, Vh _c, and D) and L(0) of the 50-state model essentially as described by Horrigan et al. 1999. In fact, this was also the case if we restricted K _O to the value estimated from our [Ca²⁺]_critical analysis such that only K _C was free to vary. We consider this confirmation of the general theory that Scheme III represents, although clearly further complexities may occur that are not represented by and therefore are not included in the form of this scheme. Such complexities might include Ca²⁺ binding steps of differing affinities in either the open or closed conformation, or direct interactions between voltage sensors and Ca²⁺ binding sites. Even without such considerations, however, the added complexity of Scheme III's voltage-sensing mechanism makes it more difficult to derive general principles for its behavior than it is for Scheme I. Simulated changes in various aspects of gating, however, indicate that this system behaves qualitatively like Scheme I (Fig. 12), thus validating our use of Scheme I in our preliminary analysis. This is perhaps not surprising since, although Scheme III's voltage-sensing mechanism is more complex than Scheme I's, Scheme III still represents a voltage-sensitive central conformational change allosterically regulated by ligand binding. Furthermore, we have found that both Schemes I and III suggest the same mechanism by which the β1 subunit alters the mSlo channel's steady state gating behavior: a decrease in ΔGº_i, a reduction in gating charge, and a subtle but important change in Ca²⁺ binding affinity. Horrigan et al. 1999 performed a detailed experimental analysis of gating and ionic currents at subnanomolar [Ca²⁺], which allowed them to estimate Q, Z, Vh _C, D, and L(0) for the mSlo_α channel. Without performing a similar analysis, we would not wish to rely on the estimates of these parameters suggested for the mSlo_{α + β1} channel by the fit shown in Fig. 12 B. We do think, however, that the broad [Ca²⁺] range we used in our experiments is sufficient to provide reasonable estimates of the mean Ca²⁺ dissociation constants for both mSlo_α and mSlo_{α + β1} under the conditions of our experiments. For mSlo_α, these estimates are: K _O = 0.8 μM and K _C = 7.4 μM; and, for mSlo_{α + β1}, they are: K _O = 0.65 μM and K _C = 9.6 μM.

It should be pointed out, however, that the mean V_1/2 and z values we report here for mSlo_α are somewhat different from those we have published previously at similar [Ca²⁺] (Cui et al. 1997). The mean V_1/2 values from our current data are on average 5–20 mV right-shifted compared with our previous report, and the mean z values are 10–30% smaller. We do not know the origin of these differences. We believe, however, that they are not due to random sampling, as the large number of experiments included in both studies render the estimates of mean V_1/2 and z reported here, and previously, precise to a standard error of <5 mV (V_1/2) and a charge of 0.1 (z). More likely, the differences are systematic, due to differences between the solutions in the two studies (we have reduced the K⁺ concentration in the internal and external solutions from 142 to 82 mM for the current study), the source or condition of the oocytes employed, or some other unknown aspects of the experimental set up. For the comparisons we have made in the present study, however, we do not believe such systematic variation to be an issue, as the mSlo_α and mSlo_{α + β1} data we report here were acquired with the same solutions, using the same experimental setup, in many cases from the same batches of oocytes. Thus, the experiments described here were specifically designed to compare mSlo_α and mSlo_{α + β1} currents, and their results are better compared with one another than with previous work.

The first β1 subunit to be isolated and characterized was bovine (Knaus et al. 1994a), and it is this subunit that we used in our experiments. During the course of this study, however, additional mammalian β1 subunits were cloned, including those from mouse and human (Dworetzky et al. 1996; Tseng-Crank et al. 1996; Jiang et al. 1999). Mouse and human β1 are ∼83% identical to one another and between 81%(mouse) and 86% (human) identical to bovine β1. Preliminary experiments we have done with mouse β1 indicate that its effects on mSlo gating are very similar to bovine β1 at micromolar [Ca²⁺], and that it produces a somewhat larger rightward G-V shift at subnanomolar [Ca²⁺]. This difference, however, is not likely to affect the main conclusions of this study.

Recently, Nimigean and Magleby 1999, Nimigean and Magleby 2000 examined the effects of bovine β1 on mSlo gating at the single-channel level. They concluded that the β1 subunit exerts its effects mainly by increasing the time the channel spends in bursting states ∼20-fold, and that this effect is evident even in the absence of [Ca²⁺]. If we suppose, as has been suggested by Rothberg and Magleby 1998, that the flickers closed that occur during a burst are to states outside the main gating structure of the channel, then the observations of Nimigean and Magleby 1999, Nimigean and Magleby 2000 fit with our observations. In this case, retention of the channel in bursting states could be accounted for by a reduction in ΔG _i, as our work suggests. Because plots of V_1/2 vs. [Ca²⁺] are not parallel for the mSlo_α and mSlo_{α + β1} channels, however, our results suggests that the β1 subunit also affects other aspects of gating.

In conclusion, results of our analysis of the mSlo_α and mSlo_{α + β1} G-V relation as a function of [Ca²⁺] suggests that the β1 subunit exerts its steady state effects by decreasing the intrinsic energy difference between open and closed conformations, thereby shifting the mSlo G-V curve at all [Ca²⁺] leftward, and by decreasing the channel's voltage sensitivity, which expands G-V spacing as a function of [Ca²⁺]. In addition, subtle but important changes to one or more of the channels' Ca²⁺ dissociation constants are required, changes that increase the power of Ca²⁺ to shift the G-V relation upon binding, an effect that is most pronounced at high [Ca²⁺]. This work should help provide a framework by which to interpret experiments designed to determine in a molecular sense how the β1 subunit makes these biophysical changes.