Abstract
The activity of 36 pairs of single motor units were recorded with intramuscular wire electrodes from m. extensor carpi radialis while subjects performed slow wrist extension and flexion movements. Periods of steady position holding were interposed between movements.
The discharge trains from pairs of motor units were analysed statistically in the time and frequency domains. During extension movements, when the muscle recorded from was the agonist, coherence between motor units was significant below 12 Hz, with a peak at 6–12 Hz in 30 of 36 pairs (83 %). The magnitude of coherence decreased during position holding compared to movements in 26 pairs, while the difference in average firing rate was small.
During movements, but not during position holding, coherence estimates between single motor units and acceleration showed a significant peak at 6–12 Hz in 56 out of 62 motor units, suggesting that a modulation of motor unit discharge contributed to angular acceleration at these frequencies. Common motor unit modulation was present at 3 Hz as well, although the coupling between motor unit activity was weaker than at 6–12 Hz.
It is concluded that a 6–12 Hz common modulation of agonist motor units is a distinguishing feature of slow voluntary wrist movements, extending the previously established notion of an 8–10 Hz rhythmic organization of slow finger movements to more proximal limb segments. It is suggested that the 6–12 Hz input is specific for movements and is normally absent or much weaker during steady maintenance of position or force.
Recurring velocity peaks with a frequency of 8–10 Hz are a common and prominent feature of slow finger movements in healthy humans (Vallbo & Wessberg, 1993), and it has been demonstrated that surface EMG and motor unit discharges from the common finger extensor muscle are correlated with angular acceleration at 8–10 Hz, suggesting a widespread correlation of motor units both in agonist and antagonist muscles (Wessberg & Vallbo, 1996; Wessberg & Kakuda, 1999). As a hypothesis, it has been proposed that this reflects the organization of motor output for voluntary slow movements in man, namely that such movements are executed in a pulsatile fashion, with a rhythmic modulation alternating between 8 and 10 Hz of the descending motor command to the agonist and antagonist muscles. However, comparable data are lacking for movements in joints where the limb has larger inertia than the fingers.
The properties of common inputs to the motoneurone pools in man have been investigated by the method of simultaneous recording of the activity of pairs of motor units, and statistical analysis of cross-correlation in the time domain (Kirkwood & Sears, 1991). The presence of rhythmic components in such common inputs has been revealed by Fourier-based frequency-domain analyses during isometric contraction (Rosenberg et al. 1989; Farmer et al. 1993), and during position holding, when the finger or wrist exhibits physiological tremor (Elble & Randall, 1976; Halliday et al. 1995).
The purpose of the present study was to investigate if agonist motor units are correlated during voluntary wrist movements, and to compare correlation during movements to that during steady position holding. Using fine wire electrodes, pairs of motor units were recorded, and a Fourier-based frequency-domain analysis was used to study the common rhythmic components of motor unit correlation during movements and position holding. It will be shown that the activity of pairs of motor units was correlated during movements, particularly at 6–12 Hz, and that correlation decreased or disappeared during steady contraction during position holding. A preliminary report of some of this work has been published in abstract form (Kakuda & Nagaoka, 1996).
METHODS
Subjects
Twelve experiments were performed on 11 healthy volunteers, four males and seven females, aged 22–45 years. All subjects, except one, were right-handed. They were medical professionals at the National Rehabilitation Centre for the Disabled, Tokorozawa, Japan and gave informed consent according to the Declaration of Helsinki. The study was approved by the ethical committee at the same institute.
Experimental set-up
The subjects sat comfortably in a reclining chair, with the left forearm supported on a platform, and the wrist clamped in mid-position between supination and pronation. The hand was fixed to a manipulandum that limited movement at the wrist joint to flexion-extension. The weight of the manipulandum was 250 g. It was connected to a servo-controlled torque motor that produced a constant torque load. Transducers of the servo system provided continuous measurement of joint angle, velocity, acceleration, and torque. All movements were performed in an isotonic condition with zero or low torque (0–0.1 N m) opposing wrist extension. A computer screen in front of the subject provided visual feedback to the subject. The upper vertical line indicated the desired position, and the lower line showed the subject's actual performance.
Surface electromyography (EMG) was recorded with two electrodes placed over the muscle belly of m. extensor carpi radialis brevis, which was located by palpation and surface electrical stimulation. Intramuscular EMG was recorded with flexible wire electrodes. Two strands of wires were inserted into a 27-gauge injection needle. The wires were cut at straight angles with a sharp wire cutter, and the distal 2–3 mm of the wires were bent over the tip of the injection needle. The muscle was impaled with two needles at a distance of several millimetres, close to the motor point as revealed by surface electrical stimulation. The needles were removed, and each pair of wires was adjusted by hand in small steps until the activity of two motor units could be recorded simultaneously with the two pairs of wires. Recruitment threshold was defined as the joint torque at which a motor unit consistently started to discharge steadily during slowly increasing voluntary contractions.
The kinematic signals were sampled at 400 Hz. Surface EMG was filtered at 1.6–800 Hz and sampled at 1600 Hz. It was digitally rectified and low-pass filtered off-line (moving average with a triangular weighting function over successive 30 bins; −6 dB cut-off at 44 Hz). Intramuscular signals were sampled at 6.4 kHz using the SC/ZOOM system (Department of Physiology, Umeå University, Sweden). Discrimination of single motor units was achieved on-line with a digital time-frequency window algorithm originally developed for the discrimination of single unit nerve discharges in microneurography recordings (Edin et al. 1988). This procedure was repeated off-line using the ZOOM/SC system. Each recorded motor unit discharge was inspected on an expanded time scale, and the invariance of the shape and regularity of firing was confirmed. Files of spike times were generated from the motor unit spike trains to allow them to be considered point processes.
Experimental protocol
The main experimental task comprised a wrist extension-flexion movement under direct visual tracking. A single trial consisted of five phases, as indicated by the command signal in Fig. 1: a position-holding phase, an extension movement of 15 deg amplitude with 5 deg s−1 overall velocity, position holding at the extended joint position for 5 s, a flexion movement with the same speed back to the starting position, and a final position-holding phase. Subjects were urged to make the movements with maximum precision and smoothness. Movements were made around a straight wrist position, corresponding to a joint angle of 180 deg.
Figure 1. Raw single motor units and kinematic data recorded during ramp-and-hold wrist extension.
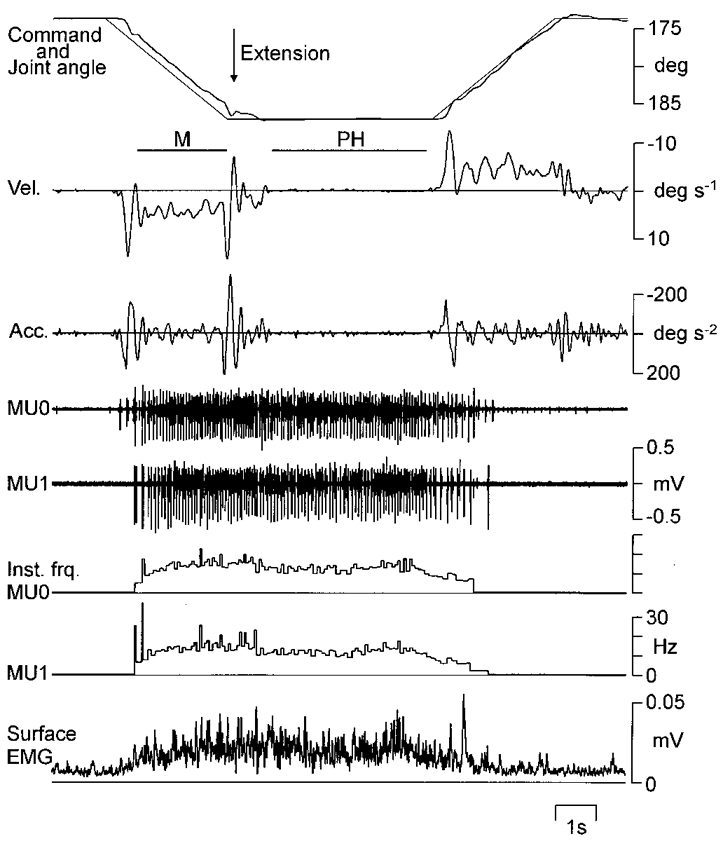
A sample trial of a slow wrist extension-flexion movement acquired by direct visual tracking. Records from above: command signal and joint angle, angular velocity (Vel.), acceleration (Acc.), intramuscular recordings of two motor units (MU0 and MU1), the corresponding instantaneous firing rates of the motor units (Inst. frq.), and rectified surface EMG from m. extensor carpi radialis. Horizontal bars indicate periods of movement (M) and position holding (PH) data selected for statistical analyses.
Because the subjects' execution of the task in many cases was slightly delayed with respect to the movements of the visual target (see Fig. 1), a number of criteria based on joint angular velocity were used during analysis to further define periods of movement and position holding: the periods of movement were defined as the period during the movement of the visual target where joint angular velocity steadily remained above or below zero. Hence, start and end points were defined as the zero crossings of the velocity limiting this period. The starting point for periods of position holding was defined as the point after the target had stopped moving and joint position remained steady. Small symmetrical deviations around the steady position were allowed, with peak velocity of no more than 1 deg s−1. The end point was when the target again started to move. In Fig. 1, the selection periods are indicated by the bars denoted as M and PH.
Data analysis
All recorded data for ongoing extension movements where two single motor units were successfully recorded simultaneously were combined for the statistical analyses. Data recorded for the same units during periods of position holding in the extended joint position were similarly combined. For the analysis, spike trains of individual motor units were regarded as realisations of stationary stochastic point processes, and the association between the two motor units was analysed by estimating the cross-intensity function and the sample coherence. Kinematic signals and rectified surface EMG were regarded as realisations of stationary time series, and were similarly analysed in the time and frequency domains (Bendat & Piersol, 1986; Rosenberg et al. 1989; Halliday et al. 1995).
The cross-intensity function for the time-domain association between two spike trains was obtained from the cross-correlation histogram, estimated for up to 100 ms on either side of the reference spike, according to the procedure described by Brillinger (1976) and Farmer et al. (1993). The cross-intensity function is proportional to the probability of a discharge from one motor unit spike train occurring in a particular time interval relative to the occurrence of a reference spike from the other train. The size of any peak around time zero was expressed as the index denoted by E/M, where M is the mean counts per bin in the control region from up to 50 ms preceding the central peak, and E is the number of counts in the peak above the expected count by chance. This index is sensitive to bin width but not to peak shape or duration (Harrison et al. 1991) and was here used for comparison of the strength of motor unit synchronization between movement and position-holding phases. The duration of the peak was defined by the time interval between inflections of the cumulative sum (cusum) constructed from the cross-correlation histogram (Davey et al. 1986).
The methods for frequency-domain analysis have been described in detail by Rosenberg et al. (1989), Farmer et al. (1993) and Halliday et al. (1995). Selected data were divided into disjoint (non-overlapping) sections of 1.28 s duration. No tapering or weighting function was used. Spike trains and time series data from each disjoint section were Fourier-transformed, giving a frequency resolution of 0.78 Hz. Auto- and cross-spectra were estimated by averaging over the disjoint sections, and coherence estimates for two jointly recorded signals were computed. The sample coherence indicates the degree of linear correlation in the frequency domain between two signals on a scale from zero to one. The statistical analysis of coherence estimates is described in Rosenberg et al. (1989). A statistically significant coherence indicates that the two signals are linearly coupled above chance level at a particular frequency. Cross-covariance functions between two jointly recorded signals were obtained by inverse Fourier transform of the cross-spectra, complementing the cross-intensity function for investigating the time relation between two signals (Bendat & Piersol, 1986). In order to facilitate comparisons, the sampling rate of the kinematic signals, 400 Hz, was used in all analyses. This is equivalent to a 2.5 ms bin width; this temporal resolution was deemed sufficient for the analyses made in the present study.
To compare the sample coherence from the same signals in two different conditions, tanh−1|RA|-tanh−1|RB| was calculated, where |RA| and |RB| are the estimates of the absolute coherency, equivalent to the square roots of the sample coherence estimates in each condition (Rosenberg et al. 1989). In the present study, this method was used to compare the magnitude of coherence of the same motor unit pair during periods of movement and position holding.
Two complementary approaches were used to investigate the time relation between motor unit activity and related kinematic signals. In the frequency domain, phase spectra were derived from the cross-spectra, and a straight line was fitted to the phase curve over the region of frequencies where the coherence was significant. The delay between the two signals was calculated from the slope of this line (Rosenberg et al. 1989; Halliday et al. 1995). In the time domain, time delay was estimated from the main deflection in the cross-covariance functions.
A 95 % confidence limit was used for all statistical analyses.
RESULTS
Motor unit recordings
Figure 1 shows the record of a sample trial. Joint position is shown along with joint angular velocity and acceleration. During the movements, recurring peaks in the angular velocity and acceleration records can be seen. Such oscillations also occur during phases of position holding, but generally with much lower amplitude. Figure 1 also shows the intramuscular recordings of two single motor units, along with surface EMG. The two motor units exhibit a similar firing pattern, which was a consistent finding for all movements and recorded motor units pairs: units were recruited early during the extension movements, discharge continued during the position-holding phase when the hand was held in an extended wrist position, and firing stopped roughly mid-way through the flexion movement phase.
In total, recordings from 36 motor unit pairs were obtained. In a few cases, the same reference motor unit could be held while more than one other motor unit was retrieved with the other intramuscular wire. In total, 62 individual motor units were recorded. All motor units were recruited early during slowly increasing voluntary isometric contractions. For 28 motor units, the recruitment thresholds were 0.006–0.15 N m (median 0.03 N m), corresponding to less than 1.5 % of the torque at maximum voluntary contraction (median). Even though the recruitment threshold was not reliably measured for the other motor units, they showed similar discharge patterns during movement under isotonic conditions. Hence, all units were tentatively classified as low-threshold, slow twitch-type units.
The number of movement trials recorded for a motor unit pair ranged from 10 to 134 (mean 35). All units showed a uniform firing pattern during the slow extension-flexion movement trials, similar to the sample units in Fig. 1. There often was a small increase in motor unit firing rate over the first few discharges after recruitment, without any consistent change in overall firing rate thereafter. This is similar to what has previously been reported for finger muscle motor units during slow movements (Wessberg & Kakuda, 1999). The average of the mean firing rates over the part of the movement where each unit was active during extension movements was 13.5 Hz (range 9.5–18.5 Hz). During steady position holding in the extended wrist position, average firing rate was 11.5 Hz (range 7.7–16.3 Hz). Average number of recorded spikes for each unit was 1183 (range 342–4873) during extension movements and 1210 (range 334–5200) during position holding. During flexion movements, units usually stopped firing similarly to the units in Fig. 1, and flexion movements were not analysed in the present study.
Common modulation of motor unit pairs during movement and position holding
Figure 2 shows the frequency- and time-domain analyses of the spike trains from all recorded wrist extension movements (112 movements) for the same motor unit pair as in Fig. 1. Figure 2A and B shows the auto-spectral density function estimates for the first and second motor unit, respectively. There is a broad peak around 15 Hz in each estimate. This corresponds to the mean firing rates for the two units, which were 14.3 and 14.7 Hz. Figure 2C shows the coherence function estimate between the motor units. There is a notable peak at 10 Hz, indicating a common modulation of the firing of the two units. In addition, there is significant coherence at lower frequency, possibly with a peak around 4 Hz. Hence, common modulation occurred at frequencies well away from the average firing rates of both motor units, reflecting one or more common inputs to the motoneurones.
Figure 2. Frequency- and time-domain analyses on the spike trains of a motor unit pair during the extension phase of movement.
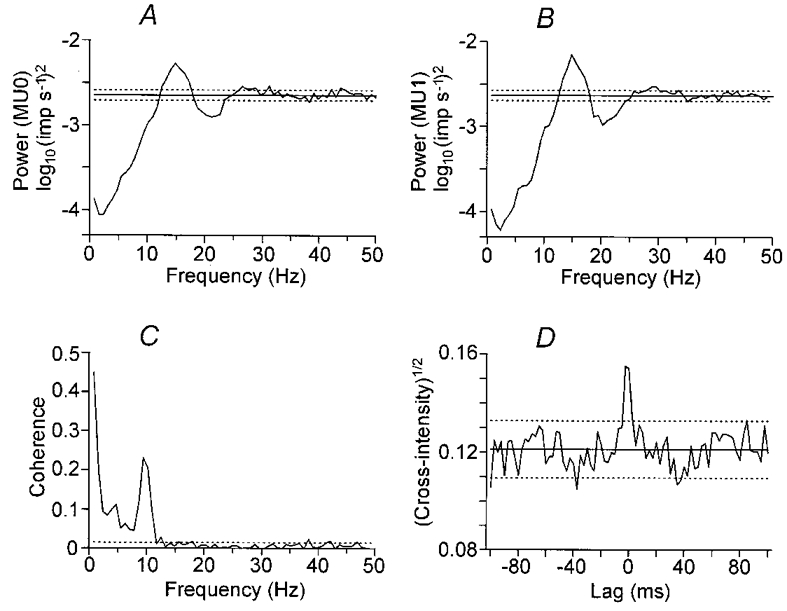
Statistical analysis of coupling between motor unit activity for a database of 112 movements performed as in Fig. 1. A and B show logarithmic auto-spectral density function estimates for each motor unit. Peaks around 15 Hz correspond to the average firing rate of the two units. C shows the estimated coherence between the motor units, revealing a strong coupling of activity at 10 Hz, as well as coupling around 4 Hz. D shows the square root of the cross-intensity function, with an 10 ms wide peak around lag time zero. Horizontal dotted lines indicate the approximate 95 % confidence limits.
Figure 2D shows the square root of the cross-intensity function estimate. This shows a narrow peak close to time zero, with a duration of about 10 ms. Hence, common modulation was near-synchronous in the two motor units.
Figure 3 shows the same analyses as in Fig. 2 for the same motor unit pair when the wrist was held in an extended joint position between movements. Auto-spectral density function estimates of individual motor units show a peak at around 12 Hz (Fig. 3A and B), corresponding to a discharge rate of 11.7 Hz in both units. This is only slightly lower than the discharge rate during movements. In contrast, the estimated coherence in Fig. 3C shows no sign of any peak at 10 Hz, with coherence around 4 Hz showing a modest decrease. The size and duration of a central peak in the square root of the cross-intensity function (Fig. 3D) during position holding are similar to those during movements (Fig. 2D).
Figure 3. Frequency- and time-domain analyses on the spike trains of a motor unit pair during position holding.
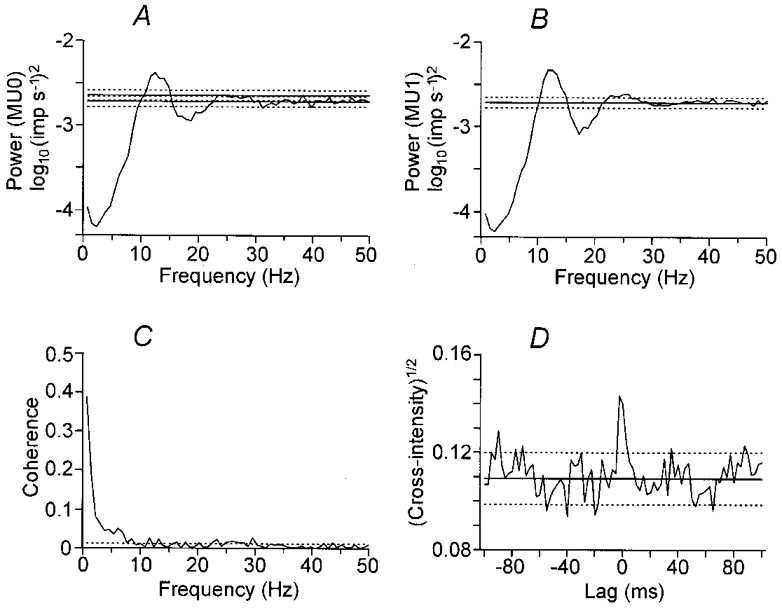
Statistical signal analysis of the inter-movements position holding phase of the same 112 trials as in Fig. 2. A-D as in Fig. 2. Coherence at 10 Hz disappears during position holding.
In Fig. 4A and B, a direct comparison of the coherence estimates for the same motor unit pair for extension movements (Fig. 2C) and position holding (Fig. 3C) has been made. In Fig. 4A the coherence estimates have been superimposed (coherence during movements in a heavy line), highlighting the disappearance of the peak around 10 Hz during position holding. Figure 4B shows the corresponding statistical analysis, the curve shows tanh−1|RA|-tanh−1|RB|, where |RA| and |RB| are the square roots of the coherence estimates from movement and position holding, respectively. The 95 % confidence limits are indicated, showing that the large change in coherence around 10 Hz is strongly significant. However, there is a significant reduction of coherence around 4 Hz as well, although the change in magnitude is not as large.
Figure 4. Comparison of coherence estimates of the same motor unit pairs between movement and position holding.
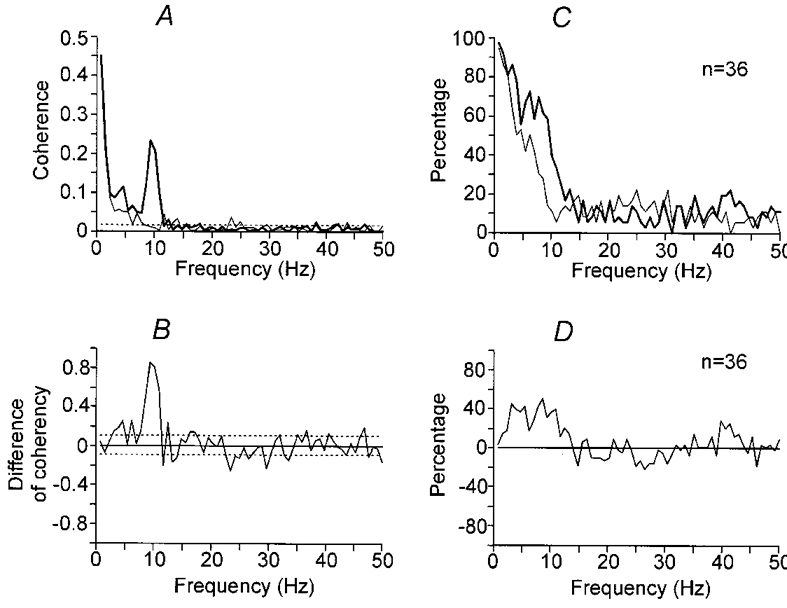
In A the coherence estimates from the same motor unit pair as in Figs 2 and 3 have been superimposed. A heavy line indicates movement and a thin line position holding. In B the difference of the transformed square root of the coherence (tanh−1|RA|-tanh−1|RB|) has been plotted. A positive deflection indicates that coherence was greater in magnitude during movement. Horizontal dotted lines indicate the 95 % confidence limits. In C and D the same analysis for 36 motor unit pairs has been summarised. In C the percentage of motor unit pairs showing a significant coherence at each frequency is indicated (P < 0.05, heavy line for movement and thin line for position holding). In D percentage of motor unit pairs showing a significant difference (tanh−1|RA|-tanh−1|RB|) at each frequency is shown.
In the 36 motor unit pairs analysed, a significant peak in the 6–12 Hz range in the coherence was present in 30 pairs (83 %) during extension movement. The magnitude of this peak was significantly reduced in 26/30 pairs (87 %) during position holding. The pattern of coherence for the whole sample is indicated in Fig. 4C, which shows the percentage of pairs exhibiting significant (P < 0.05) coherence at each frequency during movement (heavy line) and during position holding (thin line). Figure 4D shows the percentage of pairs showing a significant difference in the coherency estimates (tanh−1|RA|-tanh−1|RB|). There is a broad band of increased coherence from 2 to 12 Hz. Hence, from Figs 2–4, it may be concluded that the correlation of motor unit pairs increased in the 2–12 Hz range during voluntary slow movement at the wrist joint compared to steady position holding in the extended wrist position.
However, it can be suggested that the coherence in the 2–4 and 6–12 Hz ranges have different properties, or by extension, different origins. Notably, the magnitude of any change in coherence is not indicated by the summary in Fig. 4D, and the magnitude of the change of coherence, as shown for the sample pair in Fig. 4B, was strongest in the 6–12 Hz range. In addition, there is a notch at 5 Hz in the graph of Fig. 4D, suggesting a bimodal spectrum with separate components above and below 5 Hz. Further arguments for different origins for the 2–4 and 6–12 Hz coherence between motor units will be discussed below.
A 16–32 Hz component in the coherence, first reported by Farmer et al. (1993), was present in 32 pairs (80 %) in the present study during movement and position holding. However, this component was weak and scattered over a wide range of frequencies among different pairs so that it is not obvious in the summary graph of Fig. 4C. On the other hand, it can be noted in Fig. 4D that most points between 16 and 32 Hz are below zero, suggesting that the coherence between 16 and 32 Hz was stronger during position holding than during movement. In Fig. 4D, a small peak may be noted at 40–45 Hz as well. However, this component is very weak and not significant in Fig. 4A and C.
The peaks close to time zero in the square root of the cross-intensity function remained unchanged between movement and position holding. A significant peak was observed in 29 pairs (73 %) during both movement and position holding. The size (E/M, see Methods) of the peak during extension movements was 7.7 ± 1.9 (mean ±s.d.) compared to 7.8 ± 1.7 during position holding. The duration of peak was 11.9 ± 3.8 ms (mean ±s.d.) during movements compared to 12.4 ± 3.4 ms during position holding. These differences were non-significant (Student's paired t test, P > 0.05).
Correlated motor unit discharges contribute to angular acceleration
Angular acceleration varied around zero during position holding and movement (Fig. 1). The acceleration records from movements and phases of position holding were used as quasi-stationary time series to characterise the frequency content of the kinematics.
Figure 5 shows the frequency-domain analysis of coupling between motor unit activity and acceleration. The data base used for the analysis is the same as used in Figs 2 and 3, and for the sample record in Fig. 1. The logarithmic auto-spectral density function estimate of acceleration during extension movements is shown in Fig. 5A. There is a dominant component at 3 Hz, and a small deflection at 10 Hz. Coherence estimates between single motor unit activity and acceleration are shown in Fig. 5B and C. Both show a prominent peak around 10 Hz, and a smaller peak around 3 Hz. This corresponds to the peaks in the coherence between the motor units (Fig. 2C), suggesting that correlated motor unit discharges contributed to angular acceleration at these frequencies. As for inter-motor unit coherence, the presence of two separate peaks in the coherence between 1 and 12 Hz in Fig. 5B and C again suggests that there are two separate mechanisms contributing to coherence in this frequency range.
Figure 5. Angular acceleration and the relation with single motor units.
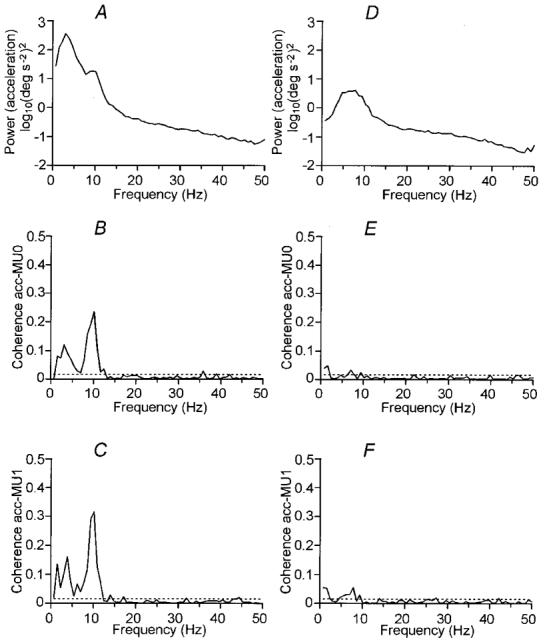
Analysis of coupling between motor units and acceleration for the same database as in previous figures. Extension movements are shown in A-C, position holding in D-F. From above, logarithmic auto-spectral density function estimates of angular acceleration (A, D), coherence estimates between the first (B, E; Coherence acc-MU0) and the second (C, F; Coherence acc-MU1) motor unit and acceleration. Horizontal lines indicate the approximate 95 % confidence limits.
Figure 5D-F shows the same analyses as in Fig. 5A-C but for the position-holding phase. The auto-spectrum of acceleration in Fig. 5D features a broad peak between 2 and 10 Hz, but due to the logarithmic scale, the power of the peak is considerably lower than the corresponding auto-spectrum for movements (Fig. 5A). In the coherence estimates between the single motor units and angular acceleration (Fig. 5E and F), only a very small peak can be seen at approximately 5–8 Hz. Because the contribution of motor unit discharge to acceleration is small, it is probable that the observed 5–8 Hz peak in the acceleration spectrum largely represents the purely mechanical oscillation close to the mechanical resonant frequency, which is weakly driven by muscle activation (Stiles & Randall, 1967; Stiles 1983; Lakie et al. 1986a,b).
The frequency content of angular acceleration was similar in all subjects. For the 62 motor units, coherence estimates showed a significant peak at 6–12 Hz in 56 motor units (90 %) during movements, and this peak decreased in amplitude during position holding in 51 units.
Estimation of time delay between motor unit activity and acceleration
After confirming a significant coupling between motor unit activity and acceleration (Fig. 5), the time relationship between these two signals was assessed. For these analyses, two complementary approaches were used. First, delay was estimated in the time domain from the location of the main deflection in the cross-covariance function. Second, delay was estimated from the phase spectrum obtained with the coherence analysis by fitting a straight line to the phase curve by weighted linear regression over the part of the spectrum where coherence is significant (Halliday et al. 1995; also illustrated in Wessberg & Kakuda, 1999). During movements, the average time delay measured in 48 motor units from the cross-covariance function was 65 ± 13 ms (mean ±s.d.). Delay measured from phase estimates in the same units was 73 ± 16 ms (mean ±s.d.). During position holding, for the 34 motor units where the delay measurements could be made, delay measured from the cross-covariance function was 45.9 ± 8.3 ms (mean ±s.d.), and from phase estimates 45.3 ± 10.6 ms (mean ±s.d.). Hence, the average delay was somewhat shorter during position holding compared to movements. A tentative reason can be found in the complex biomechanics of the wrist joint, which will be discussed below (Lakie et al. 1986b).
DISCUSSION
The main finding of this study was that discharges of pairs of motor units were correlated at 2–12 Hz during voluntary slow movement of the wrist joint. In particular, prominent coherence at 6–12 Hz was seen in 83 % of the pairs.
Modulation of motor output during slow movements in man has previously been described principally for slow finger movements, where a pulsatile modulation of muscle activity occurs at 8–10 Hz. As a hypothesis, it has been suggested that this represents a pulsatile modulation of the motor command to the muscles (Vallbo & Wessberg, 1993; Wessberg & Vallbo, 1996). The findings in the present study extend the observation of coherent motor unit modulation below 12 Hz to hand movements, and furthermore expand on the previous descriptions in several important ways. First, the biomechanics of the wrist are different from the fingers, so that the motor unit correlation at 6–12 Hz occurred in spite of the circa3 Hz oscillations of the moving limb. Second, the recording of pairs of motor units rather than surface EMG or single motor units enabled a direct analysis of common inputs to motoneurones. Third, it was shown that motor unit coherence decreased or disappeared during position holding, indicating that common 6–12 Hz motor unit modulation occurred specifically during movements.
Biomechanics of the wrist
The hand has considerably larger inertia than a finger. The biomechanical consequences are that movements at the wrist exhibit mechanical resonance at a much lower frequency, and that limb acceleration will fail to follow variations in muscle activation already at fairly low frequency compared to the fingers.
Mechanical resonance of the relaxed wrist without any external load has been reported to occur at 8–10 Hz (Stiles & Randall, 1967; Stiles, 1983; Lakie et al. 1986a). However, this may not be true for the moving limb, because the measured resonance is strongly dependent on the amplitude of displacements of the hand, and the apparent resonance may decrease down to 2 Hz if strong perturbations are used when assessing resonance (Lakie et al. 1986b). In the present study, the hand was loaded with an additional 250 g by the attached manipulandum, which should further lower the resonant frequency. During position holding, the spectrum of oscillation showed a peak around 5–8 Hz. Because the contribution of motor unit discharge to angular acceleration was found to be very small during position holding, this peak may be taken as the mechanical resonant frequency under steady contraction. During movement, average amplitudes of oscillations, as seen in velocity or acceleration records, increased by more than 10-fold, and the resonant frequency could be expected to decrease. This fits with the observed peaks in the acceleration spectra around 3 Hz in most subjects, although motor unit activity significantly contributed to this peak as well, as will be discussed below.
One further consequence of the larger inertia of the wrist than the fingers is the long electro-mechanical delay from motor unit activity to peak acceleration reported in the present study, about 70 ms during movement and 45 ms during position holding. The delay during position holding is compatible to the contraction time of human single motor units from the same muscle (30–80 ms), measured by spike-triggered averaging of the torque during weak isometric contraction (Schmied et al. 1994). (The comparable delay for fingers is about 20 ms during movements.)
The main circa3 Hz oscillations of the moving wrist occurred well below the prominent 6–12 Hz coherence of motor unit pairs, which in turn was reflected in the acceleration spectra only as a small positive deflection. Hence, in contrast to the 8–10 Hz discontinuities in finger movements, the principal 6–12 Hz motor unit modulation was mechanically uncoupled from the main oscillations of the limb. This has consequences for the possible mechanisms underlying the common motor unit modulation, discussed next.
Two different mechanisms for common modulation of motor units?
Observation of the activity of pairs of motor units rather than surface EMG or single motor units enables a direct analysis of common inputs to a pair of motoneurones (Bremner et al. 1991; Kirkwood & Sears, 1991; Farmer et al. 1993; Gibbs et al. 1995). In the present study, correlation between pairs of motor units was demonstrated both in the time and frequency domain. The notion that such correlations reflect a common input to the motoneurone pool can be further corroborated by calculation of partial coherence using surface EMG as a predictor (Halliday et al. 1995). For the data in the present study, such analyses show a significant reduction of partial coherence, indicating that the observed common modulation in each pair is shared in large part by the whole population of motor units contributing to the surface EMG (N. Kakuda & M. Nagaoka, unpublished observations). Hence, a rhythmic modulation of some input to a large proportion of the active motoneurones is implied. In principle, the source of this input may be entirely within the central nervous system, or the periphery may be involved as well.
In this context it is important to note that several observations indicate that common modulation below and above 5 Hz may have different origins. First, the 6–12 Hz component was stronger than the 2–4 Hz in most motor unit pairs. Second, the 6–12 Hz component was more prominently reduced when switching to steady contraction during position holding. Third, the coherence function between motor unit activity and acceleration during movements often was bimodal, with maxima below and above 5 Hz. Hence, we suggest that different mechanisms need to be considered for the 2–4 and 6–12 Hz common modulation.
Afferent inputs to the motoneurones
When the movements of a limb activate peripheral proprioceptive or tactile receptors, which in turn activate muscle through reflex pathways, a closed-loop feedback oscillation could in theory occur. Because of its relative strength and short conduction delay, the principal candidate would be the spinal stretch reflex. This idea has been comprehensively investigated for the phenomenon of physiological tremor during position holding (Lippold, 1970; Joyce & Rack, 1974; Elble & Randall, 1976; Burne et al. 1984). The frequency of oscillations which would be sustained has been shown to be dependent on reflex loop time, so that delay from activation of receptors to peak mechanical effect on the limb corresponds to roughly one half-cycle (Stein & Oguztöreli, 1976). When computing reflex loop time, neural conduction delay is important, but it has also been shown that mechanical factors, principally the inertia of the moving limb, will crucially affect the frequency of any reflex-driven oscillations (Prochazka & Trend, 1988).
For finger movements, the reflex hypothesis has been investigated extensively with approaches involving recording of muscle spindle afferents and analysis of responses to external perturbations applied during movements. It was concluded that a stretch reflex mechanism could not be a main factor causing the 8–10 Hz discontinuities in finger movements, but would theoretically promote oscillations at lower frequencies (Wessberg & Vallbo, 1995, 1996).
For wrist movements, although the exact patterns of activation of muscle spindle afferents are presently unknown, the electro-mechanical delay from electrical activation of muscle until the limb is accelerated will comprise a significant part of the loop, placing an upper limit on the frequency of oscillation which could theoretically be sustained. Supposing a neural conduction delay in the spinal stretch reflex of 20 ms (Deschuytere et al. 1976) and the electro-mechanical delay from motor unit firing to peak acceleration observed during movements in the present study, about 70 ms, reflex loop time would be about 90 ms. For a 90 ms half-cycle time, total cycle time is 180 ms, corresponding to 5.6 Hz. Hence, according to these theoretical calculations, a mechanism involving the spinal stretch reflex could theoretically sustain oscillations below 6 Hz at the wrist joint, but not above. This frequency would be correspondingly lower for reflexes with longer neural conduction delays, such as the transcortical stretch reflex. Hence, it may be concluded that, in theory, reflex feedback from the periphery may have contributed to the observed 2–4 Hz common modulation of motor units. However, this issue needs to be further explored in experiments involving varying the mechanical load on the hand, or perturbations of the ongoing movement.
Central inputs to the motoneurones
Visual tracking of the kind used in the present study can promote 2–3 Hz oscillations in wrist and finger movements, probably by an ongoing updating of the motor command through a loop involving visual feedback (Navas & Stark, 1968; Vallbo & Wessberg, 1993; McAuley et al. 1999). This could have contributed to the observed 2–4 Hz common modulation. It should be possible to resolve this issue in comparable experiments involving movements made without visual tracking.
For the 6–12 Hz modulation it should be noted that, while newly recruited neurones have been demonstrated to fire at frequencies in the 6–10 Hz range (Freund et al. 1975), this would not explain the observed common modulation at 6–12 Hz of pairs of motor units, individually firing at higher rates. The possible central pathways providing the common input could in theory be either spinal or supraspinal. Spinal mechanisms include Renshaw inhibition, although it has been argued that recurrent inhibition will desynchronise rather than cause correlation of motoneurones (Windhorst & Kokkoroyiannis, 1992; Maltenfort et al. 1998). For fingers, it has not been determined if the mechanism providing the modulation is spinal or supraspinal, but the observed correlation between agonist and antagonist muscles, as well as the intermittent left-right correlation at least strongly implies that a significant degree of supraspinal, possibly cortical, control is present (Wessberg, 1996; McAuley et al. 1999).
The observation of 6–12 Hz modulation of motor output during wrist movements raises the question whether circa10 Hz modulation may be a common feature of all slow movements in man. Velocity modulation at similar frequencies has recently been described for some eye movements (McAuley et al. 1999), but circa10 Hz modulation should also be sought in other movements involving limbs with larger inertia. Indeed, this has been reported for elbow movements (Conway et al. 1997). If circa10 Hz modulation of motor output is also present at these joints, this has implications for the hypotheses regarding why such modulations exist in the human motor system. We have previously argued that several different reasons may be sought at different levels in the nervous system. For the low-inertia fingers, the circa10 Hz modulation is directly reflected in the acceleration, which may provide advantages for the precise control of finger position in some motor tasks (Wessberg & Kakuda, 1999), and which means that proprioceptive feedback is modulated as well (Wessberg & Vallbo, 1996). For limbs with higher inertia, 10 Hz modulation of acceleration will be insignificant, and the possible reason for a modulation of motor output in such joints must be sought within the central nervous system. In theory, a coherent pulsatile modulation of the motor command may bring advantages such as decreased susceptibility of the command signal to corruption by extraneous neural noise. Alternatively, the circa10 Hz modulation may be the reflection of a temporal organization of the central circuitry from which the descending signal originates, without providing any particular advantage per se at the level of the descending command.
Is the 10 Hz motoneurone modulation specific for movements?
Wrist motor unit coherence was significantly reduced during periods of position holding compared to movements. Indeed, as highlighted in Fig. 4A and B, the prominent peak around 8–10 Hz sometimes disappeared entirely during steady maintenance of force during position holding. Task-dependent change of motor unit synchronization has previously been described from the cross-correlation histogram of motor unit pairs in human neck muscles (Adams et al. 1989), extrinsic hand muscles (Schmied et al. 1993), intrinsic hand muscles (Bremner et al. 1991) and leg muscles (Gibbs et al. 1995) and in cat neck muscles (Loeb et al. 1987).
It has been shown that motor units of hand muscles are coherent at circa16–32 Hz during maintenance of steady isometric contractions (Farmer et al. 1993) and that this coherence is reduced during periods when there is a change in grip force (Kilner et al. 1999). The 16–32 Hz component in the present study was much weaker than that reported in hand muscles. It is possible that this difference between hand muscles and wrist extensor muscles can be explained by a larger proportion of direct cortico-spinal projections to the motoneurone pools for the hand muscles (Farmer et al. 1993). Although weak, it is worth noting that a 16–32 Hz component in wrist muscles seems to be stronger during position holding than during movement, suggesting a task-dependent modulation similar to hand muscles.
Based on earlier findings for human finger movements and the results reported in the present study, it may be argued that during movements, the 16–32 Hz modulation of motor units is supplemented, or even replaced, by a circa 10 Hz modulation. It may be asked what parameter or aspect of the motor task would promote the 10 Hz rhythmic organization of motor units. It is interesting to note that the normally smooth force profiles in human finger grip tasks may be replaced by a circa 10 Hz stepwise pattern when the force is updated, either when subjects are unsure about the load conditions, or when force is erroneously programmed (Johansson & Westling, 1988). This suggests that the important factor promoting the circa 10 Hz input may be the modification of motor output, both during movements and isometric tasks.
The exact central mechanism responsible for the 10 Hz modulation of motor units during movements remains to be elucidated. The 16–32 Hz modulation of motor units during steady contractions is due to a modulation of the descending command from the cortex as demonstrated by direct recordings in monkeys (Baker et al. 1997) and by magnetencephalography in humans (Conway et al. 1995; Salenius et al. 1997; Kilner et al. 1999). Such fast oscillations of primate motor cortex disappear during the actual movements (Murthy & Fetz, 1996a,b; Baker et al. 1997; Donoghue et al. 1998; Kilner et al. 1999). On the other hand, previous electroencephalographic or magnetencephalographic recordings have failed to demonstrate a 10 Hz modulation of cortical activity during movements, although the type of slow precision task used in the present study remains to be comprehensively investigated during recording of the cerebral activity (Salmelin & Hari, 1994; Kilner et al. 1999). The relation between motor task, oscillations in motor cortex and common modulation of motoneurone pools would need to be further addressed in experiments with a well-controlled task comprising slow precision movements. It is also possible to speculate that the cerebellum may be involved in the 10 Hz rhythmicity of motor output during movements. It has been suggested that olivo-cerebellar activity is highly rhythmic and time-locked to movements (Llinás, 1991; Welsh et al. 1995; Welsh & Llinás, 1997).
In conclusion, the present study suggests a circa10 Hz pulsatile organization of the motor command to the agonist motoneurone pool during slow precision movements. The presence of this component is related to the motor task, but appears to be independent of the mechanics of the moving limb.
Acknowledgments
This study was supported by the Ministry of Health and Welfare of Japan. Dr Wessberg was supported by the Swedish Medical Research Council (grant 14X-3548). We would like to thank Dr David Halliday (Glasgow University, UK) for valuable comments on the manuscript.
References
- Adams L, Datta AK, Guz A. Synchronization of motor unit firing during different respiratory and postural tasks in human sternocleidomastoid muscle. The Journal of Physiology. 1989;413:213–231. doi: 10.1113/jphysiol.1989.sp017650. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Baker SN, Olivier E, Lemon RN. Coherent oscillations in monkey motor cortex and hand muscle EMG show task-dependent modulation. The Journal of Physiology. 1997;501:225–241. doi: 10.1111/j.1469-7793.1997.225bo.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Bendat JS, Piersol AG. Random Data. New York: John Wiley & Sons; 1986. [Google Scholar]
- Bremner FD, Barker JR, Stephens JA. Effect of task on the degree of synchronization of intrinsic hand muscle motor units in man. Journal of Neurophysiology. 1991;66:2072–2083. doi: 10.1152/jn.1991.66.6.2072. [DOI] [PubMed] [Google Scholar]
- Brillinger DR. Estimation of the second-order intensities of a bivariate stationary point process. Journal of Royal Statistics Society. 1976;38:60–66. series B. [Google Scholar]
- Burne JA, Lippold OCJ, Pryor M. Proprioceptors and normal tremor. The Journal of Physiology. 1984;384:559–572. doi: 10.1113/jphysiol.1984.sp015125. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Conway BA, Biswas P, Halliday DM, Farmer SF, Rosenberg JR. Task-dependent changes in rhythmic motor output during voluntary elbow movement in man. The Journal of Physiology. 1997;501.P:48–49. P. [Google Scholar]
- Conway BA, Halliday DM, Farmer SF, Rosenberg JR. Synchronization between motor cortex and spinal motoneurone pool during the performance of a maintained motor task in man. The Journal of Physiology. 1995;489:917–924. doi: 10.1113/jphysiol.1995.sp021104. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Davey NJ, Ellaway PH, Stein RB. Statistical limits for detecting changes in the cumulative sum derivative of the peristimulus time histogram. Journal of Neuroscience Methods. 1986;17:153–166. doi: 10.1016/0165-0270(86)90068-3. [DOI] [PubMed] [Google Scholar]
- Deschuytere J, Rosselle N, De Keyser C. Monosynaptic reflexes in the superficial forearm flexors in man and their clinical significance. Journal of Neurology, Neurosurgery and Psychiatry. 1976;39:555–565. doi: 10.1136/jnnp.39.6.555. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Donoghue JN, Sanes JN, Hastopoulos NG, Gaál G. Neural discharge and local field potential oscillations in primate motor cortex during voluntary movements. Journal of Neurophysiology. 1998;79:159–173. doi: 10.1152/jn.1998.79.1.159. [DOI] [PubMed] [Google Scholar]
- Edin BB, Bäckström PA, Bäckström LO. Single-unit retrieval in microneurography: A microprocessor-based device controlled by an operator. Journal of Neuroscience Methods. 1988;24:137–144. doi: 10.1016/0165-0270(88)90057-x. [DOI] [PubMed] [Google Scholar]
- Elble RJ, Randall JE. Motor-unit activity responsible for 8- to 12-Hz component of human physiological tremor. Journal of Neurophysiology. 1976;39:370–383. doi: 10.1152/jn.1976.39.2.370. [DOI] [PubMed] [Google Scholar]
- Farmer SF, Bremner FD, Halliday DM, Rosenberg JR, Stephens JA. The frequency content of common synaptic inputs to motoneurones during voluntary isometric contraction in man. The Journal of Physiology. 1993;470:127–155. doi: 10.1113/jphysiol.1993.sp019851. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Freund H-J, Bündingen HJ, Dietz V. Activity of single motor units from human forearm muscles during voluntary isometric contractions. Journal of Neurophysiology. 1975;38:933–946. doi: 10.1152/jn.1975.38.4.933. [DOI] [PubMed] [Google Scholar]
- Gibbs J, Harrison LM, Stephens JA. Organization of inputs to motoneurone pools in man. The Journal of Physiology. 1995;485:245–256. doi: 10.1113/jphysiol.1995.sp020727. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Halliday DM, Rosenberg JR, Amjad AM, Breeze P, Conway BA, Farmer SF. A framework for the analysis of mixed time series/point process data – theory and application to the study of physiological tremor, single motor unit discharges and electromyograms. Progress in Biophysics and Molecular Biology. 1995;64:237–278. doi: 10.1016/s0079-6107(96)00009-0. [DOI] [PubMed] [Google Scholar]
- Harrison LM, Ironton R, Stephens JA. Cross-correlation analysis of multiunit EMG recordings in man. Journal of Neuroscience Methods. 1991;40:171–179. doi: 10.1016/0165-0270(91)90066-9. [DOI] [PubMed] [Google Scholar]
- Johansson RS, Westling G. Coordinated isometric muscle commands adequately and erroneously programmed for the weight during lifting task with precision grip. Experimental Brain Research. 1988;71:59–71. doi: 10.1007/BF00247522. [DOI] [PubMed] [Google Scholar]
- Joyce GC, Rack PMH. The effect of load and force on tremor at the normal human elbow joint. The Journal of Physiology. 1974;240:375–396. doi: 10.1113/jphysiol.1974.sp010615. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kakuda N, Nagaoka M. The common modulation of motor units during slow wrist movement in man. Society for Neuroscience Abstracts. 1996;22:667. [Google Scholar]
- Kilner JM, Baker SN, Salenius S, Jousmäki V, Hari R, Lemon RN. Task-dependent modulation of 15–30 Hz coherence between rectified EMGs from human hand and forearm muscles. The Journal of Physiology. 1999;516:559–570. doi: 10.1111/j.1469-7793.1999.0559v.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Kirkwood PA, Sears TA. Cross-correlation analyses of motoneuron inputs in a coordinated motor act. In: Kruger J, editor. Springer Verlag Series in Synergetics: Neuronal Cooperability. Berlin: Springer Verlag; 1991. pp. 225–248. [Google Scholar]
- Lakie M, Walsh EG, Wright GW. Passive mechanical properties of the wrist and physiological tremor. Journal of Neurology, Neurosurgery and Psychiatry. 1986a;49:669–676. doi: 10.1136/jnnp.49.6.669. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lakie M, Walsh EG, Wright GW. Resonance at the wrist demonstrated by the use of a torque motor: An instrumental analysis of muscle tone in man. The Journal of Physiology. 1986b;353:265–285. doi: 10.1113/jphysiol.1984.sp015335. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lippold OCJ. Oscillation in the stretch reflex arc and the origin of the rhythmical 8–12 Hz component of physiological tremor. The Journal of Physiology. 1970;206:359–382. doi: 10.1113/jphysiol.1970.sp009018. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Llinás RR. The non-continuous nature of movement execution. In: Humphrey DR, Freund H-J, editors. Motor Control, Concepts and Issues. New York: John Wiley & Sons; 1991. pp. 223–242. [Google Scholar]
- Loeb GE, Yee WJ, Pratt CA, Chanaud CM, Richmond FJR. Cross-correlation of EMG reveals widespread synchronization of motor units during slow movements in intact cats. Journal of Neuroscience Methods. 1987;21:239–249. doi: 10.1016/0165-0270(87)90119-1. [DOI] [PubMed] [Google Scholar]
- McAuley JH, Farmer SF, Rothwell JC, Marsden CD. Common 3 and 10 Hz oscillations modulate human eye and finger movements while they simultaneously track a visual target. The Journal of Physiology. 1999;515:905–917. doi: 10.1111/j.1469-7793.1999.905ab.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Maltenfort MG, Heckmann CJ, Rymer WZ. Decorrelating actions of Renshaw interneurons on the firing of spinal motoneurons within a motor nucleus: A simulation study. Journal of Neurophysiology. 1998;80:309–323. doi: 10.1152/jn.1998.80.1.309. [DOI] [PubMed] [Google Scholar]
- Murthy VN, Fetz EE. Oscillatory activity in sensorimotor cortex of awake monkeys: Synchronization of local field potentials and relation to behavior. Journal of Neurophysiology. 1996a;76:3949–3967. doi: 10.1152/jn.1996.76.6.3949. [DOI] [PubMed] [Google Scholar]
- Murthy VN, Fetz EE. Synchronization of neurons during local field potential oscillations in sensorimotor cortex of awake monkeys. Journal of Neurophysiology. 1996b;76:3968–3982. doi: 10.1152/jn.1996.76.6.3968. [DOI] [PubMed] [Google Scholar]
- Navas F, Stark L. Sampling or intermittency in hand control system dynamics. Biophysics Journal. 1968;8:253–302. doi: 10.1016/S0006-3495(68)86488-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Prochazka A, Trend PSJ. Instability in human forearm movements studied with feed-back-controlled muscle vibration. The Journal of Physiology. 1988;402:421–442. doi: 10.1113/jphysiol.1988.sp017213. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Rosenberg JR, Amjad AM, Breeze P, Brillinger DR, Halliday DM. The Fourier approach to the identification of functional coupling between neuronal spike trains. Progress in Biophysics and Molecular Biology. 1989;53:1–31. doi: 10.1016/0079-6107(89)90004-7. [DOI] [PubMed] [Google Scholar]
- Salenius S, Portin K, Kajola M, Salmelin R, Hari R. Cortical control of human motorneuron firing during isometric contraction. Journal of Neurophysiology. 1997;77:3401–3405. doi: 10.1152/jn.1997.77.6.3401. [DOI] [PubMed] [Google Scholar]
- Salmelin R, Hari R. Spatiotemporal characteristics of sensorimotor MEG rhythms related to thumb movement. Neuroscience. 1994;60:537–550. doi: 10.1016/0306-4522(94)90263-1. [DOI] [PubMed] [Google Scholar]
- Schmied A, Ivarsson C, Fetz EE. Short-term synchronization of motor units in human extensor digitorum communis muscle: relation to contractile properties and voluntary control. Experimental Brain Research. 1993;97:159–172. doi: 10.1007/BF00228826. [DOI] [PubMed] [Google Scholar]
- Schmied A, Vedel J-P, Pagni S. Human spinal lateralization assessed from motoneurone synchronization: dependence on handedness and motor unit type. The Journal of Physiology. 1994;480:369–387. doi: 10.1113/jphysiol.1994.sp020367. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stein RB, Oguztöreli MN. Tremor and other oscillations in neuromuscular systems. Biological Cybernetics. 1976;22:147–157. doi: 10.1007/BF00365525. [DOI] [PubMed] [Google Scholar]
- Stiles RN. Lightly damped hand oscillations: Acceleration-related feedback and system damping. Journal of Neurophysiology. 1983;50:327–343. doi: 10.1152/jn.1983.50.2.327. [DOI] [PubMed] [Google Scholar]
- Stiles RN, Randall JE. Mechanical factors in human tremor frequency. Journal of Applied Physiology. 1967;23:324–330. doi: 10.1152/jappl.1967.23.3.324. [DOI] [PubMed] [Google Scholar]
- Vallbo ÅB, Wessberg J. Organization of motor output in slow finger movements in man. The Journal of Physiology. 1993;469:673–691. doi: 10.1113/jphysiol.1993.sp019837. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Welsh JP, Lang EJ, Sugihara I, Llinás R. Dynamic organization of motor control within the olivocerebellar system. Nature. 1995;374:453–457. doi: 10.1038/374453a0. [DOI] [PubMed] [Google Scholar]
- Welsh JP, Llinás R. Some organizing principles for the control of movement based on olivocerebellar physiology. Progress in Brain Research. 1997;114:469–481. doi: 10.1016/s0079-6123(08)63380-4. [DOI] [PubMed] [Google Scholar]
- Wessberg J. Significant left-right synchronization of pulsatile motor output in a human bimanual finger movement task. Society for Neuroscience Abstracts. 1996;22:428. [Google Scholar]
- Wessberg J, Kakuda N. Single motor unit activity in relation to pulsatile motor output in human finger movement. The Journal of Physiology. 1999;517:273–285. doi: 10.1111/j.1469-7793.1999.0273z.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wessberg J, Vallbo ÅB. Coding of pulsatile motor output by human muscle afferents during slow finger movements. The Journal of Physiology. 1995;485:271–282. doi: 10.1113/jphysiol.1995.sp020729. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wessberg J, Vallbo ÅB. Pulsatile motor output in human finger movements is not dependent on the stretch reflex. The Journal of Physiology. 1996;493:895–908. doi: 10.1113/jphysiol.1996.sp021432. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Windhorst U, Kokkoroyiannis T. Dynamic behaviour of alpha-motoneurones subjected to recurrent inhibition and reflex feedback via muscle spindles. Neuroscience. 1992;47:897–907. doi: 10.1016/0306-4522(92)90038-4. [DOI] [PubMed] [Google Scholar]


