Fractal bird nest distribution produces scale-free colony sizes

Abstract

The spatial distribution of organisms often differs across scales. For instance, colonial bird populations could be described, from large to small scale, as scattered clumps of otherwise regularly distributed breeding pairs. We analysed the distribution of nests of a large colonial population of white storks (Ciconia ciconia) and found a fractal pattern in each of the 4 study years. Moreover, we found that the often-observed, long-tailed frequency distribution of colony sizes was well described by a power law, regardless of the cut-off used to define colonies (from 16 to 1024 m). Thus, although storks were locally highly clumped even with tens of nests in a single tree, the population was not structured in colonies (a simple clustered distribution) as previously thought. Rather, they were distributed in a continuous hierarchical set of clusters within clusters across scales, clusters lacking the commonly assumed characteristic mean size. These quantitative solutions to previously perceived scaling problems will potentially improve our understanding of the ecology and evolution of bird coloniality and animal spacing patterns and group living in general.

1. Introduction

Ecological textbooks differentiate three possible spacing patterns of organisms: random, uniform, and clumped. Often, however, the distribution of organisms does not attach to one of these kinds, showing more heterogeneous distributions at different resolutions (Brown 1995). Moreover, in clumped distributions such as those of animal groups, the frequency distribution of different group sizes often show long-tailed patterns, i.e. with many small groups and few large ones (Krause & Ruxton 2002). Thus, we also have vague descriptions of group sizes owing to the problems of dealing with such clear no-Gaussian distributions, where the mean is of lower relevance than the variance.

Here, we report our effort to quantitatively confront these problems, improving the way we describe these patterns and thus our expectations of understanding the processes governing them. We did so by studying one of the most spectacular bird aggregation patterns: the breeding colonies of birds. A clear example are gannetries (breeding aggregations of northern gannets, Morus bassanus), where thousands of birds nest in close contact and kilometres away from the nearest colony (Mitchell et al. 2004). Colonies are used as the population units in bird coloniality research, an approach that has greatly improved our understanding of the ecology and the evolution of these birds. For instance, by studying colony size variation we know that seabird populations can be regulated by density dependence owing to food depletion around colonies (Furness & Birkhead 1984; Lewis et al. 2001; Forero et al. 2002).

Field ecologists (Berg et al. 1992; Arroyo et al. 2001) and bird monitoring teams (Mitchell et al. 2004), however, are well aware of the scale-related difficulties in defining colonies spatially. This is obvious in species that show spread distributions like lapwings (Vanellus vanellus), where an arbitrary limit is needed to decide which nests belong to a colony, and which ones are solitary settlements (Berg et al. 1992). But problems also arise in the most typical colonial species, like many seabirds. For example, the nests of black-legged kittiwakes (Rissa tridactyla) are highly clumped, but to decide the point where one colony ends and the next one starts is not a trivial task (Coulson & Dixon 1979). In other species like the adélie penguin (Pygoscelis adeliae), the problem is that nests are organized in a set of hierarchical levels labelled with different names such as ‘subcolonies’ or ‘clusters of colonies’, and could even appear as solitary settlements (Ainley et al. 2002).

Some decades ago, Coulson & Dixon (1979) posed an intriguing question: how large a gap is necessary between nesting birds before one colony becomes two? This was thought to be only a methodological problem, because the existence of colonies is considered to be beyond question. But we think that it reflects the problem that we do not know (in fact because, to our knowledge, it has been never addressed) how the distribution of nests is organized through spatial scales. Rather, much debate has centred on different ways of defining colonies, arriving at neither a convincing definition of the concept nor an objective answer to the question raised by Coulson & Dixon (1979). In this way, current ambiguity has lead to the above-mentioned, entirely arbitrary distinctions to separate breeding groups of many colonial species into colonies, subcolonies and solitary settlements. Here, we abandon verbal discussions and perform quantitative analyses of natural patterns of nest distribution through scales. We show that these scaling properties could explain by itself the current ambiguity over the ‘colony’ concept, and allow addressing of the study of patterns of colony size variation, another major unsolved scaling problem of bird coloniality research (Brown et al. 1990).

2. Material and methods

(a) Study population and data

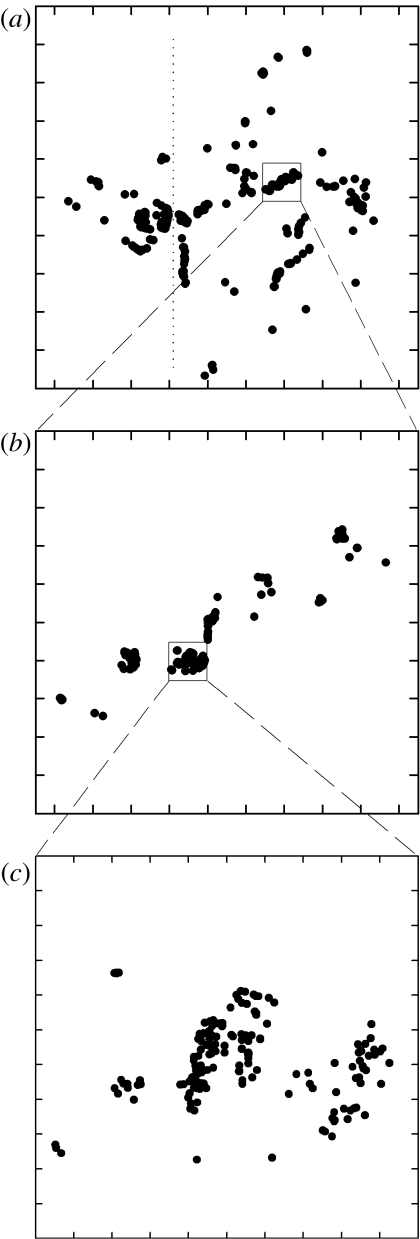
We exhaustively monitored and mapped all active nests in a large growing population of white storks (Ciconia ciconia), a large waterbird with an approximately 2 m wingspan, during four consecutive breeding seasons (year 2002, 956 nests; 2003, 1148; 2004, 1331 and 2005, 1432). The study area (3500 km² in and around Doñana National Park, south-west Spain) is composed of marshes, rice fields, Mediterranean forests and arable lands. Nests are highly conspicuous owing to their size (approx. 2.5 m radius). They were built mainly in trees (e.g. wild olive trees, eucalyptus, oaks), but some were placed in urban and rural buildings (e.g. farms, churches, houses). Approximately 100 nests were found in consecutive electric poles erected 150 m apart from one another at the west side of the study area (figure 1; figure 1 in the electronic supplementary material). After extracting these nests from the analyses, we analysed 876 nests in 2002, 1056 in 2003, 1188 in 2004 and 1274 in 2005.

Figure 1.

White stork nest distribution at different scales for the year 2005. Top of figures are oriented to the North. Nests on poles were located at the west of the dashed line in (a) (see figure 1 in the electronic supplementary material). Distance between axis tick marks are (a) 10 km, (b) 1 km and (c) 100 m.

(b) Spatial nest distribution analyses

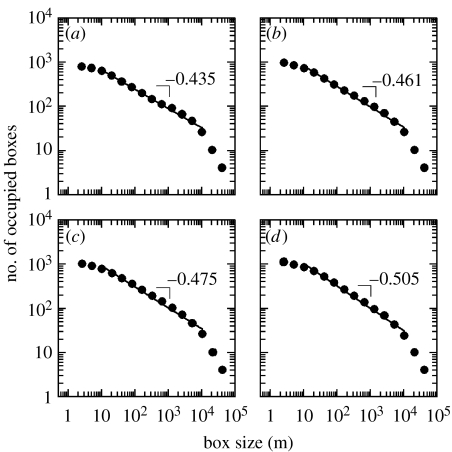
For each year we did a box-counting analysis of the spatial distribution of nests, a usual method in fractal geometry (Mandelbrot 1977; Halley et al. 2004; see figure 2 in the electronic supplementary material for an illustrative example of the following explanation). In a first step (n=1) a grid of four squares (boxes) of side length x is superimposed onto the nest distribution map. Then, in successive steps (n=2, 3, 4, …), grids with squares of side lengths x/2ⁿ⁻¹ are used. Thus, we have 4ⁿ squares for each step (i.e. 4, 16, 64, 256, …). The number of boxes occupied by atleast one nest tends to increase because boxes are increasingly smaller and thus more abundant at successive steps. In the box-counting plot the log (box side length) used in each grid is related to the log (number of occupied boxes) to quantify this rate of increase. A lineal relationship (with a non-integer slope) in this log–log plot denotes a fractal pattern, that is, a pattern that behaves alike when we look it at different scales.

Nests built on electric poles showed a regular, uniform distribution (figure 1 in the electronic supplementary material), and including them in the box-counting plot with the rest of nests produced a slight constant curve across scales (figure 3 in the electronic supplementary material), indicating the sensitivity of the method and the presumed constraint that nest substrate disposition could impose on nest distribution in some situations. Since nests occurring in a same tree could seem more clumped in two-dimensional maps than actually occurring in three dimensions, we repeated the analyses considering all the nests in a single substrate (e.g. tree, building) as a single point. Since the patterns were found to occur at higher scales than the nest substrate scale, the same results were obtained with nest substrate as the study units (results not shown).

(c) Analyses of colony size-frequency distributions

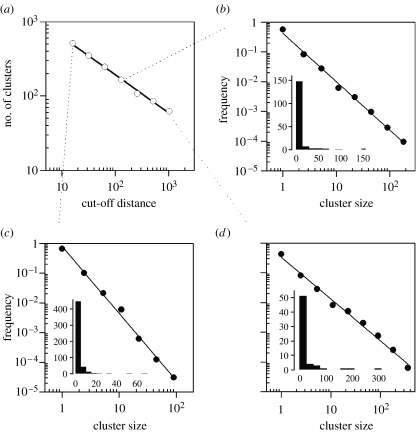
To define clusters, we used a set of different cut-off distances in powers of 2ⁿ for n=4–10, i.e. from 16 to 1024 m, with the aim of using an appropriate range around the cut-offs used to define colonies in this species (e.g. 500 m for the 2005 Spanish National White Stork census; Molina & del Moral 2005). For each distance used, clusters were defined as groups of nests interconnected by shorter distances than the cut-off point. Then, for large nest clusters, a pair of nests could be at larger distances than the cut-off if some other nests continuously fill the gap among them.

Zero frequencies cannot be plotted in log–log axis and thus (among other reasons) logarithmic bins are needed (Pueyo 2006). We used the bins [2ⁿ, 2⁽ⁿ⁺¹⁾−1] for n=0, 1, 2, …, i.e. [1,1], [2,3], [4,7],… nests, and counted the number of clusters within each bin. Then, the mean probability of finding a cluster within a given bin was calculated as the number of clusters in a bin divided by the product of the number of cluster sizes in the interval (i.e. 2ⁿ) and the total number of clusters created (Pueyo 2006). This probability was plotted against the logarithmic mid-point of the interval ${10}^{(\text{log}(2^n)+\text{log}(2^{n+1}-1))/2}$, i.e. 1, 2.45, 5.29, … In one case (figure 4 in the electronic supplementary material) bins in powers of 3ⁿ instead of 2ⁿ were used to ensure that no bin had a zero value.

3. Results and discussion

(a) Nest distribution

It seems paradoxical that although hundreds of nests of white storks may aggregate within a hectare (figure 1), with single trees being crowded with nests (figure 2) and even forming one of the largest colonies known for the species in our study area (Molina & del Moral 2005), we realized that arbitrary decisions should be taken to define colonies. On trying to gain objectivity, we first followed the common practice of placing a ‘reasonable’ cut-off distance above which a nest was said to be out of a colony, colonies being thus formed by nests interconnected by shorter gaps (e.g. Berg et al. 1992; Arroyo et al. 2001). We counted the number of clusters created by imposing a set of increasing cut-off points in successive steps and found (expectedly) that larger cut-offs created less colonies. Less trivially, the relationship between the cut-off point and the number of clusters decayed following a power law (figure 3a and figures 4a–6a in the electronic supplementary material).

Figure 2.

A cluster of white stork nests (and breeding adults) in the study area.

Figure 3.

(a) Power-law relationship between the cut-off distances applied and the number of resulting clusters in 2005. Only nests east of the dashed line in figure 1a are analysed. (b–d) Power-law (R² always greater than 0.99) distribution of nest cluster sizes created through cut-offs of 16, 128 and 1024 m in (b), (c) and (d), respectively. Inset histograms show the same data in lineal–lineal axes binned in intervals of 5, 15 and 25 nests for (b), (c) and (d), respectively. See figures 4–6 in the electronic supplementary material for the other study years.

Power laws define patterns that do not have a characteristic mean, but behave alike across scales (they are scale free, or scale invariant), displaying a lineal relationship in log–log plots (Solé & Bascompte 2006). In our case it means that there was not a single cut-off point objectively better than another. In other words, there was not a natural distance criterion to define colonies (figure 3).

A direct observation of the maps (figure 1) reinforced our uncertainty about what constitutes a colony. Considering the whole study area, much of the space was not used by storks, but nests were clumped in few places (figure 1a). However, these apparently massive clusters of nests (colonies) hid similarly heterogeneous nest distributions, with large unused suitable areas and apparently massive clusters (figure 1b), which also disintegrated in heterogeneous patterns on closer inspection (figure 1c). This suggested to us a hierarchical nest distribution resembling a fractal pattern. We confirmed this supposition through box-counting analyses (Mandelbrot 1977), finding a fractal distribution of nests across three orders of magnitude (from 10 to 10⁴ m) in the 4 study years (figure 4).

Figure 4.

Distribution of nests across scales. Log–log plot of the box-counting analyses, showing a power-law relationship between box side length and the number of occupied boxes for each year (R² always greater than 0.98). Only nests to the east of the dashed line in figure 1a are analysed. (a–d) correspond to data for the different study years ordered from 2002 to 2005. See figure 3 in the electronic supplementary material for comparing results using all the nests in the study area.

Fractal point patterns differ from simple random, uniform or aggregated ones (Mandelbrot 1977). For a clustered pattern (the one closest to the idea of a colonial population), the measures that perfectly describe the system are the distance (mean and variance) between contiguous points (nests) jointly with the distance between adjacent clusters (colonies). In a fractal point pattern, there are no characteristic mean distances, and points are spaced in a hierarchy of clusters across scales (Mandelbrot 1977). Thus, it is an aggregated pattern, but of clusters within clusters at different scales. This scale invariance is further exemplified by the power-law distribution of nearest neighbour distances between nests (figure 7 of electronic supplementary material) and the distances between all pairs of nests (figure 8 of electronic supplementary material). These power-law distributions held between four and five orders of magnitude.

Defining nest distributions using fractal geometry is not just a formal advance for understanding the spatial structure of colonial populations, but offers interesting applications that current methods could not bring us. For instance, by explicitly quantifying the scaling of nest distribution, we easily captured the pattern with a power law as y=ax^b, where b is the slope of the straight line in the box-counting plot. Thus, a messy nest distribution such as in figure 1 becomes a quantifiable pattern allowing statistical treatment and further comparative analyses. In our case, b gradually increased through years (figure 4) in parallel with a steady population growth from 876 breeding pairs in 2002 to 1274 pairs in 2005. Thus, if our study had done only in the first study year, one could have argued that the aggregation of storks was the simple outcome of the aggregation of nest sites. However, the increase in b through years demonstrates that storks were able to aggregate much more in successive years. This is because an increase in b means that in successive years the number of nests within nest clusters increased a lot, while the spread of nests to new places at broader scales was very limited. In other words, it seems that storks actively aggregated, rather than simply matched the spatial distribution of nest substrates. This is also supported by our 25-year monitoring of the spatial distribution of nests in part of our study area, showing that nest concentrations occurred even when there was a surplus of potential nest sites available nearby, e.g. only occupying a small part of a large forested area, while increasing cluster densification in consecutive years (Jovani 2006).

(b) From nest distribution to colony size variation

Conceptually, the fractal distribution of nests tells us that although storks could reach high densities in some places, even with nests touching one another (figure 2), they are not distributed in colonies as previously thought, but rather in a continuous hierarchy of clusters within clusters at different spatial scales. These scaling properties of spacing patterns challenge current practices on a central subject of bird coloniality research, i.e. the study of colony size variation, demanding a multiscale approach to this issue. Despite much interest in colony size variation (Brown et al. 1990), little has been done on formally describing colony size-frequency distributions, only knowing that they tend to be ‘long tailed’ (e.g. Götmark 1982; Brown & Brown 1996; Tella et al. 2001). It has been recently proposed (Schneider 2002), but never tested, that colony size variation could follow a power-law distribution, as also found for human cities (Batty & Longley 1994), animal groups (Bonabeau et al. 1999), bacteria colonies (Buldyrev et al. 2003) and other cluster sizes in biological systems (Solé & Bascompte 2006). Thus, we studied the scaling properties of colony size variation at different spatial scales, rather than assuming a given spatial scale to artificially define colonies.

(c) Colony size variation

Using common histograms (with lineal axis), we found the long-tailed distributions of colony sizes often reported in bird coloniality studies and other studies of animal group sizes or population sizes (Krause & Ruxton 2002; figure 3 and figures 4–6 in the electronic supplementary material). However, this says little about the shape of the distribution because different functions (e.g. exponential, lognormal, power law) display similar long-tailed patterns. Interestingly, when we plotted the same data in log–log axes the frequency distribution of colony sizes followed a power law irrespective of the cut-off used to define colonies and the year analysed (figure 3 and figures 4–6 in the electronic supplementary material). Thus, we have confirmed that power laws could be a good way of describing bird colony sizes. Moreover, we strengthen the relevance of using logarithmic bins rather than lineal ones to address frequency size distributions such as that in colony size variation (see Pueyo 2006; Pueyo & Jovani 2006).

The continuous nature of the distribution of colony sizes (including solitary birds, i.e. colony size =1; figure 3 and figures 4–6 in the electronic supplementary material), and the fractal distribution of nests (figures 1 and 4) suggests that we should understand the density of nests in which birds bred as a continuum including ‘solitary’ individuals, confronting the solitary–colonial dichotomy now used. This revisits an old, intuitive idea that there is a continuum from solitary to semi-colonial and colonial breeding (Coulson & Dixon 1979). More generally, these results intimately link colony size variation and spatial distribution, by realizing that colony size variation was the outcome of the spatial distribution of nests, but not the reverse. This supports empirically the idea that colony size variation is intrinsically related to settlement decisions of individuals (Brown et al. 1990; Serrano & Tella 2007), also reinforcing the recent suggestion that self-organization processes could be behind these (and other) clustering patterns (Camazine et al. 2001; Solé & Bascompte 2006), something than needs further study (May 1999).

4. Conclusions

We have shown that the distribution of nests of a colonial bird population can be quantitatively described using the quantitative and graphical tools of fractal geometry. Moreover, power laws and log–log plots have demonstrated to be quantitatively useful and visually appealing to describe colony size variation and unravel the interrelated hidden scaling problems on the natural patterns of bird distribution and abundance.

Supplementary Material

Supplementary figures

Figure 1, white stork nest distribution at different scales for the year 2005 (complementing figure 1 of the main text); figure 2, diagram of a hypothetic box-counting analysis; figure 3, comparison of the box-counting analysis applied to all nests against the same analysis only for nests West to the dashed line in figure 1; figure 4, nest cluster sizes in the year 2002; figure 5, nest cluster sizes in the year 2003; figure 6, nest cluster sizes in the year 2004; figure 7, frequency distribution of nearest neighbour distances between nests; figure 8, frequency distribution of distances between all pairs of nests