Zinc inhibition of rat NR1/NR2A N-methyl-d-aspartate receptors

Abstract

Zinc ions (Zn²⁺) are localized in presynaptic vesicles at glutamatergic synapses and released in an activity-dependent manner. Modulation of NMDA-type glutamate receptors by extracellular Zn²⁺ may play an important role under physiological conditions and during pathologies such as ischaemia or seizure. Zn²⁺ inhibits NMDA receptors containing the NR2A subunit with an IC₅₀ value in the low nanomolar concentration range. Here we investigate at the single-channel level the mechanism of high affinity Zn²⁺ inhibition of recombinant NR1/NR2A receptors expressed in HEK293 cells. Zn²⁺ reversibly decreases the mean single-channel open duration and channel open probability determined in excised outside-out patches, but has no effect on single-channel current amplitude. A parallel series of experiments demonstrates that lowering extracellular pH (increasing proton concentration) has a similar effect on NR1/NR2A single-channel properties as Zn²⁺. Fitting the sequence of single-channel events with kinetic models suggests that the association of Zn²⁺ with its binding site enhances proton binding. Modelling further suggests that protonated channels are capable of opening but with a lower open probability than unprotonated channels. These data and analyses are consistent with Zn²⁺-mediated inhibition of NMDA receptors primarily reflecting enhancement of proton inhibition.

The NMDA subtype of glutamate receptor ion channels is a non-selective cation channel with high calcium permeability that has been implicated as playing a critical role in both physiological and pathophysiological processes. NMDA receptor function has been demonstrated to be regulated by both intracellular modulators, such as kinases and scaffolding proteins, and extracellular modulators including redox agents and various ions (Dingledine et al. 1999; Erreger et al. 2004). Two such extracellular modulators whose range of activity probably falls within the concentrations present during physiological and/or pathological conditions are zinc ions (Zn²⁺) and protons (pH).

Zn²⁺ has been demonstrated to be localized in synaptic vesicles at glutamatergic presynaptic terminals (Salazar et al. 2005) and has been suggested to be released in an activity-dependent manner, making it an important potential modulator of glutamate receptor function (Smart et al. 2004). However, a wide range of values have been suggested for Zn²⁺ concentrations in the synaptic cleft following glutamate release, and thus the quantity of Zn²⁺ released from presynaptic terminals remains controversial (Ueno et al. 2002; Kay, 2003; Frederickson et al. 2006).

NMDA receptors containing the NR2A subunit exhibit high affinity voltage-independent inhibition by Zn²⁺ (Williams, 1996; Chen et al. 1997; Paoletti et al. 1997). The molecular determinants of high affinity zinc binding have been demonstrated to lie in the amino terminal domain of the NR2A subunit (Choi & Lipton, 1999; Low et al. 2000; Paoletti et al. 2000; Hatton & Paoletti, 2005). The extracellular amino terminal domain is present in all ionotropic glutamate receptors, and is thought to adopt a clamshell-like organization with some homology to bacterial amino acid binding proteins. Zn²⁺ binding to NR2A and polyamine binding to NR2B are the only known native ligands for the amino terminal domain (Masuko et al. 1999; Paoletti et al. 2000), although ifenprodil and its analogues are synthetic molecules that bind selectively to the NR2B amino terminal domain (Perin-Dureau et al. 2002; Wong et al. 2005). The amino terminal domain has also been suggested to play a role in subunit dimerization and receptor assembly (Leuschner & Hoch, 1999; Ayalon & Stern-Bach, 2001; Meddows et al. 2001; Ayalon et al. 2005).

Extracellular proton concentration (commonly expressed as pH =−log₁₀[H⁺]) is normally kept under tight physiological control (Chesler, 2003). However, the inside of glutamatergic synaptic vesicles has an exceptionally high proton concentration (pH 5.7) (Miesenbock et al. 1998), suggesting that vesicle release under conditions of high activity might be capable of acidifying the local extracellular environment and modifying synaptic NMDA receptor function through a pH-dependent mechanism (DeVries, 2001). In addition, activity-dependent alkalinization of the extracellular space can also influence NMDA receptor response amplitudes (Gottfried & Chesler, 1994; Makani & Chesler, 2007). NMDA receptor overactivation mediates some forms of excitotoxicity, and proton inhibition has been proposed to be one endogenous neuroprotective mechanism to attenuate NMDA receptor activation in ischaemic conditions associated with acidification and high levels of glutamate release (Tombaugh & Sapolsky, 1993). Protons inhibit NMDA receptors in a voltage-independent manner with an IC₅₀ within the physiological range: 30–120 nm H⁺ for NR1/NR2A (Low et al. 2000). Extensive site-directed mutagenesis throughout both NR1 and NR2 subunits has implicated a highly conserved region among glutamate receptors at the extracellular end of the second transmembrane domain (and excluded other parts of the protein) as an important determinant of proton inhibition (Low et al. 2003). This region contains the residue at which the ‘lurcher’ mutation was originally identified in the d-serine-binding δ2 glutamate receptor subunit (Naur et al. 2007). Mutation of this residue in δ2, GluR1, GluR6 or NR1 results in either constitutive activation in the absence of agonist or in greatly increased apparent agonist affinity, suggesting a critical role for this region in channel gating (Kohda et al. 2000; Taverna et al. 2000; Klein & Howe, 2004; Vogel et al. 2006).

Zinc and proton inhibition are recognized to share common structural determinants (Traynelis et al. 1998). Multiple lines of evidence have suggested that high affinity Zn²⁺ inhibition acts through enhancing sensitivity of NMDA receptors to inhibition by extracellular protons (Choi & Lipton, 1999; Low et al. 2000; Zheng et al. 2001; Erreger & Traynelis, 2005). That is, binding of zinc has been proposed to shift the sensitivity (pKa) of the proton sensor such that protonation is enhanced at physiological pH. Consistent with this hypothesis, we report here that Zn²⁺ and protons similarly modulate single-channel activity of recombinant NR1/NR2A by both reducing mean channel open time and reducing channel open probability. Kinetic analysis of single-channel recordings suggests that Zn²⁺ inhibition reflects enhancement of proton sensitivity.

Methods

Human embryonic kidney 293 (HEK293) cells were maintained and transiently transfected by the calcium phosphate method with cDNA encoding NR1-1a (GenBank accession numbers U11418 and U08261; pCIneo vector; hereafter called NR1), NR2A (D13211; pCIneo), and green fluorescent protein at a ratio of 1 : 2 : 1 (0.2 μg ml⁻¹ NR1) for 4–12 h, as previously described (Zheng et al. 1998). Currents from outside-out patches were digitally recorded with pClamp8 software using an Axopatch 200B amplifier (Molecular Devices, Union City, CA, USA). Single-channel records were filtered at 5 kHz using an eight-pole Bessel filter (−3 dB; Frequency Devices, Haverhill, MA, USA) and digitized at 40 kHz. Thick-walled borosilicate glass (1.5 mm outer diameter; 0.85 mm inner diameter; Warner Instruments) was fire polished to a resistance of 6–9 MΩ, and Sylgard (Dow Corning, Midland, MI, USA) was applied to the pipette tip. The extracellular solution consisted of (mm): 150 NaCl, 10 Hepes, 10 tricine, 0.5 CaCl₂, 3 KCl with 50 μm glycine and 1 mm glutamate (pH 7.3, 23°C). For some experiments, pH was adjusted to 6.7 by addition of HCl. The internal solution consisted of (mm): 110 caesium gluconate, 30 CsCl, 5 Hepes, 4 NaCl, 0.5 CaCl₂, 2 MgCl₂, 5 BAPTA, 2 Na-ATP and 0.3 Na-GTP (pH 7.35). Tricine-buffered Zn²⁺ solutions were prepared using a binding constant of 10⁻⁵m with WINMAX software (http://www.stanford.edu/~cpatton/maxc.html). The tricine concentration was 10 mm and the total Zn²⁺ concentration was 27 μm, which gives a free Zn²⁺ concentration of 300 nm. Free proton concentrations were calculated with an activity coefficient of 0.8. No voltage correction was applied for the junction potential.

Records were idealized with a segmental k-means algorithm (Qin, 2004) using QuB software (http://www.qub.buffalo.edu). Use of 0.5 mm extracellular Ca²⁺ decreased the frequency of lower subconductance level openings. All conductance levels were assumed to be equal for the analysis. In order to segment data into bursts, we calculated a critical shut time (T_crit) that would separate the three fastest shut time components from longer closed times. We calculated T_crit to minimize the total number of misclassified events (Jackson et al. 1983; Colquhoun & Sigworth, 1995; Erreger et al. 2005a). T_crit values for NR1/NR2A channel records shown in Fig. 1 were 32 ms for control conditions (< 1% events misclassified) and 37 ms for recordings made in the presence of 300 nm Zn²⁺ (3% of events misclassified). As these values are virtually indistinguishable from the T_crit value of 30 ms used in previous studies of NR1/NR2A channels (Erreger et al. 2005a,b), we used 30 ms to identify bursts here to allow more direct comparison of our analyses with these previous results. Bursts with multiple channels simultaneously open were not analysed. Dwell-time histograms were generated and fitted using Channelab (http://www.synaptosoft.com) with an imposed dead time of 50 μs. Maximal interval likelihood fitting (MIL; Qin et al. 1996) was performed with an imposed dead time of 50 μs using QuB software; similar results were obtained when a dead time of 100 μs was imposed (data not shown).

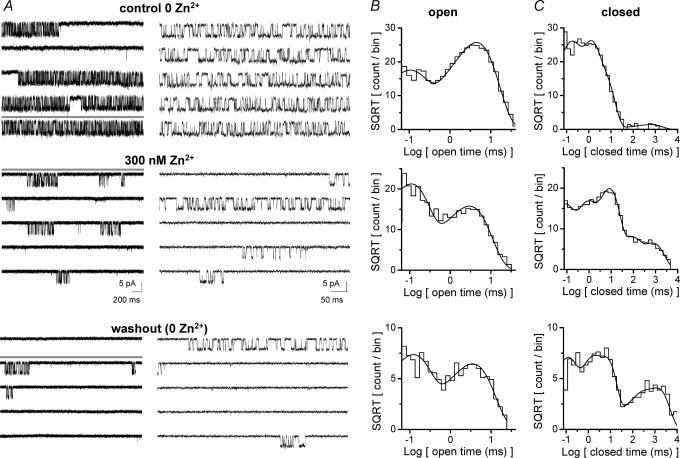
Figure 1. Zn²⁺ alters the kinetic properties of recombinant NR1/NR2A channels in outside-out patches from HEK293 cells.

A, outside-out patches were exposed to 1 mm glutamate and 50 μm glycine under control (0 Zn²⁺, 10 mm tricine), zinc (300 nm free Zn²⁺ buffered by 10 mm tricine), and washout (0 Zn²⁺, 10 mm tricine; not shown). The holding potential was −80 mV. Currents were sampled at 40 kHz and filtered at 5 kHz for analysis, but are sampled at 5 kHz and filtered at 2 kHz for display. Representative current traces from a patch with only a single active channel are displayed on two different time scales for each condition; downward deflections reflect channel openings. The thick grey line on the left trace indicates the portion displayed at a higher time resolution in the right trace. B and C, the distribution of open (B) and closed (C) duration dwell times is plotted for each condition for the same patch as panel A. The open dwell time distribution from this patch was fitted by the sum of two exponential components with time constants and relative amplitudes of τ1 0.09 ms (29%), τ2 4.5 ms (71%) for control; τ1 0.10 ms (63%), τ2 3.0 ms (37%) for 300 nm Zn²⁺; τ1 0.12 (55%), τ2 3.5 ms (45%) for washout. The closed dwell time distribution was fitted by the sum of four or five exponential components with time constants and relative amplitudes of τ1 0.13 ms (37%), τ2 1.0 ms (40%), τ3 4.0 ms (22%), τ4 590 ms (1%) for control; τ1 0.07 ms (23%), τ2 0.63 ms (22%), τ3 8.0 ms (44%), τ4 72 ms (6%), τ5 720 ms (5%) for 300 nm Zn²⁺; and τ1 0.10 ms (28%), τ2 1.0 ms (19%), τ3 6.0 ms (35%), τ4 210 ms (5%), τ5 1300 ms (13%) for washout. We recorded 7901 openings under the control condition, 5098 openings in the presence of 300 nm Zn²⁺, and 790 openings following washout of Zn²⁺ from this patch.

For patches with no double openings, the control period was analysed with the following equation (Colquhoun & Hawkes, 1995):

(1)

P is the probability of the number of observed single openings before observing a double opening in a patch with two channels. P_ON is the observed probability of being open in the experimental record; n is the number of single openings observed. We are aware that the burst structure of NR2A openings is not ideal for using this approximation to determine the number of channels in a patch. We therefore re-analysed the data based on the probability of the observed number of bursts before observing overlapping bursts in a patch with two channels (Colquhoun & Hawkes, 1995). Similar results are found with this alternate analysis, which also suggests these patches contain a single active channel.

The distribution of lengths of open periods adjacent to closed times within a specified range was determined. Conditional open duration distributions were constructed from either the preceding or following opening adjacent to a closed time of a specified length. Identical results were obtained from openings preceding or following closed periods, as expected if the channel obeys the law of microscopic reversibility. The closed duration intervals used to define the conditional open durations were chosen from critical times calculated to minimize the total number of misclassified events (Jackson et al. 1983) in the fitted shut time distribution obtained from uninterrupted recordings of NR1/NR2A channel activity in response to supramaximal co-agonist concentrations from a patch that contained only one active channel (see Fig. 1). In addition, the runs test was applied to apparent single-channel open durations as previously described (Colquhoun & Sakmann, 1985; Colquhoun & Sigworth, 1995) using a critical open time of 0.5 ms.

Statistical analysis was performed in Prism 3 (Graphpad, San Diego, CA, USA). Student's t test was employed for single comparisons and ANOVA with Tukey's post hoc test was employed for multiple comparisons. All data are expressed as mean ±s.e.m.

Results

Inhibition of NR1/NR2A channels by extracellular Zn²⁺

Recombinant NR1 and NR2A NMDA receptor subunits were transiently transfected into HEK293 cells. Patch clamp recording was performed in the outside-out patch configuration with a maximally effective concentration of glycine (50 μm) and glutamate (1 mm) at a voltage of −80 mV. Typically, control data (0 Zn²⁺, 10 mm tricine, pH 7.3) were collected for 5 min, followed by 5 min in the presence of 300 nm free Zn²⁺ (buffered by 10 mm tricine), and finally a washout back to the control condition for 5 min. A concentration of 300 nm Zn²⁺ was chosen to saturate the high affinity inhibition (IC₅₀≈ 30 nm) (Williams, 1996; Chen et al. 1997; Paoletti et al. 1997; Choi & Lipton, 1999; Low et al. 2000; Zheng et al. 2001) but not induce low affinity voltage-dependent channel block (IC₅₀≈ 30 μm) (Williams, 1996; Chen et al. 1997; Paoletti et al. 1997). Of seven patches that met our criteria for analysis, two patches exhibited no double channel openings over the course of the 15 min of recording. Based on a simple approximation of the probability of observing a given number of single openings from a patch that actually contained two channels (see Methods), we calculate this probability as P < 0.001 for the control period of each of these two patches, suggesting that they probably contain a single functional channel. One of these patches with 7901 channel opening events without any double channel openings during the control period is shown in Fig. 1. The open time distribution was fitted with the sum of two exponential components (Fig. 1B). The distribution of closed times was complex, and could be fitted with at least four exponential components (Fig. 1C). While the overall channel open probability runs down over the course of the recording, as manifested by increasing frequency of long closed times, channel properties within a burst of channel openings are identical for the initial control period compared to the washout (see Figs 2, 3 and 7 below).

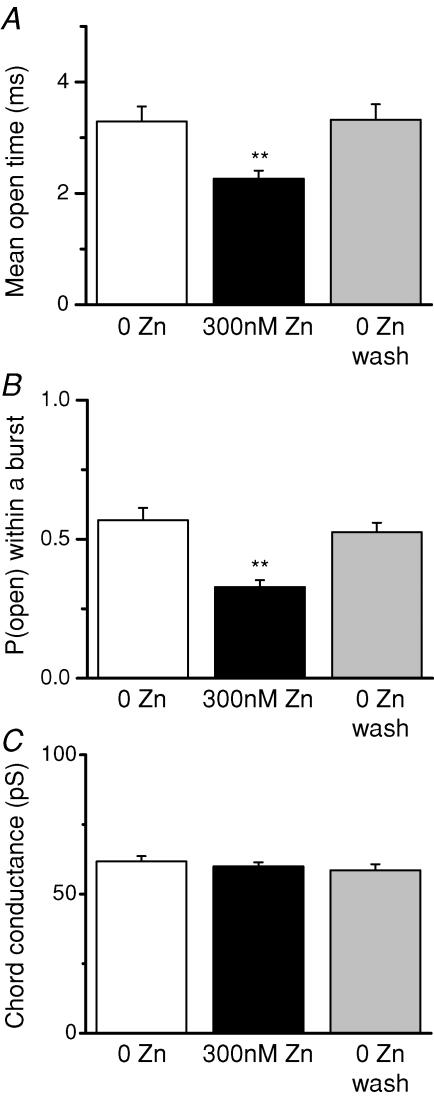
Figure 2. Zn²⁺ decreases the mean channel open time and open probability within a burst but does not alter the single-channel current amplitude.

A, the mean channel open time is reversibly decreased by 300 nm extracellular Zn²⁺ from 3.3 ± 0.3 to 2.3 ± 0.2 ms (n = 7). B, the open channel probability within a burst is reversibly decreased by Zn²⁺ from 0.57 ± 0.04 to 0.33 ± 0.02 (n = 7); burst or individual activations were identified with a 30 ms critical time (see Methods). C, the chord conductance determined at −80 mV is not altered by extracellular Zn²⁺ (n = 7). **P < 0.01, repeated-measures ANOVA with Tukey's post hoc test.

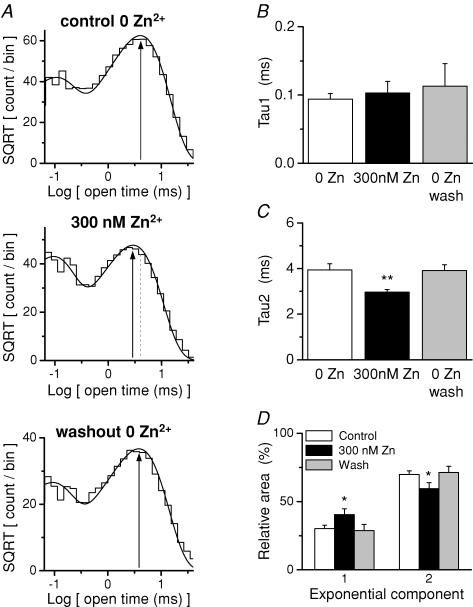
Figure 3. Zn²⁺ alters the distribution of open channel dwell durations.

A, the distribution of open times for each condition pooled among all 7 patches is shown. Vertical lines indicate the second fitted time constant, which is accelerated by 300 nm extracellular Zn²⁺. B, the mean of the briefer open time constant from fits to individual patches is displayed for all conditions. C, the mean of the longer fitted open time constant is displayed for the various conditions. D, the effect of Zn²⁺ on the mean relative amplitude of each time constant is summarized. For all panels n = 7. *P < 0.05 and **P < 0.01, repeated-measures ANOVA with Tukey's post hoc test.

Figure 7. Maximum interval likelihood fitting of Zn²⁺ and pH modulation of channel activity.

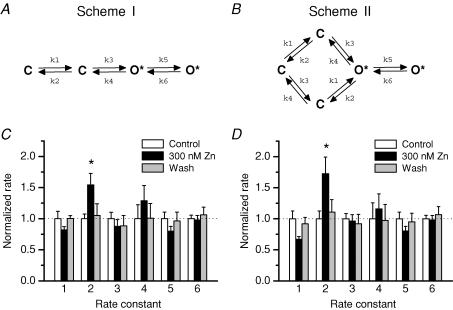
Recordings were performed in the presence of a saturating concentration of agonist and divided into segments to remove desensitization. Therefore explicit agonist binding and desensitization rates are not included in the models. A, Scheme I is a simplified model of gating with two sequential gating steps. In this scheme, ‘C’ represents closed non-conducting states and ‘O*’ represents open conducting state. B, Scheme II is a model with two independent gating steps connected in a manner to allow them to open in either order. This model has previously been suggested to represent a slow NR2-dependent step (k₁/k₂) and a fast NR1-dependent step (k₃/k₄) (Banke & Traynelis, 2003). C and D, each rate constant was normalized to the mean value of the control condition for all 7 patches in the Zn²⁺ data set. The raw values for the rate constants are given in Table 1. *P < 0.05, repeated-measures ANOVA with Tukey's post hoc test.

For all patches, the data were segmented into bursts based on a critical shut time of 30 ms as a burst terminator (Erreger et al. 2005a). Functional channel properties within a burst were then determined (Fig. 2). The similarity in results from patches that probably contain a single channel and patches with multiple channels supports our choice of 30 ms for a critical shut time for burst identification. The presence of 300 nm Zn²⁺ caused a significant 30 ± 3% reduction in the mean channel open time (P < 0.01; n = 7; ANOVA; Fig. 2A) as well as a 40 ± 5% reduction in open probability within a burst (P < 0.01; n = 7; ANOVA; Fig. 2B). The open probability was calculated as the fraction of time in the open state for all events for each patch after segmentation into bursts, and was 0.55 under control conditions, consistent with previous reports (Erreger et al. 2005a). The effects of extracellular Zn²⁺ on mean open time and on open probability were completely reversed upon washout of Zn²⁺. NR1/NR2A receptors primarily open to a single conductance level of ∼60 pS in the presence of 0.5 mm extracellular free Ca²⁺ (Fig. 2C). Zn²⁺ (300 nm) had no effect on the single-channel chord conductance at −80 mV.

The composite distribution of channel open durations pooled from all patches could be best fitted with two exponential components (Fig. 3A). Open time distributions from individual patches were also fitted with two exponential components (Fig. 3B–D). The time constant for the shorter component (τ1) was unchanged by 300 nm Zn²⁺ (Fig. 3B). By contrast, 300 nm Zn²⁺ reversibly decreased the time constant for the longer component (τ2; Fig. 3C). In addition, the relative area of τ1 in the distribution was reversibly increased by Zn²⁺ (Fig. 3D). The shift in the open time suggests that Zn²⁺-bound receptors retain the ability to open, consistent with the incomplete inhibition by saturating Zn²⁺ (Paoletti et al. 1997; Low et al. 2000; Erreger & Traynelis, 2005; Hatton & Paoletti, 2005).

Correlations between the lengths of an open time and an adjacent closed time can provide information about the mechanism of channel activation. Correlations between the duration of open and closed times have previously been described for recombinant NR1/NR2A receptors recorded in outside-out patches excised from Xenopus laevis as well as native NMDA receptors in CA1 pyramidal cells (Gibb & Colquhoun, 1991; Schorge et al. 2005; Wyllie et al. 2006). However, Zhou & Auerbach (2005) did not observe correlations in cell-attached patch recordings of NR1/NR2A receptors in response to maximal concentrations of co-agonists. To evaluate whether correlations existed in our data set and to evaluate the effect of extracellular Zn²⁺ on potential correlations, we first performed a runs test for correlations among open times in patches that contain only one active channel using a critical open time of 0.5 ms (Colquhoun & Sigworth, 1995). This analysis yielded strong support of runs of short and long duration openings for recordings both in control conditions and in the presence of 300 nm Zn²⁺ with the z statistic ranging between −8.1 and −17.7.

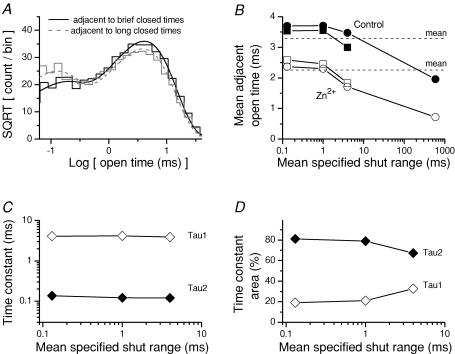
We subsequently constructed conditional distributions of adjacent intervals to examine the strength of any correlations in the data record. Figure 4A shows conditional distributions of apparent intra-burst open times adjacent to brief (0.05–0.27 ms) or prolonged (2.65–32.6 ms) intra-burst closed times. The distribution of open times adjacent to brief closed times shows similar fitted time constants as the distribution of open times adjacent to prolonged closures, but an increase in the area of the slower component. This trend could be seen in the analysis of the mean open time adjacent to closed times in a specified range. We constructed this relationship for intra-burst open times determined from all patch recordings, as well as from all open times in two patches that appear to contain a single active channel (Fig. 4B). As previously reported for recordings in outside-out patches (Gibb & Colquhoun, 1991; Schorge et al. 2005; Wyllie et al. 2006), this analysis showed a negative correlation, in that on average open times adjacent to longer closed times were shorter. The magnitude was similar to previous reports. Importantly, the correlations persisted in the presence of saturating concentration of extracellular Zn²⁺. Evaluation of the fitted time constants to the conditional open time distributions showed the change in mean open time reflected a change in the area of fitted time constants rather than any change in either time constant (Fig. 4C and D).

Figure 4. Correlations between open and closed durations of NR1/NR2A channels in outside-out patches from HEK293 cells.

A, the closed time histogram from a patch with a single active channel recorded in response to maximal concentrations of agonist (Fig. 1) was used to determine critical closed times to separate the four fitted shut time components as described in the Methods (Jackson et al. 1983). Critical times were 0.27, 2.65 and 32.6 ms. Conditional distributions were constructed from pooled data from all patches for intra-burst apparent open durations adjacent to a brief closed durations in the range of 0.05–0.27 ms (continuous black line) or adjacent to longer duration closed times in the range of 2.65–32.6 ms (broken grey line). The distributions and respective fitted exponential components for openings adjacent to brief closures were scaled to contain the same number of events as distributions for open times adjacent to long closed times. B, the mean of conditional apparent intra-burst open durations (squares) pooled from all patches recorded in control conditions or in 300 nm Zn²⁺ are plotted against the fitted time constants describing the closed time distribution (Fig. 1). The mean apparent open times determined from two patches that contained one active channel are shown as circles. The critical times defining each closed duration range were determined from the histogram in Fig. 1C, and were (in ms) 0.05–0.27, 0.27–2.65, 2.65–32.6 and 32.6–10 000. The dashed lines show the mean intra-burst open durations. C, the fitted time constants of exponential components describing the conditional open duration distributions for recordings in the absence of Zn²⁺ are shown. Fitted intra-burst time constants were (for τ1) 0.14, 0.12 and 0.12 ms and (for τ2) 4.1, 4.2 and 3.9 ms. D, the areas of the two time constants from the fitted conditional distributions are shown, and were (for τ1) 19, 21 and 33% and (for τ2) 81, 79 and 67%.

Inhibition of NR1/NR2A channels by extracellular protons

Previous studies have demonstrated a functional link between the inhibition of NR1/NR2A NMDA receptors by Zn²⁺ and protons (Choi & Lipton, 1999; Low et al. 2000). We therefore examined the effects of reduced pH (increased proton concentration) on NR1/NR2A single-channel properties. We selected a proton concentration (250 nm H⁺, pH 6.7) that was known to cause a similar level of steady-state inhibition at the macroscopic level as a saturating Zn²⁺ concentration (∼60% inhibition) when compared to the control condition (62 nm H⁺, pH 7.3; Low et al. 2000). If extracellular Zn²⁺ acts through enhancement of proton inhibition, we predict that this concentration of protons should have similar effects on single-channel properties as Zn²⁺. Figure 5A shows a representative recording from an outside-out patch at pH 7.3 and pH 6.7. Open time histograms in this patch could be fitted by the sum of two exponential components. Similar to the effects of 300 nm Zn²⁺, increasing the extracellular H⁺ concentration accelerated the second time constant in this patch (Fig. 5B).

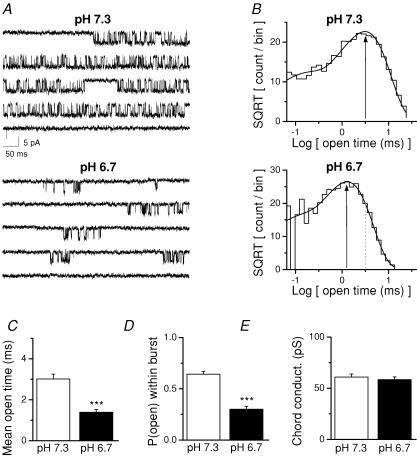
Figure 5. Increased proton concentration reduces the open probability of NR1/NR2A channels in outside-out patches.

A, representative current traces from the same patch are shown at two different extracellular pH values. B, the distribution of open dwell times is plotted for each pH for the same patch shown in panel A. The open dwell time distribution was fitted by the sum of two exponential components with time constants and relative amplitudes of τ1 0.11 ms (15%), τ2 3.1 ms (85%) for control pH 7.3 and time constants of τ1 0.07 ms (15%), τ2 1.3 ms (85%) for pH 6.7. Vertical lines show the second fitted time constant. 6140 openings were recorded at pH 7.3 and 8889 openings at pH 6.7. C, the mean channel open time (n = 5 patches) was decreased in low pH. D, the mean open channel probability within a burst was also decreased by low pH (n = 5). E, the chord conductance at −80 mV was not altered by low pH (n = 5). For all panels, ***P < 0.001, paired t test.

We subsequently analysed data from five patches in which we recorded both at pH 7.3 and 6.7. Increasing the proton concentration to 250 nm (pH 6.7) significantly decreased mean channel open time from 3.0 ± 0.2 to 1.4 ± 0.1 ms (Fig. 5C). Reduced pH also decreased the open probability within a burst from 0.64 ± 0.03 to 0.30 ± 0.03 (Fig. 5D), consistent with the effects of 300 nm extracellular Zn²⁺. In addition, reduced extracellular pH did not alter the main unitary channel conductance level recorded from NR1/NR2A receptors in the presence of 0.5 mm Ca²⁺, again consistent with the effects of Zn²⁺ (Fig. 5E). Figure 6 shows that the effect of pH on the distribution of open times pooled from all five patches is similar to the effect of 300 nm Zn²⁺ (compare with Fig. 3). That is, increased proton concentration decreased the time constant for the longer open time component (τ2). Thus, the effects of proton inhibition on single-channel currents recorded in outside-out patches largely mirror properties of Zn²⁺ inhibition on recombinant NR1/NR2A. These data are consistent with the hypothesis that Zn²⁺ binding enhances proton inhibition.

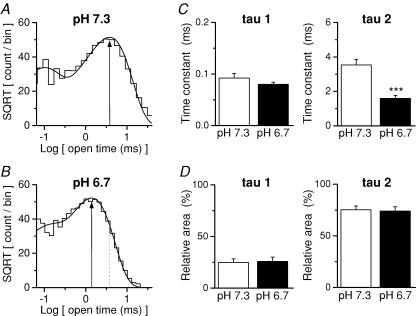
Figure 6. Increased proton concentration alters the distribution of open channel dwell durations.

A and B, the distribution of open times pooled among all 5 patches is shown at pH 7.3 (A) and pH 6.7 (B). 27 613 openings were recorded at pH 7.3 and 34 327 openings at pH 6.7. C, the mean fitted time constants for the open time distribution are displayed (n = 5). D, the mean relative areas for the fitted time constants for all five patches are shown. ***P < 0.001, paired t test.

Kinetic analysis of Zn²⁺ inhibition of NR1/NR2A channels

In order to gain some mechanistic insight into the effects of Zn²⁺ on NR1/NR2A receptor gating, we fitted the sequence of single-channel openings and closings for each patch with explicit models of channel function using maximum interval likelihood fitting (MIL, see Methods; Fig. 7A and B). Data were fitted by two models, a linear model (Scheme I) and a cyclic gating model (Scheme II), both of which were based on previously published models of NMDA receptor function (Popescu & Auerbach, 2003; Popescu et al. 2004; Erreger et al. 2005a,b; Schorge et al. 2005; Zhou & Auerbach, 2005). Two explicit open states were included and were assumed to be interconnected in the models (Popescu & Auerbach, 2003; Zhou & Auerbach, 2005). All states are fully liganded as all data fitted were in the presence of saturating agonist concentrations. Scheme I represents sequential conformational changes leading to gating (Popescu & Auerbach, 2003; Popescu et al. 2004; Zhou & Auerbach, 2005) and Scheme II represents an independent two-step gating model in which the receptor undergoes separate conformational changes required for gating in any order (Banke & Traynelis, 2003; Erreger et al. 2005a,b; Schorge et al. 2005). The models are not equivalent because the first open state encountered has different connectivity. The effect of Zn²⁺ on the fitted rate constants for each transition in both models is displayed in Fig. 7C and D. The mean rate constants averaged across all patches are given in Table 1. Average rates were virtually identical to the rates derived from global fitting of the pooled data from all patches. Extracellular Zn²⁺ significantly altered only the reverse rate of the slowest transition preceding channel opening (k₂) in both models. This is the analogous transition that is modified by partial agonists acting at the glutamate binding site (Erreger et al. 2005b) and the analogous rate that controls the main difference in gating between NR1/NR2A and NR1/NR2B receptors (Erreger et al. 2005a). Whereas the experimentally determined mean open time was reduced by 300 nm extracellular Zn²⁺, none of the average rates into or out of the open states in the model were statistically significantly different based on fits to individual patches. However, there was a clear increase in the closing rate (k₄) from the briefer gateway open state derived from the global fit of the pooled data (Table 1). The mean open times predicted from Schemes I and II changed from 2.9 and 3.1 ms in control to 1.9 and 1.9 ms in 300 nm Zn²⁺, respectively. The change in k₂ is consistent with a Zn²⁺-induced reduction in the open probability, since the reverse of the initial pre-gating step in this model is accelerated in Zn²⁺-bound receptors. As expected, the open probability was reduced for Schemes I and II from 0.56 and 0.61 in control to 0.30 and 0.29 in 300 nm Zn²⁺, respectively. These changes are consistent with our single-channel data (Fig. 2).

Table 1.

Idealized and segmented current records from the same patches were fitted with the models shown in Fig. 7A and B

	Control			300 nm Zn2+			Washout
	Mean	s.e.m.	Global fit	Mean	s.e.m.	Global fit	Mean	s.e.m.	Global fit
Scheme I
k1	460	55	430	380	28	380	460	29	450
k2	1500	120	1400	2200	310	2300	1600	370	1600
k3	2400	250	2300	2000	290	1900	2100	530	2100
k4	3800	840	2900	4600	1100	5000	3800	1200	3900
k5	6500	700	6300	5100	560	5800	6200	1200	7200
k6	880	50	910	880	70	820	930	140	830
logL/event	5.13	—	5.11	5.01	—	4.99	4.99	—	5.05
Scheme II
k1	300	38	280	200	13	210	280	31	280
k2	880	110	760	1500	240	1600	980	180	1100
k3	3200	260	3000	3000	330	2800	2900	480	2800
k4	2900	730	2200	3300	690	3400	2800	740	2900
k5	6500	690	6300	5200	480	5800	6100	900	7200
k6	880	49	900	880	69	820	940	110	830
logL/event	5.12	—	5.11	5.01	—	4.99	4.99	—	5.05

All rates have units of s⁻¹. Data from each patch were fitted independently and the mean values and standard errors are shown (n = 7). Additionally, records from all 7 patches were pooled and fitted simultaneously with the result given as the global fit. The total number of openings was 43 526 for control, 31 200 for Zn²⁺, and 16 384 for washout. Rate constants are given to two significant digits. For all tables, the logarithm of the maximum likelihood determined during MIL fitting is given normalized to the number of channel dwell times (logL/event).

Zn²⁺ enhances proton sensitivity of NR1/NR2A single channels

The kinetic analysis described in Fig. 7 suggests that Zn²⁺ binding to NR2A changes the rates of specific gating steps, rather than causing a global modification of all aspects of receptor function. However, this model does not take into account previous data (Choi & Lipton, 1999; Low et al. 2000; Erreger & Traynelis, 2005) suggesting that Zn²⁺ binding to the NR2A amino terminal domain enhances inhibition by extracellular protons. The binding of two different ions (Zn²⁺ and protons) to a complex gating scheme provides a myriad of potential models to consider (see for example Banke et al. 2005). Rather than fitting all possible kinetic schemes that include individual proton and Zn²⁺ binding steps as well as a full reaction scheme, we focused on progressively expanding the linear model shown in Scheme I (Fig. 7) by adding a protonated arm. We used a series of related models to explore how variation of the states that could be protonated, variation of open probability of protonated states, and conservation of rates between protonated and unprotonated arms impacted the ability of the models to reproduce our data.

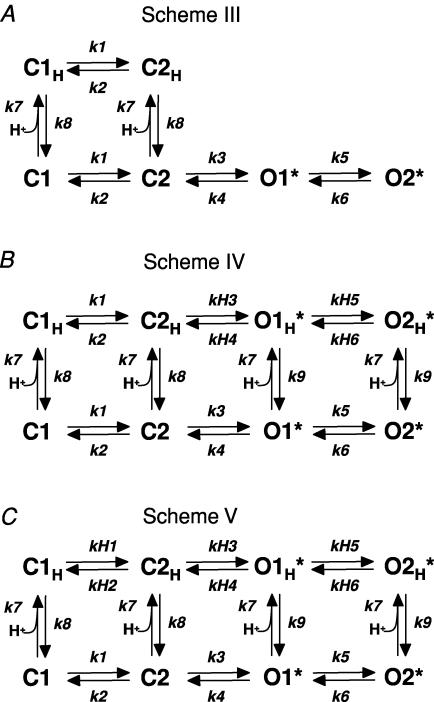
We first examined conceptual ideas of how Zn²⁺ might influence receptor protonation. We fitted the three models shown in Fig. 8 to our data set from seven patches in which we recorded channels in both control conditions and 300 nm Zn²⁺. This dataset included two patches with no double openings, and which probably contain one active channel. Recordings of channel activity in all patches were segmented into bursts, and the data were pooled and analysed as described in the Methods. Each model was fitted to data in the absence of extracellular Zn²⁺ (i.e. 10 mm tricine) and again in the presence of 300 nm Zn²⁺. We assumed that Zn²⁺ binding had reached equilibrium and was nearly saturating at 300 nm (Low et al. 2000). Each model was fitted to single-channel data using the maximum interval likelihood method (MIL, see Methods). All models reproduced our key observations of a change in open probability within a burst and shortening of the mean open time. The mean open time in control conditions was 3.6 ms, and was reduced to 2.1–2.7 ms in 300 nm Zn²⁺ for Schemes III and IV, respectively. Similarly, the open probability within a burst was reduced from 0.60–0.62 in control to 0.29–0.30 in Zn²⁺ for these models. Table 2 summarizes the rate constants derived from the global fits to the data.

Figure 8. Conceptual models of Zn²⁺ enhanced proton sensitivity.

A, Scheme III is a conceptual model that includes explicit protonation steps from closed states. B, Scheme IV is an extension of this model with protonation steps from open and closed states, with pregating rates fixed to be equal for protonated and unprotonated states. C, Scheme V is a modification of Scheme IV in which all gating rate constants are allowed to vary independently for protonated and unprotonated closed states. The proton association rate in Scheme V is fixed to that determined from proton concentration jump experiments (Banke et al. 2005); all other rates are free parameters in the model. Fitted rate constants are given in Tables 2 and 3.

Table 2.

Idealized and segmented current records from the same patches were fitted with the models shown in Fig. 8A and B

	Scheme IIl				Scheme IV
		Control	300 nm Zn2+	Zn2+/Control	Control	300 nM Zn2+	Zn2+/Control
k1 (s−1)	940	2200	2.34	k1 (s−1)	630	560	0.89
k2 (s−1)	2300	5900	2.57	k2 (s−1)	1700	2400	1.41
k3 (s−1)	2900	3500	1.21	k3 (s−1)	2800	3000	1.07
k4 (s−1)	3000	5300	1.77	k4 (s−1)	2500	2900	1.16
k5 (s−1)	6300	5800	0.92	k5 (s−1)	6400	6300	0.98
k6 (s−1)	900	820	0.91	k6 (s−1)	1000	1100	1.10
k7 (m−1 s−1)	1.5e+9	1.5e+10	10	k7 (m−1 s−1)	3.5e+7	2.6e+8	7.42
k8 (s−1)	180	380	2.11	k8 (s−1)	4.1	4.6	1.12
				k9 (s−1)	29	33	1.14
				kH3 (s−1)	820	980	1.20
				kH4 (s−1)	5200	6700	1.29
				kH5 (s−1)	5700	5100	0.89
				kH6 (s−1)	890	870	0.98
logL/event	5.12	5.00	—	logL/event	5.14	5.02	—

Records from all 7 patches were pooled and fitted simultaneously. First, data obtained in the absence of Zn²⁺ (i.e. in the presence of 10 mm tricine) were fitted, and subsequently the same model was fitted to data recorded from the same 7 patches in the presence of 300 nm extracellular Zn²⁺. The proton concentration was 62 nm (pH 7.3). All loops were constrained in all models to obey microscopic reversibility. Rates in bold indicate more than a 3-fold change in the presence of Zn²⁺. Rate constants are given to two significant digits.

Scheme III shows a linear model in which only the closed states can become protonated, with gating rates of the protonated receptor held identical to rates of the unprotonated receptor (Banke et al. 2005). All other rates were allowed to vary in the absence and presence of Zn²⁺. The dominant effect of Zn²⁺ on the fitted rate constants in Scheme III was an acceleration of the proton association rate (k₇, Table 2); there were more modest changes in channel closing rate (k₄), the forward and reverse rate constants for the initial gating step (k₁ and k₂), and the proton dissociation rate (k₈). However, this model is conceptually unsatisfactory because there is no link between the effects of protons and a reduced mean open time, as implied by data in Figs 5 and 6. We subsequently considered providing a direct protonation path from the open state that leads to channel closure, to allow acceleration of channel closure in high proton concentrations through protonation (and closure) of open channels. However, a conceptual disadvantage of this approach is the representation of two different events (protonation and channel closure) as a single step. Scheme IV circumvents this problem by separating protonation and channel closing steps. To fit this model, we forced proton association rates for closed and open states to be equal, but allowed proton dissociation rates to differ for open and closed states. This allowed variation of closing rates of protonated and unprotonated channels while maintaining microscopic reversibility; all loops were held in thermodynamic balance during fitting. The primary change in fitted rate constants between control conditions (no Zn²⁺) and 300 nm Zn²⁺ is an enhancement of proton sensitivity that is manifested as a Zn²⁺-induced acceleration of the proton association rate (k₇, see Table 2). The Zn²⁺-induced decrease in channel open time reflects the accelerated closing rate for protonated receptors (compare k₄ to kH₄ in Scheme IV, Table 2).

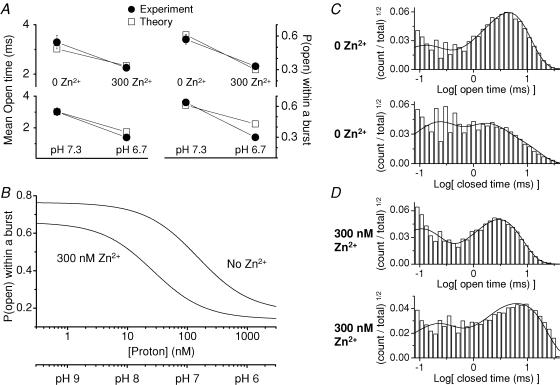
Schemes III and IV reproduced the expected Zn²⁺-induced reduction in open time, reduction in open probability, increase in occupancy of protonated states, and enhancement of proton sensitivity. Schemes III and IV additionally reproduced the open and closed time histograms (not shown). However, neither of these models provided realistic IC₅₀ values for proton inhibition in the absence of Zn²⁺. Simulated IC₅₀ values (pH 6.4 and 6.6 for Schemes III and IV, respectively) were considerably less than the experimental value of pH ≈ 7.0 (Low et al. 2000). One possible explanation for this was the lack of constraint on proton binding rates, which were allowed to vary during fitting. We therefore examined a new model (Scheme V) in which we fixed the proton association rate to a value measured for NR1/NR2B receptors (1.4 × 10⁹m⁻¹ s⁻¹; Banke et al. 2005), and allowed all other rate constants to vary during the fitting (Fig. 8C; Table 3). As observed for Schemes I and II, the magnitude of Zn²⁺-induced changes in rate constants were very similar between mean rate constants determined from fits to data from individual patches and rate constants determined from the global fits to the pooled data (Supplemental Table 1). Scheme V provided an excellent fit to the data (Fig. 9A–D), accurately represented single-channel dwell time distributions, and reproduced the key changes produced by Zn²⁺ on proton sensitivity.

Table 3.

Idealized and segmented current records from the same patches were fitted by Scheme V as shown in Fig. 8C

	Scheme V
	Control	300 nm Zn2+	Zn2+/Control
k1 (s−1)	1100	900	0.82
k2 (s−1)	2700	3200	1.19
k3 (s−1)	3800	4200	1.11
k4 (s−1)	1900	2600	1.37
k5 (s−1)	6300	6500	1.03
k6 (s−1)	1200	1200	1.00
k7 (m−1 s−1)	1.4e+9	1.4e+9	—
k8 (s−1)	47	12	0.26
k9 (s−1)	630	150	0.24
kH1 (s−1)	300	610	2.03
kH2 (s−1)	750	2200	2.93
kH3 (s−1)	900	820	0.91
kH4 (s−1)	5800	6700	1.16
kH5 (s−1)	5500	5000	0.91
kH6 (s−1)	1100	920	0.84
logL/event	5.13	5.02	—

Records from all 7 patches were pooled and fitted simultaneously. The proton concentration was 62 nm (pH 7.3). All loops were constrained to obey microscopic reversibility; proton association rate was fixed to 1.4 × 10⁹m⁻¹ s⁻¹ (Banke et al. 2005); all other rate constants were free parameters during fitting. The ratio of rate constants determined in 300 nm Zn²⁺ to those determined for control conditions (10 mm tricine, no added Zn²⁺) is given in the far right column. Rates in bold show more than a 3-fold change in the presence of Zn²⁺. Rate constants are given to two significant digits.

Figure 9. Zn²⁺ enhancement of proton sensitivity.

A, the rate constants determined by fitting Scheme V to single-channel data (Table 3) can reproduce the main features of proton and Zn²⁺ inhibition. The model predictions (‘Theory’) for mean open time and open probability are plotted compared to the experimental values for each condition. B, the model reproduced the effects of Zn²⁺ binding on the proton IC₅₀ for the open probability, assessed by fitting the simulated concentration–response data with the equation

where IC₅₀ is the concentration that produces a half-maximal response and N is the Hill slope. The fitted proton IC₅₀ for this model in the absence of Zn²⁺ was 140 nm (pH 6.9); open probability ranged between 0.19 and 0.76 and the Hill slope was 1.0. The fitted proton IC₅₀ for this model in 300 nm Zn²⁺ was 25 nm (pH 7.7); open probability within a burst ranged between 0.14 and 0.66 and the Hill slope was 1.0. C and D, the fitted rate constants can reproduce the open and closed duration single-channel histograms observed in control conditions (0 Zn²⁺, C) and in 300 nm Zn²⁺ (D). The superimposed probability density functions are calculated from the global fit of Scheme V across all patches for either the 0 nm Zn²⁺or 300 nm Zn²⁺ data set.

These Zn²⁺-induced changes in fitted rate constants reproduced the key functional features of Zn²⁺ binding to NR2A. As the proton association rate was held constant, the Zn²⁺-induced increase in proton sensitivity was manifested as a slowing of the proton dissociation rate, which led to an increased fraction of protonated receptors. Scheme V reproduced the Zn²⁺-induced changes in open time and open probability within a burst accurately, and predicted the observed changes in mean open time and open probability within a burst in patches at low pH (Fig. 9A). The Zn²⁺-induced decrease in open time reflected the faster closing rate of protonated receptors than unprotonated receptors (compare k₄ to kH₄ in Table 3). The lower open probability of protonated receptors probably involved slower forward rates for gating steps (compare k₃ to kH₃ in Table 3) in combination with faster channel closing rates. In addition, this model predicted realistic values for the proton sensitivity of the open probability (Fig. 9B). Consistent with previously reported effects of Zn²⁺ on proton IC₅₀, simulations with fitted rate constants suggest that Zn²⁺ induced a higher apparent affinity for proton inhibition. The predicted proton IC₅₀ was 140 nm (pH 6.9) in the absence of Zn²⁺ and 25 nm (pH 7.7) in the presence of 300 nm Zn²⁺. The model predicted that the K_d for proton binding to the closed states is shifted from 35 nm to 10 nm by Zn²⁺; the K_d for proton binding to the open states is shifted from 481 nm to 115 nm by Zn²⁺. The primary shortcoming of this model is the incomplete inhibition predicted by pH (Fig. 9B), which is inconsistent with previously reported data (Tang et al. 1990; Traynelis & Cull-Candy, 1990; Low et al. 2000). This may reflect engagement of other proton-sensitive residues at extremely low pH, or perhaps inaccurate representation of the open probability of protonated receptors due to oversimplification of the gating scheme. One additional caveat with this model is that it does not predict strong correlations between open and shut durations (data not shown), in contrast to observed data. This probably reflects oversimplification of the gating scheme in favour of inclusion of explicit protonation steps.

Discussion

There are two main findings of this study. First, the inhibition of single-channel currents recorded from recombinant NR1/NR2A NMDA receptors by submicromolar concentrations of extracellular Zn²⁺ and protons appears similar. Second, high affinity Zn²⁺ inhibition of NR2A-containing NMDA receptors is due to a decrease in the mean channel open duration and a decrease in the open probability within a burst of channel activity with no effect on the unitary open channel current amplitude. Kinetic modelling suggests that these effects of Zn²⁺ primarily reflect an enhancement of proton sensitivity. Moreover, the decrease in mean open time may involve an accelerated closing rate for protonated receptors. These findings provide further support for the hypothesis that Zn²⁺ inhibition of NR1/NR2A receptors reflects enhancement of tonic proton inhibition (Choi & Lipton, 1999; Low et al. 2000; Zheng et al. 2001; Erreger & Traynelis, 2005).

Mechanism of Zn²⁺-induced inhibition of NR1/NR2A receptors

There are two hypotheses that could account for the similarities in the effects of Zn²⁺ and protons on NR1/NR2A single-channel properties. The first is that Zn²⁺ and protons share a common downstream target, the channel gating machinery, and thus modify each others actions through an allosteric mechanism. The second hypothesis is that Zn²⁺ binding brings about an enhancement in the sensitivity to protons, resulting in an increase in inhibition of Zn²⁺-bound NMDA receptors by physiological concentrations of protons. Multiple lines of evidence in the literature favour the second hypothesis, that zinc binding leads to enhanced sensitivity to protons. First, the presence of 1 μm zinc shifts the IC₅₀ for proton inhibition from pH 7.0 to pH 7.3–7.6 (Choi & Lipton, 1999; Low et al. 2000). Second, inhibition by zinc is incomplete even at saturating concentrations and the degree of maximal inhibition is pH dependent, and can be accounted for by a model assuming that Zn²⁺ acts by shifting the pK_a for the proton sensor (Low et al. 2000; Zheng et al. 2001; Erreger & Traynelis, 2005). Third, the binding of phenolethanolamines to the amino terminal domain of NR2B has been shown to enhance proton sensitivity in much the same way as Zn²⁺ binding to the amino terminal domain of NR2A is hypothesized to enhance proton inhibition (Mott et al. 1998; Paoletti et al. 2000; Perin-Dureau et al. 2002).

Proton sensitivity has previously been studied in detail at the single-channel level for NR1/NR2B receptors and native NMDA receptors. A number of parallels exist between our data and this previous work. For example, we find the models that best describe our data include a protonated arm of the receptor gating scheme (Banke et al. 2005). In addition, protons decrease open probability (Traynelis & Cull-Candy, 1991; Banke et al. 2005), similar to the effects of Zn²⁺. However, the pH dependence of the mean open channel duration for NR2A-containing receptors differs from NR2B-containing receptors, which show only modest effects of protons on open times (Banke et al. 2005). Given the minimal effect of protons on NR1/NR2B open times, previous kinetic modelling did not need to incorporate mechanisms that would accelerate channel closure in low pH solutions to reproduce single-channel data. However, the more prominent effects of protons on open time for NR1/NR2A forced consideration of new potential mechanisms. The addition of protonation paths for open channels seems conceptually reasonable, and suggests that the proton sensor remains accessible and protonatable in open channels for NR1/NR2A. Indeed, the lack of protonation of any states in NR1/NR2B models previously examined might simply reflect a lower proton sensitivity of these states, so low that these steps could be omitted without compromising our ability to describe the data. Thus, we favour a unifying theory between proton sensitivity of NR1/NR2A and NR1/NR2B receptors, with minimal contribution of protonation pathways from open states of NR1/NR2B to inhibition.

Previous studies have shown that alternate RNA splicing of the NR1 subunit can influence both proton and Zn²⁺ inhibition (Traynelis et al. 1995, 1998). Inclusion of the highly charged residues encoded by the alternatively splice exon5 reduces the sensitivity to both extracellular Zn²⁺ and protons. We expect the results obtained here to apply to receptors that lack or contain residues encoded by alternative exon5. We predict that although proton binding and unbinding rates will yield lower proton sensitivity in exon5-containing receptors, that saturating concentrations of Zn²⁺ will still enhance the protonation of NR1/NR2A receptors that contain NR1 exon5.

Comparison to studies of Zn²⁺ inhibition of native NMDA receptors

The functional effects of Zn²⁺ on neuronal NMDA channels of unknown subunit composition have previously been investigated in patches from cultured cortical and hippocampal neurons and at relatively high zinc concentrations (> 1 μm) that may induce voltage-dependent channel block in addition to the high affinity voltage-independent inhibition (Christine & Choi, 1990; Legendre & Westbrook, 1990). An additional complication of these early studies is that the high affinity zinc inhibition of NR2A-containing receptors may have been obscured in these experiments by ambient levels of zinc in standard laboratory salt solutions (∼300 nm) (Li et al. 1996; Zheng et al. 1998) used as controls in the absence of a divalent buffer such as EDTA or tricine. Nonetheless, our results are in part consistent with these studies, which reported that Zn²⁺ causes a decrease in mean channel open time and a decrease in opening frequency.

Summary

In summary, this study demonstrates that high affinity Zn²⁺ inhibition and proton inhibition of NR1/NR2A NMDA receptors share a common functional signature at the single-channel level. Both protons and extracellular Zn²⁺ decrease the mean channel open duration and the channel open probability without altering the single-channel conductance. In addition, we present strong evidence for a mechanism of Zn²⁺-induced inhibition of NR1/NR2A receptors that involves Zn²⁺ enhancement of tonic proton inhibition.