Skip to main content
PMC home page
Proceedings of the Royal Society B: Biological Sciences logoLink to Proceedings of the Royal Society B: Biological Sciences
. 2008 Jun 10;275(1648):2241–2249. doi: 10.1098/rspb.2008.0512

Spatial variation in selection on corolla shape in a generalist plant is promoted by the preference patterns of its local pollinators

José M Gómez 1,*, Jordi Bosch 2, Francisco Perfectti 3, JD Fernández 1, Mohamed Abdelaziz 3, JPM Camacho 3
PMCID: PMC2603243  PMID: 18544510

Summary

An adaptive role of corolla shape has been often asserted without an empirical demonstration of how natural selection acts on this trait. In generalist plants, in which flowers are visited by diverse pollinator fauna that commonly vary spatially, detecting pollinator-mediated selection on corolla shape is even more difficult. In this study, we explore the mechanisms promoting selection on corolla shape in the generalist crucifer Erysimum mediohispanicum Polatschek (Brassicaceae). We found that the main pollinators of E. mediohispanicum (large bees, small bees and bee flies) discriminate between different corolla shapes when offered artificial flowers without reward. Importantly, different pollinators prefer different shapes: bees prefer flowers with narrow petals, whereas bee flies prefer flowers with rounded overlapping petals. We also found that flowers with narrow petals (those preferred by bees) produce both more pollen and nectar than those with rounded petals. Finally, different plant populations were visited by different faunas. As a result, we found spatial variation in the selection acting on corolla shape. Selection favoured flowers with narrow petals in the populations where large or small bees are the most abundant pollinator groups. Our study suggests that pollinators, by preferring flowers with high reward, exert strong selection on the E. mediohispanicum corolla shape. The geographical variation in the pollinator-mediated selection on E. mediohispanicum corolla shape suggests that phenotypic evolution and diversification can occur in this complex floral trait even without specialization.

Keywords: corolla shape evolution, pollinator preference, spatial variation, geometric morphometrics, nectar, pollen

1. Introduction

Angiosperms display an astonishing variety of flower shapes. Understanding the evolution of this morphological diversity has been a focus of evolutionary biology for many years (Coen et al. 1995; Donoghue et al. 1998; Endress 1999; Ree & Donoghue 1999; Kalisz et al. 2006), producing copious information gathered on macroevolution and developmental genetics of floral shape (Luo et al. 1995; Reeves & Olmstead 1998; Cubas et al. 1999; Ree & Donoghue 1999; Dilcher 2000; Cronk et al. 2005). By contrast, the selective mechanisms driving corolla shape evolution are far less understood (Herrera 1993; Campbell et al. 1996; Schemske & Bradshaw 1999; Galen & Cuba 2001). Consequently, despite many years of research, the adaptive significance of corolla shape remains largely elusive (Lloyd & Barrett 1996; Harder & Barrett 2006). According to the current models of floral evolution, corolla shape evolves in response to strong selection exerted by pollinators through an increase in flower attractiveness, which affects pollination quantity, and/or through enhanced pollen-transfer efficiency, which affects pollination quality (Möller 1995; Möller & Sorci 1998; Neal et al. 1998; Endress 1999, 2001).

Generalist plants are visited by diverse pollinators that differ in preference patterns, visitation rate and per-visit effectiveness (Johnson & Steiner 2000; Gómez & Zamora 2006). Although both quantity and quality components are essential, abundant empirical evidence suggests that the importance of a given pollinator species for the fitness of generalist plants depends strongly on its visitation rates rather than its per-visit efficiency (Galen & Newport 1987; Olsen 1997; Gómez & Zamora 1999; Vazquez et al. 2005). Pollinator visitation rate is influenced in many plants by reward quantity and quality (Smithson & Macnair 1997; Cunningham et al. 1998; Scheiner et al. 1999; Waddington 2001; Blarer et al. 2002; Plepys et al. 2002; Schaefer et al. 2004; Biernaskie & Gegear 2007; Internicola et al. 2007). Under these circumstances, pollinators may indirectly promote the selection on corolla shape if this trait covaries with reward (Stanton & Preston 1988; Young & Stanton 1990; Ashman & Stanton 1991; Campbell et al. 1991; Cohen & Shmida 1993; Stanton & Young 1994; Blarer et al. 2002; Armbruster et al. 2005; Fenster et al. 2006).

For most species with generalized pollination, the pollinator assemblage often varies geographically as a consequence of changes in abundance and identity of the flower visitors (Moeller 2005, 2006 and references therein). When pollinators differ in morphology, foraging behaviour and flower trait preferences, spatial variation in the identity of the frequent pollinators may result in concomitant spatial variation in the selection affecting floral traits (Gómez & Zamora 2000; Herrera et al. 2006).

In this study we explore the mechanisms driving corolla shape evolution as a response to pollinator selection in Erysimum mediohispanicum Polatschek (Brassicaceae). This species is a good system to study pollinator-mediated corolla shape evolution for several reasons. First, corolla shape in E. mediohispanicum is heritable (J. M. Gómez, M. Abdelaziz, J. Muñoz & F. Perfectti 2005–2007, unpublished data). Second, phenotypic selection on corolla shape has already been established (Gómez et al. 2006). Third, the species exhibits extensive quantitative variation in corolla shape in the wild (see fig. 1 in Gómez et al. 2006). Fourth, although self-compatible, E. mediohispanicum needs pollen vectors for full seed set (Gómez 2005). Fifth, E. mediohispanicum is highly generalist, being visited by more than 130 species of insects (Gómez et al. 2008). Finally, pollinator fauna, and potential selective pressures, vary significantly among localities (Gómez et al. 2006, 2008).

Flower shape has usually been studied as a qualitative trait (e.g. radial versus bilateral symmetry) or as a variable composed of several linear measurements (e.g. Neal et al. 1998; Endress 1999, 2001; Ree & Donoghue 1999; Galen & Cuba 2001). This approach generates an oversimplified description of this complex trait. To estimate corolla shape variation accurately in E. mediohispanicum, we use geometric morphometrics that treat corolla shape as a single multidimensional trait (Zelditch et al. 2004). This approach allows the application of standard quantitative genetics and evolutionary ecology tools in the study of corolla shape microevolution. Our specific objectives are to (i) estimate phenotypic selection for corolla shape, (ii) test experimentally whether different E. mediohispanicum pollinators favour different corolla shapes, (iii) ascertain whether corolla shape covaries with floral reward, (iv) explore whether variation in local pollinator fauna leads to spatial variation in selection on corolla shape and (v) assess a link between pollinator preference patterns and phenotypic selection on floral shape.

2. Material and methods

(a) Study system

Erysimum mediohispanicum is a biennial to perennial monocarpic herb, found in forests and subalpine scrublands of southeast Spain from 1000 to 2300 m elevation. Plants usually grow for 2–3 years as vegetative rosettes, and then die after producing one to eight reproductive stalks that can display a few to several hundred hermaphroditic, slightly protandrous bright yellow flowers (Gómez 2003). The most important pollinator groups of E. mediohispanicum are large bees, small bees, bee flies and beetles (Gómez et al. 2008), including species that collect pollen, nectar and both (Gómez 2003, 2005).

The study was carried out during 2005 and 2006 in three E. mediohispanicum populations located in the Sierra Nevada high mountains (Granada province, southeast Spain; table 1).

Table 1.

Location and characteristics of the E. mediohispanicum populations studied in the Sierra Nevada (southeast Spain).

population population characteristics pollinator sampling effort
latitude north longitude west altitude habitat plants minutes flowers pollinators
Em02 37°7.33′ 3°25.86′ 2099 shrubland 90 1720 2486 270
Em21 37°8.07′ 3°25.71′ 1723 forest 90 1955 1826 243
Em25 37°7.27′ 3°26.05′ 2064 shrubland 90 2195 1710 118
Open in a new tab

(b) Pollinator assemblage

Pollinator assemblage was determined in each of the three populations using a standardized methodology (Gómez et al. 2008). Throughout peak bloom (10–15 days per population), we conducted five to seven pollinator samples per population, during which the number of open flowers on each labelled plant and the number of pollinators that landed on their flowers during 5 min were noted. Thus, each survey lasted 450 min and we conducted more than 3000 min of observation per population. The number of surveys per population was fitted to the local abundance of pollinators by means of accumulation curves (Gómez et al. 2008).

We grouped the insects visiting E. mediohispanicum flowers into functional groups. We define ‘functional group’ as flower visitors that interact with flowers similarly (Fenster et al. 2004). Basically, we used criteria of similarity in size, proboscis length, foraging behaviour and feeding habits. Thus, taxonomically related species were sometimes placed in different functional groups. We established eight functional groups: (i) large bees, mostly pollen- and nectar-collecting females measuring 10 mm in body length or larger; (ii) small bees, mostly pollen- and nectar-collecting females smaller than 10 mm; (iii) wasps, including aculeate wasps, large parasitic wasps and cleptoparasitic bees collecting only nectar; (iv) bee flies, long-tongued nectar-collecting Bombyliidae; (v) hoverflies, nectar- and pollen-collecting Syrphidae and short-tongued Bombyliidae; (vi) beetles, including species collecting nectar and/or pollen; (vii) butterflies, mostly Rhopalocera, all nectar collectors; and (viii) others, including nectar-collecting ants, small flies, small parasitic wasps, bugs and other occasional flower visitors.

(c) Estimation of phenotypic selection on corolla shape

In 2005, we analysed selection occurring on E. mediohispanicum corolla shape in each population. We marked 90 plants per population at the onset of the flowering period, using aluminium tags attached to the base of the flowering stalks. Corolla shape and lifetime female fitness were estimated for each labelled plant.

Corolla shape was quantified by means of geometric morphometrics (Gómez et al. 2006). Because the four petals of E. mediohispanicum flowers are presented in a single plane (Gómez et al. 2006), we used a two-dimensional analysis to describe their shape (Zelditch et al. 2004). We took a digital photograph of one flower per labelled plant using a standardized procedure (front view and planar position). Flowers were photographed at anthesis to avoid ontogenetic effects on corolla shape. In addition, the flowers were located in the same position along the flowering stalk (central) to avoid any position effect on floral traits. For each flower, we defined 32 coplanar landmarks along the outline of the petals and the aperture of the corolla tube. The number of landmarks was chosen to provide comprehensive coverage of corolla shape (Zelditch et al. 2004; see Gómez et al. 2006 for further details). The two-dimensional coordinates of these landmarks were determined for each flower using the software tpsDig v. 1.4 (http://life.bio.sunysb.edu/morph/morphmet.html). Then, the generalized orthogonal least-squares Procrustes average configuration of landmarks was computed using the Generalized Procrustes Analysis (GPA) superimposition method, using the software tpsRelw v. 1.11 (http://life.bio.sunysb.edu/morph/morphmet.html). Following GPA, the relative warps (RWs, principal components of the covariance matrix of the partial warp scores) were computed (Walker 2000; Adams et al. 2004). Each RW is characterized by a singular value, and explains a given variation in shape among specimens. Thus, RWs summarize shape differences among specimens (Adams et al. 2004), and their scores can be used as a data matrix to perform standard statistical analyses (Zelditch et al. 2004).

Lifetime female fitness was estimated as the number of seeds produced per plant (Gómez et al. 2006). At the end of the reproductive season, we counted the number of fruits per labelled plant (90 per population, 270 plants in total) and collected between 50 and 75% of the fruits to determine the number of seeds produced per fruit in the laboratory.

Following Klingenberg & Leamy (2001) and Klingenberg & Monteiro (2005), selection for shape can be calculated using (multivariate) selection differentials (s) and gradients (β). The former is a descriptor of the total effect of selection on shape without distinguishing between direct and indirect selection. The latter provides information on how selection directly acts on each shape variable accounting statistically for the other measured variables (Klingenberg & Monteiro 2005). The selection differential was quantified as the vector of covariances between the fitness and the shape variables (the complete set of RWs in this study). We derived the vector of coefficients from a two-block partial least-squares (PLS) analysis between shape and fitness (Rohlf & Corti 2000). By means of cross-validation, we found the number of latent vectors displayed by the model with the lowest r.m.s.e. (root mean squared error) (Abdi 2007). We then determined the covariance between fitness and shape predicted by this parsimonious model. Selection gradients β for each population were computed from the standardized partial regression coefficients of a linear regression of relative fitness on all 60 RWs generated by the geometric morphometrics analyses (Lande & Arnold 1983; Klingenberg & Leamy 2001). Because we considered corolla shape as a single multidimensional trait, we included the complete set of RWs in these analyses (Adams et al. 2004). However, to improve the interpretation of the outcomes, we reduced the dimensionality of the covariance matrix (Klingenberg & Leamy 2001). For this reason, we evaluated only the first four RWs, a subset that explained most (more than 70%) of the variability in E. mediohispanicum corolla shape and that already has been shown to be subject to important selective effects (Gómez et al. 2006). In this model, we also included flower size and number as well as plant size to control for indirect selection on flower shape (Gómez et al. 2006). Flower size was estimated by means of two variables, the corolla diameter (the distance in mm between the edges of two opposite petals, using a digital calipers with ±0.1 mm error) and the corolla tube length (the distance between the corolla tube aperture and the base of the sepals). Plant size was estimated by means of three variables, the number of stalks growing from each rosette, the height of the tallest reproductive stalk (measured to the nearest 0.5 cm, the distance from the ground to the top of the highest opened flower) and the basal diameter of the tallest stalk (mm).

Selection gradients should not be visualized as shape changes (Adams & Rosenberg 1998; Klingenberg & Monteiro 2005). To visualize the expected shapes of individuals with different fitness, we followed Klingenberg & Monteiro (2005) and computed a predicted fitness score for each individual by multiplying its shape variables by the selection gradient. The regression of shape on this fitness score yields a vector of regression coefficients that can be visualized directly as changes in landmark positions (Rohlf et al. 1996).

(d) Experimental determination of pollinator preference for corolla shape

In 2005, we experimentally assessed flower shape preferences of E. mediohispanicum's pollinators in the three populations by means of choice experiments. We built artificial flowers using yellow construction paper to match (from a human perspective) the colour of E. mediohispanicum flowers. We built artificial flowers of nine different shapes. Eight of these shapes corresponded to the two extremes (positive and negative) of the four RWs that define E. mediohispanicum's corolla shape according to previous geometric morphometrics analyses (Gómez et al. 2006; see also figure 1). The ninth flower shape corresponded to the consensus shape obtained in the same analyses (Gómez et al. 2006). Artificial flowers were of the same size as natural flowers and were individually arranged in 20 cm tall wire stalks. The different shape models did not differ significantly in size, as measured by corolla diameter (F15,88=1.21, p=0.278, one-way ANOVA). In addition, to avoid any side effect of reward on pollinator behaviour, we did not add any reward to the artificial flowers.

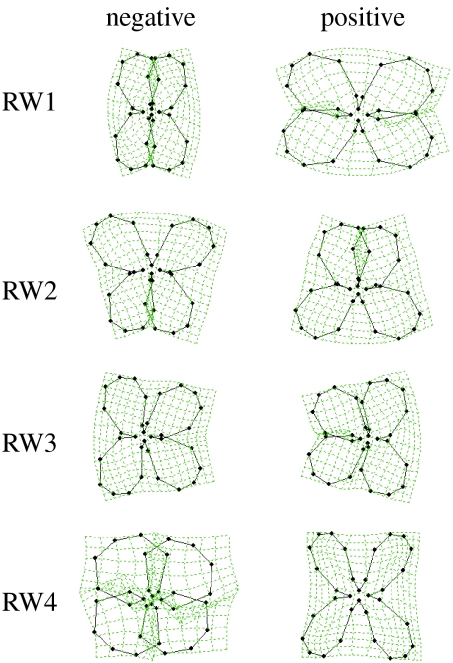
Figure 1.

Figure 1

Open in a new tab

Corolla shape variation in Erysimum mediohispanicum. Summary of the geometric morphometric analysis (2005 and 2006 data, N=420 plants) showing the variation in corolla morphology produced by RWs explaining more than 5% of the overall variation in shape (RW1: 35.2%, RW2: 19.2%, RW3: 9.7%, RW4: 6.2%). The corolla shapes corresponding to the two extreme values (negative and positive) of the distribution along each shape component (RW1–RW4) are shown.

In each population, we set up experimental arenas (80×120 cm) with 12 flowers of each of the eight extreme shapes and 48 flowers of the consensus shape. Thus, each experimental arena had 144 artificial flowers. The flowers were randomly distributed within the arenas and the experiment was conducted during peak flowering of E. mediohispanicum. During the experiments, two to three observers (located 1 m away from the arenas) noted all insects approaching the artificial flowers. We considered an approach to be successful if the insect landed on a flower or contacted it. Each pollinator was visually identified based on our 2-year experience surveying E. mediohispanicum pollinators in the area. Our analyses included only those pollinator species seen visiting natural E. mediohispanicum flowers in the three populations.

Differences among pollinator functional groups in floral choice were explored by a nominal logistic model using a likelihood ratio test. Departures from random visitation (no pollinator choice as null hypothesis) were tested for each pollinator functional group separately by chi-squared tests for goodness of fit, with expected frequencies based on the frequency of each flower morph in the experimental arenas.

(e) Determination of the relationship between corolla shape and flower reward

Pollen and nectar were quantified during 2006 for 50 plants per population. We measured pollen production, as the total volume of pollen produced per flower. One flower bud per plant was taken and preserved in 70% ethanol. Three of the six anthers of each flower (two long and one short, as E. mediohispanicum has a typical Brassicaceae tetradynamous androecium) were placed in a vial with ethanol and sonicated for 3 min to dislodge pollen grains (Kearns & Inouye 1993). A known volume of saline solution was then added to the vial and the number of pollen grains per volume was counted in a Multisizer particle counter. Pollen grain diameter was measured on pollen slides at 400×. From these two measures we obtained total pollen volume per flower. We measured nectar production as the volume produced during 24 hours by newly opened flowers. On each labelled plant we covered three to five flower buds with cellophane bags to prevent pollinator visitation. After 24 hours, we measured nectar volume in three flowers per plant using calibrated micropipettes (Kearns & Inouye 1993). Nectar volume was averaged per plant to avoid pseudoreplication. We also quantified corolla shape in these plants using the above methodology.

The relationship between flower shape and reward was analysed as the vector of covariances between reward production and flower shape variables (the complete set of RWs generated by the morphometric analyses). To accomplish this, we derived the latent vector of coefficients from a two-block PLS analysis between the complete set of 60 RWs and reward (Rohlf & Corti 2000). Each reward trait (pollen and nectar) was used separately in the reward block. By means of cross-validation, we found the number of latent vectors displayed by the model with the lowest r.m.s.e. (Abdi 2007). Then, we determined the covariance between reward and shape predicted by this parsimonious model. Prior to performing the PLS, we checked whether the corolla shape–reward relation was similar among populations by performing a general linear model (GLM) including corolla shape, population and the interaction between shape and population.

3. Results

(a) Differences between populations in pollinator fauna

The assemblage of insects visiting E. mediohispanicum flowers differed among the three populations (Χ162=108.63, p<0.0001, Monte Carlo contingency test with 100 000 iterations). The most abundant flower visitors in Em02 were beetles (42.2% of the visits), followed by large bees (18.1%), small bees (15.2%), hoverflies (8.8%) and bee flies (7.0%). In Em21, the most abundant floral visitors were small bees (44.4%), followed by bee flies (19.3%), beetles (16.1%) and large bees (5.6%). Finally, in Em25, the most abundant floral visitors were large bees (37.1%), followed by small bees (21.2%), beetles (28.8%) and bee flies (9.3%). The remaining functional groups were very scarce in all three studied populations (less than 5% of all visits).

(b) Phenotypic selection for flower shape

The geometric morphometric analysis generated 60 shape components (RWs, hereafter) of which the first four (RW1–RW4) explained more than 70% of the variation in corolla shape (figure 1, see appendix 1 in the electronic supplementary material for the complete set of RWs). In both years, RW1–RW4 were associated with the same pattern of corolla shape variation (figure 1). In addition, the outcome of the geometric morphometrics did not change when each population was analysed separately (data not shown).

The shape components did not correlate strongly with the phenotypic traits describing plant and flower size. RW4 was the only shape component correlating with any trait: it exhibited contrasting correlations with corolla diameter in two populations (Em02: r=0.371, n=50, p=0.0001; Em21: r=−0.317, n=50, p=0.002, product–moment correlation) and varied positively with corolla tube length in one population (Em02: r=0.294, n=50, p=0.005, significant after sequential Bonferroni correction; see appendix 2 in the electronic supplementary material for the complete correlation matrix). In addition, corolla shape did not vary significantly with the number of ovules produced per flower (p>0.43 for all first four RWs, linear regression).

Corolla shape experienced significant total selection in the three populations (selection differential: s=0.77, n=90, p<0.0001 (Em02); s=0.78, n=90, p<0.0001 (Em21); s=0.76, n=90, p<0.0001 (Em25); PLS analysis). However, different corolla shapes were selected in different populations (table 2). Thus, in populations Em21 and Em25, flowers with the highest female fitness had positive RW4 (Em21: β±1 s.e.=0.15±0.08, n=90, t=2.06, p=0.048; Em25: β±1 s.e.=0.28±0.11, n=90, t=2.64, p=0.01). In Em21, there was also a significant selection for flowers with positive RW1 (β±1 s.e.=0.16±0.08, n=90, t=2.06, p=0.048). Finally, although we found selection on the overall shape in population Em02, it did not occur through any of the first four RWs (table 2).

Table 2.

Standardized multivariate selection analysis on corolla shape for three E. mediohispanicum populations (n=90 plants per population). Results of only the first four RWs (RW1–RW4) are shown, although the analysis included all RWs and traits describing flower size, plant size and flower number. The corolla shapes associated with the highest female fitness in each population are also illustrated. The selected flower shapes were visualized as changes in landmark positions after computing predicted fitness scores for each individual by multiplying its shape variables by the selection gradient (Klingenberg & Monteiro 2005).

shape component β±s.e. t-ratio p-value selected flower shape
Em02
Std RW1 −0.132±0.185 2.32 0.479
Std RW2 0.059±0.119 0.50 0.622
Std RW3 −0.058±0.113 −0.51 0.609
Std RW4 0.029±0.109 0.26 0.793
Em21
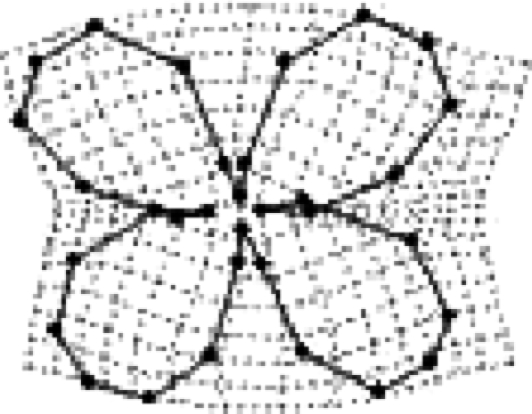Std RW1 0.159±0.077 −2.06 0.048 graphic file with name rspb20080512if01.jpg graphic file with name rspb20080512if02.jpg
Std RW2 −0.059±0.077 −0.77 0.447
Std RW3 −0.051±0.078 −0.65 0.515
Std RW4 0.158±0.077 2.06 0.048
Em25
Std RW1 −0.065±0.108 −0.60 0.555 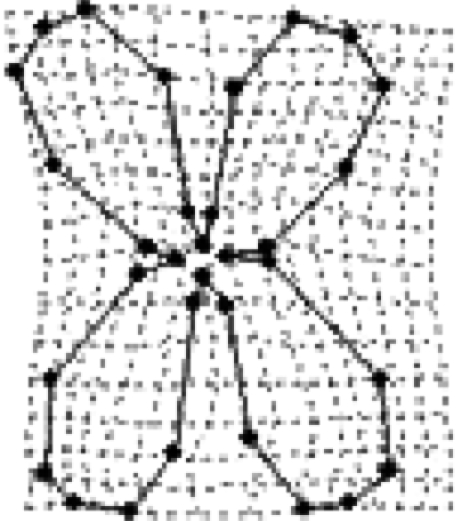graphic file with name rspb20080512if03.jpg
Std RW2 −0.111±0.101 −1.09 0.283
Std RW3 0.073±0.095 0.78 0.444
Std RW4 0.285±0.108 −2.64 0.013
Open in a new tab

With respect to other phenotypic traits, we found significant selection only for flower number in one population, Em21 (β±1 s.e.=0.64±0.17, t=3.80, p=0.0003; see appendix 3 in the electronic supplementary material for the overall selection models).

(c) Experimental determination of pollinator preference for corolla shape

A total of 1300 pollinators belonging to 66 species visited the artificial flowers. These insects belonged to the main pollinator functional groups of E. mediohispanicum: large bees (144 visits); small bees (156 visits); bee flies (405 visits); hoverflies (427 visits); and beetles (168 visits). We frequently observed pollinator flights between natural and artificial flowers.

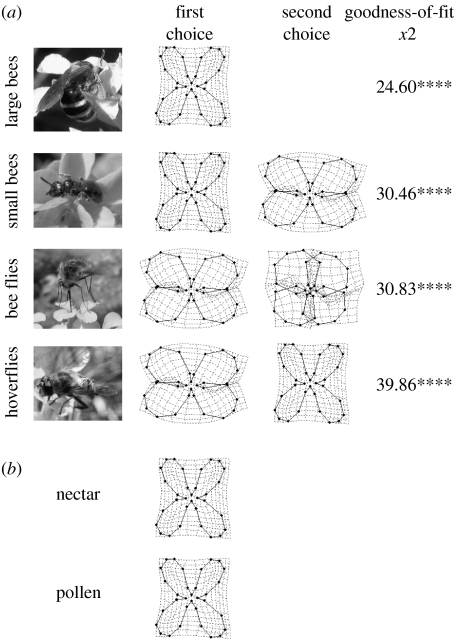
The major pollinators of E. mediohispanicum discriminated between flowers varying exclusively in corolla shape (figure 2). The results also show that different pollinator functional groups exhibited different preference patterns (likelihood ratio test=87.08, d.f.=48, p<0.0001, nominal logistic model). Beetles did not show any clear preference for any flower type (Χ82=5.80, n=168, n.s., goodness of fit), suggesting that these pollinators visited the artificial flowers at random. The remaining functional groups departed significantly from random choice (figure 2a). Large bees visited mostly flowers with positive RW4. Small bees preferentially visited flowers with positive RW4, but also flowers with positive RW1. Bee flies visited mostly flowers with positive RW1 and then flowers with negative RW4. Finally, hoverflies favoured flowers with positive RW1 and positive RW4.

Figure 2.

Figure 2

Open in a new tab

Corolla shapes associated with pollinator preference and floral rewards in Erysimum mediohispanicum. (a) Outcome of the pollinator choice experiment showing the flower shapes preferred by each E. mediohispanicum pollinator functional group. Goodness-of-fit tests assessed the departure from random choice based on the proportion of each corolla shape in the experimental arenas (see §2). ****p<0.0001. Beetles showed no significant preferences. (b) Shape of the flowers producing the most nectar and pollen (PLS analysis; see the electronic supplementary material for details).

(d) Relationship between corolla shape and reward

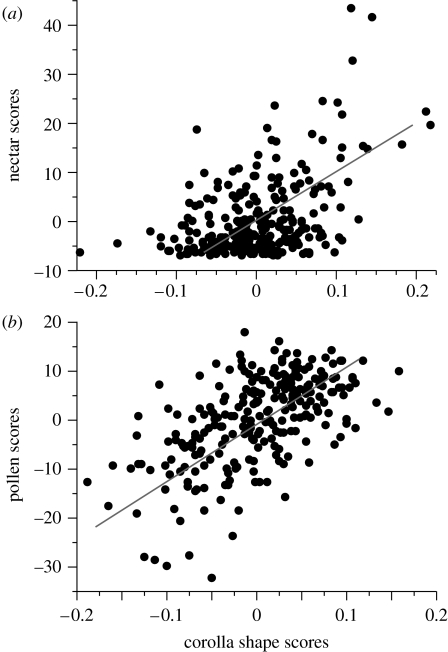
Corolla shape varied significantly with both pollen and nectar production (figure 3). The latent vector for nectar production was significant (r.m.s.e.=1.142, n=150, p<0.0001), explaining 30.1% of the variance. This vector was correlated with eight RWs, including positive correlation with RW4 (r=0.194, p<0.0001) and RW2 (r=0.412, p<0.0001; figure 2b; see appendix 1 in the electronic supplementary material for the complete set of correlation coefficients). The latent vector for pollen production was also significant (r.m.s.e.=0.953, n=150, p<0.0001), explaining 51.7% of the variance in pollen. Pollen production correlated with five RWs, again positively with RW4 (r=0.354, p<0.0001, figure 2b; see appendix 1 in the electronic supplementary material for the complete set of correlation coefficients). The GLM indicated similar relationships between corolla shape and reward among populations (no significant interaction).

Figure 3.

Figure 3

Open in a new tab

Relationship between flower reward and corolla shape. Outcomes of the PLS analysis showing the correlation between the scores of flower reward and corolla shape generated by the only significant latent vector. (a) Nectar scores (R2=0.30, p<0.0001), (b) pollen scores (R2=0.52, p<0.0001).

4. Discussion

Pollinators discriminated between different E. mediohispanicum corolla shapes and exhibited contrasting preference patterns. Whereas beetles visited indiscriminately, large and small bees preferred flowers with narrow petals and bee flies selected flowers with wide overlapping petals. These findings suggest that the shape of E. mediohispanicum corollas acts as a visual cue to flower visitors, as has been demonstrated for other species (Dafni & Kevan 1997; Neal et al. 1998; Giurfa & Lehrer 2001; Yoshioka et al. 2007). Several previous studies have established the ability of pollinators to discriminate among different models using artificial shapes (Gould 1985; Dafni & Kevan 1997; Johnson & Dafni 1998; Möller & Sorci 1998; Giurfa & Lehrer 2001), but few studies have been conducted under natural conditions and using models mimicking natural flowers (Yoshioka et al. 2007).

Our experiment could confound corolla shape and size because, as illustrated in figure 1, flowers with different values of RWs have different areas, even though they have equal corolla diameter. In particular, flowers with positive RW1 or negative RW4 had larger areas than flowers with negative RW1 or positive RW4. However, differences in areas of our artificial flowers probably influenced pollinator behaviour less than shape differences because, as observed in figure 2, the corolla shape preferred by most pollinators in the choice experiments had a small area (positive RW4), whereas studies of the effects of flower size on pollinator preference, including some previous studies with E. mediohispanicum, have shown that bees choose large flowers (Gómez 2003; Gómez et al. 2006 and references therein). Because flower shape varied largely independently of flower size and ovule production, influences of shape on pollinator behaviour and seed production probably reflect direct effects, rather than correlated responses to flower size.

It is important to uncover the factors underlying the observed pollinator preference patterns, since they will provide the mechanisms promoting corolla shape evolution. Pollinator–flower shape associations often result from a functional link between floral traits and reward production (Ashman & Stanton 1991; Campbell et al. 1991; Cohen & Shmida 1993; Möller 1995; Blarer et al. 2002; Armbruster et al. 2005; Fenster et al. 2006). We found a link between corolla shape and both pollen and nectar production in E. mediohispanicum, and the most rewarding flowers matched the corolla shape of artificial flowers preferentially visited by large and small bees. These results suggest that bees can use corolla shape as a signal for reward production in E. mediohispanicum. Since we used non-rewarding artificial flowers visited by wild experienced pollinators, these presumably learned the association between shape and reward on natural flowers (Smithson & Macnair 1997; Neal et al. 1998; Boisvert et al. 2007; Makino & Sakai 2007).

The preference of bee flies for rounded flowers cannot be explained by a functional link between corolla shape and reward. We propose that bee flies prefer rounded flowers because the corolla serves as a landing platform (Neal et al. 1998). Bee flies always fly between consecutively visited flowers, even within the same plant. Sometimes bee flies hover while collecting nectar, but other times they land on the flowers and collect nectar while standing on their second and third pairs of legs (J. M. Gómez, J. Bosch & F. Perfectti 2005–2007, unpublished data). Because hovering is energetically costly (Heinrich 1993), bee flies may choose to feed while standing on flowers that offer an appropriate landing platform (flowers with large rounded petals). Interestingly, large bee-like hoverflies visiting E. mediohispanicum behave similarly to bees, frequently walking from flower to flower, while smaller wasp-like hoverflies behave like bee flies.

Corolla shape experienced phenotypic selection in all studied populations, with two specific shapes, those associated with RW1 and RW4, selected in two populations. Previous studies of the same species in other locations also found significant selection on flower shape (Gómez et al. 2006). Our findings demonstrate that selection on corolla shape matched the preference patterns displayed by the most abundant local pollinators. Plants with the highest female fitness in population Em21 had flowers with positive RW4 and RW1, coinciding with the preference patterns of small bees, the most abundant pollinator group in this population, and of bee flies, the second most abundant groups. In population Em25, selection favoured flowers with positive RW4, in agreement with the preference pattern of large bees, the most abundant pollinator group in this population. Finally, no corolla shape was selected in population Em02, where the most abundant pollinators were beetles, the only functional group not showing preference for any corolla shape. In addition, the observed match between pollinator preference, corolla shape and reward production suggests that the evolution of corolla shape in this species with a generalized pollination system is mediated by the link between corolla shape and rewards. Corolla shape is heritable in E. mediohispanicum, due mostly to the significant heritability of two of the main shape components, RW1 and RW4 (J. M. Gómez, M. Abdeloziz, J. Muñoz & F. Perfectti 2005–2007, unpublished data), that are also associated with pollinator preferences and high fitness. Taken together, our results strongly indicate that corolla shape of E. mediohispanicum is evolving through pollinator-mediated natural selection.

The observed between-pollinator differences in preference patterns for corolla shape may have contrasting consequences for corolla shape evolution, depending on the pattern of spatial variation in pollinators. When plants in a single population are visited by many disparate pollinator species belonging to different functional groups, as occurs in some E. mediohispanicum populations (Gómez et al. 2008), between-pollinator differences in preference may lead to pollinator-mediated trade-offs. For example, any modification in corolla shape resulting in increased attraction to bees may be accompanied by a decrease in bee fly attraction, and vice versa (see also Wilson & Thomson 1996; Castellanos et al. 2004; Aigner 2006; Muchhala 2007). As a consequence, within-population variation in pollinator composition may cause disruptive selection maintaining intrapopulational phenotypic variability in corolla shape (Dilley et al. 2000; Gómez & Zamora 2006). By contrast, when the pollinator assemblage varies mostly among, rather than within populations, spatial variation in pollinators can promote variation in local selection across populations (Gómez & Zamora 2006; Herrera et al. 2006; Sargent & Otto 2006). Among-population differences in pollinator-mediated selection have been shown for some specialized systems, where an overall turnover of the main pollinator groups occurs at a geographical scale (Herrera et al. 2006). Our findings demonstrate that these differences also occur in generalized plants as a result of spatial changes in the relative abundance of different pollinators. In these kind of systems, complex floral traits, such as corolla shape, can also evolve and diversify without pollination specialization.

Acknowledgments

We are very much indebted to Dr Paul Wilson and two anonymous reviewers for improving a preliminary version of this manuscript. The Ministerio de Medio Ambiente and Consejería de Medio Ambiente of the Junta de Andalucía granted permission to work in the Sierra Nevada National Park. This study was partially supported by the Spanish MCyT (GLB2006-04883/BOS) and Junta de Andalucía PAI (RNM 220 and CVI 165).

Supplementary Material

Outcome of the geometric morphometric analysis of corolla shape

Geometric morphometric analysis of Erysimum mediohispanicum corolla shape and correlations between relative warps and PLS latent vectors for pollen and nectar contents.

rspb20080512s12.doc (122.5KB, doc)
Correlations among each shape component and the phenotypic traits

Correlation among each Erysimum mediohispanicum shape component and the phenotypic traits describing plant and flower size.

rspb20080512s13.doc (53.5KB, doc)
Standardized multivariate selection analysis on corolla shape and size

Standardized multivariate selection analysis on corolla shape and size for each E. mediohispanicum population

rspb20080512s14.doc (52.5KB, doc)

References

  1. Abdi H. Partial least square regression. In: Salkind N, editor. Encyclopedia of measurements and statistics. Sage; Thousand Oaks, CA: 2007. pp. 1–13. [Google Scholar]
  2. Adams D.C, Rosenberg M.S. Partial warps, phylogeny, and ontogeny: a comment on Fink and Zelditch (1995) Syst. Biol. 1998;47:168–173. doi: 10.1080/106351598261111. doi:10.1080/106351598261111 [DOI] [PubMed] [Google Scholar]
  3. Adams D.C, Rohlf F.J, Slice D.E. Geometric morphometrics: ten years of progress following the ‘revolution’. Ital. J. Zool. 2004;71:5–16. [Google Scholar]
  4. Aigner P.A. The evolution of specialized floral phenotypes in a fine-grained environment. In: Waser N.M, Ollerton J, editors. Plant–pollinator interactions, from specialization to generalization. University of Chicago Press; Chicago, IL: 2006. pp. 23–46. [Google Scholar]
  5. Armbruster W.S, Antonsen L, Pelabon C. Phenotypic selection on Delachampia blossoms: honest signaling affects pollination success. Ecology. 2005;86:3323–3333. doi:10.1890/04-1873 [Google Scholar]
  6. Ashman T.L, Stanton M. Seasonal variation in pollination dynamics of sexually dimorphic Sidalcea oregana spp. spicata (Malvaceae) Ecology. 1991;72:993–1003. doi:10.2307/1940599 [Google Scholar]
  7. Biernaskie J.M, Gegear R.J. Habitat assessment ability of bumble-bees implies frequency-dependent selection on floral rewards and display size. Proc. R. Soc. B. 2007;274:2595–2601. doi: 10.1098/rspb.2007.0705. doi:10.1098/rspb.2007.0705 [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Blarer A, Keasar T, Shmida A. Possible mechanisms for the formation of flower size preferences by foraging bumblebees. Ethology. 2002;108:341–351. doi:10.1046/j.1439-0310.2002.00778.x [Google Scholar]
  9. Boisvert M.J, Veal A.J, Sherry D.F. Floral reward production is timed by an insect pollinator. Proc. R. Soc. B. 2007;274:1831–1837. doi: 10.1098/rspb.2007.0445. doi:10.1098/rspb.2007.0445 [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Campbell D.R, Waser N.M, Price M.V, Lynch E.A, Mitchell R.J. Components of phenotypic selection: pollen export and flower corolla width in Ipomopsis aggregata. Evolution. 1991;45:1458–1467. doi: 10.1111/j.1558-5646.1991.tb02648.x. doi:10.2307/2409892 [DOI] [PubMed] [Google Scholar]
  11. Campbell D.R, Waser N.M, Price M.V. Mechanisms of hummingbird-mediated selection for flower width in Ipomopsis aggregata. Ecology. 1996;77:1463–1472. doi:10.2307/2265543 [Google Scholar]
  12. Castellanos M.C, Wilson P, Thomson J.D. “Anti-bee” and “pro-bird” changes during the evolution of hummingbird pollination in Penstemon flowers. J. Evol. Biol. 2004;17:876–885. doi: 10.1111/j.1420-9101.2004.00729.x. doi:10.1111/j.1420-9101.2004.00729.x [DOI] [PubMed] [Google Scholar]
  13. Coen E.S, Nugent J.M, Luo D, Bradley D, Cubas P, Chadwick M, Copsey L, Carpenter R. Evolution of floral symmetry. Phil. Trans. R. Soc. B. 1995;350:35–38. doi:10.1098/rstb.1995.0134 [Google Scholar]
  14. Cohen D, Shmida A. The evolution of flower display and reward. Evol. Biol. 1993;27:197–243. [Google Scholar]
  15. Cronk Q.C.B, Bateman R.M, Hawkins J.A. Taylor and Francis; London, UK: 2005. Developmental genetics and plant evolution. [Google Scholar]
  16. Cubas P.E, Vincent C, Coen E. An epigenetic mutation responsible for natural variation in floral symmetry. Nature. 1999;401:157–161. doi: 10.1038/43657. doi:10.1038/43657 [DOI] [PubMed] [Google Scholar]
  17. Cunningham J.P, West S.A, Wright D.J. Learning in the nectar foraging behaviour of Helicoverpa armigera. Ecol. Entomol. 1998;23:363–369. doi:10.1046/j.1365-2311.1998.00149.x [Google Scholar]
  18. Dafni A, Kevan P.G. Flower size and shape: implications in pollination. Isr. J. Plant Sci. 1997;45:201–211. [Google Scholar]
  19. Dilcher D. Toward a new synthesis: major evolutionary trends in the angiosperm fossil record. Proc. Natl Acad. Sci. USA. 2000;97:7030–7036. doi: 10.1073/pnas.97.13.7030. doi:10.1073/pnas.97.13.7030 [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Dilley J.D, Wilson P, Mesler M.R. The radiation of Calochortus: generalist flowers moving through a mosaic of potential pollinators. Oikos. 2000;89:209–222. doi:10.1034/j.1600-0706.2000.890201.x [Google Scholar]
  21. Donoghue M.J, Ree R, Baum D.A. Phylogeny and the evolution of flower symmetry in Asteridae. Trends Plant Sci. 1998;3:311–317. doi:10.1016/S1360-1385(98)01278-3 [Google Scholar]
  22. Endress P.K. Symmetry in flowers: diversity and evolution. Int. J. Plant Sci. 1999;160:S3–S23. doi: 10.1086/314211. doi:10.1086/314211 [DOI] [PubMed] [Google Scholar]
  23. Endress P.K. Evolution of floral symmetry. Curr. Opin. Plant Biol. 2001;4:86–91. doi: 10.1016/s1369-5266(00)00140-0. doi:10.1016/S1369-5266(00)00140-0 [DOI] [PubMed] [Google Scholar]
  24. Fenster C.B, Armbruster W.S, Wilson P.M, Dudash R, Thompson J.D. Pollination syndromes and floral specialization. Annu. Rev. Ecol. Evol. Syst. 2004;35:375–404. doi:10.1146/annurev.ecolsys.34.011802.132347 [Google Scholar]
  25. Fenster C.B, Cheely G, Dudash M.R, Reynolds R.J. Nectar reward and advertisement in hummingbird-pollinated Silene virginica (Caryophyllaceae) Am. J. Bot. 2006;93:1800–1807. doi: 10.3732/ajb.93.12.1800. doi:10.3732/ajb.93.12.1800 [DOI] [PubMed] [Google Scholar]
  26. Galen C, Newport M.E.A. Bumble bee behavior and selection on flower size in the sky pilot, Polemonium viscosum. Oecologia. 1987;74:20–23. doi: 10.1007/BF00377340. doi:10.1007/BF00377340 [DOI] [PubMed] [Google Scholar]
  27. Galen C, Cuba J. Down the tube: pollinators, predators, and the evolution of flower shape in the alpine skypilot, Polemonium viscosum. Evolution. 2001;55:1963–1971. doi: 10.1111/j.0014-3820.2001.tb01313.x. doi:10.1111/j.0014-3820.2001.tb01313.x [DOI] [PubMed] [Google Scholar]
  28. Giurfa M, Lehrer M. Honeybee vision and floral displays: from detection to close-up recognition. In: Chittka L, Thomson J.D, editors. Cognitive ecology of pollination. Cambridge University Press; Cambridge, UK: 2001. pp. 61–82. [Google Scholar]
  29. Gómez J.M. Herbivory reduces the strength of pollinator-mediated selection in the Mediterranean herb Erysimum mediohispanicum: consequences for plant specialization. Am. Nat. 2003;162:242–256. doi: 10.1086/376574. doi:10.1086/376574 [DOI] [PubMed] [Google Scholar]
  30. Gómez J.M. Non-additivity effect of herbivores and pollinators on Erysimum mediohispanicum (Cruciferae) fitness. Oecologia. 2005;143:412–418. doi: 10.1007/s00442-004-1809-7. doi:10.1007/s00442-004-1809-7 [DOI] [PubMed] [Google Scholar]
  31. Gómez J.M, Zamora R. Generalization vs. specialization in the pollination system of Hormathophylla spinosa (Cruciferae) Ecology. 1999;80:796–805. [Google Scholar]
  32. Gómez J.M, Zamora R. Spatial variation in the selective scenarios of Hormathophylla spinosa (Cruciferae) Am. Nat. 2000;155:657–668. doi: 10.1086/303353. doi:10.1086/303353 [DOI] [PubMed] [Google Scholar]
  33. Gómez J.M, Zamora R. Ecological factors that promote the evolution of generalization in pollination systems. In: Waser N.M, Ollerton J, editors. Plant–pollinator interactions, from specialization to generalization. University of Chicago Press; Chicago, IL: 2006. pp. 145–165. [Google Scholar]
  34. Gómez J.M, Perfectti F, Camacho J.P.M. Natural selection on Erysimum mediohispanicum flower shape. Insights into the evolution of zygomorphy. Am. Nat. 2006;168:531–545. doi: 10.1086/507048. doi:10.1086/507048 [DOI] [PubMed] [Google Scholar]
  35. Gómez J.M, Bosch J, Perfectti F, Fernández J.D, Abdelaziz M, Camacho J.P.M. Association between floral traits and reward in Erysimum mediohispanicum (Brassicaceae) Ann. Bot. 2008;101:1413–1420. doi: 10.1093/aob/mcn053. doi:10.1093/aob/mcn053 [DOI] [PMC free article] [PubMed] [Google Scholar]
  36. Gould J.L. How bees remember flower shapes. Science. 1985;227:1492–1494. doi: 10.1126/science.227.4693.1492. doi:10.1126/science.227.4693.1492 [DOI] [PubMed] [Google Scholar]
  37. Harder L.D, Barrett S.C.H. Oxford University Press; Oxford, UK: 2006. Ecology and evolution of flowers. [Google Scholar]
  38. Heinrich B. Harvard University Press; New York, NY: 1993. The hot-blooded insects. [Google Scholar]
  39. Herrera C.M. Selection on floral morphology and environmental determinants of fecundity in a hawk moth-pollinated violet. Ecol. Monogr. 1993;63:251–275. doi:10.2307/2937101 [Google Scholar]
  40. Herrera C.M, Castellanos M.C, Medrano M. Geographical context of floral evolution: towards an improved research programme in floral diversification. In: Harder L.D, Barrett S.C.H, editors. Ecology and evolution of flowers. Oxford University Press; Oxford, UK: 2006. pp. 278–294. [Google Scholar]
  41. Internicola A.I, Page P.A, Bernasconi G, Gigord L.D.B. Competition for pollinator visitation between deceptive and rewarding artificial inflorescences: an experimental test of the effects of floral colour similarity and spatial mingling. Funct. Ecol. 2007;21:864–872. doi:10.1111/j.1365-2435.2007.01303.x [Google Scholar]
  42. Johnson S.D, Dafni A. Response of bee-flies to the shape and pattern of model flowers: implications for floral evolution in a Mediterranean herb. Funct. Ecol. 1998;12:289–297. doi:10.1046/j.1365-2435.1998.00175.x [Google Scholar]
  43. Johnson S.D, Steiner K.E. Generalization vs. specialization in plant pollination systems. Trends Ecol. Evol. 2000;15:140–143. doi: 10.1016/s0169-5347(99)01811-x. doi:10.1016/S0169-5347(99)01811-X [DOI] [PubMed] [Google Scholar]
  44. Kalisz S, Ree R.H, Sargent R.D. Linking floral symmetry genes to breeding system evolution. Trends Plant Sci. 2006;11:568–573. doi: 10.1016/j.tplants.2006.10.005. doi:10.1016/j.tplants.2006.10.005 [DOI] [PubMed] [Google Scholar]
  45. Kearns C.A, Inouye D.W. University Press of Colorado; Colorado, CO: 1993. Techniques for pollination biologists. [Google Scholar]
  46. Klingenberg C.P, Leamy L.J. Quantitative genetics of geometric shape in the mouse mandible. Evolution. 2001;55:2342–2352. doi: 10.1111/j.0014-3820.2001.tb00747.x. doi:10.1111/j.0014-3820.2001.tb00747.x [DOI] [PubMed] [Google Scholar]
  47. Klingenberg C.P, Monteiro L.R. Distances and directions in multidimensional shape spaces: implications for morphometric applications. Syst. Biol. 2005;54:678–688. doi: 10.1080/10635150590947258. doi:10.1080/10635150590947258 [DOI] [PubMed] [Google Scholar]
  48. Lande R, Arnold S.J. The measurement of selection on correlated characters. Evolution. 1983;37:1210–1226. doi: 10.1111/j.1558-5646.1983.tb00236.x. doi:10.2307/2408842 [DOI] [PubMed] [Google Scholar]
  49. Lloyd D.G, Barrett C.H. Chapman and Hall; New York, NY: 1996. Floral biology, studies on floral evolution in animal-pollinated plants. [Google Scholar]
  50. Luo D, Carpenter R, Vincent C, Copsey L, Coen E. Origin of floral asymmetry in Antirrhinum. Nature. 1995;383:794–799. doi: 10.1038/383794a0. doi:10.1038/383794a0 [DOI] [PubMed] [Google Scholar]
  51. Makino T.T, Sakai S. Experience changes pollinator responses to floral display size: from size-based to reward-based foraging. Funct. Ecol. 2007;21:854–863. doi:10.1111/j.1365-2435.2007.01293.x [Google Scholar]
  52. Moellar D.A. Pollinator community structure and sources of spatial variation in plant–pollinator interaction in Clarkia xantiana spp. xantiana. Oecologia. 2005;142:28–37. doi: 10.1007/s00442-004-1693-1. doi:10.1007/s00442-004-1693-1 [DOI] [PubMed] [Google Scholar]
  53. Moellar D.A. Geographic structure of pollinator communities, reproductive assurance, and the evolution of self-pollination. Ecology. 2006;87:1510–1522. doi: 10.1890/0012-9658(2006)87[1510:gsopcr]2.0.co;2. doi:10.1890/0012-9658(2006)87[1510:GSOPCR]2.0.CO;2 [DOI] [PubMed] [Google Scholar]
  54. Möller A.P. Bumblebee preference for symmetrical flowers. Proc. Natl Acad. Sci. USA. 1995;92:2288–2292. doi: 10.1073/pnas.92.6.2288. doi:10.1073/pnas.92.6.2288 [DOI] [PMC free article] [PubMed] [Google Scholar]
  55. Möller A.P, Sorci G. Insect preference for symmetrical artificial flowers. Oecologia. 1998;114:37–42. doi: 10.1007/s004420050417. doi:10.1007/s004420050417 [DOI] [PubMed] [Google Scholar]
  56. Muchhala N. Adaptive trade-off in floral morphology mediates specialization for flowers pollinated by bats and hummingbirds. Am. Nat. 2007;169:494–504. doi: 10.1086/512047. doi:10.1086/512047 [DOI] [PubMed] [Google Scholar]
  57. Neal P.R, Dafni A, Giurfa M. Floral symmetry and its role in plant–pollinator systems: terminology, distribution, and hypotheses. Annu. Rev. Ecol. Syst. 1998;29:345–373. doi:10.1146/annurev.ecolsys.29.1.345 [Google Scholar]
  58. Olsen K.M. Pollination effectiveness and pollinator importance in a population of Heterotheca subaxillaris (Asteraceae) Oecologia. 1997;109:114–121. doi: 10.1007/PL00008811. doi:10.1007/PL00008811 [DOI] [PubMed] [Google Scholar]
  59. Plepys D, Ibarra F, Fracnke W, Löfstedt C. Odour-mediated nectar foraging in the silver Y moth Autographa gamma (Lepidoptera: Noctuidae): behavioural and electrophysiological responses to floral volatiles. Oikos. 2002;99:75–82. doi:10.1034/j.1600-0706.2002.990108.x [Google Scholar]
  60. Ree R.H, Donoghue M.J. Inferring rates of change in flower symmetry in Asterid angiosperms. Syst. Biol. 1999;48:633–641. doi:10.1080/106351599260201 [Google Scholar]
  61. Reeves P.A, Olmstead R.G. Evolution of novel morphological and reproductive traits in a clade containing Antirrhinum majus (Scrophulariaceae) Am. J. Bot. 1998;85:1047–1056. doi:10.2307/2446338 [PubMed] [Google Scholar]
  62. Rohlf F.J, Corti M. Use of two-block partial least-squares to study covariation in shape. Syst. Biol. 2000;49:740–753. doi: 10.1080/106351500750049806. doi:10.1080/106351500750049806 [DOI] [PubMed] [Google Scholar]
  63. Rohlf F.J, Loy A, Corti M. Morphometric analysis of Old World Talpidae (Mammalia, Insectivora) using partial-warp scores. Syst. Biol. 1996;45:344–362. doi:10.2307/2413569 [Google Scholar]
  64. Sargent R.D, Otto S.P. The role of local species abundance in the evolution of pollinator attraction in flowering plants. Am. Nat. 2006;167:67–80. doi: 10.1086/498433. doi:10.1086/498433 [DOI] [PubMed] [Google Scholar]
  65. Schaefer H.M, Schaefer V, Levey D.J. How plant-animal interactions signal new insights in communication. Trends Ecol. Evol. 2004;19:1–8. doi: 10.1016/j.tree.2003.11.001. doi:10.1016/j.tree.2003.11.001 [DOI] [PubMed] [Google Scholar]
  66. Scheiner R, Erber J, Page R.E., Jr Tactile learning and the individual evaluation of the reward in honey bees (Apis mellifera L.) J. Comp. Physiol. A. 1999;185:1–10. doi: 10.1007/s003590050360. doi:10.1007/s003590050360 [DOI] [PubMed] [Google Scholar]
  67. Schemske D.W, Bradshaw H.D. Pollinator preference and the evolution of floral traits in monkeyflowers (Mimulus) Proc. Natl Acad. Sci. USA. 1999;96:11 910–11 915. doi: 10.1073/pnas.96.21.11910. doi:10.1073/pnas.96.21.11910 [DOI] [PMC free article] [PubMed] [Google Scholar]
  68. Smithson A, Macnair M.R. Negative frequency-dependent selection by pollinators on artificial flowers without rewards. Evolution. 1997;51:715–723. doi: 10.1111/j.1558-5646.1997.tb03655.x. doi:10.2307/2411148 [DOI] [PubMed] [Google Scholar]
  69. Stanton M.L, Preston R.E. Ecological consequences and phenotypic correlates of flower size variation in wild radish, Raphanus sativus L. (Brassicaceae) Am. J. Bot. 1988;75:528–539. doi:10.2307/2444218 [Google Scholar]
  70. Stanton M, Young H.J. Selection for floral character associations in wild radish, Raphanus sativus L. J. Evol. Biol. 1994;7:271–285. doi:10.1046/j.1420-9101.1994.7030271.x [Google Scholar]
  71. Vazquez D.P, Morris W.F, Jordano P. Interaction frequency as a surrogate for the total effect of mutualistic animals on plants. Ecol. Lett. 2005;8:1088–1094. doi:10.1111/j.1461-0248.2005.00810.x [Google Scholar]
  72. Waddington K.D. Subjective evaluation and choice behavior by nectar- and pollen-collecting bees. In: Chittka L, Thomson J.D, editors. Cognitive ecology of pollination: animal behavior and floral evolution. Cambridge University Press; Cambridge, UK: 2001. pp. 41–60. [Google Scholar]
  73. Walker J.A. The ability of geometric morphometric methods to estimate a known covariance matrix. Syst. Biol. 2000;49:686–696. doi: 10.1080/106351500750049770. doi:10.1080/106351500750049770 [DOI] [PubMed] [Google Scholar]
  74. Wilson P, Thomson J.D. How do flowers diverge? In: Lloyd D, Barrett S.C.H, editors. Floral biology. Chapman & Hall; New York, NY: 1996. pp. 88–111. [Google Scholar]
  75. Yoshioka Y, Ohashi K, Konuma A, Iwata H, Ohsawa R, Ninomiya S. Ability of bumblebees to discriminate differences in the shape of artificial flowers of Primula sieboldii (Primulaceae) Ann. Bot. 2007;90:1175–1182. doi: 10.1093/aob/mcm059. doi:10.1093/aob/mcm059 [DOI] [PMC free article] [PubMed] [Google Scholar]
  76. Young H.J, Stanton M.L. Influences of floral variation on pollen removal and seed production in wild radish. Ecology. 1990;71:536–547. doi:10.2307/1940307 [Google Scholar]
  77. Zelditch M.L, Swiderski D.L, Sheets H.D, Fink W.L. Elsevier Academic Press; San Diego, CA: 2004. Geometric morphometrics for biologists: a primer. [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Outcome of the geometric morphometric analysis of corolla shape

Geometric morphometric analysis of Erysimum mediohispanicum corolla shape and correlations between relative warps and PLS latent vectors for pollen and nectar contents.

rspb20080512s12.doc (122.5KB, doc)
Correlations among each shape component and the phenotypic traits

Correlation among each Erysimum mediohispanicum shape component and the phenotypic traits describing plant and flower size.

rspb20080512s13.doc (53.5KB, doc)
Standardized multivariate selection analysis on corolla shape and size

Standardized multivariate selection analysis on corolla shape and size for each E. mediohispanicum population

rspb20080512s14.doc (52.5KB, doc)

Articles from Proceedings of the Royal Society B: Biological Sciences are provided here courtesy of The Royal Society

RESOURCES