Statistical mechanics and hydrodynamics of bacterial suspensions

Abstract

Unicellular living organisms, such as bacteria and algae, propel themselves through a medium via cyclic strokes involving the motion of cilia and flagella. Dense populations of such “active particles” or “swimmers” exhibit a rich collective behavior at large scales. Starting with a minimal physical model of a stroke-averaged swimmer in a fluid, we derive a continuum description of a suspension of active organisms that incorporates fluid-mediated, long-range hydrodynamic interactions among the swimmers. Our work demonstrates that hydrodynamic interactions provide a simple, generic origin for several nonequilibrium phenomena predicted or observed in the literature. The continuum model derived here does not depend on the microscopic physical model of the individual swimmer. The details of the large-scale physics do, however, differ for “shakers” (particles that are active but not self-propelled, such as melanocytes) and “movers” (self-propelled particles), “pushers” (most bacteria) and “pullers” (algae like Chlamydomonas). Our work provides a classification of the large-scale behavior of all these systems.

The world of a swimmer at low Reynolds numbers, beautifully described by Purcell in his classic 1977 paper (1), is full of surprises that defy intuition. Much attention has been devoted over the years to understanding the propulsion mechanisms that drive a single organism through a medium (2). More recently, the focus has shifted to the even richer behavior of collections of low-Reynolds-number swimmers, such as bacterial colonies (3, 4), sperm cells (5), and cell extracts of cytoskeletal filaments and motor proteins (6). These systems exhibit fascinating collective behavior, including the possibility of nonequilibrium phase transitions between disordered and ordered (possibly moving) states, novel long-range correlations, and pattern formation on mesoscopic scales. Apparently diverse phenomena, such as the large-scale swirling motion observed in bacterial suspensions and the formation of intricate aster and spiral structures in extracts of cytoskeletal filaments and motor proteins, are well described by the same continuum phenomenological hydrodynamics of active suspensions. Such collective phenomena arise from interactions among the active particles. For a complete understanding of the collective physics of these systems, it is important to elucidate the relative roles of physical interactions, such as excluded volume and medium-mediated couplings, genetically and biochemically regulated signaling, and external symmetry-breaking effects, such as chemotaxis. A first important step in this direction is understanding how activity or self-propulsion modify physical interactions among the units. In an earlier work (7), we showed that modifications of the short-range excluded volume interactions due to self-propulsion can explain nonequilibrium effects, such as anomalous number fluctuations observed in active particles on rigid substrates (8). Here we show that for active particles in a fluid, such as bacteria or collections of living cells, all the large-scale nonequilibrium phenomena described in the literature arise from the long-range nature of the hydrodynamic interactions among the active particles.

Theoretical efforts to understand the physics of active systems fall broadly into three categories. A group of researchers has analyzed the flow induced by individual or pairs of swimmers moving in a viscous fluid by studying simplified models of moving particles (9–11). A second community has focused on the physics on length scales large as compared with the size of the swimmers by proposing phenomenological hydrodynamic equations built by modifying the well-understood hydrodynamics of liquid crystal to include nonequilibrium terms that account for the activity of the system (12–15). Because of their elongated shape, active particles, like nematogens, can exhibit orientational order at high concentration (16) and have been likened to “living liquid crystals”. The phenomenological theories have yielded several important results, including the prediction of a “generic instability” of all bulk-ordered states of active suspensions (12), the description of the mesoscopic vortices and asters formed in the region where homogeneous ordered states are unstable (17), and the demonstration of novel rheological behavior (18–20). Finally, a third approach has been the derivation of the continuum hydrodynamic equations from specific microscopic models of the dynamics. This approach has included rule-based dynamical models (21, 22), inspired by the seminal work of Vicsek (23), and physical models of self-propelled rods and filaments on a substrate (7, 17, 24). Here we use a simple model of interacting swimmers in a fluid to derive continuum equations describing the large-scale behavior of active suspensions. Our model reproduces the instabilities of both isotropic and ordered homogeneous states previously discussed in the literature and uncovers new ones. It also shows that the long-range nature of hydrodynamic interactions is responsible for all such instabilities.

Bacteria propel themselves by a variety of periodic, nonreciprocating strokes that involve the motion of flagella or cilia (2). Here we are interested in the collective behavior of many swimmers on long time and length scales. With this goal in mind, the details of the mechanism of self-propulsion is not important, and we simply approximate the force distribution far from an individual swimmer as that of a static force dipole (25). Our physical model of a stroke-averaged swimmer is an asymmetric rigid dumbbell exerting a force dipole of strength |f| on the fluid, as shown in Fig. 1. The asymmetry of our active dumbbell is essential to make it a “swimmer” as opposed to a “shaker” (a particle—such as a melanocyte, which distributes pigments in the skin (16)—that is active because of intrinsic energy sources but is not self-propelled). In the Stokes regime, a swimmer needs to have a stroke that is not time-reversal invariant. In the stroke-averaged model considered here, this broken temporal symmetry translates into a broken spatial symmetry, corresponding in our case to the asymmetry of the dumbbell. An isolated dumbbell swimmer of length ℓ propels itself through a fluid of viscosity η at a velocity v_SP = v₀v̂, with v₀ = −fΔa/(8πηℓᾱ), ᾱ = (a_L + a_S)/2 and Δa = a_L − a_S. Hence, v₀ = 0 if a_L = a_S. More generally, all symmetric swimmers, defined as those for which the center of the force dipole coincides with the hydrodynamic center of the swimmer (26), are actually “shakers” with v₀ = 0. Finally, it is important to stress that although our model for a single swimmer is a static force dipole, hydrodynamic interactions do generate all multipoles in the collective flow field.

Fig. 1.

Our stroke-averaged swimmer is an asymmetric rigid dumbbell composed of a small and a large sphere of radii a_S and a_L, respectively, connected by an infinitely thin rigid rod. The swimmer has length ℓ, and its orientation is characterized by a unit vector v̂ directed along its axis from the small to the large sphere. Also shown are the equal and opposite forces f = fv̂ that the swimmer exerts on the fluid. Propulsion is centered at the hydrodynamic center (26), denoted by C, which here lies to the right of the swimmer's geometrical midpoint M, which is also the center of the force dipole.

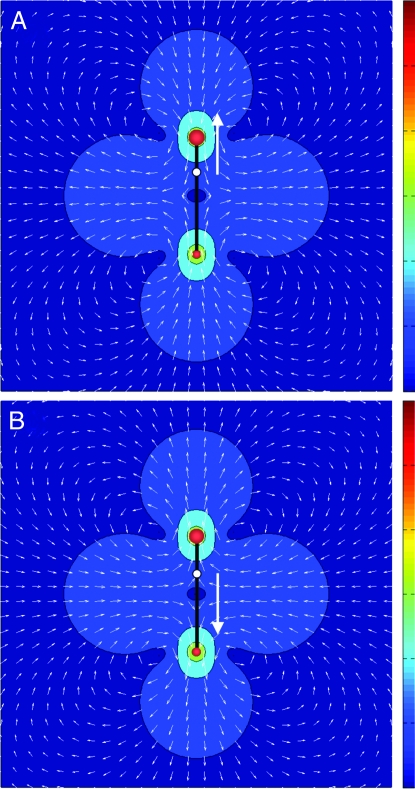
Active particles can also be classified according to the forces they exert on the surrounding fluid. Contractile swimmers or “pullers” are propelled by flagella at the head of the organism. They pull fluid in along their long axis and push fluid out along an axis normal to their midpoint (Fig. 2A). With the definition of forces shown in Fig. 1, pullers correspond to f < 0. The unicellular flagellate algae Chlamydomonas are examples of pullers. In the stroke-averaged representation, the swimmer is a puller when the hydrodynamic center lies near the “head” of the swimmer, defined with respect to its direction of self-propelled motion. Conversely, tensile swimmers with f > 0 push fluid out along their long axis and pull fluid in at their midpoint. They are propelled from the rear, hence “pushers”. Most bacteria, such as Escherichia coli, belong to this class. In this case, the hydrodynamic center of the swimmer lies near the “tail” (Fig. 2B).

Fig. 2.

Flow patterns induced by the active forces that a single puller (A) or pusher (B) exerts on the surrounding fluid. The colors denote the amplitude of the flow that decreases at large distances, albeit only as a power law (red, largest, to blue, weakest). The arrows indicate the direction of the flow. Also shown for each swimmer are the location of the hydrodynamic center (white circle) and the direction of the self-propulsion velocity (white arrow). In both cases, the flow vanishes as expected at the center of the dipole.

We derive equations for the microscopic dynamics of a collection of such swimmers in a fluid by eliminating the fluid flow velocity and recasting it explicitly in the form of long-ranged hydrodynamic interactions among the particles. We characterize the resulting interactions and identify how they depend on the swimmer's status as either a puller or a pusher. At short distances, the swimmers also interact repulsively via excluded volume effects that are known to yield orientational order at high density. These effects are understood (27), and so for simplicity we do not include them in the derivation of the continuum hydrodynamics. By using the standard tools of nonequilibrium statistical mechanics, we then obtain coarse-grained hydrodynamic equations that describe the collective dynamics of the swimmers on long length and time scales. Finally, we examine the nature of fluctuations in these systems and the underlying microscopic mechanisms that give rise to them. Before describing the derivation, we summarize some key new outcomes of our work.

1. We show that pairwise hydrodynamic interactions (within the Stokes approximation) alone do not yield homogeneous orientational order in bulk. When steric effects are incorporated in the model, the swimmers order in a nematic state at high density. This is an equilibrium effect (although modified by self-propulsion (7)). Neither steric effects nor hydrodynamic interactions yield, however, a bulk polar state.

2. We demonstrate that a uniform isotropic suspension of pullers is unstable above a critical active force |f|. This instability arises from the suppression of mass diffusion because of the attractive hydrodynamic forces along the axis of contractile swimmers. It is similar to the “bundling” instability obtained in refs. 17 and 24 for filament-motor mixtures. In that case, however, the bundling is induced by active cross-linkers; hence, by a very specific contact interaction among filaments. In the present work, the suppression of diffusion arises entirely from hydrodynamic forces among the swimmers and is therefore a general new effect.

3. It is known that uniform ordered active suspensions (swimmers, shakers, pullers, or pushers) are “generically” unstable because of the growth of orientational fluctuations (12). Our work identifies the origin of this instability in the long-ranged (∼ 1/r²) nature of the hydrodynamic interactions. This connection also suggests mechanisms for suppressing the instability, as described in Hydrodynamic Equations.

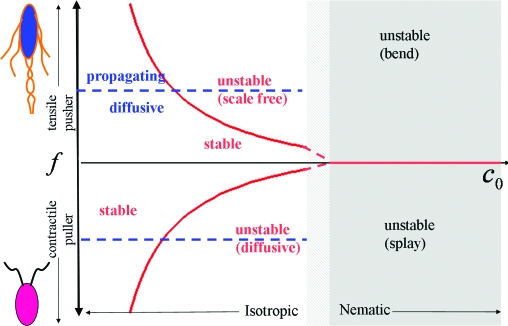
4. Our work shows that the instabilities of active suspensions arise from hydrodynamic interactions and provides the classification of such instabilities for different types of swimmers summarized in the phase diagram of Fig. 3.

5. By providing a microscopic calculation of the parameters in the hydrodynamic equations, we show that the parameter controlling the instabilities is a Péclet number defined in terms of the typical convective velocity of the suspension (rather than the individual swimmer's velocity).

Fig. 3.

A “phase diagram” for active suspensions. The vertical axis is the active force f, with f > 0 for pushers and f < 0 for pullers. The horizontal axis is the mean concentration, c₀. The red lines mark the onset of the instability of the homogeneous states, which is scale-free for pushers and diffusive for pullers. The ordered state is unstable for all f. A transition from the isotropic to the nematic state is expected to occur in the shaded region, which is not accessible to the present theory. Swimmers (but not shakers) also exhibit propagating (sound-like) density waves above a threshold value of |f| indicated by the horizontal dashed blue line.

Finally, our work also reproduces the orientational instability of a uniform isotropic suspension of pushers reported in refs. 29 and 30. Here this instability occurs because above a critical f, any inhomogeneity in the orientational order is enhanced by active hydrodynamic torques, which tend to align the swimmers when f > 0.

To address the important question of whether these instabilities may be observable experimentally, we need to estimate the parameters that control the strength of the interaction among active units. The analysis below shows that the instabilities are controlled by a dimensionless concentration of active particles, c₀^* = V_actc₀, where V_act = ℓᾱ(ℓPe) is the active volume of each swimmer. Here $Pe=\frac{v\ell }{D}$ is the Péclet number of the suspension, defined as the ratio of the convective flow velocity v ∼ |f|/ζ induced by the activity to the typical diffusion velocity, D/ℓ. If Pe > 1, diffusion is ineffective at “mixing” the suspension and activity dominates, resulting in the unstable growth of fluctuations when c₀^* > 1. The Péclet number defined here is not controlled by the self-propulsion speed of an individual organism (which vanishes for shakers) but rather by the larger speed ∼|f|/ζ that characterizes large-scale activity-induced convection. Experiments such as the one reported in ref. (3) have shown that v can exceed 100 μ m/s in dense bacterial suspensions, rendering collective nonequilibrium phenomena observable even for weak activity of the individual particles.

Model Swimmer and Hydrodynamic Interactions

Our model of a stroke-averaged swimmer is shown in Fig. 1. It is an asymmetric rigid dumbbell with spherical head and tail of radii a_L and a_S. The length ℓ of the swimmer is the distance between the centers of the two spheres. The orientation of the swimmer is described by a unit vector v̂ directed along its axis from the small to the large sphere. The swimmer exerts equal and opposite forces f = ±fv̂ on the fluid. The center of this symmetric force dipole is the swimmer's midpoint, M, located at ℓ/2. The fluid exerts equal and opposite forces on the swimmer and the net force is zero at all times. The swimmer is assumed to be at neutral buoyancy. The Reynolds number Re = ρvℓ/η for one of these swimmers, where ρ and η are the density and viscosity of the fluid, and v the typical velocity of the swimmer, can be estimated by using numbers typical for bacteria (v ∼ 10 − 30μm/sec, ℓ ∼ μm, η ∼ 10⁻² − 1Pasec) as Re ∼ 10⁻⁴ − 10⁻⁵. In this regime one can assume that, in the absence of fluid fluctuations, the swimmer is convected along with the fluid at the local fluid velocity, u(r). The dynamics of the αth rigid swimmer is then described by

where r_Lα and r_Sα denote the position of the large and small sphere with respect to a fixed origin, with r_Lα − r_Sα = ℓv̂_α to enforce the rigidity constraint. The flow velocity u(r) of the fluid at r is determined by the solution of the Stokes equation,

where

is the active force density by the swimmer on the fluid, and

describes the effect of fluid fluctuations. The random forces ξ_α^L,S(t) are Markovian white noise terms on each of the two spheres. They have zero mean and variance 〈ξ_αi^L,S(t)ξ_βj^L,S(t′)〉 = 2ζ_L,Sk_BT_aδ_ijδ_αβδ(t − t′), with ζ_L,S = 6πηa_L,S the friction of a sphere of radius a_L,S in a fluid of viscosity η and T_a an active temperature. In general, there will be noise generated by the swimmer itself. Here we assume that this can also be described as white Langevin noise and only modifies the amplitude of the fluctuations. Finally, we assume that the fluid is incompressible; hence ∇·u = 0.

The Stokes equation given by Eq. 2 can be solved with the result

where O_ij(r) is the Oseen tensor, O_ij(r) = (δ_ij + r̂_ir̂_j)/(8πηr), for |r| > a_L,S, with r̂ = r/|r| a unit vector. The Oseen tensor relating the flow around a sphere of radius a_L,S to a point force applied from the center of the sphere diverges at the origin for a point force. This divergence is eliminated in the standard way by assuming O_ij(|r| ≤ a_L,S) = δ_ij/ζ_L,S.

The dynamics of an extended rigid body in Stokes flow is conveniently described in terms of the translation of the hydrodynamic center of the body and rotations about this point. The hydrodynamic center is the point about which the net hydrodynamic torque on the body vanishes (26). The hydrodynamic center plays the same role in Stokes flow that the center of mass plays in inertial dynamics. For a rigid dumbbell in an externally imposed flow the hydrodynamic center is given by

This definition will also be used here to identify the hydrodynamic centers of our swimmers.

By using the formal solution, Eq. 5, of the Stokes equation we can eliminate the flow field from Eq. 1 and obtain closed equations of motion describing the translational and rotational dynamics of the swimmers in the fluid, in the form

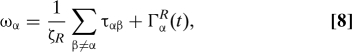where the angular velocity describing rotations about the hydrodynamic center is defined by ∂_tv̂_α = v̂_α × ω_α and ζ̄ = (ζ_L + ζ_S)/2, ζ_R = ℓ²ζ̄. The forces F_αβ and torques τ_αβ arise from hydrodynamic couplings among the swimmers. The random forces Γ_α and Γ_α^R lead to diffusion of the swimmers at large length scales. They may be expressed in terms of the random forces ξ^L,S(t) on each sphere. Neglecting for simplicity hydrodynamic effects in the noise, which have been studied (31), their correlations are given by

At low density, D_∥, D_⊥ ∼ k_BT/ζ̄ and D_R ∼ k_BT/(ζ̄ℓ²). The asymmetry of translational diffusion (D_∥ > D_⊥) is due to the hydrodynamic interactions induced by fluid fluctuations at the head and tail of the swimmer. The hydrodynamic interactions due to the random force between different swimmers yield corrections of order (k_BT_a/ζ̄)(ℓ/r₁₂)² to the diffusion constants, with r₁₂ = |r₁^C − r₂^C|. At a large Péclet number, these are negligible compared with the order (v₀²/ζ̄)(ℓ/r₁₂)² corrections from active forces.

It is clear from Eqs. 7 and 8 that an isolated swimmer propels itself in a straight line with self-propulsion velocity v₀v̂ and v₀ = −fΔa/(8πηℓᾱ).* The presence of other swimmers yields hydrodynamic forces F_αβ and torques τ_αβ by the β th swimmer on the α th swimmer. To obtain tractable expressions for the hydrodynamic forces and torques, we carry out a multipole expansion of the force distribution on the right hand side of Eq. 5 around the hydrodynamic center of each swimmer by letting r_αL = r_α^C + (ζ_S/2ζ̄)ℓv̂_α and r_αS = r_α^C − (ζ_L/2ζ̄ℓ)v̂_α and expanding about r_α^C. This expansion corresponds to an one in ℓ/r₁₂. Assuming r₁₂ ∼ c₀^−1/3, this is a small parameter at low mean concentration c₀ of swimmers. In spite of this approximation, the form of the hydrodynamic equations obtained here remains valid at high concentration, although with different values of the various parameters. We have explicitly evaluated hydrodynamic forces and torques up to octupole order in the multipole expansion. The full expressions are complicated and will not be given here. By retaining only the leading terms for each contribution and dropping numerical coefficients of order one†, we obtain

The hydrodynamic force between two swimmers is directed along the radius joining the hydrodynamic centers of the swimmers. The hydrodynamic force is nonzero for both shakers (v₀ = 0) and movers (v₀≠0) and decays as 1/r₁₂². The torque consists of two terms. The first term, proportional to fᾱ/r₁₂³, is nonzero even for shakers. This term is invariant for v̄₁ → −v̄₁ and v̂₂ → −v̂₂ and aligns swimmers regardless of their polarity. The second term, proportional to f(Δa/ᾱ)², vanishes for symmetric swimmers and tends to align swimmers of the same polarity. The force exerted by a puller (pusher) is attractive (repulsive) along the direction of its swimming axis, and repulsive (attractive) in the orthogonal direction. The torque exerted by a puller tends to align other swimmers that are located along its axis and to misalign swimmers in the orthogonal directions. The converse applies to pushers. These differences have important consequences in controlling the details of the instabilities.

Hydrodynamic Equations

The collective dynamics of many swimmers on length scales large as compared with their mean separation and time scales long as compared with the duration of the stroke of an individual swimmer is well described in terms of conserved quantities and broken symmetry variables. The only conserved quantity in our model is the density of swimmers‡, c(r,t), defined as

where the brackets denote an average over the microscopic degrees of freedom. There are two broken symmetry variables, corresponding to the possibility of polar and nematic order of the swimmers, defined as

We have used standard methods of nonequilibrium statistical mechanics to coarse grain the microscopic dynamics described by Eqs. 7 and 8 and derive continuum equations for these fields. The continuum equations are nonlinear and nonlocal. Their general form is given in the supplementary material. In the rest of the section, we will focus on some special cases.

Homogeneous States.

One of the outcomes of our analysis is that pairwise, additive, hydrodynamic interactions in the Stokes regime considered here do not yield a homogeneous ordered state in bulk. This result follows because the kernels governing the nonlocal hydrodynamic interaction have an isotropic angular dependence and vanish in mean field. If the only interactions are far-field hydrodynamic ones, the only uniform steady state of a bulk suspension of swimmers is an isotropic state, with c = c₀, P = 0, and Q = 0. Of course, at short distances steric repulsion becomes important. Due to the rod-like shape of the swimmers, these will yield a uniform nematic state (c = c₀, P = 0 and Q≠0) above a critical concentration. Excluded volume effects are not, however, sufficient to generate a homogeneous polar state (c = c₀, P≠0; hence Q≠0) (7). The identification of a physical mechanism, if any, capable of generating a macroscopic polar state in a bulk system remains an issue to be examined, one which is beyond the scope of this paper. Further investigation may require contact interactions that capture higher-order chemical processes (such as the action of motors modeled in ref. 19) or external symmetry-breaking through a chemotactic gradient or boundaries. In States with Orientational Order, we assume the existence of such a putative state and study its stability.

Isotropic State.

To study the stability of the isotropic state, we consider the dynamics of fluctuations δy_α = y_α − y_α⁰ of the hydrodynamic fields y_α = {c,P,Q} about their homogeneous values, y_α⁰ = {c₀,0,0}. We introduce a Fourier representation δỹ_α(k) = ∫_re^ik·rδy_α(r) and retain terms up to quadratic order in k in the equations by expanding the nonlocal hydrodynamic couplings. The three coupled fields that control the stability of the system are the density fluctuations δc̃, the longitudinal polarization fluctuations δP_∥ = k̂·δP̃, and the “bend” δQ_∥⊥ = k̂·Q̂·(δ−k̂k̂) and “splay” δQ_∥∥ = k̂·Q̃·k̂ components of the fluctuations in the alignment tensor. The coupled linearized equations for these four scalar fields are

where D, D_sp, and D_P are diffusion constants that can be evaluated in terms of microscopic parameters for the specific model considered here. At low density, they are of order k_BT/ζ̄, with additive corrections ∼ V_actc₀ from activity. The implications of these equations depend on the contractile or tensile nature of the swimmers.

For contractile (f < 0), swimmers ᾱ₁ ∼ ᾱ₂ ∼ fᾱℓc₀/ζ̄ < 0. Hence, splay fluctuations δQ_∥⊥ are stable and overdamped and can be neglected. On hydrodynamic time scales (large as compared with D_R⁻¹), we can neglect the left-hand side of Eq. 16 and eliminate δQ_∥∥ from Eq. 15 in terms of density fluctuations to obtain ∂_tδc̃ = ikv₀δP_∥ − D(1−V_actc₀)k²δc̃, with V_act ∼ ℓᾱ(ℓPe) the active volume of a swimmer. When there is at least one neighbor within the active volume of a given swimmer or shaker, i.e., V_actc₀ > 1, activity exceeds diffusion and density fluctuations grow without bound. This excess defines a critical concentration c_pull ∼ (ℓ²ᾱPe)⁻¹ ∼ 1/|f| above which a homogeneous isotropic suspension of pullers (swimmers or shakers) is unstable. For swimmers, the coupling of density to longitudinal polarization turns the unstable mode into one that propagates at the self-propulsion velocity, as expected from the fact that in these systems the isotropic state can support sound-like density waves (7). This instability has been discussed before for mixtures of cytoskeletal filaments and motor proteins (17, 24), where it was referred to as a “bundling” instability, because in one dimension it drives the formation of dense bundles of filaments. The instability arises from the suppression of the longitudinal diffusion constant of anisotropic particles due to activity. For cytoskeletal filaments, the suppression arises from short-range interaction because of active cross-linkers (17, 24). For swimmers in a fluid, this suppression originates from long-range hydrodynamic interactions, which are attractive at the head and tail of contractile swimmers as illustrated in Fig. 2. This suppression is absent in pushers, as in this case the hydrodynamic interaction enhances longitudinal diffusion.

For pushers, such as E.coli, the dynamics are controlled by splay fluctuations (Eq. 17). Because f > 0, ᾱ₂ > 0, splay fluctuations are unstable on all scales for ᾱ₂/4D_R > 1 and c₀ > c_push ∼ (ℓ²ᾱPe)⁻¹. The instability that occurs in pushers is scale-free, i.e., orientational correlations build up on all scales. This instability has been identified in ref. 28, and is responsible for the enhanced orientational correlations observed in simulations (29). Our analysis shows that this instability arises from the nematic components of the hydrodynamic torques.

States with Orientational Order.

In this section, we seek to characterize the nature of fluctuations in an ordered suspension, leaving aside the conditions under which such a homogeneous ordered state may exist. For compactness, we will discuss in a unified manner the stability of both polar and nematic states. In both cases, orientational order is characterized by a finite value of the magnitude of the orientational order parameter, with P = Pn̂ in a polar state and Q_ij = S(n̂_in̂_j − $\frac{1}{3}$δ_ij) in a nematic state and n̂ the director, a unit vector denoting the direction of broken symmetry. The homogeneous nematic state is symmetric for n → −n, whereas the polar state is not. We neglect fluctuations in the magnitude of the order parameters and only consider fluctuations in the director by letting n̂ = n₀ + δn, with δn · n₀ = 0. The nonlocality of the hydrodynamic interactions enters in an essential way in the continuum equations, which are more transparent when written in Fourier space. By letting k = n₀k_∥ + k_⊥, with k_⊥·n₀ = 0, we obtain§

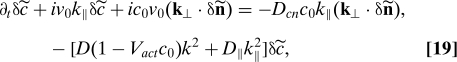where all the diffusion constants scale as k_BT/ζ̄ in the low-density limit and acquire corrections of order V_actc₀ when interactions among swimmers are included. An analysis of Eqs. 19 and 20 yields several results, enumerated below.

Generic instability.

All terms on the second line of Eq. 20 are of order k⁰. They arise from long-range hydrodynamic flows and always destabilize the homogeneous state. A homogeneous ordered state of pullers (ᾱ₂ < 0) is destabilized by the growth of splay fluctuations in the director, whereas a homogeneous ordered state of pushers (ᾱ₂ > 0) is destabilized by bend fluctuations, as predicted in ref. 12 on the basis of a phenomenological theory. The present formulation allows us to identify the origin of this instability in the nematic part of the hydrodynamic torque. The instability is “generic” and occurs on all scales, precisely because of the long-range form of the hydrodynamic interactions.

Suppressing the generic instability.

An important outcome of our formulation is that it allows us to identify the physical mechanisms that can suppress the generic instability. The instability will be eliminated by any physical mechanism capable of cutting off or screening the 1/r² decay of the flow field induced by active swimmers. Possible mechanisms include an elastic or viscoelastic component of the response of the medium and the presence of boundaries. Also, on length scales long as compared with the screening length induced by a dissipative medium, the hydrodynamic equations obtained here reduce to those for self-propelled particles on an inert substrate (32–34) that do not exhibit the generic instability. Finally, it has been noted that for swimmers that move via a stroke invariant under the combined operations of time-reversal and parity, the dipole order term in the induced flow, responsible for the 1/r² decay, vanishes (11). A collection of such swimmers will not exhibit the generic instability.

Density instabilities.

The same physics that lead to the unstable growth of density or orientational fluctuations in the isotropic state is present in ordered states, but is preempted by the generic instability.

Discussion

Our work provides a unified treatment of the physics of active suspensions. We start with a microscopic model that encompasses as special cases swimmers, shakers, pullers, and pushers and derive the coarse-grained hydrodynamics of the system. Fluctuations play a crucial role in active suspensions as they often make the putative homogeneous states unstable. The role and nature of fluctuations is summarized in the “phase diagram” of Fig. 3. At low densities, a homogeneous isotropic state of either pushers or pullers (both swimmers and shakers) is unstable above a threshold value of the active force f. The nature of the instability is different for pushers (unstable growth of large-scale density fluctuations) and pullers (unstable growth of orientational fluctuations on all scales). The interplay between instabilities driven by orientational fluctuations and density fluctuations should lead to qualitatively different patterns in suspensions of pullers and pushers and provides a rich parameter space yet to be explored. Finally, the decay of fluctuations in the concentration of swimmers (v₀≠0) in the isotropic state is controlled by propagating (as opposed to diffusive) sound-like waves above a threshold value of |f|, as predicted in ref. 7 for active particles on a substrate. The threshold value of |f| is independent of density and is solely determined by the competition between self-propulsion and diffusion. The high-density ordered states of bacterial suspensions are always unstable.

Some of the phenomena described here have been predicted by other authors with different starting points. One important result of our work is that all instabilities of active systems can be understood in a unified manner as arising from the long-ranged nature of the hydrodynamic interactions. Such instabilities are suppressed by any mechanism capable of truncating the long-range interactions. This suppression occurs, for instance, when the swimmer has a higher symmetry (e.g., a stroke that is invariant under the combined operations of time reversal and parity, such as that of the organism Spirullum Volutans), or when the medium is elastic on short time scales. In such cases, however, short-range physical interactions may provide other mechanisms with which to destabilize the homogeneous states and drive pattern formation (7, 24).

Our work is universal in that we have mapped out the consequences of the active hydrodynamic interactions on the properties of suspensions of swimmers and shakers. Any additional intrinsic interaction in these systems is short-ranged and can be included additively to the theory developed here. The exotic fluctuations characterized here will lead to pattern formation when boundaries are taken into account. For instance, the interplay between self-propulsion and diffusion that leads to propagating modes in swimmers can be shown to lead to polarization oscillations in a confined system (35). Hence, a rich parameter space remains to be explored to characterize and classify pattern formation in active systems and the relevance of such patterns to questions of biological importance. Our work constitutes an initial in this direction.

Finally, our formulation is not straightforwardly generalized to an active suspension in an externally imposed flow, u₀. To treat this case, the Stokes equation must be solved subject to the boundary condition u = u₀ far from the swimmers, which will give a modification of the Oseen tensor and hence alter the hydrodynamic interactions. In the presence of an externally imposed flow, it may be simpler to directly coarse-grain the Stokes equation (see SI Appendix) and obtain hydrodynamic equations of the form given in ref. 12 that explicitly couple to the fluid flow.