Twisting the Phenyls in Aryl Diphosphenes (Ar-P=P-Ar). Significant Impact upon Lowest Energy Excited States

Abstract

Aryl diphosphenes (Ar-P=P-Ar) possess features that may make them useful in photonic devices, including the possibility for photochemical E-Z isomerization. Development of good models guided by computations is hampered by poor correspondence between predicted and experimental UV/vis absorption spectra. An hypothesis that the phenyl twist angle (i.e. PPCC torsion) accounts for this discrepancy is explored, with positive findings. DFT and TDDFT (B3LYP) were applied to the phenyl-P=P-phenyl (Ph-P=P-Ph) model compound over a range of phenyl twist angles, and to the Ph-P=P-Ph cores of two crystallographically characterized diphosphenes: bis-(2,4,6-tBu₃C₆H₂)-diphosphene (Mes*-P=P-Mes*) and bis-(2,6-Mes₂C₆H₃)-diphosphene (Dmp-P=P-Dmp). A shallow PES is observed: the full range of phenyl twist angles is accessible for under 5 kcal/mol. The Kohn-Sham orbitals (KS-MOs) exhibit stabilization and mixing of the two highest energy frontier orbitals – the n₊ and π localized primarily on the – P=P– unit. A simple, single-configuration model based upon this symmetry-breaking is shown to be consistent with the major features of the measured UV/vis spectra of several diphosphenes. Detailed evaluation of singlet excitations, transition energies and oscillator strengths with TDDFT showed that the lowest energy transition (S₁ ← S₀) does not always correspond to the LUMO ← HOMO configuration. Coupling between the phenyl rings and central –P=P– destabilizes the π-π* dominated state. Hence, the S₁ is always n₊-π* in nature, even with a π-type HOMO. This coupling of the ring and –P=P– π systems engenders complexity in the UV/vis absorption region, and may be the origin of the variety of photobehaviours observed in diphosphenes.

Introduction

Since 1981, when Yoshifuji reported the first stable diphosphene (Mes*-P=P-Mes*)¹, a great many compounds with multiple bonds between heavy main group atoms have been described. Exploration of the synthesis, structures, coordination chemistry and reactivity of diphosphenes and related compounds is relatively advanced.^2–8 Less thoroughly investigated is the potential of diphosphenes for use as photoactive elements that is conferred upon them by their relationship to their chemical cousins azobenzene and stilbene.

The capability of photoactive molecules to impart functionality to materials has expanded greatly over the past 30 years or so. Molecular switches, memory elements, capacitors, sensors, modulators of liquid crystal optical properties and other photon-driven activities have been explored with success.^9–15 The patent literature abounds with hundreds of photoisomerization-based ideas, from portable body warmers¹⁶ to solar energy collectors and storage elements.¹⁷ Development of these technologies is still in the discovery phase, however, and diphosphenes (-P=P-) offer promising properties that are complementary to, or perhaps even superior to, those of their (-C=C-) and (-N=N-) counterparts.^18–23

A natural first step towards exploring this promise is the computational characterization of diphosphene electronic excited states. Several computational studies have been reported (vide infra), but have not revealed a consistent picture. In particular, the energetic ordering of the occupied frontier orbitals varies, and does not always reflect the experimental UV/vis absorption spectra. Careful examination of these published reports suggested to us that a deeper understanding of the impact of the phenyl twist angle (τ₁ and τ₂, Figure 1) upon the electronic behaviour in diphosphenes might shed light on these issues and foster further development of a useful framework within which to interpret ongoing and future photochemical and photophysical research.

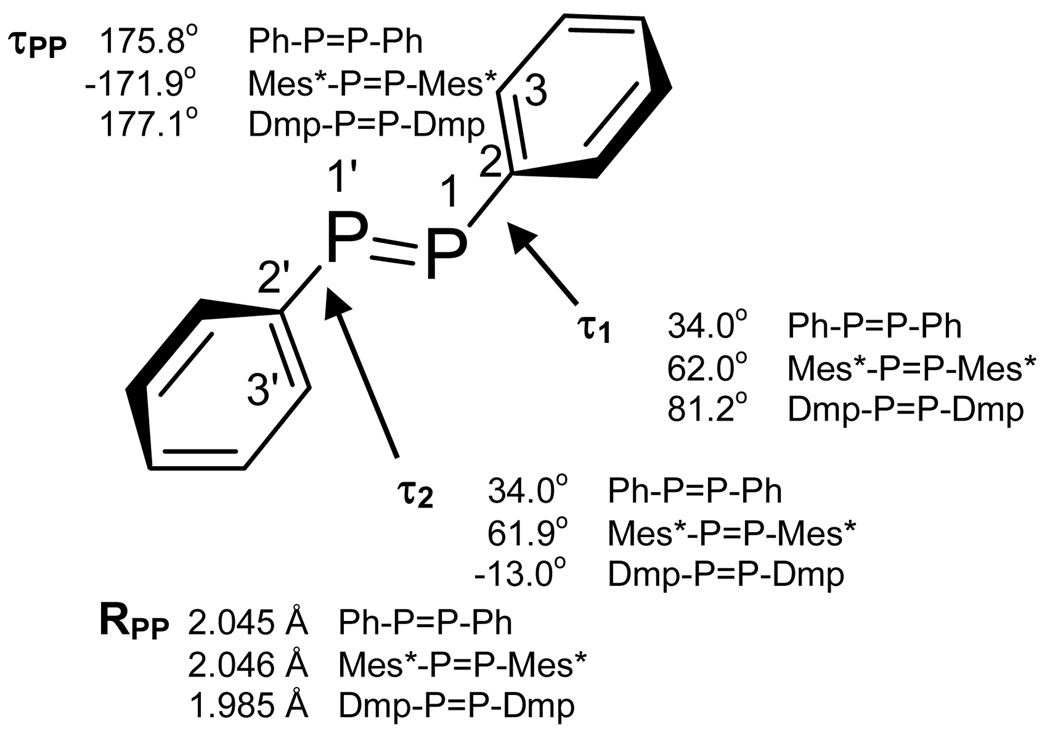
Figure 1.

Important geometric parameters of trans-diphenyl diphosphene (Ph-P=P-Ph) optimized at the B3LYP/6–311+G(2df,2p) level (Table S2). Comparisons to the crystal structures of Mes*-P=P-Mes* (reference ²⁴) and Dmp-P=P-Dmp (reference ²⁵) are provided as well. τ₁ (torsion angle 3-2-1-1') and τ₂ (torsion angle 3'-2'-1'-1) are the phenyl twist angles relative to the –P=P– core unit, that is itself nearly planar (τ_PP = torsion angle 2'-1'-1-2). R_pp is the P=P bond length. For comparison to results from optimization at the B3LYP/6–31+G(d,p), see Supplemental Information.

Here, we report upon a thorough examination of the impact of varying phenyl-twist angles on diphosphene ground and excited states using DFT and TDDFT upon the bis-phenyl-diphosphene model compound (Ph-P=P-Ph; Figure 1). Constrained geometry optimizations were used to survey the Kohn-Sham molecular orbital (KS-MO) densities and energies versus phenyl twist angles. The potential energy surface (PES) along these degrees of freedom was probed as well, to determine the ease with which diphosphenes might undergo such distortions. The central Ph-P=P-Ph cores of the crystal structures of bis-(2,4,6-tBu₃(C₆H₂))-diphosphene (Mes*-P=P-Mes*)^{1, 24} and bis-(2,6-Mes₂C₆H₃)-diphosphene (Dmp-P=P-Dmp)²⁵ were treated as well, to ground the studies in experimental behaviour.

UV/vis absorption spectra provide experimental access to electronic excited states and their energies, and insight into the participation of molecular orbitals in these photoactive states. Computational findings were therefore compared to the positions and relative intensities of the S₁ ← S₀ and S₂ ← S₀ transitions of several diphosphenes. A simple, frontier orbital model for diphosphene photobehaviour was developed and its limitations explored. The model employs a pair of single excitations, localized mainly to the phosphorus-phosphorus unit. Such orbital-based models can have considerable value by enabling predictions of electronic absorption energies and relative intensities based upon relatively simple structural ideas.

While this simple model is consistent with many experimental findings, it has significant shortcomings. TDDFT demonstrates that the phenyl rings participate actively in modulating the UV/vis transitions, sometimes beyond what could reasonably be termed a perturbation. In fact, diphosphenes exhibit a relatively uncommon effect: the order and character of the HOMO and HOMO-1 do not translate with fidelity into the dominant descriptions of S₁ and S₂, respectively. Results are interpreted in terms of the impact of phenyl twisting upon diphosphene photochemistry and photophysics.

Computational Details

All computations were performed using Gaussian03²⁶ on a PC platform. Full and constrained geometry optimizations were carried out using the B3LYP hybrid functional.^27–29 The 6-31G(d,p) basis³⁰ was utilized to calculate the potential energy surface of Ph-P=P-Ph along the τ₁ and τ₂ coordinates. A diffuse function was added (6–31+G(d,p)) for geometry optimizations at a few selected points along the diagonal τ₁ = τ₂. This level of theory was chosen as a reasonable balance between computational expense and accuracy. Calculations with a larger, more flexible basis set (6–311+G(2df,2p)) and tight optimization convergence limits showed only small (<10%) differences in both the ground and excited state findings. Results from the larger basis set are reported here, where available; a detailed comparison with the 6–31+G(d,p) basis set findings is provided in the Supporting Information.

This study focuses upon ground state structures and energies and vertical transitions to lower-lying excited states, with no chemical reactivity (i.e. bond breaking or forming), no intermolecular interactions, and no involvement of transition metals. Hence the B3LYP hybrid functional, by far the most widely used functional over the last several years³¹, is appropriate and this level of theory performs well for these target outcomes in main-group chemical systems of similar sizes and complexity.^31–33 Further, the B3LYP functional and a moderate basis set is generally sufficient for obtaining reliable TDDFT results, as long as the excitations remain well below the ionization threshold, as is the case here.³⁴ Finally, this report also treats the KS-MOs on par with ab initio molecular orbitals, as accurate reflections of the electronic behaviour of the system.³⁵

At the minimum energy structure determined by an unconstrained geometry optimization, no negative vibrational frequencies were found. With symmetry was enabled, C₂ symmetry was found for all structures except the τ₁ = τ₂ = 0° point, which was found to be in the C_2h point group. Parallel computations without symmetry constraints confirmed that the results were not distorted by exploiting the symmetry of the system. Pruned Ph-P=P-Ph versions of the experimental crystal structures of Dmp-P=P-Dmp^{25, 36} and Mes*-P=P-Mes* ²⁴ were created by removing the ligand atoms down to the base phenyl rings and substituting hydrogens. The C-H bonds were allowed to relax to minimum energy positions, and the ground state KS-MOs and their energies were calculated at the B3LYP/6–31+G(d,p) level. Transition energies and excited states were calculated upon Ph-P=P-Ph geometries and upon the Mes*-P=P-Mes* and Dmp-P=P-Dmp core geometries using TDDFT/B3LYP/6–31G+(d,p)). The steric impact of the ligand bulk upon the phenyl twist barriers was estimated with a series of ground state calculations of bis-(2,6-tBu₂(C₆H₂) diphosphene along the τ₁ = τ₂ diagonal of the PES. Molecular orbital images were generated by GaussView3.0 on a PC platform.³⁷ Fitting of KS-MO energies to y = a + b cos²(τ) was performed using Origin 7.5™.

Results and Discussion

This study began with the geometry optimization of a model compound, Ph-P=P-Ph, at a relatively high level of density functional theory (B3LYP/6–31+G(d,p)). Many experimental diphosphenes attach phenyl-based bulky groups to the phosphorus atoms to kinetically stabilize the –P=P– double bond. Hence Ph-P=P-Ph was chosen to appropriately balance computational expense and authentic molecular features such as steric repulsions and π-interactions. The surprising result was that the order of the two highest energy occupied orbitals was not consistent with experiment, leading to inaccurate predictions of UV/vis absorption and photochemical behaviour.

At the B3LYP/6–311+G(2df,2p) level of theory, the minimum energy structure of trans-Ph-P=P-Ph does not have planar C_2h symmetry, in contrast to the analogous trans-Ph-C=C-Ph and trans-Ph-N=N-Ph species. Although the central –P=P– unit is nearly planar (τ_PP = 175.8°), the phenyl rings are twisted by 34° (Figure 1), presumably to relieve repulsions between the relatively large third-shell phosphorus lone pairs and phenyl ortho-hydrogens. These steric repulsions are further exacerbated by the small C-P=P angle (102.3°), that arises from the less effective sp² hybridization that is observed in heavier main group systems.^{5, 38}

A very recent study using a complete active space self consistent field (CASSCF) approach is in agreement with these findings as well.³⁹ The minimum energy geometry on S₀ was found to have a phenyl torsion angle of 30.3°, quite similar to the B3LYP/6–311+G(2df,2p) and B3LYP/6–31+G(d,p) results reported here.⁴⁰

In experimentally characterized diphosphenes, the use of bulky groups to protect the reactive –P=P– bond leads to a wide range of phenyl twist angles (Table 2). This observation suggests that phenyl twisting provides an energetically thrifty path for the molecule to relieve steric stresses.

Table 2.

Electronic Transition Energies and Strengths of Some Real Diphosphenes.^a

Compoundb	τ1, τ2	UV-Vis Absorption			Ref.
		π-π*	n+π*	intensity ratio
Tbt-P=P-Tbt	52.8,	405 (13000)	530 (2000)	0.15	78
	60.0
Dmop-P=P-Dmopc	61.3,	346 (5620)	477 (1320)	0.23	79, 80
	58.6	347 (5500)	478 (1260)	0.23
Mes*-P=P-Mes*	61.5	340 (7690)	460 (1360)	0.18	1
Dmp-P=P-Dmp	81.2,	372 (6190)	456 (365)	0.059	25
	13.0
Bbt-P=P-Bbt	86.2,	428 (12000)	532 (1000)	0.083	78
	28.4
Dcp-P=P-Dcp	88.7	352 (8551)	448 (626)	0.073	81
Btfmp-P=P-Btfmp	91.8	277 (11640)	394 (197)	0.017	82, 83

^aτ₁ and τ₂ phenyl torsion angles defined in Figure 1, in degrees; λ in nanometers;ε in M⁻¹ cm⁻¹.

^bTbt = 2,4,6-tris[bis(trimethylsilyl)methyl]phenyl; Dmop = 2,6-di-tert-butyl-4- methoxyphenyl; Mes* = 2,4,6-tri-tert-butylphenyl; Bbt = 2,6-bis[bis(trimethylsilyl)- methyl]-4-[tris(trimethylsilyl)methyl]phenyl; Dcp = 2,6-(2,6-Cl₂C₆H₃)C₆H₃; Btfmp = 2,6- bis(trifluoromethyl)phenyl.

^cCH₂Cl₂ (upper), hexane (lower).

Energetic Cost of Phenyl Twisting

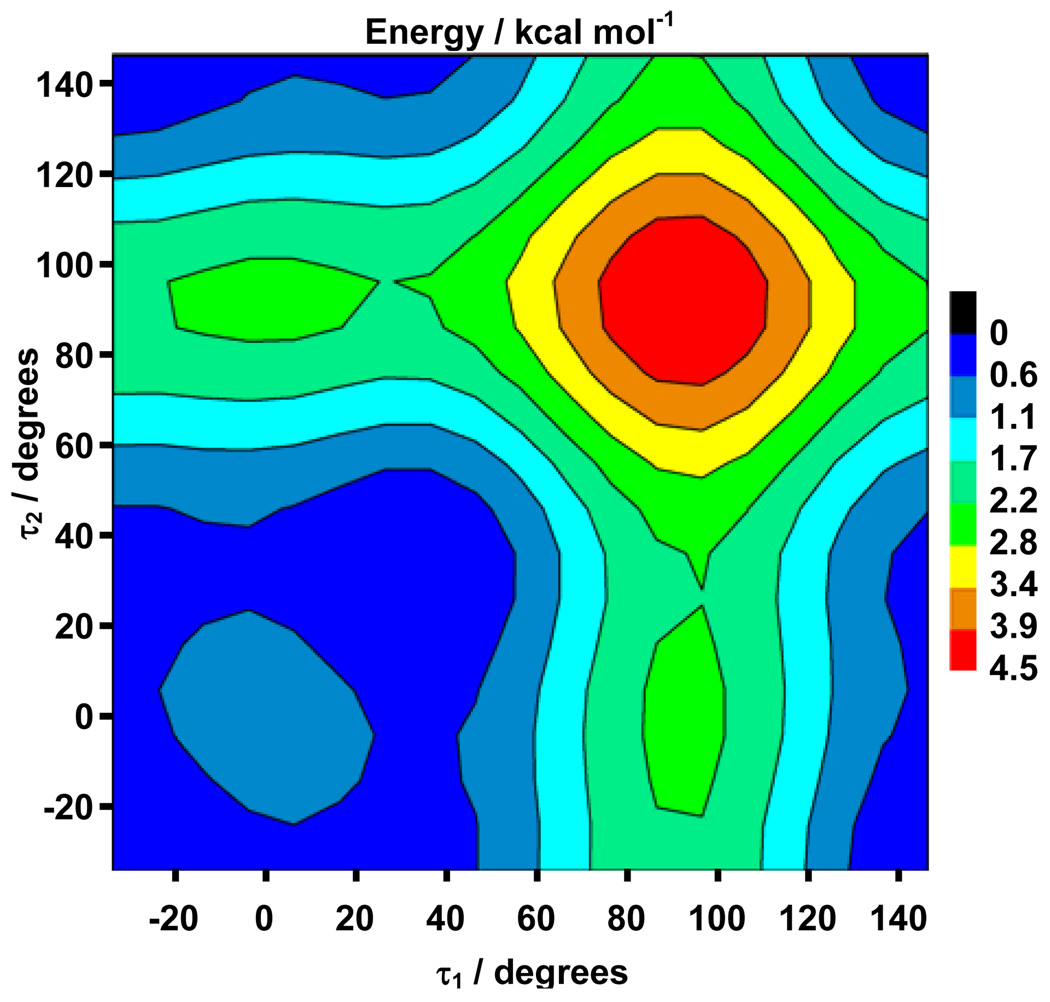
To evaluate the energetic expense of phenyl twisting, the ground state PES (361 points) was computed along τ₁ and τ₂ (B3LYP/6-31G(d,p)) (Figure 2). The shallow surface illustrates the ease with which the system can distort along these coordinates to accommodate substituents. A significantly larger, more flexible basis set (6–311+G(2df,2p) basis set was employed to examine the diagonal (τ₁ = τ₂) of the PES with higher fidelity. The energy differences between the minimum at 34° and the maxima at 0° and 90° are 0.0246 eV (0.567 kcal mol⁻¹) and 0.162 eV (3.74 kcal mol⁻¹), respectively. This energy barrier at τ = 90° is slightly greater than that found by Y. Amatatsu using a multi-reference ab initio method.^39,41 The entire range of phenyl twist angles is available for less than 4 kcal mol⁻¹, and structures from 0° to about 50° are accessible at room temperature (within ~250 cm⁻¹ of the minimum).³⁷

Figure 2.

Ground state potential energy surface along the τ₁ and τ₂ phenyl twisting coordinates. B3LYP/6-31G(d,p) level for Ph-P=P-Ph. The energy was calculated every 10° from −34° to 146° (361 points). All other geometric parameters were allowed to optimize. Energy is in kcal/mol, and is relative to the minimum.

This low barrier to phenyl twisting in Ph-P=P-Ph is significantly smaller than what is observed for azobenzene and stilbene. At the same level of theory, these compounds exhibit planar minimum energy structures and larger barriers to phenyl rotation of 0.479 eV (11.0 kcal mol⁻¹) and 0.381 eV (8.79 kcal mol⁻¹), respectively. Evidently, π-delocalization is weaker in diphosphene than in either of its lighter element cousins. That there is some conjugation in the Ph-P=P-Ph system is apparent in the increase in the P-C bond length from 1.831 to 1.847 Å as the phenyl rings are twisted from 0° to 90°. Experimental evidence for π-delocalization can be seen in an elegant study by Petsana and Power of a set of diarylboryl-substituted diphosphanes.^{42, 43} In these molecules, the P-atoms are bonded through only the σ-component of the double bond (i.e. the sp² hybrid P-P σ-bond in the absence of P=P π-bonding) and the P-C bonds are a few hundredths of an angstrom longer than the corresponding bonds in Mes*-P=P-Mes* and Dmp-P=P-Dmp. The shorter P-C bonds in the latter molecules supports the presence of π-delocalization across the Ph-P=P-Ph architecture.

In aryl-diphosphenes, π-delocalization appears strong enough to shorten P-ligand bonds and create a moderate barrier to phenyl ring twisting, but not strong enough to induce coplanarity among the phenyl rings and the central C-P=P-C unit. This begs the question: why is conjugation across the molecule less effective in diphosphene than in stilbene and azobenzene? Probably the most important factor is the relative sizes of the atomic p-orbitals involved. The <r> for the phosphorus 3p orbital is about 40 – 50% larger than that for the carbon 2p orbital³⁸, significantly limiting the magnitude of their overlap integral. The reduced π-overlap is probably compounded by the inefficient sp² hybridization at the phosphorus. In general, hybridization is less important for larger main group elements than for cognate lighter systems.^{2, 5, 38, 44} In diphosphenes, this is reflected in larger s-character for the lone-pair electrons and C-P=P bond angles that are closer to 90° than to 120°. The impact of the relatively poor π-overlap can be seen in estimates of σ and π contributions to homonuclear and heteronuclear double bonds among C, N, and P. The π-increment⁴⁵ for the CC bond is ~70 kcal/mol, and is similar in magnitude to that for NC (~65 – 86 kcal/mol); both are significantly larger than for PC (~45–50 kcal/mol).^{38, 46, 47} By extension, delocalized π-bonding across the Ar-P=P-Ar molecule might be relatively inefficient. In combination with the large extent of the phosphorus lone pairs and smaller ligand-E=E angle, this weaker conjugation induces diphenyl diphosphene to inhabit a more twisted region of the PES than do azobenzene and stilbene. The bulky ligands required to stabilize diphosphenes magnifies this tendency by increasing the barrier to phenyl rotation at τ₁ = τ₂ = 0° (vide infra).

Phenyl Twists, Frontier Orbitals, and the UV/vis Spectrum. A Simple Model

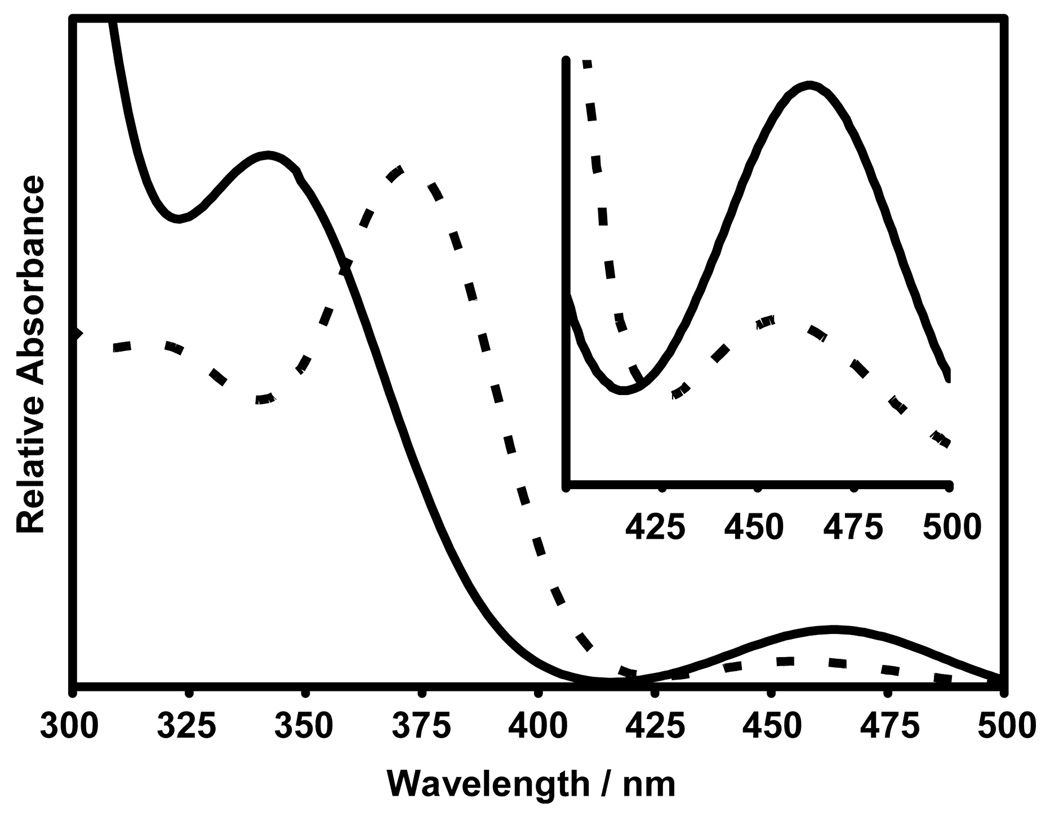
The shallow PES along the phenyl twists is noteworthy because of the marked effect this distortion has upon the frontier molecular orbitals. These can be sensitive controllers of the UV/vis spectrum and photoreactivity. Diphosphenes typically exhibit two major UV/vis absorptions (Figure 3), both assigned primarily to the P=P group. An intense (ε_max ~10,000 cm⁻¹M⁻¹) transition in the 330 – 350 nm region is attributed to a π-π* excitation; a much less intense (ε_max ~1,000 cm⁻¹M⁻¹) band at about 460 nm is assigned to the symmetry-forbidden n₊-π* transition.

Figure 3.

UV-Vis Absorption spectra of Mes*-P=P-Mes* (solid line; ε₃₄₀ = 7.69 × 10³ M⁻¹ cm⁻¹ and ε₄₆₀ = 1.36 × 10³ M⁻¹ cm⁻¹ in CH₂Cl₂) and Dmp-P=P-Dmp (dotted line; ε₃₇₂ = 6.19 × 10³ M⁻¹ cm⁻¹ and ε₄₅₆ = 365 M⁻¹ cm⁻¹ in heptane).

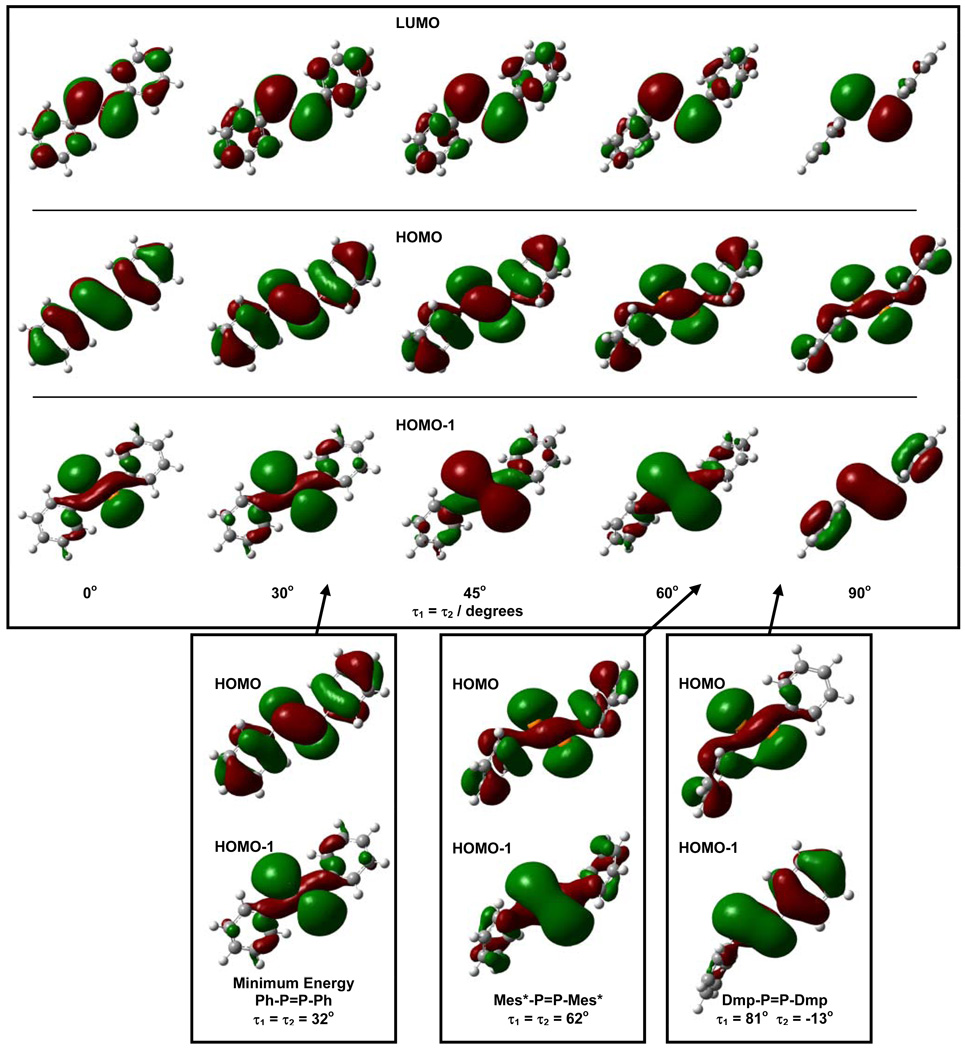
As mentioned above, the frontier orbitals of the calculated minimum energy trans-Ph-P=P-Ph (τ₁ = τ₂ = 34°) are in conflict with experimental observations: the HOMO is calculated to be largely π, the HOMO-1 is largely n₊ and the LUMO is anti-bonding π* (Figure 4). These results predict that the π-π* and n₊-π* UV/vis transitions should be reversed from the observed order. Further, these results conflict with previous DFT calculations on the crystal structure of Mes*-P=P-Mes*²⁴ and on Ph-P=P-Ph with the phenyls fixed to the twist angle (τ₁ = τ₂ = 64°) near that of the Mes*-P=P-Mes* crystal structure, both of which assigned the HOMO to be an n₊ orbital.^48,49

Figure 4.

Upper Panel: Frontier Kohn-Sham orbitals from geometry optimizations constrained along the τ₁ = τ₂ diagonal of the ground state potential energy surface. Lower Panel: Highest occupied Kohn-Sham orbitals from (left to right) unconstrained geometry optimized Ph-P=P-Ph, Ph-P=P-Ph core of the crystal structure of Mes*-P=P-Mes*, and Ph-P=P-Ph core of the crystal structure of Dmp-P=P-Dmp. All calculations performed at the B3LYP/6–31+G(d,p) level.

Several previous computational studies, mostly on H-P=P-H, also predicted that the HOMO should be a π orbital. These included several Hartree-Fock self consistent field (HF-SCF) studies ^50–52 and one that employed CASSCF.⁵³ Others indicated that the HOMO was an n₊ orbital ^54–57, and used this information to definitively assign the Mes*-P=P-Mes* photoelectron spectrum, with the n₊ as the HOMO by Koopmans’ Theorem. Allen and coworkers resolved the issue for H-P=P-H in an elegant and thorough computational study, and determined that correlation is required to obtain the appropriate orbital ordering.⁵⁸ Later, B3LYP/6-311G(d,p) calculations on H-P=P-H also found the HOMO to be n₊.⁴⁸ In light of all of these findings, it was concluded that the level of theoretical treatment employed in our B3LYP studies on Ph-P=PPh should be sufficient to accurately predict the order of frontier orbitals, though they are energetically close. A structural origin for the discrepancy was proposed, in line with a suggestion by Miqueau et al.⁴⁸

To test this hypothesis, the energies and orbitals were calculated for Ph-P=P-Ph as a function of phenyl twist angle. The KS-MOs for Ph-P=P=Ph as they are systematically distorted along the τ₁ = τ₂ diagonal of the PES are shown in Figure 4 (B3LYP/6-31+G(d,p)). For the constrained planar Ph-P=P=Ph, the two highest occupied orbitals are very clearly π (HOMO) and n₊ (HOMO-1). As expected from the C_2h symmetry, these π (a_u) and n₊ (a_g) orbitals are pure and orthogonal. Twisting about the phenyl rings, however, reduces them to the same symmetry (a) and mixing occurs. Figure 4 (upper panel) shows this progressive, structurally-induced orbital transformation.

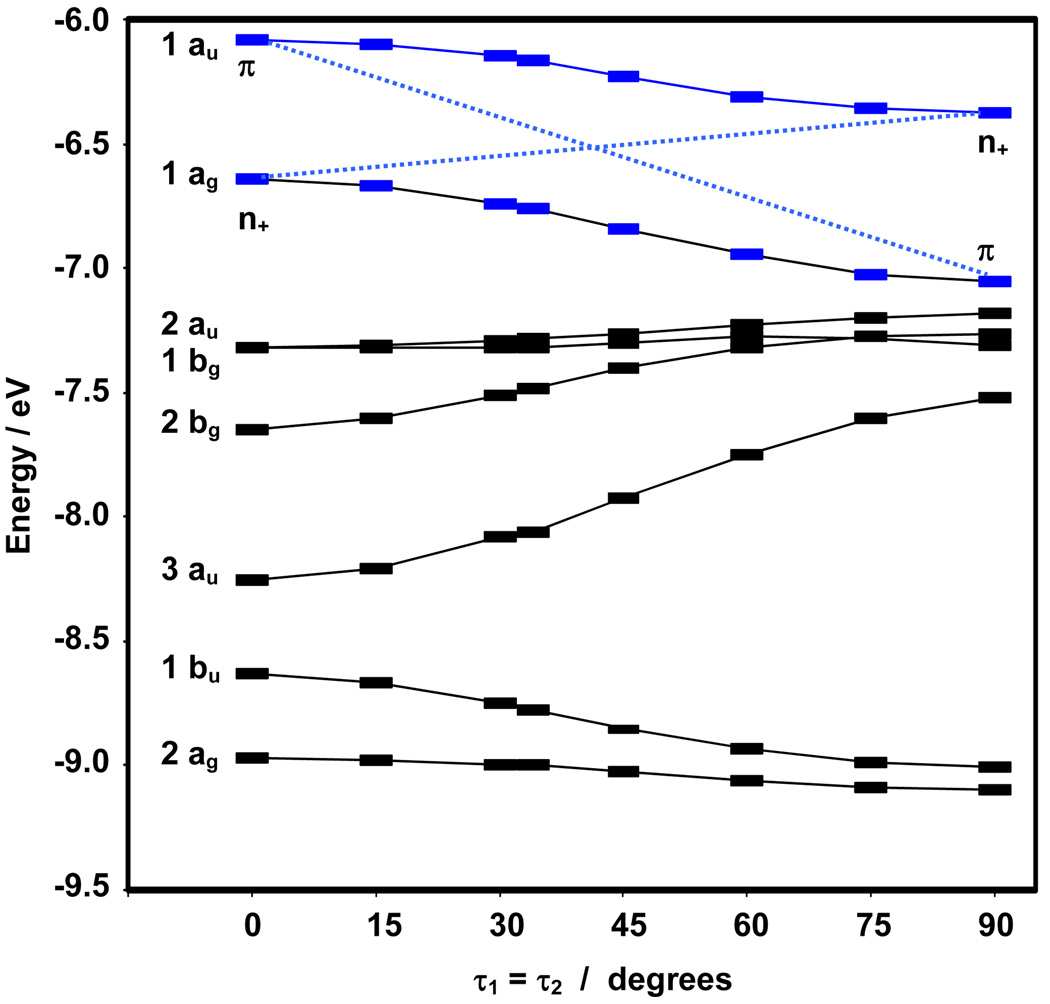
The energies of the occupied frontier orbitals depend upon the phenyl twist angle (Figure 5; B3LYP/6–311+G(2df,2p)). The HOMO and HOMO-1 are separated by 0.56 eV in the planar molecule, in good agreement with Allen et al.⁵⁸, and are stabilized roughly in parallel across the range of τ₁ = τ₂ angles. The stabilization fits a cos²τ (τ = τ₁ = τ₂) dependence (r² > 0.99), indicating that π-π overlap between the ring and central -P=P- is the prevailing influencing factor.

Figure 5.

Energies of the eight highest energy occupied Kohn Sham molecular orbitals (B3LYP/6–311+G(2df,2p)) of Ph-P=P-Ph at a series of τ₁ = τ₂ phenyl twist angles. Symmetry designations derive from the constrained planar conformation (τ₁ = τ₂ = 0°). The two frontier orbitals in the simple model, single-configuration model are in blue. For comparison to results from optimization at the B3LYP/6–31+G(d,p), see Supplemental Information.

Examination of the endpoints (τ = 0° and τ = 90°) for the HOMO and HOMO-1 in Figure 5 shows a net stabilization (~1 eV) of the π-character, and a significantly smaller destabilization of n₊. In contrast, the π* LUMO shape and energy remains largely constant with phenyl twist angle; it changes by less than 0.12 eV over the entire phenyl twist range (Table S2). Given the nodes between the –P=P– and the phenyl rings in this orbital, this insensitivity is entirely consistent with a π-overlap dependence.

It is worthwhile to consider a simple model in which the HOMO, HOMO-1, and π* LUMO determine the major features of the UV/vis spectroscopic and photochemical behaviour. Such frontier orbital models have shown excellent results in many organic photoactive systems, particularly by providing significant qualitative, physical insight into the photophysics and photochemical reactivity. First, we ascribe the dominant phenyl twist impact to the ground state, and mix the two highest-energy, occupied, frontier orbitals according to cos²(τ). Then, the UV/vis absorption spectrum is described by single electronic configurations: S₁ thus corresponds cleanly to LUMO←HOMO and S₂ corresponds to LUMO←HOMO-1. Several qualitative predictions can be made with this model:

1. Both S₁ ← S₀ and S₂ ← S₀ transitions will appear in the UV/vis absorption spectrum, except when τ₁ = τ₂ = 0° or 90°. For these geometries, one transition would correspond to pure π* ← n₊ and be symmetry forbidden. At all other angles, both excitations will have some π* ← π character, the magnitude of which would be reflected in the bands’ relative intensities. The intensities should be most similar when τ₁ = τ₂ = 45°.

2. The order of the UV/vis absorption bands will depend upon the phenyl twist angle. For τ₁ = τ₂ > 45°, the more intense band will arise at higher energy with a less intense one to the red; for τ₁ = τ₂ < 45°, the more intense transition will occur at lower energy.

3. As the phenyl twist increases, the HOMO and HOMO-1 are stabilized while the LUMO energy is unaffected; hence both bands should blue-shift as the phenyl twist increases.

Comparisons to Experimental Data

The first two predictions from the simple model above are largely borne out in experimental findings. The HOMO and HOMO-1 derived from single-point calculations of the core structures from crystal data of the diphosphenes Mes*-P=P-Mes* and Dmp-P=P-Dmp are shown in Figure 4 (lower panel). Both show a HOMO dominated by n₊ character. The experimental S₂ ← S₀ transition of Mes*-P=P-Mes* is at 340 nm (ε₃₄₀ = 7.69 × 10³ M⁻¹ cm⁻¹) and that of Dmp-P=P-Dmp is at 372 nm (ε₃₇₂ = 6.19 × 10³ M⁻¹ cm⁻¹) (Table 1). The weaker band (S₁ ← S₀) appears at lower energy for both molecules, hence the order of the UV/vis absorption transitions is qualitatively consistent with the single configuration model.

Table 1.

Computed and Experimental Electronic Transition Energies and Strengths of Mes*- P=P-Mes* and Dmp-P=P-Dmp.^a

Mes*-P=P-Mes*					Dmp-P=P-Dmp
	Calc.b		Exptc		Calc.b		Expt.c
	λ	f	λ	ε	λ	f	λ	ε
n+→π*	488	0.0345	460	1360	447	0.0006	456	365
π→π*	346	0.0630	340	7690	347	0.177	372	6190

^aλ in nanometers; f in atomic units; ε in M⁻¹cm⁻¹.

^bB3LYP/6–31+G(d,p) single point calculations of Ph-P=P-Ph core of the crystal structures, as described in the text.

^cMes*-P=P-Mes* in CH₂Cl₂; Dmp-P=P-Dmp in heptane.

The relative intensities of the bands support the single configuration model as well. Mes*-P=P-Mes*, with τ₁ = τ₂ = 62°, is closer to the fully mixed 45° model conformation than is Dmp-P=P-Dmp. Hence the relative intensity of the n₊-π* band to the π-π* should be larger for Mes*-P=P-Mes*. This is, in fact, observed: the measured ratio of band intensities ε_(n+-π*) / ε_(π-π*) is 0.18 for Mes*-P=P-Mes* and 0.059 for Dmp-P=P-Dmp.

Table 2 indicates that this simple model qualitatively captures the UV/vis absorption behaviour of other diphosphenes as well. All of these diphosphenes exhibit at least one τ₁ = τ₂ greater than 45°, and the lower energy absorption is less intense for all of them. Further, when the phenyl twists are in the 50–65° range, the (S₁ ← S₀) : (S₂ ← S₀) relative intensity ratio is 0.15 – 0.25. With at least one τ > 80°, the ratio decreases substantially to 0.02 – 0.08, as predicted.

The phenyl twists of stable diphosphenes tend to be significantly larger than the 34° equilibrium value determined for diphenyl diphosphene, a molecule that has never been isolated or observed in experiments. B3LYP/6–31+G(d,p) calculations upon bis-(2,6-tBu₂(C₆H₂)) diphosphene, a model for Mes*-P=P-Mes*, along the τ₁ = τ₂ diagonal of the PES produces a minimum energy structure at τ₁ = τ₂ = 63.4°, quite close to the experimental crystal structure value for Mes*-P=P-Mes* (Supporting Information). The barriers to phenyl rotation at 0° and 90° are 1.12 eV (25.9 kcal/mol) and 0.132 eV (3.06 kcal/mol), respectively. In these calculations, the steric crowding introduced by the bulky ligands significantly narrows the range of phenyl twist angles available to the molecule at room temperature, to between about 60° and 70° (within ~250 cm⁻¹ of the minimum). Hence the molecule does exploit the soft PES of the phenyl twist degree of freedom inherent in the Ph-P=P-Ph to accommodate bulky ligands, and the greatly increased barrier at τ₁ = τ₂ = 0° appears to constrain the molecule to a relatively narrow range of the larger phenyl twist angles. This, and the agreement between the calculated and experimental values, also suggests that crystal packing forces may have a relatively minor impact upon the phenyl twist angle, and suggests that the phenyl twist angles adopted by diphosphenes in room temperature solutions may be quite similar to those observed in crystal structures, in general.

The results thus far indicate that Ph-P=P=Ph is a good model for capturing important diphosphene photobehaviour, and the B3LYP/6–31+G(d,p) level of theory is appropriate for this system. The phenyl twist indeed appears to exert significant influence over the two highest energy frontier orbitals, and thereby upon the diphosphene UV/vis absorption spectrum. Electronic transitions dominated by single configurations thus appear to explain the major features of diphosphene UV/vis spectroscopy, once the impact of the phenyl twist upon the orbitals is taken into account, though the model has some limitations (vide infra).

Phenyl Twist and Excited States. The Picture is More Complex

The third prediction from the single-configuration model – that both S₂←S₀ and S₁←S₀ should blue shift with increasing phenyl twist angle – is not borne out by experimental findings. Limitations of the single-configuration model can be illustrated by comparing Tbt-P=P-Tbt and Dmop-P=P-Dmop.⁵⁹ These two molecules have similar phenyl twist angles, but their UV/vis absorption bands differ by more than 50 nm. Evidently, and not surprisingly, the absolute band energies reflect interactions with the protecting groups beyond the influence of the phenyl twist upon the three frontier orbitals in the simple model.

To explore the UV/vis transition energies and intensities in more detail, TDDFT (B3LYP/6–31+G(d,p)) calculations were performed upon the two truncated diphosphene model structures discussed above (Table 1). For Mes*-P=P-Mes*, less than 10 nanometers separate the TDDFT calculated and observed wavelengths for the higher energy, higher intensity transition. The n₊-π* is calculated at somewhat too long a wavelength at 488 nm, but the agreement with experiment is still good. The Dmp-P=P-Dmp lower energy band is quite well reproduced, though the (S₂ ← S₀) is slightly less well so. The oscillator strengths emulate the experimental results as well, albeit in an exaggerated fashion.

The discrepancies between experiment and computation may be partly due to the electronic influence of the extended groups that are absent from the calculations. Symmetry breaking may also play a role for Dmp-P=P-Dmp, for example. However, the TDDFT descriptions of the S₁ and S₂ states point clearly to the participation of other electronic excitations in the observed UV/vis transitions, especially those involving the phenyl ring π-systems.

For both the Dmp-P=P-Dmp and Mes*-P=P-Mes* core model structures, the S₁ state contains significant contributions from LUMO←HOMO and LUMO←HOMO-1 excitations, though the former has a 4–5 fold larger coefficient. The higher energy, more intense transition is more complex. While the LUMO←HOMO-1 is the largest contributor to the S₂ state, three other excitations also are significant; these are all excitations from ring π-orbitals to the LUMO. Also, LUMO←HOMO-1 participates in other states that exhibit appreciable oscillator strengths. This latter detail could be the origin of the exaggerated relative intensity predictions: the measured π-π* transition is likely to be a composite of several bands of varying oscillator strengths.

The limitations of the simple model were explored further by applying TDDFT/B3LYP/6–311+G(2df,2p) across the entire range (0° to 90°) of phenyl twist angles (see Supporting Information for TDDFT/B3LYP/6–31+G(d,p) results). The results indicate that although the single-configuration model is qualitatively consistent with experiment, it is overly simple. The ring π systems participate rather heavily in the “P=P” transitions, and in a τ-dependent manner, to tune the energies and transition moments of the excited states.

The most surprising finding was that the n₊-π* dominated transition is always the lower energy one – even when τ₁ = τ₂ < 45°, where the HOMO is clearly a π-type orbital. In fact, even at τ = 0°, where the HOMO is clearly a π-type orbital, the S₁ state is quite unambiguously assigned to the (n₊ ¹π*¹) configuration, and S₂ to (π¹π*¹).⁶⁰

Insight can be found by examining the occupied orbitals just below the HOMO and HOMO-1 (Figure 5). ⁶¹ At τ₁ = τ₂ = 0°, the π HOMO is 1a_u and the n₊ HOMO-1 is 1a_g. Two other a_u orbitals lie within about 2 eV of 1a_u. The 2a_u π-orbital resides wholly on the phenyls, while 3a_u has large π-bonding contribution that spans the central part of the molecule. Phenyl twisting destabilizes 3a_u, as expected by its electron density. The energies of these KS-MOs converge as the phenyl twist angle increases. By τ = 90°, the HOMO is a relatively energetically isolated n₊ orbital, 0.7 eV above the HOMO-1. Next come 5 π orbitals within an energy range of 0.4 eV. Thus the extended π-system has the energy and symmetry properties favorable for participation in the S₂ ← S₀ transition.

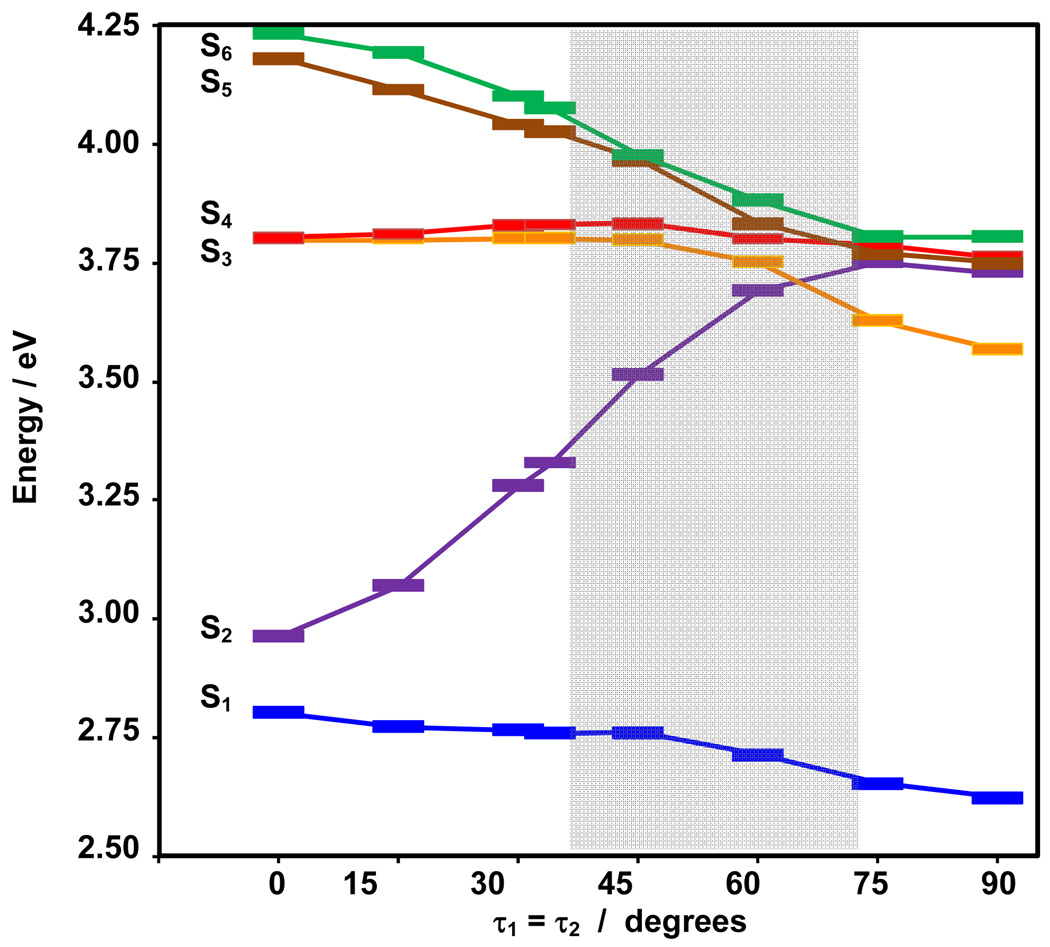
The TDDFT results confirm that the most intense band in the UV/vis absorption spectrum is not isolated to the P=P unit: by τ₁ = τ₂ = 30°, contributions from electronic excitations involving ring and ring-P π-orbitals are significant, and mixing increases with τ. In fact, as the phenyl twist increases from 60° to 90° the transitions become so mixed it becomes challenging to assign them. At 75°, for example, four singlet states with non-zero oscillator strengths are clustered within 0.06 eV of one another (Figure 6). Thus the observed band assigned to the P=P π-π* excitation is relatively complex, with multiple contributions from the ring π-system that depend sensitively upon the phenyl twist angle.

Figure 6.

Energies of the six lowest energy singlet excited states (TDDFT/B3LYP/6–311+G(2df,2p)) of Ph-P=P-Ph at a series of τ₁ = τ₂ phenyl twist angles. Excited state designations relate to the constrained planar conformation (τ₁ = τ₂ = 0°). The states corresponding to transitions traditionally assigned to n₊-π* (S₁) and π-π* (S₂) are in blue and violet, respectively. The grey area highlights the phenyl twist angles exhibited by many experimental diphosphenes. For comparison to results from optimization at the B3LYP/6–31+G(d,p), see Supplemental Information.

In contrast, S₁ remains relatively uncomplicated throughout. It contains significant contributions from only configurations involving the HOMO, HOMO-1, and LUMO throughout the range of phenyl twist angles. Presumably, it remains the lowest energy state because the mixing of the higher energy configurations with the lowest energy (π¹π*¹) destabilizes S₂. However, further study is required to fully understand this observation.

As this manuscript was being finalized, a very relevant report of ab initio calculations upon the phenyl twisting degree of freedom in Ph-P=P-Ph was published.³⁹ In those studies, CASSCF was employed to optimize the geometries of the S₀ state along this coordinate, and second-order multi-reference Moller-Plesset perturbation theory (MRMP2) energies were calculated for S₀ and S₁ at these CASSCF geometries. Although the more intense transition to S₂ was not discussed in that paper, the major features of the ab initio results agree quite well with the TDDFT B3LYP/6–311+G(2df,2p) findings for the S₁ ← S₀ excitation.

A straightforward DFT analysis has revealed important subtlety in the electronic structure of diphosphenes in just the range of phenyl twist angles that are experimentally common: 45° – 90°. While the simple, single-configuration model provides useful qualitative insight, a more detailed treatment of the coupling between –P=P– and phenyl π systems may be needed to account for details in the interaction of diphosphenes with light.

Potential Impact upon Photochemistry

The shallow nature of the PES along the two dimensional CCPP surface accounts for the broad structural diversity observed experimentally for these phenyl twist angles in diphosphenes. Concomitantly, the impact of the phenyl twist upon the energies and natures of the electronic excited states shown here may help explain the photochemical and photophysical diversity exhibited by diphosphenes.

Direct studies of diphosphene photobehaviour are relatively limited, yet the richness they reveal is considerable. Consider the molecules most thoroughly discussed here: Dmp-P=P-Dmp and Mes*-P=P-Mes*. Excitation of Mes*-P=P-Mes* in the ultraviolet induces irreversible CH bond-insertion into the Mes* group, most likely through a phosphinidene intermediate, to produce a phosphaindan product.^{62, 63} In contrast, Dmp-P=P-Dmp undergoes no irreversible photochemistry under similar conditions. Fluorescence is negligible for both at room temperature, consistent with very rapid excited state evolution. Femtosecond transient absorption confirms that the initial dynamics are sub-picosecond, however subsequent dynamics differs for the two systems.^{64, 65}

Photoisomerization from the trans to cis conformations, and back, is observed for several diphosphenes, including Mes*-P=P-Mes* under some conditions.^{63, 66–73} In some cases, the formation of a labile cyclotetraphosphane arising from the head-to-head dimerization of cis-diphosphenes is experimentally observed ^{66, 74, 75} or implied by the products formed.^{69, 73, 76} In other photochemical experiments, evidence for a transient cyclotetraphosphane is sought but not found.^{70, 72} Photoinduced bond insertion of the phosphorus into ligand C-H or C-C bonds is also observed, perhaps through a phosphinidine intermediate ^{62, 63, 69, 70}

It is unlikely that the phenyl twist is the sole variable responsible for variety observed in the photobehaviour of diphosphenes. There is ample evidence in the studies above for other influences by the bulky ligands, including electronic factors and proximity of C-C and C-H bonds to phosphorus atoms, and for temperature- and excitation wavelength-dependence in the above photoinduced reactions. However, the provocative initial computational findings reported here lead to a reasonable hypothesis that the phenyl twists may have an impact upon diphosphene photochemistry and photophysics, through moderation of the coupling between the phenyls and the central –P=P–. The flat PES may mean that diphosphenes in solution sample a broad range of the phenyl twist angles. The character of the photoexcited state(s) will affect the internal conversion and intersystem crossing events, excited state lifetimes, pathways, and product distributions. Recent reports of the importance of the phenyl ring states in critical aspects of azobenzene photochemistry provide further support for this idea.⁷⁷

Clearly, these findings are just the first step in elucidating the structure – function relationships that drive the photodynamics of diphosphenes. Further exploration of the excited state potential surfaces, and interpretation of new femtosecond transient absorption findings are underway in our group. A more rigorous study of the rotation, inversion, and dissociation pathways along the ground and excited electronic states of diphosphenes using complete active space methods will be reported separately.

Summary

DFT and TDDFT have been applied to a model diphosphene to resolve a puzzling conflict in the 25-year diphosphene computational chemistry literature. The HOMO and HOMO-1 in this system are energetically close, and while the UV-vis spectrum of all diphosphenes synthesized thus far indicates that the n₊ orbital should be the HOMO, a number of computational treatments have found otherwise. Allen et al. definitively showed that effective correlation is needed to properly order the frontier occupied orbitals in HP=PH⁵⁸. Here we report that there is a molecular structure effect operating as well, one that can affect experimental diphosphene behaviour.

In arguably the most important class of diphosphenes, Aryl-P=P-Aryl, conjugation with phenylic substituents has a significant impact upon the occupied frontier orbitals. Although the π-delocalization across the molecule is relatively weak, the near-degeneracy of the two occupied frontier molecular orbitals leads to its significant consequences. As the π-overlap between the central -P=P- unit is “tuned” by twisting the phenyl ligands, the HOMO and HOMO-1 mix and are concomitantly stabilized, resulting in a net transformation of the HOMO from π to n₊ in character, and the HOMO-1 from n₊ to π. Nonetheless, even over the range of angles where the HOMO is clearly a π-orbital, the S₁ state equally clearly arises from a primarily (n₊ ¹π*¹) excitation. Coupling between the π-systems of the -P=P- and the phenyl rings stabilizes the (π¹-π*¹) state, in a phenyl twist dependent manner.

The energy-barrier to phenyl twisting motion is quite small, and the diversity of phenyl twist angles observed experimentally indicates that many diphosphenes exploit the ease of distortion to relieve stress. As the search for new functional materials continues to intensify, understanding the photochemistry and photophysics of the heavier main group compounds becomes increasingly important. This study reveals very useful information that confirms and extends what is known about the bonding and spectroscopy of the promising diphosphene class of molecules, and helps to lay the groundwork for future studies.

Supplementary Material

1_si_001. Supporting Information Available.

References with more than 10 authors; Table S1: Selected geometric parameters and KS-MO energies for distorted Ph-P=P-Ph (B3LYP/6–31+G(d,p); Table S2: Selected geometric parameters and KS-MO energies for distorted Ph-P=P-Ph (B3LYP/6–311+G(2df,2p)); Figures S1, S2, and S3 showing comparisons of the ground state energy profile, KS-MO energies, and S₁ – S₆ excited state energies, respectively, along the τ₁ = τ₂ coordinate for the two basis sets. Figure S1 also shows the ground state energy profile along the τ₁ = τ₂ diagonal for bis-(2,6-tBu₂C₆H₂) diphosphene.