Towards extracellular Ca²⁺ sensing by MRI: synthesis and calcium-dependent ¹H and ¹⁷O relaxation studies of two novel bismacrocyclic Gd³⁺ complexes

Abstract

Two new bismacrocyclic Gd³⁺ chelates containing a specific Ca²⁺ binding site were synthesized as potential MRI contrast agents for the detection of Ca²⁺ concentration changes at the millimolar level in the extracellular space. In the ligands, the Ca²⁺-sensitive BAPTA-bisamide central part is separated from the DO3A macrocycles either by an ethylene (L¹) or by a propylene (L²) unit [H₄BAPTA is 1,2-bis(o-aminophenoxy)ethane-N,N,N′,N′-tetraacetic acid; H₃DO₃A is 1,4,7,10-tetraazacyclododecane-1,4,7-triacetic acid]. The sensitivity of the Gd³⁺ complexes towards Ca²⁺ and Mg²⁺ was studied by ¹H relaxometric titrations. A maximum relaxivity increase of 15 and 10% was observed upon Ca²⁺ binding to Gd₂L¹ and Gd₂L², respectively, with a distinct selectivity of Gd₂L¹ towards Ca²⁺ compared with Mg²⁺. For Ca²⁺ binding, association constants of log K = 1.9 (Gd₂L¹) and log K = 2.7 (Gd₂L²) were determined by relaxometry. Luminescence lifetime measurements and UV–vis spectrophotometry on the corresponding Eu³⁺ analogues proved that the complexes exist in the form of monohydrated and nonhydrated species; Ca²⁺ binding in the central part of the ligand induces the formation of the monohydrated state. The increasing hydration number accounts for the relaxivity increase observed on Ca²⁺ addition. A ¹H nuclear magnetic relaxation dispersion and ¹⁷O NMR study on Gd₂L¹ in the absence and in the presence of Ca²⁺ was performed to assess the microscopic parameters influencing relaxivity. On Ca²⁺ binding, the water exchange is slightly accelerated, which is likely related to the increased steric demand of the central part leading to a destabilization of the Ln–water binding interaction.

Electronic supplementary material

The online version of this article (doi:10.1007/s00775-007-0296-9) contains supplementary material, which is available to authorized users.

Introduction

Magnetic resonance imaging (MRI) is one of the most versatile techniques in clinical and experimental in vivo imaging. It provides excellent three-dimensional spatial resolution down to the 10-μm range, whole body coverage and the opportunity to noninvasively measure additional physiological parameters [1]. Its sensitivity is, however, 2–6 orders of magnitude lower than that of optical imaging. This can be overcome by using appropriate MRI contrast agents. Nowadays, more than 30% of MRI examinations benefit from the use of paramagnetic contrast agents [2]. By accelerating the longitudinal or transverse relaxation of water protons, they increase the signal-to-noise ratio, which, in turn, positively influences the image contrast. The currently used clinical contrast agents are mainly Gd³⁺ chelates, making use of the high magnetic moment (seven unpaired electrons) and slow electronic relaxation of this paramagnetic lanthanide ion [3, 4]. These contrast agents are, however, mostly nonspecific and provide only anatomical information. Nowadays, there is an active search for new classes of contrast agents capable of reflecting a change in biological activity in their local environment. A new field, molecular imaging, is being developed, with the aim of in vivo visualization of molecular events. Any molecular imaging procedure requires an imaging probe that is specific for a given molecular event. Examples of such “smart” potential contrast agents, sensing variables like pH [5–7], partial oxygen pressure [8, 9], ion and metabolite concentration [10–14], or enzyme activity [15, 16] have been described in the literature. They function on the basis of the modulation of one or more microscopic parameters of the Gd³⁺ chelate determining relaxivity, induced by a change in the local environment of the agent. Among the variables that influence the relaxivity of a contrast agent, the most important are the applied magnetic field (B₀), the number of water molecules directly bound to the paramagnetic center (q), their exchange rate (k_ex), the water proton–Gd³⁺ distance (r_GdH), the electron spin relaxation times (T_1e, T_2e), the rotational correlation time (τ_R), and the presence and the number of water molecules in the so-called second coordination sphere (q^2nd) [2, 4, 17, 18].

Calcium (Ca²⁺) is one of the most important metal ions for life as it controls muscular contraction, neural cell communication, hormonal secretion, etc. [19]. In the brain, Ca²⁺ acts as an important second messenger [20]. Synaptic transmission depends on the entry of calcium ions at the presynaptic terminal to cause fusion and release of vesicles. Significant changes in Ca²⁺ concentration take place during neuronal activity [21]. Tracking the dynamics of Ca²⁺ concentration changes could thus contribute to the understanding of basic aspects of neuronal regulation or to highlight the abnormalities in the diseased state [22]. Optical imaging based on fluorescent dyes possessing a calcium-dependent response has been widely used at the cellular or cell population level [23]. However, the intrinsic depth limitations associated with optical imaging restrict these applications to superficial regions. In contrast to optical imaging, MRI has no depth penetration limits and could be more adapted for tracking Ca²⁺ changes. Li et al. [11, 24] have proposed a potential MRI contrast agent, GdDOPTA, whose relaxivity was reported to be sensitive to Ca²⁺ concentration in the 0.1–10-μM range. The two macrocyclic subunits of the dimeric ligand DOPTA serve as lanthanide(III) ion chelators, while the BAPTA⁴⁻ subunit bridging the macrocycles is known for its high selectivity towards Ca²⁺ [H₄BAPTA is 1,2-bis(o-aminophenoxy)ethane-N,N,N′,N′-tetraacetic acid]. The mechanism proposed for the Ca²⁺-dependent relaxivity change of GdDOPTA accounts for changes in the number of water molecules directly bound to the paramagnetic metal ion (q) upon Ca²⁺ binding to the BAPTA⁴⁻ moiety. BAPTA⁴⁻ was proposed a long time ago as a selective fluorescent indicator for Ca²⁺ [25]. It presents several advantages for biological Ca²⁺ detection by optical methods: integrated aromatic chromophore moiety and pH insensitivity of Ca²⁺ complexation thanks to the low values of the protonation constants (6.36 and 5.47, both below physiological pH). More recently, another calcium-sensitive MRI probe was proposed by Atanasijevic et al. [20]. It is based on superparamagnetic iron oxide nanoparticles and calmodulin. In this approach, calcium-dependent protein–protein interactions lead to particle clustering and consequent changes in transverse relaxivity. Similarly to GdDOPTA, this agent had calcium-response in the micromolar Ca²⁺ concentration range. It was therefore proposed to detect elevated intracellular Ca²⁺ levels, provided it is combined to a cellular delivery system.

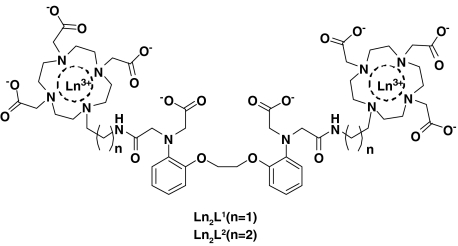
With the objective of detecting extracellular Ca²⁺ concentration changes by MRI methods, we synthesized two novel potential bismacrocyclic MRI contrast agents (Structure 1). Two Gd³⁺-loaded 1,4,7,10-tetraazacyclododecane-1,4,7-triacetic acid (DO3A) derived units were connected by a BAPTA-derived linker capable of Ca²⁺ binding. However, in comparison with BAPTA⁴⁻, the Ca²⁺ binding moiety in the central part of the ligand was designed to have a reduced affinity towards Ca²⁺ in order to shift to higher Ca²⁺ concentrations the range where the probe is expected to have a relaxivity response. The conditional stability constant of GdDOPTA–Ca at physiological pH was reported to be 1.0 × 10⁶ M⁻¹ [11]. Similarly, the fluorescent Ca²⁺ indicators used in biological applications and based on BAPTA⁴⁻ are adapted to assess the cytosolic free Ca²⁺ concentration in the micromolar range. In contrast, the extracellular concentration of Ca²⁺ is in the millimolar range. The relaxivity response of a probe with affinity of K ∼ 10⁶ M for Ca²⁺ would have already leveled off at the millimolar level. The BAPTA⁴⁻ ligand was therefore modified by replacing two carboxylates by the less strong donor amide functions. As for the macrocyclic Gd³⁺ binding site, it was designed to respond to the presence of Ca²⁺ by modulation of the hydration number with consequent change in the relaxivity.

Structure 1.

 

The proton relaxivities of the two new complexes were studied by relaxometric titrations at different Ca²⁺ concentrations and the relaxivity modulation by the Ca²⁺ concentration changes was confirmed. We specifically aimed to identify the microscopic factors responsible for the Ca²⁺-dependent relaxivity response. To this end, a detailed mechanistic study was carried out. We used UV–vis absorption and luminescence lifetime measurements on the corresponding Eu³⁺ complexes to assess the number of inner-sphere water molecules and their variation on Ca²⁺ addition. The Gd₂L¹ complex was characterized by variable-temperature ¹⁷O NMR spectroscopy and variable-field relaxivity measurements (nuclear magnetic relaxation dispersion, NMRD). The experimental data were evaluated on the basis of the Solomon–Bloembergen–Morgan theory, extended with a second-sphere contribution.

Experimental

Materials and methods

All chemicals were purchased at the purest grade commercially available and were used without further purification. Cyclen was purchased from Strem, France. Bromoethylamine hydrogen bromide, bromopropylamine hydrobromide, tert-butyl bromoacetate, N-methylpyrollidinone (dry), pyridine, H₄BAPTA, acetic anhydride and N,N-dimethylformamide (extra dry) were purchased from Sigma-Aldrich, Germany. Toluene, acetonitrile, dichloromethane and methanol were purchased as analytical grade solvents from Acros Organics, Germany. Lanthanide(III) chlorides were prepared by dissolving metal(III) oxides (Aldrich) in a slight excess of HCl (Carlo Erba, 37%) followed by evaporation of solvents and dissolution in H₂O.

High-performance liquid chromatography (HPLC) was performed at room temperature using a Varian (Australia) PrepStar instrument equipped with a PrepStar 335 photodiode array detector at 254 nm. Reversed-phase analytical HPLC was performed in a stainless steel ChromSep (length 250 cm, internal diameter 4.6 mm, outside diameter 3/8 in. and particle size 8 μm) C18 column (Varian). The ligands were purified using the following gradient:

• Method A: 40% solvent A (methanol) and 60% solvent B (water) to 100% solvent B in 5 min running isocratically at 100% solvent B for 10 min and then to 60% solvent B in the next 2 min.

• Method B: 95% solvent A (acetonitrile, 0.1% HCOOH) and 5% solvent B (water, 0.1% HCOOH) to 70% solvent B in 10 min and then 100% in the next 8 min running isocratically for 12 min after that and then to 5% in next 2 min.

The flow rate used for analytical HPLC was 1 mL min⁻¹ and for preparative 65 mL min⁻¹. All solvents used were HPLC grade filtered through a 0.45-μm nylon-66 Millipore filter prior to use.

¹H and ¹³C NMR spectra were recorded using a Bruker 400 MHz spectrometer (¹H—internal reference CDCl₃ at 7.27 ppm or D₂O at 4.75 ppm; ¹³C—internal reference CDCl₃ at 77.0 ppm or tetramethylsilane at 0 ppm). All the experiments were performed at 298 K. Electrospray ionization (ESI) low-resolution mass spectrometry (LRMS) spectra were obtained using an SL 1100 system (Agilent, Germany) with ion-trap detection in positive and negative ion mode. IR spectra were recorded with a Nicolet Impact 400 D spectrometer using neat compounds as disks with KBr and only the major bands were noted. UV–vis spectra of ⁵D₀ ← ⁷F₀ transitions of Eu₂L¹ were obtained with a PerkinElmer Lambda 19 spectrometer in the region 577–581 nm with data steps of 0.05 nm [26]. The concentrations of the samples were approximately 0.02 M and the temperature dependence was measured in the interval 288–323 K in the absence and presence of Ca²⁺. To maintain a constant temperature, thermostatizable cells with a 10-cm optical length were used. The luminescence measurements were performed with a Varian Eclipse spectrofluorimeter, equipped with a 450-W xenon arc lamp, a microsecond flash lamp and a red-sensitive photomultiplier (300–850 nm). The luminescence spectra were obtained after excitation of the ⁵L₆ ← ⁷F₀ band (394 nm). An aqueous solution (0.02 M) of Eu₂L¹ was measured at 298 K in the absence and presence of Ca²⁺.

The ¹H NMRD profiles were recorded at the Laboratory of Inorganic and Bioinorganic Chemistry, Ecole Polytechnique Fédérale de Lausanne, Switzerland, using a Stelar Spinmaster FFC fast-field-cycling relaxometer covering magnetic fields from 2.35 × 10⁻⁴ to 0.47 T (proton Larmor frequency range 0.01–20 MHz). The temperature was controlled by a VTC90 temperature control unit and fixed by a gas flow. At higher fields, the relaxivity was recorded using Bruker Minispecs mq30 (30 MHz), mq40 (40 MHz) and mq60 (60 MHz), on a Bruker 4.7 T (200 MHz) cryomagnet connected to a Bruker Avance 200 console and with a Bruker Avance 500 spectrometer (500 MHz). The temperature was measured by a substitution technique [27] or via a preliminary calibration using methanol and ethylene glycol standards [28]. The longitudinal (1/T₁) and transverse (1/T₂) ¹⁷O NMR relaxation rates were measured in the temperature range 277–344 K. The data were recorded using a Bruker Avance 500 (11.75 T, 67.8 MHz) spectrometer. The temperature was calculated according to a previous calibration with ethylene glycol and methanol [28]. The samples were measured in 5-mm NMR tubes and were enriched with tert-butanol to allow for the bulk magnetic susceptibility correction [29]. The 1/T₁ data were obtained by the inversion recovery method, while the 1/T₂ data were measured by the Carr–Purcell–Meiboom–Gill spin-echo technique. Acidified water (HClO₄, pH 3.8) was used as an external reference. Analyses of the ¹⁷O NMR and ¹H NMRD experimental data were performed with the Visualiseur/Optimiseur programs running on a MATLAB platform version 6.5 [30, 31].

Synthesis

Compound 4a was synthesized according to a previously reported procedure [32]. With use of an analogous method, compound 4b was synthesized via 3b from the substrate 1b [33].

Compound 3b. ¹H NMR (CDCl₃, 400 MHz), δ (ppm): 6.97–6.84 (m, 5H), 4.65 (s, 2H), 2.98–2.89 (m, 2H), 2.86–2.70 (m, 5H), 2.70–2.60 (m, 3H), 2.58–2.17 (m, 7H), 2.16–1.75 (m, 9H), 1.32 (br s, 2H), 1.06–1.03 (m, 27H). ¹³C NMR 172.2, 171.2, 155.2, 152.9, 135.6, 127.1, 126.4, 81.3, 81.0, 65.7, 64.7, 55.4, 50.3, 48.9, 38.5, 37.8, 28.2, 26.7, 26.5, 25.2. ESI–MS m/z: [M + H]⁺ calcd for C₃₇H₆₃N₅O₈, 705.5; found, 706.5.

Compound 4b. ¹H NMR (CDCl₃, 400 MHz), δ (ppm): 8.19 (br s, 2H), 3.42–2.99 (m, 8H), 2.85–2.20 (m, 16H), 1.61 (br s, 2H), 1.41 (s, 27H). ¹³C NMR (CDCl₃, 100 MHz), δ (ppm): 172.0, 169.8, 81.8, 81.1, 57.2, 55.9, 50.1, 49.4, 48.9, 38.5, 27.2, 27.1, 22.9. ESI–MS m/z: [M + H]⁺ calcd for C₂₉H₅₇N₅O₆, 571.4; found, 572.4 .

General method for the synthesis of compounds 6a and 6b

Compound 4a or 4b (2 mmol) was dissolved in 5 mL of dry N-methylpyrollidinone with 50 μL of dry Et₃N and heated at 333 K for 15 min. Anhydride 5 [34] was then added to this reaction mixture (295 mg, 0.67 mmol) in small lots under N₂. After complete addition of 5, the solution was kept under continuous stirring at 333 K overnight. It was then evaporated, dissolved in dichloromethane and extracted with water. The organic layer was collected and evaporated to get a yellow oil. The crude product was then purified by reversed-phase HPLC using method A to get a light yellow fluffy solid.

1,2-Bis{[2-{[({1-[1,4,7-tris(tert-butoxycarbonylmethyl)-1,4,7,10-tetraazacyclododecane-10-yl]eth-2-yl}amino)carbonyl]methyl}-(carboxymethyl)amino]phenoxy}ethane, 6a. Yield: 573 mg (55%) ¹H NMR (CDCl₃, 400 MHz), δ (ppm): 7.01–6.98 (m, 2H), 6.92–6.89 (m, 2H), 6.77–6.74 (m, 4H), 4.56 (d, J = 6.4 Hz, 2H, CHHO), 4.34 (d, J = 15.5 Hz, 2H, CHHCONH), 4.01 (d, J = 17.8 Hz, 2H, CHHCOOH), 3.91 (d, J = 6.4 Hz, 2H, CHHO), 3.48 (d, J = 17.8 Hz, 2H, CHHCOOH), 3.36–3.21 (m, 22H), 3.08–2.80 (m, 12H), 2.78–2.69 (m, 6H), 2.76–2.56 (m, 18H), 1.47 (s, 36H), 1.43 (s, 18H). ¹³C NMR (CDCl₃, 100 MHz), δ (ppm): 175.2 (CONH), 175.0 (CONH), 170.2, 170.1, 149.4, 139.2, 120.6, 119.5, 115.4, 112.1, 81.8, 81.5, 66.4 (CH₂O), 60.7 (NCH₂CONH), 58.5 (CH₂COOH), 56.5, 54.8, 53.5, 51.3, 50.3, 49.7, 47.8, 32.1 (CH₂CH₂CONH), 29.6, 28.1. ESI–MS m/z: [M + H]⁺ calcd for C₇₈H₁₃₀N₁₂O₂₀, 1,554.9; found, 1,555.9.

1,2-Bis{[3-{[({1-[1,4,7-tris(tert-butoxycarbonylmethyl)-1,4,7,10-tetraazacyclododecane-10-yl]prop-3-yl}amino)carbonyl]methyl}-(carboxymethyl)amino]phenoxy}ethane, 6b. Yield: 424 mg (40%). ¹H NMR (CDCl₃, 400 MHz), δ (ppm): 7.08–7.06 (m, 2H), 6.91–6.89 (m, 2H), 6.85–6.82 (m, 4H), 4.49 (br s, 2H), 4.31 (br s, 4H), 4.08–3.73 (m, 8H), 3.57–3.09 (m, 20H), 3.04–2.47 (m, 32H), 2.40 (br s, 4H), 2.01 (br s, 2H), 1.53 (s, 18H), 1.48 (s, 36H). ¹³C NMR (CDCl₃, 100 MHz), δ (ppm): 175.7, 173.4, 170.9, 170.2, 150.2, 139.8, 120.3, 120.2, 115.7, 112.2, 82.2, 82.0, 66.6, 61.0, 58.7, 56.5, 55.3, 49.6, 49.1, 47.6, 35.5, 28.6, 28.4, 22.2. ESI–MS m/z: [M + H]⁺ calcd for C₈₀H₁₃₄N₁₂O₂₀, 1,582.9; found, 1,584.0.

General method for the synthesis of L¹ and L²

Neat trifluoroacetic acid (70 mL) was added to the previously obtained compound 6a or 6b (0.32 mmol) and the reaction was kept at room temperature for 24 h. Trifluoroacetic acid was then evaporated, and the residue was dried under vacuum and purified by reversed-phase HPLC using method B.

1,2-Bis{[2-{[({1-[1,4,7-tris(carboxymethyl)-1,4,7,10-tetraazacyclododecane-10-yl]eth-2-yl}amino)carbonyl]methyl}-(carboxymethyl)amino]phenoxy}ethane, L¹. Yield: 220 mg (60%). ¹H NMR (CDCl₃, 400 MHz), δ (ppm): 7.06–6.99 (m, 4H), 6.94 (t, J = 7.0 Hz, 2H), 6.87–6.85 (m, 2H), 4.27 (s, 4H), 3.83 (s, 4H), 3.77 (s, 4H), 3.58 (br s, 8H), 3.45 (s, 4H), 3.28–3.12 (m, 24H), 3.02–2.97 (m, 4H), 2.82–2.75 (m, 12H). ¹³C NMR (D₂O, 100 MHz), δ (ppm): 177.6, 174.7, 170.5, 169.9, 150.1, 138.9, 122.5, 121.4, 117.4, 113.0, 67.0, 57.9, 56.7, 56.1, 55.3, 51.0, 50.6, 50.5, 49.2, 47.6, 33.5. IR (cm⁻¹): 3,426 (vs), 2,964 (m), 2,929 (m), 2,860 (m), 1,718 (s), 1,637 (vs), 1,384 (s), 1,355 (s), 1,328 (m), 1,240 (s), 1,203 (s), 762 (m), 694 (m). ESI–MS m/z: [M−H]⁻ calcd for C₅₄H₈₂N₁₂O₂₀, 1,218.57; found, 1,217.7.

1,2-Bis{[3-{[({1-[1,4,7-tris(carboxymethyl)-1,4,7,10-tetraazacyclododecane-10-yl]prop-3-yl}amino)carbonyl]methyl}-(carboxymethyl)amino]phenoxy}ethane, L². Yield: 126 mg from 400 mg of 6b (40%). 1H NMR (D₂O, 400 MHz), δ (ppm): 6.98–6.91 (m, 4H), 6.88 (t, J = 7.4, 2H), 6.82–6.80 (m, 2H), 4.20 (s, 4H), 3.78 (s, 4H), 3.74 (s, 4H), 3.57 (s, 4H), 3.39–3.31 (m, 8H), 3.27–3.20 (m, 8H), 3.13–2.92 (m, 20H), 2.89–2.83 (m, 8H), 2.65 (br s, 4H), 1.61–1.53 (m, 4H). ¹³C NMR (D₂O, 100 MHz), δ (ppm): 177.7, 174.4, 149.9, 138.5, 122.3, 121.5, 117.4, 113.2, 67.1, 57.6, 56.2, 56.1, 55.4, 50.9, 50.5, 49.6, 49.2, 36.4, 22.6. ESI–MS m/z: [M−H]⁻ calcd for C₅₆H₈₆N₁₂O₂₀, 1,246.6; found, 1,245.6.

Preparation of solutions of lanthanide(III) complexes

The complexes used for ¹H and ¹⁷O relaxometric and UV–vis measurements were prepared by mixing a slight excess (5%) of the ligand solution with the appropriate lanthanide(III) chloride solution. The pH was adjusted to 7 using KOH solution and the reaction mixture was stirred at 323 K overnight. The absence of free Ln³⁺ was checked by xylenol orange indicator in HCl/urotropine buffer (pH 5.8). For each Gd₂L sample, the Gd³⁺ concentration was determined by measuring the bulk magnetic susceptibility shifts [35].

Gd₂L¹. ESI–LRMS m/z: [M−H + Na]⁻ calcd for C₇₈H₁₂₄Gd₂N₁₂O₂₀, 1,525.1; found, 1,547.6. IR: 3,448 (vs), 1,602 (vs), 1,560 (s), 1,400 (m), 1,323 (m), 1,242 (m), 1,203 (vs), 1,085 (m), 762 (m), 723(m).

Eu₂L¹. ESI–LRMS m/z: [M−H]⁻ calcd for C₅₄H₇₆Eu₂N₁₂O₂₀, 1,156.4; found 1,155.5.

Gd₂L². ESI–LRMS m/z: [M + H]⁺ calcd for C₅₆H₈₀Gd₂N₂₀O₁₂, 1,553.1; found, 1,553.6.

Relaxometric Ca²⁺ titrations of Gd₂L¹ and Gd₂L²

The titrations were performed at 11.75 T, 298 K and pH 7.3 [maintained by N-(2-hydroxyethyl)piperazine-N′-ethanesulfonic acid buffer]. A solution of CaCl₂ of known concentration was added stepwise to the complex solution and the longitudinal proton relaxation time T₁ was measured after each Ca²⁺ addition. The initial Gd³⁺ concentrations were 7.4 and 4.0 mM for Gd₂L¹ and Gd₂L², respectively. The relaxivity r₁ was calculated from Eq. 1, using the actual Gd³⁺ concentration at each point of the titration:

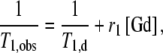1

where T_1,obs is the observed longitudinal relaxation time, T_1,d is its diamagnetic contribution in the absence of the paramagnetic substance and [Gd] is the concentration of Gd³⁺.

Relaxometric Mg²⁺ titration of Gd₂L¹

The titration was done analogously to the Ca²⁺ titration (B = 11.75 T, 298 K; initial Gd³⁺ concentration of 2.6 mM). When the Mg²⁺-to-complex ratio was 23 (in the Ca²⁺ titration, the plateau was already reached at this Ca²⁺-to-Gd₂L¹ ratio), a Ca²⁺ solution was added stepwise and the relaxivity was measured.

Results and discussion

Synthesis of ligands

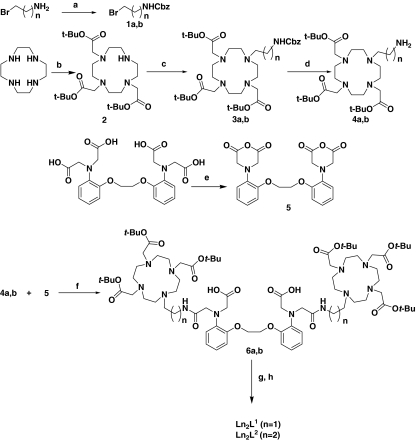
The ligands were prepared from the appropriate anhydride and amine precursors in a facile two-step procedure as shown in Scheme 1. Compounds 1a, 1b, 2 and 5 were synthesized according to reported procedures [32–34]. Protected amines 3a an 3b were synthesized by alkylating 2 with 1a or 1b in acetonitrile. Hydrogenation of the resulting products in methanol using 10% Pd on carbon as a catalyst yielded 4a and 4b. Bismacrocycles 6a and 6b were obtained by coupling of amines 4a or 4b to an anhydride 5 in N-methylpyrrolidinone and triethylamine. After the purification, they were hydrolyzed with neat trifluoroacetic acid and purified by reversed-phase HPLC giving the final ligands L¹ and L². It is interesting to note that an additional proof for the extremely high steric constraints in the bismacrocyclic systems studied was observed in 2D NMR (correlation spectroscopy, heteronuclear single quantum coherence) spectra of 6a (Figs. S13, S14). The splitting of the signals occurs on all methylene groups not belonging to the macrocyclic ring; this disappears upon the removal of tert-butyl protective groups in L¹.

Scheme 1.

Synthesis of the ligands and their lanthanide(III) complexes. Reagents and conditions: a benzyl chloroformate, K₂CO₃, water/dioxane; b tert-butylbromoacetate, NaHCO₃, MeCN, 70%; c 1a or 1b, K₂CO₃, MeCN, 60%; d H₂, Pd/C, MeOH; e Ac₂O, pyridine, 75%; fN-methylpyrollidinone, Et₃N; g trifluoroacetic acid; h LnCl₃·6H₂O

Relaxometric Ca²⁺ titrations of Gd₂L¹ and Gd₂L²

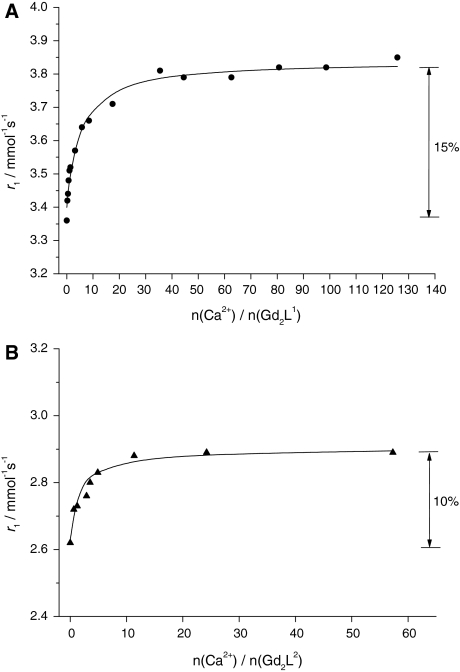
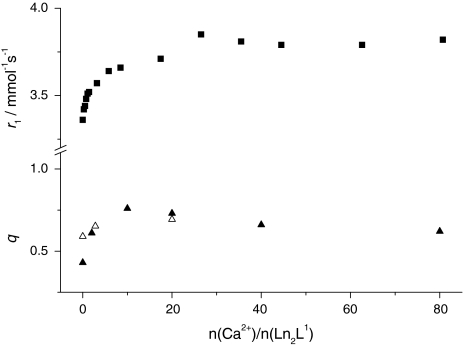
The titration curves are represented as the variation of the relaxivity of the Gd³⁺ complex solution versus the Ca²⁺ concentration (Fig. 1). The maximum relaxivity increase upon Ca²⁺ addition was 15% for Gd₂L¹ and 10% for Gd₂L². The saturation of both curves occurs at relatively high Ca²⁺ concentrations (approximately 20 equiv of Ca²⁺ for Gd₂L¹ and approximately 5 equiv of Ca²⁺ for Gd₂L²). The titration curves were fitted to obtain the apparent association constants, which are log K = 1.9 ± 0.2 and log K = 2.7 ± 0.2 for Gd₂L¹ and Gd₂L², respectively. Similarly to the case for previously investigated BAPTA-type complexes [11, 25], 1:1 binding stoichiometry has been assumed. These association constants are to be compared with the conditional stability constant, log K_cond = 6.9 of CaBAPTA²⁻ at pH 7.0, calculated by taking into account the protonation constants of BAPTA⁴⁻ [25]. The constants obtained for our systems are 3–4 orders of magnitude lower. This difference can be rationalized by the fact that even on Ca²⁺ binding, the amide groups of the ligand remain coordinated to the lanthanide as indicated by proton relaxivity and luminescence data on the Gd³⁺ and Eu³⁺ complexes, respectively (see below). Therefore, in comparison with CaBAPTA²⁻, there are two carboxylate donors fewer coordinating to the Ca²⁺ ion, which is responsible for the considerably reduced stability. It is interesting to note that the stability of the Gd₂L²–Ca complex is somewhat higher than that of Gd₂L¹–Ca. This is likely related to the higher flexibility of the Ca²⁺ binding site in Gd₂L²–Ca, where the propylene linker between the macrocycle and the Ca²⁺ binding site induces fewer steric constraints, hence ensuring a better “overwrapping” of the cation by the chelator. We have to note that for GdDOPTA-Ca, possessing an integral BAPTA⁴⁻ unit for Ca²⁺ binding, Li et al. [11] reported a much higher apparent association constant, K = 1.0 × 10⁶ M.

Fig. 1.

Relaxometric Ca²⁺ titration curves of Gd₂L¹ (a) and Gd₂L² (b) obtained at 298 K and 11.75 T. The lines correspond to the fit as explained in the text

Relaxometric Mg²⁺ titration of Gd₂L¹

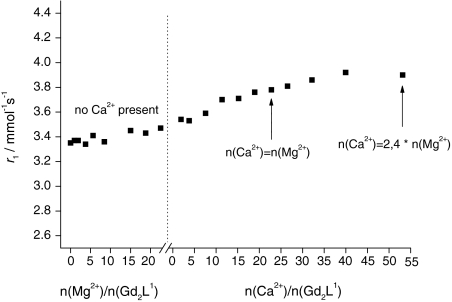
The relaxivity remains approximately constant upon addition of Mg²⁺, then it increases upon Ca²⁺ addition even in the presence of a large amount of Mg²⁺ (Fig. 2). The relaxivity increase after Ca²⁺ addition (16%) was similar to that observed in the Ca²⁺ titration without the presence of Mg²⁺. The insensitivity of Gd₂L¹ towards Mg²⁺ is due to the lower stability of BAPTA-derived complexes with Mg²⁺ compared with Ca²⁺. The titration curve shows that, though the affinity of our chelator for Ca²⁺ is diminished in comparison with BAPTA⁴⁻, the selectivity versus Mg²⁺ is conserved. The discrimination of BAPTA-type ligands towards Ca²⁺ compared with Mg²⁺ is supposed to stem from the right size of the binding cavity for the larger-sized Ca²⁺, which is already too big for Mg²⁺ and cannot constrict further to envelop snugly this smaller cation (the association constant of MgBAPTA²⁻ is log K = 1.8) [25]. Spectrophotometric measurements on the Mg²⁺–BAPTA system proved that Mg²⁺ coordination affects only half of the ligand, in contrast to Ca²⁺ coordination, where the entire ligand is involved [25].

Fig. 2.

Relaxometric titration curve of Gd₂L¹ with Mg²⁺ followed by addition of Ca²⁺ (298 K, 11.75 T)

Luminescence and UV–vis absorption studies to assess the hydration state of Eu₂L¹

In order to determine the number of water molecules coordinated to the lanthanide ion before and after Ca²⁺ binding to the complex, we performed luminescence lifetime measurements on Eu₂L¹ in H₂O and D₂O solutions [36–38]. In the absence of Ca²⁺, the luminescence lifetimes are 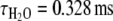and 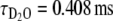. The hydration number, q = 0.4, was calculated according to the revised equation of Beeby et al. [38] which involves a correction for the contribution of second-sphere water molecules (Eq. 2):

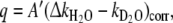2

where A′ is 1.2 ms and the correction factor for the contribution of the second sphere is −0.25 m s⁻¹. The longitudinal ¹⁷O and ¹H relaxation rates (vide supra) indicate that we cannot neglect the effect of second-sphere water molecules. The noninteger hydration number suggests the presence of both q = 1 and q = 0 species. Upon the addition of Ca²⁺ to the Eu³⁺ complex, the hydration number determined by luminescence increases to q ≈ 0.7 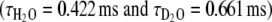(Fig. 3). Such an increase of q can be interpreted in terms of a shift towards the monohydrated species.

Fig. 3.

Dependence of the relaxivity r₁ and of the hydration number q on the Ca²⁺-to-complex ratio for Ln₂L¹. Squares correspond to the relaxivity values obtained from the relaxometric titration of Gd₂L¹ (298 K, 11.75 T). Filled triangles represent the hydration number obtained from luminescence lifetime studies on Eu₂L¹ and open triangles correspond to the molar fraction of the monohydrated species determined for Eu₂L¹ by UV–vis spectroscopy (ratio of the integrals of the absorption bands corresponding to monohydrated and nonhydrated complex; see text)

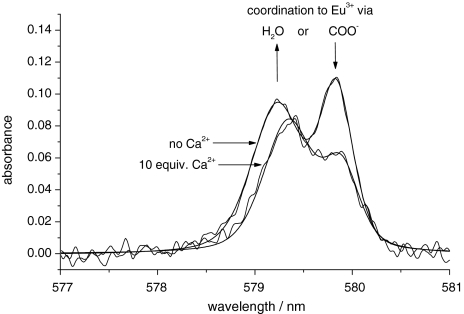
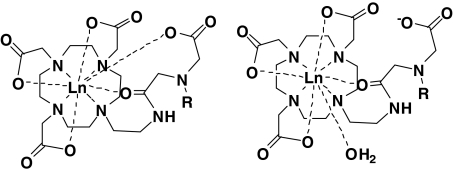
To investigate further the hydration state, variable-temperature UV–vis measurements were performed on Eu₂L¹. In general, the presence of a hydration equilibrium of europium(III) species leads to the appearance of two absorption bands for the ⁵D₀ ← ⁷F₀ transition with peak separations of more than 0.5 nm [39, 40]. High-resolution UV–vis absorption spectra of a Eu₂L¹ aqueous solution (pH 7) were recorded in the 577–581-nm region at various temperatures and Ca²⁺ concentrations. The measurements revealed two temperature-invariant absorption bands, which could be deconvoluted into two symmetrical peaks (Fig. 4). The separation of those peaks is about 0.5 nm and lies in the range typical of different coordination environments of Eu³⁺ [41, 42]. We assume that in the absence of Ca²⁺, there are two nine-coordinate species present; in both species the amines and carboxylates of the macrocycle as well as the amide oxygen are coordinated to the lanthanide, and either carboxylate from the central part or one water molecule completes the coordination sphere to a coordination number of nine, which is the usual coordination number for this type of Ln³⁺ complex (Fig. 5). The coordination of amide oxygens is supported by IR measurements performed on the ligand L¹ and its Gd³⁺ complex: the comparison of the two IR spectra (Figs. S5, S6) shows that the amide absorption is affected by complexation to the lanthanide ion.

Fig. 4.

Experimental and fitted UV–vis spectra (323 K, pH 7) of Eu₂L¹ in the absence and presence of Ca²⁺. On addition of Ca²⁺, the relative intensity of the band at lower wavelength increases, while that of the band at higher wavelength decreases

Fig. 5.

Proposed structures present in aqueous solution of Ln₂L¹. R stands for the remaining part of the bismacrocyclic complex

Among analogous structures, lanthanide complexes of monomeric DO3A derivatives bearing –(CH₂)_n–NHCO–R (n = 2, 3) amide units have been reported in the literature [43]. With Eu³⁺ and Gd³⁺, the n = 2 ligand forms monohydrated complexes, while the n = 3 ligand forms nonhydrated complexes. In contrast to this, Eu₂L¹ contains a carboxylate in the central part as a potential donor which can partially replace the water molecule in the inner coordination sphere. This leads to the presence of the two structures as proposed in Fig. 5, and consequently to a reduced hydration number.

Upon Ca²⁺ addition to a Eu₂L¹ solution, the relative intensity of the two UV–vis absorption bands changes: the band at higher wavelengths decreases, while the other band increases (Fig. 4) [44]. In parallel, the luminescence measurements indicate an increase of the hydration number. Therefore, we conclude that the band which decreases in intensity (at 579.9 nm) on Ca²⁺ addition can be attributed to the q = 0 complex, while the band which increases in intensity (579.3 nm) can be attributed to the monohydrated complex. We assume that both in the presence and in the absence of Ca²⁺, the amide oxygen remains coordinated to the lanthanide ion to preserve the overall coordination number of nine. If the amide oxygen participated also in the Ca²⁺ coordination, the concomitant increase in the hydration number and in relaxivity would be more prominent than what is experimentally observed. The Ca²⁺ binding in the central part of the complex demands the coordination of the central carboxylates, which, by leaving the coordination environment of Ln³⁺, allow a water molecule to bind to the lanthanide ion. Therefore, the Ca²⁺ binding in the central part will favor the formation of the monohydrated complex (Fig. 5).

Relation between the Ca²⁺-dependent hydration number q and relaxivity

Upon stepwise addition of Ca²⁺, we observe a good correlation between the relaxivity increase of the Gd₂L¹ complex and the increase in q determined from the luminescence lifetime measurements on the Eu³⁺ analogue (Fig. 3). In addition, we also calculated the ratio of the integrals of the two UV–vis absorption bands (attributed to q = 0 and q = 1) of Eu₂L¹ at various Ca²⁺ concentrations. The q values obtained in this way overlap with those measured by luminescence. This supports our hypothesis that the complex Ln₂L¹ exists in the form of two differently hydrated species, each with an overall coordination number of nine. As was proved by the UV–vis studies, their ratio is temperature-independent but it shifts towards the monohydrated species with increasing Ca²⁺ concentration, resulting in a relaxivity increase.

The relaxivity of the Gd₂L² complex is lower than that of the L¹ analogue, which suggests that q is also lower. In the case of the monomeric DO3A derivatives bearing –(CH₂)_n–NHCO–R amide units, with the propyl-linked (n = 3) amide q = 0 was determined for the Eu³⁺ and Gd³⁺ complexes [43]. The extra steric demand associated with the enlargement of the chelate ring incorporating the amide carbonyl group was found to be sufficient to suppress water coordination. For Gd₂L², the relaxivities suggest q > 0. Moreover, the relaxivity increases on Ca²⁺ addition, though to a smaller extent than for Gd₂L¹. Therefore, we assume that two differently hydrated species exist also for Ln₂L² complexes, with an increased proportion of the nonhydrated species compared with Ln₂L¹. The relaxivity change observed on Ca²⁺ addition indicates that the central carboxylate participates in the lanthanide coordination; on Ca²⁺ binding this carboxylate is removed from the lanthanide ion and replaced by a water molecule. The small relaxivity change shows that the participation of this carboxylate in the lanthanide coordination is limited with respect to Gd₂L¹.

For GdDOPTA, Li et al. [11, 24] reported a more important variation of q and a correspondingly greater relaxivity change on Ca²⁺ binding. This is likely related to the more flexible nature of the central BAPTA⁴⁻ part of the DOPTA ligand in contrast to the BAPTA-bisamide moiety in our case, where the monoamide functionalities are integrated in the central skeleton of the ligand and have much less flexibility to change coordination from Gd³⁺ to Ca²⁺.

¹H and ¹⁷O relaxation studies of Gd₂L¹: evaluation of the parameters influencing proton relaxivity

¹H NMRD profiles were recorded for Gd₂L¹ with and without Ca²⁺. In the presence of Ca²⁺, an increase in the relaxivity was observed at all frequencies, related to an increase in the hydration number. The relaxivity at 20 MHz and 298 K in the absence and presence of Ca²⁺ is 5.74 and 6.13 mM⁻¹ s⁻¹, respectively, slightly higher than the relaxivites of currently used MRI contrast agents [2, 4, 19, 45].

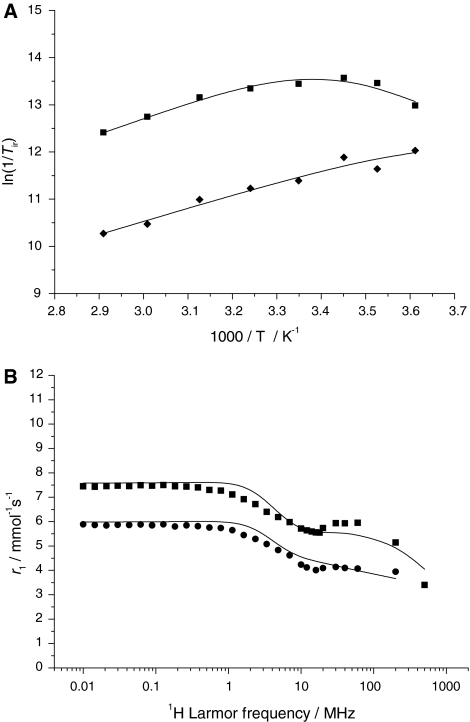
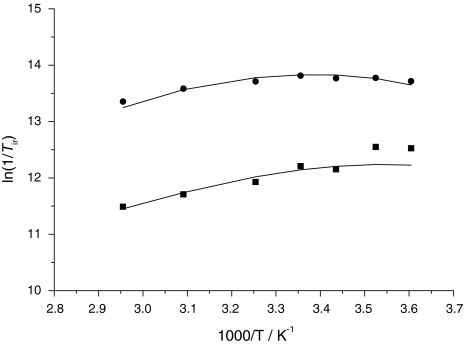
The transverse ¹⁷O relaxation rates indicate a relatively slow water exchange (Fig. 6), which is visible from the curve of ln(1/T_2r) versus inverse temperature: at low temperatures, 1/T_2r decreases with decreasing temperature. The reduced chemical shifts (Δω_r) in the absence of Ca²⁺ are smaller than would be expected for a q = 0.4 complex. Such small chemical shifts have previously been observed in systems with a significant second-sphere contribution [46]. Therefore, the chemical shifts were not included in the final fitting. For the Ca²⁺-free system, the transverse and longitudinal ¹⁷O relaxation rates and the ¹H NMRD data were analyzed simultaneously (Fig. 6) on the basis of the Solomon–Bloembergen–Morgan approach, extended by a second-sphere contribution [46, 47]. The presence of free carboxylates in the complex induces a second-sphere contribution that affects both ¹H and ¹⁷O longitudinal relaxation. In fact, by fitting the ¹⁷O 1/T₁ values without second-sphere contribution, we obtained inconceivably high rotational correlation times. The inner-sphere hydration number q was fixed to 0.4, the value found by luminescence measurements on Eu₂L¹. Since Eu³⁺ and Gd³⁺ have similar ionic radii, we expect a similar hydration mode for Gd₂L¹. To describe the second-sphere contribution, one water molecule per Gd³⁺ (q^2nd = 1) was considered during the fitting. The distance between the Gd³⁺ ion and the second-sphere water proton and oxygen was fixed to r^2nd_Gd–H = 3.5 Å and r^2nd_Gd–O = 4.1 Å, respectively, the enthalpy of activation to ΔH^#2nd = 35 kJ mol⁻¹, and the second-sphere water residence time to τ^2nd_m = 50 ps [46, 47]. Other parameters were also fixed during the fitting in order to add some constraints. They are as follows: the hyperfine coupling constant, A/ħ = −3.8 MHz; the distance between Gd³⁺ and the oxygen and the proton of the first-sphere water molecule, r_GdO = 2.5 Å and r_GdH = 3.1 Å, respectively. The distance of the closest approach of outer-sphere water molecules to Gd³⁺, a, was fixed to 3.6 Å. The quadrupolar coupling constant [χ(1 + η²/3)^1/2] was set to 7.58 MHz, the value of pure water. The activation energy E_v had to be fixed to 1 kJ mol⁻¹, otherwise the fit converged to negative values. In the fitting procedure, we used a model which considers different rotational correlation times for Gd–O and Gd–H rotating vectors (τ²⁹⁸_RO and τ²⁹⁸_RH, respectively) [48]. For geometrical reasons, their ratio (τ²⁹⁸_RH/τ²⁹⁸_RO) has to lie between 0.65 and 1. The most important parameters obtained from the best simultaneous least-squares fit to the experimental data are listed in Table 1. For the complete fitting results, see Table S9.

Fig. 6.

Variable-temperature ¹⁷O NMR [a; ln(1/T_1r) diamonds, ln(1/T_2r) squares] and ¹H nuclear magnetic relaxation dispersion (b; 298 K squares, 310 K circles) data of Gd₂L¹ in the absence of Ca²⁺. The curves correspond to the simultaneous fit as explained in the text

Table 1.

Kinetic and structural parameters obtained from the fit of ¹⁷O NMR and NMR dispersion (NMRD) data for the Gd₂L¹ complex in the absence and in the presence of Ca²⁺, compared with those of gadolinium 1,4,7,10-tetraazacyclododecane-1,4,7,10-tetraacetic acid (GdDOTA)

Parameters	Gd2L1 17O NMR + NMRD	Gd2L1 + Ca2+ 17O NMR	GdDOTA 17O NMR + NMRDa
k298ex (106 s−1)	2.4 ± 0.2	7.5 ± 1.6	4.1
ΔH‡ (kJ mol−1)	43.6 ± 3.3	43.6	49.8
ΔS‡ (J mol−1 K−1)	+23.5	+33.0	–
τ298RO (ps)	350 ± 50	1,150 ± 250	77
Er (kJ mol−1)	24 ± 1	21 ± 6	16.1
τ298v (ps)	20.6 ± 2.7	0.13 ± 0.02	11
Δ2 (1020 s−2)	0.46 ± 0.10	0.50 ± 0.05	0.16
q	0.4	0.7	1
q2 ns	1	1	–
r2981 (mM−1 s−1) 20 MHz	5.74	6.13	4.74

Parameters in italics were fixed during the fitting

^aReference [49]

The water exchange of Gd₂L¹ is slightly slower than that found for gadolinium 1,4,7,10-tetraazacyclododecane-1,4,7,10-tetraacetate (GdDOTA) [49]. It is interesting to note that for the monohydrated Gd³⁺ complex of the DO3A ligand bearing an N-linked CH₂CH₂NHCO-pyridyl pendant arm, much faster water exchange has been reported (k²⁹⁸_ex = 1.1 × 10⁸ s⁻¹) [43]. This fast water exchange has been explained by the steric destabilization of the Ln–water binding interaction by the presence of the bulky substituent. Such a destabilization effect is expected to be less important in Gd₂L¹ since the amide carbonyl is linked to a flexible –CH₂–N– moiety in contrast to the direct attachment to a pyridyl group in the previous case. This limited steric constraint around the water binding site will then be translated by a more sluggish water exchange as observed for Gd₂L¹. The more than 1 order of magnitude difference in the water exchange rate between Gd₂L¹ and the Gd³⁺ complex of the DO3A–CH₂CH₂NHCO–pyridyl ligand is a good example of the importance of the steric compression around the water binding site. Steric compression is indeed the main factor determining the rate of exchange in a dissociative water exchange mechanism, characteristic of nine-coordinate complexes [50]. The dissociative activation mode for Gd₂L¹ is indicated by the positive value of the activation entropy.

The higher τ_r value of Gd₂L¹ compared with that of GdDOTA is rationalized by the higher molecular weight and greater rigidity of the molecule. In spite of the slow rotational motion and the presence of a second hydration sphere in the bismacrocyclic system, the relaxivities are only slightly higher than those of GdDOTA, owing to the low hydration number of Gd₂L¹ (q = 0.4).

The simultaneous fit of the ¹H and ¹⁷O relaxation rates also supplies parameters that describe the electron spin relaxation of the Gd³⁺ complexes, such as τ_v, the correlation time for the modulation of the zero field splitting, its activation energy, E_v, and the mean zero field splitting energy, Δ². The values obtained for Gd₂L¹ are in the usual range for similar complexes [49].

The ¹H NMRD and ¹⁷O NMR data of the Gd₂L¹ system containing Ca²⁺ could not be fitted simultaneously because the conditions for ¹⁷O NMR and ¹H NMRD samples differed significantly in terms of the ionic strength and viscosity, and also in the Ca²⁺-to-Gd³⁺ ratio of the samples. Only the ¹⁷O ln(1/T_1r) and ln(1/T_2r) data were analyzed, and the relaxivities are given in the electronic supplementary material. The ¹⁷O relaxation rates were interpreted by using the same set of equations as for the system without Ca²⁺ (Fig. 7). Analogously, the same parameters were fixed in the fit (except for the hydration number, q  = 0.7, obtained from luminescence studies). The best fitting was obtained with the parameters listed in Tables 1 and S10. On Ca²⁺ addition, the most flagrant change is observed for the rotational correlation time as obtained from the ¹⁷O longitudinal relaxation rates (τ_R = 350 compared with 1,150 ps without and with Ca²⁺, respectively). We attribute this significant increase mainly to the high ionic strength and viscosity of the ¹⁷O NMR sample containing 1 M CaCl₂, though some rigidification of the molecule on Ca²⁺ is also expected. The water exchange rate triples on Ca²⁺ binding (k²⁹⁸_ex = 2.4 × 10⁶ compared with 7.5 × 10⁶ s⁻¹). The decreasing negative charge of the Ca²⁺-bound Gd₂L¹–Ca complex would be rather expected to diminish the exchange rate in a dissociatively activated process. Among other factors that can compensate this effect, the increased steric demand of the central part after Ca²⁺ binding can lead to a steric destabilization of the Ln–water binding interaction. Overall, the relaxivity increase upon Ca²⁺ addition is mainly related to an increase in the hydration number.

Fig. 7.

Variable-temperature, reduced longitudinal (squares) and transverse (circles) ¹⁷O relaxation rates for Gd₂L¹, in the presence of 1 M Ca²⁺. The curves correspond to the fit as explained in the text

Conclusion

With the objective of Ca²⁺ sensing by MRI in the extracellular space, we synthesized two novel bismacrocyclic ligands containing a Ca²⁺-sensitive BAPTA-bisamide moiety (Scheme 1). Their Gd³⁺ complexes exhibit relaxivities comparable to that of currently used monomeric MRI contrast agents, in accordance with their larger size but lower hydration numbers (q < 1). Upon Ca²⁺ addition, the relaxivities of Gd₂L¹ and Gd₂L² increase by 15 and 10%, respectively. These changes are likely insufficient for in vivo MRI detection. Gd₂L¹ is practically insensitive towards Mg²⁺. The apparent association constants for the Ca²⁺ interaction were obtained from the relaxometric titration curves. They are several orders of magnitude lower for our complexes than those determined for BAPTA⁴⁻ itself or other tetracarboxylate BAPTA⁴⁻ analogues. The hydration number of Eu₂L¹ was determined from luminescence lifetime measurements in the absence (q = 0.4) and presence (q = 0.7) of Ca²⁺. UV–vis measurements confirmed the presence of two coordination environments that we attribute to a monohydrated and a nonhydrated state (Fig. 5). Upon the coordination of Ca²⁺ in the central part of the ligand, the molecule undergoes a conformational change and an acetate arm, originally coordinated to the Ln³⁺ ion, binds to Ca²⁺ and leaves space for the coordination of a water molecule to the lanthanide, which shifts the hydration state towards the more hydrated species.

Electronic supplementary material

Below is the link to the electronic supplementary material.