A biology-based approach for mixture toxicity of multiple endpoints over the life cycle

Abstract

Typical approaches for analyzing mixture ecotoxicity data only provide a description of the data; they cannot explain observed interactions, nor explain why mixture effects can change in time and differ between endpoints. To improve our understanding of mixture toxicity we need to explore biology-based models. In this paper, we present an integrated approach to deal with the toxic effects of mixtures on growth, reproduction and survival, over the life cycle. Toxicokinetics is addressed with a one-compartment model, accounting for effects of growth. Each component of the mixture has its own toxicokinetics model, but all compounds share the effect of body size on uptake kinetics. The toxicodynamic component of the method is formed by an implementation of dynamic energy budget theory; a set of simple rules for metabolic organization that ensures conservation of mass and energy. Toxicant effects are treated as a disruption of regular metabolic processes such as an increase in maintenance costs. The various metabolic processes interact, which means that mixtures of compounds with certain mechanisms of action have to produce a response surface that deviates from standard models (such as ‘concentration addition’). Only by separating these physiological interactions from the chemical interactions between mixture components can we hope to achieve generality and a better understanding of mixture effects. For example, a biology-based approach allows for educated extrapolations to other mixtures, other species, and other exposure situations. We illustrate our method with the interpretation of partial life-cycle data for two polycyclic aromatic hydrocarbons in Daphnia magna.

Electronic supplementary material

The online version of this article (doi:10.1007/s10646-009-0417-z) contains supplementary material, which is available to authorized users.

Introduction

Understanding and predicting the effects of chemical mixtures is one of the great challenges of ecotoxicology and environmental risk assessment. Currently, toxicity data for mixtures are almost always analyzed using descriptive methods. For example, the framework presented by Jonker et al. (2005) allows for analyzing patterns in the data and significance testing of statistical interactions (i.e., deviations from some standard model). Such approaches may be useful as a first step, but the descriptive nature precludes a mechanistic interpretation of the results, and therefore does not provide a better understanding of mixture toxicity. Such an understanding is not only crucial from a scientific perspective, but also to make useful predictions. Clearly, it is impossible to experimentally test the toxicity of all mixtures, for all organisms, and for all relevant exposure conditions. The descriptive nature of current mixture approaches is perhaps best illustrated by the following unanswered questions. Firstly, the apparent effect of a mixture may change in time. Only very few studies actually consider this aspect, but when they do, it is clear that the conclusion on the combined effect depends on the selected exposure time (Baas et al. 2007; Van Gestel and Hensbergen 1997). Why does the mixture effect (including the interactions) change in time? Secondly, the apparent effect of a mixture can differ between endpoints in chronic tests, such as growth and reproduction (Cedergreen and Streibig 2005; Van Gestel and Hensbergen 1997). Different endpoints are a part of the response of the same individual organisms, so why does the mixture effect differ between endpoints? Few studies have so far followed mixture effects in time for multiple endpoints, but the questions posed are probably relevant to all mixtures and all organisms; especially because single toxicants already show effect patterns that change in time and differ between endpoints (Alda Álvarez et al. 2006b; Jager et al. 2006). It must be stressed that a descriptive dose–response analysis is never going to provide an answer to these questions.

To progress our understanding of mixture toxicity, we need to go beyond the use of descriptive methods. Instead of focusing on the infinite number of possible mixture combinations and exposure situations, it makes more sense to focus on the large but finite number of biological processes, and develop the models to incorporate those processes (Yang et al. 2004). Biology-based approaches are required that make explicit assumptions regarding the mechanisms governing the toxic response (OECD 2006). So far, the few attempts for a biology-based approach in mixture ecotoxicity have been restricted to the endpoint survival. Several approaches depart from the critical body residue concept (Lee and Landrum 2006; McCarty et al. 1992), and more recently, Baas et al. (2007) presented an approach based on hazard modeling, describing the entire effects surface in time.

For endpoints other than mortality, biology-based approaches for mixtures are lacking. Understanding toxic effects on growth and reproduction requires a quantitative framework for feeding, and how food is used to fuel metabolic processes such as growth, maintenance, development and reproduction. These processes are tightly coupled in organisms through the conservation laws and the rules for metabolic organization, and cannot be understood in isolation. Modeling sub-lethal effects thus requires quantitative assumptions on energy budgets, and assumptions on how the metabolic processes are affected by toxicants (Jager et al. 2006). For sub-lethal effects, the only biology-based approach currently available is the DEBtox method (Jager et al. 2006; Kooijman and Bedaux 1996). This method has been successfully applied to analyze effect patterns of single toxicants on the life-cycle of various organisms (Alda Álvarez et al. 2006b; Jager et al. 2004), also in combination with abiotic stressors such as food limitation (Pieters et al. 2006). In this contribution, we extend the DEBtox approach to deal with mixtures of toxicants. To illustrate this biology-based approach towards mixture toxicity, we analyze and interpret a dataset for two polycyclic aromatic hydrocarbons (PAHs) in Daphnia magna.

Theory

Ecotoxicodynamics

Any biology-based approach for the analysis of toxicity data should consider toxicokinetics (going from external concentration to target site) and toxicodynamics (going from target site to effects on specific endpoints). This idea is well established in mammalian toxicology, which provides excellent examples of mixture toxicokinetics and toxicodynamics (e.g., El-Masri et al. 1996; Krishnan et al. 2002). In ecotoxicology, however, toxicodynamics is rarely investigated quantitatively, which probably relates to the interest in life-history endpoints such as growth, reproduction and survival. To understand such highly integrated responses, it is essential to have a theoretical framework that links feeding, growth, development and reproduction over the life cycle. For our ecotoxicodynamics model, we focus on dynamic energy budget (DEB) theory (Kooijman 2000, 2001; Nisbet et al. 2000). This theory explains how individuals acquire and use resources over their life cycle, based on a set of simple rules for metabolic organization. Within this theory, organisms are treated as dynamic systems with explicit mass and energy balances. DEB theory formed the basis of the DEBtox approach for sub-lethal effects (Kooijman and Bedaux 1996), as included in OECD guidance (OECD 2006). The DEBtox method was extended to the simultaneous analysis of life-cycle endpoints by Jager et al. (2004).

In the DEBtox approach, toxicant effects are treated as a change in a parameter of the metabolic machinery (Fig. 1), for example, as an increase in the maintenance costs or a decrease in the assimilation of energy from food. In principle, any DEB parameter may be affected by a chemical. The DEB rules subsequently establish how a change (over time) in such a parameter affects growth, development and reproduction over the life cycle. If detailed toxicity data are available, it is usually possible to identify the affected process(es). A change in each DEB parameter has specific consequences for the life cycle, what can be called a physiological mode of action (Alda Álvarez et al. 2006a).

Fig. 1.

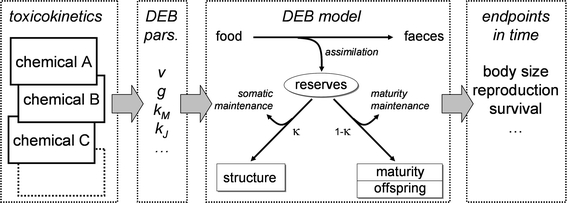
Conceptual scheme of the biology-based approach for mixture toxicity. Symbols for DEB parameters are explained in Table 1

Mixture concepts in a DEB context

Figure 1 shows the basic structure of the DEB approach for mixture toxicity. Each component of the mixture has its own toxicokinetics module, which implies that exposure to a constant mixture composition will generally lead to a time-varying mixture inside the organism. A chemical may interact with one or more sites of action within an organism; two toxicants may affect the same target site or different sites. The disruption at the site(s) of action will imply a change in one or more metabolic parameters. Two chemicals in a mixture may affect the same metabolic process (through the same or different target sites), or different processes (necessarily through different target sites). The DEB allocation rules specify the consequences of these changing parameter values over the life cycle, resulting in predictions for survival, growth and reproduction. DEB theory also provides the handles to analyze effects on other endpoints such as respiration or product formation (see Kooijman 2001). A mixture analysis in DEB context is therefore quite straightforward, conceptually.

The complexity of mixture (eco)toxicology lies in the potential for interaction between the mixture constituents. In descriptive mixture analysis, interactions are identified from the misfit of a particular reference model (e.g., a log-logistic dose response, coupled to ‘concentration addition’) to the response of an endpoint after some exposure time. In a biology-based approach we aim to understand the origin of these interactions, and therefore have to distinguish between two very different forms of interaction; chemical and physiological ones. In the realm of chemical interactions, compounds may interfere with each others toxicokinetics, by modifying each others bioavailability in the medium, or each others uptake into the organism. Inside the body, compounds may additionally reveal chemical interactions when they affect the same target site, or when they are biotransformed by the same mechanism. Such chemical interactions will be highly toxicant- and species-specific, and are thus difficult to generalize. We cannot therefore a priori include them into our model; the inclusion of these interactions requires knowledge on the environmental chemistry and biochemistry of the mixture of interest.

In contrast with the chemical interactions, physiological interactions are already included in our approach. As a consequence of the energy and mass balance in DEB theory, these interactions are in fact unavoidable as metabolic processes interact in their effect on growth and reproduction. For example, maintenance and somatic growth compete for the same share of the allocated reserves (see Fig. 1), which explains the apparent ‘synergistic’ interaction between certain toxicants and food limitation (Pieters et al. 2006). Furthermore, body size determines feeding rates and the initiation and rate of reproduction, and body size affects toxicokinetics (Eq. 1). If a chemical affects growth of the exposed organisms, it thereby automatically affects the toxicokinetics of all mixture components, and their effects on reproduction. These physiological interactions are not the direct consequences of the interactions between stressor molecules or between stressors and target sites, but interactions between physiological processes within the organisms. As such, these interactions are not chemical properties, but properties of the organism and thus should be covered by DEB theory.

The strength of our biology-based approach for mixture analysis lies in the possibility to separate chemical from physiological interactions. The model specified in this paper makes strong predictions on the mixture effects, based on the behavior of the single components and assumptions about metabolic organization. If the observed response of an endpoint differs from these expectations, the nature of the deviations should provide insights into the chemical interactions that may be underlying this response. Subsequently, we can design specific experiments to test these hypotheses, e.g., using (bio)chemical measures. If we can separate chemical from physiological interactions, we can finally hope to elucidate the similarities and differences between species and between chemical groups.

Perhaps counter-intuitively, it is the misfits from the model that will provide most information. It must be stressed that it is not our aim to provide an accurate description of a set of data, but rather to use the data to withdraw information on the underlying mechanisms of mixture toxicity. The classical approach of applying statistical interaction terms on the entire dose–response curve provides little insight into underlying mechanisms. Such descriptive methods may identify ‘interactions’ that result from rather straightforward physiological processes, such as the effects of growth on toxicokinetics and the need for energy and mass balance. Thereby, descriptive methods tend to confuse the links with underlying mechanisms, rather than clarify them. Besides, it is quite possible that the observed interactions are artifacts of applying descriptive curves to the response at a single time point (Baas et al. 2007), which may also explain the lack of reproducibility of specific interactions (Cedergreen et al. 2007).

Model implementation

The set of DEBtox equations as given by Kooijman and Bedaux (1996) followed from a simplification of DEB theory to facilitate calculations: the reserve density was assumed constant, and the length at the start of reproductive investment was fixed, just as the costs for an egg. Even though these simplifications were sensible ones, they restrict our choice of metabolic parameters that can change under the influence of toxic stress. For example, increasing the costs for somatic maintenance requires an equivalent increase in the costs for maturity maintenance to maintain a constant length at the start of reproduction. The most flexible solution is to implement a full set of DEB equations for a generic animal, with explicit calculation of the maturation process and egg costs, as was presented in detail by Kooijman et al. (2008).

The set of parameters for an ectothermic DEB animal is given in Table 1, with default values for Daphnia magna. This choice of parameters differs slightly from the list provided in Kooijman et al. (2008) to ensure that all parameters have dimensions in length and time only. We chose to treat the ratio of maturity and somatic maintenance as a parameter, as well as the ratio of the scaled maturity at puberty and birth. The reason is that these ratios are likely species-specific, and will not differ too much between experiments, whereas the absolute values will. With the change from simplified to non-simplified DEB equations, the easily interpretable compound parameters such as the maximum size and maximum reproduction rates have been replaced by more abstract parameters. However, these more abstract parameters are more closely related to the actual physiological processes, facilitating the implementation of stressor effects on every metabolic parameter. The easily measured compound parameters derive in a simple manner from the primary parameters (Kooijman et al. 2008).

Table 1.

Choice of DEB parameters for ecotoxicity purposes, defaults for Daphnia magna (Kooijman et al. 2008), and fits on controls with likelihood-based confidence intervals

Symbol	Description	Defaults Daphnia	Fits (95% CI)
g	Energy investment ratio	0.422 [–]	n.e.
v	Energy conductance	3.24 mm d−1	1.96 (1.7–2.2) mm d−1
k M	Somatic maintenance rate coefficient	1.71 d−1	1.51 (1.3–1.8) d−1
k	Ratio of maturity and somatic maintenance rate coefficient	1 [–]	n.e.
κ	Allocation fraction to soma	0.80 [–]	0.551 (0.40–0.67) [–]
κ R	Reproduction efficiency	0.95 [–]	n.e.
U pH	Scaled maturity at puberty	0.366 mm2 d	0.966 (0.61–1.4) mm2 d
U bH/U pH	Maturity at birth, relative to puberty	0.0328 [–]	n.e.
f	Scaled ingestion rate	1 [–]	n.e.

n.e. not estimated

Approach for single compounds

A toxicant first needs to be taken up from the environment (and transported to the target site) before it can exert an effect. The first step in the analysis of toxic effects is therefore a toxicokinetics model. Because toxicity tests do not contain a lot of information on toxicokinetics, we stick to a simple scaled version of the one-compartment first-order model, accounting for growth of the organism (Kooijman and Bedaux 1996). Growth affects toxicokinetics by growth dilution (the last term in Eq. 1), and by changing the surface:volume ratio of the organism (the elimination rate k _e is inversely proportional to body length, L).

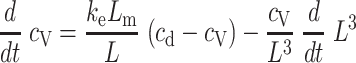1

The maximum length in the blank (L _m) is not a parameter, but follows from the primary parameters of Table 1: L _m = v/(k _M g). The scaled internal concentration (c _V) is the actual (but unknown) body residue divided by the (also unknown) bioconcentration factor (for the relevant target tissue). Thus, c _V is directly proportional to the real body residue, but it has the dimensions of the external concentration in the medium (c _d). The scaled internal concentration in steady state thus equals the external concentration. Thereby, we only have one toxicokinetics parameter to estimate from the toxicity data: the ‘elimination rate’. This rate does not necessarily reflect whole-body residues; it may instead reflect the relevant kinetics at a specific target site (Jager and Kooijman 2005, 2009).

Effects on growth and reproduction are viewed as an effect of the toxicant on the acquisition or use of resources. We introduce a ‘stress level’ (s) to link the scaled internal concentration to the value of a metabolic parameter of the DEB model (Kooijman and Bedaux 1996):

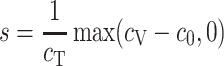2

As long as c _V is below the threshold value c ₀ (the no-effect concentration, or NEC), the stress level is zero. Above c ₀, the stress increases proportional to the concentration above the threshold. Parameter c _T can be interpreted as a tolerance (the higher its value, the lower the effect on the DEB parameter per unit of concentration above the threshold). Because c _V is the scaled internal concentration, c ₀ is a scaled threshold and c _T a scaled tolerance concentration, all with the dimensions of an external concentration.

A selection of possible modes of action is given in Table 2, and shows how the stress level of Eq. 2 is used to alter model parameters. It should be noted that k _M and g are compound parameters (Kooijman et al. 2008), which means that changes in the costs for growth affect k _M, and changes in κ affect g. Other modes of action can be envisaged, and combinations of these modes of action may also occur in practice. The implementation of the more extensive DEB formulation (Kooijman et al. 2008) allows for maximum freedom in the choice of target parameter. For example, effects on κ can now be calculated, which requires the explicit calculation of maturity.

Table 2.

Examples of physiological modes of action in DEB, where s stands for a stress factor(s) that modifies the value of a metabolic parameter

Mode of action	Affected parameter(s)
Decrease of ingestion rate	f → f (1 − s)
Increase in somatic maintenance	k M → k M (1 + s)
Increase in maturity maintenance	k J → k J (1 + s)
Increase in costs for structure (growth)	g → g (1 + s) and k M → k M/(1 + s)
Increase in reproduction costs	κ R → κ R/(1 + s)
Hazard to the developing embryo	κ R → κ R exp(−s)
Change in allocation	κ → κ (1 ± s) and g → g/(1 ± s)

We treat effects on survival (or immobility) in a similar fashion, assuming that the probability to die (through the hazard rate) increases proportional to the scaled internal concentration above a threshold (Bedaux and Kooijman 1994; Kooijman and Bedaux 1996). The proportionality constant is called the killing rate (b). The biology-based analysis of survival data for mixtures has been presented earlier by Baas et al. (2007). The method for survival data in growing animals, as required for life-cycle studies, extends the approach of Baas et al. in the sense that growth and body size influence toxicokinetics (Eq. 1).

Implementation of combined effects

We have to distinguish between chemicals that act on the same target and chemicals that act on different targets. If compounds affect the same target site, they can be treated like dilutions of each other, once they are taken up. Their toxicokinetics follows from applying Eq. 1 for each chemical, with independent values for k _e, but with the same growth pattern (L as function of time). Subsequently, we can sum the scaled internal concentrations with a weight factor (W):

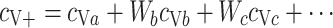3

Here, substance a is taken as the (arbitrary) reference compound for the weight factors for compounds b and c. The weight factor represents the overall efficiency with which a compound is taken up, reaches the target, and interacts with it (relative to compound a). For narcotics, we can expect the weight factors to reflect the differences in bioconcentration factors. The assumed target is the cell membrane, and what matters is the total number of molecules in there, not whether they belong to compound a or b. As a consequence, the NECs and tolerance concentrations of compounds acting through the same target cannot be independent, but are linked through the weight factor (see Jager and Kooijman 2009). A high efficiency for reaching the target results in a low NEC and a low tolerance. Because the compounds act on the same target, they also necessarily affect the same metabolic process. The total concentration (c _V+) is the total internal concentration in toxicant a equivalents, and is used to calculate the total stress level s ₊ (through Eq. 2), which in turn is used to calculate the stress on the metabolic process in Table 2. For example, if two compounds affect feeding rate through the same target site, the scaled ingestion rate under stress (f _s) becomes:

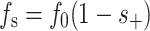4

If compounds affect different targets, they can have fully independent NECs and tolerance concentrations. For all components of the mixture, the internal concentrations are calculated independently (through Eq. 1), as well as their stress level s (Eq. 2). The stress functions in Table 2 are applied independently in the DEB model. The different targets may be linked to the same metabolic process (the DEB parameters in Fig. 1) or to different ones. If they affect the same process, the stress functions in Table 2 are multiplied. For example, if compounds a and b affect feeding rate through independent targets:

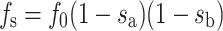5

This will lead to a different response than the ‘same-target’ assumption in Eq. 4. If the compounds affect different processes (necessarily through different targets), the stress functions from Table 2 can simply be applied independently in the DEB model. The rules for metabolic organization ensure that the combined effect is calculated in a consistent manner.

It should be noted that despite the conceptual similarities, the ‘same-target’ assumption in our method does not necessarily produce the same response surface as classical ‘concentration addition’, and the ‘different-target’ assumption will not necessarily equal the classical ‘independent action’ calculation. Apart from the fact that the classical methods do not provide a framework for effects on multiple endpoints over time, the main conceptual difference is that the classical methods apply their concentration or effect addition on the observed dose–response curve. In contrast, we apply these concepts at the level of metabolic parameters. Depending on the affected metabolic processes, the model predictions from our approach will deviate from the classical models as a result of the natural interactions caused by the need to preserve energy and mass balance (see Fig. 1), and by the effects of growth on toxicokinetics (Eq. 1).

The method we present is not specific for any particular animal. However, in our set of primary parameters, we ignored surface-related maintenance costs, which would be of particular relevance for endothermic animals (heating). Kooijman et al. (2008) provide guidance to extract the full set of DEB parameters from experimental data. The method also has no restrictions to the number of chemicals in the mixture, and allows for any combination of affected target sites. Furthermore, no specific changes are needed to deal with time-varying exposure or time-varying mixture composition (which only implies that in Eq. 1 c _d becomes a function of time).

Model calculations

The complete model was implemented in Matlab 7.3 (The MathWorks). Optimization was performed by maximizing the overall likelihood for all endpoints (see Jager et al. 2004) using a Nelder–Mead Simplex search. Confidence intervals on parameter estimates were generated using the profile likelihood (see Meeker and Escobar 1995).

Experiments with Daphnia magna

To test the biology-based mixture approach, we used a well-studied test organism (Daphnia magna), in a simple exposure medium (water), and a simple mixture of two PAHs (pyrene and fluoranthene). For D. magna, a representative set of defaults for the DEB parameters is already available (Table 1). The selected test compounds presumably share a (narcotic) mode of action, and are probably not metabolized to any great extent. The reason for selecting such a simple mixture is as ‘proof of concept’. If our approach is not able to describe the effects on all endpoints for this mixture, we have obviously missed an important step in the process, and may require additional experimentation. On the other hand, when our approach is indeed successful, we can confidently tackle more complex mixtures. More complex mixtures (e.g., metals and compounds that are biotransformed) and more complex exposure media (e.g., soil and sediment) will undoubtedly require additional model assumptions and interaction mechanisms, and thus require more detailed information than available in simple toxicity tests.

A clone of D. magna (kept under laboratory conditions for many years) was used to perform these toxicity tests. Approximately 20 organisms were kept in 1 L glass recipients containing aerated and bio-filtered tap water for daily culturing. They were held at a constant temperature of 20 ± 1°C and a photoperiod of 14 h light/10 h dark. The medium of the cultures was renewed three times a week and the water fleas were fed a mixture of Pseudokirchneriella subcapitata and Chlamydomonas reinhardtii in a 3:1 ratio (4 × 10⁵ cells/mL).

Exposure was conducted with two PAHs, fluoranthene and pyrene (Sigma–Aldrich, Belgium). Concentrations of the individual component exposures were set to 0, 0.06, 0.12 and 0.25 Toxic Units (TU), where one TU was defined as the EC50 for immobility at 48 h (when fed) of the single compound. For the binary mixtures, concentrations for a total of six combinations were determined based on a fixed ratio design (Fig. 2). Test solutions were made in OECD standard water (OECD 1992). Since both fluoranthene and pyrene were dissolved in acetone, final concentrations of 0.01% acetone were used. Besides the pure OECD water control, an additional solvent control was included. Every other day, when test solutions were renewed, organisms were fed with a mixture of P. subcapitata and C. reinhardtii in a 3:1 ratio (4 × 10⁵ cells/mL).

Fig. 2.

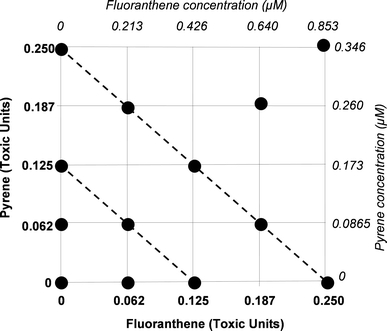
Overview of the treatments in the toxicity experiment with Daphnia magna. Toxic units based on EC50 for immobility after 2 days

Chronic tests (21 days) were performed in which survival, growth and reproduction were monitored (immobility was used as a proxy for death), starting with neonates (less than 24 h old). To evaluate survival and reproduction, ten organisms per treatment were exposed individually in 100 mL test containers. Every other day, survival and reproductive success (time to first brood; number of juveniles produced) were recorded. To assess growth, an additional set of organisms (60 organisms/treatment) was used. These daphnids were kept in a 600 mL recipient with the same algal concentration as for reproduction. Every other day, five organisms were taken out, stored in a sucrose–formaldehyde solution (4% formaldehyde; 12% sucrose) and measured (distance of carapax from head to spine) by means of a microprojector (Projectina, Switzerland).

Results and discussion

Quite a number of parameters need to be estimated from the data (Tables 1, 3). However, the data also contain a wealth of information, as one parameter set needs to describe body size, reproduction and survival simultaneously over time. Finding accurate starting values is essential, and requires a stepwise approach as clarified in the following sections.

Table 3.

Parameter estimates for the toxicological parameters, resulting from the model fits of Fig. 3, with 95% likelihood-based confidence intervals

Symbol	Parameter	Pyrene	Fluoranthene
k e	Elimination rate constant	0.195 (0.142–0.288) d−1	0.0842 (0.0513–0.120) d−1
W	Weight factor	1 (n.e.)	1.10 (0.896–1.54)
c 0s	NEC for survival	0.386 (0.341–0.428) μM
b	Killing rate	2.53 (1.77–3.66) μM−1 d−1
h	Blank hazard rate	0.0134 (0.00960–0.0184) d−1
c 0	NEC for reproduction costs	0.128 (0.114–0.140) μM
c T	Tolerance for reproduction costs	0.0160 (0.0126–0.0189) μM
c 0	NEC for growth costs	0.186 × 10−3 (0–0.134) μM
c T	Tolerance for growth costs	0.833 (0.696–1.02) μM

For the additional effect on growth costs, instantaneous steady state of the relevant internal concentration is assumed

Control response

We started by fitting the control response of growth and reproduction (control and solvent control combined as there was no clear solvent effect). Because the information on growth and reproduction at one food level is insufficient to fit all DEB parameters (Kooijman et al. 2008), we fixed several parameters to representative defaults for D. magna (Table 1). The estimated parameters (Table 1) deviate slightly from the defaults, which can be caused by the experimental setup (e.g., food quality), but also by the measure of body length that is used (for parameters that have length in their dimensions). These physiological parameters are fixed in the analysis of the response to toxicants to minimize the degrees of freedom of the model, and thereby facilitate the identification of deviations from the model predictions (which may indicate unexpected interactions in the mixture).

Single exposures

The second step is to focus on the results for the single PAH exposures, extracting initial parameter values for the mixture components. For survival, the single exposure data show little dose-related mortality (for pyrene no single dose leads to more than 50% mortality, and for fluoranthene only one dose). To improve our data basis for effects on survival, we decided to also take the acute range-finding data into account (2 days exposure, with food). The model was fit simultaneously to the acute and the chronic data set, with the same parameter values.

For the sub-lethal effects, there is little or no effect on body size, little effect on the start of reproduction, but a large effect on reproductive output (Fig. 3). This indicates a direct effect on reproduction, and an increase in the costs for reproduction (Table 2) describes this pattern best. However, a small effect on body size is apparent, especially in the mixed exposures (which reach higher combined concentrations than the single exposures, see Fig. 2), and cannot be ignored. Body size affects toxicokinetics (Eq. 1) as well as reproductive behavior, which means that growth must be described as accurately as possible. The pattern in the body size data is best described by assuming an additional effect on the costs for structure with very rapid toxicokinetics (an infinite value for k _e). Combined costs for structure and costs for reproduction has been observed in the biology-based analysis of another narcotic compound (pentachlorobenzene) in a nematode (Alda Álvarez et al. 2006b). Interestingly, the effects on reproduction costs and survival indicates slow toxicokinetics (a low k _e value, Table 3), whereas the effects on costs for structure appear much more rapidly. As explained above, k _e reflects the toxicokinetics at the site of action, and it is conceivable that these compounds have more than one site of action, with distinctly different properties. We can speculate that the effect on reproductive costs is related to PAHs accumulating in lipid membranes, whereas the effect on structural costs may relate to a receptor in the aqueous phase.

Fig. 3.

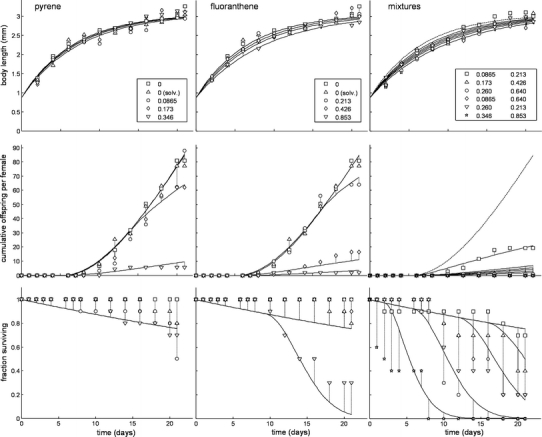
Simultaneous model fit for the effects of a binary PAH mixture on three endpoints of Daphnia magna. Dotted lines connect observations to model lines; broken lines in the mixture data indicate the blank response as reference. Concentrations in the legends are in μM. Fit for the survival data from the acute range-finding test given in supplementary material

The two PAHs have the same physiological mode of action (the same target DEB parameters), and can be described with a similar set of parameters values, which is not surprising, given their structural similarity and comparable hydrophobicity. These values act as starting values for the mixture analysis.

Mixture exposures

Finally, the entire data set is fitted, using the starting values for the toxicological parameters from the single exposures, and fixing the physiological parameters to the values obtained from the controls (Table 1). Given the similarity of the effect patterns of pyrene and fluoranthene, the ‘same target’ approach seems most likely, and indeed provides the best explanation of the mixture effects (the fit of the ‘different target’ model was clearly worse). Assuming that the two PAHs act through the same mechanism, this implies that the compounds can be viewed as dilutions of each other. Therefore, the toxicological parameters cannot vary independently. The NECs, killing rate and tolerance concentration should differ between the two compounds by a weight factor only (Eq. 3). The resulting parameter values are given in Table 3.

The graphical representation of the results of a biology-based mixture analysis is not straightforward, even for binary mixtures. The model describes a hyper plane in four dimensions (concentration A, concentration B, time and response), for three endpoints (survival, body size and reproduction). As a pragmatic solution, we plot the endpoints as a function of time, with separate plots for each endpoint, and separate plots for the single compound and the mixed exposures (Fig. 3).

The ‘same target’ assumption provides an excellent description for the mixture effects on growth and reproduction. The weight factor indicates that fluoranthene is slightly, but not significantly, more effective than pyrene in causing toxicity. The rather similar efficiency of both compounds probably relates to the similarity in hydrophobicity. The results for growth and reproduction do not suggest any form of interaction, apart from the interactions that are inherent in our approach. For survival, the fit is quite good, although the correspondence to the model predictions is perhaps less convincing than for growth and reproduction (for a clearer view, a larger number of survival plots is given in the supplementary material, including the fit on the acute range-finding test). However, it should be realized that the survival probabilities result from only ten animals per treatment. For one mixture combination (0.260 mM pyrene and 0.213 mM fluoranthene) there is a peculiar misfit of the model: there is considerable mortality that is not predicted by the model. This may be an interaction in this particular dose region, or perhaps survival requires other weight factors (Eq. 3) than sub-lethal endpoints, but without a full dose–response of pyrene this remains speculative.

Figure 4 shows the predicted iso-effect lines for 50% effect on survival and reproduction in time. This analysis shows that the effect of the mixture changes in time in a manner that depends on the endpoint (when expressed as 50% effect relative to the control). Such straight iso-effect lines will also result from classical concentration addition. The correspondence with concentration addition occurs in this particular case, as our PAHs have very similar elimination rates, and have little effect on body size. For other compound combinations, the ‘same target’ assumption in the biology-based approach will result in larger deviations from straight iso-effect lines.

Fig. 4.

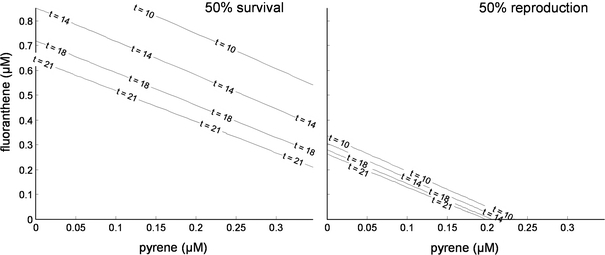
Iso-effect lines for survival and reproduction, interpolated based on the fit of Fig. 2. Lines represent the 50% effect level at varying time points

We wanted to compare our analysis to the results of the approach of Jonker et al. (2005) at the last time point. However, this statistical approach is not informative with this particular data set because for body size the effect is too small, for reproduction there is only one mixture dose with a non-zero response, and for survival one of the compounds (pyrene) does not have a dose–response relationship in the single exposures. Our biology-based approach can still withdraw information out of this data set because all of the data points in time, and the different endpoints, are used together in an integrated manner. This illustrates the advantage of a biology-based approach to make better use of the available data.

Evaluation of the experimental setup

To test the biology-based approach for mixture toxicity, we deliberately selected a well-studied test organism, a simple exposure medium, and a simple mixture. This provides an excellent opportunity to comment on the most optimal experimental setup for a biology-based analysis. Clearly, a biology-based analysis requires a detailed data set (body size, reproduction and survival over a considerable part of the life cycle), as well as a thorough knowledge of the test animal (the defaults in Table 1). For D. magna, the current setup of a 21-day test with one observation every second day is basically sufficient. However, the number and spacing of the single exposures and the mixture combinations requires careful deliberation. In our experiment, it would have been more informative had higher concentrations of the single components been tested (to better analyze effects on survival and growth), and mixtures with less sub-lethal effects (to yield more mixture combinations with a partial effect on reproduction).

More complicated mixture setups (compounds that are metabolized, specific modes of action, complex matrices such as soil or sediment) require a more elaborate set of experiments. In general, we can say that one needs to understand the single compounds in sufficient detail before attempting a biology-based analysis of a mixture. If the single compounds yield effect patterns that the model predictions cannot match, this needs to be addressed first (which may necessitate additional experiments). For more complex exposure media (e.g., soil and sediment), it is important to understand the chemical interactions that may occur in the medium before turning to the actual toxicity of the mixture. Otherwise, it will be impossible to decide whether observed interactions are the consequence of processes outside or inside the organism. Isolating these processes is of crucial importance to understand mixture toxicity, to compare results between different groups of organisms (e.g., aquatic versus soil-dwelling), and to extrapolate between exposure media (e.g., different soil types).

Conclusions

The analysis of sub-lethal mixture effects requires an ecotoxicodynamic approach capable of explaining the relations between the processes of feeding, maintenance, growth, development and reproduction. In this paper we present a biology-based method for the simultaneous analysis of mixture effects on growth, reproduction and survival in (partial) life-cycle experiments. We feel that such an integrated analysis is indispensable to gain mechanistic insights from toxicity data, and ultimately predict the toxicity of untested mixtures or untested exposure situations (e.g., time-varying concentrations or food limitation). In this modeling framework, the inevitable physiological interactions between different metabolic processes, and between these processes and toxicokinetics, are explicitly and quantitatively included. Deviations from the model predictions will help to identify other interaction mechanisms and guide further research.

The dataset for the combination of fluoranthene and pyrene illustrates how experimental data are used in a biology-based approach, and demonstrates its feasibility. The results are clearly consistent with the assumption that these PAHs have the same physiological mode of action, and act through the same target site. A relatively small set of parameters (four basic physiological parameters and ten toxicological ones) is estimated from the data, which is sufficient to explain the effect patterns over time of both single and mixed exposure, on three endpoints simultaneously. Additionally, this analysis raised fundamental questions on the toxicity of PAHs that would not have been achieved using descriptive analysis (such as the nature of the small and rapid effect on growth).

This is the first biology-based mixture analysis for sub-lethal effects. Clearly, more datasets need to be analyzed to increase confidence in the method. Nevertheless, we are convinced that understanding the effects of mixtures cannot be achieved by descriptive methods, but requires a biology-based perspective. This study is therefore a crucial first step to underpin that conviction.