Intrinsic Domain and Loop Dynamics Commensurate with Catalytic Turnover in an Induced-Fit Enzyme

SUMMARY

Arginine kinase catalyzes reversible phosphoryl transfer between ATP and arginine, buffering cellular ATP concentrations. Structures of substrate-free and -bound enzyme have highlighted a range of conformational changes thought to occur during the catalytic cycle. Here, NMR is used to characterize the intrinsic backbone dynamics over multiple timescales. Relaxation dispersion indicates rigid-body motion of the N-terminal domain and flexible dynamics in the I182-G209 loop, both at milli-second rates commensurate with k_cat, implying that either might be rate limiting upon catalysis. Lipari-Szabo analysis indicates backbone flexibility on the nanosecond timescale in the V308-V322 loop, while the rest of the enzyme is more rigid in this timescale. Thus, intrinsic dynamics are most prominent in regions that have been independently implicated in conformational changes. Substrate-free enzyme may sample an ensemble of different conformations, of which a subset are selected upon substrate binding, with critical active site residues appropriately configured for binding and catalysis.

INTRODUCTION

Arginine kinase (E.C. 2.7.3.3), a member of the phosphagen kinase family of enzymes, catalyzes reversible phosphoryl transfer between ATP and arginine, thus achieving cellular buffering of ATP levels in cells with high and/or variable energy demands (Ellington, 2001). It is chosen as a model for investigating enzyme dynamics due to its amenability to both high resolution crystallography and NMR spectroscopy.

The phosphagen kinase family of enzymes has been the focus of a large body of research resulting in significant advances in our understanding of bimolecular enzyme catalysis (Ellington, 2001). Steady-state enzyme kinetics have shown that arginine kinase undergoes a rapid equilibrium, random order bimolecular-bimolecular reaction, likely common to all phosphagen kinases, with a turnover number (k_cat) in the range of 100 to 150 s⁻¹ (Blethen, 1972; Gattis et al., 2004; Pruett et al., 2003). X-ray solution scattering experiments, which detected a decrease in the radius of gyration of arginine kinase upon substrate binding, were the first to indicate that substrate binding is accompanied by large conformational changes and raised the possibility that the change could be rate-limiting (Dumas and Janin, 1983).

X-ray crystal structures have provided the clearest view of the extent of conformational changes in Limulus polyphemus arginine kinase. Two structures of arginine kinase are available: one in a substrate-free, or ‘open’, form at 2.4 Å resolution, and one in a transition state analog complex, substrate-bound or ‘closed’, form at 1.2 Å resolution (Yousef et al., 2003; Yousef et al., 2002; Zhou et al., 1998). These structures show arginine kinase to be a monomeric, two-domain enzyme with a small, α-helical N-terminal domain (NTD) linked to a larger, primarily β-sheet C-terminal domain (CTD), with substrates binding in the cleft between these two domains. In the substrate-bound form, both N-terminal and C-terminal domains fold over the active site, along with a number of loops, analogous to a hand making a grabbing motion (Yousef et al., 2003).

Over the last decade, the concept of “dynamic domain” has been used increasingly to describe clusters of atoms whose conformational change can be approximated a common rotation/translation operator (Gerstein et al., 1994; Hayward, 1999; Hayward and Berendsen, 1998; Lee et al., 2003). A dynamic domain may include non-contiguous regions of linear sequence, so dynamic domains are an extension of the classical definition of domains as globular and linearly contiguous units. Analysis of substrate-free and transition state arginine kinase show the conformational differences can be approximated as rotations of three dynamic domains by up to 18° relative to a fixed domain with additional loop reconfigurations overlaid that bring catalytically important residues to the active site (Yousef et al., 2003). These changes may be involved in mediating substrate specificity, preventing wasteful hydrolysis of ATP, and precisely aligning substrates for phosphoryl transfer (Jencks, 1969; Jencks, 1975; Jencks and Mage, 1974; Lahiri et al., 2002).

There is growing evidence that enzymes bind substrates with a ‘conformational selection’ mechanism (Boehr et al., 2006; Boehr and Wright, 2008; Lange et al., 2008; Ma et al., 1999). In the absence of substrate, an equilibrium may exist between substrate-free states and ones that are closer to the substrate-bound conformation. Binding of substrates stabilizes the ligand-bound state, shifting the pre-existing equilibrium towards the ligand-bound form. Enzyme-substrate interactions in an ATP binding cassette (Wang et al., 2004), RNase A (Beach et al., 2005; Kovrigin and Loria, 2006), dihydrofolate reductase (Osborne et al., 2001) and other enzymes (Boehr et al., 2006), as well as antibody-antigen interactions (Bosshard, 2001), have been explained in terms of conformational selection. Conformational selection embodies statistical mechanics, in subtle contrast to Koshland’s deterministic view of induced-fit where the bound configuration is only attained when the enzyme binds a substrate molecule (Koshland Jr., 1958, 1994). The two mechanistic models represent extremes on a continuum, and there may be elements of both in the same system. Thus an excited state sampled via conformational selection may move closer to the ligand-bound state, but a substrate-initiated change may be needed to attain the final substrate-bound configuration (Sullivan and Holyoak, 2008). In this paper, we examine the timescales of backbone dynamics of an enzyme in the absence of substrates and correlate these findings with the structural changes and kinetics of enzyme turnover which occur in the presence of substrates.

RESULTS

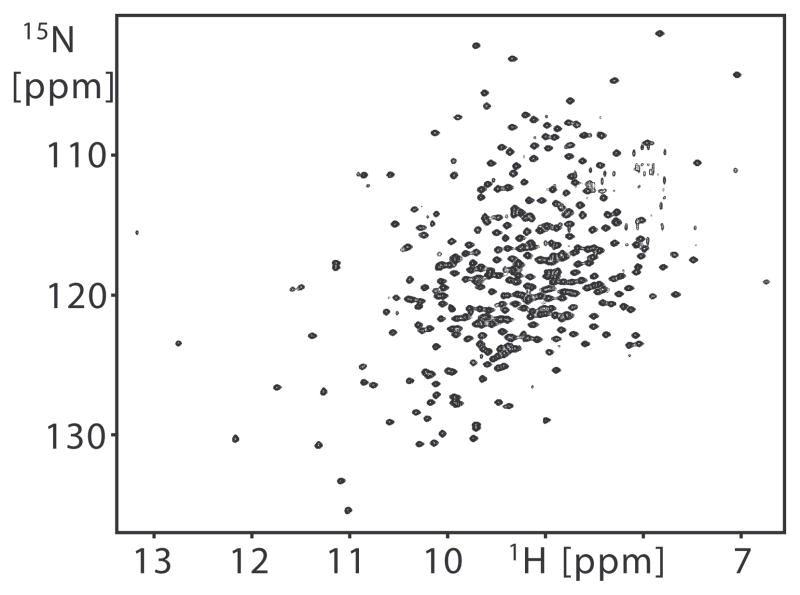
NMR ¹⁵N relaxation rate constants were measured for 303 of the 344 non-proline backbone amides in substrate-free arginine kinase. This included all assigned non-overlapping resonances (Davulcu et al., 2005). The NMR data are of high quality as illustrated by a 2D [¹⁵N,¹H] TROSY spectrum of the enzyme (Figure 1). All relaxation rate constant measurements were detected on the slow relaxing spin transition using [¹⁵N,¹H] TROSY detection schemes.

Figure 1.

2D [¹⁵N,¹H] TROSY of arginine kinase recorded at 800 MHz for the shortest T₁ delay measurement. The average signal-to-noise ratio of signals from the shortest relaxation delay time of T₁ and T_1rho measurement are 165/1 and 181/1 at 50.65 MHz, 202/1 and 200/1 at 60.78 MHz, and 370/1 and 250/1 at 81.04 MHz. The average signal-to-noise ratio from the {¹H}¹⁵N NOE reference spectrum is 165/1.

Slow (μs-ms) Timescale Dynamics

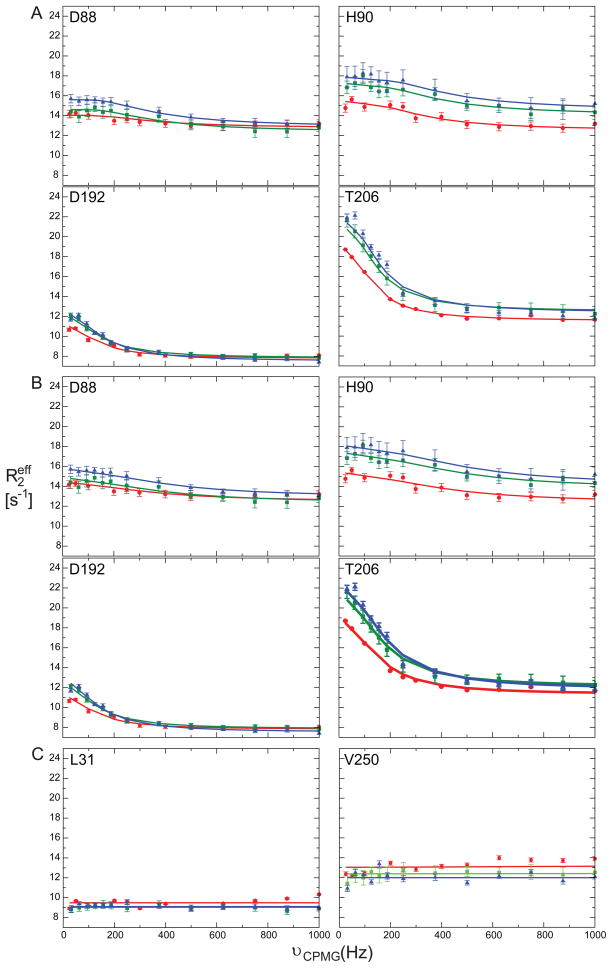
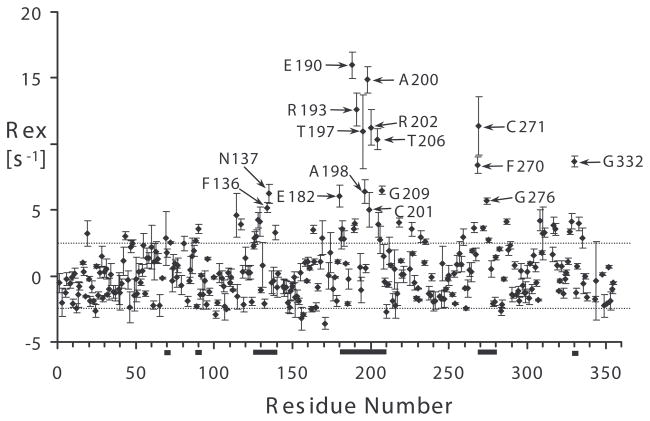
We began this study with a focus on identifying arginine kinase residues that exhibit μs-ms timescale motion. Main chain dynamics on this timescale were measured using two independent experimental approaches. Firstly, ¹⁵N relaxation dispersion identified 34 residues with R_ex > 2 s⁻¹ (Table 1). Representative ¹⁵N relaxation dispersion data at three static field strengths are shown in Figure 2. Secondly, independent R_ex measurements from a complementary NMR relaxation experiment identified 17 residues with R_ex above a conservative threshold of 5 s⁻¹ (Figure 3 & Table 1) of which just F136 had not previously been identified (Wang et al., 2003). Thus, we identified a total of 35 residues with relaxation arising from chemical exchange (R_ex) representing at least 12% of the protein.

Table 1.

Summary of two-state exchange parameters for individual and groups of residues in substrate-free arginine kinase.

			Individual Fits			Collective Fits
	Residue	2°	kex [s−1]	pB (%)	|Δω| [ppm]	kex [s−1]	pB (%)	|Δω| [ppm]
NTD HINGE	D88	α4	3200 ± 240	0.3 ± 0.1	1.9 ± 0.3	1930 ± 350	0.4 ± 0.1	3.2 ± 0.3
	H90	α4	1020 ± 480	0.4 ± 0.1	4.5 ± 0.5			4.4 ± 0.6
	G92	L4	1560 ± 760	0.3 ± 0.1	5.1 ± 0.6			3.4 ± 0.4
NTD-CTD INTERFACE	D71	L3	*	*	*	800 ± 100	1.1 ± 0.1	1.1 ± 0.1
	F136	L6
	N137	L6	1150 ± 160	1.9 ± 0.7	0.9 ± 0.6			1.7 ± 0.2
	F270	L11	2830 ± 230	0.9 ± 0.8	2.3 ± 0.5			2.4 ± 0.4
	C271	L11	*	*	*			0.9 ± 0.3
	N274	L11	1100 ± 710	0.6 ± 0.1	3.6 ± 0.7			1.6 ± 0.2
	G276	L11	1930 ± 340	1.4 ± 0.4	3.0 ± 0.6			3.8 ± 0.5
	G332	L14	1330 ± 320	1.5 ± 2.1	1.5 ± 1.0			1.5 ± 0.1
DYNAMIC DOMAIN 1 AND HINGE	R126	β1	690 ± 220	0.3 ± 0.1	2.9 ± 0.3	790 ± 70	0.4 ± 0.1	2.2 ± 0.3
	C127	β1	940 ± 550	0.2 ± 0.1	3.7 ± 0.6			1.9 ± 2.3
	R129	β1	950 ± 450	0.3 ± 0.1	5.6 ± 0.4			5.6 ± 0.2
	V220	β4	2360 ± 210	1.0 ± 0.1	1.3 ± 0.1			2.7 ± 3.0
	V222	β4	*	*	*			1.7 ± 0.6
	R229	β5	360 ± 350	0.3 ± 0.2	2.5 ± 0.4			1.7 ± 0.1
	Q234	β5	*	*	*			1.0 ± 1.0
SUBSTRATE BINDING LOOP L8	I182	α7	700 ± 420	0.5 ± 0.2	4.5 ± 0.4	790 ± 60	2.6 ± 0.6	0.8 ± 0.4
	D183	α7	*	*	*			*
	D184	α7	2730 ± 1760	0.5 ± 1.3	3.1 ± 4.5			0.6 ± 0.3
	H185	α7	1810 ± 650	0.3 ± 0.1	5.1 ± 0.6			0.8 ± 0.4
	E190	L8	990 ± 70	4.5 ± 0.6	1.6 ± 0.1			2.4 ± 0.8
	G191	L8	*	*	*			*
	D192	L8	760 ± 90	1.7 ± 0.4	1.3 ± 0.2			1.0 ± 0.2
	R193	L8	1470 ± 390	0.5 ± 0.1	4.4 ± 0.4			1.0 ± 0.5
	T197	L8	3660 ± 1360	0.5 ± 0.1	6.8 ± 1.3			1.1 ± 0.9
	A198	L8	4190 ± 1160	0.0 ± 1.2	2.6 ± 1.6			*
	A200	L8	1610 ± 440	0.7 ± 0.1	4.6 ± 0.5			1.3 ± 0.7
	C201	L8	510 ± 230	1.3 ± 0.1	1.9 ± 0.3			1.2 ± 0.1
	R202	L8	1300 ± 220	0.0 ± 0.0	0.8 ± 0.1			0.8 ± 0.6
	T206	L8	570 ± 50	2.6 ± 0.2	1.7 ± 0.1			1.7 ± 0.2
	G207	L8	1350 ± 140	0.0 ± 0.0	0.8 ± 0.1			0.7 ± 0.3
	R208	L8	920 ± 180	0.6 ± 0.1	3.8 ± 0.2			1.1 ± 0.1
	G209	β3	1450 ± 380	0.7 ± 0.2	3.3 ± 0.6			1.1 ± 0.2

Secondary structure of each residue is denoted with 2° as observed in the substrate-free crystal structure of arginine kinase. In all cases, fitting was performed assuming a two-state exchange model, where k_ex is the sum of forward and reverse rate constants, p_B is the relative population of the higher energy state, and Δω is the chemical shift difference between the two states. Asterisks denote residues for which fitting did not converge.

Figure 2.

¹⁵N spin relaxation dispersion curves for selected residues with μs-ms timescale dynamics: D88, H90, D192, and T206. (a) Individual fit dispersion curves at ¹⁵N Larmor frequencies 60.78 (red circles), 72.05 (green squares), and 81.04 MHz (blue triangles). (b) Collective fit dispersion curves for same residues: D88 and H90 with G92 and D192 and T206 with 12 other residues (Table 1). (c) ¹⁵N spin relaxation dispersion curves for residues with no R_ex.

Figure 3.

Chemical exchange in substrate-free arginine kinase identified from R_ex = R₂^β – R₁^2HzNz/2 – η_xy(κ − 1) + R₁N/2. κ = trimmed mean of 1 + (R₂^β − R₁^2HzNz/2)/η_xy = 1.43. For apparently non-exchanging residues, the average R_ex is 0.05 ± 2.5 s⁻¹, error is shown with dashed lines. The large error is partly due to residue-specific variation in the ¹⁵N CSA tensor magnitude and orientation. The solid bars represent polypeptide segments having residues with ¹⁵N spin relaxation dispersion. Error bars are from measurements in triplicate. Residues with R_ex greater than 2 standard deviations (5 s⁻¹) are labeled.

Residues with R_ex are prevalent at four prominent locations in the protein (Figures 3 & 4 & Table 1): (1) D88-G92, within a hinge linking the N- and C-terminal domains; (2) D71, F136-N137, F270-G276 and G332 which all lie at the interface between the N- and C-terminal domains; (3) R126-L131 and V220-Q234, which largely constitute the hinges of dynamic domains 1 and 2 and (4) I182-G209, a substrate binding loop.

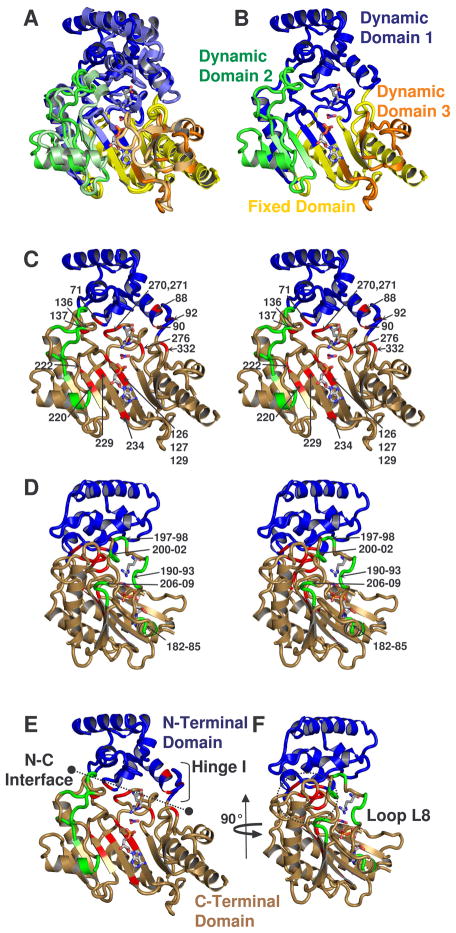
Figure 4.

(a) Comparison of the dynamic domains of substrate-free and -bound structures of arginine kinase (Yousef et al., 2003; Zhou et al., 1998). Dynamic domains 1, 2, and 3 are colored respectively blue, green and orange. The darker shade of each color is substrate-free and fixed domain is shown in yellow. (b) shows only substrate-free. (c – f) show amino acids with measurable R_ex, colored red, with the exception of residues between 180-209, near loop L8, which are shown in green. The classical N- and C-terminal domains are shown blue and sand. c & d are stereographic pairs with loop 8 residues labeled in panel d, and all others labeled in panel c. Panels d & f are rotated 90° with respect to panels c & e. e shows the interface between N- and C-terminal domains and location of N-terminal domain hinge. In all panels, substrates ADP, NO₃ anion, and arginine are shown from the -bound structure to guide the eye.

Resonances showing chemical exchange were fit to the Bloch-McConnell equation, and assuming two-state exchange, estimates of k_ex, populations p_A and p_B, and the chemical shift difference between states A and B, Δω, were obtained (Cavanagh et al., 1996; Palmer et al., 2001). Representative ¹⁵N spin relaxation dispersion curves are shown in Figure 2 and their fit exchange parameters are listed in Table 1. Conformational exchange rate constants, k_ex, of individual residues range from 400 to 4200 s⁻¹ with significantly skewed p_A/p_B ratios. The fit ¹⁵N chemical shift difference, Δω, ranges from 0.8 to 5.6 ppm. Analysis for a small number of residues did not converge, likely the result of small R_ex. Dispersion data from residues in spatially distinct locations of the enzyme were fit together assuming they share a common motion responsible for the chemical exchange with the same k_ex and relative populations p_A and p_B. The groupings and fit parameters are listed in Table 1. Further attempts to consolidate by fitting common parameters dynamic domain 1, its hinge, and flexible loop L8 were unsuccessful.

Fast (ns-ps) Timescale Dynamics

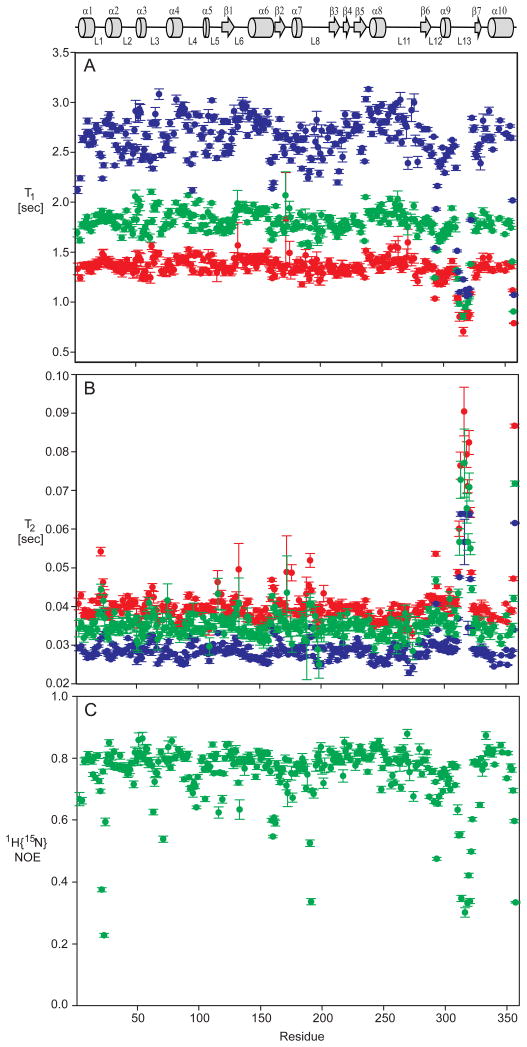
NMR spin relaxation measurements were recorded at three static field strengths, ¹⁵N ω_o = 50.65, 60.78, and 81.04 MHz, and analysed with the Lipari-Szabo model-free approach, or extended model-free, providing ns-ps dynamics information (Clore et al., 1990; Lipari and Szabo, 1982a, b). ¹⁵N spin longitudinal (T₁) and transverse (T₂) relaxation time constants were measured at each field and the heteronuclear {¹H}¹⁵N nuclear Overhauser effect (NOE) measured at 60.78 MHz only, yielding a total of seven measurements per residue. The data were recorded with high signal to noise (Figure 1), resulting in reliable T₁, T₂, and NOE values with most standard deviations < 2%. The relaxation time constants and NOE values are plotted in Figure 5 and listed in Supplemental Table 1. Mean and “trimmed” mean values, the subset used in the isotropic τ_m calculation, are listed in Table 2. The theoretical maximum NOE, in the rigid limit and τ_m = 23 ns, is 0.83 and only two residues are larger by more than 2 standard deviations, T269 and L333.

Figure 5.

¹⁵N NMR spin relaxation time constants (a) T₁, (b) T₂ and (c){¹H}¹⁵N NOE for arginine kinase at ¹⁵N Larmor frequencies 50.65 (red), 60.78 (green), and 81.04 MHz (blue). Individual values are listed in Supplemental Table 1. Error bars on each measurement represent one standard deviation. The T_1rho spin lock time at 50.65 and 60.78 MHz was shorter than optimal for the slow relaxing amide signals in loop L13 resulting in relatively large errors. A schematic diagram of arginine kinase secondary structure is shown at the top with α-helices shown as cylinders, β-strands as arrows, and loops and extended structure as lines.

Table 2.

Mean and trimmed mean ¹⁵N T₁, T₂, and heteronuclear {¹H}¹⁵N NOE values for backbone amides of arginine kinase.

Parameter	50.65 MHz	60.78 MHz	81.04 MHz
T1 [s]	1.35 ± 0.12	1.79 ± 0.17	2.61 ± 0.33
Trimmed T1 [s]	1.36 ± 0.06	1.82 ± 0.09	2.69 ± 0.16
T2 [s]	0.041 ± 0.007	0.036 ± 0.006	0.029 ± 0.005
Trimmed T2 [s]	0.039 ± 0.002	0.034 ± 0.002	0.029 ± 0.002
Trimmed T1/T2	35.34 ± 3.25	53.08 ± 5.00	96.46 ± 9.49
{1H}15N NOE		0.75 ± 0.10
Trimmed {1H}15N NOE		0.79 ± 0.04

Values are reported as the all-residue or trimmed mean ± one standard deviation. Trimmed means are determined following criteria outlined in Tjandra et al., 1995 (Tjandra et al., 1995b).

{¹H}¹⁵N NOE < 0.65 are excluded from trimmed {¹H}¹⁵N NOE mean.

NMR spin relaxation measurements were fit to isotropic and axial-symmetric anisotropic rotational diffusion models using established methods (Tjandra et al., 1995a). Evidence derived from calculation and experiment strongly suggests that the axial-symmetric diffusion model is more appropriate. First, calculation of rotational diffusion anisotropy from NMR data with the substrate-free arginine kinase coordinates, assuming axial symmetric diffusion, resulted in a ratio of rotational diffusion rate constants, D_par/D_perp of 0.86, which corresponds to an oblate ellipsoid (Yousef et al., 2003). The final diffusion tensor for 1M80.pdb was D_par = 6.50 × 10⁶ s⁻¹ D_perp = 7.54 × 10⁶ s⁻¹, theta = 1.62 rad. and phi = 0.72 rad. Secondly, the calculated ratio of rotational diffusion rate constants for substrate-free arginine kinase using HYDRONMR was 0.80, consistent with the experimental results (Garcia de la Torre et al., 2000; Yousef et al., 2003). Finally, the consensus optimal value of τ_m and the average value of τ_m for the amide resonances of the individual residues fit to isotropic models both indicate that the value of the rotational correlation time is 23 ns. In contrast, the τ_m values for the amide nuclei in individual range between 21.0 – 25.7 ns, which is inconsistent with isotropic diffusion, but consistent with data acquired for a system that exhibits anisotropic rotational diffusion but fit to an isotropic model. We therefore proceed with subsequent analysis of the NMR relaxation data using an anisotropic model.

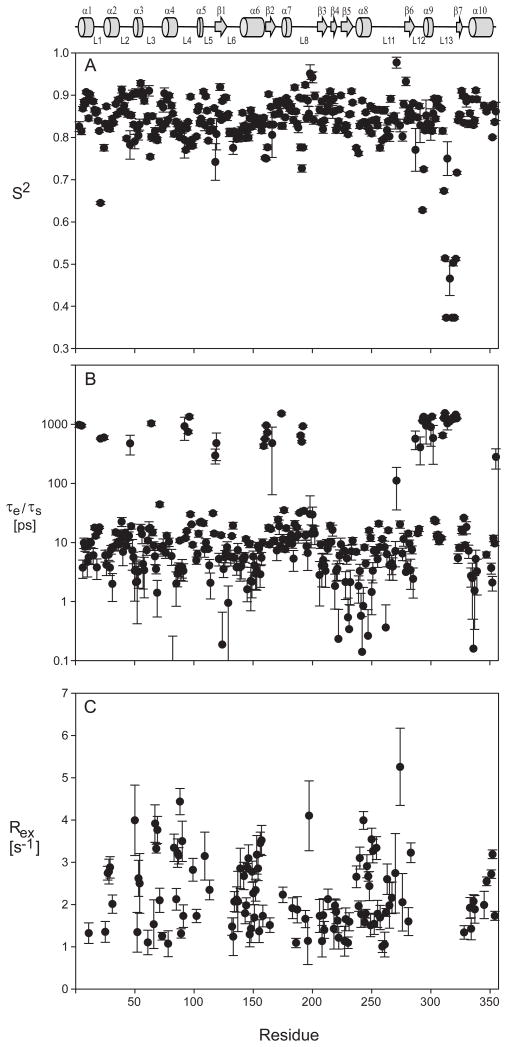
Following standard procedures and using axial symmetric diffusion, the ns-ps dynamics of each residue was analyzed and reported in Figure 6 and listed in Supplemental Table 2 (Clarkson et al., 2006; d’Auvergne and Gooley, 2003; Mandel et al., 1995). Model selection was guided by the χ² error. Where χ²could not distinguish two or more models, the simplest was chosen. Models 3 (S², R_ex) & 4 (S², τ_e, R_ex) were excluded if the fit R_ex was < 1 s⁻¹. This cutoff was chosen since the experimental error of R₂ is about 1 s⁻¹. Residues V308 - V322, which are absent in the PDB coordinates, were necessarily fit using the isotropic model. Model selection results are summarized in Table 3. About 35% of the residues were best fit using models 3 & 4, indicating even more extensive μs-ms chemical exchange (Figure 6) than reported by relaxation dispersion. Alternative analysis using an isotropic diffusion model indicates a slightly higher fraction (40%) of residues best fit to models 3 & 4 (not shown). About 14% of the residues (43) were best fit to model 5 (S²_f, S²_s, τ_s) and are most abundant in loops and turns. Most residues best fit with model 5 have a slow time constant, ^aveτ_s = 1 ± 0.4 ns. It should be appreciated that the error in estimating the local correlation time is larger than that of other fitted parameters. Furthermore, in the current analysis, a single measurement of the {¹H}¹⁵N NOE was used which decreases the precision of the estimate somewhat. Although the τ_s values are relatively large, because τ_m is also large (23 ns), the Lipari-Szabo decoupling approximation is not violated (Lipari and Szabo, 1982a, b; Vugmeyster et al., 2003). Nearly all residues best fit to model 2 (S², τ_e) & 4 (S², τ_e, R_ex) have τ_e values < 20 ps. The ^aveS² over all residues is 0.85 ± 0.06 and is significantly lower only for residues in loops L1, L12, and L13 (Figure 6 & Supplemental Table 2). Overall, the enzyme is relatively rigid on the ns-ps timescale with exception of three loops, including one, loop L13, which has previously been shown to be crucial for substrate binding.

Figure 6.

Lipari-Szabo model-free results for substrate-free arginine kinase dynamics. (a) Generalized order parameters, (b) τ_e/τ_s internal time constants and (c) R_ex at 600 MHz. All fit parameters and model selections are listed in Supplemental Table 2.

Table 3.

Summary of Lipari-Szabo model selection for substrate-free arginine kinase.

	Model	Number of Residues	Percentage
1	(S2)	5	1.7
2	(S2, τe)	142	46.8
3	(S2, Rex)	7	2.3
4	(S2, τe, Rex)	106	35.0
5	(S2f, S2s τs)	43	14.2
	Total	303	100.0

DISCUSSION

Interpretation of the main chain dynamics will be discussed with reference to classical as well as “dynamic” domain structure, dynamic domains being more appropriate in describing the differences between substrate-free and -bound crystal structures. This is not to imply that the intrinsic dynamics observed by NMR in substrate-free form must necessarily be of the same magnitude or direction as the large conformational changes revealed in the substrate-bound crystallographic structures. Furthermore, NMR can reveal motions between open and closed states, some of which may be populated at levels too low to be detectable crystallographically. Despite these caveats, there is good correspondence between the locations of residues exhibiting chemical change or increased flexibility and prediction based upon the crystal structures.

The classical description of arginine kinase is as two domains: a small, α-helical N-terminal domain (NTD) spanning residues 1-90 and a larger, primarily β-sheet C-terminal domain (CTD) spanning residues 120-357, with the active site lying in the cleft between these domains. The domains are connected with residues 91-119, a region well-defined by crystallography but lacking regular α-helical or β-strand structure. Upon binding substrates, the two domains close over the active site. Closing of the two domains positions several substrate-binding and catalytically-important residues in the active site, underlying the importance of dynamics on turnover of the enzyme (Yousef et al., 2003; Zhou et al., 1998).

Arginine kinase can also be described as three dynamic domains that rotate independently relative to a fourth, fixed domain (Yousef et al., 2003). The overall structure of dynamic domains is shown in Figure 4a,b and compared to classical domains in Figure 4c–f. Dynamic domain 1 is an extended and larger version of the N-terminal domain, while dynamic domains 2 and 3 are primarily large loops (L8 and L13) of the classical C-terminal domain.

Rigid Body Motion of the N-terminal Domain

Between the substrate-free and bound states, the (classical) N-terminal domain of arginine kinase undergoes a significant rigid-body motion - a hinged rotation of the domain about residues 88-94 (Figure 4a,b) (Yousef et al., 2003; Zhou et al., 1998). Domain rotation also causes a flexing at the NTD-CTD interface (Figure 4). The open and closed forms of the enzyme show little difference in the internal structure of the NTD. The average composite torsion angle difference, defined as (Δφ² + Δψ²)^1/2, for residues 3-90 is only 10 degrees, and the backbone RMSD is < 1 Å compared to a 6 Å overall displacement of the N-terminal domain upon substrate-binding (Yousef et al., 2003; Zhou et al., 1998). The linker between the NTD and CTD also shows sizeable differences between the open and closed-form crystal structures at residues 92-94, with composite torsion angle changes of 15°, 18°, and 29°(Yousef et al., 2003; Zhou et al., 1998).

NMR relaxation dispersion identifies μs-ms timescale motion for multiple residues in the hinge and interface (Figure 4e,f). Their distribution is consistent with a rigid body motion with little change in internal structure: the NTD core is devoid of R_ex with the exception of D71, located in the interface with the CTD (Figure 4). Assuming two-state exchange between open and closed forms, we fit exchange parameters for individual residues and groups with the Bloch-McConnell equation (Table 1). Fitting NMR relaxation dispersion for three individual hinge residues (D88, G90, G92) resulted in similar k_ex and p_B values while fitting a single collective motion yields k_ex = 1930 ± 350 s⁻¹ and p_B = 0.4 ± 0.1%. The equilibrium is highly skewed; the approximate k_open and k_close rate constants are 1920 and 10 s⁻¹, respectively. For eight NTD-CTD interface residues (D71, F136, N137, F270, C271, N274, G276, and G332) individual k_ex values range from 1200 to 2800 s⁻¹ with skewed populations, p_B < 2%, while a collective fit yields k_ex = 800 ± 100 s⁻¹ and p_B = 1.1 ± 0.1% (Table 1). Estimates of k_open and k_close are 790 s⁻¹ and 10 s⁻¹ respectively. The collective interface k_ex is about half that of the hinge. We note that the hinge and interface residues fit small relative population p_B, which can lead to uncertainty in the exact parameter values fit. A collective fit of hinge and interface residues together was unsuccessful. In summary, the hinge and interface residues exhibit μs-ms timescale dynamics, with a highly skewed equilibrium p_B ≈1%, k_open ≈ 790 – 1920 s⁻¹, and k_close ≈ 10 s⁻¹. The slower of the two rate constants is of the same order as k_cat, and potentially rate-limiting.

Additional residues exhibiting R_ex can be understood with the dynamic domain model (Figure 4a,b; Zhou et al., 1998; Yousef et al., 2003). R126, C127, R129, V220, V222, R229, and Q234 are located in the 49 residue dynamic domain 1 hinge or near the bottoms of the substrate binding pockets (Figure 4; (Yousef et al., 2003)). A collective fit for all dynamic domain 1 and hinge residues results in a reasonable k_ex = 990 ± 110 s⁻¹ but very small p_B (< 0.3%) and unreasonably large Δω and errors (not shown). Thus, even if only semi-quantitatively, NMR confirms μs-ms timescale dynamics of the NTD (or dynamic domain 1 and hinge 1) and demonstrates that the motions are intrinsic to substrate free arginine kinase, and not just substrate-gated.

On the ps-ns timescale, the entirety of the classical N-terminal domain or dynamic domain 1 and hinges are mostly rigid, with only a few locations showing increased flexibility (S² < 0.8). The average order parameter, S², for the NTD is 0.85 ± 0.04 and of the NTD loops, only L1 (residues S21-K24) shows significantly lower S² values while L2 (N41-T49), and L3 (L61-A72) reveal only slightly below average S² (supplemental Table 2; Figure 6). Loop L1 is surface exposed and distant from the active site. The average S² of hinge residues is similar to the average of the NTD residues. Loop L4, G91-G105, of the NTD-CTD linker and loops L6, S130-L140, and L11, K256-M279, at the NTD-CTD interface also show very small decreases in S², indicating some increased local flexibility.

Dynamics of Substrate Binding Loop L8, I182-G209

Substrate binding loop L8 changes conformation between open and closed structures to help close the entrance to the nucleotide binding site (Yousef et al., 2003; Zhou et al., 1998). The overall displacement of L8 is 5.4 Å, but internal to L8, the RMSD is only 1.4 Å (Figure 4). The average composite torsion angle change in loop L8 between open and closed forms of arginine kinase is 36°. Similar to the NTD, loop L8 has characteristics of a rigid body motion, but the larger composite torsion angle change and RMSD suggest a more complex motional model.

NMR relaxation dispersion shows a high fraction (60%) of loop L8 residues with R_ex. Fitting dispersion for individual residues yields a range of k_ex from 500 – 2700 s⁻¹ and skewed populations, p_B < 4.5%; a collective fit of all seventeen residues yields k_ex = 790 ± 60 s⁻¹, p_B = 2.6 ± 0.6%, k_open ≈ 770 s⁻¹, and k_close ≈ 20 s⁻¹ (Table 1) and assumes the predominant conformer is the open state. The smaller of the two rate constants is on the same order as k_cat.

NMR confirms μs-ms timescale dynamics in loop L8 are intrinsic to substrate free arginine kinase and not only substrate-gated. Backbone crystallographic B-factors for this loop in the structure of the unbound protein are elevated about two-fold. In contrast, B-factors for loop L8 in the substrate-bound form are not elevated, indicating relative ordering compared to the substrate free structure. Overall, the NMR results are consistent with the crystallographic B-factors. Loop L8 is involved with substrate nucleotide binding. The closed structure of the enzyme places loop L8 residue H185, along with H284, in a stacked arrangement with the purine ring of substrate ADP (Zhou et al., 1998). We thus speculate that the intrinsic motion present in loop L8 may sample the closed-form configuration, which is then selected with nucleotide binding.

The primary structure of loop L8 is highly conserved across the phosphagen kinase family. Crystal structures of substrate-free mitochondrial creatine kinase and transition state analog form of muscle creatine kinase show an identical B-factor pattern for the creatine kinase equivalent of loop L8 (Fritz-Wolf et al., 1996; Ohren et al., 2007). Furthermore, the results of hydrogen/deuterium exchange studies of creatine kinase are consistent with increased mobility in this loop (Mazon et al., 2004; Mazon et al., 2005). These correlations between arginine kinase and creatine kinase suggest that the phosphagen kinase family of enzymes has evolved not only in structure, but also in dynamics of this and other catalytically important regions.

On the ns-ps timescale, loop L8, and the whole of dynamic domain 2, appears relatively rigid (S² = 0.85 ± 0.03) with exception of three residues E190, G191, D192 located near but not at the loop tip, which have S² of 0.78, 0.73, and 0.78 respectively (Supplemental Table 2; Figure 4). Two of these residues, E190 and D192, exhibit measurable R_ex and also best fit model 5 (S²_f, S²_s, τ_s) and their NOEs are smaller than average (Supplemental Table 2). This suggests a more complicated loop L8 motion.

Dynamics of Substrate Binding Loop L13, V308-V322

On the ns-ps timescale, the most prominent feature of motion in the enzyme is present in substrate binding loop L13, residues V308-V322 (Figures 5 & 6). Residues in the loop are best fit with model 5 (τ_s, S²_s, S²_f) resulting in a τ_s ≈ 1 ns (Table 3). As noted above, use of a single {¹H}¹⁵N NOE measurement limits the precision of the local correlation time constant. The average S² is 0.53, substantially lower than average (Supplemental Table 2). This increased flexibility is consistent with an absence of observable electron density for this region in the substrate-free arginine kinase crystal structure (Yousef et al., 2003). The correspondence of low order parameters and the absence of electron density suggest loop disorder is not an artifact of the crystalline state, since loop L13 becomes well-ordered in the substrate-bound complex, with only slightly elevated B-factors.

The conservation of elevated measures of thermal disorder in substrate-free crystal structures of phosphagen kinases from different phyla suggests a link to function. In substrate-bound arginine kinase, the side chains from two loop L13 residues directly contact substrate, R309 with nucleotide and E314 forming a salt link with substrate arginine (Zhou et al., 1998). R309 is highly conserved in the phosphagen kinase family and E314 is less conserved, aligning with a valine in creatine kinases. An E314V mutant, in the context of swapping the arginine and creatine kinase loops, resulted in only 35% loss of activity suggesting stabilization of the substrate-bound configuration involves interactions besides E314 (Azzi et al., 2004; Pruett et al., 2003). The structural, mutagenesis, and NMR studies suggest that the conserved primary structure of loop L13 across phosphagen kinases may reflect conservation of flexibility, as opposed to stabilization of substrate-bound state, as a necessary condition for catalysis.

Dynamics and Enzyme Catalysis

Through an increasing number of examples, it is becoming apparent that dynamics is important in the turnover of many enzymes (Boehr et al., 2006). General comments have been made about the slow turnover of phosphagen kinases being consistent with rate-limiting conformational change, but it is only now, with this work, that the specific mechanistic details are beginning to emerge. Particularly intriguing is the approximate correspondence between rate constants of conformational changes determined here by NMR (3 – 20 s⁻¹) and the enzyme turnover rate, k_cat =100 –150 s⁻¹, measured earlier by experimental kinetics, suggesting that conformational changes might be rate limiting on enzyme turnover. The two regions with motions at corresponding timescales are loop L8 and the N-terminal domain (or dynamic domain 1), which moves as a (quasi-)rigid group. It is possible that the intrinsic motions are in the same direction, if not of the same magnitude as the changes seen between the substrate-free and -bound crystal structures, but the NMR analysis provides no direct evidence (Yousef et al., 2003; Zhou et al., 1998). The changes to loop L8 and the NTD contribute to the closing of the enzyme around the active site pocket. The most intriguing possibility is that turnover is gated by one or both of the motions. The difference between the slow rate constants of conformational exchange and turnover is not large, and might be brought even closer if the presence of substrates modulates dynamics. It is also possible that substrate-associated changes to loop L8 and NTD were required, and that evolutionary selection of residues that facilitate the conformational change could also have resulted in a loop that is intrinsically dynamic. In either case, it is possible that the ms timescale motions are part of an intrinsic conformational equilibrium whose populations are shifted by substrate binding towards a state required for reaction turnover (Boehr and Wright, 2008; Lange et al., 2008).

Loop L8 includes H185 that forms two interactions with the substrate nucleotide, stacking with the base, and hydrogen-bonding to the ribose (Zhou et al., 1998). While the rest of the loop is in the general area of the active site, this is the only direct interaction, and it is not proximal to where bond breakage/formation is catalyzed. The same is true for the NTD, for which the only direct substrate interaction is between the substrate-specificity loop and the end of the phosphagen substrate that is remote from the reactive atoms. Thus, if intrinsic dynamics, observed by NMR, are rate-limiting, their effects are exerted through substrate-binding or indirectly upon catalysis through propagated changes, rather than through direct influence on the catalytic chemistry.

The rate-limiting effect of protein dynamics on turnover has been shown for a number of other enzymes. Lid opening and closing has been shown to be rate-limiting upon catalysis in, for example, adenylate kinase (Wolf-Watz et al., 2004) and triose phosphate isomerase (Rozovsky et al., 2001). A single histidine residue has been implicated in coupling motions in RNase A to turnover (Watt et al., 2007). The transition from a closed to an occluded conformation in dihydrofolate reductase, marked by a loop motion, has been shown to be rate-limiting (Boehr et al., 2008). The motions in arginine kinase have analogs in these other systems. It is noteworthy that several motions are found together in arginine kinase: motion in a lid-like loop (L8), hinged domain motion (NTD), both of which are at turnover-commensurate rates, and the faster dynamics of loop L13 that folds over the active site. The presence of several modes of motions in arginine kinase, some of which might be turnover-limiting, and the conservation of the dynamic structural elements adds to a growing awareness that conformational dynamics might be more widespread and functionally relevant to enzymes than has been heretofore widely appreciated.

EXPERIMENTAL PROCEDURES

Sample Preparation

Uniformly ¹⁵N-enriched and 80% ²H-enriched Limulus polyphemus arginine kinase was prepared as previously described (Davulcu et al., 2005). The final enzyme sample was concentrated to 1.0 mM in a buffer containing 10 mM sodium citrate (pH=6.5), 0.5 mM dithiothreitol, 50 mM potassium chloride, 50 μm sodium azide, 90% H₂O, and 10% D₂O. Arginine kinase is stable for months at 4 or 20 °C, showing no signs of degradation or reduced enzymatic activity as judged by 2D [¹⁵N,¹H] TROSY spectra and enzyme activity profiles over this same timescale (not shown).

NMR Spectroscopy

NMR data were recorded on Varian INOVA NMR spectrometers operating at ¹H(¹⁵N) Larmor frequencies (ω_o) of 499.8(50.65), 599.7(60.78), 719.9(72.95), or 799.7(81.04) MHz. Each spectrometer was equipped with a HCN triple resonance probe and a Z-axis pulsed field gradient. All relaxation experiments were performed at 25 ± 0.5 °C, determined using a methanol standard (Raiford et al., 1979). Proton chemical shifts were referenced to the DSS methyl protons at 0 ppm and ¹⁵N chemical shifts referenced indirectly (Markley et al., 1998). Amide ¹H and ¹⁵N resonance assignments of arginine kinase have been reported (Davulcu et al., 2005).

¹⁵N T₁ and heteronuclear {¹H}¹⁵N NOE values were measured with relaxation experiments modified for TROSY detection (Farrow et al., 1994). T_1ρ was measured using relaxation experiments described in Korzhnev et al., 2002 (Korzhnev et al., 2002); T₂ values were calculated from T₁ and T_1ρ values. At 50.65 MHz, ¹⁵N T₁ was measured using relaxation delays of 0.151, 0.503*, 1.006, 1.509, 2.012, and 3.017 s (“*” denoting measurements duplicated for error estimation); ¹⁵N T_1ρ was measured using relaxation delays of 5, 10*, 25, 35, and 55 ms and a ¹⁵N spinlock field strength of 2.6 KHz. At 60.78 MHz, ¹⁵N T₁ was measured using relaxation delays of 0.151, 0.503*, 1.006, 1.509, 2.012, and 4.5 s; ¹⁵N T_1ρ was measured using relaxation delays of 5, 10*, 25, 35, and 55 ms, and a ¹⁵N spinlock field strength of 2.7 KHz. At 81.04 MHz, ¹⁵N T₁ was measured using relaxation delays of 0.151*, 0.504, 1.010, 1.510, 2.010*, 3.020, and 3.527 s, ¹⁵N T_1ρ was measured using relaxation delays of 5*, 15, 25*, 35, and 55 ms and a ¹⁵N spinlock field strength of 2.7 KHz. The steady-state {¹H}¹⁵N NOE was measured at 60.78 MHz using a ¹H saturation time of 11 s (5 times the longest ¹⁵N T₁). {¹H}¹⁵N NOE was calculated from the intensity ratio of an experiment recorded with and without ¹H saturation during the mixing time. Peak intensity error was estimated from the rms base plane noise and {¹H}¹⁵N NOE error from propagation of error in quadrature.

Slow timescale chemical exchange was quantified using two different experimental NMR approaches. The first approach made use of constant relaxation-time ¹⁵N spin CPMG relaxation dispersion with TROSY detection at three static fields, ¹⁵N ω₀ = 60.78, 72.95 and 81.04 MHz (Loria et al., 1999). In these experiments, the CPMG field strength (υ) is varied between 0 and 1 KHz by adjustment of the CPMG refocusing delay time. Data were recorded using the following parameters: ¹⁵N ω₀ = 60.78 MHz, constant relaxation time = 40 ms, υ = 0, 25, 50*, 100, 200, 250, 300, 400, 500, 625, 750, 875, and 1000 Hz; ¹⁵N ω₀ = 72.95 and 81.04 MHz, constant transverse relaxation time of 32 ms, υ = 0*, 31.25, 62.50, 93.75, 125*, 156.25, 187.50, 250, 375, 500*, 625*, 750, 875, and 1000* Hz. The second approach is based on measurement of ¹⁵N spin R₂^β (TROSY), R₂^α (anti-TROSY), and longitudinal two-spin order R₁^2HzNz rate constants, which are then used to estimate R_ex (Wang et al., 2003). ¹⁵N spin R₂^α, R₂^β, and R₁^2HzNz experiments were recorded at ¹⁵N ω₀ = 60.78 MHz. Experiments were recorded in duplicate at 2τ = 5/J_NH and, alternatively, the complete decay curves were recorded using relaxation time delays of 2τ = N/J_NH with N = 0, 1, 2*, 3, 6, and 12 for R₂^β; N = 0, 1*, 2, 3 for R₂^α; τ = N/J_NH with N = 0, 1, 4*, 10, 20, and 30 for R₁^2HzNz. Rate constants were taken from two-parameter single exponential fits of peak intensities to relaxation delay times using the Levenberg-Marquardt algorithm. R_ex and kappa were identical within measurement error, whether determined using a single 2τ = 5/J_NH relaxation time or by recording a full decay curve using multiple relaxation time increments.

All ¹⁵N spin relaxation data were recorded with ~100 ms ¹H acquisition time (t_2max) and 75 ms ¹⁵N acquisition time (t_1max) and processed with FELIX2004 to final matrices of 4096 (¹H) × 1024 (¹⁵N) points. Peak intensities were measured with SPARKY (Goddard and Kneller). ¹⁵N spin relaxation time constants T₁ and T_1ρ were calculated from two-parameter single exponential fits of peak intensities to relaxation delay times using the Levenberg-Marquardt algorithm. The residual χ2 values of the single exponential fits were generally less than or equal to the number of fit data points, suggesting the data were well fit.

Slow (μs-ms) Timescale Dynamics Analysis

Chemical exchange is a prevalent phenomenon in NMR spectroscopy and provides valuable information on kinetic processes occurring on the μs – ms timescale. It results from alternating an NMR spin between different magnetic environments, or chemical states. Arginine kinase residues experiencing μs – ms chemical exchange were identified using the method described in Wang, 2003 (Wang et al., 2003) and using ¹⁵N R₂ relaxation dispersion (Loria et al., 1999). For those residues with R_ex, the former approach estimates the R_ex value, while the latter depends upon analyzing the ¹⁵N R₂ rate constant as a function of effective CPMG field strength, υ. Instead of recording a full relaxation decay curve at each CPMG field strength, a two-point ¹⁵N R₂^eff is determined using a common reference experiment (I₀) and a single intensity value (I_υ): R₂^eff = (−1/τ_relax) ln (I_υ/I₀), where τ_relax is the constant relaxation time period. In the absence of chemical exchange, R₂^eff will be independent of υ, while R₂^eff will vary with υ in the presence of appropriate timescale chemical exchange. A plot of R₂^eff versus υ generates a dispersion profile that can generally be fit to a simple, two-site exchange model when ΔR₂^eff = R₂^eff (υ→0) – R₂^eff (υ = 1 KHz) > 2 s⁻¹. Values below this threshold could not be reliably identified or fit. Arginine kinase residues with relaxation dispersion of this criteria were fit with a numerical approximation of the Bloch-McConnell equation using software kindly provided by Drs. Lewis Kay and Dmitry Korzhnev (Cavanagh et al., 1996). In all cases, a two state exchange model was best-fit with the summed exchange rate constant k_ex = k_AB + k_BA, population of states A, p_A, and B, p_B, and their ¹⁵N chemical shift difference, Δω. No assumption was made regarding fast or slow exchange regime of k_ex relative to Δω.

Fast Timescale Model-Free Analyses

The Lipari-Szabo simple model-free formalism describes protein dynamics with two timescales: τ_m describes the rotational correlation time that reports on protein tumbling and τ_e describes relative rapid internal motions (Lipari and Szabo, 1982a, b). These motions are assumed to be decoupled from each other, and typically exhibit a ratio (τ_m/τ_e) greater than 10. Internal motions are described with the amplitude-dependent square of the generalized order parameter S² and a ps-ns correlation time constant, τ_e. This simple model has been extended to describe situations where fast, S₂^f and τ_f, and slow, S₂^s and τ_s, internal motions are present (Clore et al., 1990). The generalized order parameter describing this situation is expressed as the product: S² = S²_f S²_s. Model-free analysis can also include an exchange term, R_ex, that adjusts the transverse relaxation rate constant to account for motions on the μs-ms timescale. The Lipari-Szabo spectral density functions are parameterized using a NH bond length of 1.04 Å and a ¹⁵N CSA of −170 ppm.

Selection of the appropriate rotational diffusion model for substrate-free arginine kinase was established by considering the prediction of hydrodynamic behavior of the molecule based on structural coordinate using HYDRONMR (Garcia de la Torre et al., 2000), and by conducting an analysis of the χ² values derived from fits of the experimental NMR relaxation data using spectral density models for isotropic and axially-symmetric rotational diffusion. Both diffusion models were parameterized with an identical subset of residues that does not contain contributions from motion on multiple timescales. The residues excluded were those with {¹H}¹⁵N NOE < 0.75, those showing evidence of conformational exchange from T₁/T₂ ratio analyses (Tjandra et al., 1995b), those with measured R_ex, and those not in regular secondary structure elements. Using these criteria, 136 residues were retained for use in parameterization in the diffusion models. The isotropic diffusion model was determined from a least squares fit of the spectral density functions for T₁/T₂ ratios to the experimental data as described. This calculation was performed with a subroutine in the software relxn2.2 with the rvi interface (Boyer and Lee, 2008). The axially symmetric diffusion model was parameterized from the crystal structure of substrate free arginine kinase (PDB ID 1M80). This was accomplished by rotating the PDB coordinates to align the moments of inertia with the Cartesian axes and translated such that the enzyme center of mass was located at the origin. These manipulations were performed with PDBINTERTIA provided by Dr. Arthur G. Palmer (Columbia University). Direction cosines of amide bond vectors in the alignment frame were determined using software from Dr. Andrew L Lee (University of North Carolina).

The parameters S², S²_f, S²_s, τ_e, τ_s and R_ex were fit to five standard motional models: Model 1, S² only; Model 2, S² and τ_e; Model 3, S² and R_ex; Model 4, S², τ_e and R_ex; and Model 5, S²_f, S²_s and τ_s using the model-selection subroutine of relxn2.2 (Boyer and Lee, 2008). Model selection for each residue was guided by the χ² statistic and the model with the lowest χ² was usually selected (d’Auvergne and Gooley, 2003). For residues in which R_ex was not observed in relaxation dispersion experiments, model 3 or 4 was only selected if the fit R_ex was greater than the experimental error on R₂, estimated as 1 s⁻¹. Fits with χ² values > 150 were not interpreted.