Skip to main content
PMC home page
Proceedings of the National Academy of Sciences of the United States of America logoLink to Proceedings of the National Academy of Sciences of the United States of America
. 1989 Jan;86(1):352–356. doi: 10.1073/pnas.86.1.352

Consequences of stochastic release of neurotransmitters for network computation in the central nervous system.

Y Burnod 1, H Korn 1
PMCID: PMC286463  PMID: 2563165

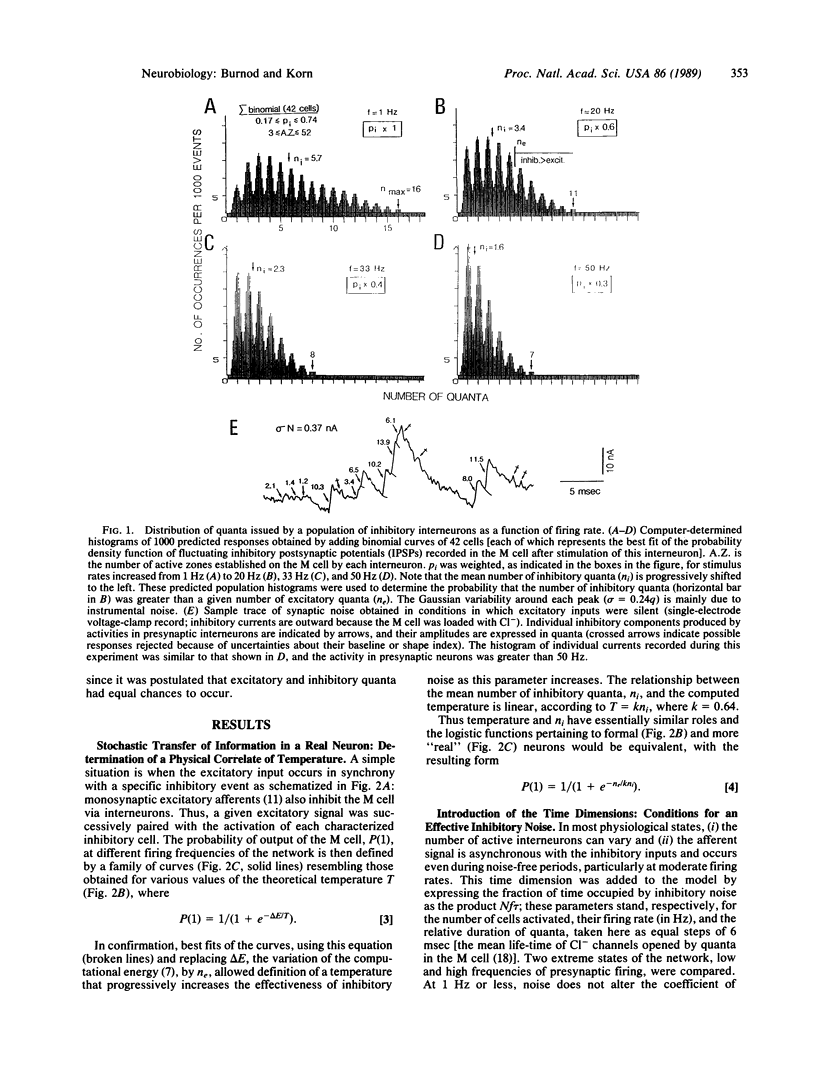
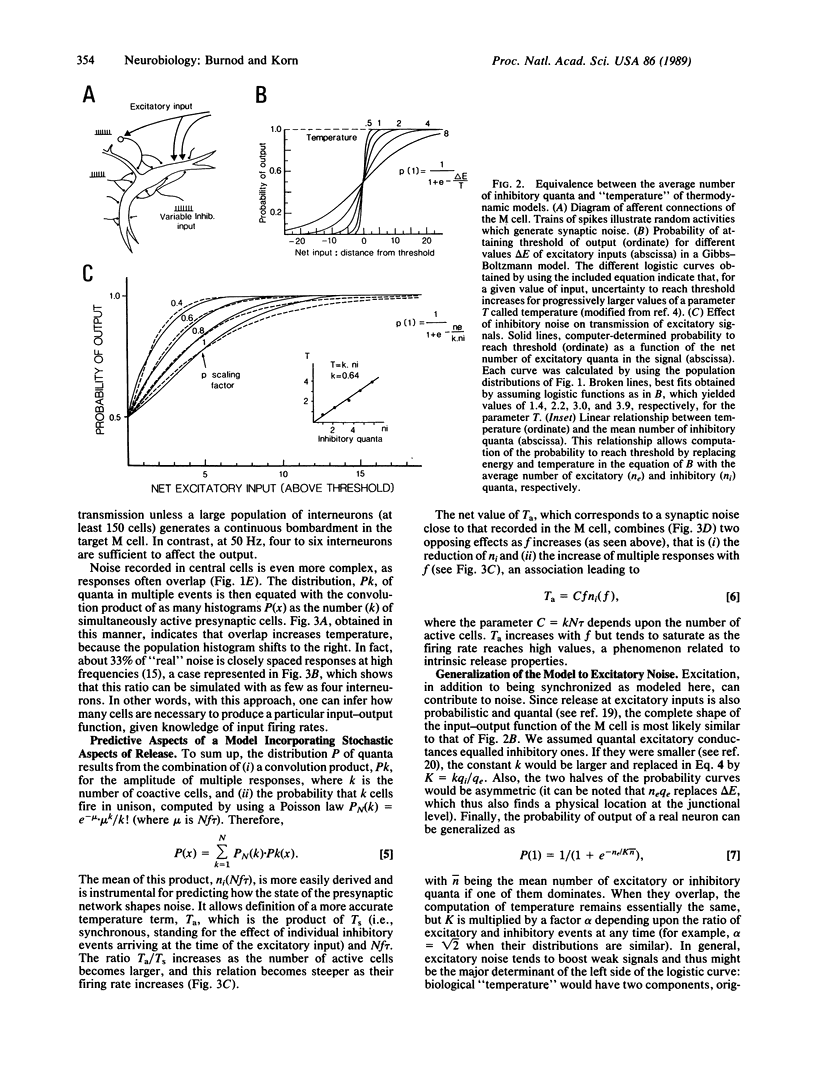
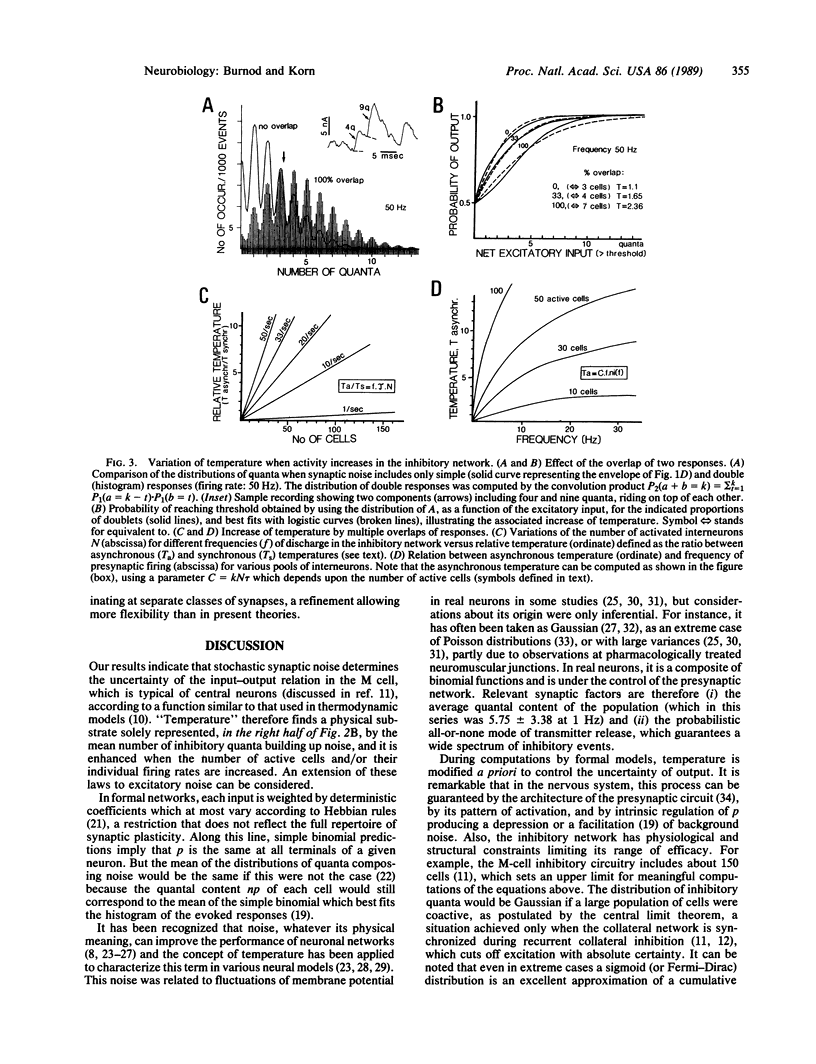
Abstract

Neuronal membrane potentials vary continuously due largely to background synaptic noise produced by ongoing discharges in their presynaptic afferents and shaped by probabilistic factors of transmitter release. We investigated how the random activity of an identified population of interneurons with known release properties influences the performance of central cells. In stochastic models such as thermodynamic ones, the probabilistic input-output function of a formal neuron is sigmoid, having its maximal slope inversely related to a variable called "temperature." Our results indicate that, for a biological neuron, the probability that given excitatory input signals reach threshold is also sigmoid, allowing definition of a temperature that is proportional to the mean number of quanta comprising noise and can be modified by activity in the presynaptic network, a notion which could be included in neural models. By introducing uncertainty to the input-output relation of central neurons, synaptic noise could be a critical determinant of neuronal computational systems, allowing assemblies of cells to undergo continuous transitions between states.

Full text

PDF
352

Images in this article

353Image
on p.353

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Amit DJ, Gutfreund H, Sompolinsky H. Spin-glass models of neural networks. Phys Rev A Gen Phys. 1985 Aug;32(2):1007–1018. doi: 10.1103/physreva.32.1007. [DOI] [PubMed] [Google Scholar]
  2. Buhmann J., Schulten K. Influence of noise on the function of a "physiological" neural network. Biol Cybern. 1987;56(5-6):313–327. doi: 10.1007/BF00319512. [DOI] [PubMed] [Google Scholar]
  3. Faber D. S., Korn H. Single-shot channel activation accounts for duration of inhibitory postsynaptic potentials in a central neuron. Science. 1980 May 9;208(4444):612–615. doi: 10.1126/science.6245449. [DOI] [PubMed] [Google Scholar]
  4. Hopfield J. J. Neural networks and physical systems with emergent collective computational abilities. Proc Natl Acad Sci U S A. 1982 Apr;79(8):2554–2558. doi: 10.1073/pnas.79.8.2554. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Jack J. J., Redman S. J., Wong K. The components of synaptic potentials evoked in cat spinal motoneurones by impulses in single group Ia afferents. J Physiol. 1981 Dec;321:65–96. doi: 10.1113/jphysiol.1981.sp013972. [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Kirkpatrick S., Gelatt C. D., Jr, Vecchi M. P. Optimization by simulated annealing. Science. 1983 May 13;220(4598):671–680. doi: 10.1126/science.220.4598.671. [DOI] [PubMed] [Google Scholar]
  7. Korn H., Burnod Y., Faber D. S. Spontaneous quantal currents in a central neuron match predictions from binomial analysis of evoked responses. Proc Natl Acad Sci U S A. 1987 Aug;84(16):5981–5985. doi: 10.1073/pnas.84.16.5981. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Korn H., Faber D. S., Burnod Y., Triller A. Regulation of efficacy at central synapses. J Neurosci. 1984 Jan;4(1):125–130. doi: 10.1523/JNEUROSCI.04-01-00125.1984. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Korn H., Faber D. S. Organizational and cellular mechanisms underlying chemical inhibition of a vertebrate neuron. Prog Brain Res. 1983;58:165–174. doi: 10.1016/S0079-6123(08)60017-5. [DOI] [PubMed] [Google Scholar]
  10. Korn H., Faber D. S., Triller A. Probabilistic determination of synaptic strength. J Neurophysiol. 1986 Feb;55(2):402–421. doi: 10.1152/jn.1986.55.2.402. [DOI] [PubMed] [Google Scholar]
  11. Korn H., Mallet A., Triller A., Faber D. S. Transmission at a central inhibitory synapse. II. Quantal description of release, with a physical correlate for binomial n. J Neurophysiol. 1982 Sep;48(3):679–707. doi: 10.1152/jn.1982.48.3.679. [DOI] [PubMed] [Google Scholar]
  12. Korn H., Triller A., Mallet A., Faber D. S. Fluctuating responses at a central synapse: n of binomial fit predicts number of stained presynaptic boutons. Science. 1981 Aug 21;213(4510):898–901. doi: 10.1126/science.6266015. [DOI] [PubMed] [Google Scholar]
  13. Lin J. W., Faber D. S. Synaptic transmission mediated by single club endings on the goldfish Mauthner cell. I. Characteristics of electrotonic and chemical postsynaptic potentials. J Neurosci. 1988 Apr;8(4):1302–1312. doi: 10.1523/JNEUROSCI.08-04-01302.1988. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Little W. A., Shaw G. L. A statistical theory of short and long term memory. Behav Biol. 1975 Jun;14(2):115–133. doi: 10.1016/s0091-6773(75)90122-4. [DOI] [PubMed] [Google Scholar]
  15. Peretto P. Collective properties of neural networks: a statistical physics approach. Biol Cybern. 1984;50(1):51–62. doi: 10.1007/BF00317939. [DOI] [PubMed] [Google Scholar]
  16. Sejnowski T. J. On the stochastic dynamics of neuronal interaction. Biol Cybern. 1976 May 17;22(4):203–211. doi: 10.1007/BF00365086. [DOI] [PubMed] [Google Scholar]
  17. Sompolinsky H. Neural networks with nonlinear synapses and a static noise. Phys Rev A Gen Phys. 1986 Sep;34(3):2571–2574. doi: 10.1103/physreva.34.2571. [DOI] [PubMed] [Google Scholar]
  18. Taylor J. G. Spontaneous behaviour in neural networks. J Theor Biol. 1972 Sep;36(3):513–528. doi: 10.1016/0022-5193(72)90006-9. [DOI] [PubMed] [Google Scholar]
  19. Toulouse G., Dehaene S., Changeux J. P. Spin glass model of learning by selection. Proc Natl Acad Sci U S A. 1986 Mar;83(6):1695–1698. doi: 10.1073/pnas.83.6.1695. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Triller A., Korn H. Transmission at a central inhibitory synapse. III. Ultrastructure of physiologically identified and stained terminals. J Neurophysiol. 1982 Sep;48(3):708–736. doi: 10.1152/jn.1982.48.3.708. [DOI] [PubMed] [Google Scholar]
  21. Tuckwell H. C. Synaptic transmission in a model for stochastic neural activity. J Theor Biol. 1979 Mar 7;77(1):65–81. doi: 10.1016/0022-5193(79)90138-3. [DOI] [PubMed] [Google Scholar]
  22. Usami H., Masaki S., Sato R. The stochastic properties of the basic neuron populations as information processing system. Biol Cybern. 1978 May 31;29(3):167–173. doi: 10.1007/BF00337353. [DOI] [PubMed] [Google Scholar]

Articles from Proceedings of the National Academy of Sciences of the United States of America are provided here courtesy of National Academy of Sciences

RESOURCES