Skip to main content
PMC home page
Philosophical transactions. Series A, Mathematical, physical, and engineering sciences logoLink to Philosophical transactions. Series A, Mathematical, physical, and engineering sciences
. 2010 Dec 28;368(1933):5605–5617. doi: 10.1098/rsta.2010.0268

Fractal structures in stenoses and aneurysms in blood vessels

Adriane B Schelin 1,*, György Károlyi 2, Alessandro P S de Moura 3, Nuala A Booth 4, Celso Grebogi 3
PMCID: PMC2981951  PMID: 21078637

Abstract

Recent advances in the field of chaotic advection provide the impetus to revisit the dynamics of particles transported by blood flow in the presence of vessel wall irregularities. The irregularity, being either a narrowing or expansion of the vessel, mimicking stenoses or aneurysms, generates abnormal flow patterns that lead to a peculiar filamentary distribution of advected particles, which, in the blood, would include platelets. Using a simple model, we show how the filamentary distribution depends on the size of the vessel wall irregularity, and how it varies under resting or exercise conditions. The particles transported by blood flow that spend a long time around a disturbance either stick to the vessel wall or reside on fractal filaments. We show that the faster flow associated with exercise creates widespread filaments where particles can get trapped for a longer time, thus allowing for the possible activation of such particles. We argue, based on previous results in the field of active processes in flows, that the non-trivial long-time distribution of transported particles has the potential to have major effects on biochemical processes occurring in blood flow, including the activation and deposition of platelets. One aspect of the generality of our approach is that it also applies to other relevant biological processes, an example being the coexistence of plankton species investigated previously.

Keywords: blood, chaotic advection, circulatory diseases

1. Introduction

Cardiovascular diseases cause about 17 million deaths worldwide each year (World Health Organization 2004). Abnormalities in blood flow, blood components and vessel wall, constituting Virchow’s triad (Lowe 2003), are the main contributors to the development of these serious diseases. In this paper, we show that chaotic motion of particles transported by blood also plays an important role in the formation of circulatory diseases. Vessel wall irregularities, being either constrictions (stenoses) or sudden enlargements (aneurysms), affect the flow field, which in turn changes in a complicated way the activation of particles like platelets.

The contribution of blood flow to the biochemical activity of particles is usually believed to be restricted to the effect of shear stress and residence time, and that both of these are easily determined by the flow velocity field. We show in this paper that this is not the case. In a time-dependent flow, the spatial distribution of the particles is not evident from the blood flow patterns. Rather, the trajectories of particles are much more complex and require further analysis. Indeed, recent advances in the field of nonlinear dynamics, and in particular in chaotic advection, show that flow pattern and the actual particle trajectories are typically and dramatically different in time-dependent flows (Aref 1984; Ottino 1989; Péntek et al. 1995; Károlyi & Tél 1997; Vilela et al. 2006). Even in laminar flows, particle motion is in general very complex displaying chaotic behaviour.

The chaotic dynamics of the transported particles leads to a peculiar filamentary distribution. For a wide range of active flows, this distribution has been shown to modify relevant chemical reactions (Toroczkai et al. 1998; Neufeld et al. 1999; Nugent et al. 2004; Károlyi & Tél 2005, 2007; Arratia & Gollub 2006) and population dynamical equations (Scheuring et al. 2003b), with applications ranging from atmospheric chemistry (Edouard et al. 1996; Grooss et al. 2005; Vilela & Motter 2007) to plankton population dynamics (Bracco et al. 2000) and evolution of life (Scheuring et al. 2003a); for a review see Tél et al. (2005).

The important conclusion from these studies is that the non-trivial, inhomogeneous, filamentary distribution of the chemically or biologically active particles has a very important consequence on their activity. In particular, the active particles spend a long time on filaments with long perimeter, hence activity is catalysed by fluid mixing. In recent years, this phenomenon has been extensively studied in relation to plankton dynamics, and it has been found that the chaotic plankton motion can give a possible explanation for the paradox of plankton (Károlyi et al. 2000). According to this paradox, the number of coexisting plankton species cannot exceed the number of limiting resources (Hardin 1960), which contradicts the obvious observation (Hutchinson 1961). The filamentary distribution of the particles results in an advantage of rarity for the weaker species that leads to coexistence. Besides the more traditional mechanisms, like environmental heterogeneity, predation, external disturbance, coevolution and seasonal variability, fluid mixing can also maintain biodiversity.

In blood, the role of transport in the biochemical activity of particles has been mostly neglected. Besides the complex distribution of particles with long residence times, the chaotic motion of particles also implies an (exponentially) strong separation of nearby particles, and hence a strong stretching is present along particle orbits. This stretching can lead to the activation of platelets, an effect that is stronger than the usually assumed influence of shear stress imposed by the flow velocity field.

Using simple numerical flow models, we show how recent results in chaotic advection affect the traditional view of transport and activation in blood flow. We also discuss the importance of different processes in blood vessels of various sizes and show examples of filamentary patterns traced out by particles transported by the flow.

The paper is organized as follows. In §2, chaotic advection in fluids is briefly reviewed. The numerical models used in our simulations are introduced in §3. In §4, we show the fractal spatial filamentary distribution of advected particles. Finally, in §5, we discuss the results and summarize our conclusions.

2. Chaotic advection

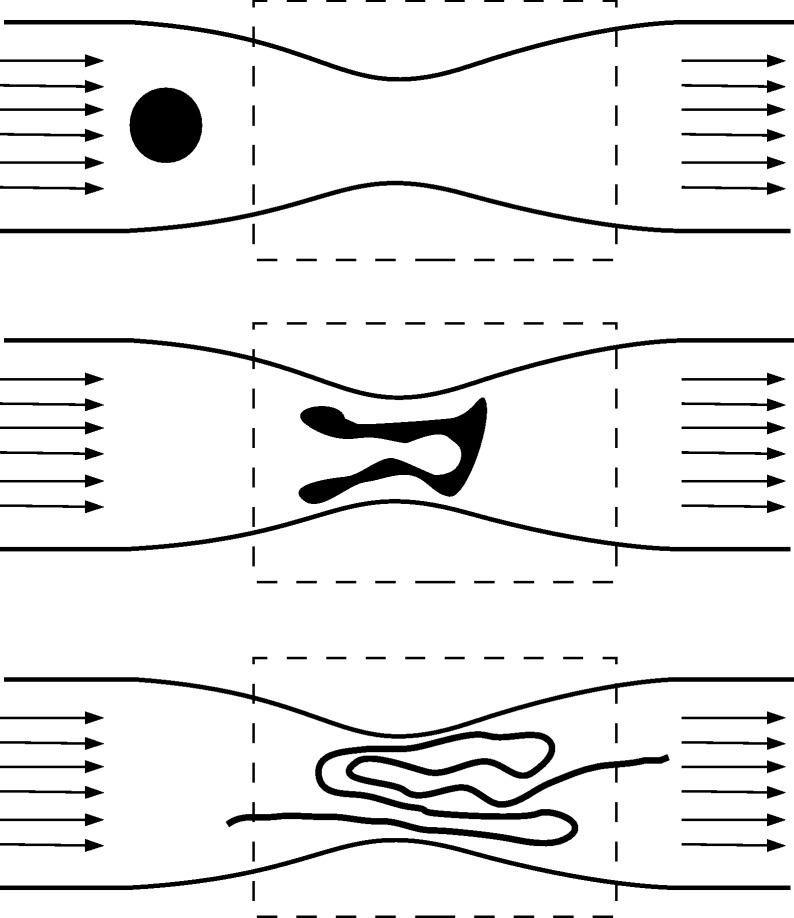
The chaotic motion of particles implies sensitive dependence on the initial conditions, that is, any two initially close particles rapidly (exponentially) deviate from each other. The average rate of this exponential divergence is measured by the Lyapunov exponent (Tél & Gruiz 2006), denoted by λ. Typically, two particles initially at a small distance δ(0) apart separate exponentially from each other, their average distance δ(t) at some time t later being given by 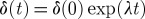Inline graphic. This exponential divergence occurs along specific curves forming the so-called unstable foliation (unstable manifold). If we take a set of particles, say a blob of dye injected into the flow, all particles rapidly diverge from each other along the unstable foliation, the dye droplet is stretched very rapidly, forming filaments in the fluid. Because the shape of the curves forming the unstable foliation is usually extremely distorted, the filaments become folded. This stretching and folding action is very typical in chaotic dynamical systems (Tél & Gruiz 2006). Figure 1 shows an illustration of such a mechanism in a constricted vessel.

Figure 1.

Figure 1.

Open in a new tab

Illustration of the unstable foliation: the schematic diagram shows the time evolution of a dye droplet that enters the region of observation (region inside the dashed rectangle). With time, the droplet deforms, by stretching and folding processes, and, after a long period, traces out the unstable manifold of the chaotic saddle.

Blood flow in vessels belongs to the category of open flows (Lamb 1932; Péntek et al. 1995). In open flows, there is a region of observation, such as the segment of the blood vessel around some irregularities (region inside the dashed rectangle in figure 1), into which the fluid transports the particles and later washes them out. Nonetheless, before leaving the region of observation, particles may exhibit a chaotic behaviour. In open flows, there is typically a set, the so-called chaotic set, composed by orbits that never leave the region of observation (Péntek et al. 1995; Tél & Gruiz 2006). The particle orbits of the chaotic set are not typical (in the sense that most other orbits leave the region of observation in finite time), and they are unstable (particles in their vicinity deviate from the chaotic set and leave the region of observation). However, their existence still governs the behaviour of the other particles. The particles that are transported close to this chaotic set will stroll in the vicinity of the trapped orbits for a long time, and eventually will leave them along the unstable foliation (Péntek et al. 1995; Tél & Gruiz 2006). Thus, particles staying for a long time close to the chaotic set, before transported downstream, trace out the unstable foliation (the unstable manifold of the chaotic set).

Geometrically, the chaotic set is a fractal set, and so is the unstable foliation (Kantz & Grassberger 1985; Péntek et al. 1995; Tél & Gruiz 2006). The unstable foliation is a strange mathematical object, consisting of an uncountable infinite number of filaments. The complexity of this object is measured by the fractal dimension (Kantz & Grassberger 1985; Tél & Gruiz 2006), a real number that generalizes the concept of ordinary dimension. For usual objects, the fractal dimension, denoted by D, is an integer number, the ordinary dimension of the object, say 1 for lines, 2 for planar objects and so on. For fractal geometrical objects D is, however, a non-integer (Tél & Gruiz 2006). Owing to the structure of the unstable foliation in two-dimensional flows—smooth curves along the foliation and fractal transverse to them—it has a dimension between 1 and 2. The dimension in smooth fluid flows is independent of time (Tél & Gruiz 2006), hence it is a valuable measure of the complexity of the unstable foliation.

3. Model

In our model, two main aspects control the advection of particles: (i) the perturbation of the flow (i.e. the shape of the vessel wall) and (ii) the time dependence of the background flow. Typically, each stage of the disease may generate a different scenario—the more severe is the disease, for instance, the more complicated the particle motion around the perturbation becomes. On the other hand, the time dependence of the flow, generated by its pulsative nature, also plays a central role in the particle dynamics. Different conditions, such as exercising or resting ones, allow for different scenarios even if the shape of the vessels is the same.

In order to simulate these different effects, we vary the stages of each disease by changing the size of the anomalies. For each size, we simulate the time dependence with exercising and resting conditions. We use simple two-dimensional models to reproduce blood flow both in stenosis in coronary arteries and in abdominal aortas with aneurysm. The diameter of the modelled coronary arteries is 4 mm; in the stenosed segment, the minimum diameter varies between 1 and 3 mm (in three dimensions, these would imply 93.75 and 43.75% occlusion, respectively). The length of the disturbed segment is 1 cm. For the model of the aneurysm, the normal diameter of the aorta is taken to be 2 cm. The maximum diameter at the aneurysm is varied between 3 and 6 cm, while the length of the dilated segment is 10 cm. Both for the stenosis and for the aneurysm the shape is sinusoidal, a form widely used in simulations and in experiments because of its simplicity (Kunov et al. 1996; Wootton et al. 2001).

To find the trajectories of particles transported by the blood, first the velocity field v(r,t) of the flow has to be computed. This is achieved by solving the Navier–Stokes equations with the available finite volume solver (Fluent), with a corresponding mesh generator (Gambit). The length of the time step used throughout the simulation is 0.001 s, fine enough to give the same result as that with smaller time steps. For such large vessels, we can consider the blood to be incompressible, Newtonian, with a constant dynamic viscosity μ=0.04 g cm−1 s−1, and the density of the blood is approximated to be ρ=1.06 g cm−3 (Ku 1997).

For the coronary artery, the model of the time dependence of the inlet velocity is given by idealized approximations of measured flow rates shown by Berry et al. (2000) for one heartbeat, those for the aorta are based on Taylor et al. (1999; see also Schelin et al. 2009). In the far upstream region, the inflow velocity is taken to be constant across the cross section, but the expected velocity profile has been checked to develop close to the inlet of the disturbance. During one heartbeat period, the inlet velocity v in our model is time-dependent, it varies between 0.4 m s−1 (peak velocity in case of systole in exercise) and a small back-flow component (diastole). This way, the Reynolds number Re=vdρ/η, where d is the blood vessel diameter, in our simulations falls between 0 (during diastole) and 2200 (during systole with peak velocity in the aorta). The higher end of this range is comparable with the transition to turbulence in pipe flows, and we indeed see irregular vortical structures in the fluid velocity field in our simulations.

Once we have the velocity field, v(r,t), the motion of the passively advected particles is simulated using the fourth order Runge–Kutta method, with a small fixed time step, to solve the equation

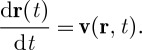3. 3.1

Passive advection implies that the particles take on the velocity of the fluid instantaneously, hence the inertia and the size of the particles are neglected. This is a reasonable assumption for platelets (of size of the order of a few micrometres) in arteries (of diameter above a few millimetres). An important dimensionless quantity that describes the importance of diffusion is the Péclet number Pe=dv/Ddiff, where Ddiff is the diffusion coefficient of the particle. In the case of platelets, Ddiff=10−7 cm2 s−1, which results in Pe=2×108 in blood vessels of diameter 1 cm with average velocity of 0.2 m s−1. This very large value indicates that the effects of diffusion are negligible when compared with the transport by fluid motion. This ideal view is somewhat modified by the presence of larger particles, like red blood cells, but in the large blood vessels we consider neglecting their presence for simplicity.

4. Results

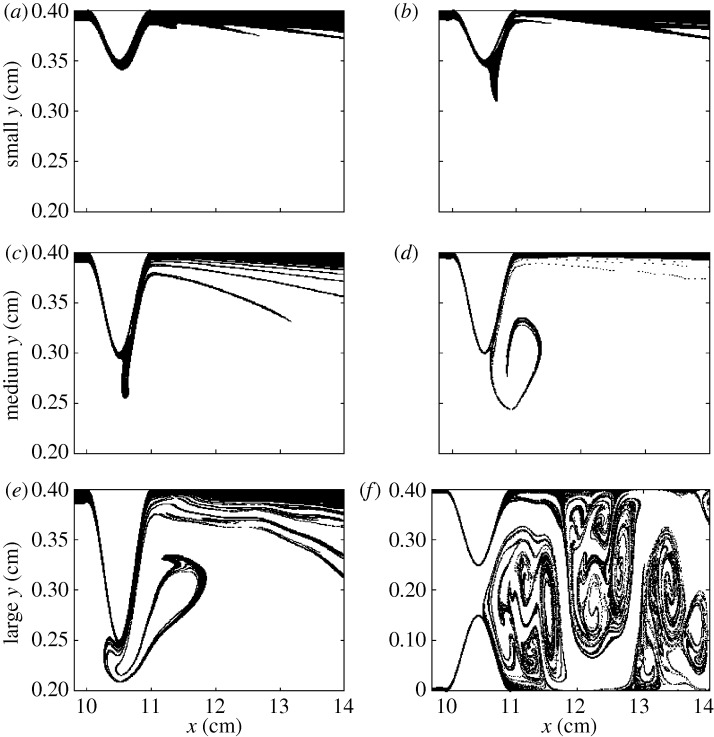
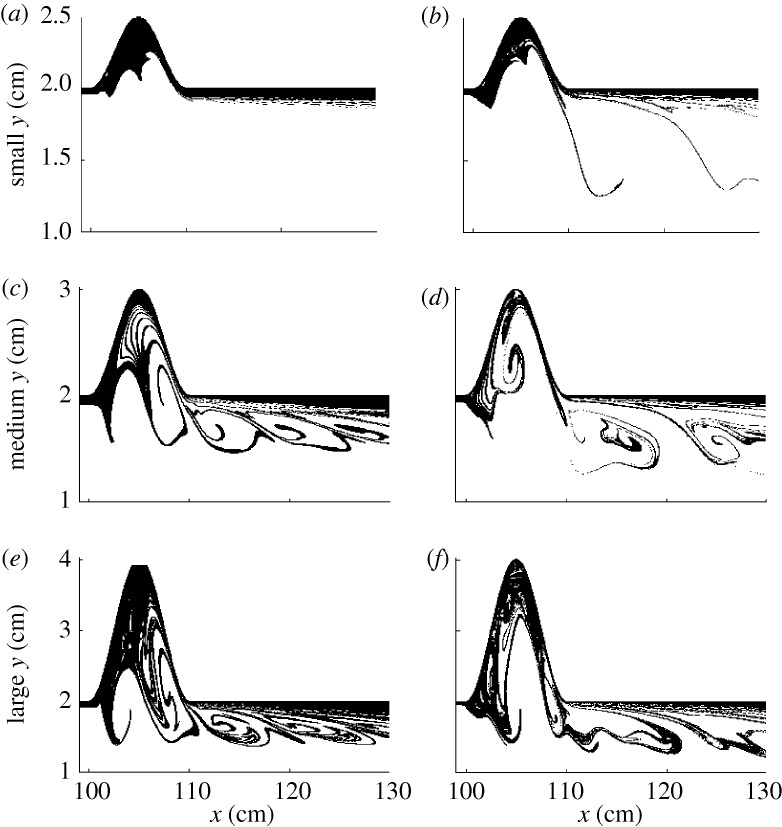
To see if there is chaotic advection in blood flow even in our simplified model, we follow the trajectories of many particles. To find numerically the unstable foliation (discussed in §2), we inject particles into the flow on a rectangular grid covering the region of observation. Then we plot the final position of those particles that stay for a long time, that is, that are not transported downstream in a set period of time. The patterns traced by this group of particles are shown in figure 2 for the model of the stenosis, and in figure 3 for the model of the aneurysm.

Figure 2.

Figure 2.

Open in a new tab

Snapshots of the unstable foliation for stenosed coronary arteries. The three rows correspond to small, medium and large stenosis, respectively, for (a,c,e) resting and (b,d,f) exercise condition. The scales on the vertical and horizontal axes are different for better visualization of the filaments. Flow is from left to right. Except for the large stenosis in exercise, only the upper half of the blood vessel segment is shown, the whole segment being symmetric to the y=0.2 cm line.

Figure 3.

Figure 3.

Open in a new tab

Snapshots of the unstable foliation for aorta arteries with aneurysms. The three rows correspond to small, medium and large aneurysms, respectively, for (a,c,e) resting and (b,d,f) exercise condition. The scales on the vertical and horizontal axes are different for better visualisation of the filaments. Flow is from left to right. Only the upper half of the blood vessel segment is shown, the whole segment being symmetric to the y=1 cm line.

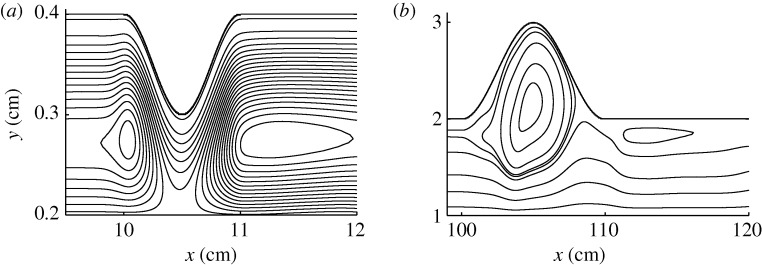
Figure 4a,b shows snapshots of the streamlines, characterizing the flow patterns, for a medium stenosis and aneurysm, respectively, during exercise. Despite the relative simplicity of the flow field, the particle distribution around the diseased area is generally very complex, as shown in figures 2d and 3d. Traditionally, studies on the contribution of blood flows to the biochemical processes involving particles, such as platelets, were restricted to analysis using simulations of the (Eulerian) velocity field. By comparing figure 4a,b with figures 2d and 3d, respectively, we can see that our (Lagrangian) approach deals with the particles themselves, which are the relevant entities in this problem.

Figure 4.

Figure 4.

Open in a new tab

Snapshots of the streamlines of blood flow in (a) a stenosed coronary artery and (b) an aorta with aneurysm. The scales on the vertical and horizontal axes are different for better visualisation. Flow is from left to right. Only the upper half of the blood vessel segment is shown, the whole segment being symmetric to the (a) y=0.2 cm and (b) y=1 cm line.

From figure 2, we can see that as the size of the obstacle increases, and also in exercise conditions, the trapped particles accumulate along filamentary structures detached from the wall. For resting conditions, there is a thick coverage of the vessel wall with particles spending a long time there, while in exercise conditions the particles with a significant residence time are to be found along filaments. Exercise thus generates a filamentary distribution of the transported particles, and destroys the non-filamentary component of the long-time distribution.

Similar observations can be made in the case of aneurysms. In figure 3, the snapshots of the unstable foliation are shown for aneurysms of different sizes and conditions. The filamentarity of the particle distribution that spent a long time within the vessel wall dilation increases as the size of the aneurysm increases (from top to bottom) and as we use exercise conditions instead of resting conditions. In the case of resting, large compact areas of long residence time are visible inside the vessel wall dilation. These break up and give way to the formation of more filamentary structures in the case of exercising. The presence of an underlying fractal structure suggests that extended particles, such as platelets, have their covalent bonds being severely stretched, resulting in their activation.

The degree of filamentarity of the unstable foliation is quantified by measuring the fractal dimension, D. To calculate D, we initialize many particles along a straight line, and measure the distribution of particles having long residence time. The number N of segments of length ε that, on the initial straight line, cover the initial position of particles with long residence time scales as N≈ε−D1 (Tél & Gruiz 2006), where D1 is the fractal dimension of the cross section of the unstable foliation. By measuring N numerically for various segment lengths ε, we can compute D1 for the various stenosis geometries and for resting and exercise conditions. From D1, the fractal dimension D of the whole unstable foliation can be obtained as D=D1+1. The results are summarized in table 1. The fractal dimension for both anomalies increases with the flow disturbance, and is larger in exercise than in resting condition. This shows that the filamentary fractal structures are more pronounced with the increase of the blood velocity and with the size of the disturbance.

Table 1.

Fractal dimension of the unstable manifold.

stenosis
 aneurysm
resting exercise resting exercise
small 2.00±0 1.59±0.02 2.00±0 1.69±0.01
medium 1.60±0.02 1.66±0.04 1.58±0.01 1.67±0.02
large 1.62±0.01 1.72±0.02 1.69±0.01 1.70±0.01
Open in a new tab

We also measured the mean residence time τ of the particles exhibiting chaotic motion before they are washed downstream. In our model, the residence time is the time it takes the particles to reach a pre-set distance downstream of the vessel wall irregularity. As the motion is chaotic while the particles are close to the stenosis or aneurysm, this is also the time while the particles exhibit chaotic motion. The results for the residence time are shown in table 2 for the stenoses and aneurysms of various severity both in resting and exercise conditions. For small disturbance in resting conditions, there was no visible chaotic motion. We see that the chaotic residence time decreases with exercise, which is the expected behaviour owing to increased blood velocity. It is less intuitive, however, that residence time decreases with the severity of the disturbance. The explanation of this observation is that the fluid fluctuates more wildly in the presence of large disturbances, which destroys the large vortices and washes out the bulk of the particles more rapidly.

Table 2.

Residence time (s).

stenosis
 aneurysm
resting exercise resting exercise
small — 82.0±1.3 — 5.32±1.80
medium 42.0±0.6 30.6±0.5 11.1±0.10 2.37±0.03
large 23.2±0.3 19.2±0.2 3.27±0.06 2.01±0.02
Open in a new tab

To measure the Lyapunov exponent λ, we use the data shown in tables 1 and 2. The residence time of a particle depends sensitively on the initial position of a particle. This means that the mean residence time τ of many particles depends strongly on the complexity of this dependence on the initial conditions, which is characterized by the Lyapunov exponent λ. This implies a relationship between the average time τ the particles spend in the region of observation, the Lyapunov exponent λ characterizing the dependence on initial conditions and the fractal dimension D describing the geometry of the unstable foliation as λ=1/(τ(2−D)) (Kantz & Grassberger 1985). The computed values of λ are shown in table 3. The more severe the flow disturbance is, the higher the value of the Lyapunov exponent becomes, and hence the more chaotic the particle motion is, the more rapidly the initially close particles deviate from each other. Exercise also increases the value of the Lyapunov exponent by imposing a more pulsating flow.

Table 3.

Lyapunov exponent (s−1).

stenosis
 aneurysm
resting exercise resting exercise
small 0 0.0297 0 0.608
medium 0.0594 0.0641 0.216 1.295
large 0.113 0.187 0.973 1.648
Open in a new tab

5. Discussion and conclusions

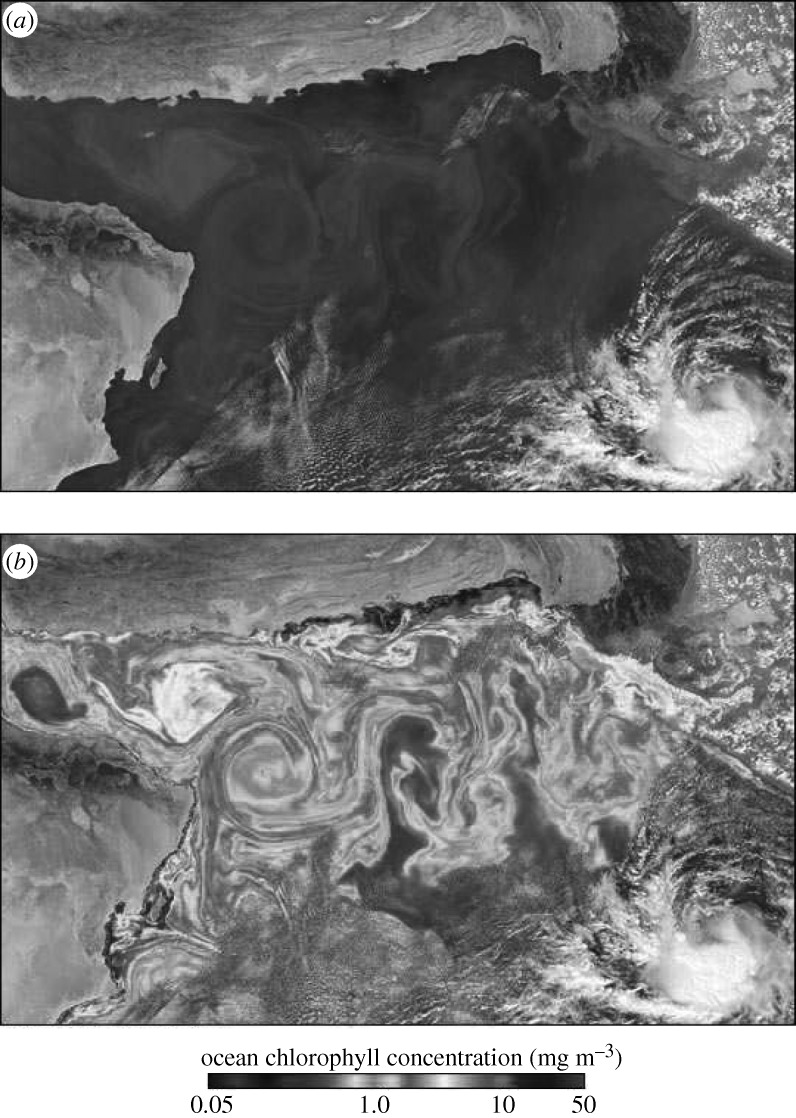
We have shown that filamentary fractal structures are present in blood flow in many situations, both in resting and exercise conditions. A more complete model could include a precise description of other biological factors of the blood flow. The important fact, however, should remain: that chaotic advection changes the way particles interact with each other and, thus, affects the development of circulatory diseases. The existence of fractal filaments has been shown to have a major effect on active processes in fluid flows. For instance, filamentary patterns emerge in the case of plankton populations. As an example, figure 5 shows a satellite image of a plankton bloom at the throat of the Arabian Sea. The situation is overall similar to a large stenosis, the pattern generated by the oceanic flow is reminiscent of unstable foliation observed in our experiments. This similarity is a consequence of the fact that fractal objects do not have a characteristic scale, they look similar on all scales, be it in a blood vessel (millimetre scale) or in oceanic currents (hundreds of kilometres).

Figure 5.

Figure 5.

Open in a new tab

Plankton bloom at the throat of the Arabian Sea, provided by the EOS Project Science Office, NASA Goddard Space Flight Center; see http://visibleearth.nasa.gov/view_rec.php?id=19638. (a) Natural colour and (b) chlorophyll concentrating.

In the case of plankton blooms, the role of advection has been uncovered during the last decade (Abraham 1998; Bracco et al. 2000; Károlyi et al. 2000; Neufeld et al. 2002; Martin 2003; Scheuring et al. 2003b). The fractal skeleton (Toroczkai et al. 1998) of the filamentary patterns traps the advected particles for a long time, where mixing is strong and the boundary between materials is very long. This results in a dramatic increase in the access of plankton species to resources, which alters the traditional population dynamical equations (Scheuring et al. 2003b). The reproduction rate P becomes dependent on the fractal dimension D of the unstable foliation in a non-trivial way, as P∼c−β where c is the concentration of plankton and β=(D−1)/(2−D) is a positive number. This is the advantage of rarity: the smaller the concentration is, the higher the reproduction rate becomes (Károlyi et al. 2000; Tél et al. 2005).

At the other end of the length scales, in blood vessels, there are different kinds of activity. As a particular example, we discuss the activation and deposition of platelets that play an important role in blood clotting and thrombus formation. Platelets can be activated by high shear in the blood (Kroll et al. 1996; Savage et al. 2002; Nesbitt et al. 2006), for example, by stretching the bond between the von Willebrand factor and platelet (Ruggeri 2002). We have seen that one consequence of the chaotic particle transport in fluid flows is the high stretching. One can speculate that this chaos-induced stretching can enhance platelet activation along the chaotic set, where the Lyapunov exponent is positive. Deposition is associated with stagnant regions in the flow: in these regions, the Lyapunov exponent has lower values, hence stretching is also lower. These processes should contribute to the further development of circulatory diseases and thus must be taken into account in the modelling of platelet dynamics.

Acknowledgements

This work was supported by the MRC discipline Hopping Award. A.B.S. was supported by FAPESP. C.G. and A.P.S.M. were supported by BBSRC under grant nos BB-F00513X and BS-G010722. G.K. was supported by OTKA grant nos K68415 and NK72037.

Footnotes

References

  1. Abraham E. The generation of plankton patchiness by turbulent stirring. Nature. 1998;391:577–580. ( doi:10.1038/35361) [Google Scholar]
  2. Aref H. Stirring by chaotic advection. J. Fluid Mech. 1984;143:1–21. ( doi:10.1017/S0022112084001233) [Google Scholar]
  3. Arratia P. E., Gollub J. P. Predicting the progress of diffusively limited chemical reactions in the presence of chaotic advection. Phys. Rev. Lett. 2006;96:024501. doi: 10.1103/PhysRevLett.96.024501. ( doi:10.1103/PhysRevLett.96.024501) [DOI] [PubMed] [Google Scholar]
  4. Berry J., Santamarina A., Moore J., Roychowdhury S., Routh W. Experimental and computational flow evaluation of coronary stents. Ann. Biomed. Eng. 2000;28:386–398. doi: 10.1114/1.276. ( doi:10.1114/1.276) [DOI] [PubMed] [Google Scholar]
  5. Bracco A., Provenzale A., Scheuring I.2000Mesoscale vortices and the paradox of the plankton Proc. R. Soc. Lond. B 2671795–1800(doi:10.1098/rspb.2000.1212) [DOI] [PMC free article] [PubMed] [Google Scholar]
  6. Edouard S., Legras B., Lefevre F., Eymard R. The effect of small-scale inhomogeneities on ozone depletion in the arctic. Nature. 1996;384:444–447. ( doi:10.1038/384444a0) [Google Scholar]
  7. Grooss J., Konopka P., Mueller R. Ozone chemistry during the 2002 Antarctic vortex split. J. Atmos. Sci. 2005;62:860–870. ( doi:10.1175/JAS-3330.1) [Google Scholar]
  8. Hardin G. The competitive exclusion principle. Science. 1960;131:1292–1298. doi: 10.1126/science.131.3409.1292. ( doi:10.1126/science.131.3409.1292) [DOI] [PubMed] [Google Scholar]
  9. Hutchinson G. The paradox of the plankton. Am. Nat. 1961;95:137–147. ( doi:10.1086/282171) [Google Scholar]
  10. Kantz H., Grassberger P. Repellers, semi-attractors, and long-lived chaotic transients. Physica D. 1985;17:75–86. ( doi:10.1016/0167-2789(85)90135-6) [Google Scholar]
  11. Károlyi G., Tél T. Chaotic tracer scattering and fractal basin boundaries in a blinking vortex-sink system. Phys. Rep. 1997;290:125–147. ( doi:10.1016/S0370-1573(97)00063-X) [Google Scholar]
  12. Károlyi G., Tél T. Chemical transients in closed chaotic flows: the role of effective dimensions. Phys. Rev. Lett. 2005;95:264501. doi: 10.1103/PhysRevLett.95.264501. ( doi:10.1103/PhysRevLett.95.264501) [DOI] [PubMed] [Google Scholar]
  13. Károlyi G., Tél T. Effective dimensions and chemical reactions in fluid flows. Phys. Rev. E. 2007;76:046315. doi: 10.1103/PhysRevE.76.046315. ( doi:10.1103/PhysRevE.76.046315) [DOI] [PubMed] [Google Scholar]
  14. Károlyi G., Péntek A., Scheuring I., Tél T., Toroczkai Z.2000Chaotic flow: the physics of species coexistence Proc. Natl Acad. Sci. USA 9713661–13665(doi:/10.1073/pnas.240242797) [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Kroll M., Hellums J., McIntire L., Schafer A., Moake J. Platelets and shear stress. Blood. 1996;88:1525–1541. [PubMed] [Google Scholar]
  16. Ku D. N. Blood flow in arteries. Annu. Rev. Fluid Mech. 1997;29:399–434. ( doi:10.1146/annurev.fluid.29.1.399) [Google Scholar]
  17. Kunov M. J., Steinman D. A., Ethier C. R. Particle volumetric residence time calculations in arterial geometries. J. Biomech. Eng. 1996;118:158–164. doi: 10.1115/1.2795954. ( doi:10.1115/1.2795954) [DOI] [PubMed] [Google Scholar]
  18. Lamb H. Hydrodynamics. Cambridge, UK: Cambridge University Press; 1932. [Google Scholar]
  19. Lowe G. Virchow’s triad revisited: abnormal flow. Pathophysiol. Haemost. Thromb. 2003;33:455–457. doi: 10.1159/000083845. ( doi:10.1159/000083845) [DOI] [PubMed] [Google Scholar]
  20. Martin A. Phytoplankton patchiness: the role of lateral stirring and mixing. Progr. Oceanogr. 2003;57:125–174. ( doi:10.1016/S0079-6611(03)00085-5) [Google Scholar]
  21. Nesbitt W., Mangin P., Salem H., Jackson S. The impact of blood rheology on the molecular and cellular events underlying arterial thrombosis. J. Mol. Med. 2006;84:989–995. doi: 10.1007/s00109-006-0101-1. ( doi:10.1007/s00109-006-0101-1) [DOI] [PubMed] [Google Scholar]
  22. Neufeld Z., Lopez C., Haynes P. H. Smooth-filamental transition of active tracer fields stirred by chaotic advection. Phys. Rev. Lett. 1999;82:2606–2609. ( doi:10.1103/PhysRevLett.82.2606) [Google Scholar]
  23. Neufeld Z., Haynes P. H., Garcon V., Sudre J. Ocean fertilization experiments may initiate a large scale phytoplankton bloom. Geophys. Res. Lett. 2002;29:1534–1537. ( doi:10.1029/2001GL013677) [Google Scholar]
  24. Nugent C. R., Quarles W. M., Solomon T. H. Experimental studies of pattern formation in a reaction–advection–diffusion system. Phys. Rev. Lett. 2004;93:218301. doi: 10.1103/PhysRevLett.93.218301. ( doi:10.1103/PhysRevLett.93.218301) [DOI] [PubMed] [Google Scholar]
  25. Ottino J. M. The kinematics of mixing: stretching, chaos, and transport. Cambridge, UK: Cambridge University Press; 1989. [Google Scholar]
  26. Péntek A., Toroczkai Z., Tél T., Grebogi C., Yorke J. A. Fractal boundaries in open hydrodynamical flows: signatures of chaotic saddles. Phys. Rev. E. 1995;51:4076–4088. doi: 10.1103/physreve.51.4076. ( doi:10.1103/PhysRevE.51.4076) [DOI] [PubMed] [Google Scholar]
  27. Ruggeri Z. M. Platelets in atherothrombosis. Nat. Med. 2002;8:1227–1234. doi: 10.1038/nm1102-1227. ( doi:10.1038/nm1102-1227) [DOI] [PubMed] [Google Scholar]
  28. Savage B., Sixma J. J., Ruggeri Z. M.2002Functional self-association of von Willebrand factor during platelet adhesion under flow Proc. Natl Acad. Sci. USA 99425–430(doi:10.1073/pnas.012459599) [DOI] [PMC free article] [PubMed] [Google Scholar]
  29. Schelin A. B., Károlyi G., de Moura A. P. S., Booth N. A., Grebogi C. Chaotic advection in blood flow. Phys. Rev. E. 2009;80:016213. doi: 10.1103/PhysRevE.80.016213. ( doi:10.1103/PhysRevE.80.016213) [DOI] [PMC free article] [PubMed] [Google Scholar]
  30. Scheuring I., Czárán T., Szabó P., Károlyi G., Toroczkai Z.2003aSpatial models of prebiotic evolution: soup before pizza? Orig. Life Evol. Biosph. 33319–355.(doi:10.1023/A:1025742505324) [DOI] [PubMed] [Google Scholar]
  31. Scheuring I., Károlyi G., Toroczkai Z., Tél T., Péntek A.2003bCompeting populations in flows with chaotic mixing Theor. Popul. Biol. 6377–90.(doi:10.1016/S0040-5809(02)00035-7) [DOI] [PubMed] [Google Scholar]
  32. Taylor C. A., Hughes T. J., Zarins C. K. Effect of exercise on hemodynamic conditions in the abdominal aorta. J. Vasc. Surg. 1999;29:1077–1089. doi: 10.1016/s0741-5214(99)70249-1. ( doi:10.1016/S0741-5214(99)70249-1) [DOI] [PubMed] [Google Scholar]
  33. Tél T., Gruiz M. Chaotic dynamics. Cambridge, UK: Cambridge University Press; 2006. [Google Scholar]
  34. Tél T., de Moura A., Grebogi C., Károlyi G.2005Chemical and biological activity in open flows: a dynamical system approach Phys. Rep. 41391–196(doi:10.1016/j.physrep.2005.01.005) [Google Scholar]
  35. Toroczkai Z., Károlyi G., Péntek A., Tél T., Grebogi C. Advection of active particles in open chaotic flows. Phys. Rev. Lett. 1998;80:500–503. ( doi:10.1103/PhysRevLett.80.500) [Google Scholar]
  36. Vilela R. D., Motter A. E. Can aerosols be trapped in open flows? Phys. Rev. Lett. 2007;99:264101. doi: 10.1103/PhysRevLett.99.264101. ( doi:10.1103/PhysRevLett.99.264101) [DOI] [PubMed] [Google Scholar]
  37. Vilela R. D., de Moura A. P. S., Grebogi C. Finite-size effects on open chaotic advection. Phys. Rev. E. 2006;73:026302. doi: 10.1103/PhysRevE.73.026302. ( doi:10.1103/PhysRevE.73.026302) [DOI] [PubMed] [Google Scholar]
  38. Wootton D., Markou C., Hanson S., Ku D. A mechanistic model of acute platelet accumulation in thrombogenic stenoses. Ann. Biomed. Eng. 2001;29:321–329. doi: 10.1114/1.1359449. ( doi:10.1114/1.1359449) [DOI] [PubMed] [Google Scholar]
  39. World Health Organization. Cardiovascular disease: the atlas of heart disease and stroke. 2004. See http://www.who.int .

Articles from Philosophical transactions. Series A, Mathematical, physical, and engineering sciences are provided here courtesy of The Royal Society

RESOURCES