Abstract
Coherent control of quantum states is at the heart of implementing solid-state quantum processors and testing quantum mechanics at the macroscopic level. Despite significant progress made in recent years in controlling single- and bi-partite quantum systems, coherent control of quantum wave function in multipartite systems involving artificial solid-state qubits has been hampered due to the relatively short decoherence time and lack of precise control methods. Here we report the creation and coherent manipulation of quantum states in a tripartite quantum system, which is formed by a superconducting qubit coupled to two microscopic two-level systems (TLSs). The avoided crossings in the system's energy-level spectrum due to the qubit–TLS interaction act as tunable quantum beam splitters of wave functions. Our result shows that the Landau–Zener–Stückelberg interference has great potential in precise control of the quantum states in the tripartite system.
 Coherent control of solid-state multi-qubit systems is
highly desirable for quantum information. Here the authors show coupling, and control through
Landau–Zener interference, of a superconducting qubit and two microscopic two-level systems, creating
an interesting platform for quantum computation.
Coherent control of solid-state multi-qubit systems is
highly desirable for quantum information. Here the authors show coupling, and control through
Landau–Zener interference, of a superconducting qubit and two microscopic two-level systems, creating
an interesting platform for quantum computation.
As one of three major forms of superconducting qubits1,2,3, a flux-biased
superconducting phase qubit4,5 consists of a superconducting loop with
inductance L interrupted by a Josephson junction (Fig. 1a). The
superconducting phase difference ϕ across the junction serves as the quantum variable
of coordinate. When biased close to the critical current I0, the qubit can
be thought of as a tunable artificial atom with discrete energy levels that exist in a
potential energy landscape determined by the circuit design parameters and bias (Fig. 1b). The ground state |0 and the first excited state |1
and the first excited state |1 are usually chosen as the computational basis states of the phase qubit.
The energy difference between |1
are usually chosen as the computational basis states of the phase qubit.
The energy difference between |1 and |0
and |0 , ω10, decreases with flux bias. A
TLS is phenomenologically understood to be an atom or a small group of atoms tunnelling
between two lattice configurations inside the Josephson tunnel barrier, with different wave
functions |L
, ω10, decreases with flux bias. A
TLS is phenomenologically understood to be an atom or a small group of atoms tunnelling
between two lattice configurations inside the Josephson tunnel barrier, with different wave
functions |L and |R
and |R corresponding to different critical currents (Fig. 1c). Under the interaction picture of the qubit–TLS system, the state
of the TLS can be expressed in terms of the eigenenergy, with |g
corresponding to different critical currents (Fig. 1c). Under the interaction picture of the qubit–TLS system, the state
of the TLS can be expressed in terms of the eigenenergy, with |g being the ground
state and |e
being the ground
state and |e the excited state. When the energy difference between |e
the excited state. When the energy difference between |e and |g
and |g ,
ħωTLS=Ee−Eg, is close to
ℏω10 (ħ≡h/2π, where h is Planck's constant),
coupling between the phase qubit and the TLS becomes significant, which could result in
increased decoherence4,5. On the other hand, one can use strong qubit–TLS
coupling to demonstrate coherent macroscopic quantum phenomena and/or quantum information
processing6,7,8. For instance, recently, a tetrapartite system formed by
two qubits, one cavity and one TLS, has been studied5. However, although
multipartite spectral property and vacuum Rabi oscillation have been observed, coherent
manipulation of the quantum states of the whole system has not yet been demonstrated.
,
ħωTLS=Ee−Eg, is close to
ℏω10 (ħ≡h/2π, where h is Planck's constant),
coupling between the phase qubit and the TLS becomes significant, which could result in
increased decoherence4,5. On the other hand, one can use strong qubit–TLS
coupling to demonstrate coherent macroscopic quantum phenomena and/or quantum information
processing6,7,8. For instance, recently, a tetrapartite system formed by
two qubits, one cavity and one TLS, has been studied5. However, although
multipartite spectral property and vacuum Rabi oscillation have been observed, coherent
manipulation of the quantum states of the whole system has not yet been demonstrated.
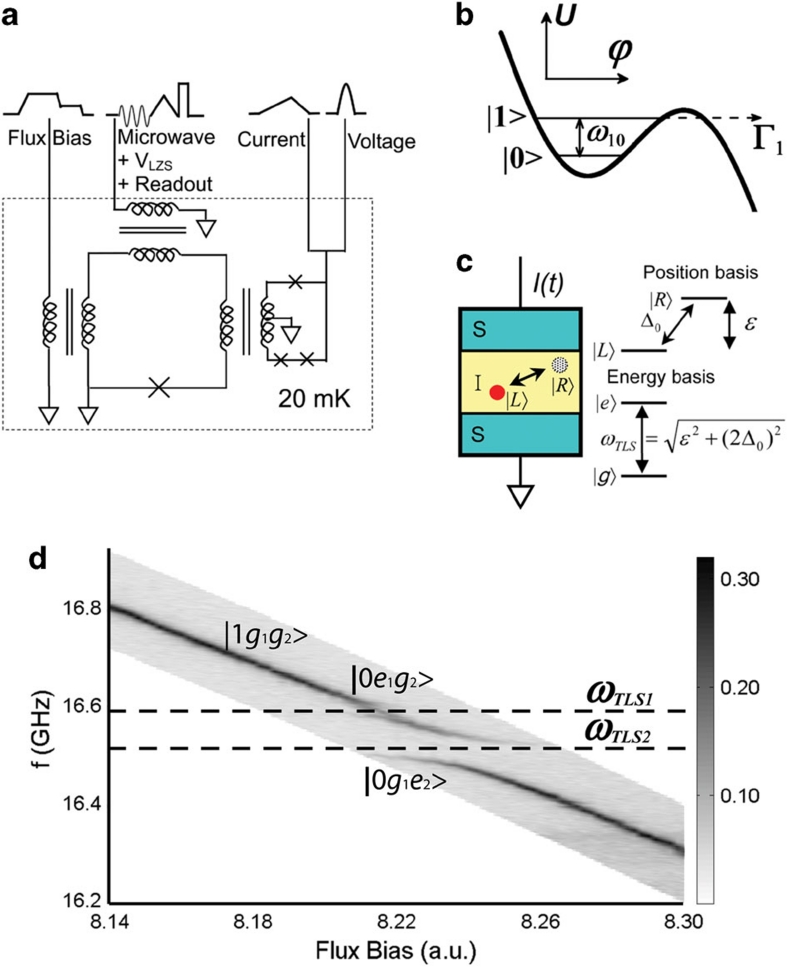
Figure 1. Qubit circuit and experimental procedure.
(a) Schematic of the qubit circuitry. Josephson junctions Al/AlOx/Al are denoted
by the X symbols. The flux bias, microwave and readout dc-SQUID are inductively coupled
to the qubit with inductance L≈770 pH, capacitance C≈240 fF and critical
current I0≈1.4 μA. (b) Principle of the operation and
measurement of the phase qubit. The two lowest eigenstates, |0 and |1
and |1 , form the qubit
with transition frequency ω10, which can be adjusted by changing the
flux bias. A microwave pulse is used to manipulate the qubit state and readout pulse and
then lower the potential energy barrier to perform a fast single-shot readout.
(c) Schematic of a two-level state located inside the insulating tunnel barrier
of a Josephson junction and its eigenstates in different bases. (d) Spectroscopy
of the coupled qubit–TLS system with corresponding quantum states labelled. Two avoided
crossings centered at ωTLS1 and ωTLS2 are
observed.
, form the qubit
with transition frequency ω10, which can be adjusted by changing the
flux bias. A microwave pulse is used to manipulate the qubit state and readout pulse and
then lower the potential energy barrier to perform a fast single-shot readout.
(c) Schematic of a two-level state located inside the insulating tunnel barrier
of a Josephson junction and its eigenstates in different bases. (d) Spectroscopy
of the coupled qubit–TLS system with corresponding quantum states labelled. Two avoided
crossings centered at ωTLS1 and ωTLS2 are
observed.
In our experiments, we use two TLSs near 16.5 GHz to form a hybrid tripartite9,10,11 phase qubit–TLS system and demonstrate Landau–Zener–Stückelberg (LZS) interference in such a tripartite system. The avoided crossings due to the qubit–TLS interaction act as tunable quantum beam splitters of wave functions, with which we could precisely control the quantum states of the system.
Results
Experimental results of LZS interference
Figure 1d shows the measured spectroscopy of a phase qubit. The
spectroscopy data clearly show two avoided crossings resulting from qubit–TLS coupling.
As, after application of the π-pulse, the system has absorbed exactly one microwave
photon and the subsequent steps of state manipulation are accomplished in the absence of
the microwave, conservation of energy guarantees that one and only one of the qubit, TLS1
and TLS2, can be coherently transferred to its excited state. Thus, only 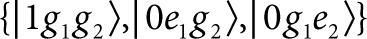 as marked in Figure 1d, are involved in the dynamics of the system. Notice that these three
basis states form a generalized W state10,11,12,
as marked in Figure 1d, are involved in the dynamics of the system. Notice that these three
basis states form a generalized W state10,11,12, 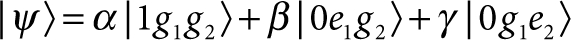 which preserves entanglement
between the remaining bipartite system even when one of the qubits is lost and has been
recognized as an important resource in quantum information science13. The
system's effective Hamiltonian can be written as
which preserves entanglement
between the remaining bipartite system even when one of the qubits is lost and has been
recognized as an important resource in quantum information science13. The
system's effective Hamiltonian can be written as
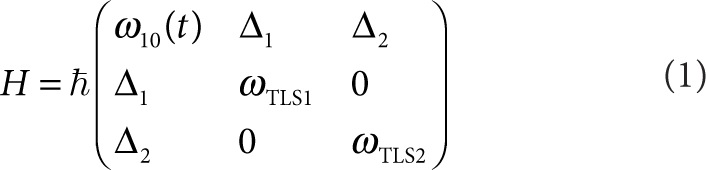 |
1 |
where Δ1 (Δ2) is the coupling strength between the qubit and TLS1
(TLS2). ωTLS1 (ωTLS2) is the resonant frequency of
TLS1 (TLS2). ω10(t)=ω10,dc−sΦ(t),
with ω10,dc being the initial energy detuning controlled by the dc flux
bias line (that is, the second platform holds in the dc flux bias line),
s=|dω10(Φ)/dΦ| being the diabatic energy-level slope of state
|1g1g2 and Φ(t) being the time-dependent
flux bias (Fig. 1a).
and Φ(t) being the time-dependent
flux bias (Fig. 1a).
In our experiment, coherent quantum control of multiple qubits is realized with LZ
transition. When the system is swept through the avoided crossing, the asymptotic
probability of transmission is exp(−2π(Δ2/ν)), where
ħν≡dE/dt denotes the rate of the energy spacing change for
noninteracting levels, and 2ħΔ is the minimum energy gap. It ranges from 0 to 1,
depending on the ratio of Δ and ν. The avoided crossing serves as a beam splitter
that splits the initial state into a coherent superposition of two states14. These two states evolve independently in time, while a relative phase is accumulated,
causing interference after sweeping back and forth through the avoided crossing. Such LZS
interference has been observed recently in superconducting qubits15,16,17,18,19,20,21,22. However, in these experiments the avoided
crossings of the single-qubit energy spectrum are used, and microwaves, whose phase is
difficult to control, are applied to drive the system through the avoided crossing
consecutively to manipulate the qubit state. Here we use a triangular bias waveform with
width shorter than the qubit's decoherence time to coherently control the quantum state of
the tripartite system. The use of a triangular waveform, with a time resolution of 0.1 ns,
ensures precise control of the flux bias sweep at a constant rate and thus the quantum
state. The qubit is initially prepared in |0g1g2 . A
resonant microwave π-pulse is applied to coherently transfer the qubit to
|1g1g2
. A
resonant microwave π-pulse is applied to coherently transfer the qubit to
|1g1g2 . A triangular flux bias, Φ(t), with
variable width T and amplitude ΦLZS
. A triangular flux bias, Φ(t), with
variable width T and amplitude ΦLZS
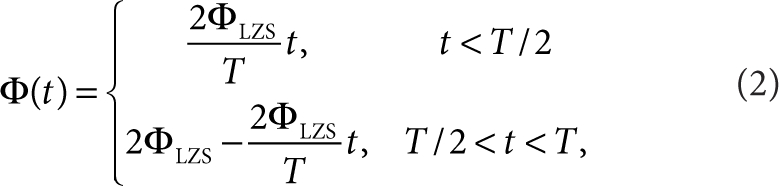 |
2 |
is then applied immediately to the phase qubit to induce LZ transitions (Fig. 2d). This is followed by a short readout pulse (about 5 ns) to determine
the probability of finding the qubit in the state |1 , that is, the system in the state
|1g1g2
, that is, the system in the state
|1g1g2 .
.
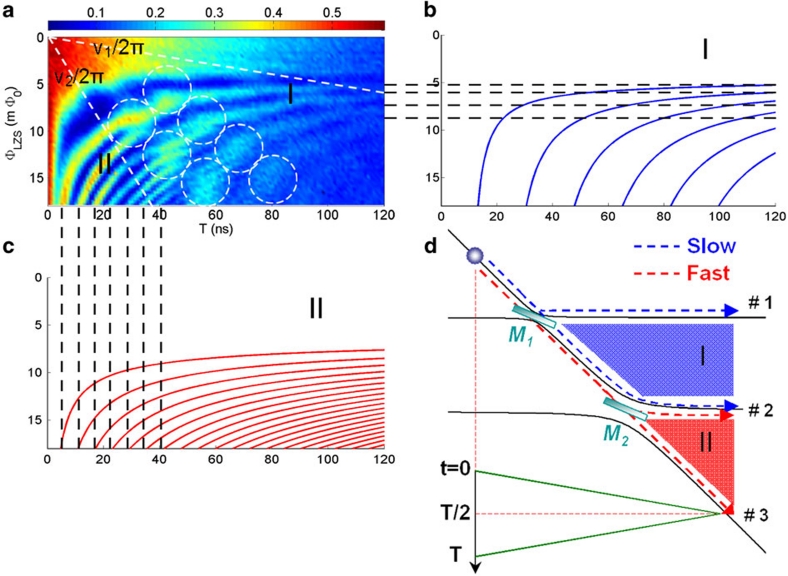
Figure 2. LZS interference in a phase qubit coupled to two TLSs.
(a) The population of |1 measured immediately (a few ns) after the triangular
flux pulse is plotted as a function of the width and amplitude of the triangular flux
bias waveform. The oblique dotted lines are lines of constant characteristic sweeping
rates, ν1 and ν2, defined in the text. The white
circles mark the 'hot spots', where the interference fringes generated by
M2 tend to fade out and the interference fringes generated by
M1 dominate. (b, c) Analytically calculated
constructive interference strips in regions I and II, respectively. The horizontal and
vertical dotted lines indicate the corresponding locations of interference strips.
(b, c) have the same axis labels as (a). (d) Schematic of
generating LZS interference with tunable beam splitters in a phase qubit coupled to two
TLSs. M1 and M2 correspond to the TLSs with smaller
and larger avoided crossings in Figure 1d, respectively.
measured immediately (a few ns) after the triangular
flux pulse is plotted as a function of the width and amplitude of the triangular flux
bias waveform. The oblique dotted lines are lines of constant characteristic sweeping
rates, ν1 and ν2, defined in the text. The white
circles mark the 'hot spots', where the interference fringes generated by
M2 tend to fade out and the interference fringes generated by
M1 dominate. (b, c) Analytically calculated
constructive interference strips in regions I and II, respectively. The horizontal and
vertical dotted lines indicate the corresponding locations of interference strips.
(b, c) have the same axis labels as (a). (d) Schematic of
generating LZS interference with tunable beam splitters in a phase qubit coupled to two
TLSs. M1 and M2 correspond to the TLSs with smaller
and larger avoided crossings in Figure 1d, respectively.
Figure 2a shows the measured population of |1 as a function of
T and ΦLZS. On the top part of the plot, the amplitude is so small
that the state could not reach the first avoided crossing M1. Therefore,
no LZ transition could occur and only a trivial monotonic behaviour is observed. When the
amplitude is large enough to reach M1, the emerging interference pattern
can be qualitatively divided into three regions with remarkably different fringe
patterns.
as a function of
T and ΦLZS. On the top part of the plot, the amplitude is so small
that the state could not reach the first avoided crossing M1. Therefore,
no LZ transition could occur and only a trivial monotonic behaviour is observed. When the
amplitude is large enough to reach M1, the emerging interference pattern
can be qualitatively divided into three regions with remarkably different fringe
patterns.
Quantitative comparison with the model
To quantitatively model the data, we calculate the probability to return to the initial state P1 by considering the action of the unitary operations on the initially prepared state. Neglecting relaxation and dephasing, we find
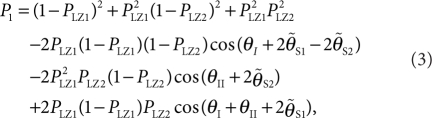 |
3 |
where PLZi (i=1,2) is the LZ transition probability at
the ith avoided crossing Mi, and θI and
θII are the phases accumulated in regions I and II, respectively
(Fig. 2b). The phase jump 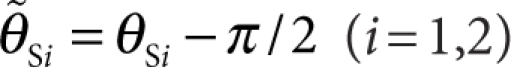 at the ith avoided crossing is due to the Stokes phase16,22
θSi, which depends on the adiabaticity parameter
ηi=Δi2/ν in the form
θSi=π/4+ηi(ln
ηi−1)+arg Γ(1−iηi), where
Γ is the Gamma function. In the adiabatic limit θS→0, while in
the sudden limit θS=π/4. In order to give a clear physical
picture, hereafter we adopt the terminology of optics to discuss the phenomenon and its
mechanism. First of all we define two characteristic sweeping rates of
ν1 and ν2 from
2πΔi2/νi=1 (i=1,
2). From the spectroscopy data, we have Δ1/2π=10 MHz and
Δ2/2π=32 MHz; thus,
ν1/2π=3.94×10−3 GHz ns−1 and
ν2/2π=4.04×10−2 GHz ns−1,
respectively. These lines of constant sweeping rate characteristic to the system are
marked as oblique dotted lines in Figure 2a. The avoided crossings
M1 (M2) can be viewed as wave function splitters
with controllable transmission coefficients set by the sweeping rate ν.
ν1 and ν2 thereby define three regions in the
T−ΦLZS parameter plane that contain all main features of the measured
interference patterns:
at the ith avoided crossing is due to the Stokes phase16,22
θSi, which depends on the adiabaticity parameter
ηi=Δi2/ν in the form
θSi=π/4+ηi(ln
ηi−1)+arg Γ(1−iηi), where
Γ is the Gamma function. In the adiabatic limit θS→0, while in
the sudden limit θS=π/4. In order to give a clear physical
picture, hereafter we adopt the terminology of optics to discuss the phenomenon and its
mechanism. First of all we define two characteristic sweeping rates of
ν1 and ν2 from
2πΔi2/νi=1 (i=1,
2). From the spectroscopy data, we have Δ1/2π=10 MHz and
Δ2/2π=32 MHz; thus,
ν1/2π=3.94×10−3 GHz ns−1 and
ν2/2π=4.04×10−2 GHz ns−1,
respectively. These lines of constant sweeping rate characteristic to the system are
marked as oblique dotted lines in Figure 2a. The avoided crossings
M1 (M2) can be viewed as wave function splitters
with controllable transmission coefficients set by the sweeping rate ν.
ν1 and ν2 thereby define three regions in the
T−ΦLZS parameter plane that contain all main features of the measured
interference patterns:
(I) ν ν1 and ν
ν1 and ν ν2: M1 acts as a beam splitter and
M2 acts as a total reflection mirror, that is,
PLZ1
ν2: M1 acts as a beam splitter and
M2 acts as a total reflection mirror, that is,
PLZ1 1/2 and
PLZ2
1/2 and
PLZ2 0. In
this case, equation (3) can be simplified as
0. In
this case, equation (3) can be simplified as
| 4 |
Apparently, only path 1 and path 2 contribute to the interference. The phase accumulated in region I can be expressed as
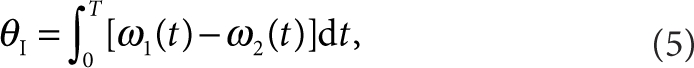 |
5 |
where ωi(t) (i=1, 2) denotes the energy frequency corresponding to path i (i=1, 2). It is easy to find that P1 is maximized (constructive interference) in the condition
| 6 |
from which we can obtain the analytical expression for the positions of constructive interference fringes
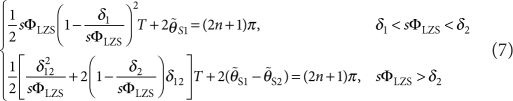 |
7 |
where δ1=ω10,dc−ωTLS1, δ2=ω10,dc−ωTLS2, and δ12=ωTLS1−ωTLS2.
In Figure 2b we show the calculated constructive interference strips, which agree well with the experimental results. Especially, in the limit of sΦLZS>>δ2, δ12, equation (7) can be simplified as
| 8 |
Intuitively, this result is straightforward to understand, as in the large-amplitude limit the accumulated phase θ1 is two times the area of a rectangle with length T/2 and width ωTLS1−ωTLS2.
(II) ν ν2 and ν>>ν1:
M1 acts as a total transmission mirror and M2 acts
as a beam splitter, that is, PLZ1
ν2 and ν>>ν1:
M1 acts as a total transmission mirror and M2 acts
as a beam splitter, that is, PLZ1 1 and PLZ2
1 and PLZ2 1/2. In this case, equation (3) can be simplified as
1/2. In this case, equation (3) can be simplified as
| 8 |
Only path 2 and path 3 contribute to the interference. Using the same method in dealing with region I, we obtain the analytical formula governing the positions of constructive interference fringes:
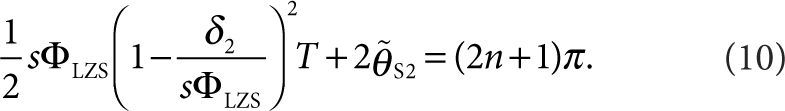 |
9 |
As shown in Figure 2c, the positions of the constructive interference fringes obtained from equation (10) agree with experimental results very well. Similarly, in the limit sΦLZS>>δ2, equation (10) has the simple form,
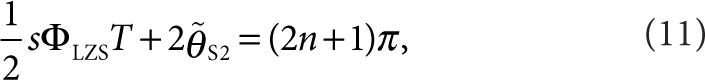 |
11 |
which is also readily understood because in the large-amplitude limit the accumulated phase θII is two times the area of a triangle with base length T/2 and height sΦLZS.
(III) ν1<ν<ν2: This region is more interesting and complex. Here, M1 acts as a beam splitter, while M2 can act either as a beam splitter or as a total reflection mirror. This effect cannot be described by the asymptotic LZ formula because in this region LZS interference occurs only in a relatively small range around the avoided crossings. As the analytical solution is extremely complicated and does not provide clear intuition about the underlying physics, we use a numerically calculated LZ transition probability PLZ corresponding to the transmission coefficient of M1 and M2 for comparison with the experimental data. We find that for certain sweeping rates, LZ transition probability resulting from M2 is quite low. Therefore, M2 can be treated as a total reflection mirror, while M1 is still acting as a good beam splitter. The interference fringes generated by M2 thus disappear (the fringes tend to fade out) and the interference fringes generated by M1 dominate, displayed as a chain of 'hot spots' marked by the circles in Figure 2a.
When both M1 and M2 can be treated as beam splitters, all three paths (1, 2, and 3) contribute to the interference. According to equation (3), P1 is maximized in the condition
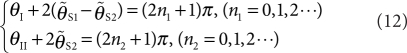 |
12 |
It is noted that under this condition the term 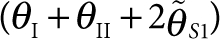 in equation (3) equals 2nπ. Considering different weights in
each path, it is more convenient to obtain a theoretical prediction from a numerical
simulation. Here we utilize the Bloch equation to describe the time evolution of the
density operator of the tripartite system:
in equation (3) equals 2nπ. Considering different weights in
each path, it is more convenient to obtain a theoretical prediction from a numerical
simulation. Here we utilize the Bloch equation to describe the time evolution of the
density operator of the tripartite system:
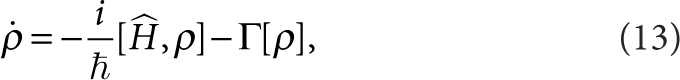 |
13 |
where Γ[ρ] includes the effects of energy relaxation. Figure
3a shows the calculated population of |1 as a function of T and
ΦLZS. Figure 3b shows the extracted data for different
T and ΦLZS values. The agreement between the theoretical and
experimental results is remarkable. In order to better understand the origin of the 'hot
spots', we also plot the probabilities of LZ transition as a function of the pulse width
at fixed amplitude ΦLZS=10mΦ0 (Fig.
3c). Notice that both LZ transition probabilities oscillate with T, which
are quite different from the general asymptotic LZ transition probabilities. The
transition probability at M1 is always greater because Δ1 is
much smaller than Δ2. The three oblique dotted lines in Figure
3a represent lines of constant sweeping rate. The 'hot spots' are located on
these lines, where the transition probability of M2 is a minimum.
M2 thereby acts as a total reflection mirror, resulting in the 'hot
spots' in transition probability. This feature further confirms that the avoided crossings
play the role of quantum mechanical wave function splitters, analogous to continuously
tunable beam splitters in optical experiments. The transmission coefficient of the wave
function splitters (the avoided crossings) in our experiment can be varied in situ
from zero (total reflection) to unity (total transmission) or any value in between by
adjusting the duration and amplitude of the single triangular bias waveform used to sweep
through the avoided crossings.
as a function of T and
ΦLZS. Figure 3b shows the extracted data for different
T and ΦLZS values. The agreement between the theoretical and
experimental results is remarkable. In order to better understand the origin of the 'hot
spots', we also plot the probabilities of LZ transition as a function of the pulse width
at fixed amplitude ΦLZS=10mΦ0 (Fig.
3c). Notice that both LZ transition probabilities oscillate with T, which
are quite different from the general asymptotic LZ transition probabilities. The
transition probability at M1 is always greater because Δ1 is
much smaller than Δ2. The three oblique dotted lines in Figure
3a represent lines of constant sweeping rate. The 'hot spots' are located on
these lines, where the transition probability of M2 is a minimum.
M2 thereby acts as a total reflection mirror, resulting in the 'hot
spots' in transition probability. This feature further confirms that the avoided crossings
play the role of quantum mechanical wave function splitters, analogous to continuously
tunable beam splitters in optical experiments. The transmission coefficient of the wave
function splitters (the avoided crossings) in our experiment can be varied in situ
from zero (total reflection) to unity (total transmission) or any value in between by
adjusting the duration and amplitude of the single triangular bias waveform used to sweep
through the avoided crossings.
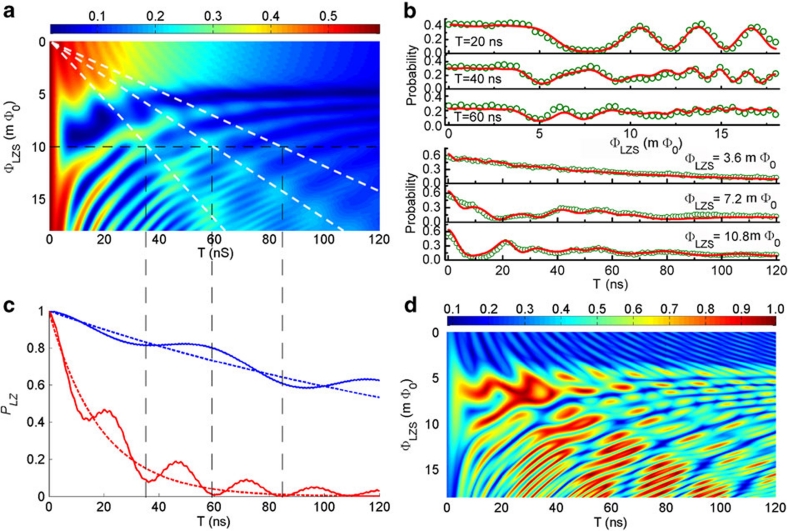
Figure 3. Numerically simulated LZS interference pattern and control of a generalized W state in a phase qubit coupled to two TLSs.
(a) The numerically simulated population of |1 after the triangular flux pulse
is plotted as a function of the width and amplitude of the triangular flux bias. The
horizontal dotted line indicates the location of ΦLZS=10 mΦ0 and
the vertical dotted lines indicate the locations of 'hot spots' at ΦLZS=10
mΦ0. The oblique dotted lines are lines of constant sweeping rate. The
parameters used are determined experimentally: ω01,dc/2π=16.747
GHz,
after the triangular flux pulse
is plotted as a function of the width and amplitude of the triangular flux bias. The
horizontal dotted line indicates the location of ΦLZS=10 mΦ0 and
the vertical dotted lines indicate the locations of 'hot spots' at ΦLZS=10
mΦ0. The oblique dotted lines are lines of constant sweeping rate. The
parameters used are determined experimentally: ω01,dc/2π=16.747
GHz, 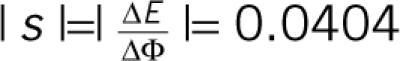 GHz/mΦ0,
ωTLS1/2π=16.590 GHz,
ωTLS2/2π=16.510 GHz, Δ1/2π=10 MHz,
Δ2/2π=32 MHz,
GHz/mΦ0,
ωTLS1/2π=16.590 GHz,
ωTLS2/2π=16.510 GHz, Δ1/2π=10 MHz,
Δ2/2π=32 MHz,  γ(deph)=(45 ns)−1. (b) The upper
panel shows the dependence of population of |1
γ(deph)=(45 ns)−1. (b) The upper
panel shows the dependence of population of |1 on ΦLZS at T=20, 40
and 60 ns, respectively. The lower panel shows the dependence of population of |1
on ΦLZS at T=20, 40
and 60 ns, respectively. The lower panel shows the dependence of population of |1 on
T at ΦLZS=3.6, 7.2 and 10.8 mΦ0, respectively. The
circles represent the experimental data and the lines from the theory. (c) LZ
transition probabilities of M1 (blue line) and M2
(red line) at ΦLZS=10 mΦ0 as a function of pulse width. They are
quite different from the asymptotic LZ transition probabilities (blue dotted line and
red dotted line). (d) The resulting w as a function of T and
ΦLZS.
on
T at ΦLZS=3.6, 7.2 and 10.8 mΦ0, respectively. The
circles represent the experimental data and the lines from the theory. (c) LZ
transition probabilities of M1 (blue line) and M2
(red line) at ΦLZS=10 mΦ0 as a function of pulse width. They are
quite different from the asymptotic LZ transition probabilities (blue dotted line and
red dotted line). (d) The resulting w as a function of T and
ΦLZS.
Precise control of the quantum states in the tripartite system
We emphasize that the method of using LZS interference for the precise quantum state
manipulation described above is performed within the decoherence time of the tripartite
system, which is about 140 ns. Through coherent LZ transition, we can thus achieve a high
degree of control over the quantum state of the qubit–TLS tripartite system. For example,
one may take advantage of LZS to control the generalized W state,
|ψ =α|1g1g2
=α|1g1g2 +β|0e1g2
+β|0e1g2 +γ|0g1e2
+γ|0g1e2 ,
evolving in the sub-space spanned by the three product states during the operation of
sweeping flux bias. In order to quantify the generalized W state, we define
,
evolving in the sub-space spanned by the three product states during the operation of
sweeping flux bias. In order to quantify the generalized W state, we define 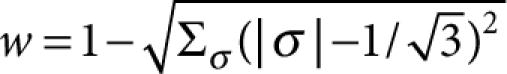 where σ=α, β, γ.
In Figure 3d, w is plotted as a function of T and
ΦLZS. Note that with precise control of the flux bias sweep, the states with
w=1, which are generalized W states with equal probability in each of the
three basis product states, are obtained, demonstrating the effectiveness of this new
method. It should be pointed out that when one of the three qubits is lost, the remaining
two qubits are maximally entangled.
where σ=α, β, γ.
In Figure 3d, w is plotted as a function of T and
ΦLZS. Note that with precise control of the flux bias sweep, the states with
w=1, which are generalized W states with equal probability in each of the
three basis product states, are obtained, demonstrating the effectiveness of this new
method. It should be pointed out that when one of the three qubits is lost, the remaining
two qubits are maximally entangled.
Discussion
Our tripartite system includes a macroscopic object, which is relatively easy to control and read out, coupled to microscopic degrees of freedom that are less prone to environment-induced decoherence and thus can be used as a hybrid qubit. The excellent agreement between our data and theory over the entire T−ΦLZS parameter plane indicates strongly that the states created are consistent with the generalized W states. The coherent generation and manipulation of generalized W states reported here demonstrate an effective new technique for the precise control of multipartite quantum states in solid-state qubits and/or hybrid qubits6,8.
Methods
Experimental detail
Figure 1a shows the principal circuitry of the measurement. The
flux bias and microwave are fed through the on-chip thin film flux lines coupled
inductively to the qubit. The slowly varying flux bias is used to prepare the initial
state of the qubit and to read out the qubit state after coherent state manipulation. In
the first platform of the flux bias, the potential is tilted quite asymmetrically to
ensure that the qubit is initialized in the left well. Then we increase the flux bias to
the second platform until there are only a few energy levels, including the computational
basis states |0 and |1
and |1 in the left well. A microwave π-pulse is applied to rotate
the qubit from |0
in the left well. A microwave π-pulse is applied to rotate
the qubit from |0 to |1
to |1 . This is followed by a triangular waveform with adjustable width
and amplitude applied to the fast flux bias line, which results in LZ transition. A short
readout pulse of flux bias is then used to adiabatically reduce the well's depth so that
the qubit will tunnel to the right well if it was in |1
. This is followed by a triangular waveform with adjustable width
and amplitude applied to the fast flux bias line, which results in LZ transition. A short
readout pulse of flux bias is then used to adiabatically reduce the well's depth so that
the qubit will tunnel to the right well if it was in |1 or remain in the left well if it
was in |0
or remain in the left well if it
was in |0 . The flux bias is then lowered to the third platform, where the double-well
potential is symmetric, to freeze the final state in one of the wells. The state in the
left or right well corresponds to clockwise or counterclockwise current in the loop, which
can be distinguished by the dc-SQUID magnetometer inductively coupled to the qubit. By
mapping the states |0
. The flux bias is then lowered to the third platform, where the double-well
potential is symmetric, to freeze the final state in one of the wells. The state in the
left or right well corresponds to clockwise or counterclockwise current in the loop, which
can be distinguished by the dc-SQUID magnetometer inductively coupled to the qubit. By
mapping the states |0 and |1
and |1 into the left and right wells, respectively, the
probability of finding the qubit in state |1
into the left and right wells, respectively, the
probability of finding the qubit in state |1 is obtained. We obtained
T1
is obtained. We obtained
T1 70 ns from
energy relaxation measurement (Supplementary Fig.
S1a), TR
70 ns from
energy relaxation measurement (Supplementary Fig.
S1a), TR 80 ns from Rabi oscillation (Supplementary
Fig. S1b), T2*
80 ns from Rabi oscillation (Supplementary
Fig. S1b), T2* 60 ns from Ramsey interference fringe (Supplementary Figs S1c and S1d) and T2
60 ns from Ramsey interference fringe (Supplementary Figs S1c and S1d) and T2 137 ns from spin-echo (Supplementary Fig. S1e) in the region free of
qubit–TLS coupling.
137 ns from spin-echo (Supplementary Fig. S1e) in the region free of
qubit–TLS coupling.
Hamiltonian in our tripartite system
For the coupled qubit–TLS system, the Hamiltonian can be written as23,24
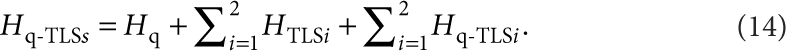 |
14 |
In the two-level approximation the effective Hamiltonian of the qubit is 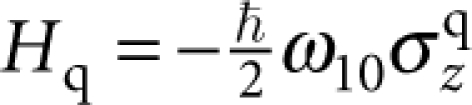 here the flux bias (Φ) dependent
energy-level spacing of the qubit,
ħω10=E1−E0, can be obtained
numerically by solving the eigenvalues problem associated with the full Hamiltonian of the
phase qubit25. The Hamiltonian of the ith TLS can be written as
here the flux bias (Φ) dependent
energy-level spacing of the qubit,
ħω10=E1−E0, can be obtained
numerically by solving the eigenvalues problem associated with the full Hamiltonian of the
phase qubit25. The Hamiltonian of the ith TLS can be written as 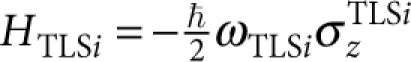 where
ħωTLSi is the energy-level spacing of the ith TLS. The
interaction Hamiltonian between the qubit and the ith TLS is
where
ħωTLSi is the energy-level spacing of the ith TLS. The
interaction Hamiltonian between the qubit and the ith TLS is 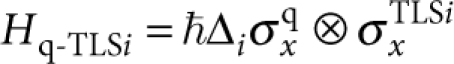 where Δi is the coupling
strength between the qubit and the ith TLS and
where Δi is the coupling
strength between the qubit and the ith TLS and 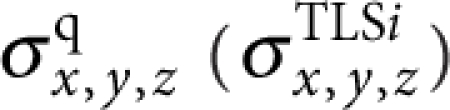 are the Pauli operators acting on the states of the
qubit (the ith TLS). By adjusting the flux bias, the qubit and TLSs can be tuned
into and out of resonance, effectively turning on and off the couplings. Below |0
are the Pauli operators acting on the states of the
qubit (the ith TLS). By adjusting the flux bias, the qubit and TLSs can be tuned
into and out of resonance, effectively turning on and off the couplings. Below |0 and |1
and |1 (|gi
(|gi and |ei
and |ei ) are used to denote the
ground state and excited state of the qubit (the ith TLS). In our experiment the
initial state is prepared in the system's ground state
|0g1g2
) are used to denote the
ground state and excited state of the qubit (the ith TLS). In our experiment the
initial state is prepared in the system's ground state
|0g1g2 . When the couplings between the qubit and
TLSs are off, we use a π-pulse to pump the qubit to |1
. When the couplings between the qubit and
TLSs are off, we use a π-pulse to pump the qubit to |1 (thus the system is in
|1g1g2
(thus the system is in
|1g1g2 ). We then sweep the flux bias through the
avoided crossing(s) to turn on the coupling(s) between the qubit and the TLS(s). Since
after the application of the π-pulse the system has absorbed exactly one microwave
photon and the subsequent steps of state manipulation are accomplished in the absence of
the microwave, conservation of energy guarantees that one and only one of the qubit, TLS1
and TLS2, can be coherently transferred to its excited state. Therefore, states with only
one of the three subsystems in excited state, |1g1g2
). We then sweep the flux bias through the
avoided crossing(s) to turn on the coupling(s) between the qubit and the TLS(s). Since
after the application of the π-pulse the system has absorbed exactly one microwave
photon and the subsequent steps of state manipulation are accomplished in the absence of
the microwave, conservation of energy guarantees that one and only one of the qubit, TLS1
and TLS2, can be coherently transferred to its excited state. Therefore, states with only
one of the three subsystems in excited state, |1g1g2 ,
|0e1g2
,
|0e1g2 , and
|1g1e2
, and
|1g1e2 , are relevant in discussing the subsequent
coherent dynamics of the system. In the subspace spanned by these three basis states, the
Hamiltonian (14) can be written explicitly as Hamiltonian (1) in the main text.
, are relevant in discussing the subsequent
coherent dynamics of the system. In the subspace spanned by these three basis states, the
Hamiltonian (14) can be written explicitly as Hamiltonian (1) in the main text.
Unitary operation in our tripartite system
We use the transfer matrix method16,22 to obtain the probability of
finding the system in |1g1g2 at the end of the
triangular pulse. We use |a
at the end of the
triangular pulse. We use |a =[1,0,0]T,
|b
=[1,0,0]T,
|b =[0,1,0]T and |c
=[0,1,0]T and |c =[0,0,1]T to denote the
instantaneous eigenstates of the time-dependent Hamiltonian (14), as shown in Supplementary Figure S2. It is noted that at the
initial flux bias point, which is far from the avoided crossings, the system is in
|a
=[0,0,1]T to denote the
instantaneous eigenstates of the time-dependent Hamiltonian (14), as shown in Supplementary Figure S2. It is noted that at the
initial flux bias point, which is far from the avoided crossings, the system is in
|a =|1g1g2
=|1g1g2 . At the crossing times
t=t1 and t=t2, the incoming and
outgoing states are connected by the transfer matrix:
. At the crossing times
t=t1 and t=t2, the incoming and
outgoing states are connected by the transfer matrix:
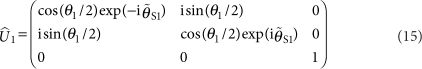 |
15 |
and
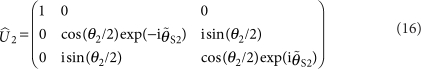 |
16 |
respectively. Here
sin2(θi/2)=PLZi
(i=1, 2) is the LZ transition probability at the ith avoided crossing. 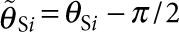 where θSi is
the Stokes phase16,22, the value of which depends on the adiabaticity
parameter ηi=Δi2/υ in the
form of θSi=π/4+ηi (ln
ηi−1)+arg Γ(1−iηi), where
Γ is the Gamma function. In the adiabatic limit θS→0, and in
the sudden limit θS=π/4. At crossing times
t=t3 and t=t4, we have
where θSi is
the Stokes phase16,22, the value of which depends on the adiabaticity
parameter ηi=Δi2/υ in the
form of θSi=π/4+ηi (ln
ηi−1)+arg Γ(1−iηi), where
Γ is the Gamma function. In the adiabatic limit θS→0, and in
the sudden limit θS=π/4. At crossing times
t=t3 and t=t4, we have 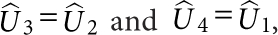 respectively. The outgoing state
at t=ti and the incoming state at
t=ti+1 (i=0, 1, 2, 3, 4) are thus connected by
the propagator
respectively. The outgoing state
at t=ti and the incoming state at
t=ti+1 (i=0, 1, 2, 3, 4) are thus connected by
the propagator
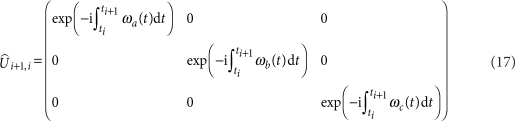 |
17 |
where ωi(t) is the energy-level spacing frequency of
|i (i=a, b, c) at time t. The net effect of a
triangular pulse is to cause the state vector to evolve according to the unitary
transformation
(i=a, b, c) at time t. The net effect of a
triangular pulse is to cause the state vector to evolve according to the unitary
transformation
| 18 |
The probability of finding the system remaining at the initial state is
P1=| 1g1g2|Û|1g1g2
1g1g2|Û|1g1g2 |2.
Its concrete form is equation (3), in which
|2.
Its concrete form is equation (3), in which  and
and 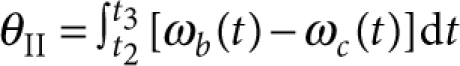 are
the relative phases accumulated in regions I and II, respectively, as shown in Supplementary Fig. S2. The LZS in our experiment
can be viewed as interferences among the three paths, which are labelled 1, 2 and 3,
starting from the same initial state:
are
the relative phases accumulated in regions I and II, respectively, as shown in Supplementary Fig. S2. The LZS in our experiment
can be viewed as interferences among the three paths, which are labelled 1, 2 and 3,
starting from the same initial state:
path 1: 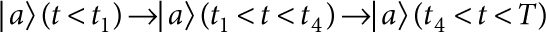
path 2: 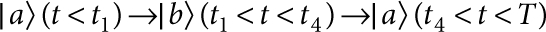
path 3: 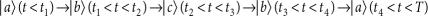
Denoting ωi(t) as the energy-level spacing frequency
corresponding to path i (i=1, 2, 3), then θI and
θII have the forms 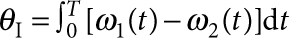 and
and 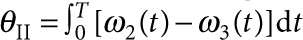 respectively.
respectively.
Numerical simulation of LZS interference in the bipartite qubit–TLS system
For the bipartite qubit–TLS system discussed here, the qubit is coupled only to a single TLS. The quantum dynamics of the system, including the effects of dissipation, is described by the Bloch equation of the time evolution of the density operator:
 |
19 |
where
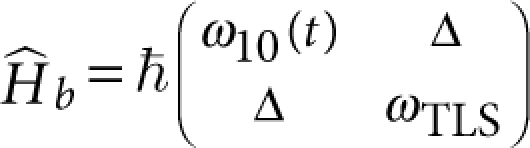 |
where ω10(t)=ω10,dc−νt,
ν≡2sΦLZS/T is the energy sweeping rate and Δ is the
qubit–TLS coupling strength. The second term, Γ[ρ], describes the relaxation
process to the ground state |0g and dephasing process phenomenologically. In a
concrete expression, equation (19) can be written as (for ease of discussion, we relabel
|1g
and dephasing process phenomenologically. In a
concrete expression, equation (19) can be written as (for ease of discussion, we relabel
|1g and |0e
and |0e as |a
as |a and |b
and |b , respectively)
, respectively)
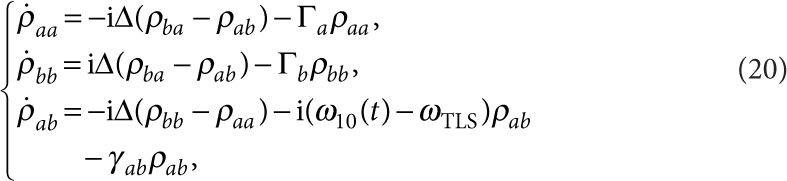 |
20 |
with ρba=ρab*. Here
Γα (α=a, b) is the relaxation rate from state
|α to the ground state |0g
to the ground state |0g . The decoherence rate
. The decoherence rate 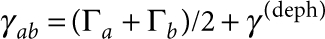 includes contributions from both relaxation
and dephasing. Supplementary Figures S3a and
S3b give the numerically simulated LZS interference pattern for the qubit coupled
with the first TLS and second TLS, respectively. To calculate the transmission coefficient
of Mi (i=1, 2), that is, the LZ tunneling probability
PLZ, as shown in Figure 3c, we cannot directly
use the asymptotic LZ formula, which is based on sweeping the system across the avoided
crossing from negative to positive infinities. In contrast, in our experiment the LZS
occurs near the avoided crossings. Therefore, our numerical results are obtained by
solving the Bloch equations directly.
includes contributions from both relaxation
and dephasing. Supplementary Figures S3a and
S3b give the numerically simulated LZS interference pattern for the qubit coupled
with the first TLS and second TLS, respectively. To calculate the transmission coefficient
of Mi (i=1, 2), that is, the LZ tunneling probability
PLZ, as shown in Figure 3c, we cannot directly
use the asymptotic LZ formula, which is based on sweeping the system across the avoided
crossing from negative to positive infinities. In contrast, in our experiment the LZS
occurs near the avoided crossings. Therefore, our numerical results are obtained by
solving the Bloch equations directly.
Numerical simulation of LZS interference in the tripartite qubit–TLS system
For the tripartite qubit–TLS system discussed below, the qubit is coupled resonantly to
two TLSs (TLS1 and TLS2) with different excited state energies ħωTLS1
and ħωTLS2. The Hamiltonian in the basis of
|1g1g2 ,
|0e1g2
,
|0e1g2 , |0g1e2
, |0g1e2 is Hamiltonian (1) in the main text. The Bloch equations that govern the evolution of the
density operator can be written as (for simplicity, we relabel
is Hamiltonian (1) in the main text. The Bloch equations that govern the evolution of the
density operator can be written as (for simplicity, we relabel 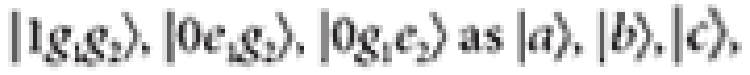 respectively)
respectively)
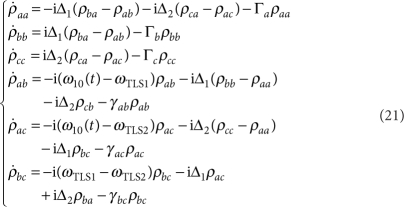 |
21 |
where the diagonal elements ρii are the populations,
off-diagonal elements ρij(i ≠ j) describe
coherence, and 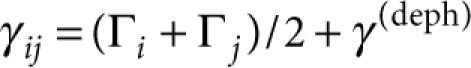 are the rates of
decoherence. The remaining three elements' equations are determined by
ρij*=ρji. The numerically simulated
LZS interference pattern is shown in Figure 3a, which agrees with
the experimental results excellently.
are the rates of
decoherence. The remaining three elements' equations are determined by
ρij*=ρji. The numerically simulated
LZS interference pattern is shown in Figure 3a, which agrees with
the experimental results excellently.
Author contributions
G.S. and S.H. conceived the experiments; G.S. carried out the measurements with the help of B.M. and analysed the data with the help of X.W., Y.Y., J.C., P.W. and S.H.; X.W. performed the numerical calculations; G.S., Y.Y. and S.H. wrote the paper.
Additional information
How to cite this article: Sun, G. et al. Tunable quantum beam splitters for coherent manipulation of a solid-state tripartite qubit system. Nat. Commun. 1:51 doi: 10.1038/ncomms1050 (2010).
Supplementary Material
Supplementary Figures S1-S3
Acknowledgments
This work is partially supported by NCET, NSFC (10704034,10725415), 973 Program (2006CB601006), the State Key Program for Basic Research of China (2006CB921801) and NSF Grant No. DMR-0325551. We thank Northrop Grumman ES in Baltimore, MD, for technical and foundry support and thank R. Lewis, A. Pesetski, E. Folk and J. Talvacchio for technical assistance. We thank B. Ruzicka for editing the paper.
References
- Makhlin Y., Schön G. & Shnirman A. Quantum-state engineering with Josephson-junction devices. Rev. Mod. Phys. 73, 357–400 (2001). [Google Scholar]
- You J. & Nori F. Superconducting circuits and quantum informations. Phys. Today 58, 42–47 (2005). [Google Scholar]
- Clarke J. & Wilhelm F. K. Superconducting quantum bits. Nature 453, 1031–1042 (2008). [DOI] [PubMed] [Google Scholar]
- Martinis J. M. Superconducting phase qubits. Quantum Inf. Process. 8, 81–103 (2009). [Google Scholar]
- Simmonds R. W. et al. Coherent interactions between phase qubits, cavities, and TLS defects. Quantum Inf. Process. 8, 117–131 (2009). [Google Scholar]
- Zagoskin A. M., Ashhab S., Johansson J. R. & Nori F. Quantum two-level systems in Josephson junctions as naturally formed qubits. Phys. Rev. Lett. 97, 077001 (2006). [DOI] [PubMed] [Google Scholar]
- Wei L. F., Johansson J. R., Cen L. X., Ashhab S. & Nori F. Controllable coherent population transfers in superconducting qubits for quantum computing. Phys. Rev. Lett. 100, 113601 (2008). [DOI] [PubMed] [Google Scholar]
- Neeley M. et al. Process tomography of quantum memory in a Josephson-phase qubit coupled to a two-level state. Nature Phys. 4, 523–526 (2009). [Google Scholar]
- Xu H. et al. Spectroscopy of three-particle entanglement in a macroscopic superconducting circuit. Phys. Rev. Lett. 94, 027003 (2005). [DOI] [PubMed] [Google Scholar]
- Dür W., Vidal G. & Cirac J. I Three qubits can be entangled in two inequivalent ways. Phys. Rev. A 62, 062314 (2000). [Google Scholar]
- Roos C. F. et al. Control and measurement of three-qubit entangled states. Science 304, 1478–1480 (2004). [DOI] [PubMed] [Google Scholar]
- Häffner H. et al. Scalable multiparticle entanglement of trapped ions. Nature 438, 643–646 (2005). [DOI] [PubMed] [Google Scholar]
- Bennett C. H. & DiVincenzo D. P. Quantum information and computation. Nature 404, 247–255 (2000). [DOI] [PubMed] [Google Scholar]
- Petta J. R., Lu H. & Gossard A. C. A coherent beam splitter for electronic spin states. Science 327, 669–672 (2010). [DOI] [PubMed] [Google Scholar]
- Oliver W. D. et al. Mach-Zehnder interferometry in a strongly driven superconducting qubit. Science 310, 1653–1657 (2005). [DOI] [PubMed] [Google Scholar]
- Sillanpää M., Lehtinen T., Paila A., Makhlin Y. & Hakonen P. Continuous-time monitoring of Landau-Zener interference in a cooper-pair box. Phys. Rev. Lett. 96, 187002 (2006). [DOI] [PubMed] [Google Scholar]
- Wilson C. M. et al. Coherence times of dressed states of a super conducting qubit under extreme driving. Phys. Rev. Lett. 98, 257003 (2007). [DOI] [PubMed] [Google Scholar]
- Izmalkov A. et al. Consistency of ground state and spectroscopic measurements on flux qubits. Phys. Rev. Lett. 101, 017003 (2008). [DOI] [PubMed] [Google Scholar]
- Rudner M. S. et al. Quantum phase tomography of a strongly driven qubit. Phys. Rev. Lett. 101, 190502 (2008). [DOI] [PubMed] [Google Scholar]
- Sun G. et al. Population inversion induced by Landau–Zener transition in a strongly driven rf superconducting quantum interference device. Appl. Phys. Lett. 94, 102502 (2009). [Google Scholar]
- LaHaye M. D., Suh J., Echternach P. M., Schwab K. C. & Roukes M. L. Nanomechanical measurements of a superconducting qubit. Nature 459, 960–964 (2009). [DOI] [PubMed] [Google Scholar]
- Shevchenko S., Ashhab S. & Nori F. Landau-Zener-Stückelberg interferometry. Phys. Rep. 492, 1–30 (2010). [Google Scholar]
- Ku L. -C. & Yu C. C. Decoherence of a Josephson qubit due to coupling to two-level systems. Phys. Rev. B 72, 024526 (2005). [Google Scholar]
- Lupascu A., Bertet P., Driessen E. F. C., Harmans C. J. P. M. & Mooij J. E. One- and two-photon spectroscopy of a flux qubit coupled to a microscopic defect. Phys. Rev. B 80, 172506 (2009). [Google Scholar]
- Han S., Rouse R. & Lukens J. E. Generation of a population inversion between quantum states of a macroscopic variable. Phys. Rev. Lett. 76, 3404–3407 (1996). [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Supplementary Figures S1-S3