Shear rheology of lipid monolayers and insights on membrane fluidity

Abstract

The concept of membrane fluidity usually refers to a high molecular mobility inside the lipid bilayer which enables lateral diffusion of embedded proteins. Fluids have the ability to flow under an applied shear stress whereas solids resist shear deformations. Biological membranes require both properties for their function: high lateral fluidity and structural rigidity. Consequently, an adequate account must include, in addition to viscosity, the possibility for a nonzero shear modulus. This knowledge is still lacking as measurements of membrane shear properties have remained incomplete so far. In the present contribution we report a surface shear rheology study of different lipid monolayers that model distinct biologically relevant situations. The results evidence a large variety of mechanical behavior under lateral shear flow.

One fundamental concept of cell biology is the recognition that the cell membrane is a fluid assembly of amphiphilic molecules into a two-dimensional liquid crystalline structure (1). This structure is rigid enough to form a stable container but is fluid enough to allow lateral transport of the different membrane components and a differential permeability essential for cell homeostasis (2, 3). The membrane fluidity entails lipids and membrane proteins mobility, which is essential for their mutual interplay and function (2, 4, 5). Indeed, adequate protein function requires an energetically efficient conformational dynamics, which is only possible in a mechanically adapted membrane medium (6–8). Lipid bilayers are generally assumed to behave as simple Newtonian fluids, however they possess a bending and a compression elasticity (6, 9), thus the question of the existence of a shear elasticity comes to mind. Very recently, Harland et al. showed that bilayers of single phospholipids are not purely viscous, but viscoelastic, with an elastic modulus that diverges at the fluid-gel transition (10). Although the fluid mosaic picture allows for a satisfactory description of the molecular dynamics of the embedded objects (8, 11, 12), lacking of viscoelastic contributions could result in an incomplete understanding of membrane processes. A rigorous picture of the mechanical behavior of a membrane includes the resistance to shear, compression, and bending. Furthermore, the concept of membrane fluidity, when referred to an ability of the membrane to flow under an applied shear stress, should be distinguished from the concept of molecular mobility inside the membrane (13), or of the local microviscosity measured from changes in the mobility of a molecular fluorescent probe (14). In this sense, the high diffusion mobility typical of disordered lipid phases might be consistent with a finite macroscopic shear viscosity.

The present work addresses the fundamental question of membrane fluidity with a systematic study of the surface shear rheology of model Langmuir monolayers. Marsh demonstrated that the properties of monolayers and bilayers are equivalent when the monolayer surface pressure equals the hydrophobic free energy of the bilayer per unit area (π ≈ 30–35 mN/m) (15). However, the work of Marsh focused on single component bilayers. For two components or more, interactions across the leaflets can generate correlations that lead to demixing in bilayers whereas monolayers remain monophasic, as shown very recently in the case of ternary lipid mixtures by Ziblat et al. (16). The structure of the bilayers can then be appreciably different from that of the corresponding monolayers, especially at large cholesterol content, thus with a possible influence on their dynamics. In our study, we focused on single phase monolayers, containing amounts of cholesterol smaller than the one leading to phase separation in bilayers (see ref. 17 for a study), to minimize possible differences with the properties of bilayers.

Membrane flow behavior is characterized by an intrinsic surface viscosity (η) relevant for in-plane motion and an intermonolayer friction opposing velocity gradients across the layer (18). For thermal motions, intermonolayer slippage hardly affects η, which is primarily determined by the lateral packing inside the monolayers (18). Thus, interdigitation and intermonolayer coupling effects might consequently impact less surface viscosity than phase behavior, provided such a coupling does not lead to phase separation and situations such as formation of cholesterol crystals reported in ref. 16.

Model Monolayers and Surface Shear Rheology

Here, we focus on the shear viscoelasticity of homogeneous lipid phases spanning a large range of membrane fluidity relevant to different biological states (Table 1). Oscillatory rheology experiments were performed at a surface pressure of 30 mN/m and at 37 °C, which is the representative state of lipid packing in physiological conditions (6, 15).

Table 1.

Lipid systems studied in this work with specification of their melting temperature, T_m, and the physical state of the bulk lamellar phase at 37 °C.

System	Tm (°C)	Lyotropic phase	Thermal criterion*
DPPC	41	gel	solid
POPC	3	ld	fluid
POPC/chol (70∶30)	≈3	lo	fluid
E. coli polar lipid extract	2–4	ld	fluid
Egg sphingomyelin (eggSM)	39	gel	solid
eggSM/chol (70∶30)	≈39	lo	fluid
eggSM/POPC/chol (1∶1∶1)	≈25	lo	fluid
Egg ceramide (eggCer)	90	solid	solid

^*The thermal criterion for fluidity is linked to T_m (T > T_m, fluid; T < T_m, solid).

There are two main families of membrane lipids, namely glycerophospholipids and sphingolipids (3, 19). Although a variety of acyl chains are present in cells, lipids of the first class are found predominantly monounsaturated, the palmitoyl-oleyl-sn-glycero-phosphatidylcholine (POPC) being a main component of the fluid matrix of most eukaryote membranes. The fully saturated component, dipalmitoyl-sn-glycero-phosphatidylcholine (DPPC) melts well above room temperature. Sphingomyelin (SM) is composed by fully saturated sphingosine mainly localized in cholesterol-rich domains. These lipids (POPC and SM) have been mutually mixed with cholesterol (Chol) at near physiological proportions (about 30% mol) (2, 3). The different mixtures were chosen to represent different states of plasma membranes in prokaryote cells. The monolayers of a native lipid extract of the inner membrane of Escherichia coli have been studied to model prokaryote membranes. Ceramide (Cer), an essential messenger in the apoptosis pathway, has also been studied.

Linear Viscoelasticity and Flow Dynamics

Disordered Liquid Phases: High Fluidity.

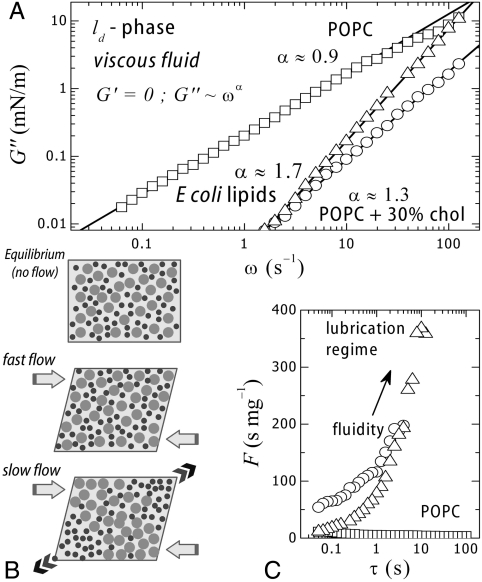
Unsaturated glycerolipids are known to assemble into bilayers in water, forming liquid crystalline phases (l_d). The acyl chains show in-plane nematic order but lack short-range translational and orientational order, consequently, they do not resist shear. We will first consider the case of the monounsaturated phosphocholine (POPC), a typical fluid glycerolipid well above the melting temperature (T_m ≈ 3 °C) (Table 1). Fig. 1A shows data for POPC monolayers sheared at small amplitude (1%) well within the linear regime (SI Text). The POPC monolayers behave as a fluid, with no storage modulus (G^′ ≈ 0) and relatively high loss modulus (G^′′≫0): G^′′ ∼ ω^1.0±0.1, where ω is the frequency of the deformation. This case corresponds to a constant shear viscosity, nearly independent of the frequency (η = G^′′/ω ≈ constant). Further, the stress–strain curve displays linear behavior up to large deformations (SI Text). POPC monolayers are therefore two-dimensional pure Newtonian fluids (undergoing flow with a constant viscosity independent of the shear rate: η ∼ ω^α-1 with α = 1, thus G^′′ = ωη ≈ ω¹). Fig. 1A also shows data for other monolayers in a fluid state (G^′ = 0): (A) POPC mixed with cholesterol (30% mol), the eukaryote regulator of membrane fluidity (20, 21), and (B) a native extract from the inner membrane of E. coli. Interestingly, these two multicomponent systems exhibit significant lower loss moduli than POPC alone, assigning an active role to lipid complexity as a regulator of membrane fluidity (significantly enhanced in the biomimetic mixtures). Unlike POPC monolayers (G^′′ ∼ ω^1.0), the multicomponent layers show a nontrivial frequency dependence of the loss moduli (G^′′ ∼ ω^α, α = 1.3 ± 0.1 for POPC + 30% cholesterol and α = 1.7 ± 0.2 for E. coli lipids) (Fig. 1A). Discovering the origin of this rheological complexity is a question that deserves further attention (SI Text). Shear thickening fluids are characterized by a viscosity coefficient that increases with the rate of shear (η ∼ ω^α-1, thus G^′′ = ηω ∼ ω^α with α > 1). This shear thickening effect is encountered in concentrated dispersions of particles and assigned to reorganization under flow (22). At low frequencies, the liquid filler acts as a lubricant and the fluid flows easily. At higher frequencies, this liquid is unable to refill the gaps created by flow, thus friction increases causing an effective increase of the viscosity. Similar mechanisms could operate in the multicomponent monolayers.

Fig. 1.

(A) Frictional losses of different fluid monolayers (G^′ = 0) upon oscillatory shear flow performed at increasing frequency in the linear regime (1% strain) (□, POPC; ○, POPC + 30% cholesterol; △, E. coli lipids). (B) Lubrication flow of a composite medium made of small objects in a less mobile matrix (see main text). (C) Experimental fluidity coefficient (F = η^-1 = ω/G^′′) measured in a shear flow at different characteristic times (τ = 2π/ω).

Especially interesting is the time dependence of the fluidity coefficient F (Fig. 1C), defined as the inverse shear viscosity (F = η^-1 = ω/G^′′), with time defined as the period of the oscillatory shear deformation (τ = 2π/ω). Fig. 1C shows that F is constant and low (F ≤ 10 s/mg) in POPC layers. An increase of fluidity is observed for the multicomponent systems. For POPC monolayers with 30% of added cholesterol (mimic of the fluid matrix of the eukaryote plasma membrane), the instantaneous fluidity F₀ (at τ → 0) is several times higher than for single POPC layers. Furthermore, a drastic increase in fluidity is observed with slower flow rates, the system reaching a lubrication regime at longer flow rates (F ∼ τ¹), suggesting that cholesterol molecules act as a lubricant able to reduce friction under slow shear flow. A similar fluidity enhancement is observed for E. coli lipids which are as fluid as POPC at short times but undergo a lubrication transition at long times, similar to the effect of cholesterol in POPC.

As for other transport phenomena, membrane fluidity stems from the velocity correlations inside the membrane (Green–Kubo causality relationships) (23). Consequently, higher complexity (compositional and distributional) should result in a higher correlation of the motion in the membrane, to account for a higher fluidity. It is broadly assumed that lipid mixing leads to optimal chain packing (24–26), thus to an evolutionary optimized functional structure and phase behavior of natural membranes (27). The present data suggests that lipid complexity also provides optimized dynamic behavior.

Ordered Liquid Phases (Lipid Rafts).

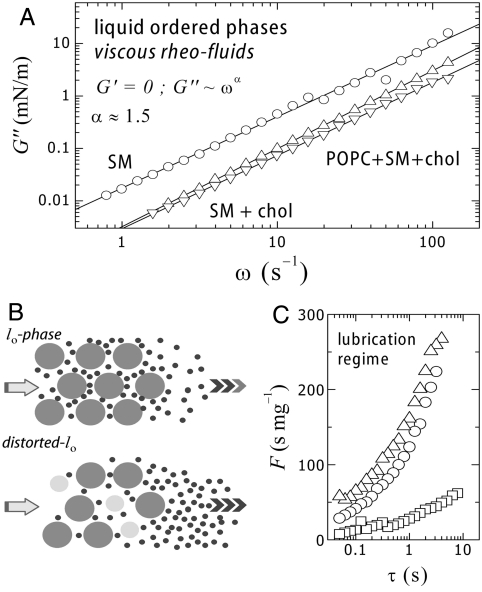
Certain tissues are enriched in SM (brain, egg yellow, etc.), which is also a major lipid component of the plasma membrane of eukaryote cells. These SMs, predominantly saturated, are found highly packed together with proteins and a high amount of cholesterol in membrane domains usually referred to as lipid rafts (28). Natural and synthetic SMs and their mixtures with cholesterol are known to exist in an ordered liquid state (l_o), in monolayers (29), and in bilayers (30, 31). It has been suggested to constitute the native structural state of lipid rafts (32), where proteins found a functional medium fluid enough to allow for conformational changes but structurally compact enough to provide adequate mechanical support. The l_o-phase lacks short-range positional order, which provides liquid character, but is characterized by a large extent of orientational order (3, 19). Here, we consider egg sphingomyelin (eggSM), a predominantly saturated natural sphingolipid with a relatively high melting temperature (T_m ≈ 39 °C). Despite the ordered character of these dense assemblies (19, 33), they appear fluid-like under shear with a vanishing shear rigidity (G^′ ≈ 0).

Fig. 2 shows experimental data for the shear loss modulus of different homogenous monolayers based on eggSM at the physiological packing state (π = 30 mN/m, T = 37 °C < T_m ≈ 39 °C). Values for the single lipid monolayer (eggSM) correspond to a moderate fluidity (G^′′ ≈ 10 mN/m at 10 Hz), similar to those exhibited by the unsaturated phospholipid POPC in the high frequency limit. Unlike POPC (a Newtonian fluid, G^′′ ∼ ω¹), eggSM exhibits shear thickening (G^′′ ∼ ω^1.5). Similar to multicomponent l_d layers, the exponent α (≈1.5), implies a lubrication regime at long times (Fig. 2B). Egg sphingomyelin is a natural lipid extract predominantly saturated (eggSM, 90% hexadecanoyl C16∶0 and 10% of other chain lengths). Consequently, eggSM layers intrinsically possess a certain degree of compositional and structural disorder, unlike high purity synthetic homologues (27). This heterogeneity explains why eggSM exhibits fluid-like features at experimental temperatures below T_m, but also justifies friction regulation by the small length components with a higher mobility. As expected, further mixing of eggSM with eukaryote fluidity regulators (such as Chol and POPC), causes an additional decrease of the shear losses (Fig. 2A). Added Chol (30% mol) causes G^′′ to decrease by more than a factor of three. An additional decrease is observed upon POPC addition (eggSM∶chol∶POPC/1∶1∶1, monophasic at π > 20 mN/m). These results point out that compositional complexity favors high fluidity. The observed rheology is compatible with the hypothesis that cholesterol-rich membrane rafts based on sphingolipids arrange in an ordered but fluid phase, the l_o-phase (33, 28, 32). The basic idea is that SM provides larger free area than phosphatidylcholine (PC) lipids, so promoting fluidity and probably important functional consequences in accommodating conformational changes involved in protein function. Cholesterol, like in the previous case, might work as space filler and fluidity regulator in the l_o-phase.

Fig. 2.

(A) Frictional shear losses of fluid monolayers (G^′ = 0) at the liquid ordered state [○, eggSM; △, eggSM + 30% cholesterol; ▽, eggSM + cholesterol + POPC (1∶1∶1)]. (B, Top) Lubrication flow in an ordered matrix, (Bottom) lubrication flow favored by distortions caused by an additive (see main text). (C) Experimental fluidity coefficient measured in a shear flow at different characteristic times.

The Gel Phase (Saturated PCs).

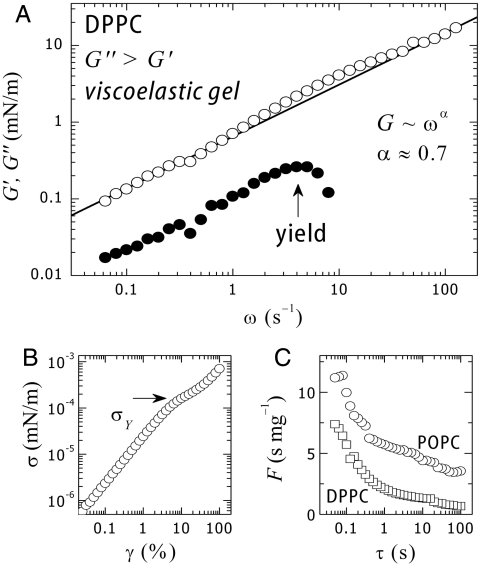
Below T_m, all phospholipids undergo a freezing transition to a solid state usually referred to as the “gel” phase. In this ordered phase (with short-range, positional, and orientational order), lateral diffusion is significantly constrained, thus one expects a much lower mobility and a smaller fluidity, similar to bulk gels. However, an adequate definition of a gel implies high viscosity and finite shear rigidity. To our better knowledge, nothing was known until recently (10) about the precise values of the shear modulus of PC layers. To investigate viscoelasticity in gel phases, we used DPPC, a saturated phosphocholine. Fig. 3 shows the frequency dependence of the shear parameters measured for DPPC monolayers in physiological conditions (T ≈ 37 °C < T_m, pH 7, π = 30 mN/m).

Fig. 3.

(A) Shear modulus (○, G^′) and frictional shear losses (○, G^′′) of monolayers of DPPC at the gel state. (B) Stress–strain plot at 1 Hz. A yield stress defines a plastic plateau at σ_Y. (C) Fluidity as a function of the characteristic flow time (τ = 2π/ω). DPPC is significantly pasty compared to its unsaturated fluid homologue, POPC.

The observed behavior resembles that of a viscoelastic gel with finite shear rigidity and high viscosity (G^′′ ≈ 5G^′). In the case of a single relaxation process, viscoelasticity is described by the Maxwell model (at low frequencies G^′ ∼ ω², G^′′ ∼ ω). However, those frequency dependences are not found in the present case. DPPC monolayers exhibit a weaker time dependence (G^′, G^′′ ∼ ω^0.7), which suggests a glassy-like dynamic typical for soft solids (34). Data in Fig. 3A shows that the system undergoes a softening transition above a critical yield stress (σ_Y = (G^′γ)_Y ≈ 0.2 mN/m), followed by plastic behavior. The plastic yield is associated with an extra energy dissipation shown as a bump in the loss modulus (Fig. 3A). The yield point is clearly visible in the stress–strain plot (Fig. 3B), which displays a plastic plateau at σ_Y ≈ 0.2 mN/m. The yield point defines an upper limit for preserving structural rigidity. Above σ_Y structural softening occurs, then the gel undergoes flow (Fig. 3 A and B). To our knowledge, the present results constitute unique proof for a genuine gel-like mechanical behavior in a model membrane in the gel state.

Solid Phases (Ceramide).

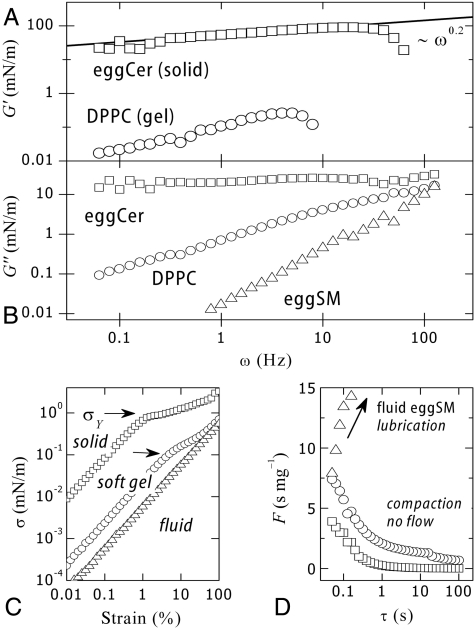
Compared to other lipids, ceramide melts at very high temperature (T_m ≈ 90 °C) and forms anisotropic domains with flat-shaped edges characteristic of solid crystalline phases (35). Fig. 4 shows results from shear rheology experiments performed on monolayers of egg ceramide (eggCer) in the solid state (eggCer, π = 30 mN/m and T = 37 °C ≪ T_m ≈ 90 °C). Nonlinear effects emerge at very small deformation (yielding is already found at 1% strain, Fig. 4C), thus experiments were performed in this case at γ = 0.5%. The shear modulus is high (G^′ ≈ 30–100 mN/m), and typical of 2D solids (36). This solid is characterized by weak frequency dependences (G^′ ∼ ω^0.2) and high yield stress [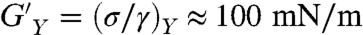], much larger than observed for DPPC (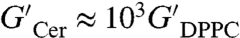, Fig. 4A). As expected for solids, viscous losses are lower than elasticity (G^′ > G^′′) although high (G^′′ ≈ 25 mN/m) and essentially constant (G^′′ ∼ ω⁰). Similar to the gel-phase (DPPC), the solid monolayer (eggCer) yields above a critical stress (σ_Y ≈ 1 mN/m), giving rise to a plastic plateau (Fig. 4C). A comparison between the fluidity of different monolayers is shown in Fig. 4D. Note that in principle there should be no long-range translational order in 2-D because of thermal fluctuations. Theory (37) showed, however, that lacking long-range positional order does not preclude orientational order, thus lipids can freeze in 2-D either as a hexatic phase with short-range positional order or a true solid with quasi long-range translational order allowing for the formation of 2-D microcrystals (38).

Fig. 4.

Comparative plot of (○) eggSM, (○) DPPC, and (○) eggCer. (A) Shear modulus (G^′) and (B) loss modulus (G^′′). (C) Stress–strain plots at 1 Hz. Arrows mark yield stress. (D) Fluidity as a function of flow time. Ceramide is a compact paste as compared to gel DPPC.

For years, a mere structural function was assumed for ceramides and membrane sphingolipids in general, although different functional roles have been recognized later (35, 39). Perhaps one of the most fascinating aspects of ceramides is their capacity to work as a signaling molecule, specifically, of the programmed cell death. The hydrolysis of membrane sphingosynes into ceramides is catalyzed by the enzyme sphingomyelinase, which starts a cascade of cellular events guiding apoptosis (39). In view of the present data, membrane metabolism in a fluid sphingomyelin environment might become strongly repressed after hydrolysis to ceramide. As membrane homeostasis is based in a high fluidity, sphingosyne conversion to solid ceramide can be plausibly viewed as a breakdown of the membrane metabolism, thus as a possible ceramide-mediated physical pathway for the apoptosis mechanism (35).

Shear Flow and Membrane Fluidity

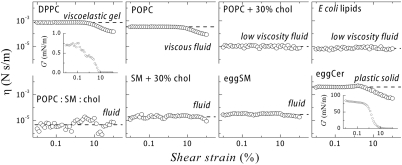
Fluidity is a material property that characterizes the response of a material under shear flow. Translation of an object (e.g., a protein) embedded in a membrane is only possible if the membrane is fluid (G^′ = 0). In the case of Brownian diffusion in a fluid membrane the shear deformation involved in thermal motion is small compared to the size of the object (the shear strain is γ ≈ l/R, where l is the typical displacement and R the object size). In this case, the relevant friction is properly accounted for the linear value of G^′′ (with a Stokes friction factor ξ ≈ η₀R defined by the Newtonian shear viscosity; i.e., η = G^′′/ω, measured at γ → 0). However, larger displacements could eventually involve nonlinear effects, so non-Newtonian values of the shear viscosity could be relevant. Consequently, only a study of the dependence of the shear viscosity on the amplitude of the deformation could allow a valid analysis of the effective fluidity “felt” upon large strength membrane motions. With this purpose, we have performed rheology experiments at a constant frequency (1 Hz) and at variable amplitude in a broad range of shear strains (0.01 ≤ γ ≤ 100). Fig. 5 shows the amplitude dependence of the shear viscosity measured for the different lipid systems.

Fig. 5.

Surface shear viscosity of the different lipid monolayers studied in this work. A low shear viscosity (η ≤ 10^-5 N s/m) defines the system as a highly fluid membrane (glycerolipids and sphingolipids mixed with cholesterol and E. coli lipids). Pasty fluids (POPC), viscoelastic gels (DPPC), or solids (eggCer) are characterized by much higher (non-Newtonian) viscosities.

Single Glycerolipids.

Monolayers of single phosphocholines (DPPC and POPC) under shear exhibit a Newtonian linear regime up to a 10% strain. Newtonian viscosities are found higher for the gel phase (for DPPC, η₀ = 9 × 10^-4 N s/m) than for the fluid phase (for POPC, η₀ = 3 × 10^-4 N s/m). Surface viscosity is primarily determined by correlations in the normal pressure profile (25), consequently larger values are expected at increasing molecular packing, as experimentally observed. Above a 10% strain, nonlinear effects emerge (shear thinning). In this regime, the viscosity decreases by a factor of 10 at the highest deformation (γ ≈ 100%), implying an increase of fluidity for large scale displacements.

Mixed Glycerolipids.

Binary mixtures of unsaturated phosphocholines with cholesterol (POPC + cholesterol) or complex native mixtures (E. coli lipids) exhibit an enhanced fluidity characterized by a very low value of the shear viscosity (Fig. 5). These systems, representative of biological fluid membranes, are highly fluid, with an optimized value of the shear viscosity (η₀ ≈ 10^-5 N s/m). Unlike single glycerolipids, nonlinear effects are now strongly inhibited, which enables Newtonian linear flow even at very large deformations (SI Text).

Sphingolipids.

Egg sphingomyelin, a prototype of natural sphingolipid, exhibits a flow behavior typical of a low viscosity fluid with a Newtonian regime extending up to large deformations (η₀ ≈ 3 × 10^-5 N s/m). Similar to glycerolipids, association with cholesterol and unsaturated components results in a fluidity enhancement, with a very low and constant viscosity coefficient similar to that of cholesterol-regulated fluid phases (η ≈ 10^-5 N s/m).

Ceramide.

This highly hydrophobic lipid self-assembles in a very dense solid state (G^′ > 0). Flow is highly restricted by structural stiffness and high friction, resulting in a behavior typical of plastic solids (34). In the linear regime (γ < 0.9%) the system behaves as hard solid (G^′ ≈ 85 mN/m) with a practically null fluidity (the shear viscosity takes values as high as η₀ ≈ 4 × 10^-3 N s/m, three orders of magnitude higher than typical fluid phases). Above a 1% strain, nonlinear effects emerge with viscous shear thinning followed by a sharp structural softening characterized by a drop of the storage modulus (from the linear value, 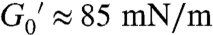, typical of a hard solid down to a vanishing value, G^′ ≈ 0 at γ≥10%, typical of a viscous fluid). Consequently, in an enriched-ceramide membrane, Brownian motion should be largely impeded.

Molecular Foundations.

In molecular terms, the shear viscosity is expected to increase with the strength of the net attractive potential (mainly dependent on tail length) and to decrease with increasing molecular area (18). Data show this general trend: The condensed phospholipid DPPC is more viscous than the unsaturated homologue POPC, whereas charged E. coli lipids (dominated by the electrostatic repulsions) represent the highest fluidity case. Sphingosine is larger than the glycerol head thus sphingolipids might, in general, exhibit smaller viscosities than glycerolipids, as experimentally observed. Ceramide polar heads are less bulky than those of sphingomyelin, because they do not have phosphate groups, thus enabling much higher lateral cohesion and closer packing. Ceramide lipids can therefore pack into a solid structure characterized by high shear stiffness and extremely high viscosity. In general, lipid mixing increases fluidity, even concomitantly with significant structural condensation (e.g., induced by cholesterol) (19–21). Molecular disorder and lubrication effects induced by mixing are thus believed to underlie the functional dichotomy—compact, but fluid—of optimized biological membranes.

Biological Implications.

This elastic dichotomy may have profound consequences in protein function: Protein lateral translation in a fluid membrane is not impeded although it takes place at constant area, but proteins undergoing conformational transitions should exert net forces on the lipid surrounding, which reacts with an equivalent compression force. Systematic studies of surface rheology might contribute to a better understanding of this mechanical interplay existing in biological membranes, although it is secularly ignored in protein energetics.

Furthermore, biological membranes are heterogeneous complex media where the different lipids are locally organized depending on the precise membrane function (2–4). Indeed, organisms invest substantial resources in synthesizing tens of different lipids and selectively arranging them to produce the different membrane structures forming the cell (3). Contrary to a randomized lipid distribution driven by simple entropic spontaneity, specific interactions are assumed to construct a complex organization of lipids. The biological principle that structure attends for function implies that there must be evolutionary functional advantages for such a complex lipid repertoire and distribution. Since their existence proposed by Simons and Ikonen, lipid rafts have been frequently invoked as specialized platforms necessary for optimal protein function and envisaged to constitute a unique element of membrane structure superposed to the well-known protein mosaicity (28–40). A fundamental question arises in this context: What is the subtle interplay of interactions between the different lipids that endows such a unique mechanical behavior of biological membranes (8)? If lipid domains are present in real membranes, not only energetic contributions arising from line tension and long-range domain interactions must be explicitly considered in the mechanical kernel of the membrane (25, 41) but also kinetic effects related to the multiphase transport (42, 43) could nontrivially shape the functional dynamics in such heterogeneous membranes.

Conclusions

A systematic study of the surface flow properties has been performed with monolayers of different membrane lipids. We propose that the concept of membrane fluidity is linked to the ability to flow under shear, to the absence of shear modulus (G^′ = 0) and to low frictional losses characterized by a low viscosity coefficient. Such a fluid-like behavior has been evidenced for lipid layers mimetic of different biological membrane structures: prokaryote (E. coli polar lipid extract) and eukaryote (POPC and SM mixed with cholesterol). These fluid layers have been evidenced to undergo Newtonian flow characterized by a low shear viscosity (η₀ ≈ 10^-5 N s/m). In only two particular cases solid-like rigidity was encountered: (a) the gel-phase of single saturated phospholipids (viscoelastic, G^′′ > G^′ > 0) and (b) solid ceramide (typically solid, G^′ > G^′′). Consequently, only under the strong fluidity requirement (G^′ = 0), membrane proteins might be able to undergo lateral translation/rotation in diffusion processes. Although caution is necessary before generalizing our results to biologically relevant situations, they capture the essentials of mechanical behavior for the different lipid phases found in biological membranes. They might influence the current wisdom at three levels: (a) molecular, adequate lipid mixing and consequent phase behavior enables functional rheology; (b) biophysical, highly fluid, moderately fluid, viscoelastic or solid-like rheology can be observed for different membranes or membrane sites; and (c) biological, specific membrane function entails adequate—evolutionally optimized—membrane rheology.

Methods

Monolayer Preparation.

Lipids were purchased from Avanti Polar Lipids and used without further purification. Lipid monolayers are spread dropwise on a buffered aqueous subphase (50 mM Hepes pH7, 0.1 mM EDTA in Milli-Q water) from a chloroform solution (ca. 1 mg/mL). All substances were purchased from Sigma Aldrich at the highest purity.

Surface Shear Rheology.

We used an Anton Paar Physica model MCR-301 rheometer, equipped with a biconical bob (68.3 mm diameter, 5° cone angle). A plane shear strain is applied and normal axial torque is measured in the oscillatory mode, γ = γ₀ sin ωt (γ₀ is the strain amplitude). Using the theoretical treatment of interfacial flow established by Oh and Slattery (44), after correction from bulk water contributions, G^′ and G^′′ are calculated as the material parameters defining the interfacial stress σ(t) = γ₀ (G^′ sin ωt + G^′′ cos ωt), the surface shear viscosity being η = G^′′/ω. Temperature was controlled with a Peltier element.