Paul trapping of charged particles in aqueous solution

Abstract

We experimentally demonstrate the feasibility of an aqueous Paul trap using a proof-of-principle planar device. Radio frequency voltages are used to generate an alternating focusing/defocusing potential well in two orthogonal directions. Individual charged particles are dynamically confined into nanometer scale in space. Compared with conventional Paul traps working in frictionless vacuum, the aqueous environment associated with damping forces and thermally induced fluctuations (Brownian noise) exerts a fundamental influence on the underlying physics. We investigate the impact of these two effects on the confining dynamics, with the aim to reduce the rms value of the positional fluctuations. We find that the rms fluctuations can be modulated by adjusting the voltages and frequencies. This technique provides an alternative for the localization and control of charged particles in an aqueous environment.

Trapping a single molecule or colloidal particle offers an unique opportunity to study the intrinsic individual characteristics rather than the averaged ensemble properties (1). Over the last decades, various techniques have been developed to achieve trapping in liquids, including optical tweezers (2), acoustic tweezers (3), and magnetic tweezers (4). Electrical forces for manipulating small objects in an aqueous solution include electrophoresis (EP) and dielectrophoresis (DEP) (5). Electrophoretic forces arise from the interaction of the object’s fixed charge and an external electric field, whereas DEP arises from the object’s polarizability in a spatially inhomogeneous electric field. To date, the electrical trapping of objects in solution has been done primarily by DEP (6, 7). Even though most macromolecules (DNA molecules, for example) suspended in aqueous solutions develop net electric charges (by either the dissociation of chemical groups or the adsorption of ions or molecules from the solution), utilization of the direct charge-field interaction to trap objects in aqueous solution has been rarely explored. It was realized five decades ago that charged particles (e.g., ions) can be trapped and confined in inhomogeneous, oscillatory electric fields. The best-known examples are quadrupole Paul traps (8), which have been used in many fields, such as mass spectrometry (9), analytical chemistry (10), and quantum information processing (11). Moreover, nanoscale Paul traps may be capable of trapping a single electron (12), ions in aqueous solutions (13), as well as a long DNA polymer (14). In contrast to the 3D Paul traps, a linear Paul trap is compatible with standard microfabrication technology and can thus be mass produced (15). Linear Paul traps confine the ions radially by a 2D rf field and transport the ions axially by an applied axial electric field (16). However, all Paul traps and the variants (11, 15, 17) experimentally realized so far have only been operated either in vacuum or in gaseous phase. An aqueous Paul trap remains an unexplored area. In fact, there are contradictory predictions whether a Paul trap is applicable in an aqueous environment (13, 17, 18).

This work serves as a first step toward realizing an aqueous Paul trap. We use a planar Paul trap device to experimentally demonstrate the feasibility of trapping charged particles in an aqueous solution, as previously predicted by molecular dynamic simulations (13). Our device functions similarly to a conventional linear Paul trap (16), with the addition of an advantageous damping term due to the fluid viscosity. We also investigate the impact of the Brownian noise on the confining dynamics, aiming to reduce the rms value of the positional fluctuations. We find that, by using finely tuned driving parameters, the rms fluctuations can be significantly modulated and minimized.

Materials and Methods

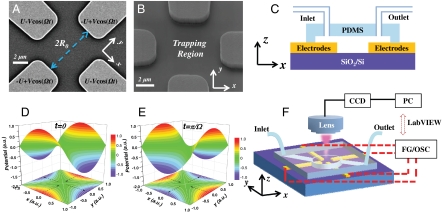
The planar aqueous Paul trap (PAPT) devices are produced by conventional microfabrication methods on an insulating SiO₂ substrate. Quadruple microelectrodes define the confinement region (Fig. 1 A and B; 2R₀ denotes the device’s physical size, which varies between 2 and 8 μm in this work). The devices are assembled with polydimethylsiloxane microfluidic chambers to form the functional devices (Fig. 1C). Detailed fabrication process is presented in the SI Appendix, section S1. The assembled devices are wire-bonded and mounted onto a printed circuit board. Voltages in the form of ± (U - V cos Ωt) are used to perform the trapping experiments (Fig. 1A). Here U is the dc voltage in series with the time-varying rf sinusoidal voltage of amplitude V and angular frequency Ω (= 2πf, and f is the frequency in hertz). The carefully controlled fabrication process results in smooth electrode sidewall profiles (Fig. 1B). Particles in the microfluidic chamber move freely in the x-y plane but are constrained mechanically in the z direction by the chamber height (2.5 μm in this experiment, molded using SU-8 2002) (Fig. 1C). Future designs could incorporate an additional electric field perpendicular to the trapping field, if the particle translocation through the trap is desired.

Fig. 1.

PAPT devices and experimental platform. (A) SEM of PAPT devices before integration with a microfluidic interface. The ac/dc voltages are applied such that the potentials of any two adjacent electrodes are of same magnitude but opposite sign. The physical size of the device is denoted 2R₀. (B) Finely controlled processing results in smooth sidewalls of the electrodes, which helps to minimize the stray electric fields. (C) Sketch of a functional device with microfluidics integrated (not drawn to scale). PDMS, polydimethylsiloxane. (D and E) Illustration of working principles for the device shown in A under a pure ac case (U = 0). The x and y axes are normalized by R₀. The z axis is normalized by V. At t = 0, the resulting electric forces (dashed arrows) will focus positively charged particles along the y direction and defocus them along the x direction. Half an rf period later, the potential polarity is reversed and opposite electric forces are thus generated. If the ac potential changes at the right frequency, the charged particles become stuck in this rapid back-and-forth motion. (F) Schematic of the experimental setup. The whole setup is built around a microscope. A LabVIEW (National Instruments) program controls the function generator (FG) to create the ac/dc voltages. The real voltage applied to the device is measured by an oscilloscope (OSC) and recorded by the same LabVIEW program. The electrical connections are through Bayonet Neill-Concelman cables (dashed lines). The videos taken by CCD are stored in personal computer (PC) memory in real time.

The basic principle of the PAPT device is shown in Fig. 1 D and E. At time t = 0, the applied voltage creates a saddle shape potential such that positive charges will be pushed into the center of the device along the y direction but will be pulled away from the center along the x direction (Fig. 1D). After half an rf cycle (t = π/Ω), the polarity of the potential is reversed and the positive charges are subject to opposite forces (Fig. 1E). The focusing/defocusing forces alternate between x and y directions with the applied sinusoidal voltage. If the polarity of the voltage changes fast enough, charged particles become stuck in a rapid back-and-forth motion. Note that this mechanism also works for negatively charged particles in a similar way. Because the electric field intensity is at a minimum in the trap center (x = y = 0), an effective pseudoforce will push the charged particles (either positively or negatively charged) toward the center, where they become confined in the x-y plane.

The motion of the charged particles is monitored by an optical microscope (Olympus BX51) and the video is taken by a high-sensitivity digital CCD camera (Olympus DP70) with the highest shutter speed as fast as 1/44,000 s (Fig. 1F). Video processing is performed using the National Institutes of Health ImageJ platform (see SI Appendix, section S2 for details).

Charged particles used to verify the working principles are polystyrene beads (Polysciences) of two diameters (0.481 ± 0.004 μm and 0.982 ± 0.013 μm). The surfaces of these particles are functionalized with carboxylate groups (-COOH). These COOH surface groups are the origin of the negative charges (-COOH⇄COO^- + H⁺). SEM reveals that all the particles have a pronounced spherical shape.

The solutions used in our experiment are repeatedly washed with deionized (DI) water (milli-Q grade, resistivity 18 MΩ·cm) to obtain a low solution conductivity. The detailed protocol of solution preparation is described in the SI Appendix, section S3. A lower solution conductivity is preferred for the Paul trap effect (which is an ac electrophoretic effect) to dominate over the DEP effect (see SI Appendix, section S4 for a detailed discussion).

Results and Discussion

Confinement.

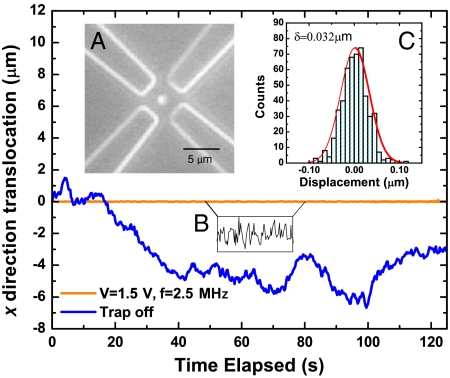
Fig. 2 presents the trapping results with PAPT devices. Inset A of Fig. 2 shows a typical image for a single trapped charged bead (of mean radius 490 nm). Individual particles can be stably held in the center of the device for up to 4 h (due to insignificant change over this time, we did not explore longer). Trapping mostly occurs for a single particle (instead of ensembles) due to interparticle Coulomb repulsion. The orange curve in Fig. 2 describes the time trace of the particle trajectory in the x direction when trapped under conditions of V = 1.5 V, U = 0 V, and f = 2.5 MHz. The blue curve depicts the Brownian motion when the trap is off (no electrical connection). We observe that the particles are not stationarily trapped but trapped with fluctuations (inset B of Fig. 2). Inset C shows the normal distribution of displacements derived from the orange trajectory in Fig. 2. A Gaussian fit yields an effective trap stiffness k = k_BT/δ² in the x direction as 4 pN/μm (k_B is Boltzmann constant and T is the absolute temperature). The motion in the y direction shows a similar property. Note that the confinement into 32-nm range is achieved with a 2R₀ = 8 μm device. We note that the trap stiffness of 4 pN/μm is not a characteristic value of this Paul trap. In fact, the Paul trap stiffness depends on the operation frequency, voltage, charge, and mass of the objects.

Fig. 2.

Particle trajectories when the trap is on (orange line) and off (blue line) in the x direction. Inset A shows a snapshot of a single particle confined in the center of the device. Inset B shows a magnification of fluctuations. Inset C is the histogram of the displacements for the orange curve. A Gaussian fit yields a trap stiffness of 4 pN/μm.

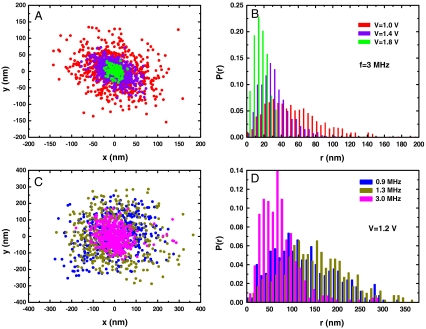
Most importantly, the rms fluctuations of the trapped particles can be tuned by externally applied voltages (U and V) and frequencies (f). Fig. 3 A and B shows the x-y positions of a trapped particle and the radial probability distributions at a fixed frequency (f = 3 MHz) and three different ac voltages. By adjusting the voltage, the degree of the radial confinement can be modulated. We observe a decrease of rms fluctuations with increasing the ac voltages (V) within ranges we can experimentally achieve. Fig. 3 C and D shows the x-y positions of a trapped particle and their radial probability distributions under a fixed voltage (V = 1.2 V) and three different frequencies. A slight decrease of rms fluctuations when increasing the frequency is visible for the data presented. However it is not necessarily true that increasing the frequency will reduce the rms fluctuations. As a matter of fact, because of the complexity of achieving an impedance match for the rf circuit, it is very difficult to maintain a fixed voltage for various frequencies during the experiments. Therefore, the frequency dependence can not be decoupled from the voltage dependence (Fig. 3A and B). We do not have a conclusive trend for the frequency dependence at the current stage. Nevertheless, we can experimentally achieve a tight or loose confinement by adjusting the applied voltages and frequencies (Movie S1). In addition, we are also able to repel a confined particle from the trap and to resume confinement after the particle escapes from the trap (Movie S1).

Fig. 3.

Effect of the applied voltages and frequencies on the confinement of particles. Experiments are performed with 491-nm radius particles and R₀ = 4-μm devices. No dc voltages are applied (U = 0). (A) The x-y positions of a single particle trapped under a fixed frequency (f = 3 MHz) and three different voltages. (B) Radial probability histograms (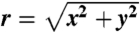) corresponding to the datasets in A. P(r) is defined such that ∫P(r)2πrdr = 1. (C) The x-y positions of a single 490-nm radius particle trapped under a fixed voltage (V = 1.2 V) and three different frequencies. (D) Radial probability histograms corresponding to the datasets in C.

Theoretical Modeling.

Unlike the case of charged particles in a vacuum Paul trap, which has been extensively studied and described by Mathieu equations (16), the motion of charged particles in an aqueous environment is governed not only by the external electric fields but also by additional damping forces and thermally induced fluctuations (i.e., Brownian motion). The last two forces always appear together according to the fluctuation-dissipation theorem (19). This kind of system, as suggested by Arnold et al. in their study of trapping microparticles in the atmosphere near standard temperature and pressure, necessitates a stochastic approach (20).

Assuming an ideal planar rf/dc quadrupole electric potential, resulting from the applied voltages as shown in Fig. 1A,

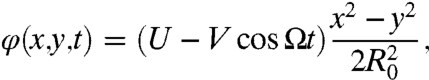[1]

the motion of a homogeneous charged particle with mass M, radius r_p, and net charge Q in the presence of a stochastic force can be written as (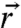 is the particle radial position vector in x-y plane, ),

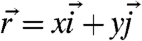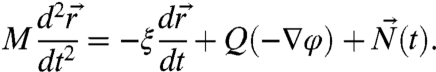[2]

The three terms on the right-hand side of Eq. 2 are the damping force, the electric driving force, and the Brownian noise force, respectively. The Stokes’ drag coefficient ξ can be approximated by ξ = 6πηr_p, where η is the dynamic viscosity of the aqueous solution. 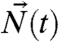is a random force due to thermal fluctuation, with the properties 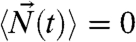and 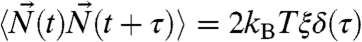, where δ(τ) is the Dirac delta function.

Rewriting Eq. 2 into a parametric dimensionless form, the motion in the x and y direction takes the form of a Langevin equation,

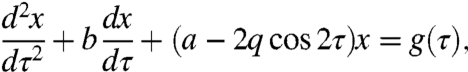[3a]

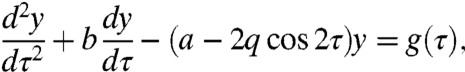[3b]

where τ = Ωt/2 is a dimensionless scaled time, 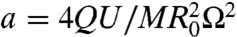is the scaled dc voltage, 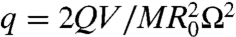is the scaled ac voltage, b = 2ξ/MΩ is the scaled damping coefficient, and g(τ) is the scaled thermal fluctuation force, following a Gaussian distribution with zero mean and standard deviation of .

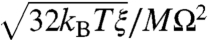It is worth noting that the geometry of our PAPT devices is not an ideal 2D structure, which would require high aspect ratios for the four electrodes (16). However, 3D calculations (SI Appendix, section 6) show that the analysis will not be affected significantly as long as the particle remains within the height of the electrodes. Above the electrodes, the potential changes as if the device radius R₀ is increased. As a result, we can deal with this nonideal 2D situation by adopting an effective device radius 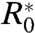. Moreover, the potential profile of the PAPT device is not exactly an ideal quadruple field because of the existence of higher-order components. Taking these two effects into account, a correction factor Γ should be introduced in the expression of a and q,

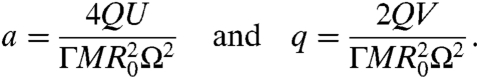[4]

The solutions of Eq. 3 will determine the dynamics of particles inside the trap. As is well known for Paul traps in vacuum [b = 0 and g(τ) = 0], stable trapping will only occur when parameters (q,a) are within certain regions in the q - a diagram (where Eq. 3 has convergent solutions) (8). If a viscous medium is present (b > 0, for example, air or water), the stable region in the q - a diagram will not only be shifted but also be extended (21). The deterministic damped Mathieu equation without taking thermal fluctuations into consideration reads as the homogeneous part of Eq. 3 [with g(τ) = 0]. With this deterministic system, the particles should settle toward the center of the device (x = y = 0) and eventually be trapped without moving when time t → ∞ if the (q,a) parameters are inside the stability region. This prediction is, however, not true in our experiment, where positional fluctuations are observed (Fig. 2). The fluctuations of the trapped particles confirm the necessity to include the stochastic Brownian effect to study the PAPT device. The questions that arise are how this white Brownian noise affects the stability of the trapping dynamics and the rms value of the position fluctuations. We will address these two aspects in the following discussion.

Brownian Noise Effect on Trapping Stability.

Zerbe et al. (22) theoretically showed that the variance of position displacement fluctuations remains bounded for (q,a) parameters that are located within the stability zones of the damped deterministic equation [Eq. 3 with g(τ) = 0]. As a result, the trapping stability is solely determined by the behavior of the deterministic system and the Brownian noise would not affect the stability boundaries. The (q,a) stability region for various damping factors b can be numerically determined using Hasegawa and Uehara’s method (21). It is thus very interesting to experimentally map out the stability boundary and compare it with the theoretical predictions. The principal problem here is that, unlike atomic ions, the particles are neither identical in mass nor charge. Therefore, the boundary mapping requires that all points in the stability boundary be derived from a specific single bead throughout the experiment. We are able to record each boundary point in (V,U) coordinates successfully without losing the single trapped particle by carefully adjusting the ac and dc voltages at a fixed frequency and by recognizing when the motion is on the verge of no longer being stable. Eq. 4 translates the measured boundary from (V,U) coordinates into (q,a) coordinates by using a fitting parameter Q/ΓM (effective charge to mass ratio), where Γ is the correction factor mentioned above.

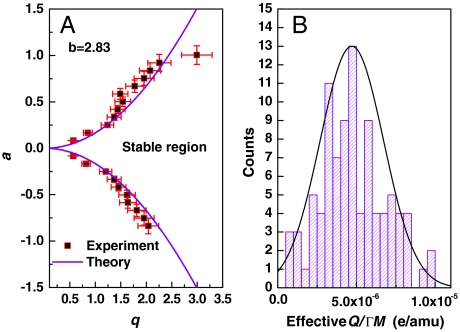
As shown in Fig. 4A, the resulting measured limits of the (q,a) stability boundary reproduce the theoretical calculated boundary very well. This remarkable agreement between the theoretical boundary and experimental data strongly proves that the trapping dynamics are dominated by the Paul trap mechanism, because a DEP trap would not have such a (q,a) stability boundary. The DEP forces only contribute small perturbations near the (q,a) origin (SI Appendix, section 7.1). Determination of boundary points becomes difficult for large a values because this requires higher dc voltages, and we find experimentally that dc voltages beyond 2.2 V (corresponds to a = 1.34 using Q/ΓM = 4 × 10^-6 e per atomic mass unit and R₀ = 4 μm) will result in detrimental electrochemical reactions of the metal electrodes. Surface modifications or passivations of the electrodes may improve the tolerance of high dc voltages.

Fig. 4.

(A) Experimental points on the boundary curves of the stability diagram as observed from a single charged particle (experiments performed with a radius of 0.491 ± 0.0065 μm at a fixed frequency f = 2 MHz). The solid line is the theoretically calculated stability boundary for Q/ΓM = 4 × 10^-6 e per atomic mass unit. The dimensionless damping coefficient b = 2.83 is calculated by b = 2ξ/MΩ, using known parameters. (B) Gaussian distribution of fitting Q/ΓM for a total of 121 beads from the same solution. The mean value is 4.77 × 10^-6 eper atomic mass unit and standard deviation is 1.95 × 10^-6 e per atomic mass unit.

By using the fitting techniques described above, we are able to evaluate the distribution of effective charge to mass ratio (Q/ΓM) for a collection of beads. We analyzed a total of 121 beads from the same suspension solution and extracted the Q/ΓM for each single bead. Fig. 4B shows a Gaussian distribution for the extracted Q/ΓM, with a mean value of 4.77 × 10^-6 e per atomic mass unit. This value corresponds to around 10⁶ elementary charges on a single bead, which is two orders of magnitude lower than the number of carboxylate surface groups. This discrepancy may be due to the partial dissociation of carboxylate groups in solution and the charge renormalization effect (23).

Brownian Noise Effect on rms Fluctuations.

Although the serial dc voltages (U) can be used to tune the trapping stability and thus the dynamics in PAPT devices (Fig. 4A), a pure ac field (U = 0) is experimentally favorable due to the obvious advantages of an ac over a dc electric field in solution. In particular, electroosmosis flow does not develop in the bulk, and electrochemical reactions can be avoided. Thermal convection can also be suppressed because the heating effect of an ac field is less (24). As a result, ac fields are of more practical interest in the context of aqueous solutions.

The rms fluctuation in the x and y directions for the ac only case (a = 0) can be expressed as (25),

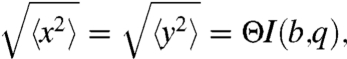[5]

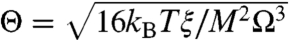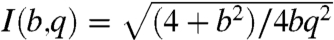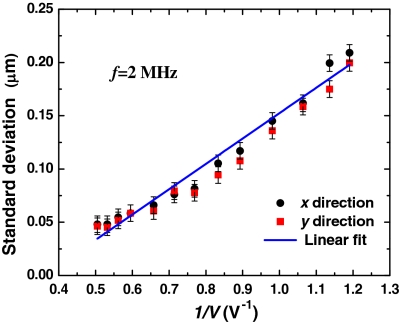
where and I(b,q) is a function of dimensionless parameters b and q. The rms fluctuations as a function of applied ac voltages (V) at a fixed frequency (f = 2 MHz) observed in the experiment is given in Fig. 5. Because I(b,q) can be approximated as for small q (20) (note that the working parameter b and maximum possible q are calculated to be 2.83 and 0.604, respectively), the rms fluctuations thus have the dependence on the ac voltage as 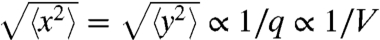for a fixed frequency (fixed damping factor b) in small q region. The linear fitting curve in Fig. 5 demonstrates a remarkable agreement with the predicted linear dependence of rms fluctuations on 1/V. We perform this experiment under several frequency conditions and all of them show the same linear dependence (SI Appendix, section 8). This dependence is intuitively correct (stronger field gives a tighter trap). However, it is not necessarily true for the whole ac voltage range. Theoretical studies showed that there always exists a minimal rms fluctuation if proper working parameters q are chosen within the stability region (25). By taking the experimental parameters as f = 2 MHz, Q/ΓM = 4 × 10^-6 e per atomic mass unit, and b = 2.83, we can calculate that the minimal rms fluctuations corresponds to q = 2.78 and V = 9 V. This ac voltage is beyond our instrument’s ability (V_max = 5 V) and therefore we only experimentally observe a decrease of rms fluctuations when increasing ac voltages are within ranges we can achieve (Fig. 3 A and B).

Fig. 5.

Dependence of the standard deviation of position fluctuations of trapped bead on ac voltage at fixed frequency (2 MHz). The reduced χ² value for the linear fitting is calculated as 1.4.

The rms fluctuation dependence on the driving parameters when a = 0 is theoretically studied in several works (22, 25–27). The magnitude of the minimal fluctuation (which determines the size of a virtual nanopore) can be expressed as 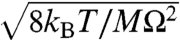, which is dependent only on the environment temperature T, the particle mass M, and the tunable working frequency Ω. This minimal fluctuation will happen when (25). The existence of such a minimum in the positional fluctuation of the stochastically confined motion is of considerable importance because one can significantly reduce the thermal noise effect on the positional uncertainty of the motion. It is noteworthy that the operating parameters (q,a) must be inside of the stability region to achieve this minimal fluctuation. The minimal fluctuation for the particle shown in Fig. 5 would be 0.63 nm (with M = 520 fg and working frequency f = 2 MHz). It is apparent that higher frequency can be adopted to suppress the positional uncertainty to the greatest extent for the reduced M, if the parameters q, a, and b are kept within the stability region. For example, for a 1,000-bp dsDNA (650 Da/bp, charge to mass ratio 3 × 10^-3 e per atomic mass unit), when the working frequency is increased to 442 MHz, the minimal achievable fluctuation is around 2 nm (close to the size of a physical nanopore; refs. 28–30). By careful rf circuit design, this frequency could be experimentally achievable. The practical limits of the confinement is determined by the highest frequency that can be applied without causing detrimental heating or device damage.

Conclusions

In summary, we experimentally demonstrate the feasibility of a Paul-trap-type planar device working in aqueous solutions. An oscillating quadrupole electric field generates a pseudopotential well and the charged particles are dynamically confined to a nanometer scale region, whose size can be externally tuned by driving parameters (voltages and frequencies). This technique opens up the possibility of spatially controlling the object in a liquid environment and can lead to lab-on-a-chip systems controlling single molecules that often appear charged when submerged in water. Further investigations such as the impact of variation of the solution’s ionic composition, concentrations, and pH on the trapping performance are needed for a better understanding for biomolecular applications.