Potentiometric Sensors Based on Fluorous Membranes Doped with Highly Selective Ionophores for Carbonate

Abstract

Manganese(III) complexes of three fluorophilic salen derivatives were used to prepare ion-selective electrodes (ISEs) with ionophore-doped fluorous sensing membranes. Because of their extremely low polarity and polarizability, fluorous media are not only chemically very inert but also solvate potentially interfering ions poorly, resulting in a much improved discrimination of such ions. Indeed, the new ISEs exhibited selectivities for CO₃²⁻ that exceed those of previously reported ISEs based on non-fluorous membranes by several orders of magnitude. In particular, the interference from chloride and salicylate was reduced by two and six orders of magnitude, respectively. To achieve this, the selectivities of these ISEs were fine-tuned by addition of non-coordinating hydrophobic ions (i.e., ionic sites) into the sensing membranes. Stability constants of the anion–ionophore complexes were determined from the dependence of the potentiometric selectivities on the charge sign of the ionic sites and the molar ratio of ionic sites and the ionophore. For this purpose, a previously introduced fluorophilic tetraphenylborate and a novel fluorophilic cation with a bis(triphenylphosphoranylidene)ammonium group, (R_f6(CH₂)₃)₃PN⁺P(R_f6(CH₂)₃)₃, were utilized. The optimum CO₃²⁻ selectivities were found for sensing membranes composed of anionic sites and ionophore in a 1:4 molar ratio, which results in the formation of 2:1 complexes with CO₃²⁻ with stability constants up to 4.1 × 10¹⁵. As predicted by established theory, the site-to-ionophore ratios that provide optimum potentiometric selectivity depend on the stoichiometries of the complexes of both the primary and the interfering ions. However, the ionophores used in this study give examples of charges and stoichiometries previously neither explicitly predicted by theory nor shown by experiment. The exceptional selectivity of fluorous membranes doped with these carbonate ionophores suggests their use not only for potentiometric sensing but also for other types of sensors, such as the selective separation of carbonate from other anions and the sequestration of carbon dioxide.

Introduction

Because HCO₃⁻, CO₃²⁻, and CO₂ coexist in aqueous solutions, accurate and quick determinations of so-called “total CO₂ content” are required for clinical, physiological, industrial, and environmental analysis. To date, almost all CO₂ determinations are performed with Severinghaus-type potentiometric sensors after sample acidification to pH < 5.5, permeation of CO₂ through a gas-permeable membrane, and redissolution in an inner solution compartment.^1,2 While widely employed, this method suffers from slow responses and the complexity associated with sample acidification. In comparison, ion-selective potentiometry offers the advantage of direct ion measurements.^3–7 However, due to the high free energies of hydration of HCO₃⁻ and CO₃²⁻ (335 kJ/mol and 1315 kJ/mol, respectively),⁸ phase transfer of these ions from aqueous into water-immiscible phases is energetically very unfavorable. Consequently, ionophore-free ion-exchanger electrodes show only weak responses to both anions.

To enhance the response and selectivity, an ionophore that binds HCO₃⁻ or CO₃²⁻ and thereby enhances sensor selectivity is needed.⁹ While analysis of HCO₃⁻ would be favored by the fact that it is the most abundant CO₂ species in blood at physiological pH, an ionophore for HCO₃⁻ that can be used in ion-selective electrodes (ISEs) has yet to be reported. Several ionophores for CO₃²⁻ were described and used to measure CO₃²⁻ in blood. Trifluoroacetyl-p-alkylbenzenes have been the most promising carbonate ionophores.^10–13 Unfortunately, poor selectivities for CO₃²⁻ over Cl⁻ and interference from salicylate (Sal⁻) limit real-life applications of ISEs based on trifluoroacetophenone derivatives. Substantial work was performed to improve the selectivity for CO₃²⁻, e.g., by variation of the substituents on the benzene ring.^14–17 Higher CO₃²⁻ selectivities were also obtained by molar ratios of ionophore and added ionic sites that favor 1:2 complexes of CO₃²⁻ and the ionophore,^18,19 or by use of tweezers-type ionophores with two trifluoroacetobenzoyl groups (unfortunately, thermodynamically meaningful selectivities were not reported for the latter).²⁰ The use of membranes with a hydrophilic, porous layer on top of the ionophore-doped membrane^21–24 or plasticized silicone rubber or sol-gel matrixes^25–27 were also reported to improve the discrimination of lipophilic anions such as Sal⁻, decreasing their interference. However, ionophore-based CO₃²⁻ ISEs have not yet met the selectivity requirements of routine analysis in clinical chemistry and are not used currently in major commercial clinical analyzers.

Consequently, ionophores with improved CO₃²⁻ selectivities are still in need. We discovered in the work described here a promising class of ionophores not previously recognized as binding CO₃²⁻. In earlier work, three fluorophilic manganese(III) complexes of salenes (Mn-1, Mn-2, and Mn-3) were shown to catalyze epoxidation reactions in fluorous media.^28–30 Since porphyrins with Mn(III) centers had been reported as successful Cl⁻ ionophores for use in non-fluorous ISE membranes,³¹ we suspected that Mn-1, Mn-2, and Mn-3 might provide good selectivities for Cl⁻ when used in fluorous ISE membranes. However, preliminary studies of fluorous ISE membranes doped with these compounds showed intriguing CO₃²⁻ selectivities, leading us to optimize the potentiometric selectivity for this ion. Indeed, from the view of hard-soft acid base (HSAB) theory, the high selectivity for CO₃²⁻ is not too surprising since Mn(III) is a hard Lewis acid and has a high affinity for oxygen ligands such as CO₃²⁻.

The selectivity of the thus obtained ISEs is significantly enhanced by use of fluorous membrane matrixes, which are the least polar and polarizable condensed phases known.^32–36 Since ionic species that do not form ionophore complexes are poorly solvated in fluorous media and strong binding between the ionophore and the target ion is favored in these weakly coordinating matrixes,³⁷ chemical sensors with fluorous sensing membranes are highly selective. An ionophore-based H⁺ ISE, e.g., was shown to be more selective than the well known pH glass electrodes.³⁸ Also, an ISE with a fluorophilic Ag⁺ ionophore exhibited selectivities over many heavy metal ions exceeding those of the selective non-fluorous ISEs by a factor of 100 and more, permitting Ag⁺ detection at the low parts-per-trillion level.³⁹ This work shows significant enhancements in CO₃²⁻ selectivities of fluorous sensing membranes based on receptors Mn-1, Mn-2 and Mn-3. In the course of the optimization of the sensing membranes, the stoichiometries and stabilities of the complexes with CO₃² and interfering anions were determined. These results shed new light on the molecular recognition of carbonate and provide hints as to how new host compounds could be designed to exhibit even higher carbonate selectivity.

Experimental Section

Reagents and Materials

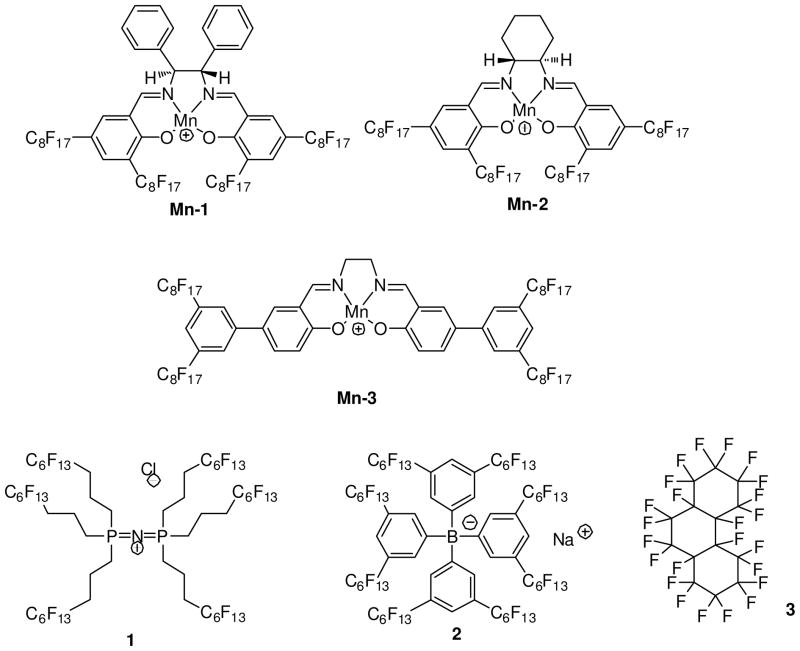
The perfluoroalkylated salen manganese(III) complexes Mn-1, Mn-2, and Mn-3 were prepared by refluxing solutions of the salen ligands in ethanol with an excess of Mn(OAc)₂·4H₂O under aerobic conditions, as reported previously.²⁹ Sodium terakis[3,5-bis(perfluorohexyl)phenyl]borate, 2, was prepared according to previously described procedures.⁴⁰ Perfluoroperhydrophenanthrene, 3, and tris(hydroxymethyl)amino-methane (Tris) were obtained from Alfa Aesar (Ward Hill, MA). Fluoropore membrane filters (pure polytetrafluoroethylene, 47 mm diameter, 0.45 μm pore size, 50 μm thick, 85% porosity) were purchased from Millipore (Bedford, MA). All salts and sulfuric acid were obtained from Mallinckrodt Baker (Paris, KY). Deionized and charcoal-treated water (18.2 MΩ·cm resistance) purified in a Milli-Q PLUS reagent-grade water system (Millipore) was used for all sample solution preparations.

Synthesis of 1 ((R_f6(CH₂)₃)₃PN⁺P(R_f6(CH₂)₃)₃PCl⁻, bis(tri[(perfluorohexyl)propyl]phosphine)iminium chloride)

This compound was prepared by a modification of the literature procedure for (Ph₃P)₂N⁺Cl⁻.⁴¹ A Schlenk flask was charged with P((CH₂)₃Rf₆)₃ (0.820 g, 0.73 mmol) in 7 mL tris(perfluoropentyl)amine ((CF₃CF₂)₄)₃N, bp 215 °C). Finely powdered PCl₅ (0.104 g, 0.50 mmol) was added to the mixture with stirring, and the homogeneous mixture was heated to 110–115 °C for 1 h. The byproduct PCl₃ was distilled off under vacuum (3 × 10⁻² mbar) after the system was cooled down to 85–90 °C. NH₂OH·HCl (0.018 g, 0.26 mmol) was added, and the stirred mixture was heated to 150–155 °C for 15 h with a reflux condenser connected to a concentrated H₂SO₄ guard tube. The thus obtained homogeneous transparent but lightly yellow solution was cooled to room temperature, and the white precipitate that formed was removed. The solvent, tris(perfluoropentyl)amine, was evaporated under oil pump vacuum at 120–125 °C, and the remaining white residue was washed three times with 5 mL perfluorohexanes. Ethanol was added, and the sample heated to 60 °C with stirring, and conc. HCl was added dropwise. Two layers formed. The lower, sticky layer was separated, washed several times with water, and dried by oil pump vacuum. The remaining sticky liquid gave a waxy white solid on standing overnight (0.413 g, 0.18 mmol, 70%). ¹H NMR (CF₃C₆H₅/CDCl₃, 2:1 v/v), δ 2.24–2.33 (m, 12 H, CH₂CF₂), 2.00 and 1.90 (br, 12 H, CH₂CH₂, and 12 H, PCH₂). ³¹P NMR (CF₃C₆H₅/CDCl₃, 2:1 v/v), 47.0 (s). MS (MALDI-TOF, m/z): 2242.4 ((R_f6(CH₂)₃)₃PN⁺P(R_f6(CH₂)₃)₃P (100%), 1129.1 ((R_f6(CH₂)₃)₃PNH⁺, 26%); for the spectrum see the Supporting Information Figure S1.

Electrodes

Fluorous sensing phases were prepared by addition of ionophore and ionic sites into perfluoroperhydrophenanthrene, 3 (for the concentrations of these components, see Table 1), and stirring of the resulting mixtures overnight with a magnetic stirring bar to ensure complete dissolution. To prepare membrane supports, porous Fluoropore filters were sandwiched in between two note cards and cut with a 13 mm diameter hole punch. The addition of approximately 20 μL of the liquid sensing phase onto a stack of two filter supports changed the appearance of the latter from opaque white to translucent with a shiny surface. The thus obtained supported liquid membranes were mounted into custom-machined poly(chlorotrifluoroethylene) electrode bodies, which were sealed with screw caps that exposed the sensing membranes to the sample solutions through an 8.3 mm diameter hole. All electrodes contained two liquid compartments, i.e., an outer filling solution in contact with the backside of the sensing membrane, and an inner filling solution in contact with a AgCl-coated Ag wire. The two compartments were separated by a small cotton plug that was tightly packed into a tapered plastic pipette tip.⁴² The inner filling solution was always 1 mM in KCl. To measure CO₃²⁻ responses and selectivities measurements relative to CO₃²⁻, the outer filling solutions contained 4 mM NaHCO₃ buffered with 10 mM Tris-H₂SO₄ to pH 8.75. In those cases where selectivities were determined with respect to an anion other than CO₃²⁻, the outer filling solution contained a 1 mM sodium salt of the respective anion (i.e., SCN⁻ or NO₃²⁻). In all cases, the electrodes were conditioned for 2–3 h in solutions of the target ion (primary ion) prior to measurements.

Table 1.

Composition and properties of sensing membranes doped with one of the three ionophores Mn-1, Mn-2 and Mn-3 in different ratios of ionic sites and ionophore.

Sensing Membrane No.	Ionophore (L)	Ionic sites (RzR,char)		Detection Limits/M	CO32− selectivity ( $log{K}_{{co}_3^{2-},J}^{\mathit{pot}}$)
		zR,char	[RzR,char]/Ltot		SCN−	HO−	BPh4−
1a	Mn-1	+1	2:3	2.0 × 10−5	−1.59 ± 0.16	1.07 ± 0.16	8.54 ± 0.22
2a	Mn-1	+1	4:3	1.7 × 10−5	5.60 ± 0.20	3.21 ± 0.24	17.30 ± 0.33
3b	Mn-1	−1	1:4	1.9 × 10−5	3.28 ± 0.20	5.14 ± 0.13	3.72 ± 0.12
4a	Mn-2	+1	2:3	5.2 × 10−5	0.46 ± 0.14	3.90 ± 0.15	12.04 ± 0.18
5a	Mn-2	+1	4:3	1.5 × 10−5	2.54 ± 0.14	3.06 ± 0.32	14.16 ± 0.30
6b	Mn-2	−1	1:4	3.0 × 10−4	−2.78 ± 0.20	7.23 ± 0.07	0.22 ± 0.17
7b	Mn-3	+1	1:4	2.0 × 10−4	1.02 ± 0.22	6.27 ± 0.18	4.78 ± 0.10

^aConcentration of ionophore: 1.5 mM.

^bConcentration of ionophore: 4.0 mM.

Potentiometric Measurements

Potentiometric measurements were carried out using a double-junction type external reference electrode (DX200, Mettler Toledo, Switzerland; 3.0 M KCl saturated with AgCl as inner filling solution, and 1.0 M LiOAc as bridge electrolyte) and an EMF 16 potentiometer (input impedance 10 TΩ) controlled with EMF Suite 1.03 software (Lawson Labs, Malvern, PA). Calibrations for CO₃²⁻ were determined by stepwise dilution of buffered NaHCO₃ solutions with pure pH buffer (10 mM Tris-H₂SO₄, pH 8.75) and continuous EMF monitoring. The CO₃²⁻ concentrations were calculated as suggested by Herman and Rechnitz.¹² Single-ion activity coefficients for CO₃²⁻ and HCO₃⁻ were determined with a two-parameter Debye-Hückel approximation.⁴³ Calibration curves for other anions were obtained analogously by successive dilution of concentrated solutions with water. Nernstian responses were confirmed for all anions of interest in the concentration range where selectivities were measured. All EMF values were corrected with the Henderson equation for liquid-junction potentials,⁴⁴ and activity coefficients were calculated as described in ref. 43. Selectivity coefficients were determined with the separate solution and fixed interference methods;^45,46 reported values are averages for three electrodes (typical deviation, ±0.3).

Results and Discussion

The three fluorophilic ionophores Mn-1, Mn-2, and Mn-3 are derivatives of salen, whose name is derived from the reagents from which it is prepared (i.e., salicyl aldehyde and ethylenediamine). Tetradentate salen ligands bind Mn(III) with a geometry similar to that of the roughly planar metalloporphyrins, and coordination of one or two additional ligands to the metal center results in square pyramidal or octahedral coordination spheres, respectively.⁴⁷ Indeed, both pentacoordinated and hexacoordinated Mn(III) complexes are common. In the following, the additional (non-salen) ligands will be referred to as pseudo-axial ligands, and the symbol L⁺ will be used to represent the Mn(III) salen cores of Mn-1, Mn-2, and Mn-3 without these pseudo-axial ligands.

The molar ratio of non-coordinating hydrophobic ionic sites and the ionophores Mn-1, Mn-2, and Mn-3 can be used to control the potentiometric selectivity^48–50 of membranes containing these compounds. For a more thorough discussion, the reader unfamiliar with site theory will have to consult the specialized literature.^48–50 Briefly, both the ionophore and the ionic sites are so hydrophobic that they are confined to the hydrophobic (water immiscible) sensing membrane. In an appropriately formulated ISE membrane, the only other ionic species are the target ions (typically referred to as primary ions) for which the ISE is selective. The potentiometric response of the ISE can be explained by the selective, ionophore-assisted transfer of these target ions from the aqueous sample into the sensing membrane, resulting in a sample–membrane phase boundary potential that depends logarithmically on the target ion activity in the aqueous sample. However, because of the principle of electroneutrality in the bulk of the sensor membrane, the charges and concentrations of the ionophore and ionic sites control the ratio of ionophore and target ions in the bulk of the membrane. If this ratio is large enough, there is an excess of ionophore, and the ISE membrane exhibits enhanced selectivity for the target ions. On the other hand, if all ionophore occurs in the form of complexes and no free ionophore is available, the ISE selectivity is low.

Site theory predicts ratios of ionic sites and ionophore that are likely candidates for highest selectivity.^48,49 However, the case of the CO₃²⁻-selective ionophores Mn-1, Mn-2, and Mn-3 is more complicated than most comparable systems described in the past because CO₃²⁻ may not only form 1:1 complexes (i.e., L⁺–CO₃²⁻) but it may also bind as a pseudo-axial ligand to two ionophore molecules (L⁺–CO₃²⁻–L⁺), and because these ionophores may also bind two pseudo-axial ligands to attain a hexacoordinated Mn(III) coordination sphere (CO₃²⁻–L⁺–CO₃²⁻). Consequently, the formation of 2:1, 1:1, and 1:2 complexes had to be considered when experimentally exploring which ionic site-to-ionophore ratio provides the highest potentiometric selectivity with Mn-1, Mn-2, and Mn-3. In the following, we first describe the logic that led us to test specific ratios of ionic sites and ionophores. Note that the discussion of the results that follows afterwards gives a detailed quantitative picture of the host guest chemistry in these sensing membranes, making it easier to understand this rather complicated system.

If an ionophore with the charge +1 forms complexes of 1:1 stoichiometry with, on one hand, the doubly charged CO₃²⁻ as primary ion and, on the other hand, a singly charged interfering anion, the highest selectivity for CO₃²⁻ is expected with cationic sites in a molar ratio of 62:100 to the ionophore (i.e., with a concentration of 62 mol % of cationic sites relative to the ionophore).^48,49 On a qualitative level, this optimum site ratio can be understood readily considering the concentration of free ionophore. If a sensing membrane is exposed only to the singly charged interfering anions, the 62 mol % cationic sites in the membrane enforce a 162:100 ratio of singly charged anions and ionophore in the membrane. Consequently, a large fraction of the singly charged anions do not get the chance to interact with the ionophore. To the contrary, if the sensing membrane is exposed to doubly charged CO₃²⁻, the molar ratio of ionic sites and ionophore in the membrane results in a 81:100 ratio of CO₃²⁻ and ionophore, leaving 19% of all ionophore is in its uncomplexed form; this results in a high CO₃² selectivity. Similarly, if 2:1 complexes may be formed in which the anion binds to two singly charged ionophore molecules, and if such bridged complexes are formed both for CO₃²⁻ and the singly charged interfering anion, 27 mol % of anionic sites can be predicted as the optimum ionic site concentration.^48,49 Finally, if the singly charged ionophore forms 2:1 complexes with CO₃²⁻ but 1:1 complexes with the singly charged interfering anion, site theory predicts that there is no distinct selectivity maximum; instead, the potentiometric selectivity gradually increases with the molar ratio of anionic sites and ionophore until a critical point is reached where counterion interference occurs.⁴⁸ To test which complex stoichometries are formed by the ionophores Mn-1, Mn-2, and Mn-3, sensing membranes containing ≈62% cationic or ≈27% anionic sites and one of the three CO₃²⁻ ionophores were prepared, and their potentiometric selectivities were determined (see Table 1).

Because of the ability of Mn(III) to form complexes with an octahedral coordination sphere, binding of two CO₃²⁻ ligands to one ionophore molecule, L⁺, had to be considered too. For electrostatic reasons, it was expected that the first CO₃²⁻ ligand would bind more strongly to the Mn(III) center ( ${\text{L}}^{+}+{\text{CO}}_3^{2-}\leftrightarrow {\left[{\text{LCO}}_3\right]}^{-}$) than the second CO₃²⁻ ligend ( ${\left[{\text{LCO}}_3\right]}^{-}+{\text{CO}}_3^{2-}\leftrightarrow {\left[\text{L}{{(\text{CO}}_3)}_2\right]}^{3-}$). In such a case, the [LCO₃]⁻ complex is considered formally by site theory as a negatively charged ionophore for CO₃²⁻, forming the complex [L(CO₃)₂]³⁻. However, the complex formed in the same ionophore-doped sensing membrane when the membrane is exposed to a solution of a singly charged interfering ion (e.g., SCN⁻) is formally an electrically neutral ionophore (e.g., [LSCN]). Cases in which the active ionophore is formally a charged ionophore when the ISE membrane is exposed to solutions of the primary ion, but an electrically neutral ionophore when the ISE membrane is exposed to solutions of an interfering ion (or vice versa) have not been discussed explicitly in the literature in the past. However, applying the same logic as it has been the basis of reported site theory, it can be predicted that the optimum selectivity for CO₃²⁻ over a singly charged interfering anion (e.g., SCN⁻) is expected for membranes containing cationic sites in a range of 100 to 300 mol % to the ionophore. In such a membrane, there is a considerable concentration of the species that formally acts as the ionophore (i.e., [LCO₃]⁻) when the membrane is exposed to CO₃²⁻ solutions, but the concentration of the species that acts as the free ionophore (e.g., [LSCN]) is extremely low when the sensing membranes are exposed to solutions of the singly charged interfering ion. (In the case of SCN⁻, the major membrane species would be [L(SCN)₂]⁻.) Based on these considerations, ISE membranes containing 133% cationic sites and one of the three fluorophilic CO₃²⁻ ionophores were prepared in addition to the membranes already mentioned in the preceding paragraph, and their potentiometric selectivities were determined too (see Table 1).

The fluorophilic salts bis(tri[(perfluorohexyl)propyl]phosphine)iminium chloride, 1, and sodium tetrakis[3,5-bis(perfluorohexyl)phenyl]borate, 2, were used to provide for cationic and anionic sites, respectively. As compared to salts of fluorophilic cations used in previous potentiometric studies, the bis(triphenylphosphoranylidene)ammonium cation of 1 has the advantage of a higher chemical stability in the presence of HO⁻ than previously reported fluorophilic phosphonium cations⁵¹ and does not bind coordinatively to analyte anions. Fluorous membranes doped with cationic site 1 did not show any sign of deterioration even when exposed to strongly alkaline solutions, such as 0.1 M NaOH, and exhibited no more interference from OH⁻ than expected for a non-specific ionophore-free ion exchanger membrane with Hofmeister⁵² selectivity (see also p 1595 of ref. 9). The logarithm of the potentiometric selectivity coefficient for hydroxide with respect to chloride, $log{K}_{{Cl}^{-},{OH}^{-}}^{\mathit{pot}}$, was determined to be −3.03, which means that the potentiometric response to hydroxide is 10^3.03 times weaker than to chloride (for a discussion of K^pot, see, e.g., refs. 3, 4, 45,48,46). As the fluorous membrane matrix, perfluoroperhydrophenanthrene, 3, was chosen because this matrix is a good solvent for both 1 and 2, and because its use excluded possible effects from impurities present in small concentrations in perfluoropolymers,⁵³ a possible complication that we wanted to avoid at this stage to explore the maximum selectivity obtainable with these new ionophores.

Optimized Potentiometric Responses and Selectivities

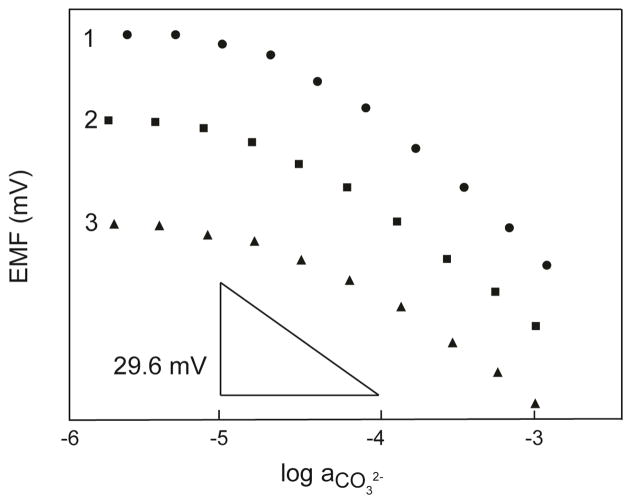
The CO₃²⁻ responses at pH 8.75 (10 mM Tris-H₂SO₄) of three electrodes (No. 1, 2, and 3 in Table 1) based on sensing membranes containing ionophore Mn-1 with three different site-to-ionophore ratios are shown in Figure 1. All three electrodes exhibited responses close to the expected theoretical (“Nernstian”) responses of −29.6 mV per tenfold change of the activity of CO₃²⁻ in the aqueous samples. The detection limits of the three electrodes, as determined according to IUPAC,⁵⁴ were 2.0 × 10⁻⁵, 1.7 × 10⁻⁵, and 1.9× 10⁻⁵ M, respectively. The observed response times were on the order of a few seconds, and were most likely limited only by the speed of complete sample change⁵⁵ in these experiments; they provide no information about the dynamics of complex formation, which indeed is desirable for potentiometric applications.

Figure 1.

Potentiometric CO₃²⁻ response of an ISE based on a liquid membrane with perfluoroperhydrophenanthrene doped with (1) 1.0 mM cationic sites, 1, and 1.5 mM ionophore Mn-1, (2) 2.0 mM cationic sites, 1, and 1.5 mM ionophore Mn-1, and (3) 1.0 mM anionic sites, 2, and 4.0 mM ionophore Mn-1. Sample solutions contained sodium bicarbonate buffered with 10 mM Tris-H₂SO₄ to pH=8.75. Response curves were shifted vertically relative to one another for enhanced clarity.

The selectivities of these electrodes for CO₃²⁻ over SCN⁻ and tetraphenylborate (BPh₄⁻) are shown in Table 1. The SCN⁻ anion was chosen for this purpose as a practically relevant interfering ion that binds to the ionophore, and BPh₄⁻ was chosen as a non-coordinating reference ion expected not to interact with the ionophore, permitting the determination of complex stoichiometries and binding constants. The highest CO₃²⁻ selectivity of the ISEs over BPh₄⁻, as seen from the lowest value for $log{K}_{{co}_3^{2-},{\mathit{BPh}}_4^{-}}^{\mathit{pot}}$, was obtained with the electrode membranes doped with anionic sites and ionophore Mn-1 in a molar ratio of 1:4. However, those sensors also exhibited relatively high interference from SCN⁻. Indeed, the selectivity for CO₃²⁻ over SCN⁻ for this kind of sensing membranes is not high enough to prevent significant interference from SCN⁻ (present in the blood of patients who smoke⁹) when applied to clinical analysis.

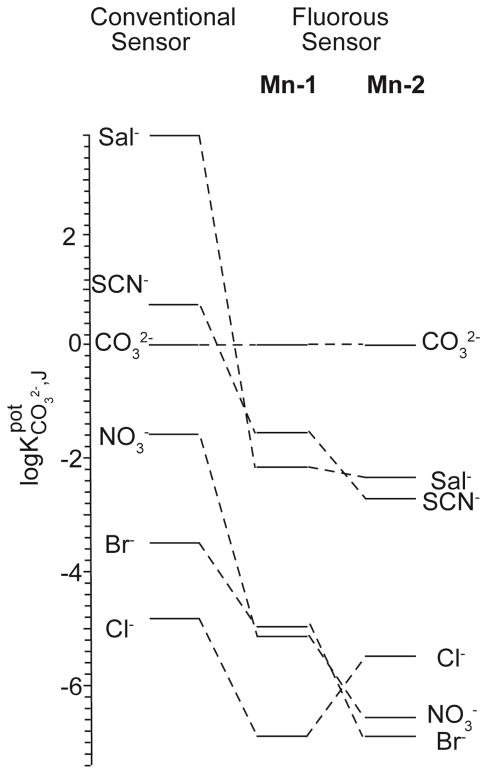
Instead, electrode membranes with 67 mol % cationic sites, 1 (No. 1, Table 1) exhibited significantly better CO₃²⁻ selectivity over SCN⁻, and are more promising for CO₃²⁻ measurements in clinical samples. Consequently, the selectivities for CO₃²⁻ over physiologically relevant anions, including Cl⁻, Sal⁻, and Br⁻ (the latter two present in the blood of patients who take certain drugs) were determined. Figure 2 compares the selectivities of the fluorous sensing membrane No. 1 based on the fluorophilic ionophore Mn-1 and cationic sits 1 with those of conventional membranes based on the ionophore heptyl 4-trifluoroacetylbenzoate, a typical example of a trifluoroacetophenone-type ionophore.¹⁴ The fluorous ISE membrane gives significant improvements in CO₃²⁻ selectivities over all interfering ions, ranging from a 30 fold improvement for Br⁻ to a 10⁶ fold improvement over salicylate (see Supporting Information Table S1 for the numerical values).¹⁴ The strong interference from the highly lipophilic anion salicylate in the case of ISEs based on conventional trifluoroacetophenone ionophores ( $log{K}_{{co}_3^{2-},{\mathit{Sal}}^{-}}^{\mathit{pot}}$ is typically in the range from +5.2 to +1.7) is one of the main limitations for its application in clinical analyzers. However, with the fluorous sensing membrane No. 1, Sal⁻ could be discriminated with a $log{K}_{{co}_3^{2-},{\mathit{Sal}}^{-}}^{\mathit{pot}}$ of −2.19. To the best of our knowledge, this is the lowest value reported to date for a carbonate selective electrode.

Figure 2.

Logarithmic representation of selectivities for CO₃²⁻ of a conventional ISE based on the ionophore heptyl 4-trifluoroacetylbenzoate¹⁴ and fluorous ISEs with 1.0 mM cationic sites 1 and 1.5 mM fluorophilic ionophore Mn-1 (center, No. 1 in Table 1), and 1.0 mM anionic sites, 2, and 4.0 mM fluorophilic ionophore Mn-2 (right, No. 6 in Table 1).

Electrode membranes doped with ionophore Mn-2 and the three different ionic site-to-ionophore ratios (electrodes No. 4 to 6, Table 1) also exhibited the theoretically expected Nernstian responses to CO₃²⁻. The membranes with ionophore Mn-2 and the anionic site-to-ionophore ratio of 1:4 (electrode No. 6) showed another tenfold increase compared to that of membranes doped with Mn-1 and the optimum ionic site-to-ionophore ratio (No. 1). Indeed, they exhibited among all electrodes tested in this study the by far best CO₃²⁻ selectivity over SCN⁻ ( $log{K}_{{co}_3^{2-},{\mathit{SCN}}^{-}}^{\mathit{pot}}=-2.78$). Therefore, selectivities for CO₃²⁻ over other interfering anions, including Cl⁻, Br⁻, and Sal⁻, were measured too. They are compared in Figure 1 to those of trifluoroacetophenone-type membranes and the fluorous sensing membrane No. 1 (see Table S1 in the Supporting Information for numerical values). There were significant improvements in CO₃²⁻ selectivities for sensors based on fluorophilic ionophore Mn-2 with the 1:4 anionic sites-to-ionophore ratio over the conventional sensors, from approximately 10 fold for Cl⁻ to 10⁶ fold for Sal⁻ ( $log{K}_{{co}_3^{2-},{\mathit{Sal}}^{-}}^{\mathit{pot}}=-2.36$). Whereas the selectivity for CO₃²⁻ over Cl⁻ was not as good as that for membrane No. 1, selectivities for CO₃²⁻ over SCN⁻, NO₃⁻, Br⁻ and Sal⁻ were further enhanced by using fluorous membrane No. 6 based on Mn-2. Since ionophore Mn-2 discriminates HO⁻ less than Mn-1, the detection limits of the electrode membranes doped with ionophore Mn-2 in Tris-H₂SO₄ solutions buffered to pH=8.75 were dominated by HO⁻ interference and were worse than the detection limits of the electrode membranes doped with Mn-1. Because CO₃²⁻ is a divalent and HO⁻ a monovalent anion, HO⁻ interference is less significant at lower pH. Therefore, if measurements were carried out in physiological samples with a pH of 7.4, the interference from OH⁻ as calculated from $log{K}_{{co}_3^{2-},{OH}^{-}}^{\mathit{pot}}=7.23$ would result in a detection limit of the No. 6 electrode membranes (doped with ionophore Mn-2) of 0.93 μM; this is well below the concentration range of CO₃²⁻ in blood samples (28 – 38 μM).⁵⁶

With the anionic site-to-ionophore ratio of 1:4, the electrodes based on ionophore Mn-3 had worse CO₃²⁻ selectivities over SCN⁻ than that the electrodes based on ionophore Mn-2 and worse CO₃²⁻ selectivities over HO⁻ than the electrodes based on ionophore Mn-1 (No. 7 in Table 1). Therefore, further experiments to determine the effect of the site-to-ionophore ratio on sensing membranes doped with ionophore Mn-3 were not performed.

Working Mechanism of Electrodes Based on Mn-1 and Mn-2: Stoichiometry and Stability of Carbonate Complexes

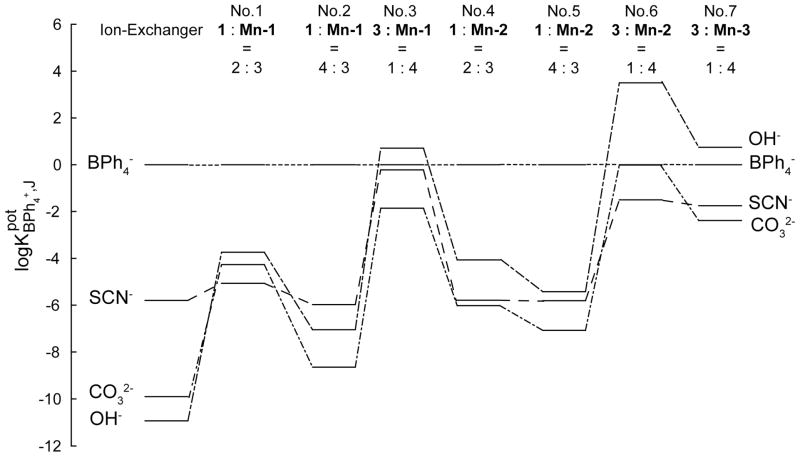
To quantitatively assess the stability and stoichiometry of the complexes between the ionophore and the primary and interfering anions, the selectivities of all fluorous ionophore-based sensing membranes relative to BPh₄⁻ (assumed to be an anion not interacting selectively with the ionophore) were compared to the selectivities of ionophore-free ion-exchanger membranes prepared by dissolving 2 mM cationic sites, 1, in perfluoroperhydrophenanthrene (Figure 3; see Supporting Information Table S2 for the numerical values). In the following, this comparison is presented for the different types of membranes along with a molecular level interpretation of the observed selectivity based on the composition of the sensing membranes when exposed to either CO₃²⁻ solutions or to solutions of interfering ions.

Figure 3.

Logarithmic representation of selectivities with respect to BPh₄⁻ of (from left to right) fluorous ion-exchanger ISEs based on 2.0 mM cationic sites, 1, and fluorous ionophore-based ISEs doped with 1 (1.0 mM) and ionophore Mn-1 (1.5 mM); 1 (2.0 mM) and Mn-1 (1.5 mM); 1 (1.0 mM) and Mn-1 (4.0 mM); 1 (1.0 mM) and ionophore Mn-2 (1.5 mM); 1 (2.0 mM) and Mn-2 (1.5 mM); 1 (1.0 mM) and Mn-2 (4.0 mM); anionic sites, 2 (1.0 mM) and Mn-3 (4.0 mM).

Note that in many cases these membranes contain primary or interfering anions in the form of more than one species. For example, some of these membranes contain more than one type of ionophore complex, and some also contain primary or interfering anions in a free form not bound to the ionophore. As it is intuitively easy to understand, the potentiometric selectivity of each membrane is limited by the anions that interact most weakly with the membrane components and can, therefore, most readily transfer from the sensing membrane into the sample solution. In some membranes, the anions that are least stabilized by interaction with membrane components are the anions not bound to ionophore. In other membranes, all the anions are bound to ionophore, but not all anions undergo the same level of stabilization in the sensing membrane because they form complexes of different stoichiometries with the ionophore.

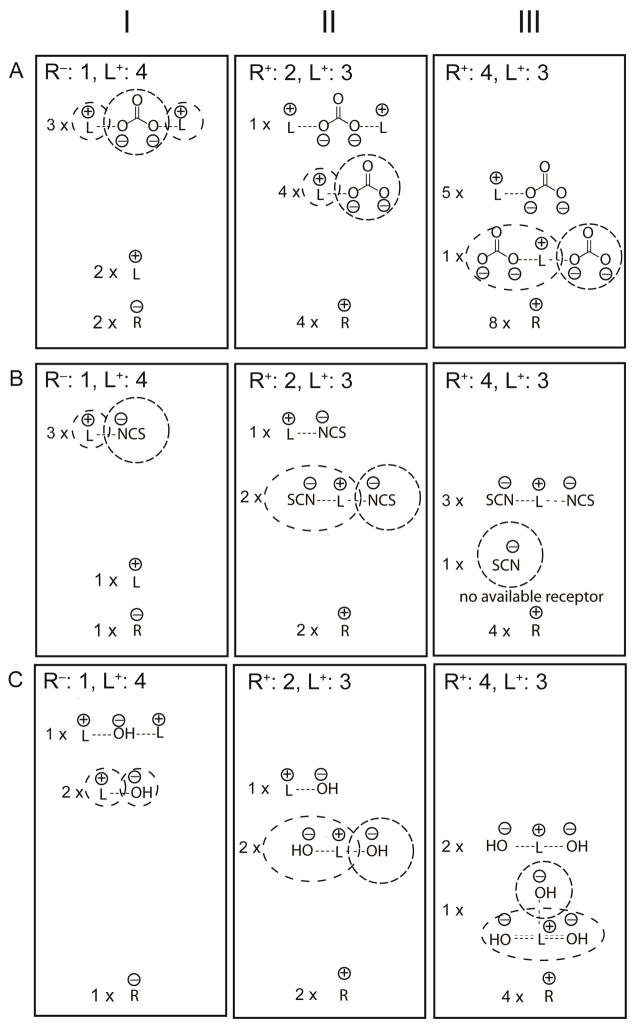
Consider first the membranes with a 4:3 cationic site-to-ionophore ratio when equilibrated with aqueous CO₃²⁻ solutions. As illustrated by the schematic on the right hand side of panel A in Figure 4 (panel A-III), in the bulk of the sensing membrane the positive charge of both the cationic sites and the ionophore needs to be balanced by an equal concentration of anion charges, which results in a large total concentration of CO₃²⁻ in these membranes. Both 1:1 and 1:2 ionophore–anion complexes are formed, and the weakest bound CO₃²⁻ is expected to be the second CO₃²⁻ in the complex [L(CO₃)₂]³⁻. Based on the concept of ionic site theory, [LCO₃]⁻ may be considered formally to be an ionophore for CO₃²⁻. Figure 3 shows that the selectivity for CO₃²⁻ over BPh₄⁻ of these membranes was slightly larger than that of the ionophore-free membranes. This confirms that [L(CO₃)₂]³⁻ complexes are indeed formed but that [LCO₃]⁻ is a weak ionophore for CO₃²⁻. Using a method first described by Pretsch and Bakker according to which the potentiometric selectivities of ionophore-doped and ionophore-free membranes for a complexing over a noncomplexing ion are used to compute the stability of the ionophore complexes,^57–59 the stabilities of the complexes between [LCO₃]⁻ and CO₃²⁻ were calculated to be 3.2×10² M⁻¹ and 4.2×10⁴ M⁻¹ for ionophores Mn-1 and Mn-2, respectively (Table 2; see Supporting Information for the derivation of the equations for the special case of the charges and stoichiometries discussed here). Given the very similar electronic environments of the [L(CO₃)₂]³⁻ complexes of Mn-1 and Mn-2, the stronger binding of the second CO₃²⁻ to Mn-2 in the latter case appears to be the result of steric hindrance from the phenyl groups of Mn-1, which interfere with the binding of two pseudo-axial CO₃²⁻ ligands.

Figure 4.

Schematic representation of the ratios of ionophore and ionophore complexes in fluorous sensing membranes with different ionic site-to-ionophore ratios for the primary ion CO₃²⁻ (A) and the interfering ions SCN⁻ (B) and HO⁻ (C). For each membrane composition, the anion stabilized the least in the sensing membrane (i.e., the most readily exchangeable anion) is circled.

Table 2.

Binding Constants for ionophores with CO₃²⁻

Ionophore	$log{K}_{L^{+}-{co}_3^{2-}}({\text{M}}^{-1})$	$log{K}_{L^{+}{co}_3^{2-}+L^{+}}({\text{M}}^{-1})$	$log{K}_{{co}_3^{2-}{L}^{+}-{co}_3^{2-}}({\text{M}}^{-1})$
Mn-1	9.8 ± 0.2	4.0 ± 0.1	2.5 ± 0.5
Mn-2	9.8 ± 0.2	5.8 ± 0.1	4.6 ± 0.3

Improved carbonate selectivity as compared to ion exchanger membranes was observed both for membranes with 2:3 cationic site-to-ionophore ratio and membranes with 1:4 anionic site-to-ionophore ratio (see Figure 3). This indicates that both [LCO₃]⁻ and [L₂CO₃] complexes can be formed. As Figure 4 illustrates, in the membranes with the cationic sites there is not enough ionophore to form [L₂CO₃] complexes only (panel A-II and A-III), but in the membranes with anionic sites (panel A-I), there is plenty of ionophore to form [L₂CO₃] complexes exclusively. The higher observed selectivities in the latter case (see Figure 3) confirm that [L₂CO₃] complexes are indeed formed. Using the numerical values of the potentiometric selectivities over BPh₄⁻, the stabilities of the complexes resulting from CO₃²⁻ binding to L⁺ and of L⁺ binding to [LCO₃]⁻ were calculated to be 6.7 × 10⁹ M⁻¹ and 1.1 × 10⁴ M⁻¹ for Mn-1, respectively, and 6.3 × 10⁹ M⁻¹ and 6.5 × 10⁵ M⁻¹ for Mn-2, respectively (Table 2; see the Supporting Information for the derivation of the binding constant equations). In comparison to membranes with ionophore Mn-1, the membranes with ionophore Mn-2 exhibited a higher CO₃²⁻ selectivity for the 1:4 anionic site-to-ionophore ratio but a lower CO₃²⁻ selectivity for the 2:3 cationic site-to-ionophore ratio. Since the selectivities of the membranes with the 1:4 anionic site-to-ionophore ratio are dominated by the 2:1 complexes, while the selectivities of the membranes with the 2:3 cationic site-to-ionophore ratio are dominated by the 1:1 complexes, the different selectivities appear to result from the smaller extent of steric hindrance in the [L₂CO₃] complexes of Mn-2. This conclusion is supported by the fact that binding of L⁺ to [LCO₃]⁻ to give [L₂CO₃] is notably stronger in the case of Mn-2 than that for Mn-1, as evidenced by the more than hundred fold larger binding constant.

Stoichiometry and Stability of Complexes with Interfering Ions

To extend the discussion of the working mechanism of these CO₃²⁻ ISEs to their response to interfering ions that have the ability to bind to the Mn(III) center of the ionophores, the case of the clinically very relevant SCN⁻ was considered first. As shown in panel B-III of Figure 4, the membranes with the 4:3 cationic site-to-ionophore ratio are predicted to contain free SCN⁻ even if it is assumed that L⁺ can bind up to two SCN⁻ ligands. Not surprisingly, this ratio of cationic sites and ionophore gave for both Mn-1- and Mn-2-doped sensing membranes the same SCN⁻ selectivities over BPh₄⁻ as it was observed for ionophore-free ion exchanger membranes (Figure 3). Moreover, there was no significant change in the selectivity for SCN⁻ over BPh₄⁻ when the concentration of cationic sites to ionophore was decreased to 2:3, which shows that [LSCN] is a poor ionophore at most, and that the membrane composition of the corresponding membranes is better represented by three equivalents of [LSCN] and two equivalents of free SCN⁻ rather than what is depicted schematically in panel B-II in Figure 4. The best selectivities for SCN⁻ over BPh₄⁻ were observed for the 1:4 anionic site-to-ionophore ratio, which is consistent with L⁺ as an ionophore forming 1:1 complexes with SCN⁻.

Most importantly, the highest selectivity for CO₃²⁻ over SCN⁻ was observed for membranes that contained ionophore Mn-1 and cationic sites in a ratio of 3:2, which coincides with the prediction from ionic site theory for an ionophore that prefers 1:1 stoichiometry.⁴⁸ This is one of the first examples that shows that existing ionic site theory needs further refinement for cases in which the ionophore may form complexes of multiple stoichiometries.

In the case of Mn-2, where binding of two ionophores to one CO₃²⁻ is slightly more favorable, the 25% of anionic sites gave among all three ionic site concentrations tested the highest selectivity for CO₃²⁻ over SCN⁻. This result is compatible with ionic site theory; when an ionophore L⁺ that can form [L₂CO₃] complexes binds one interfering monoanion (e.g., [LSCN]), the selectivity is predicted to steadily increase with the increasing concentration of anionic sites up to the onset of Donnan failure (this case is referred to as selectivity maximum of type I in ref. 48).

While the selectivities for other interfering anions, such as Cl⁻, Br⁻ and Sal⁻, followed the same trend as SCN⁻, a marked exception was observed for HO⁻. Figure 3 shows that the sensing membranes with Mn-1 or Mn-2 and a cationic site-to-ionophore ratio of 4:3 provided a much stronger response to HO⁻ in comparison to BPh₄⁻ than the ion exchanger membrane. This indicates that the ionophore can bind three equivalents of HO⁻, which may suggest a heptacoordinated Mn(III) center. Although not very common, heptacoordination for Mn(III) is known and has been observed crystallographically.^60,61 Alternatively, the third HO⁻ might attack a C=N bond or the arene ring, activated by the perfluoroalkyl groups.

Another unique feature related to the pH was the observation of super-Nernstian response slopes of −103 and −74 mV/decade in the SCN⁻ activity range from 10⁻¹ to 10^−2.5 M for sensing membranes with a 1:4 ratio of anionic sites and ionophore Mn-1 or Mn-2, respectively (see Supporting Information, Figure S2A). This may be explained by a similar type of complex stoichiometries as they have been previously reported for so-called apparently twice-Nernstian responses, i.e., the independent but simultaneous complexation of the ionophore with two different types of ions.^62,63 In the specific case here, there appears to be a range of SCN⁻ and HO⁻ concentrations in which the sensing membranes contain both ionophore complexes of 1:1 stoichiometry with SCN^−. and ionophore complexes of 2:1 stoichiometry with HO⁻ (i.e., [L₂OH]), but the membranes contain no free ionophore. Substantial lowering of the HO⁻ to SCN⁻ ratio in the aqueous sample leads to complete elimination of HO⁻ from the sensing membrane by exchange with SCN⁻ (and consequently Nernstian SCN⁻ responses), and substantial raising of the HO⁻ to SCN⁻ ratio in the sample leads to complete elimination of SCN⁻ from the sensing membrane by replacement with HO⁻ (an interpretation supported by the pH-dependent lower detection limit of the SCN⁻ response). Both effects were experimentally confirmed (see Supporting Information, Figure S2). However, the concentration range in which the SCN⁻ and OH⁻ complexes of Mn-1 and Mn-2 co-exist in membranes with a 1:4 ratio of anionic sites and ionophore is too narrow to exhibit the full twice-Nernstian response of −116 mV/decade, which explains the observed slopes of −103 and −74 mV/decade. Similar observations were reported previously for electrodes with the same site ratio and Mn(III) porphyrins.⁶⁴ Note that in the current case of Mn-1 and Mn-2, the ionophores appear to form not only 2:1 but also 1:1 complexes with HO⁻ (see Panel C of Figure 4), as indicated by the stronger preference for HO⁻ over BPh₄⁻ for the sensing membranes with the anionic site-to-ionophore ratio of 1:4 than in the case of the ion-exchanger electrodes (see Figure 3). Alternatively, the HO⁻ selectivity of these electrodes may be the result of more extended complexes of the type [L_n(OH)_n-1]⁺.

Because of such super-Nernstian responses, unbiased selectivity coefficients over the interfering ions SCN⁻, Cl⁻, Br⁻ and Sal⁻ for membranes with the anionic site-to-ionophore ratio of 1:4 were measured at pH 3 (0.5 mM H₂SO₄), where Nernstian responses were observed for those anions. In biological samples with a pH of approximately 7.4, the interference from SCN⁻, Cl⁻, Br⁻, and Sal⁻ on membranes with a 1:4 ratio of anionic sites and ionophore would be decreased by this twice-Nernstian phenomenon in comparison to pH 3. It also follows from this discussion that the selectivity for CO₃²⁻ over HO⁻ may be improved if binding of more than one Mn(III) salen to HO⁻ were prevented by some type of capping or covalent attachment of the ionophore to a polymer backbone. Similar efforts have been successful in the case of porphyrins.⁶⁵

Conclusions

This paper describes the first use of perfluoroalkylated (salen)Mn(III) complexes as ionophores for CO₃²⁻, which resulted in selectivity improvements of several orders of magnitude over previously reported ISEs. Measurements of selectivities relative to BPh₄⁻ for ionophore-based membranes with different site-to-ionophore ratios made it possible to quantify the underlying complex stoichiometries and stabilities. The improved selectivities suggest that these CO₃²⁻-selective electrodes can be used for carbonate measurements in various applications, most notably in clinical analyzers or to monitor spatial and temporal variations of carbonate and total CO₂ in the environment.^66–69 Having gained a full understanding of the fairly complicated working mechanism of these Mn(III) salenes, we now plan to optimize the detection limits of these sensors, improve their mechanical robustness by use of perfluoropolymer matrixes, and test their use in real life samples. However, the extremely selective binding of carbonate to these fluorophilic Mn(III) salen receptors should also be of considerable interest for applications outside of the field of electrochemical sensors, such as for anion separations or the sequestration of carbon dioxide.

Chart 1.

Structure Formulae of Ionophores and Other Membrane Components