Why the bigger live longer and travel farther: animals, vehicles, rivers and the winds

Abstract

Here we show that constructal-law physics unifies the design of animate and inanimate movement by requiring that larger bodies move farther, and their movement on the landscape last longer. The life span of mammals must scale as the body mass (M) raised to the power 1/4, and the distance traveled during the lifetime must increase with body size. The same size effect on life span and distance traveled holds for the other flows that move mass on earth: atmospheric and oceanic jets and plumes, river basins, animals and human operated vehicles. The physics is the same for all flow systems on the landscape: the scaling rules of “design” are expressions of the natural tendency of all flow systems to generate designs that facilitate flow access. This natural tendency is the constructal law of design and evolution in nature. Larger bodies are more efficient movers of mass on the landscape.

It is well known that larger animals live longer¹,²,³,⁴,⁵,⁶,⁷,⁸: the life span (t) increases with the body mass M roughly as the power function M^γ. For mammals, observations show that the γ values are around 0.2⁴ and 0.22⁷, with considerable scatter around the t ~ M^γ curve. This information is empirical and awaits a purely theoretical prediction.

The theoretical framework in this paper is based on the view that animals are not the only moving bodies for which the larger size translates into a longer life span. Inanimate streams such as rivers and atmospheric currents persist longer in time when they are larger. They also travel farther during their lifetimes, and this unveils a second aspect of animal design that is not nearly as well known as the body size effect on life span: bigger animals travel farther during their lives than smaller animals.

This unifying view of the flow of mass on the landscape (animate and inanimate) is expressed by the constructal law of design in nature⁹,¹⁰: “For a finite-size flow system to persist in time (to live) its configuration must evolve in such a way that it provides easier access to its currents”¹¹. According to the constructal law, “life” is defined as a flow system that morphs freely over time toward easier movement and access to the available space. This definition of life makes the life span vs. body size a problem of physics, i.e. of everything, not just of biology. In the next four sections, we explore this idea as a sequence of four categories of mass flows, two inanimate and two animate: air jets, rivers, human vehicles and animals.

Results

Jets and plumes

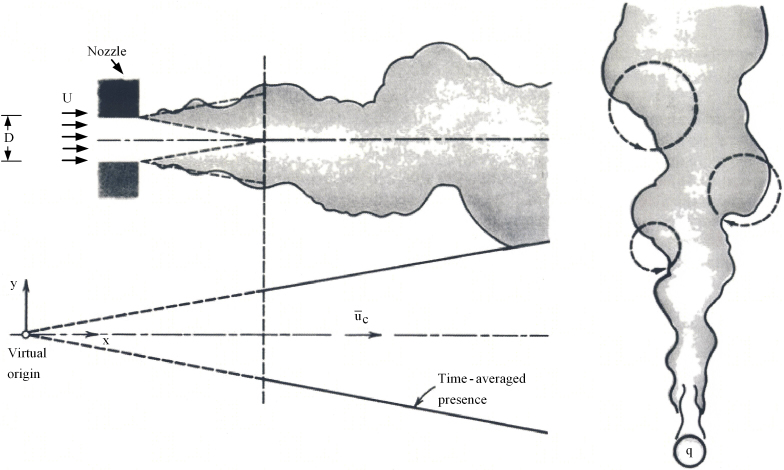
Atmospheric and oceanic currents can be modeled as turbulent fluid-in-fluid jets and plumes (Fig. 1). Here we use the simplest description, which is in terms of time-averaging the turbulent flow field¹². We focus on the life and travel of a fluid packet that issues from the nozzle of the jet. The fluid mechanics of turbulent jets issuing from round nozzles is well established¹², cf. Fig. 1. The time-averaged presence of this turbulent flow is a conical volume with an angle of roughly 20^°.

Figure 1. Turbulent jets and plumes: the time averaged flow field occupies a cone with fixed angle¹².

The time-averaged turbulent jet has two dimensions, the nozzle diameter D, and the mean fluid velocity at the nozzle, U. The following analysis refers to the self-similar region, which resides downstream of 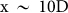, where x is measured from the virtual origin of the cone. The time-averaged fluid speed on the centerline 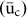decreases downstream,

We define the travel of the jet as the distance x = L where 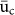has become so small that 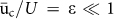where is a small constant fixed for the purpose of comparison, for example 0.01. It follows that the reach (the territory) of this live system is

The time of travel to the distance L is obtained by integrating 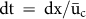from the nozzle 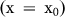to x = L, which yields 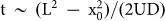. Because 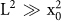when 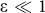, the life span of the moving fluid packet is of order

In summary, larger turbulent jets travel farther and last longer, Eqs. (2) and (3). These two conclusions do not change if instead of a turbulent jet with round cross section we consider the scales of a jet with flat cross section. Such a jet develops downstream from a slit-shaped nozzle of spacing D, and it has the same time-averaged presence as in Fig. 1. In this case, the jet mixing region is two-dimensional—a wedge with an angle of approximately 20^°. Repeating the preceding steps, we can show (Supplementary Information A) that the territory and life span of two-dimensional turbulent jets are essentially the same as in Eqs. (2) and (3). Turbulent plumes are jets driven by the force of buoyancy. The two conclusions reached for jets also apply to plumes: larger plumes have longer life spans and travel farther (Supplementary Information B).

The fact that these conclusions emerge analytically is the result of the time-averaging of the real flow field, which is turbulent, with local time-dependent features (large and small) that deviate from the pattern, which is time-averaged. The coexistence of the pattern with the deviations (the outliers) is an integral part of the pattern and diversity that characterizes design in nature⁹,¹⁰,¹¹. The coexistence of pattern with diversity is a feature of all the flow systems analyzed next: rivers, vehicles and animals.

Rivers

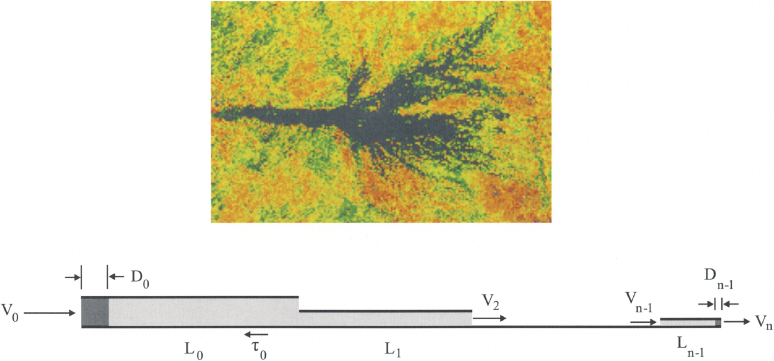
Rivers are analogous to turbulent jets, except that they are fluid-in-solid streams, or fluid in erodible solid that stabilizes the tendency of every turbulent stream to become unstable, with bulging elbows that become eddies. The stabilized tendency to develop elbows is visible as meanders, which are not static but morph and move along the stream. This analogy also means that the turbulent jet (Fig. 1) is like a river delta in which all its channels have become so unstable that they generate a hierarchy of eddies (few large and many small) similar to the hierarchy of the river channels themselves (Fig. 2).

Figure 2. The spreading of a river on an area is analogous to the spreading of a jet into a fluid reservoir (Fig. 1).

The upper image is the Okavango delta (NASA photo).

In rivers, the flow of water is driven by gravity along sloped channels. Here we rely on a simpler model that brings the description of river flow closer to that of jet flow. The main feature of the river channel model is that it is horizontal: the water packet flows because of its kinetic energy, which decreases because of friction against the river bed. This flow model is illustrated by the Okavango Delta, where the river arrives from Angola and invades a finite-size area of the Kalahari desert (Fig. 2). The river has the speed V₀, length L₀, and transversal length scale D₀, which characterizes the width and depth of its cross-section. A body of water of mass 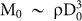enters the big channel with the kinetic energy 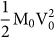and exits with a lower kinetic energy, 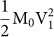. The decrease equals the work dissipated by friction against the river bed, 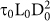, where the bed shear stress is 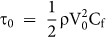, the friction force on M₀ is 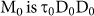, and C_f is constant and of order 10⁻². From this equality follows the decrease in speed along the channel,

Mass is conserved, because downstream of the big channel (L₀, D₀), the water mass M₀ splits into several smaller masses of size M₁, each traveling along channels of length L₁ and thickness D₁. The decrease in speed along L₁ is similar to Eq. (4), namely V₂/V₁ = (1 − C_fL₁/D₁)^1/2. This scenario is repeated n times, where n is the number of branching levels. The last generation of channels has the length scale L_n–1, thickness D_n–1, inlet speed V_n–1, and outlet speed V_n, which are related by

The reach of the river tree is the location where 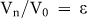, where for the purpose of comparing large and small rivers is fixed, and 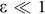. In view of Eqs. (4) and (5), this definition becomes

The channel sizes D_i are related (approximately) to the sizes of their neighbors 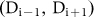but the exact relation is not important. We get a sense of it by noting Horton's scaling rule of stream numbers¹³ for river basins, which states that the number of daughter channels corresponding to one mother channel is between 3 and 5. This means that M_i/M_i+1 is between 3 and 5, and that D_i/D_i+1 is between 3^1/3 and 5^1/3, the average of which is 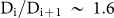For the ratio L_i/L_i+1, we note Horton's scaling rule of stream lengths, which states that L_i/L_i+1 should take values in the range 1.5–3.5. For simplicity, we set 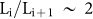and find that Eq. (6) becomes

In the limit 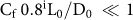, this expression yields 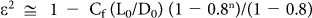and, when n ≫ 1 and ε ≪ 1,

The total length traveled by the water packet (originally as M₀, finally as M_n−1) is 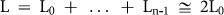. After combining this with Eq. (8), we find that a bigger river (D₀) flows farther (L).

The second conclusion is about the life span of the water packet,

in which we used the approximation 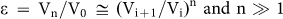Combining Eqs. (10) and (9), we find that bigger rivers (D₀) live longer,

The two conclusions reached for rivers are in complete accord with what we found for turbulent jets. Equations (9) and (11) are nearly the same as Eqs. (2) and (3). They represent the pattern that exists hand-in-glove with individual cases that deviate locally or temporarily from the pattern.

Vehicles

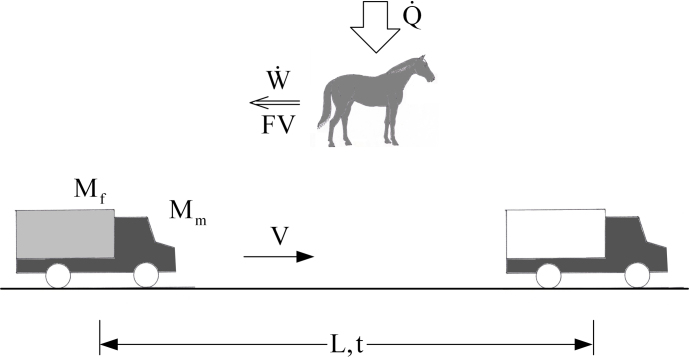
Here we predict the same trends for human vehicles. We use the model shown in Fig. 3, which is analogous to Fig. 2. The vehicle travels the distance L, while consuming the amount of fuel M_f. The vehicle mass M has two main components, the fuel mass M_f, and the motor vehicle M_m.

Figure 3. The spreading of the mass of vehicles and animals is completely analogous to the flow of water in river channels.

The burning of M_f delivers the heat input Q = M_fH to the motor, where H is the heating value of the fuel. The work produced from Q is destroyed during the L travel, namely 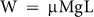, where 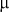is an effective friction coefficient, and Mg is the weight of the loaded vehicle. This W formula holds (with different 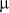values) for all modes of transportation: land, sea and air⁹,¹⁰.

The energy conversion efficiency of the vehicle 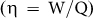exhibits a size effect that is valid for all power generators and power users: larger machines are more efficient than smaller machines because they operate with less friction (with wider passages for fluid flow) and less heat transfer irreversibility (with larger surfaces for heat transfer)¹⁴. This effect is expressed as (cf. Supplementary Information C)

where C₁ and α are constants, and 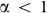, cf. Table 1. Combining the Q, W and 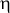expressions, we find that the total movement of mass on the landscape (ML) scales as

Because of the total mass constraint M = M_m + M_f, the product 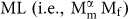is maximal when 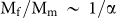, constant.

Table 1. The larger move farther and live longer: the effect of size on the distance of movement (L) and life span (t).

	L	t
Round jet, flat jet	D	D
Rivers	D0	D0
Vehicles	Mα,	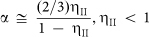Mα – β,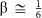
Animals	M5/12	M1/4

In conclusion, there must be a proportionality between the size of the motor vehicle and the size of the fuel used by the vehicle. This prediction is supported by all transportation systems (and animal designs), which have evolved such that larger fuel loads belong on larger vehicles. Both M_m and M_f are represented by the scale of their sum, which is M, and Eq. (13) becomes

where the group containing 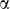is a constant smaller than 1 (Supplementary Information C). The range of the vehicle (L) varies in proportion with 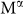: larger vehicles travel farther, and cover greater territories.

The life span of vehicle travel is t ~ L/V, where L is given by Eq. (14) and the vehicle speed tends to be greater when the vehicle is larger. For example, the speed data for aircraft designs over the M range 10³ – 10⁶ kg fall in the vicinity of the speed-mass scaling for all animal fliers, 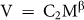, where 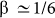(cf. Ref. 15). For the life span we obtain

Because 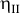is in the range 0.3–0.4¹⁶, the exponent () is in the range 0.3–0.45. In conclusion, larger vehicles also have longer life spans in their movement on the surface of the earth. This is the theoretical trend (the pattern), and its prediction invites future statistical studies of the persistence (life time and travel) of vehicles of all types and sizes on the landscape.

Animals

The size effect that unites the dominant movers of mass on earth (Figs. 1–3) also unites the minor mass movers (animals). This unification is transparent because the analysis constructed for vehicles applies unchanged to animals of all kinds (land, air, water).

Viewed as vehicles with motors, larger animals must have higher thermodynamic efficiencies. With reference to Fig. 3, the animal vehicle is analyzed on a per unit of time basis, with the heat input rate 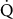(watts) and the power output 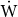(watts). The heat input 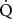is proportional to the metabolic rate, which is predictable to be proportional to M^{3/4 8},¹⁷. The power output 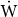is equal to the horizontal force F times the speed V. The force F scales as the body weight, and is proportional to M. The speed scales as M^1/6 for animal locomotion in all media¹⁸, although many outliers exist (e.g., turtles, human) that deviate from this and other scaling laws, for various reasons: habitat, body armor, brain size, etc. The scaling law refers to the trend in the broad sense, in the unifying sense, and the best illustration of it is that it unites the animal speed-mass scaling with the speed-mass scaling of the evolution of sports (running, swimming)¹⁹. It follows that the power output (FV) scales as M raised to the power 1 + 1/6 = 7/6.

The efficiency of the animal as a vehicle for moving animal mass is the ratio 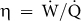and, according to the proportionalities 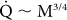and 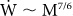, the efficiency 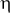increases with body mass as M^5/12. This conclusion is consistent with Eq. (12), and now the exponent α has the value 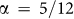which is in the same range as the α values obtained for vehicles.

The analysis following Eq. (12) continues to apply: an animal moves motor mass (M_m) and food mass (M_f) to a distance L during the lifetime t. The analysis shows that M_m ~ M_f ~ M, and this leads to Eq. (14) and the conclusion that the range of the animal movement L increases as M^α, which means that the lifetime travel scales as L ~ M^5/12. There was no theory of lifetime animal travel before the present theory, and this is why the biology literature does not offer an empirical correlation between L and M. Yet, the increase in L with body size is supported by data reported in the literature²⁰,²¹,²². One example²⁰ is the study of the travel of three types of monkeys in Kenya (small vervet, large vervet, patas), which showed that the individual travel during 30 minutes was significantly greater in the larger animals, in this sequence: small vervet, large vervet and patas.

The life span of animal mass movement to the distance L scales as t ~ L/V, where V increases as M^β, with β = 1/6. This leads to the conclusion reached earlier in Eq. (15), which is that the lifetime of this movement (t) increases M^{α – β}, where the exponent is

In conclusion, the observed statistical proportionality⁴,⁷ between lifetime and M^γ (with 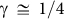) is predicted from the constructal-law physics of animal mass flow on the landscape. As in the preceding classes of inanimate and animate systems (jets, rivers, vehicles), the predicted scaling t ~ M^1/4 unifies the pattern in the broad sense, with diversity (deviations, outliers) accompanying the pattern.

Discussion

In this paper we made three theoretical advances. First, we constructed a theoretical framework in which we unified the movement of inanimate mass flow (fluid jets, rivers) with the movement of animate mass flows (human transportation, animals). This unifying theory is based on the constructal law⁹,¹⁰,¹¹ and shows that inanimate and animate mass flows exhibit the same size effect on life span and territory covered by movement: larger bodies move to greater distances during longer life times.

Second, we relied on this unifying theory to predict the size effect on animal life span, namely t ~ M^1/4, Eq. (16). We showed that this scaling has the same constructal-law origin as the speed-mass scaling of all locomotion (land, sea, air, sports)¹⁵,¹⁶,¹⁸,¹⁹.

Third, we predicted that larger bodies should cover larger distances during lifetime. Although obvious intuitively, the size effect on distance traveled is not documented and correlated in biology. The present theory should stimulate the collection and correlation of such data.

The predicted effect of size on distance traveled and lifetime is summarized in Table 1 for the inanimate and animate mass flow systems considered. Most interesting is that the size effect is essentially the same in inanimate and animate flow systems. For example, the nozzle size for the jet (D) is the length scale of the fluid packet that issues from the nozzle. The mass of this packet is M ~ ρD³, and this means that in Table 1 we can replace D (and D₀) with M^1/3. In this way, we obtain a table in which the size effect on travel (L) and life time (t) unites the inanimate with the animate with the same firmness as the unification of all the other phenomena of design in nature⁹,¹⁰,¹⁶.

Supplementary Material

Supplementary Information

Supplementary Information