Explaining differences in the lifespan and replicative capacity of cells: a general model and comparative analysis of vertebrates

Abstract

A better understanding of the factors that govern individual cell lifespan and the replicative capacity of cells (i.e. Hayflick's limit) is important for addressing disease progression and ageing. Estimates of cell lifespan in vivo and the replicative capacity of cell lines in culture vary substantially both within and across species, but the underlying reasons for this variability remain unclear. Here, we address this issue by presenting a quantitative model of cell lifespan and cell replicative capacity. The model is based on the relationship between cell mortality and metabolic rate, which is supported with data for different cell types from ectotherms and endotherms. These data indicate that much of the observed variation in cell lifespan and cell replicative capacity is explained by differences in cellular metabolic rate, and thus by the three primary factors that control metabolic rate: organism size, organism temperature and cell size. Individual cell lifespan increases as a power law with both body mass and cell mass, and decreases exponentially with increasing temperature. The replicative capacity of cells also increases with body mass, but is independent of temperature. These results provide a point of departure for future comparative studies of cell lifespan and replicative capacity in the laboratory and in the field.

1. Introduction

The processes governing the birth and death of cells are fundamental to survival, growth and reproduction in multi-cellular organisms [1]. These processes have a major impact on both the progression of disease and the decline in health and performance with age [2,3]. Thus, a better understanding of the processes that govern cell birth and death is important for addressing a multitude of public health challenges [4,5].

To date, a great deal of research in this area has focused on examining the lifespan of individual cells in vivo, or the replicative lifespan of cell lines in culture, but no clear picture has emerged regarding the factors that control cell birth or death rates. Substantial heterogeneity has been observed for both cell lifespan and the replicative lifespan of cell lines. In vivo, individual cell lifespan varies from hours to years both across different cell types within a species [6] and for the same cell type across species [7,8]. Also, it is not at all clear how the processes thought to control cell death (e.g. apoptosis, accrual of cellular damage) may act to explain these vast differences in times [9]. In culture, the replicative capacity of cell lines (i.e. the number of mitotic events in the lifetime of a dividing cell), known as Hayflick's limit [10], is also unpredictable [1]. While most human cell lines have a finite replicative capacity, under certain conditions the capacity of lines from other species appear to be immortal [11].

However, in recent years, some intriguing observations on cell lifespan and cell replicative capacity have emerged that hint at the underlying causes of the heterogeneity in these measures. Across species, for a given cell type, both cell lifespan and cell replicative capacity have been shown to correlate strongly with body size and lifespan in mammals [12,13]. Within species, both have been shown to correlate with factors, such as donor age (i.e. age of subject at time of cell harvest [14]). Given the well-established relationships between metabolism, lifespan, temperature and body size, this has raised questions about the possible role of metabolism in controlling cell death rates [15,16]. In particular, the free radical theory of ageing [17] is often cited as a possible explanation for these observations [18–22]. Still, the processes that underlie these patterns remain unclear, in part because non-mammals (e.g. ectotherms) do not appear to conform to otherwise general patterns [23].

Here we develop and test a model that aims to explain heterogeneity in individual cell lifespan and the replicative capacity of cells for both ectotherms and endotherms. The model is based on a hypothesis regarding the relationship between the rate at which a cell uses energy (i.e. cellular metabolic rate) and the lifespan of cells or cell lines. We begin by deriving the relationship between cellular metabolic rate, organismal size, organismal temperature and individual cell size. We then relate cell lifespan and the replicative capacity of cells to cellular metabolic rate. The quantitative model yields a series of first-order predictions regarding how cell lifespan and cell replicative capacity should vary with cell size, organism size and organism temperature both within and across species.

We evaluate these predictions by performing broad-scale comparative analyses of cell lifespan and cell replicative capacity using data from a diversity of ectotherms and endotherms. Together, the model and analyses provide a step towards a more synthetic understanding of the factors that govern the birth and death of cells in multi-cellular organisms.

2. Model development and predictions

(a). Cellular metabolic rate

We first consider the metabolic rate of a single cell as a function of three factors: organism body mass, organism temperature and cell mass. With respect to the first two, previous work has shown that the mass-specific metabolic rate of an organism (B/M) can be described as a function of organism mass (M) and body temperature (T) as

2.1

where b₀ is a normalization constant in units of power/mass^3/4 [24]. Equation (2.1) describes how the metabolic rate of a given mass of tissue varies as a power law with body mass (M^−1/4) and exponentially (e^−E/kT) with temperature. The temperature term is defined such that E represents the average activation energy of the respiratory complex, k is Boltzmann's constant (1.381 × 10⁻²³ J K⁻¹, or 8.62 × 10⁻⁵eV K⁻¹) and T is absolute temperature in Kelvin (K). We then extend equation (2.1) to describe the metabolic rate for a single cell (B_c) with mass m_c as

2.1

or in terms of body size and temperature as

2.2

Equation (2.2) is derived from equation (2.1) by assuming the normalization constant b₀ decreases with increasing cell size as 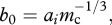where a_i may vary depending on cell type, i. This assumption is based on the observation that the mass-specific metabolic rate of individual cells is limited by diffusion across available surface area such that cellular metabolic rate increases with cell mass as m_c^2/3 [25–29].

(b). Cell lifespan

To address cell lifespan, we begin with equation (2.1), which has been used previously to predict the lifespan of species under the simplifying assumption that the lifetime mass-specific energy use of a species is constant [30]. This assumption, which follows from Pearl's ‘rate of living’ hypothesis and the work of others [31,32], implies that whole organism lifespan (LS_o) is inversely proportional to mass-specific metabolic rate such that

2.3

Data from diverse invertebrate and vertebrate animals, as well as plants, are consistent with equation (2.3) [30]. These data support the two main predictions from equation (2.3), namely that on average (i) the lifespan of species increases by about 2.5-fold for every 10°C decrease in temperature, and (ii) lifespan increases about one order of magnitude for every four orders of magnitude increase in body mass. We can apply equation (2.3) to address cell lifespan in vivo by assuming that the rate of living theory applies not only to whole organisms, but also to individual cells. As such, the lifespan of a cell is related to body size, metabolic rate, temperature and cell size as

2.4

(c). Cell replicative capacity

To address cell replicative capacity, we use equation (2.2) and add three additional simplifying assumptions. First, we assume that cellular metabolic rate in culture is independent of species' body size, but that it shows the cell size and temperature dependence described by equation (2.2) (i.e. B_c ∝ m_c^2/3 e^−E/kT). Second, we assume that the cell cycle time of cells in culture (G) is inversely proportional to mass-specific cellular metabolic rate such that G ∝ 1/(m_c^−1/3 e^−E/kT) [33]. Third, we assume that the replicative age of a cell line (t), defined as the total time from birth to senescence of cells in culture, scales with body mass and temperature as t ∝ M^1/4 e^E/kT m_c^1/3. In other words, we assume that, unlike the metabolic rate of cells in culture [34], the replicative age of a cell line is fixed for a given cell size, based on the body mass of the species from which it originated. This latter assumption, while perhaps not intuitive, is supported by recent data from mammalian fibroblasts [13]. However, replicative age is still expected to be temperature-dependent given the effects of temperature on biochemical kinetics as described by equation (2.2). Together, these assumptions imply that the replicative capacity of a cell line (N) can be described by the following formula:

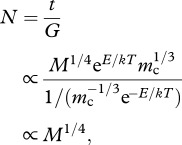2.5

where t is replicative age and G is cell cycle time.

(d). Model predictions

Equations (2.3)–(2.5) provide four testable predictions regarding cell lifespan in vivo and cell replicative capacity in culture based on their proposed relationships to cellular metabolic rate. First, equation (2.3) predicts that the natural logarithm of temperature-corrected cell lifespan should scale linearly with the natural logarithm of body mass raised to the one-fourth power. Second, equation (2.3) predicts that the natural logarithm of body mass-corrected cell lifespan should be a linear function of inverse absolute temperature with a slope of 0.65. Third, equation (2.4) predicts that, after normalizing for differences in body mass and temperature, the natural logarithm of cell lifespan should increase linearly with the natural logarithm of cell mass raised to the one-third power. Finally, equation (2.5) predicts that the natural logarithm of cell replicative capacity should scale linearly with the natural logarithm of body mass raised to the one-quarter power.

3. Material and methods

We evaluated the body mass and temperature dependence of cell lifespan in vivo (predictions 1 and 2) using published data on average red blood cell lifespan, as this is the most commonly measured cell type. In most studies, average lifespan was reported as the time at which 50 per cent of labelled cells had expired, or the mean of all measures. In cases where only maximum lifespan was reported, we converted maximum lifespan to average lifespan by multiplying by ln(2), assuming exponential mortality. This dataset consisted of 49 species ranging in size from 7.0 × 10⁻³ kg (Taricha granulosa) to 486 kg (Equus caballus), and in temperature from 12°C (Oncorhynchus mykiss) to 43.5°C (Passer domesticus; electronic supplementary material, appendix S1). The dataset included 21 mammal, seven bird, three reptile, five fish and three amphibian species. Adult body sizes for this and all subsequent analyses were taken from the study or, when not reported, from an independent source. Temperatures were estimated based on the average environmental temperature cited in the study for ectotherms, and on published estimates of body temperature for endotherms.

We evaluated model predictions on the cell size dependence of cell lifespan (prediction 3) by combining the red blood cell data for which we had cell sizes with additional data for different cell types with greater variation in size and lifespan. While in vivo estimates of cell lifespan for other cell types were limited, we collected all available data (to the best of our knowledge) in which modern labelling methods were used. This dataset was composed of several different cell types, which varied in size by over three orders of magnitude. Cell mass was calculated from estimates of cell volume by assuming the density of water. Cell volumes, when not reported, were estimated based on the linear dimension(s) reported assuming that cells are spherical or spheroid in shape.

Finally, we tested the predictions regarding cell replicative capacity by compiling all available estimates, to the best of our knowledge, from both ectotherms and endotherms. The dataset of 18 species, which consisted of endotherms and ectotherms, was largely composed of muscle cells that were harvested from adults. All model predictions were evaluated by performing ordinary least-squares regression in R.

4. Results and discussion

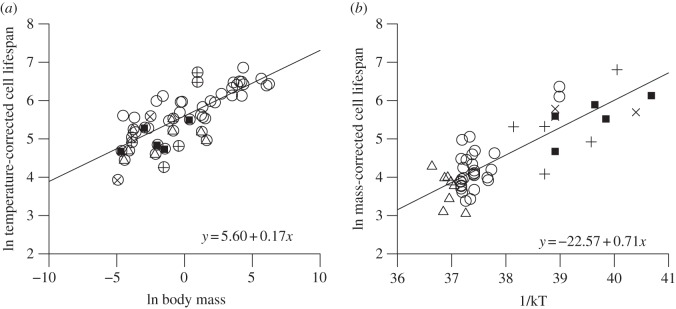
The predicted relationships between red blood cell lifespan, body mass and temperature were largely supported by the data. The natural logarithm of temperature-corrected cell lifespan was linearly related to the natural logarithm of body mass with a slope of 0.17 (figure 1a; 95% CI: 0.13–0.22; n = 49; r² = 0.58; p < 0.0001), though the slope was significantly different from the predicted slope of one-quarter. Consistent with prediction 2, the natural logarithm of mass-corrected cell lifespan was negatively related to inverse absolute temperature, with a slope of 0.71 (figure 1b; 95% CI: 0.57–0.86; n = 49; r² = 0.67; p < 0.0001), which was not significantly different than the predicted value of 0.65. This indicates that, all else being equal, cell lifespan decreases about 2.5-fold for every 10°C increase in body temperature.

Figure 1.

The body mass and temperature dependence of red blood cell lifespan in vivo. (a) The natural logarithm of temperature-corrected red blood cell lifespan versus the natural logarithm of body mass. (b) The natural logarithm of mass-corrected red blood cell lifespan versus inverse absolute temperature. Plots were body mass- and temperature-corrected based on equation (2.3). Circles, mammals; triangles, birds; plus symbols, reptiles; cross symbols, amphibians; squares, fishes.

The predicted relationship of cell lifespan with cell mass was also supported by the data. Consistent with prediction 3, the natural logarithm of body mass- and temperature-corrected cell lifespan was linearly related to cell mass, with a slope of 0.34 (figure 2; 95% CI: 0.26–0.42; n = 52; r² = 0.58; p < 0.0001), which was statistically indistinguishable from the predicted value of one-third. The lifespan of the human neutrophil was an outlier, perhaps due to the greater free radical production in this cell type [35], though it had little effect on the observed slope. Note, too, that no relationship with cell size was observed among red blood cells alone—perhaps due in part to the relatively limited range of red blood cell sizes.

Figure 2.

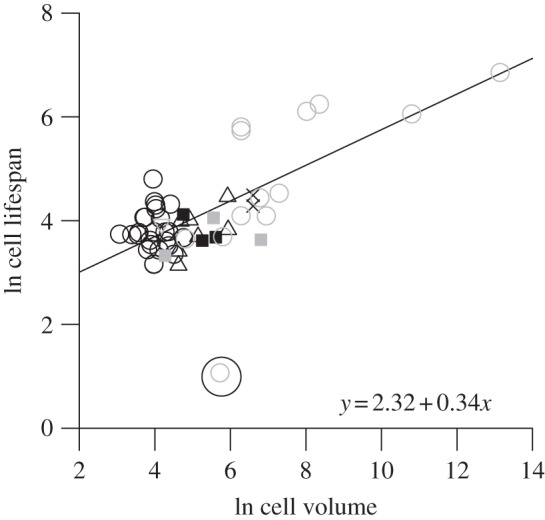
The cell size dependence of cell lifespan in vivo, after correcting for the effects of body size and temperature based on equation (2.4). Black symbols represent red blood cells, whereas grey symbols represent other blood cell types. The circled point represents the human neutrophil, an outlier that was not included in the regression analysis. Circles, mammals; triangles, birds; plus symbols, reptiles; cross symbols, amphibians; squares, fishes; inverted triangles, invertebrates.

Finally, the predicted relationship between cell replicative capacity and organism mass was supported by the data. Consistent with prediction 4, the natural logarithm of cell replicative capacity was linearly related to the natural logarithm of body mass with a slope of 0.18 (figure 3; 95% CI: 0.10–0.26; n = 19; r² = 0.52; p < 0.001), a value that was statistically indistinguishable from the predicted value of 0.25.

Figure 3.

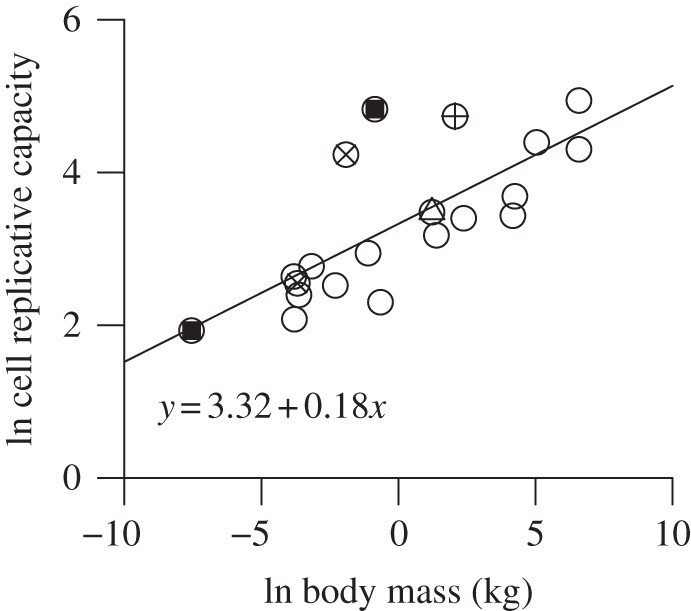
The natural logarithm of cell replicative capacity in culture as a function of the natural logarithm of body mass. Circles, mammals; triangles, birds; plus symbols, reptiles; cross symbols, amphibians; squares, fishes.

Overall, the results presented in figures 1–3 point to a primary role of cell metabolic rate in controlling cell lifespan and replicative capacity. This control is almost certainly indirect, through the effects of metabolic rate on rates of apoptosis and/or free radical production and telomere length. Previous work has shown that organisms with higher metabolic rates incur higher rates of cell damage [36–40].

While a primary role for metabolic rate has long been proposed to control cell lifespan, less appreciated is what this implies about the relationship between cell lifespan and the factors that govern cellular metabolic rate, particularly body temperature and cell size. Our results indicate that, all else being equal, cell lifespan decreases about 2.5-fold for every 10°C increase in body temperature. This temperature dependence appears to explain the considerable differences in red blood cell lifespan between ectotherms and endotherms. Our results also indicate that there is a nonlinear dependence of cell lifespan on cell size, as shown in figure 2. This appears to explain why certain cells in humans, such as hepatocytes and neurons, live much longer than other cell types that are smaller, such as lymphocytes. These results also suggest that the lifetime energy budget of a cell is roughly constant, much as for whole organisms. In other words, Pearl's rate of living hypothesis may apply to individual cells in vivo. This does not, however, appear to be the case for cells in culture. Instead, the model and data suggest that cell replicative capacity and metabolic rate are independent of one another in culture—cell replicative capacity maintains body size dependence, whereas cell metabolic rate does not.

Still, while the proposed model and results are generally in agreement, further research is clearly needed on this topic. It remains unclear how generally applicable this model is to the large variety of cell types that are present in vertebrates given available data. For example, multi-nucleated cells, such as those found in muscles, may deviate from model predictions. Moreover, our analysis was largely based on red blood cells, which are non-dividing cells, and enucleated in the case of mammals.

Nevertheless, the theory and data presented here provide a point of departure for more comparative analyses on cell lifespan and cell replicative capacity. Our hope is that the deliberately simplified models presented here could be fruitfully combined with comparative work on the relevant cellular-level mechanisms to develop a truly general theory of the lifespan of cells and cell lines.