Fluid Circulation Determined in the Isolated Bovine Lens

Abstract

Purpose.

In 1997, a theoretical model was developed that predicted the existence of an internal, Na⁺-driven fluid circulation from the poles to the equator of the lens. In the present work, we demonstrate with a novel system that fluid movement can be measured across the polar and equatorial surface areas of isolated cow lenses. We have also determined the effects of ouabain and reduced bath [Na⁺].

Methods.

Lenses were isolated in a chamber with three compartments separated by two thin O-rings. Each compartment, anterior (A), equatorial (E), and posterior (P), was connected to a vertical capillary graduated in 0.25 μL. Capillary levels were read every 15 minutes. The protocols consisted of 2 hours in either open circuit or short circuit. The effects of ouabain and low-Na⁺ solutions were determined under open circuit.

Results.

In 21 experiments, the E capillary increased at a mean rate of 0.060 μL/min while the A and P levels decreased at rates of 0.044 and 0.037 μL/min, respectively, closely accounting for the increase in E. The first-hour flows under short circuit were approximately 40% larger than those in open-circuit conditions. The first-hour flows were always larger than those during the second hour. Preincubation of lenses with either ouabain or low-[Na⁺] solutions resulted in reduced rates of fluid transport. When KCl was used to replace NaCl, a transitory stimulation of fluid transport occurred.

Conclusions.

These experiments support that a fluid circulation consistent with the 1997 model is physiologically active.

Few studies have attempted to directly detect fluid transport by the lens. We herein demonstrate a novel system with which fluid movement can be measured across three separate lens surface areas (anterior, equator, and posterior) of isolated bovine lenses under both open- and short-circuited conditions.

Introduction

In 1997, Mathias et al. reviewed the physiological properties of the normal lens and proposed theoretical ideas that later became known as the lens fluid circulation model (FCM).^1–3 They suggested, based primarily upon the existence of electrolyte currents measured by Patterson and coworkers around the surface of the lens,^4–7 that such currents represent the external portion of a circulating ionic current that drives an internal microcirculatory system. This microcirculation was envisaged to maintain fiber cell homeostasis and therefore the transparency of the avascular lens. Recently, the validity of the FCM has been challenged on the basis that the mature fiber cells are relatively inactive metabolically and thus the lens may not require an internal convective fluid flow to deliver nutrients to, and remove metabolic waste from, the deep fiber cells within the organ.⁸ Proponents of this view suggest that simple diffusion to and from the mature fibers might be sufficient to maintain these cells given their low metabolism, and emphasize that fluid circulation coupled to circulating currents exists only in theory because no studies have demonstrated a linkage between these phenomena.

Our laboratories have measured both the circulating currents around the rabbit lens⁹ and, in preliminary work, the net movements of fluid into the lens across the anterior polar region and out across the equatorial surface and posterior surfaces in cow lenses (Candia OA, Gerometta R. IOVS 2003;44:ARVO E-Abstract 3455).¹⁰ The latter observations were in general agreement with the FCM, which proposes that Na⁺ and fluid entry into the polar regions is balanced by Na⁺ and fluid efflux at the lens equator, maintaining steady-state electrolyte levels and volume. Few studies have attempted to directly detect fluid transport by the lens. Fowlks¹¹ and Fischbarg et al.¹² obtained antithetical results that were contrary to our initial findings (Candia OA, Gerometta R. IOVS 2003;44:ARVO E-Abstract 3455). We have now obtained additional data from recently performed experiments (on cow lenses) that corroborate, and substantiate, our earlier results. Our new data, presented herein, include the effects of short-circuiting and ion substitutions on volumetrically measured lens fluid movement exhibited by cow lenses isolated in a novel three-compartment chamber. The results of these experiments provide additional support for the concept that a fluid circulation, consistent with the Mathias FCM, is physiologically active.

Materials and Methods

Procurement and Isolation of Cow Lenses

Experiments using cow eyes were done at the laboratory of Rosana Gerometta at the School of Medicine of Universidad Nacional Del Nordeste (UNNE) in Corrientes, Argentina, because of the proximity of the municipal slaughterhouse to the school. Enucleated cow eyes were transported on ice to UNNE within 0.5 to 1 hour postmortem, a requirement for these physiological experiments.

Lenses were dissected free of the eye by a posterior approach, which entailed placement of the eye globes anterior side down on gauze, an incision made near the optic nerve, and cutting of the sclera from the posterior surface of the eye to nearly the corneal limbus. Such cutting was repeated (four to six cuts) until the sclera could be folded forward, exposing the posterior aspect of the lens with adhering vitreous. The vitreous was blotted with filter paper while being trimmed from the lens until it was entirely removed. The lens was then dissected from the ciliary body, leaving a thin ring of zonular fibers around the equator that served as a reference. Care was taken to ensure that any metal instruments did not touch the lens. Isolated lenses were transferred using plastic-covered, curved forceps and placed in a physiological bathing medium, described below.

Mounting of Lenses in Chamber for Volumetric Measurements of Fluid Movement

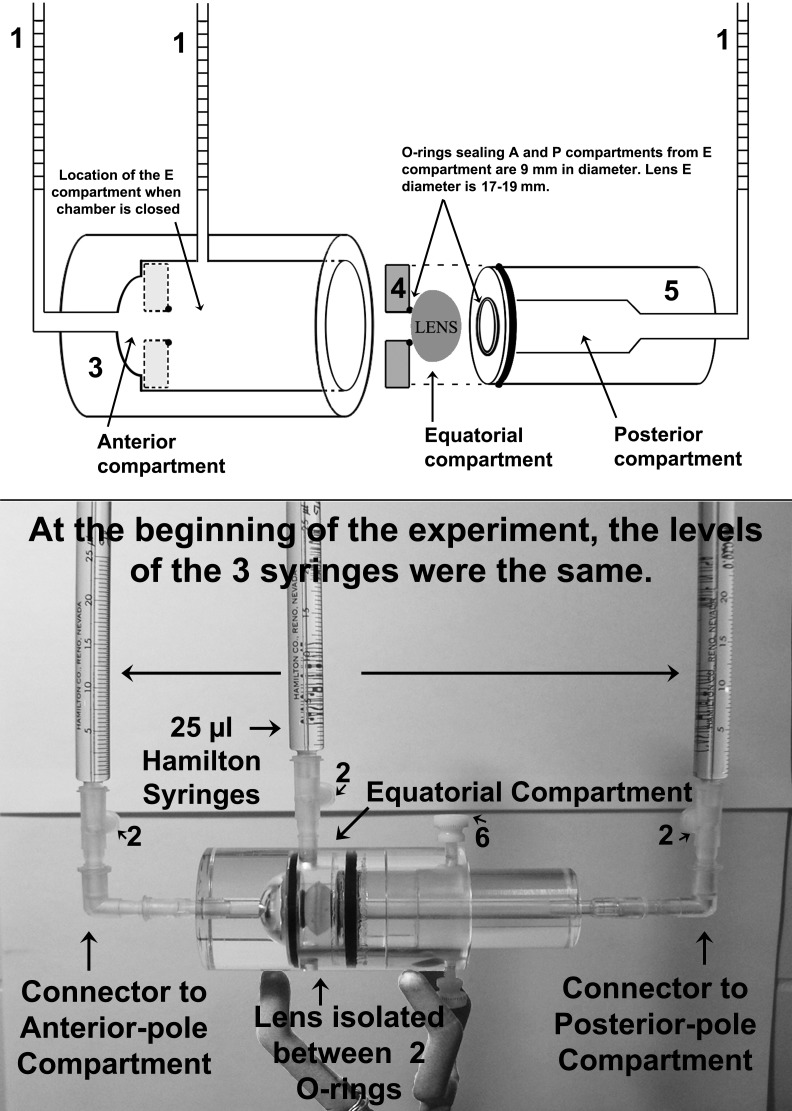
After acclimation in the bathing medium (usually 5–10 minutes in most experiments, but as long as 3 hours in others, as described in Results), the lenses were mounted within a novel three-compartment Ussing-type chamber that was fabricated from the milling of Lucite blocks (schematic outline of design shown in Fig. 1, upper panel). The chamber design essentially represents an outer cylindrical cavity (component 3 in Fig. 1) that accommodates a piston (component 5 in Fig. 1). Both the cavity and piston components contained ports for the attachment of graduated capillaries (i.e., 25 μL Hamilton syringes), as well as O-rings that sealed the lens. This design was based on the premise that O-rings of appropriate sizes can separate and seal different zones of the lens, thereby isolating associated fluid compartments. With two O-rings, one placed on the anterior face a few millimeters anterior to the equator, and the other posterior to the equator on the posterior aspect, three zones are demarcated. The inside diameter of the O-rings was 9.6 mm (measured from the central point of contact between the O-ring and the lens). Procured lenses had equatorial diameters ranging between 17 and 19 mm and were obtained from 3- to 4-year-old cows weighing ≈350 kg.

Figure 1.  .

Schematic diagram (upper panel) and photograph of replete chamber assembly with lens isolated within the three-compartment chamber used for these volumetric measurements (lower panel). Numbers in the diagram and photograph label the components in the chamber assembly, which are (1) 25 μL Hamilton syringes with 0.25 μL divisions; (2) leakproof Luer connectors; (3) body of the Lucite chamber with cylindrical cavity to accommodate piston (component 5); (4) washer that can accommodate O-rings of different diameters to adapt to the size of the lens—it lies flat against the bottom of the cylinder held by a layer of grease; (5) piston with its central O-ring that touches the lens and seals the center (equatorial) compartment from the posterior compartment (see Fig. 2); (6) screw to hold piston in place after all adjustments are made. Abbreviations: A, anterior; E, equatorial; P, posterior.

Placement of the lenses within the chamber initially entailed prefilling the piston (component 5 in Fig. 1) with physiological bathing solution with a syringe while the piston was oriented in a vertical position, and then gently depositing the lens with its posterior face downward upon the O-ring affixed to the end of the piston (Fig. 2). The cylindrical part of the chamber (component 3 of Fig. 1) was then lowered over the lens and piston, bringing the O-ring within the cylinder close to the epithelial surface. Additional bathing solution was then gently injected with the syringe into the piston, which resulted in a slight elevation of the posterior surface from the posterior O-ring as fluid entered and surrounded the equatorial and anterior aspects of the lens. Then the cylinder was further lowered over the piston until the O-rings on the piston and within the cylinder sealed by touching the lens, resulting in the isolation of the three compartments. Each O-ring touched the lens with just enough pressure to prevent leaks without distorting the shape of the lens. A screw (component 6 of Fig. 1, lower panel) was used to hold the piston in place relative to the outer cylinder after the lens was sealed.

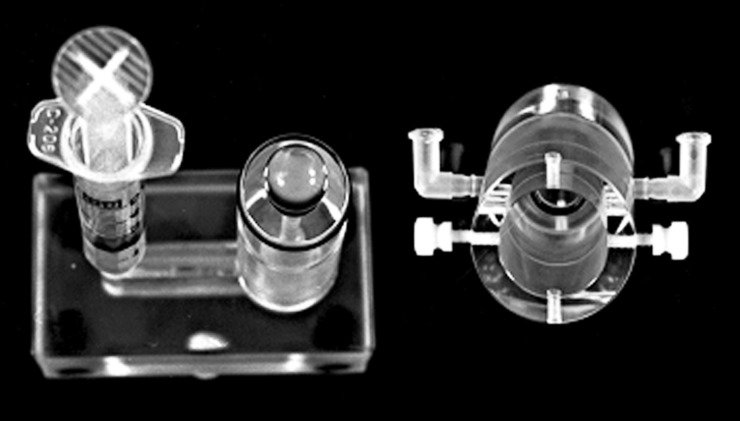
Figure 2.  .

Photograph of lens sitting on the piston's O-ring with anterior epithelial surface facing upward, and, to the right, the cylinder that will be slid over the lens-piston prior to connecting the Hamilton syringes.

At this point, the assembly was oriented horizontally; the filling syringe was removed from the piston and replaced with a Leur connector (component 2 of Fig. 1, lower panel) and a 25 μL Hamilton syringe (component 1 of Fig. 1) prefilled with bathing solution. Prefilled Hamilton syringes were also placed within the Leur connectors of the equatorial and anterior compartments that had been preinserted into the Lucite chamber walls within tapered apertures machined to snuggly accept the connectors without leaks. Bubbles were then removed, and the fluid levels in the Hamilton syringes were adjusted via introduction of a thin needle that fit into the cavity of the syringes. Applying a slight pressure with a piston to any one of the Hamilton syringes made it possible to determine if the three compartments were isolated by checking the levels of the other two syringes. In addition, in some experiments, a food colorant was added to one of the compartments for an alternative assurance that there were no leaks around the O-rings.

On average, the total surface area of the cow lenses that were isolated in the above arrangement was ≈8 cm².¹³ Assuming the cow lens is a perfect sphere, approximately 1 cm² of the anterior surface and 1 cm² of the posterior surface interfaced with bathing media within the respective compartments, while ≈6 cm² of lens surface was bathed within the center compartment.

Measurements of spontaneous fluid movement were made by recording the levels in the capillaries using the scales (0.25 μL divisions) on the Hamilton syringes. The syringes were pretreated with a hydrophobic agent (Rainix) to prevent fluid from creeping up the surface of the glass and distorting readings. Without leaks, changes in the level of the three capillaries must be such that their addition equals zero. This would be independent of possible small changes in the volume of the lens, since movement of fluid between the lens and any of the compartments will not affect the total volume of the system. For example, if the level of the capillary connected to the center compartment goes up, it should be associated with an equal decrease in one or both of the others.

The lens–chamber assembly was kept at room temperature (RT) of 26 ± 1°C. The volume of the anterior chamber (cylinder side) was 1.0 mL, and that of the posterior chamber (piston side) was 0.8 mL. The center (or equatorial) compartment had the shape of a donut around the lens, and its volume varied between 1.5 and 2.0 mL depending on the size of the lens. Using the 2.0 mL value for the center compartment, the total volume of the chamber was ≈3.8 mL, indicating that any putative 1° change in RT represented a ≈0.99 μL change in the specific volume of water, which would be distributed proportionally among the three capillaries.

In practice, small leaks occurred in some experiments (as discussed in Results). Thus, we arbitrarily decided that if the sum of the levels varied more than 3 μL, the experiment would be discarded because this indicated either a leak or an erroneous reading. At the start of the experiments, the fluid in the three capillaries was adjusted to the same horizontal level and read every 15 minutes by two independent observers, who did not share their observations until the end of the protocol. Results indicating marked discrepancies between observers were also discarded. All experiments lasted 2 hours.

Short-Circuiting of Lenses in the Three-Compartment Chamber

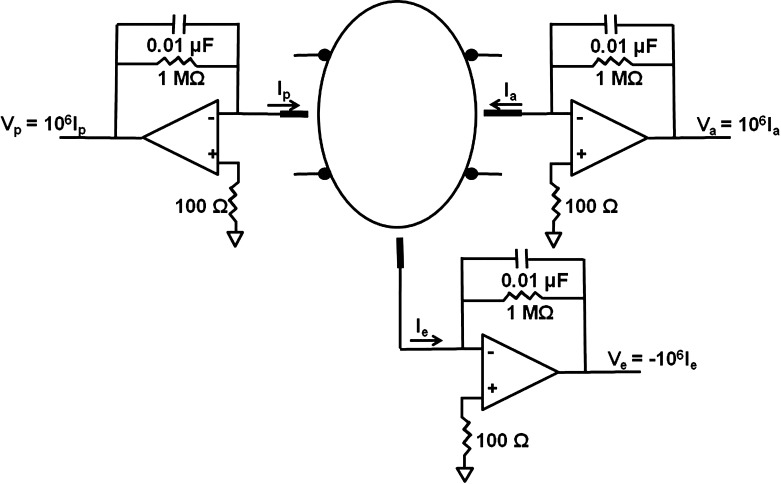
Because of the separation of the lens surface into three general lens zones (anterior, equatorial, and posterior), during isolation within the chamber, a potential difference (PD) is expected to develop between the bathing solutions of the compartments of the replete lens–chamber assembly. This is due to known differences in the electrolyte transport properties of these distinct lens surface regions.⁹ To determine if this spontaneous in vitro PD affected the measured rates of fluid movement between chamber compartments, some experiments involved short-circuiting the three compartments simultaneously so that the PD in each bath was the same. In these experiments, a T-shaped connector (seen in Fig. 3) was placed between the Lucite chamber and the capillary to enable the connection into each bathing compartment of a Ag/AgCl electrode that was in turn connected to a short-circuiting device. Figure 4 shows a simplified diagram of the main electronic components used to short-circuit the lens. The positive terminal of each operational amplifier is grounded, so the negative terminals, attached to the three chambers, are clamped to virtual ground by negative feedback, thus short-circuiting the three chambers to ground potential.

Figure 3.  .

Photograph of two chambers set up during an open-circuit experiment; that is, the T-connectors between the glass Hamilton syringes and the Lucite chamber were closed with plugs (seen at a right angle to the glass capillaries). For short-circuit experiments, the plugs were removed and Ag/AgCl electrodes were inserted into the connectors (not shown).

Figure 4.  .

Circuit diagram for the short-circuiting device that was used.

Most of our protocols consisted of either 2 hours under open-circuit conditions, 2 hours under short-circuit conditions, 1 hour each open and short, or 1 hour each short and open. In addition, other experiments tested the effects of electrolyte substitutions and ouabain under open circuit.

Composition of Bathing Media

The physiological medium used to bathe the lenses within the chambers had the following composition (mM): 1.8 calcium gluconate, 1.2 MgCl₂, 2 NaH₂PO₄, 4 KCl, 110 NaCl, 25 NaHCO₃, 10 glucose, and 15 HEPES hemi-sodium salt (i.e., 7.5 mM N-2-hydroxyethyl-1-piperazine-N′-2-ethanesulfonic acid plus 7.5 mM N-2-hydroxyethyl-1- piperazine-N′-2-ethanesulfonate-Na). When gassed with 5% CO₂, the pH of this solution was ≈7.5, and its osmolality measured 290 ± 5 mOsm/kg water. Gas bubbling of the bathing medium with CO₂ was not maintained within the relatively enclosed chambers, from which CO₂ could gradually evolve, albeit slowly, to the atmosphere via the small diameters of the three open capillaries (i.e., the Hamilton syringes). During the relatively short, 2-hour time frame of the experiments, the hemi-Na⁺ HEPES buffer (pKa = 7.5) contributed toward maintaining medium pH, which was measured at 7.6 to 7.7 at the end of the experiments.

Two other bathing solutions with reduced [Na⁺] were also used. In one, the 110 mM NaCl of the control solution was replaced by 110 mM N-methyl-D-glucamine HCl (NMDG-HCl), thereby lowering medium Na⁺ from the 144.5 mM concentration of the control bath to 34.5 mM; all other components were identical. For the second solution with reduced Na⁺, 110 mM KCl and 25 mM KHCO₃ were used in lieu of NaCl and NaHCO₃, respectively, resulting in a high-[K⁺] medium with 139 mM K⁺ and 9.5 mM Na⁺; all other components were identical to those in the control solution.

Statistics

Changes in the measured fluid levels in the capillaries were analyzed as two-tailed data using Student's t-test, with P < 0.05 chosen as the level of significance.

Results

Upon isolation of cow lenses within the three-compartment chambers, spontaneous changes in the fluid levels in each of the three capillaries were observed within 10 to 15 minutes. During the course of the present study, these changes invariably involved declines in the levels of the capillaries connected to the compartments bathing the anterior (A) and posterior (P) polar surfaces, while the fluid level of the equatorial (E) capillary increased.

Table 1 compiles the raw data from a typical experiment (upper rows), as well as providing calculations of the rates of fluid movement between compartments from these representative data (lower rows). In this and all experiments, the capillary levels of each compartment were read every 15 minutes for 2 hours. At t = 0 of this experiment, the respective A, E, and P capillary levels were adjusted to 15.00, 11.00, and 14.75 μL (Table 1, top row) for a total capillary reading of 40.75 μL (Table 1, last column). These initial, individual readings were set so that the fluid levels in the three capillaries were in the exact same horizontal plane, given that the plastic connectors joining the Lucite chamber to the glass capillaries were not of identical length (Fig. 1, lower panel). No additional adjustments were made to the capillary levels during the remainder of the 2-hour experiment. Fifteen minutes after the setting of the initial capillary levels, the readings of the A and P capillaries declined by 1.00 and 0.75 μL, respectively, whereas the level in the E capillary rose by 1.50 μL (Table 1, second row). The total reading in the capillaries declined by 0.25 μL in this 15-minute period, from 40.75 to 40.50 μL (Table 1, top, last column), indicating a very small, finite leak somewhere within the arrangement. When observed, leaks commonly occurred around the plastic connectors between the glass capillaries and the Lucite chambers. Nevertheless, the apparent leak in the representative experiment (Table 1) was markedly less than the gain in volume within the E capillary.

Table 1 .

Raw Data from a Typical Experiment

Time, min	A	Δ μL	E	Δ μL	P	Δ μL	A+E+P
0	15.00	0	11.00	0	14.75	0	40.75
15	14.00	−1.00	12.50	1.50	14.00	−0.75	40.50
30	13.00	−2.00	13.75	2.75	13.00	−1.75	39.75
45	12.25	−2.75	14.75	3.75	12.50	−2.25	39.50
60	11.50	−3.50	16.00	5.00	12.00	−2.75	39.50
60	11.50	0	16.00	0	12.00	0	39.50
75	11.00	−0.50	17.00	1.00	11.50	−0.50	39.50
90	10.50	−1.00	17.50	1.50	11.25	−0.75	39.25
105	10.00	−1.50	18.25	2.25	11.00	−1.00	39.25
120	9.75	−1.75	18.50	2.50	10.75	−1.25	39.00
Rate Calculations from Readings Listed Above
Time Interval	A μL/min		E μL/min		P μL/min		A+E+P
0 to 15	−0.067		0.100		−0.050		−0.017
15 to 30	−0.067		0.083		−0.067		−0.050
30 to 45	−0.050		0.067		−0.033		−0.017
45 to 60	−0.050		0.083		−0.033		0.000
Average:	−0.058		0.083		−0.046		−0.021
60 to 75	−0.033		0.067		−0.033		0.000
75 to 90	−0.033		0.033		−0.017		−0.017
90 to 105	−0.033		0.050		−0.017		0.000
105 to 120	−0.017		0.017		−0.017		−0.017
Average:	−0.029		0.042		−0.021		−0.008

Values are capillary readings (μL) from the anterior (A), equatorial (E), and posterior (P) compartments and changes in readings (Δ μL) with respect to baseline values over 15-minute intervals. The t = 60-minute capillary readings were taken as baseline for the second hour of the experiment. Changes in capillary readings were markedly slower during the second hour. The A+E+P column in the top part of the table indicates that the system lost 1.75 μL over the course of the 2-hour experiment, within our 3 μL exclusion criterion. The A+E+P column in the bottom part indicates that there was a tendency in this experiment for the A and P compartments to lose more fluid than the E compartment acquired. A value of zero indicates that the gain in the E compartment was exactly matched by the loss of fluid from the A and P compartments.

It was also apparent that the rates of change of the capillary levels declined with time. To document this, the t = 60-minute capillary readings were taken as a new baseline for calculating the rates of change within the three capillaries for the second hour.

The lower rows of Table 1 compile the rates of change within the three capillaries for the eight 15-minute intervals that were recorded. The average of the rates for the first hour is markedly higher than that for the second hour. Yet the volume of the E capillary always increased throughout the 2-hour experiment, while the A and P capillary volumes decreased. The reasons for the time-dependent decline in the rate of fluid transport are not known. However, they are probably related to placement of the lens in the chamber (Fig. 1), since in later experiments we maintained lenses in an open saline bath for 3 hours and then, upon their mounting in the chamber, observed the same rate of fluid flow as shown here. Thus the decline is not simple time-dependent rundown.

Moreover, it can be seen from the A+E+P column of the upper rows of Table 1 that the system lost 1.75 μL during the 2-hour experiment (from 40.75 to 39.00 μL). This loss was within our arbitrary exclusion criterion of 3 μL, and much less than the total volume of fluid transferred from the polar compartments to the equatorial compartment. Over the 2 hours, the A and P capillaries, respectively, lost 5.25 and 4.00 μL, and the E capillary gained 7.50 μL.

The A+E+P column of the lower rows of Table 1, where the rate calculations are presented, reiterates that there was a tendency in this experiment for the A and P compartments to lose more fluid than the E compartment acquired, given that this algebraic addition was never larger than zero. A value of zero would indicate that the gain in the E compartment was exactly matched by the loss of fluid from the A and P compartments.

The movement of fluid from the polar baths to the equatorial bath, described above, putatively occurred by the transport of fluid through the lens and not as the result of a leak of fluid around the O-rings isolating the lens surfaces within the chamber, given the absence of a hydrostatic pressure gradient within the in vitro arrangement. On the contrary, the flow of fluid was toward the E capillary even after its height was larger than that of the A and P capillaries. Additional experiments involving the use of (1) short-circuiting, (2) the Na⁺/K⁺ pump inhibitor ouabain, and (3) reduced bath Na⁺ levels were done to test the hypothesis that the net movement of fluid toward the E compartment involved an active transport mechanism.

Table 2 compiles the rates of fluid changes in the three capillaries of nine cow lenses under open-circuit conditions (upper rows) and six lenses under short-circuit conditions (lower rows). As indicated in the description of the typical experiment, flow rates were higher during the first hour. It was also apparent that there was a tendency for the flow to be higher under short-circuit conditions (unpaired data) in the set of lenses shown in Table 2. But variability and low number of experiments precluded statistical significance at the 5% level.

Table 2.  .

Rate of Fluid Flow in the Anterior, Equatorial, and Posterior Capillaries over 2 Hours under Open- and Short-circuit Conditions

Lens No.	Anterior First Hour	Anterior Second Hour	Equatorial First Hour	Equatorial Second Hour	Posterior First Hour	Posterior Second Hour
Open Circuit
1	−0.046	−0.046	0.100	0.058	−0.083	−0.046
2	−0.029	−0.017	0.088	0.050	−0.083	−0.046
3	−0.033	−0.017	0.038	0.033	−0.042	−0.021
4	−0.029	−0.013	0.104	0.033	−0.171	−0.092
5	−0.038	−0.017	0.063	0.042	−0.058	−0.033
6	−0.038	−0.017	0.071	0.063	−0.083	−0.058
7	−0.033	−0.013	0.054	0.025	−0.046	−0.029
8	−0.038	−0.021	0.021	0.017	−0.017	−0.008
9	−0.021	−0.008	0.050	0.017	−0.033	−0.013
Mean:	−0.034	−0.019*	0.065	0.038*	−0.068	−0.038*
SEM:	±0.002	±0.004	±0.009	±0.006	±0.015	±0.009
Short Circuit
10	−0.075	−0.046	0.092	0.054	−0.058	−0.033
11	−0.046	−0.033	0.100	0.067	−0.104	−0.042
12	−0.013	−0.013	0.100	0.050	−0.108	−0.042
13	−0.046	−0.029	0.038	0.050	−0.029	−0.013
14	−0.033	−0.042	0.079	0.050	−0.054	−0.017
15	−0.029	−0.029	0.083	0.042	−0.046	−0.021
Mean:	−0.040	−0.032	0.082	0.052*	−0.067	−0.028*
SEM:	±0.009	±0.005	±0.009	±0.003	±0.013	±0.005

Values are μL/min.

^* Significantly smaller than flow rate during the first hour as two-tailed, paired data, P < 0.05.

In another set of experiments (not shown), an attempt was made to determine the effects of short-circuiting as paired data. In these experiments, eight lenses were maintained under open circuit during the first hour and under short circuit during the second hour. In addition, the rates of fluid flow in the capillaries were measured in nine lenses under short circuit during the first hour and under open circuit during the second hour. However, the natural decline in the flow rates in the second hour (Tables 1 and 2) precluded interpreting these data. Yet, if we consider only the flow rates during the first hour from these experiments, plus the first-hour values from the experiments shown in Table 2, a statistically significant ≈40% increase in flow into the equatorial capillary (from 0.060 to 0.084 μL/min) can be shown as unpaired two-tailed data (Table 3). The flow rates in the capillaries of the polar baths did not change as saliently as that of the equatorial bath for reasons that are unclear; but as before, the decline in polar capillary volume exceeded the volume acquired by the equatorial compartment in both the open- and short-circuited conditions. With the latter condition, this discrepancy was less pronounced (see Table 3 notes, which show the effects of short-circuiting on a per hour basis). Overall, net fluid gains by the equatorial compartment exceeded losses in the system due to leaks, and the short-circuited condition stimulated fluid movement into the equatorial compartment.

Table 3.  .

Comparison of Flow Rates in the Anterior, Equatorial, and Posterior Capillaries of Lenses Exposed to Open- versus Short-circuit Conditions during the First Hour

	Anterior		Equatorial		Posterior
	Open	Short	Open	Short	Open	Short
	−0.044	−0.054	0.078	0.083	−0.004	−0.029
	−0.071	−0.067	0.088	0.079	−0.021	−0.017
	−0.035	−0.046	0.044	0.067	−0.006	−0.021
	−0.019	−0.083	0.046	0.117	−0.004	−0.033
	−0.046	−0.061	0.100	0.071	−0.083	−0.017
	−0.029	−0.088	0.088	0.121	−0.083	−0.050
	−0.033	−0.067	0.038	0.067	−0.042	−0.008
	−0.029	−0.046	0.104	0.083	−0.171	−0.017
	−0.038	−0.029	0.063	0.083	−0.058	−0.042
	−0.038	−0.075	0.071	0.092	−0.083	−0.058
	−0.033	−0.046	0.054	0.100	−0.046	−0.104
	−0.038	−0.013	0.021	0.100	−0.017	−0.108
	−0.021	−0.046	0.050	0.038	−0.033	−0.029
	−0.058	−0.033	0.058	0.079	−0.008	−0.054
	−0.100	−0.029	0.067	0.083	−0.017	−0.046
	−0.063		0.054		0.000
	−0.058		0.075		−0.038
	−0.033		0.075		−0.033
	−0.038		0.017		−0.008
	−0.079		0.046		0.000
	−0.029		0.021		−0.017
Mean:	−0.044	−0.052	0.060	0.084*	−0.037	−0.040
SEM:	±0.004	±0.006	±0.005	±0.005	±0.009	±0.008

Values are μL/min. (anterior open + posterior open) per hour = (−0.044–0.037) × 60 = −4.87 μL/h; equatorial open = 3.60 μL/h. (anterior short + posterior short) per hour = (−0.052–0.040) × 60 = −5.67 μL/h; equatorial short = 5.04 μL/h.

^* Significantly 40% greater than open-circuit value as unpaired two-tailed data, P < 0.005.

Given the difficulty in performing paired experiments with this method (i.e., a control hour followed by a second hour with a perturbation), the effects of ouabain were determined on an unpaired basis. For this, five lenses were pretreated for 45 minutes with 0.1 mM ouabain in a beaker containing the control bathing solution plus the Na⁺/K⁺ pump inhibitor. Then the lenses were mounted in the chamber under open-circuit conditions with the inhibitor maintained in the bath, and the capillary levels were monitored for 1 hour. Table 4 shows that the rate of fluid gained by the equatorial compartment in the presence of the inhibitor (0.011 μL/min) was ≈83% lower than that observed under open-circuit control conditions (0.065 μL/min) as two-tailed unpaired data. These data suggest that Na⁺/K⁺ pump activity is required to transport fluid to the equatorial compartment.

Table 4.  .

Rate of Fluid Flow (μL/min) in the Equatorial Capillary of Lenses Preincubated with 0.1 mM Ouabain for 45 Minutes prior to Mounting in Chamber

Lens No.	First Hour with 0.1 mM Ouabain Maintained in Bathing Solution
1	0.004
2	0.012
3	0.017
4	0.013
5	0.008
Mean:	0.011*
SEM:	0.002

The flow rates in the capillaries of the anterior and posterior compartments of the five lenses exposed to 0.1 mM ouabain were −0.007 ± 0.002 and −0.008 ± 0.003, respectively.

^* Significantly smaller than control value of 0.065, as two-tailed unpaired data, P < 0.001.

A second set of experiments was conducted with ouabain applied at a higher concentration. In this case, 12 lenses were pretreated for 3 hours with 1.0 mM ouabain prior to isolation in the chamber under open-circuit conditions. Table 5 shows that the fluid gained by the equatorial compartment during the first hour in the presence of the 1.0 mM concentration of the drug was virtually identical to that observed with the lower dose, suggesting that the maximal effect on fluid transport had been obtained with the lower concentration. Moreover, a residual Na⁺/K⁺ pump activity might be active in the large cow lens.

Table 5.  .

Rate of Fluid Flow (μL/min) in the Equatorial Capillary of Lenses Preincubated with 1.0 mM Ouabain for 180 Minutes prior to Mounting in Chamber

Lens No.	First Hour with 1.0 mM Ouabain Maintained in Bathing Solution
1	0.008
2	0.004
3	0.008
4	0.000
5	0.021
6	0.033
7	0.013
8	0.008
9	0.038
10	0.033
11	0.021
12	0.033
Mean:	0.018*
SEM:	0.004

The flow rates in the capillaries of the anterior and posterior compartments of the 12 lenses exposed to 1.0 mM ouabain were −0.013 ± 0.003 and −0.009 ± 0.002, respectively.

^* Significantly smaller than control value of 0.065, as two-tailed unpaired data, P < 0.001.

Additional experiments tested the effects of reduced bath Na⁺ levels on the rate of fluid transport to the equatorial compartment under open-circuit conditions. For these, Na⁺ was reduced by replacement with either NMDG or potassium. In the former situation, fluid transport was virtually eliminated by the NMDG-HCl substitution for NaCl (Table 6).

Table 6.  .

Rate of Fluid Flow (μL/min) in the Equatorial Capillary with Low Na⁺ (using NMDG Bathing Solution)

Lens No.	First Hour Low Na+	Second Hour Low Na+
1	0.00	0.00
2	0.01	0.00
3	0.01	0.00
4	0.04	0.00
5	0.01	0.00
6	0.02	0.00
7	0.02	0.00
8	0.00	0.00
9	0.01	0.00
10	0.01	0.00

Fluid flow in the NMDG bathing solution was barely detectable and essentially zero. NMDG bathing solution had 34.5 mM Na⁺ and 110 mM N-methyl-D-glucamine. NMDG-HCl replaced NaCl; all other components were the same as in the normal physiological medium. Changes in the capillary levels of the anterior and posterior compartments of these 10 lenses bathed with NMDG were also barely detectable.

In contrast, Na⁺ reduction by replacement with potassium salts elicited a transient biphasic phenomenon. With lenses initially exposed to the low-Na⁺ (9.5 mM)/high-K⁺ (139 mM) solution upon the mounting in the chamber, the rate of fluid transported into the equatorial bath during the first hour (0.089 μL/min) was significantly greater than that observed with control solution (0.065 μL/min), as shown in Table 7. The rate of fluid transport during the second hour was reduced (to 0.025 μL/min), as usually observed (Table 7). This reduced flow into the equatorial compartment during the second hour was marginally lower than the respective equatorial flow during the second hour under control conditions, 0.038 μL/min (Table 2), P < 0.09 as unpaired two-tailed data.

Table 7.  .

Rate of Fluid Flow (μL/min) in the Equatorial Capillary with Low Na⁺ (using High-K⁺ Bathing Solution)

Lens No.	First Hour Low Na+	Second Hour Low Na+
1	0.088	0.033
2	0.075	0.038
3	0.100	0.017
4	0.075	0.013
5	0.104	0.038
6	0.088	0.021
7	0.075	0.021
8	0.108	0.021
Mean:	0.089	0.025*
SEM:	0.005	0.003

Mean rate of equatorial fluid flow under control conditions was 0.065 μL/min during the first hour. The flow rate obtained with the high-K⁺ solution during the first hour was significantly greater than that obtained with control solution, as two-tailed, unpaired data, P < 0.05. The high-K⁺ bathing solution had 9.5 mM Na⁺ with potassium salts used to replace NaCl and NaHCO₃; all other components were the same as in the normal physiological medium. The flow rates in the capillaries of the anterior and posterior compartments of these eight lenses exposed to high-K⁺ solution were −0.044 ± 0.009 and −0.076 ± 0.008, respectively, during the first hour.

^*Significantly lower than first-hour value as paired two-tailed data, P < 0.005.

In a permutation of this condition, cow lenses were preincubated for 3 hours in either the control, normal physiological medium or the low-Na⁺/high-K⁺ solution. Then the lenses were mounted in the chamber with their respective baths, and fluid flow was monitored for 2 hours under open-circuit conditions. Data from these lenses are compiled in Table 8. The rate of fluid transported during the first hour toward the equatorial compartments of control lenses preincubated in normal physiological solution was not different than that observed in control lenses mounted without preincubation (Table 2 versus Table 8, left-side columns). Thus the 3-hour preincubation did not adversely affect the rate of fluid movement observed during the first hour in the chamber. The rate of fluid transport into the equatorial compartment of lenses preincubated for 3 hours in low-Na⁺/high-K⁺ solution was 0.036 μL/min, a value 47% lower than that observed with the normal solution (0.068 μL/min; Table 8), suggesting that depletion of the normal Na⁺ and K⁺ gradients necessary for driving the apparent fluid transport occurs relatively slowly with the large cow lens.

Table 8.  .

Effect of 3 Hours of Preincubation before Mounting of the Cow Lens in the Chamber on the Rate of Fluid Flow (μL/min) in the Equatorial Capillary

Preincubation and Bathing in Chamber with Normal, Control Solution			Preincubation and Bathing in Chamber with Low-Na+/High-K+ Solution
Lens No.	First Hour Control Solution	Second Hour Control Solution	Lens No.	First Hour Low Na+/High K+	Second Hour Low Na+/High K+
1	0.058	0.038	8	0.021	0.001
2	0.046	0.042	9	0.017	0.000
3	0.046	0.038	10	0.038	0.004
4	0.092	0.063	11	0.025	0.004
5	0.113	0.033	12	0.033	0.008
6	0.088	0.021	13	0.063	0.038
7	0.033	0.008	14	0.033	0.033
			15	0.063	0.029
			16	0.029	0.008
Mean:	0.068*	0.035	Mean:	0.036†	0.014†
SEM:	0.011	0.007	SEM:	0.006	0.005

For lenses 1 through 7, the flow rates in the capillaries of the anterior and posterior compartments during the first hour were −0.035 ± 0.003 and −0.073 ± 0.009, respectively; for lenses 8 through 16, these respective rates were −0.021 ± 0.004 and −0.026 ± 0.005.

^*Value not different than 0.065 rate observed in normal solution without 3-hour preincubation, P > 0.86, as two-tailed, unpaired data.

^†Value significantly lower than respective flow rate in normal solution after 3-hour preincubation, P < 0.025, as two-tailed, unpaired data.

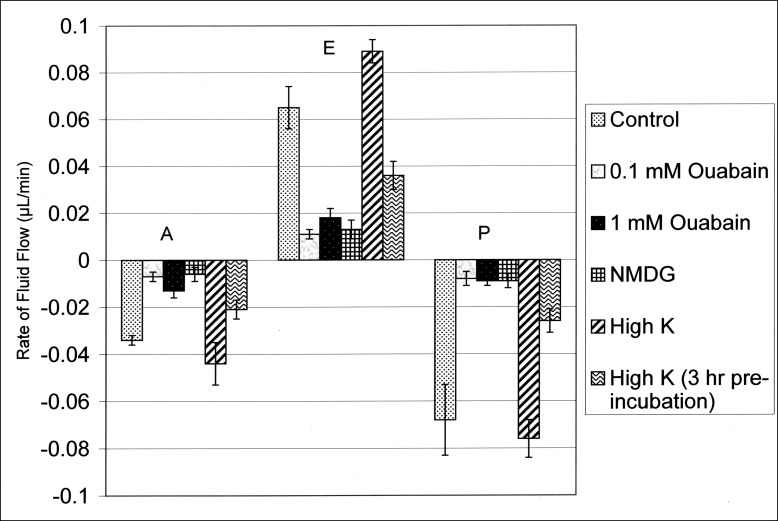
The results of the experimental perturbations described above and presented in Tables 4 through 8 are summarized in Figure 5. This figure plots the mean effects of the perturbations on fluid flow in all three compartments during the first hour. With the exception of the high-K⁺ condition described in Table 7, fluid flows in the capillaries of each compartment in the presence of an experimental perturbation were significantly lower than the respective control values of the A, E, and P compartments (data from Table 2) as unpaired two-tailed data (P < 0.05). As described above, the high-K⁺ condition (sans the 3-hour preincubation) elicited a stimulation in the flow into the equatorial compartment. While there was a tendency for the flows to simultaneously increase into the lens from the anterior and posterior compartments under this condition, these flows were not significantly larger than their respective control values (P > 0.55 as unpaired data).

Figure 5.  .

Summary plot of data (means ± SEMs) from Tables 4 through 8 for fluid flows across the anterior (A), equatorial (E), and posterior (P) surfaces during the first hour. Control values are from Table 2. Fluid flow in each compartment in the presence of an experimental perturbation is significantly different from its respective control value as unpaired two-tailed data (P < 0.05), except for the values of the flows in the A and P compartments for the high-K⁺ condition, where the flows are indistinguishable from their respective control values, P > 0.55, as unpaired two-tailed data. The flow in the E compartment for this latter condition (0.089 μL/min) is significantly higher than its control value, 0.065 μL/min (P < 0.05).

Discussion

The lens is an asymmetrical organ, both structurally and functionally, with localized transport properties. Although the identification and localization of specific transporters and ion channels are among topics of contemporary studies,¹⁴ the recognized asymmetrical nature of the lens provided the underpinning for the microcirculation model of Mathias et al. for fluid movement within the lens.^1–3 These authors proposed not only that epithelial electrolyte transport leads to the observed currents circulating around the lens, but also that such ionic transport provides the driving force for the intralenticular circulation of nutrients and metabolites due to a hypothetical fluid entry across the polar regions, followed by fluid circulation within the lens and equatorial exit, as further detailed below. To date, published studies have not directly demonstrated such fluid circulation by the lens or shown that such putative fluid circulation is indeed linked to circulating Na⁺ currents.

In the present study, we determined, using a volumetric technique that we developed, that a spontaneous fluid movement occurs across isolated surfaces of the cow lens upon mounting of the organ system within a unique chamber design with three separate compartments. Under our conditions, the compartmental volumes of the bath interfacing the anterior and posterior surfaces gradually decreased, while the bath volume interfacing the equatorial surface simultaneously increased. These observations indicate a fluid movement through the lens from poles to equator consistent with the Mathias et al. FCM. However, these data are clearly not sufficient to demonstrate that fluid circulation is indeed occurring due to net flows entering the internal lens cortex via the paracellular pathway and exiting the lens through a transcellular pathway prior to equatorial outflow, which is a requisite of the FCM. Nevertheless, the observed fluid circulation in the cow lens appears linked to a circulating Na⁺ current as evidenced by the stimulatory effects of short-circuiting and the inhibitory effects of ouabain and reduced levels of Na⁺ in the bath.

Briefly, the FCM of Mathias et al.^1–3 suggests that a circulation of Na⁺ within the lens draws a fluid flow capable of convecting essential nutrients (e.g., amino acids, glucose, antioxidants) into the deeper cortical and nuclear fibers. As proposed, a net inflow of Na⁺ likely enters the lens at the polar regions. Across the anterior epithelial aspect, Na⁺ inflow may occur both trans- and paracellularly. A transcellular Na inflow may occur given the low levels of Na⁺-K⁺ adenosine triphosphatase (ATPase) activity in the polar epithelium and the absence of ouabain-sensitive, Na⁺-K⁺ pump currents in this region.^9,15 A paracellular Na⁺ inflow into the lens may also occur given that the tight junctions in the polar region structurally resemble those commonly found in “leaky” epithelia,¹⁶ such as the corneal endothelium. This contrasts with the tight junctions found at the lens equator, which morphologically resemble those of high-resistance epithelia,¹⁶ with the cells in the equatorial region stratified as in a multilayered epithelium. The net movement of Na⁺ into the virtual space directly beneath the polar epithelium is predicted to create an osmotic gradient for water movement into the lens. Such movement could also occur both trans- and paracellularly. The FCM also posits that Na⁺ would continue to flow between the fiber cells and gradually enter the cellular space via nonselective cation channels in the fiber membranes. This translocation favors water entry into the fiber cells likely via aquaporin subtype 0 (AQP0).

The fiber cell membranes also exhibit the transporters necessary for the uptake of nutrients thought to be convected by the putative fluid movement¹⁴; and these transporters are presently presumed to be functional.

The FCM further proposes that the Na⁺ and fluid entry into the lens is balanced by an equal amount of Na⁺ and fluid efflux at the lens equator, maintaining steady-state lens electrolyte levels and volume. The efflux of Na⁺ and fluid is posited to occur transcellularly as a result of a “funneling” of Na⁺ movement from cell to cell via gap junctions toward the equatorial surface epithelial cells where the Na⁺-K⁺ ATPase activity is concentrated. As such, the lens microcirculation is thought to occur paracellularly in one direction and transcellularly in the other, thereby completing the circulatory loop.^2,3 Moreover, the FCM predicts the existence of a large intracellular hydrostatic pressure gradient (several hundred millimeters of mercury) that would drive the intracellular flow of fluid from the central cells to the surface cells through the gap junctions. Gao et al. recently measured this intracellular hydrostatic pressure in mouse lenses and found that it varied from 335 mm Hg in the central fiber cells to 0 mm Hg in the surface cells.¹⁷

While there are experimental data in support of the FCM,^1–3 few studies have attempted to directly detect fluid transport by the lens, and antithetical results have been obtained. Fowlks qualitatively ascertained that fluid moved across the rabbit lens in the posterior-to-anterior direction,¹¹ while Fischbarg et al. quantified a net fluid movement of ≈10 μL/h/cm² across the rabbit lens in the opposite direction.¹² The latter study also measured net fluid movement across cultured mouse and bovine epithelial cells in the basolateral-to-apical direction (≈3 μL/h/cm² and ≈5 μL/h/cm², respectively), thereby obtaining results consistent with the direction of the net flow that they determined across the intact lens. With the intact lens, these authors isolated the lenses within a chamber that occluded the equatorial surface, and speculated that the magnitudes of the circulating currents are insufficient to elicit substantial equatorial fluid efflux. This suggestion is contrary to the Mathias et al. model¹ and to our findings for substantial cationic currents at the equatorial zone, which are larger than at other areas of the lens surface.⁹

Subsequently, we developed the chamber design described in this paper to empirically measure fluid movement across the relatively large (≈18 mm equatorial diameter) freshly isolated cow lens and presented preliminary results in 2003 at the annual ARVO meeting (Candia OA, Gerometta R. IOVS 2003;44:ARVO E-Abstract 3455). We reasoned that a relatively large mammalian lens would be needed to allow detection of the expected rates of fluid transport by a volumetric method such as ours. In this regard, analogous experiments done to date on rabbit lenses have not resulted in detectable rates of trans-lens fluid movement between the three compartments of a virtually identical chamber scaled for the smaller lens of this species (Candia OA, unpublished data, 2010). We think that a rate of fluid circulation proportional to the rabbit lens volume would be undetectable by our method.

We further discussed the 2003 cow lens data in a review article on fluid transport by ocular epithelia¹⁰ but did not formally submit these findings for publication. The results described in the present study are from new, recently completed experiments and do not include the original data from 2003. In this regard, our current measurements indicate flow rates approximately twice those seen earlier, presumably due to improved experience with the method.

A consistency between the present results and those from 2003, however, is that fluid entered the cow lens from the anterior pole and exited from the equatorial surface. Across the posterior surface, the flow rates were smaller and more variable than those measured across the other two isolated surfaces in the older data set, whereas in the current experiments, fluid was always observed to enter the lens across the posterior surface, albeit with a large variability, likely due to limitations of the method. Sources of variability include the fact that the defined surfaces are arbitrary, that is, defined by the O-rings used to isolate the lens surface, such that individual lenses with different surface curvatures result in placement of a portion of the transporting surface on either one side of the O-ring or the other, by happenstance. Secondly, at the posterior surface, the thoroughness of the removal of vitreous may not have been identical in all of the lens specimens that were assayed. In addition, there was a decline in the rate of fluid transport in the second hour and even at the end of the first hour of most experiments, for reasons that are unknown. Despite this, we used the mean of all readings from the first hour for comparisons since it included four data points, thereby reducing overall variability given the limited resolution and precision of the individual readings. The decline in the rate of fluid transport with time in the chamber varied among lenses and was more variable in the flows recorded across the posterior surface.

Interestingly, we also observed a gradual decline in spontaneous fluid transport across isolated rabbit and cow ciliary epithelial preparations under conditions in which the detected fluid movement reflected solely the secretory activity of the isolated ciliary epithelium,¹⁸ since our in vitro arrangement precluded contributions from ultrafiltration, as well as externally applied osmotic or pressure gradients, which is also the case with the presently described cow lens conditions. The gradual decline in fluid transport observed under control conditions (Table 1) is not necessarily a consequence of a deterioration of the excised tissue within this time frame. Transepithelial electrical parameters of various lens preparations are stable over several hours,^9,19,20 suggesting that the driving force for spontaneous fluid transport is not lost within this period in vitro. If this is the case, the decline in fluid transport might result from a loss in membrane water permeability. Hypothetically, water channels could close or be gradually removed from lens membranes. In addition, it is not clear if the hydrostatic pressure needed to drive fluid from internal cortical cells toward the surface via gap junctions is maintained for a prolonged period in the in vitro chamber with the cow lens. Putatively, squeezing the lens into the chamber might disrupt and cause dissipation of the hydrostatic pressure gradients.

Nevertheless, the present observations suggest that the observed fluid movement (from polar compartments to equatorial compartment) through the cow lens is linked to a circulating Na⁺ current, given the increase in the short-circuited flow and the inhibitory effects of ouabain and reduced levels of Na⁺ in the bathing solutions. Na⁺ replacement with NMDG completely eliminated the fluid movement, whereas Na⁺ replacement with K⁺ elicited a time-dependent phenomenon. Short exposure (≤1 hour) to low-Na⁺/high-K⁺ solution resulted in a 37% stimulation of fluid movement. In contrast, after a 3-hour preincubation with the low-Na⁺/high-K⁺ solution, the measured fluid movement was 47% lower than that observed with control solution. These transient effects with low-Na⁺/high-K⁺ solution likely result from an initial increase in Na⁺ outflow through equatorial Na⁺/K⁺ pumps, thereby stimulating the fluid circulation. As intra-lens Na⁺ is depleted and the high K⁺ depolarizes the intra-lens voltage, Na⁺ circulation is inhibited by the loss of the electrochemical gradient for Na⁺ entry, and fluid circulation declines. The size of the relatively large cow lens might account for the length of time required to observe the reduction in fluid transport, which occurs secondary to the inhibition of Na⁺ circulation. Presumably a longer preincubation time would be necessary for a complete inhibition of Na⁺ and fluid circulation by the large cow lens. Microelectrode measurements from deep within the cow lens cortex are important for a further and more rigorous analysis.

As pointed out earlier by Mathias et al.,² our 2003 measurements of fluid movement by the cow lens (Candia OA, Gerometta R. IOVS 2003;44:ARVO E-Abstract 3455)¹⁰ may have significantly underestimated the amount of fluid moving through the lens in vivo. The reason is that the in vivo lens naturally exists in a short-circuited state, whereas our compartmentalization (i.e., separation) of the anterior polar, equatorial, and posterior polar regions enabled a PD to develop between each of the three compartments in our chamber. Based on the current density that we measured earlier,⁹ we would expect the PD of the equatorial bath (PD_eq) to be more positive than that of the anterior polar bath (PD_a), which is in turn more positive than the PD of the posterior polar bath (PD_p); thus, PD_eq > PD_a > PD_p. These PDs theoretically reduce the Na⁺ flux within the microcirculatory loop and putatively result in a lesser amount of fluid transported, as briefly explained below.

Because the anterior polar, equatorial, and posterior polar surfaces are not isolated within separate compartments in the eye, these surfaces interface with extracellular solutions at the same voltage; that is, the natural in situ lens is short-circuited in vivo. This is a unique situation, since all other epithelia transport electrolytes and fluid in the open-circuit state in vivo. In this latter situation there is a steady-state PD between the apical-side solution and the basolateral-side solution, the magnitude of which is directly proportional to the resistance of the paracellular pathway. In the case of the Cl⁻-secreting corneal epithelium, for example, Cl⁻ is actively transported transcellularly under open circuit and secreted into the apical (or tear)-side solution, while Na⁺ moves paracellularly in the same direction to neutralize the Cl⁻ charge and create a nearly isotonic fluid at the apical surface.

Likewise with the lens, under open circuit (as in our original in vitro situation for fluid measurements), we can predict that Na⁺ cannot move faster than Cl⁻, which is close to electrochemical equilibrium in the lens,² and thus the flux of Na⁺ within the microcirculatory current loop might be much less than that in the short-circuited state (the in vivo situation). In the present study we tested this possibility by measuring lens fluid transport under both open- and short-circuited conditions. Consistent with the theoretical concept, the observed fluid movement from the polar compartments toward the equatorial compartment was ≈40% larger under short-circuit conditions, suggesting that fluid circulation may occur in vivo.

Beebe and Truscott⁸ have aptly noted that our 2003 measurements of fluid inflow across the anterior epithelium and outflow across the equatorial and posterior surfaces (Candia OA, Gerometta R. IOVS 2003;44:ARVO E-Abstract 3455)¹⁰ do not reveal the pathway taken by water through the lens, or whether the water traverses the fiber cell cytoplasm as predicted by the FCM. As alluded to above, this is a valid criticism. Our method cannot demonstrate the pathways taken by water within the lens. However, our present experiments suggest that the observed fluid movement (inward at the poles and outward across the equator) may be linked to the circulating currents and is not a separate phenomenon, because the measured fluid transport rates changed in a manner consistent with the model upon alteration of the bath Na⁺ levels and the short-circuit status of the lens. Moreover, Vaghefi et al. used magnetic resonance imaging to demonstrate that the directionality of intracellular water movement in cow lenses is as predicted by the FCM, and substitution of external K⁺ for Na⁺ eliminated the directionality.²¹ Gao et al. showed that intracellular hydrostatic pressures varied from 335 mm Hg at the mouse lens center to 0 mm Hg at the surface, as predicted by the FCM, and this gradient could be eliminated by substitution of external K⁺ for Na⁺ or reduced by external ouabain.¹⁷ These experiments, in connection with our direct measurement of fluid circulation, provide compelling support that a fluid circulation consistent with the Mathias FCM is physiologically active.