The hummingbird's tongue: a self-assembling capillary syphon

Abstract

We present the results of a combined experimental and theoretical investigation of the dynamics of drinking in ruby-throated hummingbirds. In vivo observations reveal elastocapillary deformation of the hummingbird's tongue and capillary suction along its length. By developing a theoretical model for the hummingbird's drinking process, we investigate how the elastocapillarity affects the energy intake rate of the bird and how its open tongue geometry reduces resistance to nectar uptake. We note that the tongue flexibility is beneficial for accessing, transporting and unloading the nectar. We demonstrate that the hummingbird can attain the fastest nectar uptake when its tongue is roughly semicircular. Finally, we assess the relative importance of capillary suction and a recently proposed fluid trapping mechanism, and conclude that the former is important in many natural settings.

1. Introduction

Capillary action was one of several mechanisms proposed to account for hummingbird nectar uptake in the nineteenth century [1,2]. The question received renewed attention in the 1930s, and despite disagreements regarding the relative importance of capillarity [3,4] and a mechanism relying on the combined action of the bill and the tongue [5,6], a consensus emerged that capillarity plays at least a partial role [7]. According to this hypothesis, once the tongue tip touches a nectar reservoir, surface tension drives the nectar flow through the tongue's grooves, loading the tongue before its subsequent withdrawal and unloading.

The distal portion of the hummingbird's tongue forms two parallel C-shaped grooves that split in a bifurcated end [8] (figure 1a). These grooves consist of keratinized membranes on the order of 25 µm in thickness, which curl around a relatively rigid, keratinized rod [9]. The free edge of the groove ends in fine fringes or lamellae that are generally attributed to wear [10,11]. Because vascular and nervous tissues recede at the tip of the tongue [9,8], no active change of shape of these grooves is possible: tongue groove deformation can only be driven by hydrodynamic forces arising from interaction with the nectar.

Figure 1.

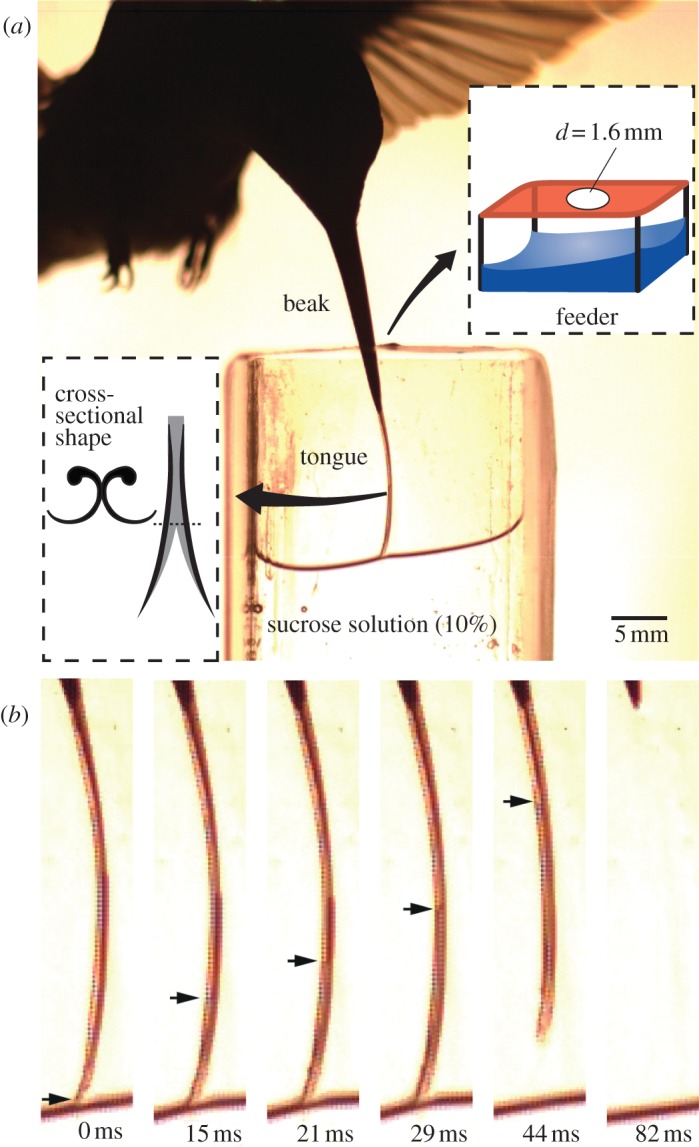
A hummingbird (Archilochus colubris) drinking from a transparent feeder. (a) A photograph of the drinking bird. Insets: schematic of the hummingbird's tongue (left) and feeder (right). The feeder is made of glass plates, and wrapped with red paper in order to attract the bird. (b) High-speed images of the hummingbird drinking from a feeder. Owing to the transparency of the tongue, the meniscus of the rising nectar (arrows) is observable. As the tongue tip touches the surface, interfacial forces drive the liquid along the tongue at speeds of approximately 20 cm s⁻¹ (see electronic supplementary material, movie S1).

Flexible solids in the presence of liquids can be deformed by interfacial forces [12]. Py et al. [13] presented the first example of capillary origami, demonstrating that thin sheets with bending stiffness B can be folded up by the surface tension σ of a water droplet placed on them, provided that the largest sheet dimension exceeds the elastocapillary length l_E = (B/σ)^1/2. Recently, Rico-Guevara & Rubega [11] demonstrated that a hummingbird tongue closes around nectar, thus representing a natural example of capillary origami [14]. Their high-speed videography indicates that when the tongue is withdrawn from the nectar, the formerly immersed portion of the tongue changes shape, so that the thin membrane curls inwards and traps liquid inside its grooves. They thus described the drinking mechanism as a fluid trap: surface tension causes the trap to close, after which the fluid is transported mouthward by tongue retraction.

An open question remains concerning how the nectar fills the entire tubular grooves, each of which is on the order of 1 cm in length and 150 µm in radius [8]. Specifically, what is the relative importance of fluid trapping and capillary suction in the loading of the tongue? We note that the former does not preclude the latter, and so expect both to be significant. Because the nectar reservoirs of many of the hummingbirds' target flowers are shorter than the tongue groove length, the entire groove cannot always be immersed in the reservoir [15]; in such cases, fluid trapping without capillary suction would not optimally load the tongue.

Kingsolver & Daniel [16] proposed the first dynamic model for capillary suction in a solid tube (see also Daniel et al. [17]). By demonstrating that this model successfully predicts the dependence of nectar intake rate on nectar concentration, Kim et al. [18] recently provided a rationale for the optimal concentrations [19] for the fastest energy uptake—33 per cent for suction feeders, as measured in a laboratory setting. Here, we present in vivo observations of capillary suction in a hummingbird tongue using high-speed videography [20,21]. We also report direct observations of elastocapillary behaviour [12,13,22,23], as the tongue is deformed by capillary forces during nectar uptake. We then develop a theoretical model for the hummingbird's drinking process, thereby elucidating how the elastic deformation of the tongue affects the nectar dynamics.

2. Results

(a). In vivo observations

We filmed captive ruby-throated hummingbirds (Archilochus colubris) drinking a sucrose solution (10% by mass) from a feeder (see figure 1 and electronic supplementary material, movie S1). A 1.6 mm diameter hole on the top of the feeder allows the bird to insert only the distal portion of its beak into the feeder. We adjusted the level of the sucrose solution so that the bird had to extend its tongue tip approximately 13 mm out of its bill to reach the reservoir. Figure 1b indicates that as soon as the tongue tip touches the free surface of the reservoir, the liquid begins to rise along the tongue groove, thus clearly demonstrating capillary suction of the sucrose solution along the hummingbird's tongue. The rise continues until the tongue is extracted from the liquid, and the tongue is retracted into the beak.

For quantitative analysis of the capillary suction and tongue deformation, the tongue is observed at a higher magnification (see figure 2 and electronic supplementary material, movie S2). Before entering the liquid, the tongue tips adhere to each other owing to surface tension, indicating that the tongue is prewetted with either nectar or saliva. Upon contact with the fluid, the immersed tips separate, and the sucrose solution starts to climb up along the tongue, as indicated by the rising menisci in figure 2a. The menisci advance at a speed u ∼ 20 cm s⁻¹ in response to capillary action, and the tongue is moving at a speed of less than 7 cm s⁻¹ while the tongue tip is immersed in the nectar. After being loaded by capillary suction, the tongue retracts with peak speeds of 33 cm s⁻¹. The tongue protrusion and retraction are repeated at a frequency of approximately 6 Hz.

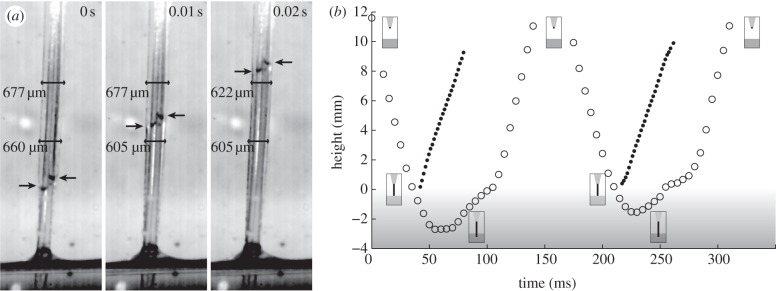
Figure 2.

Capillary suction through the hummingbird's tongue. (a) A dorsal view of the tongue of a hummingbird drinking sucrose solution of 20% concentration by mass. The arrows indicate the two menisci of the rising nectar. The tongue width becomes smaller after the inner surface is wetted by the nectar. (b) The position of the tongue tip (open circles) and meniscus (filled circles) during two consecutive licks. The measurement is interrupted when the meniscus moves beyond the field of view. The nectar rise speed is approximately 20 cm s⁻¹ (see electronic supplementary material, movie S2). The capillary rise of the nectar clearly precedes the tongue retraction.

Examining dorsal views of the tongue during capillary suction indicates the deformation of the tongue because the tongue's lateral extent becomes smaller after its inner surface is wetted by the nectar (figure 2a). Our measurements reveal that the outer tongue diameter decreases by approximately 10 per cent near the advancing meniscus, but we observe that surface tension does not cause the complete closure of the groove. A schematic illustration of the deformation of one of the two tongue tubes is presented in figure 3.

Figure 3.

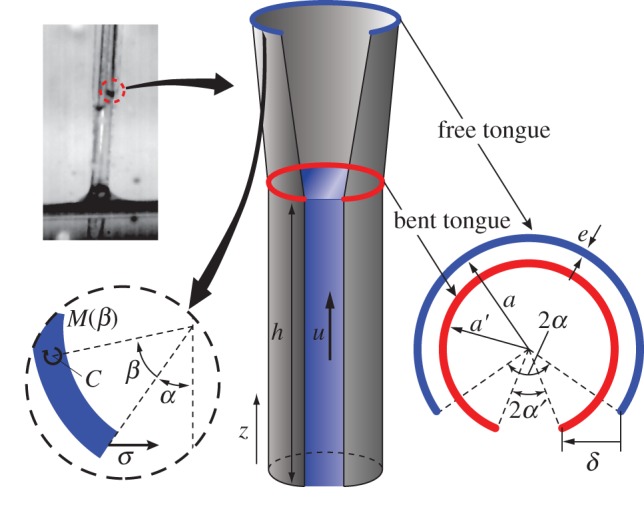
A schematic of nectar rise along the flexible tongue of the hummingbird, which closes in response to the surface tension. For the sake of clarity, only one of the tongue's two grooves is illustrated.

(b). Tongue deformation

Drinking in hummingbirds involves a sequence of nectar loading and unloading events. It has been demonstrated that hummingbirds unload nectar by squeezing their tongues between their upper and lower bills [24]. The tongue must be flexible for this unloading process. In addition, when only small quantities of nectar are available in the target flowers, a flexible tongue may make it easier for the tongue lamellae to sweep the corolla tube [11,25]. Hummingbirds feed from plants with a variety of floral morphologies; jewelweed (Impatiens capensis) even has forward-pointing nectar spurs that require the tongue to bend at a 180° angle [26]. We thus infer that the tongue's flexibility is advantageous in both accessing and unloading the nectar.

We proceed by examining its role in nectar transport. We model the tongue as an open circular groove without longitudinal variation (see figure 3), with radius a ∼ 150 µm, thickness e ∼ 25 µm, length l ∼ 1 cm and opening angle 2α ranging from 0 to π [8,9]. The ratio of hydrostatic to capillary pressures is prescribed by the Bond number Bo = ρgal/σ, where ρ is the density of the nectar, g the gravitational acceleration and σ the surface tension. Owing to the weak dependence of σ and ρ on sucrose concentration [16], we treat σ ∼ 0.07 N m⁻¹ and ρ ∼ 1000 kg m⁻³ as constants with respect to sucrose concentration. Because Bo attains a maximum value of 0.2 for the worst-case scenario of a vertical tongue, we infer that tongue deformation is caused principally by surface tension applied along its lateral edges rather than by hydrostatic suction along its length.

To estimate the tongue deformation, we consider a lateral segment of the groove of length βa, as shown in figure 3, where the angle β is measured clockwise from the edge. Balancing moments about the point C yields the bending moment M(β) per unit length at the cross section, aβ away from the edge: M(β) = σa[cosα − cos(α + β)], where a clockwise moment is defined as positive. Then, applying Castigliano's theorem [27] yields the maximum tongue displacement δ at the edge:

2.1

where B ∼ e³Y is the bending stiffness per unit length, Y the tongue's Young's modulus and 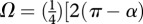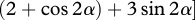. The dimensionless deformation thus scales as δ/a ∼ Γ, where Γ = a²σ/B represents the control parameter of the system. By measuring Y ∼ 300 kPa (see §5), we estimate Γ ∼ 0.3 and thus consider the regime of weak deformation, which is consistent with our direct observations of the tongue diameter contracting by approximately 10 per cent near the advancing meniscus.

(c). Elastocapillary suction

We define the Weber number We = ρau²/σ, the ratio of inertial to curvature pressures, and the reduced Reynolds number Re = ρua²/μl, the ratio of inertial to viscous forces. We use the empirical dependence of nectar viscosity on sucrose concentration [28], noting that 0.001< μ<0.1 for sucrose concentrations between 0 and 65 per cent. For typical rise speeds u ∼ 0.1 m s⁻¹ (figure 2b), we thus estimate We ∼ 0.02 and 0.002 < Re < 0.2. Therefore, inertial effects are negligible, and the nectar flow through the hummingbird's tongue is described by Stokes's equation: 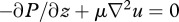, where P is the pressure. The pressure gradient may thus be expressed by 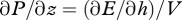, where V is the nectar volume inside the tongue, and E the total system energy contained inside the tongue of height h (see figure 3)—specifically, the free surface energy E_s plus the bending energy E_b.

For nectar rise by an infinitesimal height Δh from h to h + Δh, the free surface energy associated with the inner surface of the tongue increases by 2(π − α)(γ_SL − γ_SV)aΔh, where γ_SL and γ_SV are the interfacial energy per unit area between solid and liquid, and between solid and gas, respectively. Also, owing to the interfacial area between liquid and gas at the gap of both edges, the free surface energy increases by 2a′sinα′σΔh, where a′ and α′ are, respectively, the radius of the groove and the half-opening angle after deformation (see figure 3). Using Young's equation, γ_SV − γ_SL = σ cos θ_c, the increase in free surface energy ΔE_s can thus be expressed by ΔE_s = 2[−(π − α)a cosθ_c + a′sinα′]σΔh, where θ_c is the contact angle. The bending energy is principally associated with the change of the cross-sectional tongue shape. Because the strain energy per unit longitudinal length is 2σδ = 2σaΓΩ, the increase in the strain energy for deformation over a length Δh is given by ΔE_b = 2a σΓΩΔh.

Substituting into Stokes's equation yields

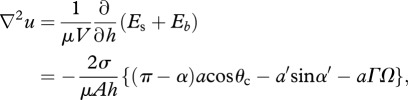2.2

where A = (π − α′ + cosα′sinα′)a′² denotes the cross-sectional area of the rising nectar. The no-shear and no-slip boundary conditions are applied at, respectively, the meniscus between the edges and the inner wall of the tongue (see figure 3).

The solution of Stokes's equation gives the velocity distribution of u over the cross-sectional area A. From the computation of the velocity field via the finite element method (FEM), we estimate the average flow speed and the nectar rise h(t) (see §5). For a licking frequency f, the energy per volume of nectar c and the time of contact with the nectar τ for each lick, the energy intake rate ɛ predicted by our model is .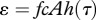

We restrict our attention to a given f and τ, nectar properties (μ, σ, c), and lateral perimeter of the tongue. While the driving capillary pressure decreases with increasing α, the cross-sectional area through which nectar flows increases with α, and the resistance to flow decreases. One thus anticipates an optimal opening angle for which the hummingbird attains the fastest energy intake. In figure 4, we plot the energy intake rates for tongues that have the same perimeter but different Γ. The energy intake rates are normalized by that obtained for α = 0, Γ = 0 and a = 150 µm, and α_c represents a limit below which the two lateral edges come into contact after bending. Although the optimal opening angle depends on Γ, the normalized energy intake rate is maximized at opening angles between 140° and 170°. For parameters relevant for the hummingbird, we conclude that opening angles 140° < 2α < 170° optimize energy uptake. Because the results presented in figure 4 are independent of the frequency, we expect them to be valid even at higher licking frequencies, which can be as large as 17 Hz when the distance between the beak tip and the nectar is smaller [24].

Figure 4.

The dependence of the energy intake rates on the opening angle 2α for different Γ = σa²/B, where a is the undeformed radius and B is the bending stiffness per unit length. Here, the energy intake rate is scaled by that for the tongue of α = 0, Γ = 0 and a = 150 µm. The closed circles represent α_c, a limit below which the two lateral edges come into contact after bending. The tongue perimeter is the same for all cases. The optimal opening angles 2α that maximize energy intake rates are indicated by the dashed line and lie between 140° and 170°.

3. Capillary suction versus fluid trapping

Recently, the role of capillary action in hummingbird feeding has been questioned: a new model proposes that instead of being taken up via capillary suction, fluid is captured through entrainment by the tongue following submergence, a mechanism called ‘fluid trapping’ [11]. Arguments against the role of capillary action include the observation that nectar intake rates are only weakly dependent on flower orientations (pendulous or erect). However, as previously noted, the value of Bo ∼ 0.2 indicates that gravitational effects are negligible in nectar uptake. Another potential inconsistency of the capillary model is that it predicts optimal nectar concentrations (30–40%)—specifically those that maximize energy uptake rate—that are different from preferred concentrations (45–60%), as deduced from the statistics of visit frequency to feeders with different nectar concentration [29,30]. However, a bird's preferred concentration may depend on factors such as gustatory preferences [31] or physiological state [32], and thus may not correspond to the optimal concentrations.

We note that fluid trapping does not inherently preclude capillary suction; so hummingbirds can use both mechanisms. In our experimental set-up, we can assess the importance of each mechanism. To permit visualization of the tongue outside the bill and in a vertical orientation (figure 2), we positioned the level of the sucrose solution (20% by mass) in the feeder sufficiently far from the opening of the feeder that the tongue must extend approximately 15 mm from the bill to be immersed by an amount h_i ∼ 2 mm in the sucrose solution. We measured a ∼ 150 µm, τ ∼ 50 ms, μ ∼ 2 mPa · s; so the length loaded via capillary suction in a time τ is given by h_c ∼ (σaτ/(2μ))^1/2 ∼ 1 cm (see §5). We thus deduce h_c/h_i ∼ 5, and so assess that capillary suction is the dominant nectar-loading mechanism in this experimental setting.

Our experimental set-up probably resulted in a longer tongue extension and slower licking frequency than may occur in natural feeding bouts. The relative importance of capillary suction and fluid trapping in the wild will in general depend on the depth of the nectar reservoir of the visited flower as well as on the licking frequency of the hummingbird. Very few data exist for heights of nectar, and estimating this is challenging: nectar volume can vary temporally, as well as within and among populations [33–37], and data on corolla diameter are scarce [38]. Moreover, the diameter at the base of the corolla tube may be different from the distal corolla diameter.

We list corolla measurements of six species of flowers visited by ruby-throated hummingbirds (see electronic supplementary material, table S1). Using images obtained from the digital herbaria of New York Botanical Garden and Missouri Botanical Garden, as well as specimens from the Gray Herbarium (Harvard University Herbaria), we obtained a rough measurement of proximal corolla width, which we used to estimate a possible upper-bound value for nectar height (see electronic supplementary material, table S2 and figures S1–S5). Owing to the uncertainties associated with estimates of nectar volume, as well as internal corolla shape, these calculations are approximations; yet it appears that some plants may have a small nectar height H compared with the tongue groove length of l ∼ 1 cm in the ruby-throated hummingbird [8]. In addition, during a natural drinking bout, the volume of nectar in a flower will decrease progressively. Thus, partial immersion of the tongue (h_i ∼ H ∼ 1 mm) may commonly arise in the wild.

The capillary loading length h_c depends critically on μ and τ. The loading time τ may be bounded by the licking frequency f, which ranges from 6 Hz (our observations) to 17 Hz [24], and has been shown to depend on the tongue's extrusion length and corolla shape [39]. In our observations (figure 2), τ ∼ 50 ms is approximately 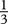of the period of the full licking cycle 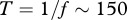ms. If we were to assume similar tongue kinematics of 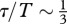for different licking frequencies, we would expect that 20 < τ < 50 ms for 6 < f < 17 Hz. By using the empirical dependence of μ on sucrose concentration c [28], we deduce the dependence of h_c on c for different τ (see figure 5). As the concentration of the hummingbird flowers is typically 20 to 25 per cent [40], the model results presented in figure 5 indicate that when a hummingbird drinks nectar from a flower with a shallow nectar reservoir of H₁ = 1 mm, the majority of its nectar is loaded via capillary suction for biologically relevant values of τ. Fluid trapping becomes appreciable when the hummingbird drinks from deeper nectar reservoirs (i.e. H₃ = 10 mm) or with high licking frequencies. While the relative importance of capillary suction and fluid trapping will thus in general depend on feeding rates, tongue morphology and plant morphology, available estimates indicate that hummingbirds may benefit from capillary suction in many natural settings.

Figure 5.

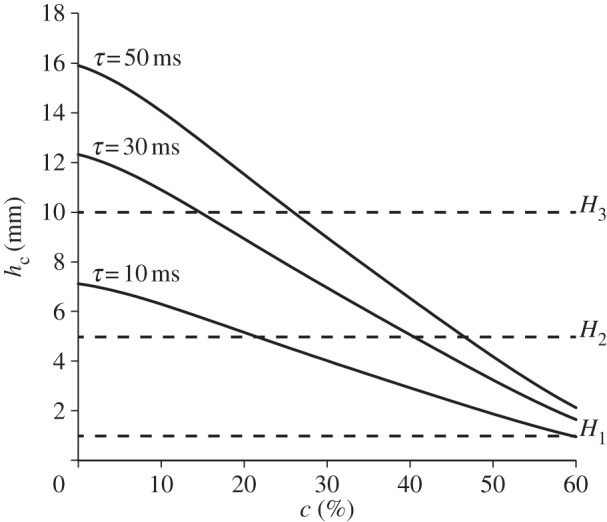
The dependence of the tongue length loaded by capillary suction, h_c, on sucrose concentrations c for a range of biologically relevant loading times τ. We represent three different nectar reservoir depths of H₁ = 1 mm, H₂ = 5 mm and H₃ = 10 mm by dashed lines. Provided that the tongue immersion depth h_i ∼ H, curved and dashed lines allow for a comparison between nectar volumes loaded via capillary suction and fluid trapping.

Previous laboratory experiments on hummingbird drinking [8,15,29,41,42] lend support to the capillary suction model [16]. These indicate that the observed dependence of nectar intake rates on nectar concentrations are satisfactorily rationalized by the capillary suction model [18]. Moreover, the fact that the average volumetric uptake rate decreases with nectar concentrations [15] is consistent with the capillary suction model, but inconsistent with the fluid trap model, which suggests that nectar uptake rates are independent of nectar concentration.

4. Discussion

We have presented in vivo observations of hummingbird drinking that indicate both the elastocapillary deformation of the hummingbird's tongue and capillary suction along its length. The hummingbird's tongue may thus be best described as a self-assembling capillary syphon. Our observations clearly indicate that fluid trapping and capillary suction are complementary rather than mutually exclusive mechanisms. While both are viable mechanisms for nectar uptake, we conclude that capillary suction is important in many natural settings. Nectar reservoirs are often shallow, relative to the tongue's groove length, thus precluding tongue submergence, in which case capillary suction is predominantly used. Moreover, the dependence of nectar uptake rates on nectar concentrations reported in the biological literature [8,15,29,41,42] for the hummingbird are well rationalized by the capillary suction model [18].

Guided by our observations, we have developed a theoretical model for the elastocapillary suction of nectar. The model suggests that the hummingbird can maximize the energy uptake rate when the opening angle of its tongue is roughly 150°. Our model thus provides new rationale for the shape of the tongue: specifically, the fact that each of the two grooves of a hummingbird's tongue is nearly semicircular (figure 1a). The results of our model (figure 4) suggest that, for a given opening angle, a rigid tongue (Γ = 0) enables the hummingbird to maximize the energy uptake. Nevertheless, tongue flexibility Γ ∼ 0.3 presumably evolved because it is advantageous for both accessing and unloading nectar.

Floral nectar is the primary energy source of hummingbirds, and their inter-flower movement serves to pollinate flowers. This mutual reliance can result in coevolution of bill shape and floral morphologies [38,43,44]. Thus, the detailed shape of the hummingbird's tongue may also be affected by the corolla morphology and nectar attributes of its target flowers. Nevertheless, flexible tongues with semicircular cross-sectional shapes are characteristic of many hummingbirds, as well as sunbirds and honeyeaters [25], which may rely on similar nectar uptake styles.

5. Methods

(a). In vivo high-speed imaging

The movies were taken at the Concord Field Station, in Concord, MA. We filmed four individuals (of the species Archilochus colubris), feeding during flight in a cage (figure 1) or while held in the hand (figure 2). The birds fed spontaneously. The feeders contain a sucrose solution of concentration 10 per cent (figure 1) or 20 per cent (figure 2) by mass, which is sufficiently far from the opening of the feeder for the birds to have to extend their tongues out of their bill in order to reach the liquid. We filmed the dynamics of the tongue and nectar with high-speed cameras (Phantom V5.2 running at frame rates of 1000 fps for figure 1 and Photron Fastcam 1024 PCI running at frame rates of 2000 fps for figure 2).

(b). Tension stress experiment

We prepared a rectangular sample (1.0 × 0.2 mm) from the tongue tip of a deceased hummingbird (Archilochus colubris). We stuck the extremities of the samples on a plastic holder. By using a high-precision dynamometer, we recorded the force exerted on the sample while extending it. By measuring a deformation rate in the elastic regime, we calculated the Young's modulus. Although the thickness of the tongue is not uniform, we assumed a thickness of 25 µm in order to calculate a value of Y ∼ 300 kPa.

(c). Finite element method

The linearity of Stokes's equation enables us to find the solution via numerical solution of

5.1

with the boundary conditions

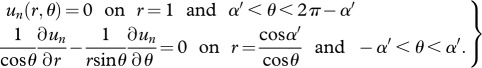5.2

By using the FreeFEM++ software (see http://www.freefem.org), the velocity distribution of u_n is found. The dimensionless volumetric flow rate Q_n through A_n, the cross-sectional area bounded by conditions (5.2), depends only on α′. The volumetric flow rate Q for the tongue dimensions is then given by 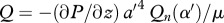. Because Q is the product of the cross-sectional area A and the rise speed dh/dt, one readily obtains the differential equation

5.3

which we solve with the initial condition h(0) = 0. For the prewetted tongue (θ_c = 0), the solution of equation (5.3) yields the dependence of rise height on time:

5.4

The lateral perimeter of the tongue groove does not change through bending: (π − α)a = (π − α′)a′ and 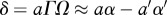. Solving these equations for a′ and α′ yield a′ = (1 − ΓΩ/π)a and α′ = [1 − (π − α)/(π − ΓΩ)]π in equation (5.4). For a rigid, circular tube (Γ = 0, α′ = α = 0, a = a′), Q_n(0) = π/8 and h(t) = (aσt/(2μ))^1/2.