Plant invasions and extinction debts

Abstract

Whether introduced species invasions pose a major threat to biodiversity is hotly debated. Much of this debate is fueled by recent findings that competition from introduced organisms has driven remarkably few plant species to extinction. Instead, native plant species in invaded ecosystems are often found in refugia: patchy, marginal habitats unsuitable to their nonnative competitors. However, whether the colonization and extinction dynamics of these refugia allow long-term native persistence is uncertain. Of particular concern is the possibility that invasive plants may induce an extinction debt in the native flora, where persistence over the short term masks deterministic extinction trajectories. We examined how invader impacts on landscape structure influence native plant persistence by combining recently developed quantitative techniques for evaluating metapopulation persistence with field measurements of an invaded plant community. We found that European grass invasion of an edaphically heterogeneous California landscape has greatly decreased the likelihood of the persistence of native metapopulations. It does so via two main pathways: (i) decreasing the size of native refugia, which reduces seed production and increases local extinction, and (ii) eroding the dispersal permeability of the matrix between refugia, which reduces their connectivity. Even when native plant extinction is the deterministic outcome of invasion, the time to extinction can be on the order of hundreds of years. We conclude that the relatively short time since invasion in many parts of the world is insufficient to observe the full impact of plant invasions on native biodiversity.

Introduced species are often considered a leading threat to native biodiversity (1, 2). However, recent syntheses show that competition from introduced species, and plant invaders in particular, has only rarely resulted in extinction (3–6). This trend has emerged because, in the short term at least, invasive plants do not completely extirpate native plant species but rather reduce their distribution and abundance, often restricting them to isolated habitat refugia (7–9). Despite well-established cases of native plants occupying distinct refugia and outperforming invasive plant species in those habitats (8, 10, 11), the long-term dynamics of native species in these refugia are poorly understood. Given the global prevalence of plant displacement by invasions, it is important to develop a general method for predicting how extinction debts may develop following invasions.

The metapopulation framework, which considers a network of isolated populations connected via dispersal, provides an excellent starting point for understanding the long-term consequences of invasions. When native populations are relegated to spatially isolated refugia, their long-term persistence is regulated by the colonization and extinction dynamics in their entire metapopulation (12). A large body of work suggests that even a partial loss of habitat in metapopulations, such as might arise from invasion, can deterministically drive the system to extinction (13, 14). However, due to slow colonization and extinction dynamics, this outcome often occurs many generations after habitat loss, generating an extinction debt in the meantime (15–17).

General metapopulation models indicate that reductions in colonization rates or increases in extinction rates reduce the viability of the metapopulation, and may therefore lead to an extinction debt (18). However, these models have yet to incorporate the mechanistic links between the local impacts of invasive species and the global persistence of the metapopulation. This prevents us from understanding the relative importance of different types of invader impacts on native persistence, and which native species will be most sensitive to these impacts at the metapopulation scale. In this study, we first present a theoretical model for understanding how extinction debts arise in invaded landscapes. We then parameterize and apply this model to understand the potential for invasive plant impacts on native annual plant persistence in a spatially heterogeneous serpentine soil landscape in California. Invasions are widespread in these landscapes, and they have likely reduced the connectivity and extent of local native patches within the broader metapopulation (9, 19). Serpentine landscapes support a disproportionate number of rare and threatened plant species, and therefore have a high conservation value (20). We show that invader impacts on the size and/or quality of native refugia and the permeability of the matrix between refugia can greatly reduce native plant metapopulation persistence and force extinction hundreds of years after the invasion is complete.

Model Framework and Application to the Focal System

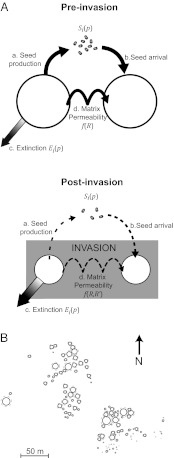
Our modeling framework builds on recent advances in metapopulation theory (13, 14) to quantify and partition invader impacts on the viability of native metapopulations in real landscapes. We assume that as invasions increasingly relegate native species to isolated patches (7, 9, 19), they can generate extinction debts via two main impacts (Fig. 1A): effects on patch size and effects on the dispersal permeability of the habitat matrix between patches. Reducing patch size reduces the number of seed-producing individuals, and thereby depresses the colonization probability in the metapopulation (Fig. 1A, arrow a). This impact is particularly severe when the invader eliminates the most favorable habitat. Reducing patch size also hinders colonization by making patches effectively further from one another (Fig. 1A, arrow b) and increases the stochastic extinction rate by reducing the number of individuals in a patch (Fig. 1A, arrow c).

Fig. 1.

Metapopulation viability in the study system following invasion. (A) Metapopulation dynamics preinvasion and postinvasion, with the width of arrows signifying the strength of the process. Invaders reduce colonization rates through decreased seed production and by altering competitor composition between patches (matrix permeability). Reduced local population sizes also increase local extinction rates. (B) Spatial layout of the study system. Black lines represent present-day distributions, and dashed gray lines represent one preinvasion scenario in which the habitat of native annuals was double the present-day area.

The second pathway of impact occurs when the invader alters the dispersal permeability of the matrix between suitable patches (Fig. 1A, arrow d). Although the matrix may not support stable populations, it may support transient sink populations. These sinks may provide critical intermediate steps for dispersal between patches by allowing many incoming seeds to produce a few plants whose seeds may then disperse onward in the next generation. Many plant species have extremely limited dispersal (9, 21); thus, this “multigenerational dispersal” can help overcome dispersal limitation.

To model these processes, we begin with methods developed by Hanski and Ovaskainen (13, 14) to analyze spatially explicit metapopulations with dynamics in discrete time. In what follows, we first describe the model and then show how one can obtain mathematical expressions for metapopulation persistence with this model structure. Next, we show how these expressions can be modified to incorporate the different ways invaders might affect native metapopulations (Fig. 1) and how their influence on metapopulation persistence can be numerically evaluated. We then apply these techniques to our field system to determine the impacts of invaders on the metapopulation dynamics of several focal species. Finally, we use simulations of the model to estimate times to extinction.

Consider a vector of patch occupancy probabilities, where each element corresponds to a specific patch in the metapopulation. The change in a species’ probability of occurrence (p) in patch i is the difference between the probability of patch colonization (C_i) and its probability of extinction (E_i):

We model the colonization probability as a Monod function that approaches one with high seed arrival from other patches []: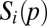

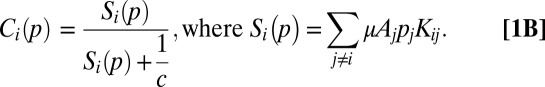Seed arrival to patch i is the sum of the contributions from all occupied patches j (p_j = 1). The contribution of each occupied patch j is the product of the number of seeds produced (seeds produced per unit area, μ, multiplied by patch area (A_j) and the probability of dispersing from patch j to i. This dispersal probability is defined by the dispersal kernel (K_ij), which is a function of the distance between patches and other metapopulation characteristics described below. The parameter c regulates how rapidly the probability of colonization increases with seed arrival.

We assume that the extinction probability, 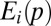, is inversely related to the size of the population in a patch:

Specifically, the extinction rate is the product of e, the extinction probability for a patch with a single individual; 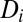, the inverse of patch population size; and 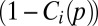, the probability the patch is not immediately recolonized (a rescue effect).

Returning to the colonization rate, the connectivity of the metapopulation is determined by the dispersal kernel, K_ij, which is influenced by the distance between patches (d_ij), the size of the recipient patch (the “target area”; Fig. S1), mean dispersal distance (σ), and the matrix permeability, measured as the annual plant’s finite rate of increase in the matrix (R; Fig. 1A, arrow d). Because species in our system can make seeds in the matrix habitat but not enough to replace themselves (0 < R < 1), some colonization of other patches might arise from multigenerational spread through the matrix. We therefore model dispersal as a random walk allowing a focal seed produced in patch j to disperse directly to patch i or to make offspring that land in the matrix but eventually disperse to patch i. Each step in the walk, apart from the initial dispersal from patch j, is taken with probability R [the average number of offspring per seed in the matrix (<1)]. The kernel that defines the per-seed probability of dispersing to focal patch i in exactly n generations (Q_n) can then be expressed (SI Materials and Methods, Dispersal function):

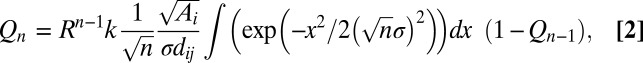where k is a normalization constant and the integral is evaluated over the range of d_ij ± radius_i. The probability of dispersing, K_ij, in any number of generations (1 to ∞) becomes .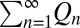

Having specified the model, we follow the approach of Hanski and Ovaskainen (13, 14) to analyze metapopulation persistence, the ability to recover from a drop to low patch occupancy. Doing so requires first defining a function g that describes the expected contribution of a patch to metapopulation persistence. Defined as the colonization probability (Eq. 1B) divided by the extinction probability (Eq. 1C) for patch i, g_i is somewhat analogous to a local growth rate that results from the colonization and persistence of immigrants from other patches in the metapopulation. We then build a (mathematical) matrix M, where each element (m_ij) is the partial derivative ∂g_i(p)/∂p_j evaluated at P = 0, in other words, how metapopulation “growth” from a low probability of occupancy in patch i changes with occupancy in patch j. For our model (Eq. 1), calculation of this partial derivative generates elements m_ij equal those of the spatially explicit Levins model (13, 14):

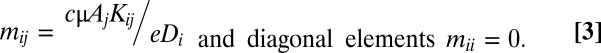To persist, the metapopulation must show increasing occupancy when occupancy drops to very low levels. This persistence criterion is met when the leading eigenvalue (λ) of M is greater than 1 (13, 14).

Having specified the persistence criteria for the metapopulation, we can now model how invasion effects on different parameters in the colonization and extinction functions (the various arrows in Fig. 1A) have an impact on metapopulation persistence. Specifically, we consider the effects of reduced refugia area, lowered seed density when invasion removes the most favorable habitat, and reduced dispersal permeability of the grassland matrix between refugia. When these three changes are incorporated into the colonization and extinction functions, and substituted into Eq. 3, the off-diagonal elements in M become:

Here, H_F is the fraction of habitat remaining after invasion, A signifies the preinvasion patch area, and 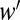is the seed density after invasion divided by before invasion (Table S1). R is the finite rate of increase in the matrix before invasion, and R′ is the R after invasion divided by before invasion (Table S2). Importantly, Eq. 4 can be partitioned into two multiplicative components: The first half incorporates the effect of invasion on native persistence through its impact on seed production, and the second half (the function f, which is the kernel K_ij following invasion) incorporates its impact on connectivity.

After incorporating these invader impacts, native plant persistence in the metapopulation is predicted when λ_postinvasion > 1. Empirically estimating λ, and thus predicting extinction debts, is challenging because the presence of an extinction debt precludes standard estimation techniques for metapopulations at equilibrium (12, 22), and several of the parameters required to parameterize λ accurately are difficult to attain precisely for most species. However, the criteria for metapopulation persistence can be expressed in terms of the ratio of λ′s preinvasion and postinvasion. This ratio does not depend on some of the parameters that are more difficult to measure (e.g., c, e), and it provides a continuous measure of the contribution of invasion to reduced persistence. A metapopulation enters an extinction debt when:

Combined with the determinants of λ in invaded and uninvaded systems (Eqs. 3 and 4), the ratio in Eq. 5 allows empiricists to scale the local impacts of invasion on patch size and matrix permeability to the expected proportional change in metapopulation persistence (λ) (the mathematics are presented in SI Materials and Methods, Incorporating invasion into the model). Whether this change is enough to force eventual extinction depends on the species’ spatially weighted patch occupancy before invasion, 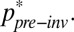p∗_pre−inv. Although our lack of knowledge of this value ultimately prevents us from identifying which species suffer from extinction debts, we can use the left-hand side of Eq. 5 to predict the impact of invasions on the degree to which metapopulations are buffered from extinction.

We used the model to predict the impacts of invasion on metapopulation persistence in an edaphically heterogeneous California landscape. The habitat is derived from serpentine parent material but is topographically heterogeneous, with rocky hummocks interspersed by more finely textured clay soils. Native annual forbs and native perennial grasses dominated this area before European annual grass invasion (9, 23, 24), but the forbs now occur on small rocky refugia, surrounded by a matrix of exotic grasses (8, 9, 19) (Fig. 1B). Species in similar habitats have previously been shown to exhibit colonization and extinction dynamics typical of metapopulations (25, 26), and native species’ distributions in our study area are consistent with predictions for metapopulations (Fig. S2).

To demonstrate that invasion has definitely driven extinction debts in a native community, one requires patch occupancy, colonization, and extinction dynamics before and after the invasion. Such data are simply unavailable for nearly all invaded systems. We argue, however, that the absence of such information should not prevent ecologists from exploring extinction debts in invaded landscapes, even if considerable uncertainty surrounds such efforts. To show how this might be accomplished, we field-parameterize the model for seven experimentally tractable focal annual plants (Table S3). We then predict the influence of invasion on their metapopulation persistence and explore the sensitivity of these results to variation in model parameters, such as loss of habitat to invasion and dispersal ability.

Some model parameters were assumed to be unaffected by invasion, including the spatial location of the centroid of suitable habitats, seed production per unit area in current refugia habitat (μ), and species’ dispersal distances (σ). Seed production per unit area, μ, was measured in plots sown with the focal species evenly spaced along transects through the refugia. Seed dispersal rates, σ, were parameterized with empirical relationships between dispersal distances, plant height, and dispersal syndrome from the literature (21), and were then validated with field data. According to published relationships, mean dispersal distances for our species range from 0.1 to 0.5 m, with the lower estimate for the shortest plant with no obvious dispersal mechanism and the higher estimate for the tallest of the wind-dispersed plants. Seeds trapped at various distances from parental populations at our field site confirmed these extremely low mean dispersal distances (SI Materials and Methods and Table S4). To predict invader effects on metapopulation persistence conservatively, we assumed a mean dispersal distance of 0.8 m (σ = 1) for all species but also explore more restricted dispersal (mean distance = 0.4 m, σ = 0.5; SI Materials and Methods).

The parameters affected by invasion were (i) the relative quality of the habitat from which the native species were displaced (which determines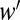), (ii) native per capita population growth rates in the matrix area between habitat patches (R′), and (iii) the area of each patch from which native species were displaced (A * H_F). To parameterize i, the relative quality of the habitat lost, we grew a community of the native annual plants in a refugia habitat and in a habitat adjacent to the refugia in plots where we experimentally removed exotic grasses.

Estimating how invasion altered native annual growth rates in the matrix and the size of each patch is more complicated. Although the details of the preinvasion landscape are uncertain, native bunchgrasses very likely dominated the matrix habitat between outcrops before exotic grass invasion. This common assumption for California grasslands (9, 24) is supported by the frequent occurrence of the native bunchgrass Stipa pulchra in the matrix habitat even today (19, 23). Thus, to estimate native annual growth rates in the matrix (R and R′), we measured the population growth rates of focal native annuals sown into matrix plots dominated by either European annual grasses or native perennials. Bunchgrass dominance of the matrix would mean that native forbs suffered the negative effects of a fragmented landscape even before invasion. Given that the native forbs themselves may have once dominated the matrix, our assumption conservatively predicts the impact of invasion.

In addition to changing the nature of the matrix, exotic grass invasion likely reduced the size of the rocky hummocks by invading their margins (9, 24); however, if it did so, the extent is unknown. We therefore explored a range of invasion scenarios. At one extreme, invasive grasses only displaced native bunchgrasses in the matrix between refugia, and therefore did not have an impact on refugia area (H_F = 1). At the other extreme, we assume that in addition to replacing the native bunchgrass matrix, European annual grasses encroached into the rocky hummocks from the margins, reducing their area by up to 50% (Fig. 1B, dashed vs. solid lines; H_F = 0.5). This second extreme means that less than 10% of the area currently occupied by nonnative plants would have formerly been native annual habitat.

Results and Discussion

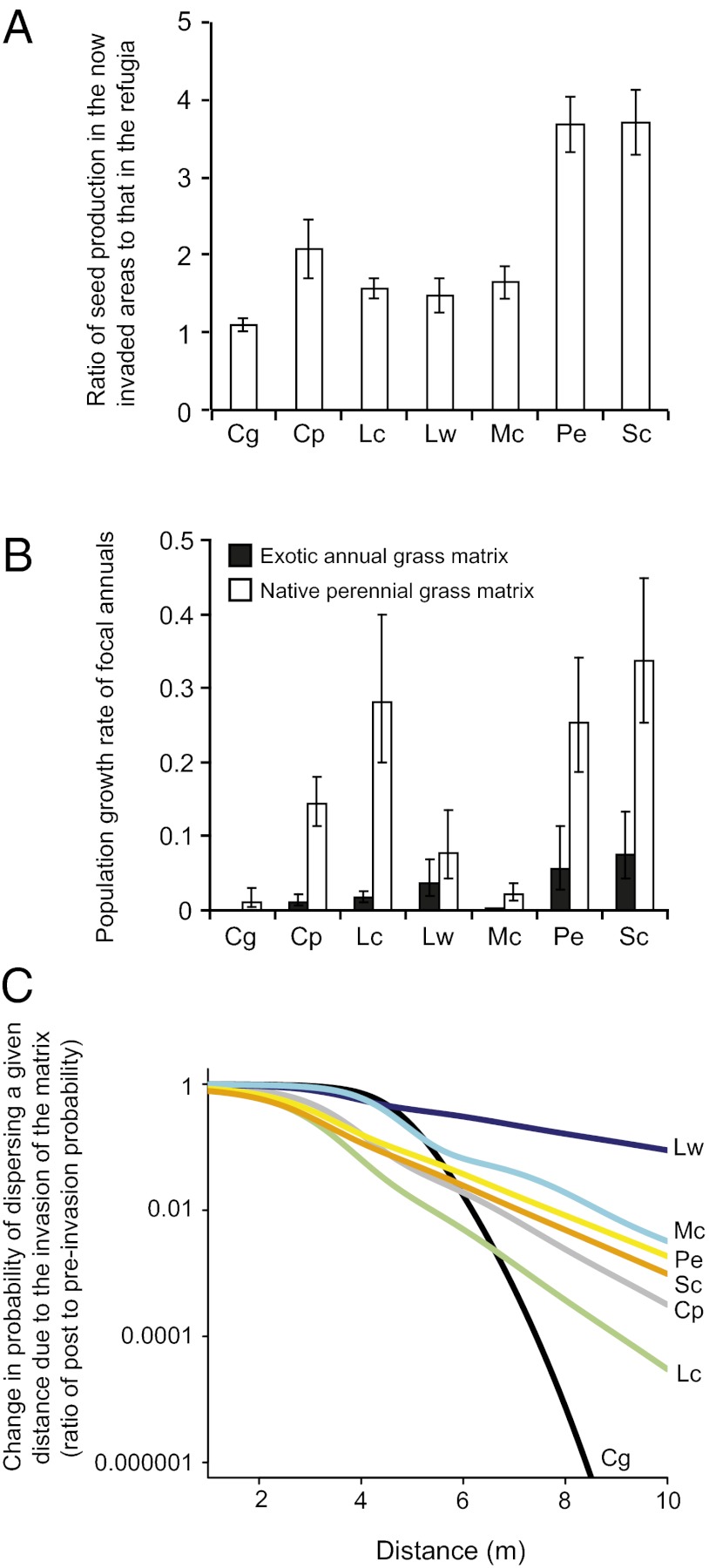
We found that finite rates of increase of native annual species were up to 3.5-fold greater in habitat now dominated by European grasses than in their current habitat (generalized least squares, P < 0.05; Fig. 2A and Table S1). This indicates that not only does European grass encroachment of the patches, to the extent that it occurs, remove habitat suitable to the focal native annual plant species but it removes what is otherwise superior habitat.

Fig. 2.

Impact of invasion on local population sizes and dispersal. (A) When competing only against other native annual species, the focal annuals have significantly higher finite rates of increase in the area now occupied by invasive grasses than in their current refugia. Bars show mean ratio ± SE, with ratios greater than 1 indicating that areas now dominated by invasive species are optimal for the native plants. (B) Native annuals had higher finite rates of increase among native bunchgrasses than among invasive grasses (mean R ± SE) in the matrix habitat. Data are not presented as ratios because the finite rate of increase of Chaenactis among exotic grasses was zero. (C) Lower finite rates of increase of native annuals in invaded habitat greatly reduce connectivity by decreasing multigenerational dispersal through the matrix. Curves show the effect on the probability of dispersal of lowering species’ finite rates of increase in the matrix after invasion, given a mean dispersal distance of σ = 1. The effect of dispersal through the matrix is calculated assuming that offspring from a parent plant could not persist for more than 30 y in the matrix (i.e., n_max = 30 in Eq. S5). Cg, Chaenactis galibriuscula; Cp, Chorizanthe palmerii; Lc, Lasthenia californica; Lw, Lotus wrangelianus; Mc, Micropus californicus; Pe, Plantago erecta; Sc, Salvia columberiae.

Even if preinvasion patches were double in size, a large grass matrix remains (Fig. 1B). However, the ability of the focal annual plants to disperse through the matrix, and thereby colonize other patches, was greater before invasion. We found that all native annual plants had finite rates of increase less than 1 in matrix plots dominated by native perennial bunchgrasses, the presumed former dominant (Fig. 2B). Thus, before European grass invasion, much of the matrix between patches was too competitive for the persistence of the focal species. However, finite rates of increase (R) were significantly higher among native bunchgrasses than invasive grasses (mean: R = 0.16 and R = 0.03, respectively; Fig. 2B and Table S2). Because the dispersal permeability of the matrix depends on these growth rates (Eq. 2 and Eq. S5), parameterizing Eq. 2 with R values preinvasion and postinvasion suggests that European grass invasion of the matrix alone imposes an order of magnitude reduction in the probability of colonizing a patch only several meters away (Fig. 2C and Fig. S3).

We can partition invader effects on metapopulation persistence (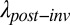/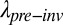) into the multiplicative effects of reduced seed production (affecting both colonization and stochastic extinction) and reduced connectivity (Eqs. 4 and 5, Fig. 3, and Eq. S6B). We found that for all species and degrees of area loss, the reduction in metapopulation persistence due to reduced connectivity (red line in Fig. 3) was greater than the reduction due to lost seed production (blue line in Fig. 3, which lies above the red line in all panels of Fig. 3). Of note, the y intercept of the red line (zero patch area lost) shows the effect of reduced landscape permeability caused by the replacement of the native bunchgrass matrix with European grasses in the absence of any change in patch size, one of the invasion scenarios for this system. This effect reduces metapopulation persistence by up to an order of magnitude, and it was variable across species (Fig. 3 and Fig. S4). It was strongest for species like Lasthenia, which grew much better in the matrix with native perennial bunchgrasses than with European annuals. The negative slope to the connectivity line reflects the effect of increasing isolation of patches as their area is lost. Holding connectivity constant, the metapopulation persistence of all species declined with the reduced seed production associated with habitat area loss (blue line in Fig. 3). This effect was most severe for species like Plantago and Salvia (Fig. 3 E and F) that grew relatively well in the lost habitat area (Fig. 2A).

Fig. 3.

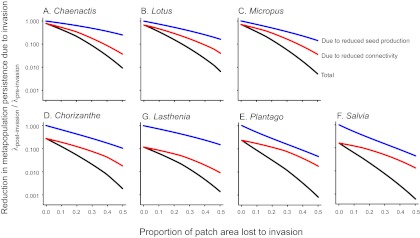
Impact of loss of seed production (blue line) and reduction in habitat connectivity (red line) on metapopulation viability (black line; joint contribution) for the seven focal species. Seed production alters both colonization and extinction dynamics, whereas loss in connectivity results from a reduction in habitat size and alteration of dispersal through the matrix. Panels correspond to the species listed by genus (full names are given in Fig. 2).

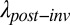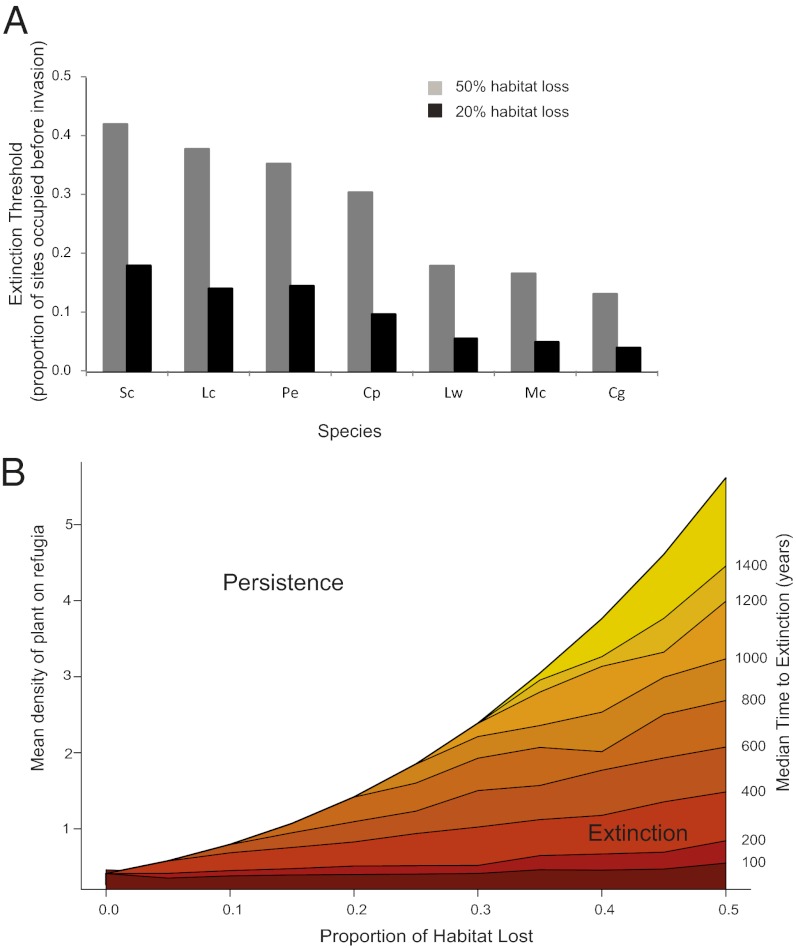
We can also explore the long-term impact of invasion under the scenario in which European grasses replace the native bunchgrasses in the matrix and also reduce the size of the native annual patches to varying degrees. Assuming a 50% loss of habitat due to European grass invasion, these collective invader impacts reduced metapopulation persistence (/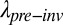; Eqs. 4 and 5 and Eq. S6B) by two to more than three orders of magnitude for the seven focal annual plants (black lines in Fig. 3 and Fig. S4). Although their preinvasion patch occupancy is unknown, all would persist if they occupied more than 45% of patches before invasion and none would persist having occupied only 10% of patches (Fig. 4A using Eq. 5). If we assumed that grass invasion reduced patch area by only 20%, we still predict a roughly one order of magnitude decline in metapopulation persistence; all populations with more than 18% preinvasion occupancy would persist (Fig. 4A). Given that most metacommunities consist of species that occur in a low proportion of potential sites (26–28), the local extinction of many native plant species is likely when invader impacts are as great as seen in this ecosystem.

Fig. 4.

Extinction thresholds and times to extinction for species in an extinction debt. (A) Extinction threshold is the minimum proportion of habitat that a species must occupy before invasion to avoid falling into an extinction debt following invasion. Estimates are based on incorporating both species-specific responses to invaders (black lines in Fig. 3) and the level of habitat loss shown into Eq. 5. Species abbreviations are given in Fig. 2. (B) Median time to extinction for an average species with a given mean density and proportion of habitat lost to invaders. Estimates are based on simulations for an “average” species containing the mean trait values of the seven focal species.

Next, we show that for species that cannot persist with invasion, their time to extinction can still be on the order of hundreds of years in this landscape. Because the model used does not predict times to extinction, we used simulations to generate extinction time lines for an “average” species following invasion (SI Materials and Methods, Model Simulations and Numerical Solutions). This average species possesses the average of all demographic rates from the seven common taxa but not their local density, which we varied in our simulations (Table S5). Consistent with earlier results, we found that only some combinations of patch area loss and local density led to extinction (Fig. 4B). When the species fell below the extinction threshold (Eq. 1), extinction happened rapidly if the species was sparse and the habitat loss too great. However, for a wide range of habitat area loss and population densities, times to extinction were long, upward of several hundred years (Fig. 4B). Long extinction times after habitat destruction are characteristic of many metapopulations (18) and were also found with very different values of c and e, two model parameters that we can only approximate (SI Materials and Methods).

Finally, we discuss the sensitivity of our metapopulation persistence predictions to the uncertainty that naturally arises with many of the parameters. The estimated dispersal distances were short, which can greatly affect the impact of connectivity (compare Figs. 2C and 3 with Figs. S3 and S4). We therefore calculated patch connectivity with upper bound dispersal distances that conservatively estimate the impact of changes in connectivity (Figs. 2C and 3) and with estimated mean dispersal distances (Figs. S3 and S4). Increasing the mean dispersal distance significantly increases metapopulation viability, more so than changing the dispersal kernel to one with a “fatter tail” (SI Materials and Methods, Model assumptions). Similarly, uncertainty in the fraction of the patch area lost motivated us to explore the effects of a range of plausible losses of patch area, and results differ as shown in Figs. 3 and 4. Our inclusion of seven focal species, all with their own vital rates (Fig. 2 A and B and Tables S1 and S2), also gives an indication of how results vary across parameter combinations found for species in the system. Finally, the sensitivity of extinction debt time lines to parameters c and e was explored. In several cases, the sensitivity analyses indicate consistent predictions across a range of parameter values (e.g., Fig. S5). We found, for example, a greater effect of reduced connectivity vs. reduced seed production on metapopulation persistence in invaded landscapes for all focal species, regardless of the mean dispersal distance incorporated (Fig. 3). Other results were more sensitive to parameter values, as suggested by the variation among species in their overall sensitivity to invader impacts (Fig. 3 and Fig. S4). A final source of uncertainty arises from the fact that our study examines only a subset of the processes that negatively affect native metapopulations following invasion. Other factors, such as large-scale environmental stochasticity, demographic stochasticity at the scale of the entire metapopulation, and changes to pollinator dynamics following greater fragmentation, should all exacerbate the effects that we report (29).

We conclude that plant invasions relegating native populations to isolated patches can greatly reduce their metapopulation viability. Even under low levels of invasion, most species in our system that occupy less than 10% of patches may enter an extinction debt (Fig. 4A). In studies of metapopulations around the world, plant species most commonly fall into this low-occupancy range (28). Moreover, these extinction debts may take hundreds of years to play out. In a world with a rapidly changing climate, it is tempting to regard invader impacts that occur with 100-y time lags as a lower priority concern. However, invasions that reduce metapopulation viability by limiting connectivity or local population size may exacerbate the effects of climate change because these factors also limit opportunities for migration (30) and evolution (31), which are key processes for persistence in a changing world. Recent suggestions that plant invasions fail to drive native plant extinctions may be premature.

Materials and Methods

We conducted experiments in an 8-ha area at the northern edge of the Sedgwick Reserve (34° 44’ 20” North, 120° 01’ 34” West). The area has a natural metapopulation structure, with refugia of native annual plants occurring on slightly raised mounds with coarse soils (9, 19). We selected seven native annual species that were abundant enough to provide sufficient seed for our experiments (species are listed in Fig. 2 and SI Materials and Methods). The area between refugia is almost completely covered with exotic grasses, mainly Avena fatua, Avena barbata, and Bromus sp. Pockets of native bunchgrasses (mainly S. pulchra) persist in small patches among invasive grasses. Initial categorization of the landscape was performed using images from Google Earth, according to the method of Gram et al. (19). We subsequently performed detailed mapping of a portion of the site using a global positioning system and ground measurements, and we used geographic information system (GIS) tools (ArcGIS) to calculate patch areas and centroids; the resulting detailed map (Fig. 1B) was used for all analyses.

Habitat Quality Experiment.

We evaluated the relative seed production in current refugia vs. invaded habitat by sowing 3 g⋅m⁻² of native seed per species into 20 × 20-cm plots cleared of competitors on refugia and also immediately adjacent to refugia in invaded habitat. In total, we had 96 plots (48 in each habitat type) distributed across 12 of the larger refugia in the study area. We used half of the plots in each habitat type to estimate germination rates and the other half to estimate per capita seed production. Finite rates of increase were calculated for each sown species by summing its seed production and the carryover of ungerminated seeds in the seed bank (SI Materials and Methods) and dividing through by the seeds added. Seed bank carryover was the product of the number of added seeds, one minus the germination rate, and the seed survival fraction (estimated by measuring seed viability before and after a year of burial in mesh bags). Mean seed density on refugia (µ) was estimated for each species from the sum of seed production and seed bank carryover in refugia plots.

Matrix Permeability Experiment.

Matrix permeability (R) was estimated by sowing 3 g⋅m⁻² of seed per species into 10 sets of paired plots that were placed less than 1.5 m from each other. Two plots were placed in each type of grass (native perennial or exotic annual) at each location: high-density plots, sown with seed densities from natural refugia, and low-density plots in which only small numbers of native annual seed were added. Because the two densities gave similar results, they were combined for analysis. We estimated R as the seed production in each plot divided by the number of seeds added. Results from matrix permeability and habitat quality experiments were first tested with nested distance-based permutation multivariate ANOVA and, following significant results, with separate generalized linear mixed models for each species.

Seed dispersal rates were first estimated from well-established relationships between dispersal distance, plant height, and dispersal syndrome (21). We used two empirical methods to test the validity of these estimates. We created “false refugia” by clearing circular 50-m² areas of invasive grasses, with edges ranging from 0.5 to 7 m from the nearest refugia. Germinants of our focal species were counted the year after these refugia were created. In addition, we chose two refugia that contained all species and placed seed traps (28 × 52 cm, 92 seed traps total) at distances up to 8 m from the refugia edge.