Evidence of recovery of Juniperus virginiana trees from sulfur pollution after the Clean Air Act

Significance

This study shows that red cedar trees growing in the Central Appalachian Mountains of West Virginia are recovering from decades of acidic pollution. Our study shows the efficacy of the Clean Air Act in a region where acidic pollution levels were some of the highest in the United States before the Clean Air Act. We demonstrate that a large portion of the increase in water use efficiency of trees that is often attributed to increasing atmospheric CO₂ over the last century may be caused by acid deposition. This study has important implications for carbon cycling in forests, showing an interaction between decreasing SO₂ emissions and increasing CO₂ that is not currently accounted for in biosphere–atmosphere models of climate change.

Abstract

Using dendroisotopic techniques, we show the recovery of Juniperus virginiana L. (eastern red cedar) trees in the Central Appalachian Mountains from decades of acidic pollution. Acid deposition over much of the 20th century reduced stomatal conductance of leaves, thereby increasing intrinsic water-use efficiency of the Juniperus trees. These data indicate that the stomata of Juniperus may be more sensitive to acid deposition than to increasing atmospheric CO₂. A breakpoint in the 100-y δ¹³C tree ring chronology occurred around 1980, as the legacy of sulfur dioxide emissions declined following the enactment of the Clean Air Act in 1970, indicating a gradual increase in stomatal conductance (despite rising levels of atmospheric CO₂) and a concurrent increase in photosynthesis related to decreasing acid deposition and increasing atmospheric CO₂. Tree ring δ³⁴S shows a synchronous change in the sources of sulfur used at the whole-tree level that indicates a reduced anthropogenic influence. The increase in growth and the δ¹³C and δ³⁴S trends in the tree ring chronology of these Juniperus trees provide evidence for a distinct physiological response to changes in atmospheric SO₂ emissions since ∼1980 and signify the positive impacts of landmark environmental legislation to facilitate recovery of forest ecosystems from acid deposition.

A key uncertainty in coupled biosphere–atmosphere models of climate change is the long-term effects of increasing atmospheric CO₂ on carbon uptake and storage in terrestrial ecosystems (1, 2). Forest ecosystems play a fundamental role in the global C cycle; they contribute ∼50% of terrestrial net primary production (3, 4), account for ∼45% of terrestrial C (5), and are a major part of the terrestrial C sink that removes nearly 30% of anthropogenic C emissions each year (6). Consequently, state-of-the-art climate models require a mechanistic understanding of how simultaneous changes in key environmental variables affect C cycling in trees and forest ecosystems. Although experiments have examined how numerous environmental factors affect tree growth and forest productivity, accurately forecasting future tree growth and C cycling requires a better understanding of complex environmental interactions across spatial and temporal gradients in natural systems.

For over a century, the combustion of fossil fuels resulted in the widespread alteration of the composition of the atmosphere. For example, though atmospheric CO₂ concentrations increased by almost 40% since the mid-19th century and are continuing to increase at an unprecedented rate (7, 8), SO₂ emissions in the United States also increased rapidly initially but then declined following US environmental legislation (the Clean Air Act) in 1970 and its subsequent amendment in 1990 (Fig. 1) (9, 10). Although the direct effects of elevated CO₂ are positive for plants, generally increasing photosynthesis and decreasing water loss by reducing stomatal conductance (11), SO₂ emissions are transformed into acidic deposition (Fig. S1), which has numerous negative impacts on ecosystem productivity (12).

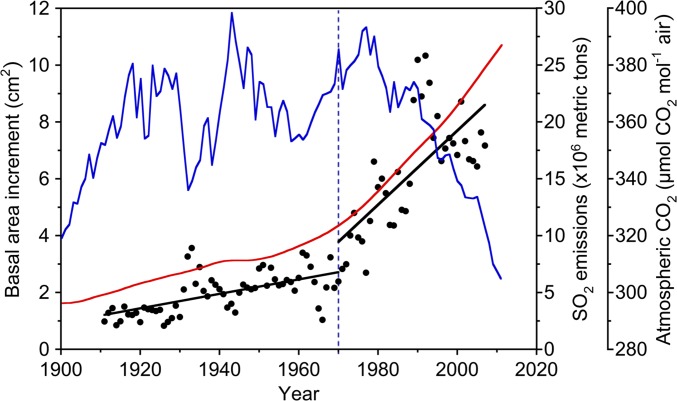
Fig. 1.

Basal growth of 118- to 480-y-old J. virginiana trees in a stand located in the Central Appalachian Mountains over the past century (n = 5 trees). The vertical blue dotted line represents the approximate date of the Clean Air Act (1970). Before 1970, y = 0.025x − 47.4, r² = 0.38, P < 0.0001; after 1970, y = 0.130x – 253.1, r² = 0.49, P < 0.0001. The slopes of the relationships between BAI and year before and after 1970 are different (P < 0.0001). Superimposed over BAI are trends of atmospheric CO₂ concentrations (red line) (7, 8) and historical sulfur emissions in the United States (blue line) (9, 10).

The Central Appalachian Mountains have historically received some of the highest rates of acid deposition in the United States due to their downwind proximity to the abundance of coal-fired power plants in the Ohio River Valley (12). Despite these historical rates of acid deposition and the potentially long-lasting effects on ecosystems, there are many examples of tree species in the temperate deciduous forest in the eastern United States that have shown recent unexplained increases in growth (13–17). In this study, we used dendroisotopic techniques to examine simultaneous environmental influences on Juniperus virginiana trees growing in the eastern panhandle of West Virginia. Using this approach, we were able to estimate how key physiological processes have changed across the past century, and the extent to which temporal changes in atmospheric CO₂ concentrations and acid deposition are responsible.

Results and Discussion

Here, we found that the basal area increment (BAI) of Juniperus in an old-growth stand in West Virginia has increased significantly since the enactment of the Clean Air Act of 1970 (Fig. 1), despite being older (118–480 y old) than the age of trees that normally exhibit rapid growth (18, 19). A recent tree ring chronology of J. virginiana, incorporating our site and others in this region, indicates an increasing growth trend for these trees since the late 20th century that is greater than any other time over the past 450 y (20). We performed a multivariate correlation analysis using historical climate variables (21), atmospheric CO₂ concentrations (7, 8), and US SO₂ and NO_x emissions (9, 10), and found that the growth of these Juniperus trees over the last century is explained best by increases in atmospheric CO₂ and NO_x emissions, and decreases in SO₂ emissions (Table S1).

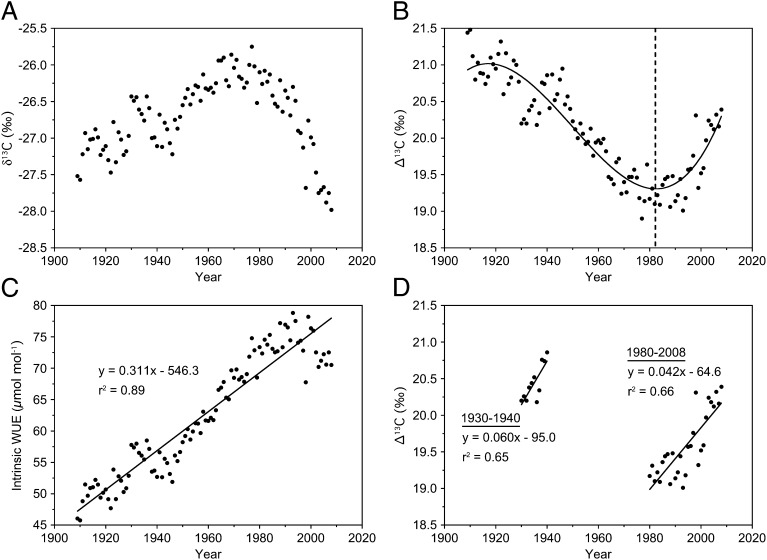
To examine changes in leaf physiology that influence tree growth over time, whole wood from tree rings from the years 1909–2008 was analyzed for δ¹³C (Fig. 2A), corrected to account for the differences between leaf and wood isotopic signatures, converted to ∆¹³C (isotopic discrimination) to account for the anthropogenic increase in isotopically light atmospheric δ¹³CO₂, and used in standard equations to calculate leaf physiological characteristics (22). We found a striking nonlinear trend in ∆¹³C (Fig. 2B) that indicates a fundamental shift in foliar gas exchange of these Juniperus trees, resulting in a 66% increase in intrinsic water use efficiency (iWUE), or the ratio of carbon gained through photosynthesis (A) to the stomatal conductance to water (g_w), over the 100-y time period (Fig. 2C). This isotopic signal indicates a progressive reduction in the discrimination against the heavy C isotope across the tree-ring chronology until later in the 20th century, when increased isotopic discrimination resulted in a lighter C isotopic signature being incorporated into the wood (Fig. 2B). We fit a third-order polynomial function to the sequence of ∆¹³C to estimate the year when the isotopic trend changed from enrichment to depletion of the heavy C isotope (y = 0.00001227x³ − 0.07179x² + 139.9x – 90869; r² = 0.86, P < 0.0001). The breakpoint was identified as 1982, which corresponds to the approximate time when SO₂ emissions began to decline in the United States. This result was surprising because it is counter to the widely observed stomatal response of plants to increasing CO₂ in the 20th century (11, 23). Here, decreasing ∆¹³C before ∼1980 suggest stomatal closure as if the trees were responding to increasing atmospheric CO₂, yet the reversal and increases in ∆¹³C after 1980 suggest reduced stomatal regulation to CO₂ (Fig. 2B). Similar isotopic trends in trees growing in the northern hemisphere have been observed by other investigators who have suggested that these recent shifts in δ¹³C are indicative of a physiological change in guard cells where stomata become uncoupled from CO₂ concentration as atmospheric CO₂ began to increase rapidly in the 1980s (24). After converting ∆¹³C to C_i/C_a (Fig. S2A), the ratio of internal leaf CO₂ to atmospheric CO₂, a metric of leaf physiology that provides a relative assessment of the stomatal limitation of photosynthesis, it is clear that stomatal limitations for these Juniperus trees increased until ∼1980, at which point the trend reversed and stomatal limitations to CO₂ uptake are reduced. Several lines of evidence point to the declining levels of SO₂ emissions around the 1980s that drive these stomatal responses in Juniperus rather than a decoupling of stomatal sensitivity to atmospheric CO₂.

Fig. 2.

(A) Chronology of leaf-corrected δ¹³C from tree rings of J. virginiana, (B) ∆¹³C, (C) iWUE derived from C_a and C_i, and (D) a comparison of ∆¹³C from 1930 to 1940 and after 1980. The vertical dashed line at 1982 in B represents the year predicted from a third-order polynomial where the shift in ∆ occurs.

The first line of evidence is the close correspondence between the timing of the change in direction of C_i/C_a with the decline in SO₂ emissions that occurred in the United States following the Clean Air Act. The directional change in the isotopic signal follows reductions in SO₂ emissions that began in the 1970s, but precedes reductions in NO_x emissions by over a decade (Fig. S3) (10). Although changes in tropospheric ozone might also impact C_i/C_a over time, a direct assessment of the influence of O₃ is not possible because historical records of O₃ are not available for the same time period as our tree-ring chronology. Regardless, because NO_x is a precursor to O₃ (25), it is reasonable to assume similar historical patterns of O₃ and NO_x, and because contemporary historical NO_x emissions do not show a breakpoint around the year 1980, it is unlikely that changes in O₃ explain the pattern of C_i/C_a in our tree-ring chronology. In addition, the open canopy and relatively high light levels of this Juniperus/hardwood forest suggests that canopy closure, or increased shading, around 1980 was not likely the cause for this chronology of C_i/C_a. Likewise, we found no evidence of fire, timber harvesting, or any other type of disturbance that may have increased the light levels in this forest, thereby influencing the isotopic signatures of the tree rings. There has been an increase in yearly precipitation in this region in West Virginia over 1909–2008 (∼1.2 mm⋅y⁻¹; P < 0.012), and there has been a trend toward warmer temperatures (∼0.4 °C; P < 0.057), but year-to-year variability of both of these climatic factors were high (Fig. S4). If these changes in climate were responsible for patterns of C_i/C_a, we would expect a gradual change in the isotopic signature over the entire century rather than the observed breakpoint at ∼1980.

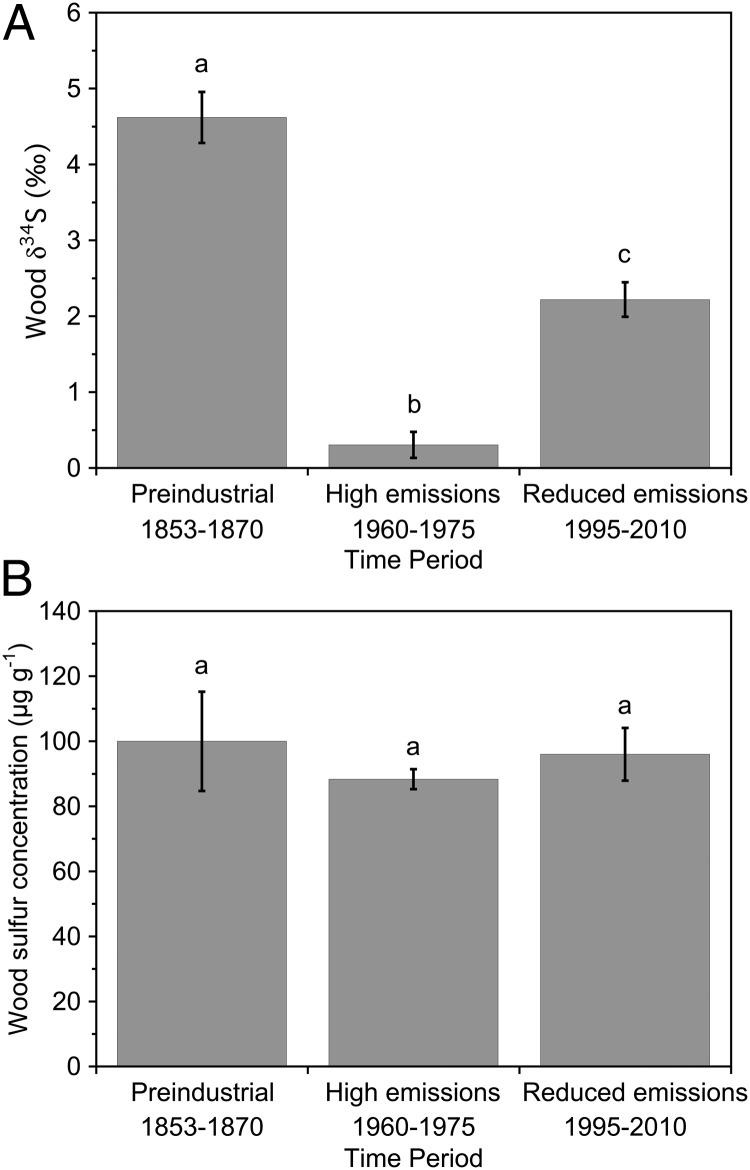
To further examine the consistency between the timing of the increase in C_i/C_a in the tree rings of Juniperus with the timing of the decline in US SO₂ emissions, we compared δ³⁴S in tree rings formed during preindustrial years to δ³⁴S in tree rings before and after 1980 (Fig. 3). Wood δ³⁴S is infrequently used in dendroisotopic studies because of the very low concentration of S in wood (26, 27). Sulfur can enter the plant through their roots or leaves, but either route of uptake results in very little fractionation when S is incorporated into biomass, allowing δ³⁴S to typically reflect the sources of S incorporated by the tree (28). In this study, the sources of S used by these trees over the tree-ring chronology are unknown, but we assume that the δ³⁴S from 1853 to 1870 shows little anthropogenic influence. The large depletion of the heavy S isotope between the preindustrial period and 1960–1975, as US SO₂ emissions were increased by ∼260% compared with the mid-1800s (9), reflects a strong anthropogenic S input at the same time that δ¹³C indicates a decline in leaf C_i/C_a. The increase in δ³⁴S between 1960–1975 and 1995–2010, as US SO₂ emissions declined by ∼41% (9, 10) and SO₄ deposition declined by ∼66% (29), indicates a reversal back toward preindustrial δ³⁴S signatures and a reduced anthropogenic influence. Thus, just as the tree ring δ¹³C after ∼1980 indicates C_i/C_a increased at the leaf level, tree ring δ³⁴S indicates a synchronous change in the sources of S used at the whole-tree level. Sulfur concentrations in wood were constant across the chronology despite large changes in SO₂ emissions, reflecting the fixed physiological processes that regulate S deposition in wood, as has been shown previously (27).

Fig. 3.

Mean (A) δ³⁴S and (B) S concentrations of J. virginiana tree rings from three time periods: 1853–1870, 1960–1975, and 1995–2010. Error bars denote ±1 SE; n = 3 for 1853–1870, and n = 5 for 1960–1975 and 1995–2010. Values designated by the same letter are not different at the 0.05 level of significance, using a Tukey–Kramer HSD post hoc test.

The second line of evidence is that the changes in the C isotopic signature of Juniperus tree rings late in the 20th century were not unique to this time period. Instead, the patterns of change in δ¹³C, ∆¹³C, and C_i/C_a after 1980 were not significantly different from the isotopic signatures found during the 1930s, when SO₂ emissions were sharply reduced during the US Great Depression (Fig. 2D and Fig. S5). As with the C isotopic signatures after 1980, no other environmental factors explain the observed changes during the 1930s. In addition, the growth of these Juniperus trees during 1930–1940 was inversely correlated to SO₂ emissions (y = −0.46× + 6.48; r² = 0.72, P < 0.0009), suggesting that the trees experienced a short-term recovery from acid deposition during the Great Depression years (Fig. S6).

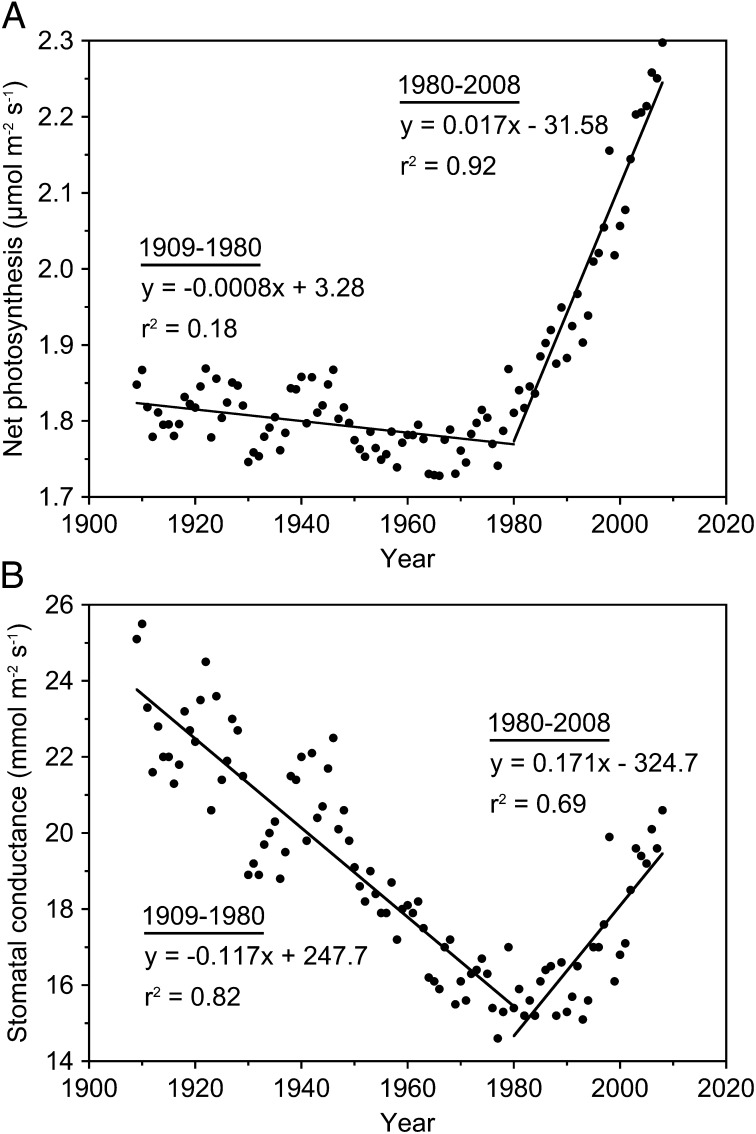
The third line of evidence is from the chronology of stomatal conductance to CO₂ (g_c) of the Juniperus foliage inferred from tree ring C isotopes. Acid misting experiments and field-based isotopic studies have indicated that SO₄ deposition affects stomatal function, including reducing g_c of leaves (30–32). Based on these studies, we postulated that g_c would decrease before 1980 when SO₂ emissions were high, but would subsequently increase in response to reductions in SO₂ emissions. The chronology of C_i/C_a suggests a strong link between increased SO₂ emissions and the regulation of stomata, but C_i/C_a incorporates both A and g_c. To evaluate drivers of C_i/C_a independently, we performed a simulation analysis of changes in A and g_c using foliar C_i derived from tree ring ∆¹³C of the Juniperus trees (Fig. S2B), atmospheric CO₂ concentrations (7, 8), and the physiological relationship between light-saturated A and C_i of Juniperus foliage measured onsite.

The chronology of A and g_c in Juniperus shows that A changed very little from 1909–1980 (a 3% decline), whereas g_c decreased by 35% over the same time period (Fig. 4 A and B). After 1980, large increases in both A (27%) and g_c (33%) occurred. Increases in A after 1980 were correlated with increases in atmospheric CO₂ and decreases in SO₂ emissions (Fig. S7 A and B). Likewise, g_c of Juniperus leaves after 1980 increased as SO₂ emissions declined, despite increasing atmospheric CO₂ (Fig. S7 C and D). Additionally, during 1930–1940, A increased 5.9% and g_c increased 14.9% (Fig. S8), providing further support to consider that changes in the isotopic signal of Juniperus tree rings late in the 20th century and during the 1930s are both related to the same phenomenon. The chronology of A and g_c binned by decade reveals that the only two time periods when there were net positive changes in A and g_c of Juniperus were during the 1930s and after the 1980s (Table 1), and these correspond to time periods when increases in growth of the trees were observed (Fig. 1). During both of these time periods, g_c increased to a greater extent than A, explaining why iWUE declined during the 1930s and after ∼1990 in the tree ring chronology (Table 1 and Fig. S5D).

Fig. 4.

Simulated seasonally integrated (A) net photosynthesis and (B) stomatal conductance of J. virginiana trees across the tree ring chronology.

Table 1.

Changes (%) in the photosynthetic variables (PV), net photosynthetic rates (A) and stomatal conductance to CO₂ (g_c) of J. virginiana trees over the 100-y chronology derived the simulation analysis using C_i from tree-ring isotopic data, atmospheric CO₂ (7, 8), and the relationships between A and C_i measured on J. virginiana foliage in 2009

PV	1909–1920	1920–1930	1930–1940	1940–1950	1950–1960	1960–1970	1970–1980	1980–1990	1990–2000	2000–2008
A	−1.9	−2.0	5.9	−2.5	0	−1.7	0	5.9	10.5	11.1
gc	−11.3	−10.8	14.9	−7.5	−5.6	−12.1	−4.6	3.1	15.1	18.4

Despite decreased iWUE of Juniperus during the 1930s and after ∼1990, iWUE increased 66% over the entire chronology (Fig. 2C) due to a 23% increase in A and an 18.5% decrease in g_c. Many dendroisotopic studies have concluded that the small but progressive increases in atmospheric CO₂ over the last century have improved iWUE (23, 33), and suggest that a fundamental way that increasing atmospheric CO₂ stimulates tree growth and forest productivity is by improving the efficiency that trees are using water. Here, acid deposition over many decades contributed to a large portion of the increase in iWUE of Juniperus, and these data signify that the effects of increasing CO₂ on iWUE can be greatly overestimated if other environmental influences are not considered in these estimates. The mechanisms for increased iWUE over the entire chronology differed between 1909–1980 (small decreases in A, large decreases in g_c) and 1980–2008 (greater increases in A than g_c initially, but followed by greater increases in g_c than A ∼1990, whereby iWUE decreases; Table 1). If we assume that the stomatal response between 1909–1980 incorporates stomatal closure due to both a CO₂ response and a response to SO₄ deposition (resulting in a 35% decline in g_c), and after 1980 there is recovery from SO₄ deposition, then the difference in g_c between 1909–2008 reflects the effect of increasing CO₂ (a 18.5% decline in g_c; Fig. S9). Thus, if the recovery of g_c from the effects of acid deposition was complete by 2008, it appears that the effects of SO₄ deposition on g_c of Juniperus between 1909–1980 were greater than that of atmospheric CO₂ during the same time period.

SO₂ emissions and acid deposition have been effectively reduced in the United States since the Clean Air Act was enacted, but despite this, acid deposition remains a considerable problem in the United States, as well as other locations globally (34). Though aquatic ecosystems in the United States have shown some improvements as acid deposition has declined (34, 35), recovery of terrestrial ecosystems is expected to be much slower than aquatic systems, and could take decades (12, 36, 37). Our findings indicate that the productivity of this stand of Juniperus growing in the Central Appalachian Mountains is recovering from acid deposition, and confirm the prediction that recovery of trees occurs over long periods of time. It is clear that it will be important to examine the generality of these results from Juniperus to understand how widespread these responses to reduced SO₂ emissions are among co-occurring tree species within eastern temperate forest communities (38). Regardless, the recovery of Juniperus from long-term acid deposition involves gradual stomatal opening over several decades, and thus provides evidence for a physiological response to reductions in SO₂ emissions that is distinct from the response to increased atmospheric CO₂. The slower rate of increase in g_c, but faster rate of increase in A, that occurred after 1980 compared with the short-term recovery during the 1930s (Fig. S8 A and B) likely reflect the widely observed effects of increased CO₂ concentrations on g_c and A (11). If we consider A as a diffusive process described by Fick’s law, where 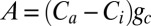, then as the recovery from acid deposition of these Juniperus trees occurs, carbon uptake is enhanced by both increased C_a as atmospheric CO₂ increases and increased g_c as SO₂ emissions decline. Taken together, these results highlight the complexities of significant interactions between atmospheric CO₂ and acid deposition on stomatal conductance, photosynthesis, and tree growth that have important consequences for forest C cycling in many northern hemisphere forests.

Materials and Methods

Tree Selection and Growth Measurements.

The study site is a stand of J. virginiana (red cedar) trees located along the south branch of the Potomac River in southern Grant County, WV, on a northwestern facing limestone outcrop located above Smoke Hole Canyon (38° 53′02′′ N 79° 14′12′′ W; 670 m above sea level). This site is in the Ridge and Valley Physiographic Province of northeastern West Virginia and has been defined as a cedar glade (39). The soil in this glade woodland has little organic matter and is primarily composed of broken limestone. In addition to Juniperus trees, the overstory vegetation is comprised of Quercus muehlenbergii, Quercus alba, Fraxinus americana, and Juglans nigra, and the open overstory allows most trees to receive high sunlight throughout the day.

Five Juniperus trees were randomly selected along a transect running parallel to the bluff at least 6 m from the edge. An exception to the random sampling was that trees with obvious strip bark were avoided. Tree rings used in this study were obtained in 2008. To minimize bias from uneven radial growth, we took two increment cores from each tree parallel to the contours of the slope and recorded the diameter at 1 m above ground level. Increment cores were returned to the laboratory, processed according to standard dendrochronological techniques (40), and cross-dated using both WinDENDRO (Regent Instruments Inc.) and a master chronology created previously for the site using >20 Juniperus trees (20). All tree-ring series correlated with the master chronology, having interseries correlations >0.50. The expressed population signal, a measure of the common variance in a chronology, was >0.75 for the current residual chronology for the duration of the 20th century, suggesting that the trees had similar growth patterns. Once each core was measured, cross-dated, and statistically validated, we assigned a calendar year to each annual tree ring. The five trees were aged to be 119, 138, 166, 308, and 480 y old. Because tree-ring width declines as trees mature, and growth trends are not readily detectable based on changes in ring widths alone, we converted the cross-dated tree-ring series into annual BAI to characterize growth trends from 1911 to 2007 using

where R is tree radius for a given year and n is the year of ring formation.

Carbon Isotopic Analysis of Tree Rings.

Tree rings for years 1909–2008 were collected for isotopic analysis by scalpel dissection of cores under a dissecting microscope at the boundary of late wood and early wood. Wood samples were cut to 1 mg and packed into tin capsules for carbon isotopic analysis. Within years, earlywood and latewood were combined for sample analysis to ensure enough material for peak detection in the isotopic analysis. Whole-wood samples were analyzed for δ¹³C with a ThermoFinnigan ConFlo III interface and Finnigan Delta-plus Continuous Flow Stable Isotope Ratio Mass Spectrometer. Values of δ¹³C in parts per million (‰) were calculated using the following formula:

where R is the ratio of ¹³C:¹²C and PeeDee belemnite (PDB) from the Pee Dee River Formation (Hemingway, SC) was the standard to which the samples were compared. In-house laboratory standards (apple leaves) had a within-run variation of <0.04‰ (n = 50), whereas the laboratory standard (flour) used to assess variability between runs analyzed over time had variation <0.07‰ (n = 25).

Wood samples were corrected by −3.2‰ to account for the differences between leaf and wood isotopic signatures. Leaf δ¹³C was measured in the upper and lower portions of the Juniperus trees across the growing season to attain a seasonally integrated δ¹³C_leaf value of −27.79 (n = 65). Our correction value was calculated from the difference between this integrated δ¹³C_leaf value and δ¹³C of the outer most ring of radial growth (2008) of the five tree ring samples (range = −2.4 to −4.3). Similar estimates of the offset between wood tissue and leaf tissue have been found (41, 42).

Carbon isotope discrimination (∆¹³C) accounts for the anthropogenic increase in isotopically light atmospheric δ¹³CO₂ and was calculated according to Farquhar et al. (22),

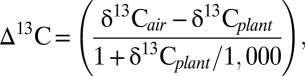where δ¹³C_plant values were leaf-corrected values from each tree ring, and δ¹³C_air values were atmospheric values for the specific year of the tree ring. Values of δ¹³C_air from 1909 to 2003 were taken from McCarroll and Loader (43), who interpolated yearly values taken from the Law Dome ice cores (44). Values of δ¹³C_air from 2004 to 2008 were decreased incrementally by 0.02‰ y⁻¹ (45).

The seasonally integrated C_i/C_a ratio (the ratio of the leaf intercellular CO₂ concentration, C_i, to the CO₂ concentration in the atmosphere, C_a) for each year was calculated from ∆¹³C according to ref. 22, using

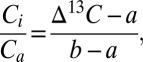where a is the fractionation constant due to diffusion of CO₂ through the stomatal aperture (4.4‰) (46) and b is the fractionation constant due to ribulose-1,5-bisphosphate carboxylase–oxygenase (27‰) (47). Values of C_i for each year were calculated from the C_i/C_a ratios, using C_a values from the Law Dome ice cores and direct atmospheric measurements (7, 8). Intrinsic water-use efficiency, the ratio of photosynthesis (A) to stomatal conductance of water vapor (g_w), compares photosynthetic properties independent of evaporative demand and reflects integrated carbon uptake and water loss over the time of tissue development. Using C_i and C_a, iWUE was calculated as

where Fick’s law of diffusion of CO₂ into the leaf, 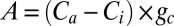, is rearranged to solve for A/g_c, and g_c is converted to g_w using 0.625 as the constant for differences between diffusion of CO₂ and H₂O (48).

Simulation of Photosynthesis and Stomatal Conductance over the Chronology.

A simulation analysis of changes in net photosynthetic rates (A) and stomatal conductance (g_c) of J. virginiana trees over the 100-y chronology was conducted using foliar C_i derived from ∆¹³C, C_a (7, 8), and the relationship between A and C_i (A–C_i curves) of Juniperus foliage measured onsite in 2009. We measured 55 A–Ci curves on 10 Juniperus trees (ages 98–481 y) from April to September 2009. Values of isotopically derived C_i were used with A–C_i curves to estimate A for each year. Stomatal conductance to CO₂ (g_c) was estimated for each year using the methods in ref. 49. For this simulation, we made two assumptions: first, that A was in the CO₂-limited region of the A–C_i curve for the chronology, i.e., atmospheric CO₂ was never at saturating concentrations (11), and second, that there was little photosynthetic acclimation to CO₂ by the trees over the chronology, consistent with results from Free Air CO₂ Enrichment experiments and CO₂ meta-analyses (11, 50–52). The meta-analysis by Ainsworth and Rogers (11) indicates that, of all plant functional types, trees have shown the least potential for photosynthetic acclimation in experiments when CO₂ is doubled from current-day concentrations. As such, it is unlikely that photosynthetic rates of these Juniperus trees have acclimatized to the ∼29% increase in CO₂ between 1909 and 2008.

The A–C_i relationship was determined using an open-flow gas exchange system with an attached red/blue light-emitting diode light source (LI6400; Li-Cor, Inc.). Small branches from 10 Juniperus trees were cut from the upper canopy and immediately placed into containers filled with water (floral water picks). Foliage from the end of branches was used for these analyses. Measurements were made between 1000 and 1600 Eastern Standard Time on sunny days to minimize diurnal effects with saturating light (1,500 μmol⋅m⁻²⋅s⁻¹). To generate A–C_i curves, light-saturated photosynthesis was measured at 10 CO₂ concentrations between 50 and 1,500 μL CO₂⋅L⁻¹ air. The initial CO₂ concentration was maintained at 380 μL⋅CO₂⋅L⁻¹ air. After an equilibration of ∼5 min at each CO₂ concentration, A and C_i were recorded. After the gas-exchange analyses, all leaves were taken to the laboratory and scanned to determine projected leaf area (CanoScan 9950F; Canon), and gas exchange of Juniperus was expressed on a total leaf area basis (53).

Sulfur Isotopic Analysis of Tree Rings.

Five additional Juniperus trees from the same stand were randomly selected and cored in 2013 as described above for BAI and C isotopes. Each core was cross-dated with existing cores left over from the 2008 analysis, and we assigned a calendar year to each annual tree ring. Tree rings for years 1853–1870, 1960–1975, and 1995–2010 were collected for isotopic analysis by scalpel dissection of cores under a dissecting microscope at the boundary of late wood and early wood. Rings from each time period were pooled for each tree and then ground into a fine powder for sulfur isotopic analysis. An n = 5 was used for 1960–1975 and 1995–2010, and an n = 3 was used for 1853–1870.

Wood samples were analyzed for δ³⁴S using a PYRO cube elemental analyzer (Elementar) operated in carbon, nitrogen, sulfur mode with extra O₂ added (100 mL/min for 70 s) to account for the high C:S of wood and the small sample mass available (∼80 mg dry weight). Trapped SO₂ gas was delivered to a Delta XP isotope ratio mass spectrometer (ThermoFinnigan) for analysis. Values of δ³⁴S were corrected according to the international atomic energy standards S-1 and S-2 (−0.3 and 22.7‰, respectively) and an internal laboratory standard of argentite (S-6). Analytical precision for δ³⁴S was ± 0.4‰. Analyses of δ³⁴S were performed in the G. G. Hatch Stable Isotope Laboratory at the University of Ottawa in Ontario, Canada.

Statistics.

To determine if there had been any significant changes in climatic conditions, we examined the linear trends of monthly, seasonal, and yearly means of precipitation and temperature using the 100-y period from 1909 to 2008. Precipitation and temperature data were obtained from the National Climatic Data Center for West Virginia climate region 6 (21), which includes the portion of West Virginia in the Ridge and Valley physiographic province and contains our study site. We used annual average of the atmospheric CO₂ concentrations reconstructed from ice cores (7) and recorded at Mauna Loa observatory since 1953 (8); overlapping values were averaged. Atmospheric CO₂ concentrations increased from 299 µL⋅L⁻¹ in 1910 to 386 µL⋅L⁻¹ in 2008. Historical US SO₂ emissions and historical US NO_x emissions were taken from refs. 9 and 10; overlapping values were averaged.

Linear regression was used to identify significant temporal trends in BAI of Juniperus trees over the tree-ring chronology (1911–2007). Due to the nonnormal distribution and autocorrelation in BAI, we used Kendall’s tau, a nonparametric correlation coefficient, to identify explanatory variables with the best relationships with BAI. All statistical analyses used single-year BAI data and were not detrended or smoothed, providing interpretable yearly levels of growth rather than a standardized index. Analysis of covariance was used to test for differences in BAI trends for before (1909–1970) and after (1970–2008) the Clean Air Act legislation.

Simple regression analyses were used to examine trends in tree ring ∆¹³C, C_i/C_a, and iWUE, as well as simulated A and g_c over the 100-y tree chronology (1909–2008). Although we only used five individual trees in our isotopic analysis, this number is typically sufficient to characterize stand-level tendencies given the typical uniformity of environmental signals reflected in tree rings for a given species and site (33). Analysis of covariance was used to test for differences in isotopic trends for 1930–1940 and 1980–2008.

One-way analysis of variance was used to examine whether S isotopes differed in tree rings from 1853–1870, 1960–1975, and 1995–2010. Differences between the means of each time category were assessed using a Tukey–Kramer honestly significant difference (HSD) test.

All statistical analyses were performed using JMP software for Macintosh v. 10.0 (SAS Institute).