Study of the γD-Crystallin Protein Using Two-Dimensional Infrared (2DIR) Spectroscopy: Experiment and Simulation

Abstract

Cataracts is a misfolding protein disease in which one of its major components is the γD-crystallin protein. The conformational structure of the aggregated γD-crystallin and the interactions that cause aggregation are largely unknown. A recent experimental two-dimensional infrared (2DIR) spectroscopy study determined that the C-terminal domain has a high propensity to form β-sheets whereas the N-terminal domain forms a disordered structure in the fiber state. We present a combined computational molecular dynamics (MD) and infrared spectroscopy study of the local dynamics of these domains. The computed 2DIR signals agree remarkably well with experiment. We show that both domains having a Greek key structural fold experience different electrostatic environments, which may be related to the fact that the C-terminal domain is more structurally stable than the N-terminal domain. We correlate the vibrational couplings to known energy dissipation mechanisms and reveal their origin.

Introduction

Many NMR, FTIR and X-ray Crystallography studies have been used to characterize the structures of the Crystallin proteins aggregates (1–5). Cataracts are misfolded protein diseases that cause blindness and affect elderly population over the age of 65 (National Eye Institute). Its treatment is invasive, expensive and performed only when the disease has severely damaged the eye lens. Pathology of human cataractous lenses reveals that cataracts are composed of protein aggregates called Crystallin proteins. These proteins are degenerative during adulthood, and are damaged by radiation exposure and other chemical modifications that obstruct the passage of light through the lens which cause blurred vision that worsens with time (6–9). Even though the causes of cataracts are known, the aggregation mechanism and the three-dimensional structure of these aggregates remain unknown.

There are three classes of Crystallins: α, β and γ-Crystallins. Their solubilities and stabilities in their native states are critical to keep the transparency of the lens for a lifetime since Crystallin are not regenerated (7, 9, 10). α-Crystallins are oligomers that exhibit chaperone activity, interact with unfolded proteins and prevent aggregation (11–15). β and γ-Crystallins proteins are believed to be structural proteins involved in aggregation and share similar structures composed of antiparallel β-sheets. Their secondary structures are separated into two domains, labeled as N-terminal domain (N-td) and C-terminal domain (C-td) and further clustered into four subdomains called Greek Key Motifs that contain antiparallel β-strand (See Fig. 1A). A key biological difference between β and γ Crystallin proteins is that β-Crystallin forms domain-swapped dimers while the γ-Crystallins are monomeric in solution (1–3, 16).

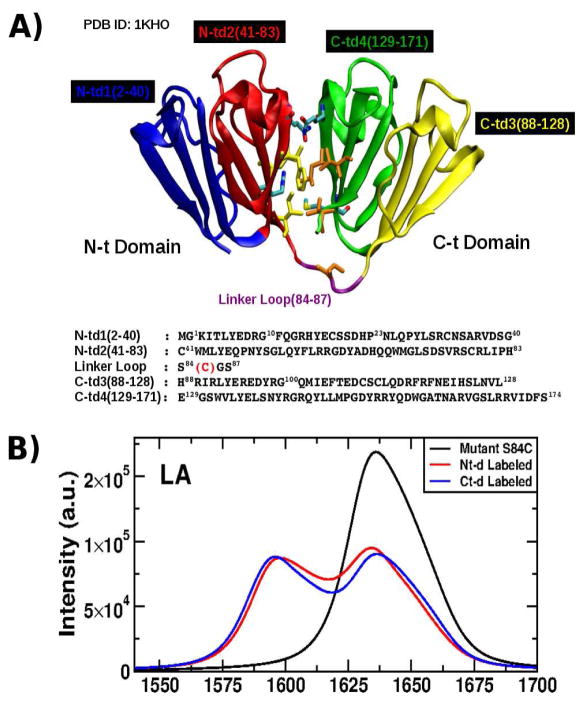
Figure 1.

A: Structure of the γD-Crystallin protein showing its four Greek Motifs divided into the two domains Nt (blue and red) and Ct (green and yellow) connected by a loop (purple) and their respective sequence as shown in the crystal structure (PDB ID: 1KHO) with the mutation S84C indicated (red). Residues located in the interdomains (in licorice) are responsible in the stability of the entire structure. B: Linear absorption (LA) (top) in the infrared regime of the mutant S84C-HγD-Crystallin for the full sequence (black), N-td labeled (red) and C-td labeled (blue).

Wild-type γ-Crystallins are stable eye lens proteins in vitro with higher free-energies of unfolding than the other Crystallin proteins. The two domains of a γ-Crystallin protein interact intermolecularly across a hydrophobic domain interface that helps stabilize the protein (17, 18). Human γD-Crystallin (HγD-Crys) is the third most abundant γ-Crystallin found in young human eyes. HγDCrys is a 173 amino-acid protein with a high structural homology with other γ-Crystallins (10, 19). Its secondary structure is composed of antiparallel β-sheets and loops that are grouped in two domains connected by a loop linker. Mutagenesis studies revealed that single amino acid substitutions in the HγD-Crys sequence are linked to aggregation and juvenile-onset cataracts (20–26). The aggregation mechanism in the aging lens differs from that of the juvenile-onset cataracts because they present a large number of modifications such as Methionine and Cysteine residues oxidation, deamidation, and glycation (27–30)

Fluorescence studies in triple tryptophan mutants of HγD-Crys by Kosinski-Collins et al. (31) found that the C-td is more structurally stable than the N-td. Studies of unfolding/folding experiments under physiological conditions demonstrated that the protein refolds through sequential structuring of its domains, where the C-td folds first and domain interface residues subsequently mediate the refolding of the N-td. Unfolding/folding kinetics studies by Flaugh et al. (17, 18) revealed the formation of partially folded intermediate structure containing the C-td in its native state and the N-td in its random state. They further suggested that hydrophobic residues in the intercore domain are critical in the unfolding/refolding process where the refolded C-td plays the role as a nucleating center for the N-td.

Two-dimensional ultrafast spectroscopy has been used to characterize geometrically ordered structures such as hairpins, amyloid fibrils and helical dimers for many proteins (32–38). One of the advantages of this techinique is its high temporal resolution; detailed information about the kinetics of the proteins can be obtained on timescale regimes inaccessible by others techniques. It can also be applied continously to study amyloid and protein folding kinetics on timescales of months to hours. The QM/MM computation of 2D spectroscopy signals has become a valuable tool in the study of the internal dynamics occurring in biological complexes such as amyloid fibrils (37, 39–43). Often, computations are necessary to fully interpret experimental 2D spectra. A recent study of γD-Crystallin using isotope labeling of domains and 2DIR spectroscopy by Moran et al. (44), determined that the fibril nucleation of the Crystallin occurs exclusively in the C-td. The authors further proposed a structural model of the fibrils composed of a segment of β-sheets formed by the C-td and another segment of random structure formed by the N-td.

In this article, we present a simulation study of 2DIR spectroscopy of natively folded γD-Crystallin and compared with the experimental results of Moran et al. (44). This comparison of experiment and simulation provides new insight into differences in how the native domains are predisposed to misfolding and aggregation.

To the best of our knowledge, this is the first successful comparison of 2DIR simulation with experiment on a protein of this size. We find excellent agreement between the two. Details of the experimental conditions may be found in ref. (44).

Materials and Methods

Protein Model

The HγD-Crys crystal structure at 1.25 Å resolution was solved and is available in the Protein Data Bank (PDB ID: 1HKO) (19). Its sequence consists of 173 amino acids which are grouped in the N-td and C-td domains which each has 2 sub-groups called Greek Key Motif with the labels: N-td1(2–40), N-td2(41–83), C-td3(88–128), and C-td4 (129–171) as shown in 1A. In order to mirror the experimental studies, the mutant S84C HγD-Crys(S84C-HγD-Crys) was created and the MET residue added in their N-Terminus using the MODELLER (45) package.

Simulation Protocol

Molecular dynamics (MD) trajectories of the S84C-HγD-Crys were obtained by using the NAMD Package 2.6 (46) with the CHARMM27 force field (47). Simulations were carried out in aqueous solutions (TIP3P water model) (48) with cubic periodic boundary conditions. The particle-mesh Ewald protocol was used to calculate the long-range electrostatic interactions and a 12 cutoff distance was used for nonbonded interactions. 20 ns MD simulations were performed after 2 ns equilibration with a time step of 1 fs at T=300K. 100 MD snapshots with a time interval of 2 ps from the last 2 ns were harvested to compute the signals. The simulated linear absorption (LA) and the 2DIR signals were computed using the SPECTRON package (49, 50).

A 2DIR experiment uses four input pulses k₁, k₂, k₃ with k_s satisfying k_s = ±k₁ ±k₂ ±k₃ that interact with the protein sample with delays t₁, t₂ and t₃. The signal is defined as the transmission change of the k_s beam is recorded. The signals were computed using the Fluctuating Vibrational-Exciton Hamiltonian (FVE) (51) which is based on the Frenkel Exciton Model. The FVE Hamiltonian, H●_FVE, has the form:

$${\widehat{H}}_{\mathit{FVE}}={\widehat{H}}_S+{\widehat{H}}_F,$$ (1)

where H●_S is the hamiltonian of the system:

$${\widehat{H}}_S=\sum _m{\epsilon }_m{\widehat{B}}_m^{†}{\widehat{B}}_m+\sum _m{J}_{mn}{\widehat{B}}_m^{†}{\widehat{B}}_n-\frac{1}{2}\sum _m{\mathrm{\Delta }}_m{\widehat{B}}_m^{†}{\widehat{B}}_m^{†}{\widehat{B}}_m{\widehat{B}}_m,$$ (2)

and H●_F is the interaction with the optical field, E(t):

$${\widehat{H}}_F=-\mathbf{E}(t)·\sum _m{\mu }_m({\widehat{B}}_m^{†}+{\widehat{B}}_m).$$ (3)

${\widehat{B}}_m^{†}$ and B●_m are the bosonic annihilation and creation operators for the m-th amide I mode, ε_m is the frequency, Δ_m is the anharmonicity, J_mn are the harmonic intermode couplings between modes m and n, and μ_m is the transition dipole moment. J_mn remains the same for all isotopomers, as known from experiments on HγD-Crys.

H●_S and H●_F were evaluated for each snapshot using the fluctuating parameters for each amide I unit using the vibrational map of Hayashi et al. (52). Vibrational maps connect the frequencies and transition dipoles of molecular vibrations with the fluctuating local electrostatic environment. Δ_m = 16 cm⁻¹ were used fro the anharmonicities (53). The intermode coupling, J_mn, is calculated using the transition dipole coupling scheme (54). Once the Hamiltonian is constructed and diagonalized, the $S_{{\mathrm{\nu }}_4{\mathrm{\nu }}_3{\mathrm{\nu }}_2{\mathrm{\nu }}_1}^{{\mathbf{k}}_s}$ signal is computed using equations 115, 119 and 121 in ref. (50):

$$\begin{array}{l}{S}_{{\mathrm{\nu }}_4{\mathrm{\nu }}_3{\mathrm{\nu }}_2{\mathrm{\nu }}_1}^{{\mathbf{k}}_s}({\mathrm{\Omega }}_3,t_2=0,{\mathrm{\Omega }}_1)=2i\sum _{e_4\dots e_1}{\langle {\mu }_{e_4}^{{\mathrm{\nu }}_4}{\mu }_{e_3}^{{\mathrm{\nu }}_3}{\mu }_{e_2}^{{\mathrm{\nu }}_2}{\mu }_{e_1}^{{\mathrm{\nu }}_1}\rangle }_0{I}_{e_1}^{\ast }(-{\mathrm{\Omega }}_1)I_{e_4}({\mathrm{\Omega }}_3)\times \\ {\mathrm{\Gamma }}_{e_4{e}_3{e}_2{e}_1}({\mathrm{\Omega }}_3+{\epsilon }_{e_1}+i{\gamma }_{e_1}){\mathcal{J}}_{e_3{e}_2}({\mathrm{\Omega }}_3+{\epsilon }_{e_1}+i{\gamma }_{e1}),\\ I_e(\mathrm{\Omega })=\frac{i}{\mathrm{\Omega }-{\epsilon }_e+i{\gamma }_e},\\ {\mathcal{J}}_{e{e}^{\prime }}(\omega )=\frac{i}{\omega -{\epsilon }_e-{\epsilon }_{e^{\prime }}+i({\gamma }_e+{\gamma }_{e^{\prime }})}.\end{array}$$

where I_e(Ω) and 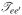are the one and two-exciton Green’s functions, respectively. In this study, we set the broadening factor γ_e = 5.5 cm⁻¹. Γ_e4e3e2e1 (Ω) is the exciton scattering matrix with two incoming (e₁ and e₂) and two outcoming excitons (e₃ and e₄) as defined in ref. (50), equation 325.

The signals are plotted by using two-dimensional Fourier transform of the times t₁ → Ω₁ and t₃→Ω₃. The signals can be either non-chiral or chiral depending on the polarization configuration of the laser pulses. In the dipole approximation, there are three independent signals to leading zeroth order in k: xyxy, xyyx, and xxyy (where x and y are the polarization of the laser pulses). The absorptive all-parallel signal, xxxx, is given by the linear combination xxxx = xyxy + xyyx + xxyy. Some combinations of non-chiral induced signals can be used to enhance desired features. The xyxy-xyyx combination supresses the population pathways and reveals the coherent quantum dynamics through crosspeaks (50). The combination xxyy-xyxy highlights the population dynamics and eliminates the coherent pathways in stimulated emission. For t₂ = 0, we have xyxy=xxyy, and the combination xyxy-xyyx would only reveal excited state absorption.

We have simulated the absorptive 2DIR signals S^k_I + S^k_II. Here k_I = −k₁ + k₂ + k₃ and k_II = k₁−k₂ + k₃. We focused on the amide I band, which corresponds to the backbone carbonyl vibrational motions. The xxxx, the xxyy signals are compared with experiment. We further predict the combination xyxy-xyyx signal. The 2D non-chiral spectra used to compare with the experimental signals are plotted using a linear scale.

Results

Linear Absorption Spectra of the γD-Crys Protein

The linear absorption (LA) signals in the IR regime for the γD-Crys protein with its respective labeled domains are displayed in 1B. The labeling protocol was implemented in this study as described by Moran et al. (44): One domain is ¹³C isotopically labeled and the other domain remains ¹²C intact. In the LA signal, the unlabelled protein displays a peak centered at 1638 cm⁻¹. When one of the domains is isotope labeled, the signal acquires a bimodal shape with two peaks around at 1595 cm⁻¹ and 1638 cm⁻¹ (1B). The LA signal for the labeled Ct-d shows that the peak at 1638 cm⁻¹ moves slightly to the higher frequency making the bimodal shape more pronounced than that of the labeled Nt-d.

2DIR Signals

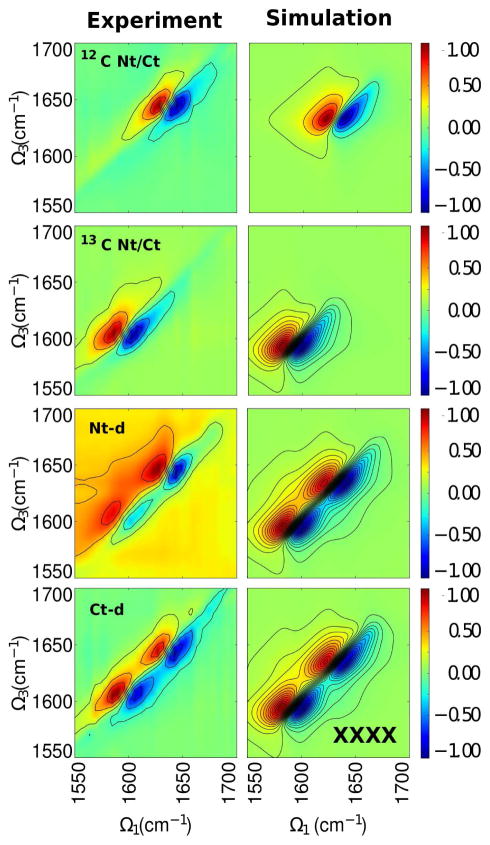
The 2DIR signals for the S84C-HγD-Crys and their respective labeled domain samples were computed at t₂ = 0. The experimental and simulated all parallel xxxx spectra of the mutant protein and its respective labeled domains are compared in 2. For the unlabeled and labeled full protein (2, ¹²C Nt/Ct and ¹³C Nt/Ct, respectively), a pair of negative and positive peaks are observed in each spectrum: At Ω₃ = 1638 cm⁻¹ for the unlabeled protein and at Ω₃ = 1595 cm⁻¹ for the labeled protein. These frequencies correspond to a structure with antiparallel β-sheets (55). The negative peak results from ground state bleach and excited state emission pathways while the positive peak results from excited state absorption. The broadening of these peaks is due to contributions of other secondary structures formed in the protein. These peaks are reproduced in the simulations (2, right panels). These pairs are shifted around by 40 cm⁻¹ which is consistent with the effective mass replacement of the carbon. The node slopes (44) were calculated for both of these states to compare the contributions of inhomogeneous versus homogeneous broadening (Table S1). A node slope of one represents a perfectly inhomogeneously broadened peak while a value of infinity represents a perfectly homogeneously broadened peak. The node slope of the unlabeled peak pair is in good agreement with experiment, while the node slope for the labeled full protein shows a 15% deviation from experiment. This deviation may be because while the isotope labeling was accounted for in the Hamiltonian (equation 2), the MD simulations used for conformational sampling did not use a heavy carbon, which may have a slight influence on the dynamics.

For the proteins with labeled domain, two pairs of positive and negative peaks appear in the spectra. The experimental spectra of the labeled domains (2, Nt-d and Ct-d) show two pairs of peaks near Ω₃ = 1640 cm⁻¹ and Ω₃ = 1605 cm⁻¹ in each spectrum. Differences between the experimental signals can be seen. The peak pairs in the labeled Nt-domain signal are less intense and more separated than those of the labeled Ct-domains (see Table S1), where the high frequency pair in the labeled Ct-domain are elongated and broader than those of in the labeled Nt-domain signal. For the low frequency pair, the labeled Nt-domain signal shows difused peaks, especially the negative peak, while the positive peak is well defined and centered at Ω₃ = 1605 cm⁻¹. In the labeled Ct-domain signal, the low frequency peak pair is intense and are centered at Ω₃ = 1605 cm⁻¹.

Some of these features are reproduced in the simulated labeled domain signals (2, right panels third and fourth rows). Both show a similar profile with two pairs of peaks separated by 40 cm⁻¹. In the labeled Nt-domain signal, the negative peaks are less broad than those of in the labeled Ct-domain signal. The peak pairs in the labeled N-td signal are more separated than those of the labeled C-td signal, as observed in experiment and the simulated linear absorption spectra. Comparing the simulated and experimental node slopes, we see that the node slopes attributed to the unlabeled domains are in good agreement with experiment while those attributed to the labeled domains show a larger deviation from experiment. Again, we believe this larger deviation from experiment may be because all MD simulations used for conformational sampling used ¹²C in the amide backbone.

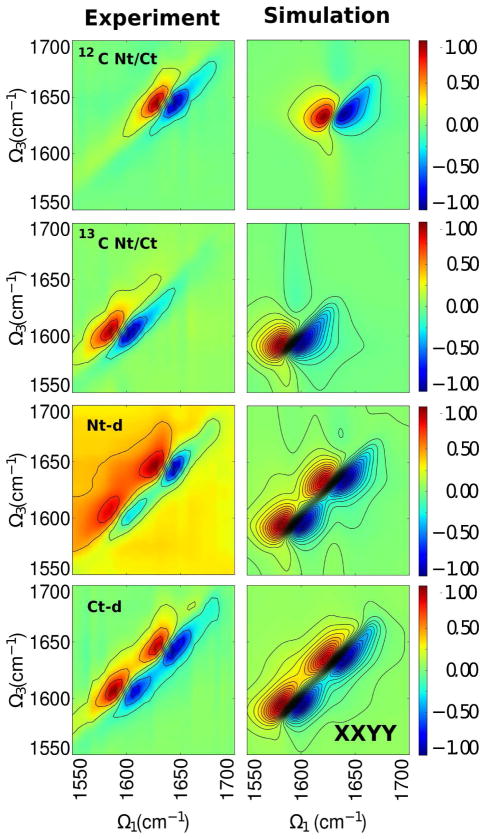
We next turn to the xxyy signal (3, left panels). All spectra share similar profile as in the xxxx spectra but with less intense peaks at Ω₃ = 1640 cm⁻¹ and Ω₃ = 1605 cm⁻¹. This pattern is observed in the simulated data as well. However, the peak pairs in the labeled full protein are stronger and broader than in the unlabeled full protein which indicates that the N-td follows different dynamics where its eigenstates exhibit a wider frequency range despite the high homology in the backbone geometry. We note that in the simulated spectra, we are able to better resolve the low intensity cross peaks better than in experiment because of the lack of experimental (white) noise in the simulations.

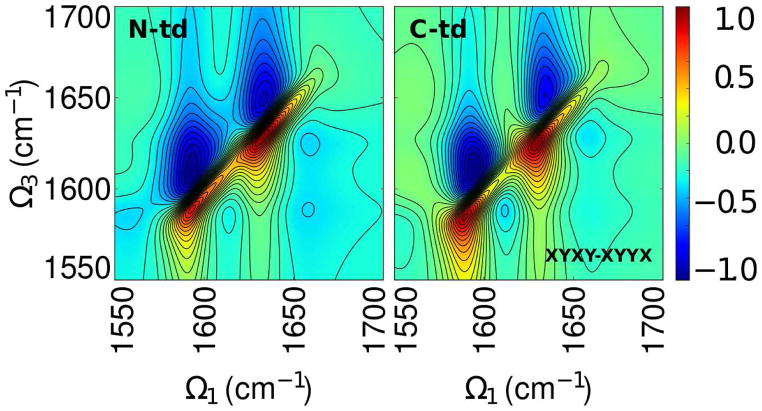
The simulated combination xyxy-xyyx spectrum (4) for labeled domains share the same pairs of peaks as observed in the xxxx combination. However, the N-td shows more elongated positive peaks along the diagonal than the positive peaks in the C-td. The spectra also show cross-peaks at Ω₁ = 1610, 1660 cm⁻¹ that are more diffuse in the Ct-Domain than the N-td.

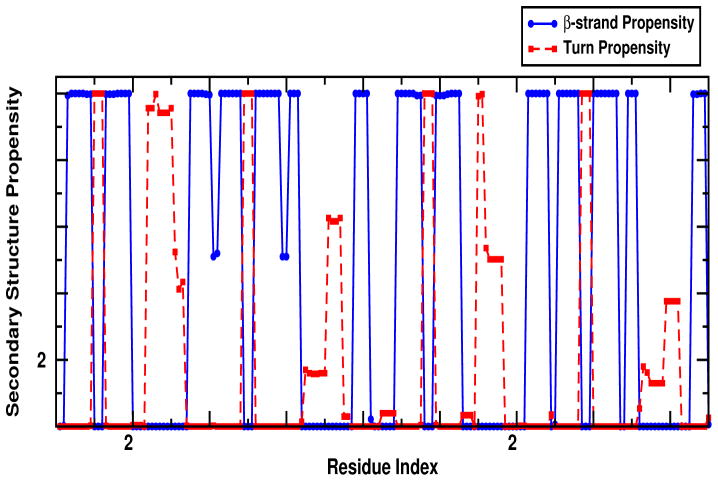
Both experiment and simulation show clear differences in the 2DIR spectra when the N-t or C-t domain are labeled, despite their high structural homology. The experimental and simulated spectra show some weak cross peaks which suggest couplings between the backbone amide states. These couplings depend strongly on the geometry of the protein. The domains are connected by a flexible linker loop and one domain is essentially the reflection of the other domain. The secondary structure propensity for the protein displayed in 5 showed that reflection among domains especially where the propensity to form β-strand is high except in the residues 40–41 and 60–61 from the N-td2 motif, and their corresponding reflections in the C-td2 motif (residues 129–130 and 147–148). Other differences are observed in the turn propensities where the loop between N-td1 and N-td2 motifs are higher than that of among C-td1 and C-td2 motifs. These differences in secondary structure indicate that the interactions among the sub-domains may affect the overall stability of each domain.

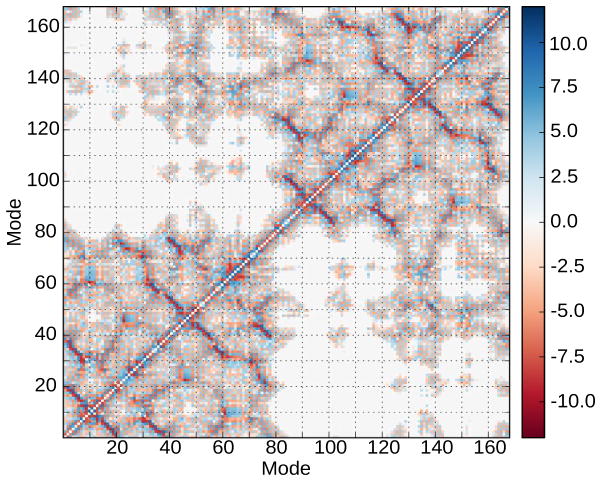
The coupling matrix (shown in 6) may help reveal how the various residues are relevant in the folding and stability of the domains because the couplings are sensitive to distance and orientation. Couplings are observed from intramolecular interactions between residues that form β-sheets structures (anti-parallel to the diagonal). Residues in the N-td (residue indexes 1 to 83) have weaker couplings when compared with the corresponding section in the C-td (residue indexes 88 to 168). In the N-td, R36 is strongly coupled to T4 and K2. These surface residues are solvent-exposed and it is known that mutant R36S is linked to congenital juvenile onset-cataracts (56). Interestingly, Q54 and R58 show a strong coupling with those residues in the segment where R36 is located. The image residue for Q54 in the C-td is Q143. Q54 and R58 are solvent-exposed residues that form one of the β-strands in their respective domains and belong to the set of residues in the interface core. Deamidation of Q54 and Q143 introduce a negative charge at physiological pH into the domain interface and destabilizes HγD-Crys, lower the unfolding kinetic barrier and leads to the formation of partially unfolded intermediate with structured C-td and a unstructured N-td (29). The R58H mutation, which results in aculeiform cataract (20), and the R36S mutant have lower solubilities and crystallize more readily than the wild-type (19).

A cluster of couplings seen in the map corresponds to the hydrophobic interdomain region located between Nt-d2 (residues M43, Q54, F56, R79, I81) and Ct-d4 (V132, Q143, L145, and M147). These residues play a role in the stabilization of the domains and a decrease of the refolding rate of the N-td as indicated in alanine screening experiments (17).

HγD-Crys protein has, on the other hand, a large number of aromatic residues (14 tyrosine, 6 phenylalanine, and 4 tryptophan), few of them are lone residues and the rest are distributed in pairs or set of three residues that maintain the protein structure (57). In the coupling map, we indicate two pairs of tryptophan of interest: W42 and W68 in the N-td and their images residues, W131 and W157, in the C-td. W42 and its image W131 are buried at the bottom of their respective domains. The map indicates that W42 couples with Y28, and W131 couples with D114 but the pair (W42,Y28) displays a weaker coupling than that of the pair (W131,D114). W42 and its image W131 have coupling with W68 and W157, respectively. (W42,W68) has a weaker coupling intensity than (W131,W157) where the latter is surrounded by strong couplings generated by other residues. The other two tryptophan, W68 and its image W157, located at the top of the structure, exhibit some couplings. For W68, we have the pairs (W68,Y6), (W68,Y28) and (W68,Y50) and for W157, we have (W157,H88), (W157,F117), and (W157,W132). It is well established that UV radiation may contribute to the origin of cataract due to the absorption by aromatic residues (57). These tryptophan residues, W42, W68, W131 and W157, have been studied using fluorescence quenching (31) and determined that the local environment around W68 and W157 originate the native state quenching of the protein fluorescence emission. During the refolding/unfolding process, W42 and W68 unfolded at lower concentrations of GdnHCl than their images residues, W131 and W157, respectively. Futher analysis of unfolding and refolding of the tryptophan mutants determined an intermediate state along the wt-HγD-Cryst folding pathway with a structured C-td and unstructured N-td which could be a suitable conformational model for cataract formation.

Even though the two domains are geometrically homologous, they make different contributions to the 2DIR signals. The large separation between densities of states for the pair Q54 and Q143 could be due to their exposure to the solvent and their location in the interface domain which could change the strengths of the interactions with other residues. The tryptophan residues considered here are buried in their respective domains. Although both domains share basically identical backbone geometry, the frequency shifts upon domain labelling suggest that the kinetics of these domains are different where the folding in the N-td depends on the transitions occurring in the C-td, which agrees well with experimental reports (17, 30, 59)

Discussion and Conclusions

Moran et al. used a combined approach of isotope labeling and 2DIR spectroscopy (44) to study the γD-Crystallin protein. They demonstrated that the use of this combined tool is valuable for the characterization of the kinetics and structure in proteins that help to obtain additional information not available from standard methods. In their studies, they expressed labeled and unlabeled domains of the protein separately, and the full length of γD-Crystallin protein was constituted by protein ligation using a covalent linker residue located in the connecting loop. They determined tha C-td of the γD-Crystallin protein is the leading domain to form amyloid fibrils and the use of this covalent linkage between domains plays a role in determining the fibril structure which is a striking result considering that each individual domain can form fibrils on its own (58).

We note that our simulations are limited to the computation of 2DIR spectra of the monomeric form of HγD-Crys, and do not offer a direct comparison with the 2DIR kinetics experiments. We used a computational approach to compliment these results at the level of the native state of the mutant S84C-γD-Crystallin monomers. The computed spectra are in excellent agreement with experiment. Although the N-t and C-t domains are geometrically similar with highly conserved residues in their sequences, the average coupling map analysis shows an asymmetric contribution of some relevant residues and their corresponding image residue to the signals when one of the domains is isotopically labeled. Experiments made by Mills et al. (59) concluded that the isolated N-td was less stable than the full-length proteins and less stable than the C-td. A computational study by Das et al. (60) confirmed that the stability and the folding mechanism of the N-td are regulated by the interdomain interactions.

Our findings are consistent with the conclusions that Moran et al. stated about the role of the covalent linkage between domains in determinining the fibril structure and the prevalent dynamics that the C-td has over the N-td in the fibril formation. It will be interesting to use 2DIR spectroscopy to analyze the coupling between domains at different environmental conditions and mutations.

As stated in the introduction, we can consider HγD-Crys in terms of two coupled domains. In a very simple approximation, it is possible to think of this as a coupled two-mode exciton system:

$$H\approx \left(\begin{array}{cc}{\epsilon }_i& J_{ij}\\ J_{ij}& {\epsilon }_j\end{array}\right)$$

where ε_i is the mean site-energy of domain i and J_ij is the coupling between modes i and j. The eigenvalues are:

$${\epsilon }_{\pm }=\frac{1}{2}\left[{\epsilon }_i+{\epsilon }_j\pm \sqrt{4J_{ij}^2+{({\epsilon }_i-{\epsilon }_j)}^2}\right]$$

When neither domain is isotope labeled or both domains are labeled, ε_i ≈ε _j, and we have (roughly) a linearly coupled homodimer. When either the N-td or the C-td is isotope labeled, we then have a linearly coupled heterodimer. In this approximation, we expect to see two peaks at ε₊ and ε₋ in the linear absorption spectrum. These peaks are separated by $\mathrm{\Delta }\omega =\sqrt{4J_{ij}^2+{({\epsilon }_i-{\epsilon }_j)}^2}$. If the system is symmetric, then (ε_i − ε _j)² = (ε _j − ε_i)² and the N-td and C-td labeled isotopomers will produce equivalent linear absorption spectra (61). However, as seen from the calculated linear absorption spectra, this is not the case as the peaks in the N-td labeled isotopomer are less separated compared to the peaks in the C-td labeled isotopomer. Since J_ij is the same for the two isotopomers, this indicates that (ε_i − ε _j)² is greater for the C-td labeled isotopomer. This is likely related to the fact that the C-td has a better defined structure, which leads to a lower approximate site energy

While the diagonal elements of the Hamiltonian are decreased by isotopic substitution, the off-diagonal elements remain the same because the transition dipoles of the individual amide I modes, which determine the coupling, do not change upon isotopic substitution. This was verified experimentally by comparing the diagonal cross-sections of the 2DIR spectra of the unlabeled and uniformly labeled ¹³C proteins.

However, as noted by Fang et. al (62), the cross peaks are a direct measure of the off-diagonal an-harmonic coupling. Since we assume zero mechanical off-diagonal anharmonicity, all off-diagonal anharmonicity must result from electrostatic coupling. In the weak-coupling limit, which is appropriate here, this off-diagonal anharmonicity can be approximated as:

$${\mathrm{\Delta }}_{ij}=-\frac{4{\mathrm{\Delta }}_{ii}}{{({\epsilon }_i-{\epsilon }_j)}^2}$$

Where Δ_ij is the off-diagonal anharmonicity and Δ_ii is the diagonal anharmonicity (16 cm⁻¹). Therefore, systems with a smaller (ε_i − ε _j)² will have stronger cross peaks. In our case, the N-td labeled isotopomer has the smaller value and therefore has stronger cross peaks.

Using this very simple model, we conclude that the asymmetry in the linear absorption and 2DIR spectra is caused by the differences in the site-energies in the two domains. The differences in these site-energies are caused by the differing local electrostatic environments of residues within these two domains and is likely related to the secondary structure propensity in each domain.

Figure 2.

All-parallel signal xxxx S_kI + S_kII experiment (left) and simulation (right). From top to bottom: full mutant protein (12C Nt/Ct), labeled full mutant protein (13C Nt/Ct), labeled N-td and the labeled C-td.

Figure 3.

Same as in 2 but for polarization configuration xxyy.

Figure 4.

Simulated xyxy-xyyx signals of the isotopically labeled domains.

Figure 5.

Secondary structure propensity of the domains of γD-Crystallin indicates the segments of the sequence that have the probability to form β-strands or turns.

Figure 6.

Coupling matrix of the γD-Crystallin protein. Inter-domains interactions are represented by a red-blue color scale indicating their strength.