Magnetic wall decoupling method for monopole coil array in ultrahigh field MRI: a feasibility test

Abstract

Ultrahigh field (UHF) MR imaging of deeply located target in high dielectric biological samples faces challenges due to the reduced penetration depth at the corresponding high frequencies. Radiative coils, e.g., dipole and monopole coils, have recently been applied for UHF MRI applications to obtain better signal-noise-ratio (SNR) in the area deep inside the human head and body. However, due to the unique structure of radiative coil elements, electromagnetic (EM) coupling between elements in radiative coil arrays cannot be readily addressed by using traditional decoupling methods such as element overlapping and L/C decoupling network. A new decoupling method based on induced current elimination (ICE) or magnetic wall technique has recently been proposed and has demonstrated feasibility in designing microstrip transmission line (MTL) arrays and L/C loop arrays. In this study, an array of two monopole elements decoupled using magnetic wall decoupling technique was designed, constructed and analyzed numerically and experimentally to investigate the feasibility of the decoupling technique in radiative coil array designs for MR imaging at 7 T. An L-shaped capacitive network was employed as the matching circuit and the reflection coefficients (S₁₁) of the monopole element achieved –30 dB or better. Isolation between the two monopole elements was improved from about –10 dB (without decoupling treatment) to better than –30 dB with the ICE/magnetic wall decoupling method. B₁ maps and MR images of the phantom were acquired and SNR maps were measured and calculated to evaluate the performance of the ICE/magnetic wall decoupling method. Compared with the monopole elements without decoupling methods, the ICE-decoupled array demonstrated more independent image profiles from each element and had a higher SNR in the peripheral area of the imaging subject. The experimental and simulation results indicate that the ICE/magnetic wall decoupling technique might be a promising solution to reducing the EM coupling of monopole arrays for UHF MRI.

Introduction

Ultrahigh field (UHF) MRI is able to provide a high signal-noise-ratio (SNR) and a high contrast for human imaging (1-8). However, attenuation of radiofrequency (RF) signals increases at higher frequencies, which could degrade the sensitivity in the deep area of the high dielectric, conductive biological samples, such as human body (9). To increase the SNR gain in the area located deep inside the human head or body, radiative coil arrays including dipole (10,11) and monopole (12,13) arrays have been proposed for UHF MRI applications, showing better SNR performance in the deep area (10,13).

Reducing the electromagnetic (EM) coupling among coil elements is critical to the coil array designs, given that better decoupling performance usually means higher SNR and better parallel imaging ability (8,14-19). Several methods are widely employed to reduce the mutual EM coupling of coil elements including the use of low input impedance preamplifiers, overlapping of adjacent loop elements (20), transformers (21) and L/C decoupling networks (22-28). However, the traditional methods, such as element overlapping and L/C decoupling network, face challenges and are not readily feasible for dipole or monopole transceiver arrays. A new decoupling method based on induced current elimination (ICE) or magnetic wall technique has recently been proposed and has demonstrated its feasibility in designing microstrip transmission line (MTL) arrays (29-32) and traditional L/C loop arrays (33-35). The magnetic wall decoupling method uses an independent decoupling element which forms a metamaterial to eliminate the current induced by EM coupling. This method is more general and does not need physical connection between coil elements, which is essential for dipole and monopole transceiver arrays.

In this study, we aim to test the feasibility and investigate the performance of the ICE/magnetic wall decoupling method for the monopole array (13). A two-channel ICE-decoupled monopole array was designed and fabricated for MR imaging at 7 T. In the array, an L-shaped capacitive network was employed to address the impedance mismatch of monopole elements, an issue currently faced in monopole RF coil designs. Bench test and MR imaging results obtained from the ICE-decoupled array demonstrate its capability of obtaining a high decoupling between monopole elements while original B₁ profiles of the individual monopole elements are maintained. To further validate the ICE/magnetic wall decoupling method in monopole arrays, Q values, S parameters, B₁⁺ maps, phantom images and SNR maps of the ICE-decoupled monopole elements were evaluated and compared with the monopole elements without decoupling methods. Additionally, full-wave EM simulations were applied to analyze the proposed design. Simulated B₁⁺ maps and gradient recalled echo (GRE) images were compared with experimental results.

Materials and methods

Design and construction of the two-channel ICE-decoupled monopole array

For the two monopole elements without decoupling methods (as shown in Figure 1A), the S₁₁ of each monopole element could still achieve about –12 dB even no matching circuits was employed (13). However, the S₁₁ of each monopole element was only about –2 dB when the decoupling element was added in practice. This was because the self-impendence of each monopole element was totally changed by the decoupling element. Therefore, matching circuits had to be employed for the ICE-decoupled monopole elements. An L-shaped capacitive network (C_s and C_p, as shown in Figure 1B and 1C) were chosen as the matching circuit considering that trimmer capacitors were easier to be obtained. To make a fair comparison, the matching circuit was also applied for the two monopole elements without decoupling methods (Figure 1B). To compensate the induced capacitance of C_s, the length of the matched monopole elements was a little longer (about 25 cm) than unmatched monopole elements (about 20 cm) (13). The series capacitor C_s could also be used to finely tune the resonant frequency of the monopole elements.

Figure 1.

Schematics of two monopole elements: (A) Without matching circuits and without the decoupling element; (B) With matching circuits and without the decoupling element; (C) With matching circuits and with the decoupling element.

Two monopole elements with and without the decoupling element were mounted on a cylindrical acrylic former with an outer diameter of 26 cm, as shown in Figure 2A and 2B. The distance of the two elements were 8 cm and the decoupling element was symmetrically placed between them. All conductor strips were made of the copper tape (3M, St, Paul, MN) with a thickness of 100 µm and a width of 10 mm. The length of monopole elements and the decoupling element were about 25 cm. Two capacitors C_s and C_p (Johanson Manufacturing corp., NJ, USA) were applied for matching and finely tuning. For the two ICE-decoupled monopole elements, a monopole series with a capacitor (referred to as the decoupling capacitor C_d) was served as the decoupling element, as shown in Figure 1C and Figure 2B. The upper corners of the ground plate, which had a dimension of 40×40 cm², were cut to fit in our MRI bore. To reduce the eddy currents, the ground plate was cut by a number of slots and chip capacitors with a value of 1 nF were connected across slots. A cylindrical water phantom with an outer diameter of 16 cm and a length of 37 cm was placed 2 cm below the monopole elements. The electromagnetic parameters of the water phantom were measured by a dielectric probe (DAK-12, Speag, Switzerland): conductivity σ =0.59 s/m; relative permittivity ε_r =78.

Figure 2.

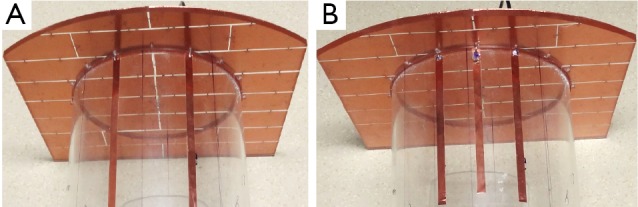
Constructed two monopoles with matching circuits. (A) Without the decoupling element; (B) With the decoupling element.

Bench tests and MR imaging experiments

The magnetic wall decoupling method for the monopole array was validated by bench tests and MR imaging experiments on the water phantom. All MRI experiments were performed on a whole-body MRI scanner (7 T MAGNETOM, Siemens Healthcare, Erlangen, Germany). Reflection coefficient (S₁₁) and transmission coefficient (S₂₁) of the two monopole elements with and without the decoupling method were measured with an Agilent E5071C network analyzer. The reflection coefficient measurements were also used to calculate the coil’s unloaded Q value (Q_UL) and loaded Q value (Q_L).

To demonstrate the decoupling performance of the magnetic wall decoupling method, B₁⁺ maps and image profiles of the water phantom in the transverse plane using the two monopole elements with and without the decoupling element were compared. The transverse plane chosen for MR imaging was 4 cm apart from the GND. During the MR imaging experiments, one monopole element was used for both transmit and receive with the other one terminated with 50 Ω load. The sequence and parameters used for imaging acquisition were GRE, flip angle (FA) =25 deg, TR =150 ms, TE =20 ms, field of view (FOV) =180×180 mm², matrix =256×256, slice thickness =5 mm, bandwidth =260 Hz/pixel, and phase encoding is in the y direction. In this comparison, the experimental setup, including imaging sequence and parameters, was exactly the same. SNR maps were generated by dividing the signal intensities (SI) of the images by the standard deviation (SD) of the noise (36): SNR= SI⁄SD ×0.66. The B₁⁺ map was measured with a Turbo FLASH method and scaled to angle (37,38). During B₁⁺ map measurement, all parameters were set the same for quantitative comparisons.

EM simulations

The three dimensional (3-D) EM and RF circuit co-simulation approach was used to investigate the performance of the magnetic wall decoupling method for the monopole array (28,32,39-41). Full-wave EM modeling (HFSS, ANSYS, Canonsburg, PA, US) was chosen as the EM simulation tool because of its fast speed (42).

Two monopole elements with and without the decoupling element were modeled in HFSS for simulation, as shown in Figure 3A and 3B. In the simulation, all parameters including dimensions of the coil and phantom, electromagnetic parameters and position of the phantom were exactly the same with the practical situation. The distance of the boundary and monopole elements was larger than λ/2. Manual mesh was used to accelerate simulation convergence and the convergence condition ∆S was set to 0.002 to achieve more reliable results. In the simulation, one port was excited with 1 W with the other port terminated with 50 Ω. B₁ maps (transmission field B₁⁺ and reception field B₁^–) were extracted from simulation by Eq. [1] (43). Image or SI profiles of each coil element were calculated from simulation by Eq. [2] (44).

Figure 3.

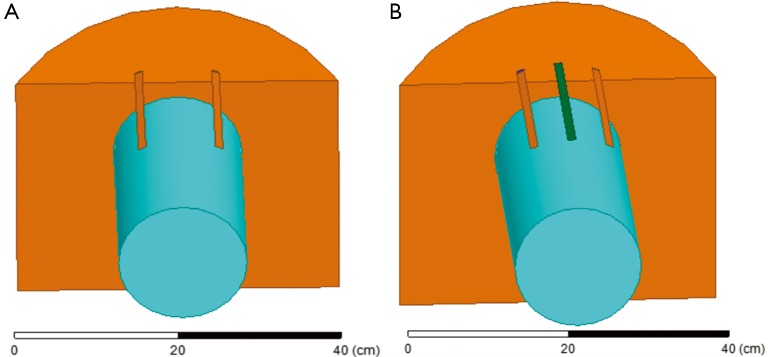
Simulation geometries of two monopoles and the water phantom. (A) Without the decoupling element; (B) With the decoupling element. All parameters including dimensions of the coil and phantom, electromagnetic parameters and position of the phantom were exactly the same with the practical situation.

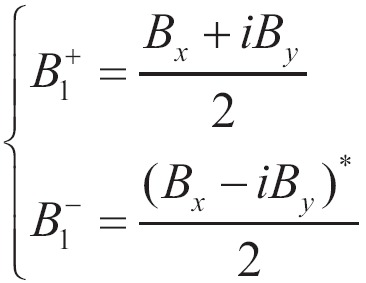[1]

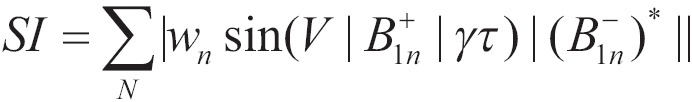[2]

where N is the total number of the voxels within the FOV; γ denotes the gyromagnetic ratio; W_n is the water content percentage of the nth voxel, and τ is the excitation duration. V, the normalization factor, is determined by: 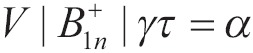, where α is the flip angle.

Results

Measured S parameters and Q values

For two monopole elements without matching circuits and without the decoupling element, the measured reflection coefficients (S₁₁) loaded with the water phantom were only –12.7 dB, as shown in Figure 4A. The S₁₁ of the monopole element could be improved to better than –28 dB when the L-shaped matching circuit was added, as shown in Figure 4B and 4C. This indicated the impedance could be well matched to 50 Ω by using the proposed matching circuit. The impedance was closer to 50 Ω, indicating that the noise figure (NF) of the conventional 50 Ω preamplifier was better and the SNR could be improved by using the matching circuit.

Figure 4.

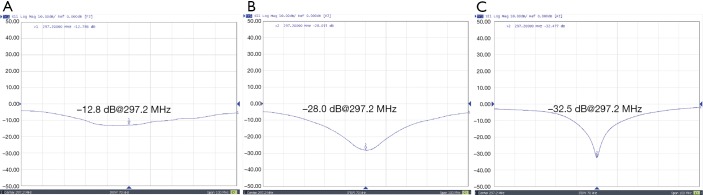
Measured S₁₁ plots of two monopoles loaded with the water phantom. (A) Without matching circuits and without the decoupling element; (B) With matching circuits and without the decoupling element; (C) With matching circuits and the decoupling element. The S₁₁ of the coil elements could be improved to better than –28 dB with matching circuits added, indicating the monopole elements were well matched by using the L-shaped capacitive networks.

For the two monopole elements without the decoupling method, the transmission coefficient S₂₁ between the two coil elements was about –10 dB, as shown in Figure 5A. The average Q_UL and Q_L of a single element were about 9.3 and 3.8, respectively.

Figure 5.

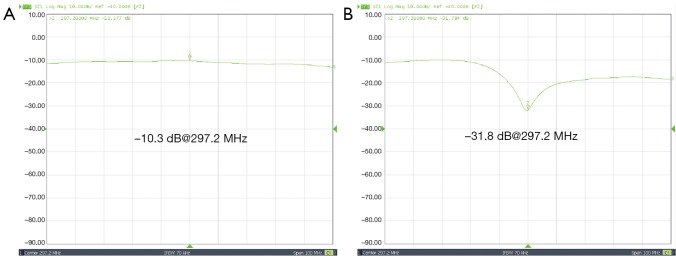
Measured S₂₁ plots vs. frequency of two monopole elements loaded with the water phantom. (A) Without the decoupling element; (B) With the decoupling element. The isolation between the two elements can be reduced from about –10 dB to about –32 dB, indicating the strong decoupling ability of the ICE/magnetic wall method for the monopole array.

For the ICE-decoupled two monopole elements, the transmission coefficient S₂₁ between the two coil elements was about –32 dB, as shown in Figure 5B. This means the coupling of two monopoles can be well reduced by using ICE/magnetic wall decoupling method. The average Q_UL and Q_L of a single element were 18 and 6.2, respectively. The increased Q value is probably because of the better decoupling performance and the shielding effect of the decoupling element. The improved Q_L could also be observed clearly from the comparison of S₁₁ plots in Figure 4B and 4C.

B₁ maps, MR images and SNR maps

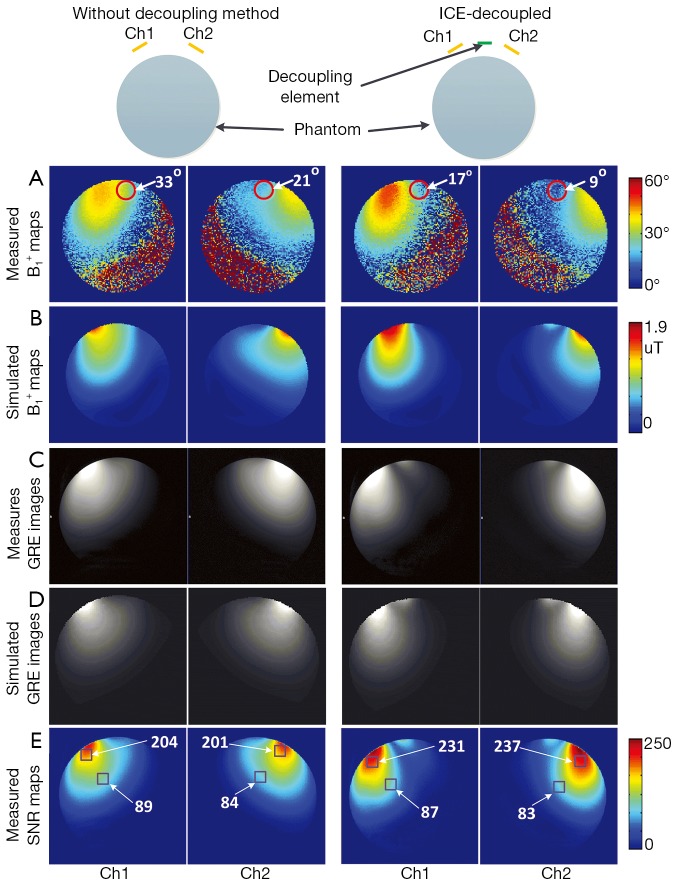
Measured and simulated B₁⁺ maps of the two monopole elements without and with the decoupling element were shown in Figure 6A and 6B. For both measured and simulated results, B₁⁺ map of each channel of the ICE-decoupled array was more independent and weaker at the neighborhood of the two elements, indicating better decoupling performance was obtained by the ICE/magnetic wall decoupling method. From the measured results, the average angles, which were corresponding to average B₁⁺, of the neighborhood (red circle area in Figure 6A) were reduced from 33 degree to 17 degree and from 21 degree to 9 degree for channel 1 and channel 2, respectively. In Figure 6A, the B₁⁺ field of ICE-decoupled elements was stronger at the peripheral area of the water phantom, which was probably due to the shielding effect of the decoupling element.

Figure 6.

Measured and simulated results of the two monopole elements without and with the decoupling element. (A) Measured B₁⁺ maps scaled to angle; (B) Simulated B₁⁺ maps; (C) Measured GRE images; (D) GRE images calculated from simulation; (E) Measured SNR maps. For both measured and simulated results, B₁⁺ maps and GRE images of ICE-decoupled elements were more independent. ICE-decoupled elements have better SNR at the peripheral area with an improvement of about 15% and meanwhile kept similar SNR at the center area of the phantom. The consistency between the simulation and experiment has also demonstrated that the simulation results are reliable.

Measured and calculated GRE images from simulation without and with the decoupling element were shown in Figure 6C and 6D. Well defined image profiles of the two ICE-decoupled monopole elements were obtained, indicating sufficient electromagnetic decoupling between the two elements. Note that nulls of the images of the ICE-decoupled elements at the peripheral area was because of the different distribution of the transmit field and receive field. The agreement between the simulation and experiment also demonstrated that the simulation results are reliable.

Measured SNR maps of the two monopole elements with and without the decoupling element were shown in Figure 6E. Two squares (gray squares in Figure 6E) with 15 pixels at the peripheral and center areas were chosen for the quantitative comparison of the SNR. The ICE-decoupled elements had better SNR at the peripheral area with an improvement of about 15% and meanwhile kept similar SNR at the center area of the phantom. This improvement can be verified by the comparison of B₁⁺ map as described above and was consistent with the previous work (33,35).

Discussion and conclusions

In this study, the feasibility of ICE/magnetic wall decoupling method for the monopole radiative coil array has been validated through bench tests, MR imaging experiments and EM simulations. With this decoupling technique, coupling between two monopole elements is able to be reduced from about –10 dB to a sufficiently small value (better than –30 dB). Due to the better decoupling performance and shielding effect of the decoupling element, the ICE-decoupled monopole elements demonstrate more independent image profiles and achieve a higher SNR at the peripheral area of the water phantom over the monopole array without decoupling treatment.

Additionally, an L-shaped capacitive matching circuit for the monopole element is proposed and investigated, showing a significant improvement of the reflection coefficients S₁₁ (from –12.7 to –28 dB). Better impedance matching could result in increased transmit efficiency, higher SNR, and decreased NF of the conventional 50 Ω preamplifier. Therefore this design can be expected to have a possible transmit power reduction and the SNR improvement over the monopole elements without matching circuits.

Although the ICE/magnetic wall decoupling method is validated for only the monopole array in this work, this decoupling method is more general and should be suitable for other kinds of radiative arrays, e.g., dipole coil arrays. This study has paved the way for designing ICE-decoupled multi-channel volume-typed radiative array for human MR imaging.