Population shift of binding pocket size and dynamic correlation analysis shed new light on the anticooperative mechanism of P_II protein

Abstract

P_II protein is one of the largest families of signal transduction proteins in archaea, bacteria, and plants, controlling key processes of nitrogen assimilation. An intriguing characteristic for many P_II proteins is that the three ligand binding sites exhibit anticooperative allosteric regulation. In this work, P_II protein from Synechococcus elongatus, a model for cyanobacteria and plant P_II proteins, is utilized to reveal the anticooperative mechanism upon binding of 2-oxoglutarate (2-OG). To this end, a method is proposed to define the binding pocket size by identifying residues that contribute greatly to the binding of 2-OG. It is found that the anticooperativity is realized through population shift of the binding pocket size in an asymmetric manner. Furthermore, a new algorithm based on the dynamic correlation analysis is developed and utilized to discover residues that mediate the anticooperative process with high probability. It is surprising to find that the T-loop, which is believed to be responsible for mediating the binding of P_II with its target proteins, also takes part in the intersubunit signal transduction process. Experimental results of P_II variants further confirmed the influence of T-loop on the anticooperative regulation, especially on binding of the third 2-OG. These discoveries extend our understanding of the P_II T-loop from being essential in versatile binding of target protein to signal-mediating in the anticooperative allosteric regulation. Proteins 2014; 82:1048–1059.

INTRODUCTION

The P_II protein, as one of the most widely distributed families of signal transduction proteins, has key functions in terms of regulation of central metabolic processes by sensing signals from the carbon, nitrogen and energy status within the cells.^1,2 It is able to regulate the activity of not only key metabolic and regulatory enzymes, but also transcription factors and even transport proteins.^3–5 The control of P_II protein on nitrogen-storage metabolism is due to the binding of P_II protein toward N-acetyl-l-glutamate kinase (NAGK) in cyanobacteria and plants which controls the biosynthesis of arginine.^6–8 The binding of P_II is a response to the cellular concentration of central metabolites ATP, ADP, and 2-oxoglutarate (2-OG), which reflect the cellular energy, carbon and nitrogen abundance.^2,5,9 These metabolites bind to P_II in an interdependent manner, thereby transmitting metabolic information into structural states.^2,10 Another intriguing characteristic is that the three ATP binding sites and the three 2-OG binding sites each exhibit negative cooperativity, which allows P_II to sense a wide range of metabolite concentrations.^10–13

As revealed from numerous crystal structures, P_II proteins show a highly conserved three-dimensional structure. It is composed of homotrimers with each subunit of 12 to 13 kDa and two ferredoxin-like fold-containing cores (βαβ-βαβ) (Fig. 1).^2,14 There is a long and flexible T-loop extending outward from each subunit and it is believed that the flexibility of these T-loops is essential to mediate the versatile functions of P_II protein.² Furthermore, each subunit comprises two small loops (B and C loop) in the intersubunit clefts, which take part in the binding of ATP or ADP.^15–17 In the presence of Mg-ATP, up to three 2-OG molecules can bind per trimer with the exception that Arabidopsis thaliana P_II, which binds 2-OG also in the presence of ADP.^18,19 From the crystal structure of the Synechococcus elongatus P_II protein in complex with ATP, Mg²⁺, and 2-OG, the site of 2-OG was located in the vicinity between the subunit clefts and the base of the T loop.¹¹ More importantly, besides P_II trimers with all the three sites fully occupied, structures with one or two 2-OG molecules bound per P_II trimer were also obtained, which make it possible to reveal the basis for the anticooperative allosteric regulation upon binding of 2-OG.

Figure 1.

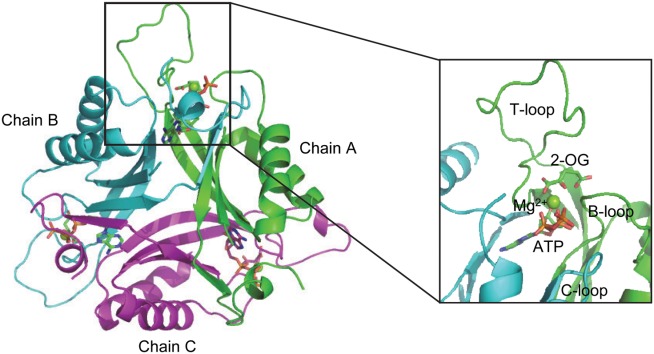
Overall structure of P_II protein with ligand in the binding pocket. Chain A, B and C are shown in cartoon and colored in green, cyan and purple, respectively. ATP and 2-OG are shown in sticks; Mg²⁺ in sphere.

In this work, the P_II protein from S. elongatus is utilized to reveal the anticooperative allosteric mechanism from the point of view of population shift. Population shift or re-distribution of protein conformational states has been proved to be a powerful concept for rationalizing binding mechanisms and allosteric regulations.^20–23 It emphasizes that the process of ligand binding merely shifts the population of conformational states in a dynamic ensemble of the protein. Recent experiments also show that conformational states in a pre-existing equilibrium can influence protein function.^24–26 To this end, we first identified residues that contribute greatly to the binding of 2-OG and based on this, we then proposed a method to define the binding pocket size. The anticooperative mechanism can be well revealed by considering the population shift of the binding pocket size. Furthermore, a new algorithm is developed based on dynamic correlation analysis and used to identify residues that mediate the allosteric regulation upon the binding of 2-OG with high probability. Predictions concerning “unexpected” roles of residues on the P_II T-loop for signal mediation in the anticooperative process are experimentally verified.

MATERIALS AND METHODS

Structures and systems preparation

Crystal structures of P_II protein with different number of bound 2-OG (PDB code: 2XZW) were employed. Residues missed in the original PDB file were added using Modeller 9v2²⁷ and the enumeration algorithm²⁸ was used to increase the accuracy of the calculated structure. Three P_II protein structures with different states of ligand binding were constructed: P_II protein with 2-OG only in the binding pocket of chain A (P_II^OG1); P_II protein with 2-OG in both chain A and B (P_II^OG2); P_II protein with 2-OG in both chain A, B and C (P_II^OG3). For each case, ATP and Mg²⁺ exist in each of the subunits.

Each structure was first neutralized by adding sodium counter ions randomly with the xLeap module of Amber 10.0²⁹ and then solvated in a rectangular box of TIP3P water molecules³⁰ with a solute-wall distance of 12 Å. The solvated systems were energy-minimized prior to the molecular dynamics simulations. Each system was minimized by six consecutive rounds with each round of 1500 steps. Harmonic constraints were applied to all non hydrogen atoms with the strength of 500, 400, 300, 200, 100, and 0 kcal mol⁻² at each round. After that, the system was slowly heated from 0 K to 300 K in 120 ps. Then the systems were conducted in a NVT ensemble for 50 ps followed by a 100 ps simulation in a NPT ensemble.

Molecular dynamics simulations

The molecular dynamics simulations were performed with the Sander module Amber 10.0 using the force field developed by Cornell et al.³¹ with a periodic boundary condition in the NPT ensemble at 300 K. Langevin dynamics was used with the collision frequency of 1.0 ps⁻¹ and constant pressure of 1 atm. The Shake algorithm³² was applied to fix all covalent bonds. The Particle Mesh Ewald (PME) method³³ was used to treat long-range electrostatic interactions. A residue-based cutoff of 10 Å was applied to the noncovalent interactions. No constraint was applied during molecular dynamics simulations which lasted 1.0 ns for each system to get an equilibrium state. A time step of 2.0 fs was used and the coordinates of the simulated complexes were saved every 1.0 ps.

Binding free energy calculations

The MM-GBSA approach,³⁴ which is implemented in the Amber program, was applied to compute the binding free energy (ΔG_binding) of ligand. The ΔG_binding was determined according to the following equations:

1

2

3

where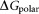 is the free energy for the polar interaction; 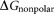is for the nonpolar interaction; 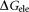is the electrostatic interaction free energy; 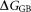is the electrostatic solvation free energy; 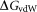is the van der Waals interaction energy, 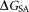is the nonpolar solvation free energy. Default parameters were used to calculate the overall binding free energy and its decomposition at residue level.

Hydrogen-bond interactions analysis

Since the molecular dynamics simulations include hydrogen atoms, it is possible to make an analysis of hydrogen-bond properties. The hydrogen-bond interactions, which were performed with the Ptraj module of Amber 10.0, are assumed to be present if the participating heavy atoms are 3.5 Å apart and the angle formed between the heavy atoms and donated hydrogen is larger than 120°.

Dynamic correlation analysis

Dynamic correlation analysis was employed to identify residues that mediate the signal transduction during the anticooperative process. This approach is based on the correlation coefficient between each pair of residues obtained from the trajectory of molecular dynamics simulations. The correlation coefficients are calculated according to the following equation:

4

where C(R_s,R_t) is the correlation coefficient between residues s and t. R_s and R_t are the coordinates of residues s and t; <R_s>_N and <R_t>_N are the average coordinates of residues s and t calculated based on the whole trajectory and N is the total number of snapshots recorded in the trajectory during the molecular dynamics simulations.

The residue m that mediates the signal transduction between residues s and t with the largest probability is identified according to the following equation:

5

where C(R_s,R_i,R_t) is the correlation coefficient between residues s and t mediated by residue i; C(R_s,R_i) is the correlation coefficient between residues s and i; C(R_i,R_t) is the correlation coefficient between residues i and t; | | indicates absolute value; N is the total residue number of the protein. The residue holding the maximal value of C(R_s,R_i,R_t) is considered to mediate the signal transduction between residues s and t with the largest probability (C(R_s,R_m,R_t)). After the first round, residues mediate the signal transduction between residues s and m with the largest probability is to be discovered and the same for residues m and t. The procedure is continued until no more residues could be found.

Overexpression and purification of P_II variants

Site-directed mutagenesis of glnB and cloning into the Strep tag fusion vector pASK-IBA3 (IBA GmbH, Göttingen, Germany) was performed and described previously.³⁵ The vectors were transformed into Escherichia coli strain RB9060³⁶ and purified using affinity chromatography as described earlier.⁷

ITC measurements

ITC experiments were performed using a VP-ITC microcalorimeter (MicroCal) in buffer containing 10 mM Na₂HPO₄, 1.8 mM KH₂PO₄, 25 mM NaCl, 10 mM KCl, 1 mM MgCl₂ and 1 mM ATP (pH 7.5); 2 mM of the titrant 2-OG were dissolved in the same buffer. After an initial 2 µL injection 6 µL were injected 45 times into the 1.4285 mL cell with stirring at 155 rpm at a temperature of 25°C. The P_II concentration in the cell was 33 µM (trimer concentration). The binding isotherms were calculated from received data and fitted to a three-site binding model with the MicroCal ORIGIN software (Northampton, MA) as indicated.

RESULTS

Definition of 2-OG binding pocket size

Structures of P_II trimers with all three sites fully occupied and with one or two bound molecules of 2-OG not only shed first light on structural basis of anticooperativity but help revealing the anticooperative mechanism from perspectives of dynamics. The population shift of binding pocket size employed here to demonstrate the allosteric effects upon 2-OG binding, is defined by identifying residues that contribute greatly to the binding of 2-OG through decomposition of binding free energy followed by analysis of hydrogen-bond interactions.

It is reported that 2-OG is bound to a Mg²⁺ ion, which is coordinated by three phosphates of ATP, and by ionic interactions with the highly conserved residues Gln39 and Lys58 together with the B- and T-loop backbone interactions.¹¹ Table 1 lists the overall binding free energy for structures with different ligand binding states. The slight differences among their overall binding free energy mainly result from the polar interactions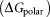, namely the electrostatic interaction energy 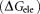and the electrostatic solvation free energy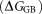. When examining the energy contribution of each residue [Fig. 2(A)], it can be seen that Arg9, sequence Phe36-Gly41 of the P_II T-loop, Lys58 and Gly87 are the main contributors to the energy differences upon 2-OG binding. Among these residues, Arg9 makes contributions to the binding of the first and third 2-OG although it does not make large contributions when the second 2-OG binds to the binding pocket. Gln39 influences the ligand binding most in the sequence Phe36-Gly41. It is evident that both Lys58 and Gly87 make large and positive contributions to the ligand binding. Therefore, Arg9, Gln39, Lys58, and Gly87 were finally chosen as the residue-level indicators to describe the size of the binding pocket.

Table I.

Binding Free Energies Computed by the MM-GBSA Method upon Binding of the First, Second, and Third 2-OG Toward the Binding Pocket of Chain A, B, and C of P_II Protein (Unit: kcal mol⁻¹)

Energy terms	PIIOG1	PIIOG2	PIIOG3
ΔGele	−295.3	−344.0	−236.4
ΔGvdw	−2.6	−2.7	−4.2
ΔGMMa	−297.9	−346.7	−240.6
ΔGnp	−2.7	−2.7	−2.7
ΔGGB	226.4	277.4	181.8
ΔGsolvb	223.7	274.7	179.0
ΔGbindingc	−74.1	−72.0	−61.6

^aΔG_MM = ΔG_ele + ΔG_vdw.

^bΔG_solv = ΔG_np + ΔG_GB.

^cΔG_binding= ΔG_MM + ΔG_solv.

Figure 2.

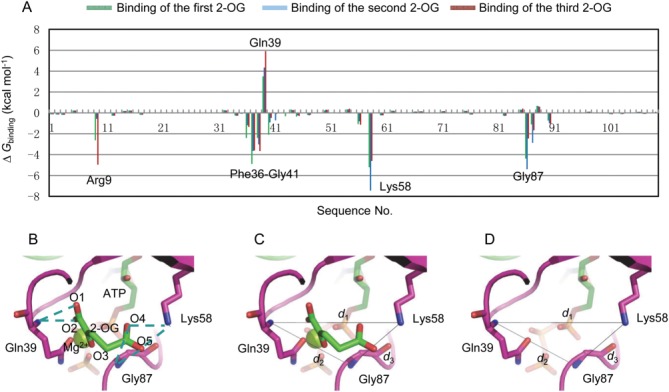
Definition of the binding pocket size. (A) Binding free energy decomposed at residue level for P_II protein. (B) Hydrogen bonds interactions (dashed lines) between Gln39, Lys58, Gly87, and 2-OG. Definition of binding pocket size by d₁, d₂ and d₃ with (C) or without (D) the binding of 2-OG. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

Since hydrogen-bond interactions always play an important role for ligand binding, hydrogen-bond interactions analysis is employed to identify atoms that can be used to describe the size of the binding pocket at an atomic level. The analysis was conducted between the residues Arg9, Gln39, Lys58, Gly87, and 2-OG. As shown in Figure 2(B), hydrogen-bonds are formed between the main chain N atom of Gln39 and O1 (O2) of 2-OG, the side chain N atom of Lys58 and O4 (O5) of 2-OG. There are also hydrogen-bonds between the main chain N atom of Gly87 and the O4 (O5) of 2-OG. However, no hydrogen-bond is formed between Arg9 and 2-OG due to their long distance although Arg9 also makes contributions to the binding of 2-OG because of its positively charged side chain. Thus, the main chain N atom of Gln39, the side chain N atom of Lys58 and the main chain N atom of Gly87 are selected as the atom-level indicators to describe the binding pocket size. Then three distance parameters d₁ (the distance between the main chain N atom of Gln39 and the side chain N atom of Lys58), d₂ (the distance between the main chain N atom of Gln39 and the main chain N atom of Gly87) and d₃ (the distance between the side chain N atom of Lys58 and the main chain N atom of Gly87) are defined to reflect the size of the binding pocket with or without the binding of 2-OG [Fig. 2(C,D)].

Population shift of binding pocket size upon 2-OG binding

With the definition of the binding pocket size, it is possible to quantitatively investigate the population shift of the binding pockets in response to the binding of 2-OG. In order to investigate the anticooperativity in the context of distribution shift from unbound to bound P_II, molecular dynamics simulations of unbound P_II trimer (PDB code: 1QY7) were carried out and the distributions of the binding pocket size (d₁, d₂, d₃) were calculated (Fig. 3). It is found that although the distances measured from the crystal structure show that they are the same among the monomers (purple dots in Fig. 3), the simulation results show some difference. For instance, d₁ is 10.07 Å and 10.05 Å for chain A and chain B, whereas it is 9.65 Å for chain C. d₂ of chain A (9.85 Å) is somewhat larger than that of chain B (8.10Å) and chain C (8.17Å). There are also difference for d₃ among chain A, chain B and chain C.

Figure 3.

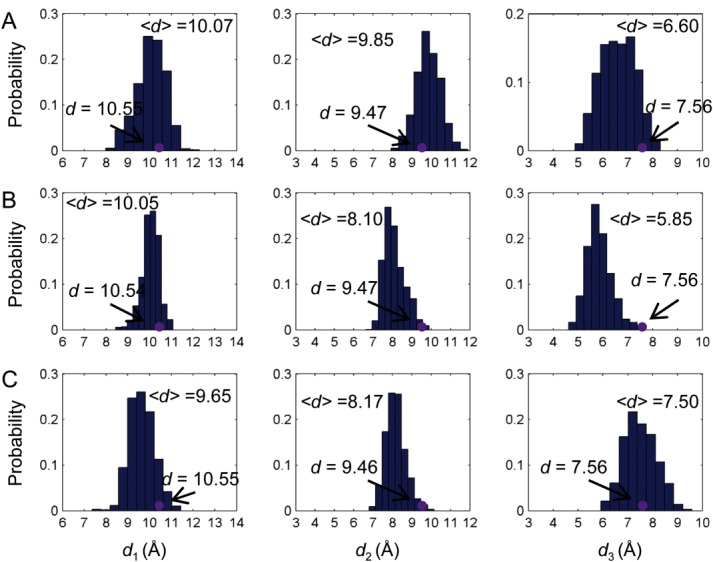
Distribution of the binding pocket size without ligand binding. Distance distributions of d₁, d₂ and d₃ for chain A (A), chain B (B), and chain C (C). Purple dots are distances measured based on the crystal structures. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

Upon binding of the first 2-OG towards chain A of P_II trimer, the shift of the binding pocket size of chain B and C is determined and compared with that of chain A. As shown in Figures 3(B) and 4(A), the pocket size of chain B becomes larger as indicated by the distance parameters defined above. In particular, distance d₁ increases from 10.05 Å to 11.47 Å although d₂ and d₃ show little changes. By contrast, the binding pocket of chain C becomes smaller upon the binding of the first 2-OG. This can be seen from the distance d₂, which decreases from 8.17 Å to 6.29 Å and d₃ which deceases from 7.50 Å to 5.62 Å [Figs. 3(C) and 4(B)]. There are only slight changes in terms of d₁.

Figure 4.

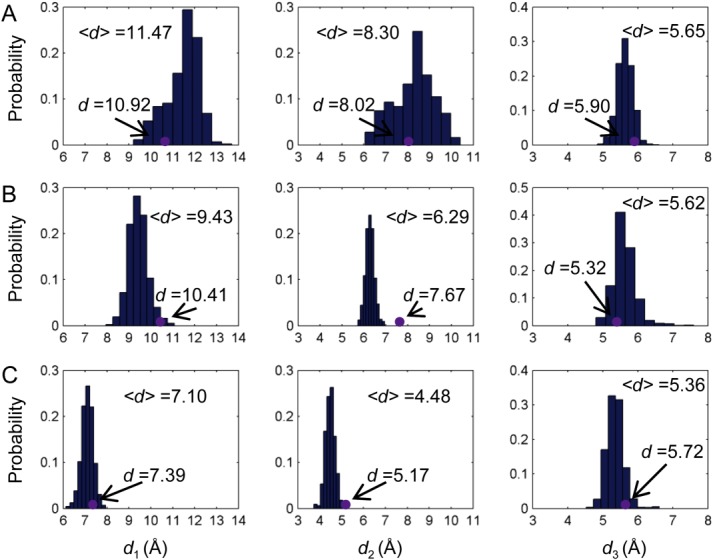
Distribution of the binding pocket size upon ligand binding. Distance distributions of d₁, d₂, and d₃ for chain B (A) and chain C (B) when the first 2-OG binds towards the binding pocket of chain A as well as distance distributions for chain C (C) when the second 2-OG binds toward the binding pocket of chain B. Purple dots are distances measured based on the crystal structures. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

When the second 2-OG binds to chain B, the binding pocket size of chain C shrinks further because of the decrease of d₁ from 9.43 Å to 7.10 Å and d₂ from 6.29 Å to 4.48 Å [Fig. 4(C,D)]. Again the distance change of d₃ is very small, only from 5.62 Å to 5.72 Å. The small distance change of d₃ upon the binding of either the first or the second 2-OG may be due to the fact that Lys58 and Gly87 are located in more stable structure areas than Gln39, which resides in the flexible T-loop. The large distance shifts of d₁ and d₂ in response to the ligand binding illustrate the role of P_II T-loop in the anticooperative process as well as providing further proof of its role in the ligand binding process.

By defining the binding pocket size with residues that make great contributions to the ligand binding, the anticooperative mechanism of P_II protein in response to 2-OG can be well explained as a population shift of the binding pocket size (Fig. 5). Shifts of the binding pocket to become either bigger (in the case of chain B upon the binding of the first 2-OG) or smaller (in the case of chain C upon the binding of the first or the second 2-OG) will definitely decrease the binding opportunities of 2-OG and result in the negative cooperativity of P_II trimer. Results from the ITC measurements suggest that the binding of 2-OG to the first binding site is driven by both enthalpy and entropy (ΔH < 0, TΔS > 0) with the enthalpy having the bigger impact. The situation is similar for the binding to the second binding site, but here the entropy has the bigger impact. The binding of 2-OG to the third binding site, on the other hand, is clearly driven by the enthalpy (ΔH < 0, TΔS < 0). The thermodynamic considerations fit well with the binding model illustrated by the change of the binding pocket size. They imply that occupation of the first and second site lead to a more disordered conformation because of the change of the binding pocket size, whereas occupation of the third site gives a more ordered state because all three sites are now occupied with 2-OG. That is why this reaction is exclusively enthalpy driven.

Figure 5.

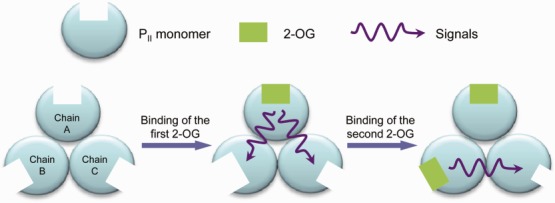
Sketch of the change of binding pocket size upon the binding of 2-OG. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

Residues mediating the anticooperative signal transduction

Dynamic correlation analysis [Eq. 4] was first used to obtain the correlation coefficients between each pair of residues when the first 2-OG binds toward the binding pocket of chain A [Fig. 6(A)] and the second 2-OG toward chain B [Fig. 6(B)]. The number of residues correlated with high correlation coefficient (>0.5) provides a quantitative parameter about the signal-mediating function of each residue [Fig. 6(C,D)]. Then, residues that mediate the signal transduction process with high probability were identified using the algorithm derived from the dynamic correlation analysis with residues identified to illustrate the binding pocket size as R_s or R_t in Eq. (5).

Figure 6.

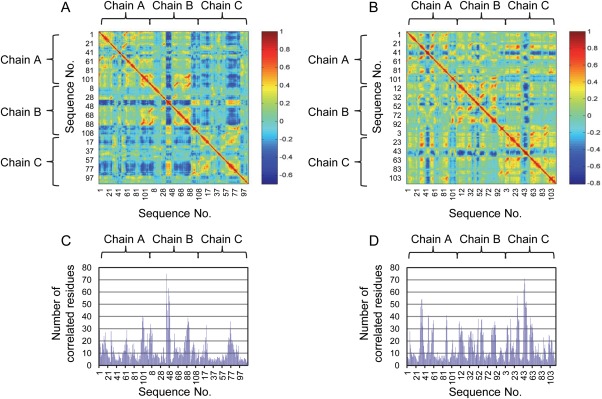
Dynamic correlation analysis of P_II protein upon binding of 2-OG. Correlation coefficients for each pair of residues upon the binding of the first (A) and the second (B) 2-OG in the case of wild-type P_II protein. Number of correlated residues with the correlation coefficient value over 0.5 upon the binding of the first (C) and the second (D) 2-OG. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

By comparing Figure 6(A,C), we found that the sequence 41 to 50 of chain B shows the largest numbers of correlated residues upon the binding of the first 2-OG. Besides, the sequence 81 to 94 of chain B and the sequence 70 to 81 of chain C also show large numbers of correlated residues. Particularly, 21 residues are primarily involved in the signal transduction process from the binding pocket of chain A to that of chain B, of which 7 belong to chain A and 14 belong to chain B [Table 2 and Fig. 7(A)]. In the case of signaling process from chain A to C, 13 residues contribute to the process, wherein 8 of them belong to chain A and 5 of them belong to chain C [Table 2 and Fig. 7(B)]. Among these residues, Gln39, Lys40, Ser49, Lys58, Leu59, Gly87, and Asp88 are involved in the signal transduction from chain A to both chain B and C. In the case when the second 2-OG binds towards the binding pocket of chain B [Fig. 6(B,D)], the sequence 43 to 51 of chain C shows the largest numbers of correlated residues. Besides, the sequence 29 to 40 of chain A also shows large numbers of correlated residues. In particular, 14 residues contribute largely to the signal transduction process from chain B to C, of which 6 belong to chain B and 8 belong to chain C [Table 2 and Fig. 7(C)].

Table II.

Major Residues Mediating the Signal Transduction from the Binding Site of Chain A to that of Chain B and Chain C upon Binding of the First 2-OG as well as from Chain B to Chain C upon Binding of the Second 2-OG

Residue no.	Chain A to B			Chain A to C			Chain B to C
	A	B	C	A	B	C	A	B	C
Phe36a,b				√
Gly37a,b				√
Arg38a,b	√	√
Gln39a,b	√	√		√		√		√	√
Lys40		√		√
Gly41a,b		√
Gln42a,b		√
Thr43		√
Glu44a		√
Tyr46a,b									√
Arg47a,b									√
Gly48a,b	√								√
Ser49a,b	√			√				√	√
Leu56a				√
Lys58a,b	√	√		√		√		√	√
Leu59a,b		√				√
Ile86		√						√
Gly87a,b	√	√		√		√		√	√
Asp88a,b	√					√			√
Lys90		√
Ile91		√
Phe92		√
Arg103 a								√

The symbol √ indicates to which chain the residue belongs to in each case.

^aResidues identified within the time period of 1.0 and 1.5 ns.

^bResidues identified within the time period of 1.0, 1.5, and 2.0 ns.

Figure 7.

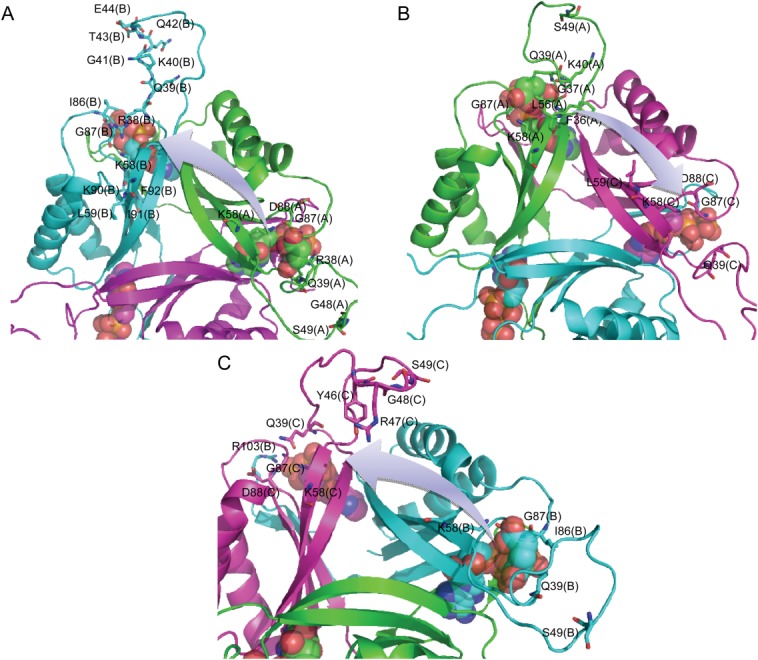
Major residues mediating the signal transduction from the binding site of chain A to that of chain B (A) and chain C (B) upon binding of the first 2-OG as well as from chain B to chain C (C) upon binding of the second 2-OG. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

It is not surprising to find that Gln39, Lys58, and Gly87 are involved in each case of ligand binding state due to the fact that they comprise the binding pocket. Besides these three residues, Ser49 and Asp88 are also involved in not only the signaling process from the binding pocket of chain A to B and C upon the binding of first 2-OG but also from chain B to C upon the binding of second 2-OG. Especially, it is interesting to find that Ser49, which is located at the T-loop of P_II protein and is an important site to regulate the binding of S. elongatus P_II toward its target protein through phosphorylation^7,37 also plays a key role in the signal transduction process. In addition, residues Lys40 and Leu59 take part in the signaling process from the binding pocket of chain A to B and C upon the binding of first 2-OG. Residues Gly48 and Ile86 take part in the signaling process from the binding pocket of chain A to B upon the binding of first 2-OG and chain B to C upon the binding of second 2-OG.

Since allosteric proteins undergo structural fluctuations over a wide range of timescales (from femtoseconds to milliseconds or longer), residues mediating signal transduction may differ during different periods of time. The signal-mediating residues identified in this work are based on correlation matrix obtained within the first nanosecond upon binding of ligand. To test whether similar signal-mediating residues can be indentified from correlation matrix obtained from a longer simulation, molecular dynamics simulations were carried out for another nanosecond. Then signal-mediating residues were identified within the time period of 1.5 and 2.0 separately and compared with the results from the first nanosecond (Table 2). As expected, most of the residues keep their function in the signal transduction process. Among the 23 residues identified within the time period of 1.00 ns, 17 are identified within the time period of 1.0 and 1.50 ns. Fourteen are identified within the time period of 1.00, 1.50, and 2.0 ns. The identification of P_II T-loop residues, especially Ser49 and its neighboring residues, indicates their significant roles in the signaling process during the alloteric regulation.

Anticooperativity of P_II variants by 2-OG

Considering the specificity of Ser49 in the function of P_II protein, two variants P_II-Ser49Gly and P_II-Ser49Asp were constructed and their anticooperativity was measured by isothermal titration calorimetry (ITC) approach. The former is constructed to investigate the role of the side chain in the signal transduction process and the later is used to mimic the phosphorylation modification of Ser49. As shown in Figure 8, the anticooperativity of P_II-Ser49Gly is decreased by approx. 19 % upon the binding of the second 2-OG (Kd₂/Kd₁ from 3.2 to 2.6). The anticooperativity is weakened by approximately 31% upon the binding of the third 2-OG (Kd₃/Kd₁ from 22.1 to 15.3). In the case where Ser49 is replaced by aspartate acid, the anticooperativity (Kd₂/Kd₁) is slightly changed from 3.2 to 3.5 upon the binding of the second 2-OG. However, the anticooperativity is strengthened by 19.9% upon the binding of the third 2-OG (Kd₃/Kd₁ from 22.1 to 26.5) which means P_II-Ser49Asp is able to sense a wider range of metabolite concentrations than the wild type if it is expressed in the organism. Moreover, the influence of site mutation on the binding of the third 2-OG is stronger than the second 2-OG as expected by the dynamic correlation analysis.

Figure 8.

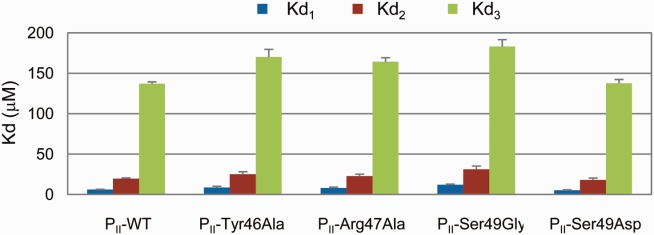
Anticooperativity of P_II variants by 2-OG measured using ITC method. [Color figure can be viewed in the online issue, which is available at wileyonlinelibrary.com.]

In order to further confirm the role of T-loop in the anticooperative allosteric regulation of P_II, two neighboring sites (Tyr46 and Arg47) that are also located in the mid of the T-loop were chosen and tested. Since these two sites hold large and even positive charged (in the case of Arg47) side chain, both of them were mutated to alanine to decrease the contributions of their side chains and the anticooperativity of the variants were measured using the ITC method. From Figure 8, it can be seen that both variants show the same influence on the anticooperativity upon the binding of the second 2-OG (Kd₂/Kd₁ from 3.2 to 2.9 and 2.8, respectively). However, Tyr46Ala shows a little stronger influence than Arg47Ala on the anticooperativity upon the binding of the third 2-OG. Tyr46Ala decreases the anticooperativity (Kd₃/Kd₁) from 22.1 to 19.6 while the anticooperativity is decreases from 22.1 to 20.5 in the case of Arg47Ala. Compared with Ser49, Tyr46 and Arg47 show less influence on the anticooperativity of P_II. This is also expected by the dynamic correlation analysis. From these experimental results it can be seen that although the T-loops are long and extend outward from each subunit, they indeed take part in the anticooperative allosteric regulation of P_II upon binding of 2-OG. Modifications of residues located at the T-loop can influence the anticooperativity positively or negatively.

DISCUSSION

The concept of protein allostery went back to the MWC model (also known as the concerted model or symmetry model) put forth by Monod et al. and the KNF model (also known as the sequential or “induced fit” model) described by Koshland et al.^38,39 Both sought to account for allostery based on gross properties of the transition between two well-defined end states. The two models differ most in their assumptions about subunit interaction and the preexistence of both states. The concerted model of allostery postulates that subunits are connected in such a way that a conformational change in one subunit is necessarily conferred to all other subunits. Thus, all subunits must exist in the same conformation. The sequential model of allosteric regulation assumes that ligand molecules bind via an induced fit protocol, which converts a subunit from one state to another. However, it does not propagate the conformational change to adjacent subunits. Instead, ligand-binding at one subunit only slightly alters the structure of other subunits so that their binding sites are more receptive or rejective to the ligand. In the case of P_II protein, it is known that 2-OG binds in a sequential manner and the binding of 2-OG at one subunit decreases the binding of 2-OG at the other subunits.¹¹ Thus, it seems that the KNF model is more suitable to describe the anticooperativity of P_II by 2-OG. Nevertheless, the underlying mechanism behind the sequential binding of 2-OG is still unclear. Thus it is necessary to get a deeper understanding of the anticooperative allosteric regulation of P_II protein in order to reveal the underlying mechanism. To this end, new models and approaches are needed to shed more light into the anticooperativity of P_II by 2-OG.

Considering the fact that the function of a protein is ultimately governed by its dynamics in most cases, proteins are inherently dynamical molecules that undergo structural fluctuations over a wide range of timescales.^40,41 More recent thermodynamic models of allostery has expanded the understanding and definition of allostery by emphasizing that rather than only in two conformational states, proteins exist in ensembles. Thus, allosteric regulation is considered to be accompanied by population shift in conformational ensembles.^42–44 In this work, the concept of binding pocket size is proposed to describe the conformational ensembles. From the re-distribution of the distances that define the binding pocket size, the population shifts proceeding upon binding of 2-OG are well characterized. These discoveries shed now light into the anticooperative allosteric regulation of P_II protein by first confirming that the binding of 2-OG at one subunit changes the population of the binding pocket site of the other subunits, which subsequently influence the binding of 2-OG at the other subunits. Furthermore, this influence is conducted in an asymmetric manner due to the different population shifts of the second and the third binding pocket size upon binding of the first 2-OG.

To uncover signal transduction processes within allosteric proteins, several approached have been developed so far. A co-evolutionary approach, which is based on sequence information of the protein family, was employed to identify amino acid interactions in each protein family.^45,46 Another novel approach to reveal intramolecular signal transduction networks was proposed based on a new protein dynamics model of energy dissipation.^47,48 Since correlations in the atomic displacements (dynamic correlation analysis based on fluctuation dynamics) indicate collective motion and are therefore of potential relevance to protein function, correlated motions have been detected in molecular dynamics simulations and used to make deductions concerning dynamical aspects of protein function.^49–54 In a recent study, molecular dynamics simulation was coupled with co-evolutionary analysis to identify a key residue-residue interaction network which is responsible for the allosteric regulation and signal transduction within allosteric enzymes.^55,56 In this work, a new approach derived from dynamic correlation analysis is utilized to reveal how the signal is transferred from the binding site of one subunit to the other subunits upon binding of ligand. This approach highlighted amino acid residues mediating the signaling process with high probability. It was surprising to find the important role of P_II T-loop in the signal transduction process since the long T-loop extends outward from each subunit and is believed to be responsible for mediating the binding of P_II with its target proteins.^35,57 In particular, it is found that Ser49 also takes part in the allosteric process. The particular impact of this site relies on the fact that it is an important site to regulate the binding of P_II towards its target protein N-acetyl-l-glutamate kinase through phopshorylation.⁷ Experimental results further confirmed the role of T-loop in influencing the anticooperativity of P_II, especially on binding of the third 2-OG. These resuls not only indicate that the population shift of the binding pocket size is highly correlated to the ligand binding through protein dynamics but also illustrate the versatile function of P_II T-loop in the anticooperative allosteric regulation. From the perspective of application, it is possible to alter the sensitivity of P_II protein to a much wider range of metabolite concentrations by modifying the residues discovered through the new dynamic correlation analysis approach.

CONCLUSIONS

The dynamics of the binding pocket is well characterized by defining its size with residues that contribute greatly to the binding of 2-OG. The anticooperative mechanism of P_II protein upon the binding of 2-OG can be well explained by population shift of the binding pocket size. Residues mediating the anticooperative regulation with high probability are discovered by using the new algorithm developed based on dynamic correlation analysis. It is found that Ser49, which is located in the flexible T-loop of P_II, plays an important role in the allosteric signaling, as illustrated by experimental results of the anticooperativity of the variants P_II-Ser49Gly and P_II-Ser49Asp. Overall, the results presented here extend our understanding of the P_II T-loop from being essential in versatile binding of target protein to signal-mediating in the anticooperative allosteric regulation.