Skip to main content
PMC home page
Nature Portfolio logoLink to Nature Portfolio
. 2015 Jan 13;6:6028. doi: 10.1038/ncomms7028

Engineering two-dimensional superconductivity and Rashba spin–orbit coupling in LaAlO3/SrTiO3 quantum wells by selective orbital occupancy

Gervasi Herranz 1,a, Gyanendra Singh 2, Nicolas Bergeal 2, Alexis Jouan 2, Jérôme Lesueur 2, Jaume Gázquez 1, María Varela 3,4, Mateusz Scigaj 1,5, Nico Dix 1, Florencio Sánchez 1, Josep Fontcuberta 1
PMCID: PMC4308716  PMID: 25583368

Abstract

The discovery of two-dimensional electron gases (2DEGs) at oxide interfaces—involving electrons in narrow d-bands—has broken new ground, enabling the access to correlated states that are unreachable in conventional semiconductors based on s- and p- electrons. There is a growing consensus that emerging properties at these novel quantum wells—such as 2D superconductivity and magnetism—are intimately connected to specific orbital symmetries in the 2DEG sub-band structure. Here we show that crystal orientation allows selective orbital occupancy, disclosing unprecedented ways to tailor the 2DEG properties. By carrying out electrostatic gating experiments in LaAlO3/SrTiO3 wells of different crystal orientations, we show that the spatial extension and anisotropy of the 2D superconductivity and the Rashba spin–orbit field can be largely modulated by controlling the 2DEG sub-band filling. Such an orientational tuning expands the possibilities for electronic engineering of 2DEGs at LaAlO3/SrTiO3 interfaces.

Inline graphicTwo-dimensional electron gases at oxide interfaces induce exotic behaviours. By studying samples with different crystal orientation, Herranz et al. show that the extension and anisotropy of the oxide quantum well properties can be controlled through selective sub-band filling via orientational tuning.

The confinement of electron orbitals over small scales provides a pathway to tailor the electronic properties of a quantum system. Restricting the motion of electrons within planes of different crystal orientation affords additional routes to reorganize the electronic band structure. Such strategies have been used to engineer the electronic and optical properties of II–VI and III–V semiconductor quantum wells, where s- and p- orbitals are involved1,2,3. Yet, two-dimensional (2D) electron gases (2DEGs), comprising d-electrons instead of s or p, have come into the limelight over about the last 10 years4,5,6,7,8,9,10,11, opening novel perspectives that are inaccessible for more traditional materials. In particular, the narrow bandwidth of d-states in transition metal oxide quantum wells promote correlated states—for example, magnetism and superconductivity—that are unseen in conventional semiconductors. Interestingly, the extreme confinement, over just a few unit cells, enables full electrostatic control of correlated 2DEG states, allowing access to new physics and paving the way to new device concepts. Particularly, these 2D electron systems have been found to be superconductive7,12,13,14, with 2D superconductivity largely modulated by electric gates15,16. The 2D character of the superconductivity has led to phenomena not observed in the 3D regime, such as magnetic enhancement of superconductivity17, violation of the paramagnetic Pauli limit for the upper critical fields18, quantum phase transitions19 or multiple quantum criticality20. The intricacy of all these complex phases and the evidence of the role of electron correlations have often prompted the use of the concept of electron liquids to designate these electron systems21.

The interface between LaAlO3 and SrTiO3 is the oxide quantum well par excellence. Initially, the research on LaAlO3/SrTiO3 quantum wells was restricted to the (001)-plane of the perovskite unit cell4,14,15. Remarkably, recent investigations have uncovered that interface conductivity also appears along other directions, such as (110) (refs. 22, 23) and (111) (ref. 22). The selective confinement of electrons within planes of different crystal orientation expands vigorously the possibility of fine-tuning the 2DEG sub-band hierarchy and, thereof, the physical properties. Along this line, we have recently demonstrated that crystal symmetry is an extra degree of freedom to realize different 2DEG band reconstructions at the LaAlO3/SrTiO3 interface, by imposing distinctive orbital hierarchies on (001)- and (110)-oriented quantum wells and enabling the selective occupancy of states of different symmetry24. More specifically, we have uncovered that the degeneracy within the t2g sub-band—which forms the backbone of the 2DEG structure in LaAlO3/SrTiO3 wells—is broken in reversed ways depending on the crystal orientation: for (001)-oriented 2DEGs the dxy orbitals have the lowest energy, while along (110) the bottommost levels have instead a dxz/dyz character24. Recent experiments on uncapped (110) SrTiO3 surfaces also found the same hierarchy25. This orbital reconfiguration provides an excellent playground to test the link between orbital symmetry and complex correlated states, provided that we understand exactly the implications that such 2DEG band engineering has for the physical properties of the quantum wells.

In this work, we present evidence that the selection of the orbital symmetries in the 2DEG sub-band structure triggers some nontrivial and extensive modifications of the electronic properties of quantum wells at the LaAlO3/SrTiO3 interface. First, we demonstrate that the orbital reconfiguration implies a modulation of 2DEG spatial extension and, as a result, the anisotropy of the 2D superconductivity is largely affected by crystal orientation. Second, we show that the effects of sub-band engineering are influential on the spin–orbit coupling and the concomitant Rashba effect, opening new pathways to tune the spin–dependent transport in LaAlO3/SrTiO3 quantum wells. These findings open fresh perspectives to understand the fundamental connection between orbital symmetry and the electronic phases at LaAlO3/SrTiO3 interfaces.

Results

Structural characterization

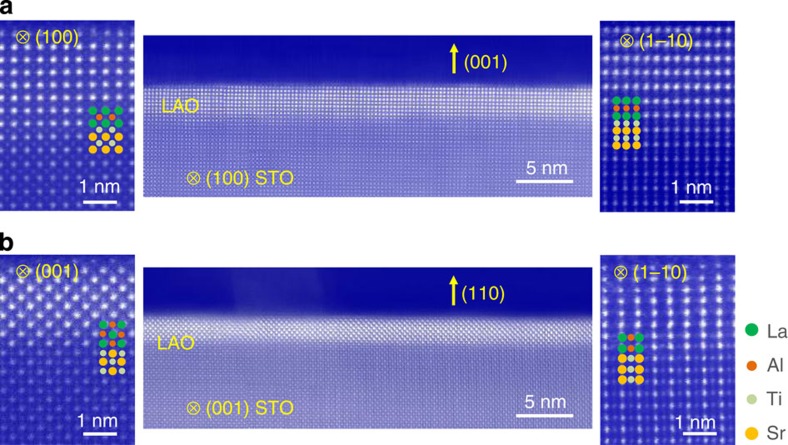
The samples analysed here were obtained by pulsed laser deposition of LaAlO3 thin films on TiO2-terminated (001)-SrTiO3 substrates (LaAlO3 thickness t=10 monolayers (MLs), corresponding to t~3.8 nm) as well as on thermally treated (110)-oriented SrTiO3 substrates (t=7–14 MLs, t~1.9–3.8 nm), see details in Methods and (refs 22, 26, 27). We carried out cross-sectional scanning transmission electron microscopy (STEM) in the high-angle annular dark field (HAADF) imaging mode, in which, to a good approximation, the intensity of an atomic column is proportional to the square of the atomic number (Z), so elements can be deduced by tracking column intensities28. Brighter atomic columns correspond to the heavier elements, La and Sr, whereas fainter columns correspond to Ti and Al. Atomic-scale structural characterization shows a coherent and epitaxial growth of both heterostructures and atomically flat interfaces—Fig. 1a,b for (001) and (110), respectively— Besides, regarding the (110)-oriented sample, along the [001] zone axis the (110) ionic stacking across the interface can be readily appreciated, see Fig. 1b. Therefore, in spite of the higher surface energies of (110)-planes with respect to (001), the STEM-HAADF study rules out altogether the formation of (100) microfacets at the (110)-interface23,29,30.

Figure 1. Atomic-resolution STEM characterization.

Figure 1

Open in a new tab

(a) HAADF-STEM images of the LaAlO3/SrTiO3 (001) interface. The left and right panels are magnified views of the interface observed from [100] and [1–10] zone axes, respectively. (b) HAADF-STEM images of the LaAlO3/SrTiO3 (110) interface. Left and right panels are magnified views of the interface observed from [001] and [1–10] directions, respectively. Both LaAlO3 layers are continuous within the analysed region (of the order of 1 μm). The images in the central panels a and b have been Fourier filtered to reduce background noise. The positions of La and Sr are indicated by green and orange circles, whereas Al and Ti are shown in red and light green. Note that for both orientations the interfaces are atomically flat and that the (110) interface does not show any local (100) microfacet.

Spatial extension and anisotropy of 2D superconductivity

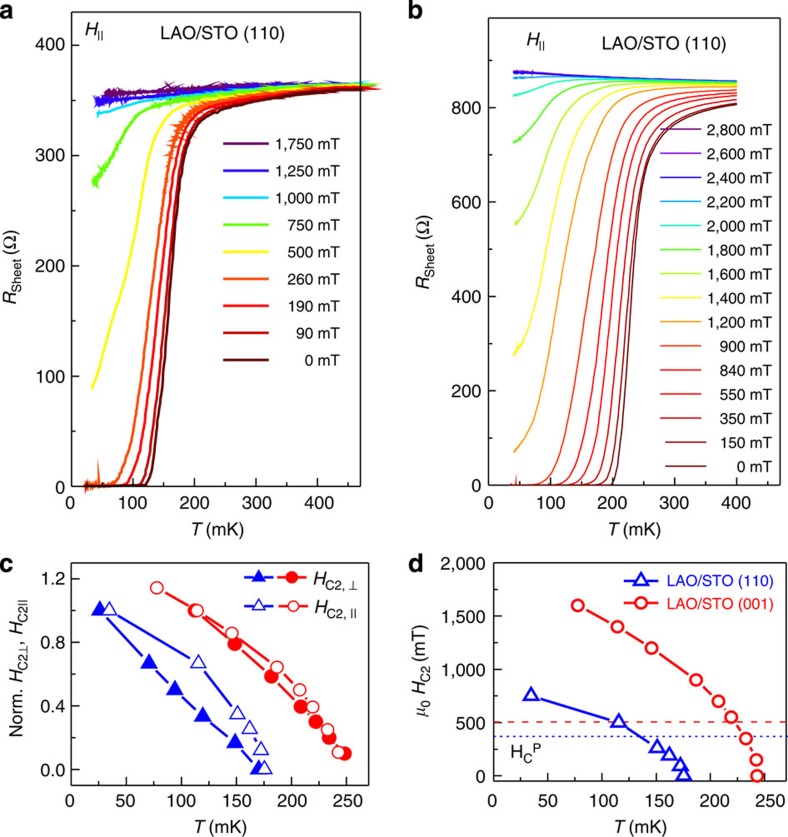
We discuss first the implications of band reconstruction on the 2DEG superconductivity. In line with previous reports on (001) (refs 14, 15, 19), we show that the (110)-interface is also superconductive and has a 2D character. Yet, we uncover that the anisotropy of the 2D superconductive state is considerably larger for (001) than for (110). Such a conclusion is readily apparent from the sheet resistance curves measured under the magnetic fields applied in-plane (Fig. 2a,b). It is known that as the 2D limit is approached, increasingly higher in-plane fields are required to suppress the superconductivity, since vortex entry is impeded by the low dimensionality13. Therefore, higher in-plane critical fields imply stronger anisotropy. Inspection of Fig. 2a,b shows that the (001) interface requires much higher in-plane fields (μ0Hc2,‖≈2,200 mT) than the (110) interface (μ0Hc2,‖≈1,000 mT) to induce the transition to the normal state. We conclude, thus, that the 2D anisotropy is larger for (001) than for (110), anticipating a smaller spatial extension of the quantum well along (001).

Figure 2. Anisotropy of the 2D superconductivity at the (001) and (110) interfaces.

Figure 2

Open in a new tab

Sheet resistance of (a) the (110)-interface with t=14 MLs and (b) the (001)-interface with t=10 MLs, under magnetic fields applied parallel to the interface. The field values are indicated in the panels. Panel (c) shows the temperature dependence of out-of-plane μ0Hc2,⊥ and in-plane μ0Hc2,‖ critical fields of (001)—red circles—and (110)—blue triangles—interfaces, corroborating the 2D character of the superconductivity for both orientations. (d) The upper critical fields are displayed as a function of the temperature for both the orientations (field in-plane). The dotted and dashed straight lines indicate the Pauli-limited critical fields 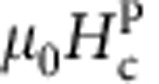Inline graphic. The observation of higher critical fields for the (001) interface is consistent with the larger anisotropy of the 2DEG superconductivity and stronger spatial confinement for (001).

For a quantitative estimation of both the superconductive layer thickness d and the in-plane superconductive coherence length ξ, we carried out an analysis based on the Landau–Ginzburg formalism31. For that purpose, the out-of-plane μ0Hc2,⊥ and in-plane μ0Hc2,‖ critical fields were determined by defining quantitative criteria for the field-induced transitions. Thus, a drop resistance of 90% from the normal resistance state at T=400 mK was established to ascertain the evolution of the transition temperature TC. We consider first the (110) sample with LaAlO3 thickness t=14 MLs. The out-of-plane critical field, extrapolated to T=0 K, was μ0Hc2,⊥≈160 mT (Supplementary Fig. 1), leading to an in-plane coherence length 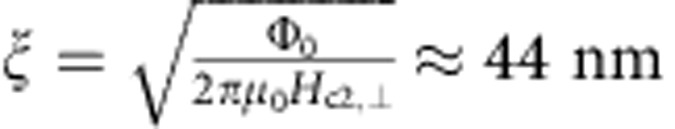Inline graphic (Φ0 is the flux quantum)31. In addition, from the in-plane critical field μ0Hc2,‖≈1,000 mT we could estimate the superconductive thickness 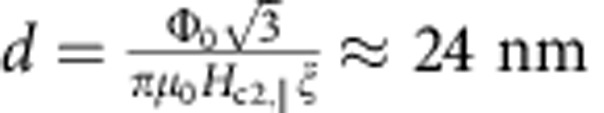Inline graphic. Since the coherence length is well above the superconductive thickness (d<ξ), the superconductivity is shown to be 2D. Applying the same protocol analysis to the other (110)-interfaces in this study, with thickness in the range t=7–10 MLs (Supplementary Fig. 2), we find that always the in-plane coherence length (ξ≈40–75 nm) is significantly larger than the superconductive thickness (d≈24–30 nm), thus confirming the 2D character of the superconductivity at the (110) interface. This is also corroborated by the analysis of the temperature dependence of the resistance, showing that the transition to the superconductivity at (110) interfaces belongs to the Berezinskii–Kosterlitz–Thouless (BKT) universality class32,33,34 (Supplementary Fig. 3). In addition, the out-of-plane and in-plane critical fields follow the temperature dependence expected for 2D superconductors (Fig. 2c), that is, 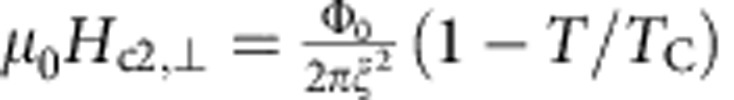Inline graphic and Inline graphic, respectively13.

We applied also the Landau–Ginzburg analysis to a (001) LaAlO3/SrTiO3 sample using the same growth conditions as those used for the (110)-oriented samples. The analysis of the experimental data concludes that the coherence length is ξ≈40 nm and the superconducting thickness is d≈13 nm, in close agreement with the values previously reported14,35. We, thus, demonstrate in a quantitative manner that the spatial extension of superconductive (110) interfaces (d≈24–30 nm) is considerably larger than the one usually reported for (001) interfaces (d≈10–13 nm) (refs 14, 35).

The wider spatial extent of the (110)-2D state is also inferred from the analysis of the Pauli paramagnetic limit of the upper critical fields. For high-enough magnetic fields, the paramagnetic susceptibility induces a parallel alignment of the Cooper pair spins that eventually breaks them apart, giving a higher bound for the upper critical fields18,36. This value can be assessed as 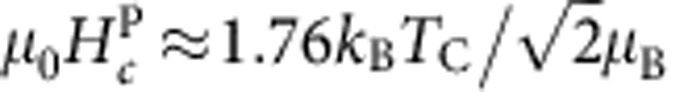Inline graphic, where kB is the Boltzmann’s constant and μB is the Bohr magneton (assuming a g factor of 2)18,36. Although this upper bound is generally fulfilled, it is violated in some cases. One example is the case of ultrathin SrTiO3 2D superconducting layers for which the values of μ0Hc2,‖ were found to exceed largely the Pauli limit. This was explained by the large intrinsic spin–orbit coupling at interfaces, which becomes a prominent energy scale as the thickness is reduced18. The correlation between the spatial confinement and the anisotropy of the 2D superconductivity is also borne out in the (001) and (110) LaAlO3/SrTiO3 interfaces. Figure 2d summarizes this observation: the upper critical fields μ0Hc2,‖ measured in (001) interfaces are significantly higher than those measured in (110) samples at any temperature. As a matter of fact, for the (001) interface the Pauli limit is already violated at temperatures below T≤220 mK, close to TC. Instead, the Pauli limit is only surpassed at temperatures T≤110 mK for the (110) interface, further away from the transition (Fig. 2d). Again, this is an indication of stronger 2DEG confinement at the (001) interface.

Electrostatic modulation of 2D superconductivity

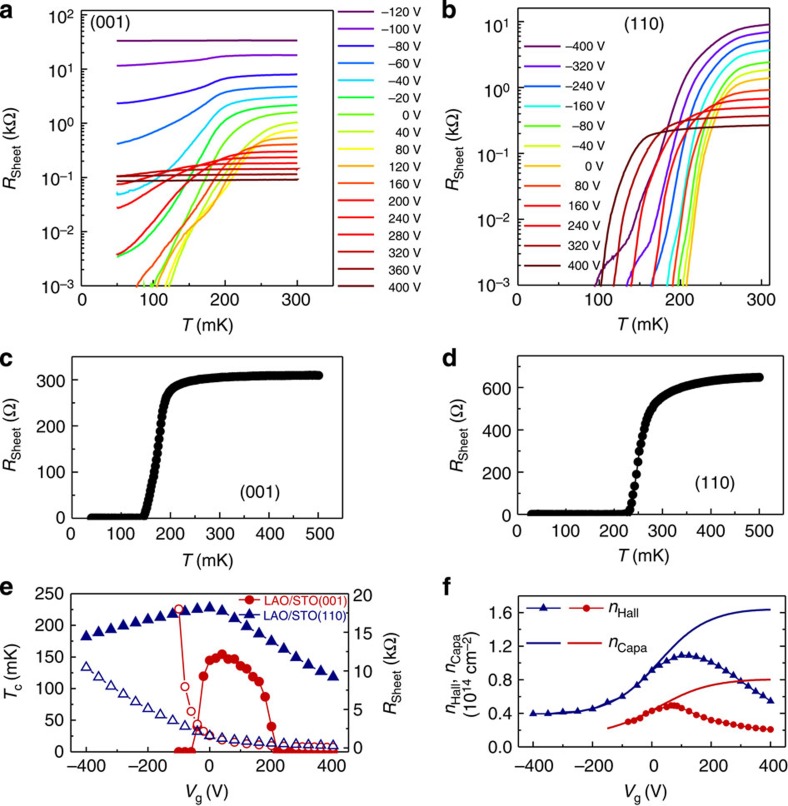
The different 2DEG spatial extent has also consequences on the electrostatic modulation of the superconductivity. We performed electrostatic gating experiments in (001)- and (110)-oriented samples that were contacted by top and backgate electrodes and electric fields were applied in the range of Vg=±400 V (Fig. 3). Positive/negative voltages correspond to the accumulation/depletion of electrons at the interface, respectively. Hall and capacitance experiments allowed us to obtain the sheet carrier density modulation as a function of the voltage Vg for both the film orientations. The curves of carrier density that we extract from Hall measurements exhibit a reduction of nHall for positive Vg (Fig. 3f). Such a feature is the hallmark of multiband conduction, in which high- and low- mobility carriers participate in the transport in the regime of accumulation, whereas only one type of carrier is relevant in the regime of depletion (Vg≪0) (ref. 16). The total carrier density nS, comprising both heavy and light electron bands, can be obtained by experiments that measure the capacitance between the backgate and the 2DEG. In this case, the value of ns is extracted by integration over the voltage range 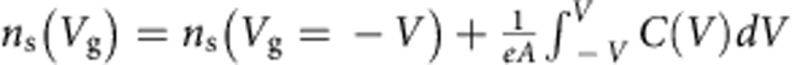Inline graphic, where A is the area of the capacitor. Note that, in agreement with the two-carrier scenario, only one band is involved in transport at negative Vg and nS is superimposed to nHall within this range of applied voltages (Fig. 3f). Instead, in the regime of accumulation, Vg>0, two bands are involved and nS and nHall differ significantly16,37.

Figure 3. Electrostatic modulation of the 2D-superconductvity at the (001) and (110) interfaces.

Figure 3

Open in a new tab

Temperature dependence of the resistance as a function of gate voltage for (a) (001)—and (b) (110)-oriented LaAlO3/SrTiO3 interfaces, respectively. In panels (c) and (d) we show the superconducting transitions in linear scale, for zero applied voltage, for (001) and (110) interfaces, respectively. (e) Superconducting TC (filled symbols) and sheet resistance (open symbols) of (001)—and (110)-interfaces plotted against the gate voltage. (f) Hall carrier density of (001)- (circles) and (110)- (triangles) interfaces. Solid lines correspond to the carrier density obtained from the analysis of the capacitance.

Figure 3e summarizes the results of the electrostatic gating experiments, where the superconducting transition temperature TC and the resistance Rsheet at the normal state are plotted as a function of the gate voltage Vg. We see that the carrier density is largely modulated for both orientations, with variations Δns=0.2−0.8 × 1014 cm−2 and Δns=0.4−1.6 × 1014 cm−2 for (001) and (110) interfaces, respectively. However, despite similar modulations of the carrier density for both orientations, their effects on the superconductivity are dramatically different depending on the crystal orientation. More specifically, the superconductivity of the (001)-interface could be suppressed for a range of applied fields (Fig. 3a), in agreement with previous reports15. At the (001) interface the TC(Vg) curve exhibits a dome-like shape (Fig. 3e), indicating that superconductivity is suppressed at fields above Vg≈+200 V and below Vg≈−50 V. Instead, for (110) interfaces the superconducting state is never switched off by electric fields (Fig. 3b) and the transition temperature is modulated by at most about 50% (Fig. 3e). The much larger tunability of (001) interfaces with respect to (110) is again consistent with the narrower extension of 2DEGs at (001) wells.

Modulation of the Rashba spin–orbit field

Previous works have demonstrated that there is a strong spin–orbit field that stems from a Rashba-type interaction at the LaAlO3/SrTiO3 interface38,39. As a result, an effective magnetic field BSO is felt by electrons moving relativistically under the influence of the interface intensive electric fields E0=−∇V(r). Remarkably, the intensity of BSO is directly related to electron hopping between t2g orbitals that, although forbidden in the unperturbed system away from interfaces, are however allowed in the presence of the field E0 (ref. 40). In particular, E0 induces a polarization of the atomic orbitals, which break their symmetry and, as a consequence, allows a hybridization within the t2g manifold in the metal–oxygen network that contributes to BSO (refs 40, 41). Because of the different 2DEG band structure along (001) or (110), the spin–orbit field BSO is expected to have a strong orientational dependence.

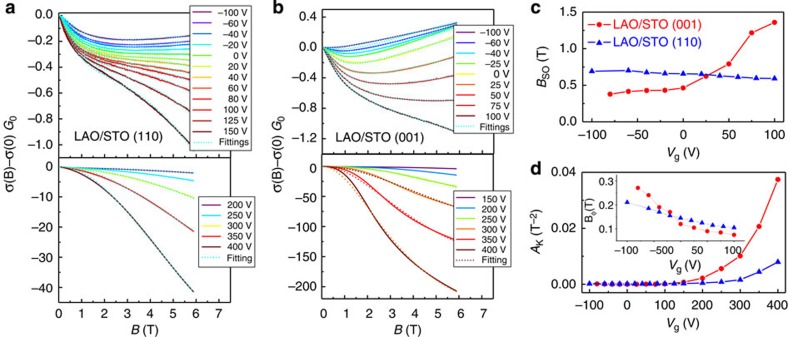
To probe the effects of orientational reconstruction on the spin–orbit term BSO, we analysed the field dependence of the magnetoconductance at the normal state recorded at a temperature T=3.3 K under applied electric fields (Fig. 4a,b). The experimental data were fitted to the expression38,42

Figure 4. Analysis of the spin-orbit coupling at the (001) and (110) interfaces.

Figure 4

Open in a new tab

The field-dependent magnetoconductance Δσ(B) normalized by the quantum of conductance G0 was measured under different gate voltages Vg. Data measured at T=3.3 K are shown for the (a) (110) and (b) (001) interfaces. Dashed lines are the fittings to equation (1) and the values of the applied voltage are indicated in the panels. The dependence of the spin–orbit term BSO on the gate voltage Vg is plotted (c). The Kohler AK and inelastic Bφ terms obtained from fittings to equation (1) are plotted in (d) and inset, respectively.

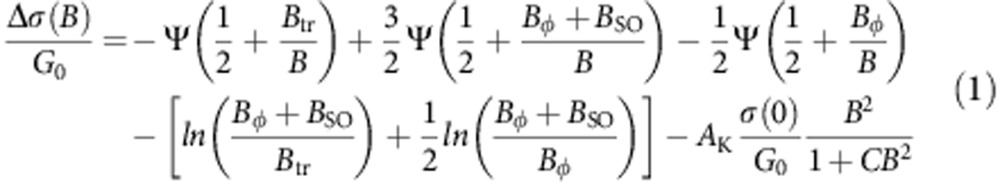graphic file with name ncomms7028-m7.jpg

that describes the change of conductivity with field Δσ(B) normalized by the quantum of conductance G0=e2/πh (refs 38, 42, 43). In equation (1), quantum corrections to the conductance in the 2D limit are described by the four first terms, where Ψ(x) is the digamma function, and Btr, Bφ and BSO are the effective fields related to the elastic, inelastic and spin–orbit scattering terms, respectively43. Finally, the last term in equation 1, involving the parameters AK and C, is the Kohler term that gives an account of orbital magnetoresistance. Fittings of the experimental data to equation (1) were excellent, as shown in Fig. 4a,b for both orientations and for different electric fields. The parameters 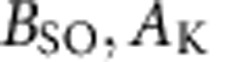Inline graphicand Bφ extracted from these fittings are shown in Fig. 4c,d. It turns out that the Kohler term AK became rather large at positive fields Vg>+100 V (Fig. 4d), making difficult a precise evaluation of the spin–orbit term in the regime of strong electron accumulation. For that reason in Fig. 4c we plotted the evolution of the term BSO restricted to the range Vg=±100 V, where accurate values of the spin–orbit contribution can be obtained.

For the (001) sample, the values of BSO that we obtained from fittings to equation (1) are in the same range as reported previously for the same orientation, with a similar asymmetric dependence of the spin–orbit field with Vg (refs 38, 43). In the regime of depletion (Vg<0 V), the values of the spin–orbit field are BSO<0.5 T, whereas for electron accumulation (Vg>0 V) the spin–orbit term rises up to BSO≈1.5 T. We thus observe a strong asymmetric field dependence of BSO for the interfaces along (001). In contrast, the electrostatic modulation of BSO is very weak along (110), and the spin–orbit field is largely unaffected by the electrostatic gating, with values restricted within a much narrower range BSO≈0.6–0.7 T (Fig. 4c). In brief, our analysis demonstrates that the Rashba spin–orbit fields at the (110) interfaces are substantially different from those along (001). This observation illustrates how band engineering based on crystal symmetry can be exploited to tailor the spin–dependent transport along SrTiO3-based quantum wells44,45.

Discussion

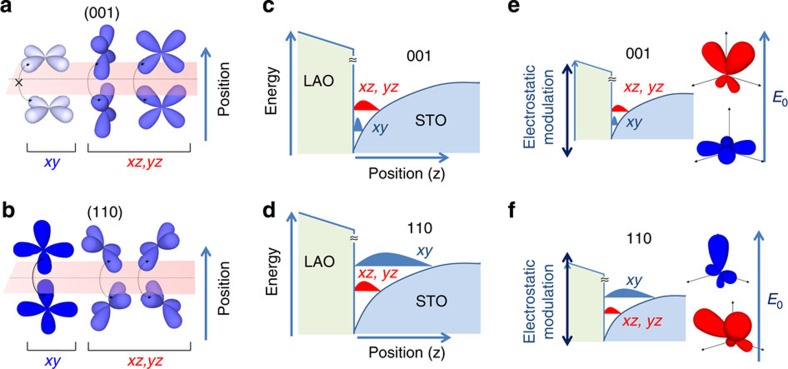
The different spatial extension of the quantum wells along (001) and (110) and the different behaviour of the Rashba spin–orbit fields can be elucidated on the grounds of the modulation of the 2DEG sub-band structure observed in the experiments24 that, in turn, can be understood using the fundamental concepts of quantum physics of solids. When we consider the orbitals of t2g electrons that are confined along (001) or (110), the quantum well entrapment of dxy, dxz and dyz wavefunctions produces an energy splitting between the different eigenstates that is inversely proportional to their effective masses along the confinement direction46. Figure 5 illustrates schematically the arguments that we expose in the following. Note that although the full complexity of the quantum sub-band structure47,48 is ignored in this Figure—as we depict only one sub-band for each type of orbital— the essential physics is captured. More specifically, for confinement along (001), π-type bonding between dxy states leads to small wavefunction overlapping and large effective mass, while along (110) σ-like bonds between dxy orbitals lead to much smaller effective mass (Fig. 5a,b). Instead, the overlapping of dxz/dyz states has intermediate values for both the orientations. This results in a hierarchy of out-of-plane effective masses given by 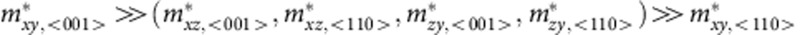Inline graphic that, in turn, yields the energy orbital landscape outlined in Fig. 5c,d, which is in agreement with the 2DEG sub-band hierarchy observed in X-ray linear dichroism experiments46,49.

Figure 5. Energy landscape and orbital symmetries of 2DEGs at (001) and (110) LaAlO3/SrTiO3 interfaces.

Figure 5

Open in a new tab

(a) Along (001), the overlapping of dxy orbitals is very small, while it is moderately large for dxz/dyz states. (b) Along (110), the overlapping of dxy orbitals is the largest. Since the effective mass along the confinement direction is inversely proportional to the orbital overlapping, the hierarchy of masses is given by 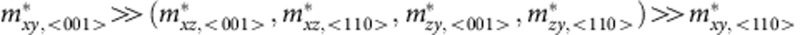Inline graphic. The rules of quantum physics in solids dictate the energy landscape in the quantum wells: (c) Orbitals with symmetry dxy lie at the bottom of (001) quantum wells, while dxz/dyz are higher in energy; (d) Along (110), the dxz/dyz states have lower energy and the dxy levels are at the top. The different energy hierarchy of orbitals determines a larger spatial extension for 2DEGs along (110) as compared with (001). (e) Sketches how the dxy and dxz/dyz orbitals are distorted by the inversion symmetry breaking field E0 at the interface. Along (001), dxy states have a small projection along the out-of-plane direction and they are weakly polarized by E0, whereas dxz/dyz states project along the direction of confinement and are largely polarized. (f) Instead, along (110), both kinds of orbitals are affected similarly by the interface fields. Within the range of applied fields, the electrostatic modulation has dissimilar effects on the spin–orbit fields BSO. Along (001), only dxy orbitals are filled at Vg<0, whereas at Vg>0 the dxz/dyz states are progressively occupied. Thus, at low electrostatic doping, BSO is smaller because of the relatively small atomic orbital polarization of dxy states, whereas the population of dxz/dyz orbitals at positive Vg increases significantly BSO. Instead, along (110) the dependence of BSO on the applied fields is weak, because all t2g orbitals are expected to undergo polarizations of similar strength.

As a consequence of the observed rearrangement of orbital symmetries, the spatial extension of the 2DEG must change significantly with the crystal orientation. In this respect, Fig. 5c,d plot schematically the carrier spatial distributions of dxy, dxz and dyz states: along (001) the first dxy sub-band is expected to be at the bottom of the well, with little spatial spread; on the contrary, along (110) the dxy level raises its energy above the dxz/dyz states, and its spatial extent is considerably larger. In addition to orbital occupancy, contributions from the anisotropic character of the dielectric constant tensor may also influence the 2DEG spatial extent. Therefore, the modulation of the orbital hierarchy described here provides a natural explanation for the distinct anisotropy of the 2D superconductivity and spatial extension for quantum wells oriented along (001) and (110).

By the same token, the redistribution of the orbital sub-band hierarchy also explains the distinctive dependence of the Rashba spin–orbit fields on the orientation. As mentioned above, the interface electric field E0 induces a polarization of the atomic orbitals that breaks their symmetry along the direction of the quantum well. This enables new covalent channels within the t2g manifold and the oxygen network that contributes to BSO (refs 40, 41). The key point is to recognize that orbitals with large projections over the normal to the interface are those more sensitive to the inversion symmetry breaking fields E0, giving larger Rashba effects40,41. Such atomic orbital polarization is graphically depicted in Fig. 5e in the form of spatially distorted orbitals.

In the light of these observations, the asymmetric modulation of BSO with field along (001), Fig. 4c, can be explained because dxy orbitals are weakly polarized due to their minimal projection along the confinement direction, while dxz/dyz states have much stronger spatial asymmetry (Fig. 5e). As a result of the 2DEG sub-band hierarchy along (001), the electrostatic modulation of orbital occupancy is anticipated to give a significant variation of BSO as a function of the orbital occupancy: at Vg<0 V only dxy orbitals are populated; the orbital polarization is weak (Fig. 5e) and BSO is relatively small. In contrast, as we enter the regime of accumulation and dxz/dyz bands start to be filled, the spin–orbit term begins to increase significantly, in agreement with the significantly larger orbital polarization of these orbitals.

The situation is radically different for the (110) interface. Now, the electrostatic modulation of BSO is very weak and the spin–orbit field is largely unaffected by the electrostatic gating. This behaviour, which may seem surprising in the light of the modulation of carrier density with electrostatic gating (Fig. 4d), can be well understood on the grounds of the similar atomic orbital polarizations of dxy and dxz/dyz orbitals along (110), Fig. 5e. Indeed, along (110), all t2g orbitals are expected to undergo similarly strong polarizations (Fig. 5e) and, therefore, the spin–orbit field BSO is expected to have a rather weak dependence on the applied field, as confirmed by the experiments.

In summary, we have shown that the orbital reconstruction that occurs for LaAlO3/SrTiO3 quantum wells confined along two different directions, (001) and (110) has a deep impact on the physical properties of these 2DEGs. We claim that the different energy landscapes and hierarchy of orbital symmetries are behind the observed differences in the 2DEG spatial extensions and spin–orbit fields. The analysis of the 2D superconductivity is consistent with 2DEGs extending spatially over (110) at larger distances than at (001) interfaces. At the same time, electrostatic gating experiments have provided relevant clues to understand the distinctive spatial distribution of t2g states with respect to the interface that results from the modified energy sub-band hierarchy and the renormalization of the associated effective band masses. Our work shows that crystal symmetry is an extra degree of freedom to realize different 2DEG band reconstructions at the LaAlO3/SrTiO3 interface, thus allowing a selective occupancy of states of different symmetry. Such new perspective for 2DEG band engineering is very alluring, as it opens new research fields to extend our current understanding of the link between orbital symmetry and magnetism and superconductivity at LaAlO3/SrTiO3 quantum wells.

Methods

Sample preparation

For the growth of (110)-oriented samples, the SrTiO3 substrates were treated in a dedicated furnace at 1,100 °C for 2 h under ambient conditions26,27. Samples with (001) orientation were grown on TiO2-terminated SrTiO3 substrates. The TiO2 termination of the SrTiO3(001) single crystals was obtained by chemical treatment followed by thermal annealing50,51. LaAlO3 thin films were grown by pulsed laser deposition (λ=248 nm) monitored by high pressure reflection high-energy electron diffraction. The substrates were heated from room temperature to deposition temperature (850 °C) in an oxygen partial pressure PO2=0.1 mbar. During deposition, the LaAlO3 was grown under a pressure PO2=10−4 mbar and 1-Hz repetition rate, with laser pulse energy of around 26 mJ. Films with thickness 7, 8, 10 and 14 MLs were prepared on (110) substrates, whereas the (001)-oriented sample had a LaAlO3 thickness of 10 MLs. At the end of the deposition, samples were cooled down in an oxygen rich atmosphere to minimize the formation of oxygen vacancies that could lead to extrinsic mechanisms of conduction. More specifically, the samples were cooled from T=850 to 750 °C under a pressure PO2=0.3 mbar and under PO2=200 mbar from T=750 °C down to room temperature, including a dwell time of 1 h at 600 °C.

Magnetotransport

The electrical characterization was performed by using six-contact arrangement in Hall geometry, from which the sheet resistance, sheet carrier density and electron mobility were extracted as a function of temperature and gate voltage. The current was injected along the in-plane (001) direction in (110)-interfaces. The LaAlO3/SrTiO3 interface was contacted via ultrasonic wire bonder with Al wires. Measurements at temperatures below 1.8 K were measured in a dilution cryostat by applying 50 nA AC current of frequency 13.67 Hz. For the estimation of critical field, the magnetic field was applied parallel and perpendicular to the sample plane with sweep rates of 1.6 mT s−1. For the measurement of the parallel critical magnetic field of the 110 samples, the field was applied in the same direction than the current, that is, along the in-plane (001). Electric fields were applied using voltage source. No leakage current (<5 nA) was detected up to largest applied voltages ±400 V.

Transmission electron microscopy

STEM-HAADF images were acquired with a NION UltraSTEM, equipped with a 5th order NION aberration corrector and operated at 200 kV, and in a FEI Titan (60–300 kV) STEM operated at 300 kV, equipped with a probe Cs corrector from CEOS, a monochromator and a high-brightness field-emission gun (X-FEG). HAADF signals for the samples were collected from the detector inner-angles of ~86 and ~60 mrad for the NION and FEI Titan microscopes, respectively. Specimens for STEM were prepared by conventional methods, by grinding, dimpling and argon ion milling.

Author contributions

G.H. designed and conceived the experiments with help from J.F., N.B. and J.L. Low-temperature magnetotransport experiments were done by G.S., A.J. and N.B. The analysis of low-temperature magnetotransport data was done by G.S., N.B. and G.H. The thin film preparation, in situ reflection high-energy electron diffraction characterization and structural and surface morphology analysis were done by M.S., N.D. and F.S. J.G., and M.V. made all the characterization and analysis of structural characterization by STEM-HAADF. G.H. wrote the paper. All authors discussed the data and commented on the paper.

Additional information

How to cite this article: Herranz, G. et al. Engineering two-dimensional superconductivity and Rashba spin–orbit coupling in LaAlO3/SrTiO3 quantum wells by selective orbital occupancy. Nat. Commun. 6:6028 doi: 10.1038/ncomms7028 (2015).

Supplementary Material

Supplementary Information

Supplementary Figures 1-3 and Supplementary References

ncomms7028-s1.pdf (644.3KB, pdf)

Acknowledgments

This work was supported by the Spanish MAT2011-29269-C03 project, the RyC-2012–11709 contract of J.G. and the Generalitat de Catalunya (2014 SGR 734 project) and by the Region Île-de-France in the frame-work of CNano IdF programme. The HRTEM microscopy work was in part conducted in the ‘Laboratorio de Microscopías Avanzadas’ at the Instituto de Nanociencia de Aragón-Universidad de Zaragoza. We acknowledge the LMA-INA for offering access to their instruments. J.G. is grateful to Dr A. Ibarra for the help at LMA-INA. Electron microscopy observations at ORNL were supported by the U.S. Department of Energy (DOE), Basic Energy Sciences (BES), Materials Sciences and Engineering Division.

References

  1. Park S.-H. Crystal orientation effects on electronic properties of wurtzite InGaN/GaN quantum wells. J. Appl. Phys. 91, 9904–9908 (2002). [Google Scholar]
  2. Feneberg M. & Thonke K. Polarization fields of III-nitrides grown in different crystal orientations. J. Phys. Condens. Matter 19, 403201 (2007). [DOI] [PubMed] [Google Scholar]
  3. Park S.-H. & Ahn D. Crystal orientation effects on electronic and optical properties of wurtzite ZnO/MgZnO quantum well lasers. Opt. Quant. Electron. 38, 935–952 (2006). [Google Scholar]
  4. Ohtomo A. & Hwang H. Y. A high-mobility electron gas at the LaAlO3/SrTiO3 heterointerface. Nature 427, 423–426 (2004). [DOI] [PubMed] [Google Scholar]
  5. Ohtomo A., Muller D. A., Grazul J. L. & Hwang H. Y. Artificial charge-modulation in atomic-scale perovskite titanate superlattices. Nature 419, 378–380 (2002). [DOI] [PubMed] [Google Scholar]
  6. Jang H. W. et al. Metallic and insulating oxide interfaces controlled by electronic correlations. Science 331, 886–889 (2011). [DOI] [PubMed] [Google Scholar]
  7. Biscaras J. et al. Two-dimensional superconductivity at a Mott insulator/band insulator interface LaTiO3/SrTiO3. Nat. Commun. 1, 89 (2010). [DOI] [PubMed] [Google Scholar]
  8. Hotta Y., Susaki T. & Hwang H. Y. Polar discontinuity doping of the LaVO3/SrTiO3 interface. Phys. Rev. Lett. 99, 236805 (2007). [DOI] [PubMed] [Google Scholar]
  9. Perna P. et al. Conducting interfaces between band insulating oxides: The LaGaO3/SrTiO3 heterostructure. Appl. Phys. Lett. 97, 152111 (2010). [Google Scholar]
  10. Moetakef P. et al. Transport in ferromagnetic GdTiO3/SrTiO3 heterostructures. Appl. Phys. Lett. 98, 112110 (2011). [Google Scholar]
  11. Chen Y. Z. et al. A high-mobility two-dimensional electron gas at the spinel/perovskite interface of γ-Al2O3/SrTiO3. Nat. Commun. 4, 1371 (2013). [DOI] [PubMed] [Google Scholar]
  12. Ueno K. et al. Discovery of superconductivity in KTaO3 by electrostatic carrier doping. Nat. Nanotechnol. 6, 408–412 (2011). [DOI] [PubMed] [Google Scholar]
  13. Kozuka Y. et al. Two-dimensional normal-state quantum oscillations in a superconducting heterostructures. Nature 462, 487–490 (2009). [DOI] [PubMed] [Google Scholar]
  14. Reyren N. et al. Superconducting interfaces between insulating oxides. Science 317, 1196–1199 (2007). [DOI] [PubMed] [Google Scholar]
  15. Caviglia A. D. et al. Electric field control of the LaAlO3/SrTiO3 interface ground state. Nature 456, 624–637 (2008). [DOI] [PubMed] [Google Scholar]
  16. Biscaras J. et al. Two-dimensional superconducting phase in LaTiO3/SrTiO3 heterostructures induced by high-mobility carrier doping. Phys. Rev. Lett. 108, 247004 (2012). [DOI] [PubMed] [Google Scholar]
  17. Gardner H. J. et al. Enhancement of superconductivity by a parallel magnetic field in two-dimensional superconductors. Nat. Phys. 7, 895–900 (2011). [Google Scholar]
  18. Kim M., Kozuka Y., Bell C., Hikita Y. & Hwang H. Y. Intrinsic spin-orbit coupling in superconducting δ-doped SrTiO3 heterostructures. Phys. Rev. B 86, 085121 (2012). [Google Scholar]
  19. Schneider T., Caviglia A. D., Gariglio S., Reyren N. & Triscone J.-M. Electrostatically-tuned superconductor-metal-insulator quantum transition at the LaAlO3/SrTiO3 interface. Phys. Rev. B 79, 184502 (2009). [Google Scholar]
  20. Biscaras J. et al. Multiple quantum criticality in a two-dimensional superconductor. Nat. Mater. 12, 542–548 (2013). [DOI] [PubMed] [Google Scholar]
  21. Warusawithana M. P. et al. LaAlO3 stoichiometry is key to electron liquid formation at LaAlO3/SrTiO3 interfaces. Nat. Commun. 4, 2351 (2013). [DOI] [PubMed] [Google Scholar]
  22. Herranz G., Sánchez F., Dix N., Scigaj M. & Fontcuberta J. High mobility conduction at (110) and (111) LaAlO3/SrTiO3 interfaces. Sci. Rep. 2, 758 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. Annadi A. et al. Anisotropic two-dimensional electron gas at the LaAlO3/SrTiO3 (110) interface. Nat. Commun. 4, 1838 (2013). [DOI] [PMC free article] [PubMed] [Google Scholar]
  24. Pesquera D. et al. Two-Dimensional electron gases at LaAlO3/SrTiO3 interfaces: orbital symmetry and hierarchy engineered by crystal orientation. Phys. Rev. Lett. 113, 156802 (2014). [DOI] [PubMed] [Google Scholar]
  25. Wang Z. et al. Anisotropic two-dimensional electron gas at SrTiO3(110). Proc. Natl Acad. Sci. 111, 3933 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Bachelet R., Valle F., Infante I., Sánchez F. & Fontcuberta J. Step formation, faceting, and bunching in atomically flat (110) surfaces. Appl. Phys. Lett. 91, 251904 (2007). [Google Scholar]
  27. Sánchez F., Ocal C. & Fontcuberta J. Tailored surfaces of perovskite oxide substrates for conducted growth of thin films. Chem. Soc. Rev. 43, 2272–2285 (2014). [DOI] [PubMed] [Google Scholar]
  28. Pennycook S. J. & Nellist P. D. Scanning Transmission Electron Microscopy Springer (2011). [Google Scholar]
  29. Bando H., Aiura Y., Haruyama Y., Shimizu T. & Nishihara Y. Structure and electronic states on reduced SrTiO3(110) surface observed by scanning tunneling microscopy and spectroscopy. J. Vac. Sci. Technol. B 13, 1150–1154 (1995). [Google Scholar]
  30. Bottin F., Finocchi F. & Noguera C. Facetting and (n × 1) reconstructions of SrTiO3(1 1 0) surfaces. Surf. Sci. 574, 65–76 (2005). [Google Scholar]
  31. Tinkham M. Introduction to Superconductivity 2nd ed. Dover (1996). [Google Scholar]
  32. Kosterlitz J. M. & Thouless D. J. Ordering, metastability and phase transitions in two-dimensional systems. J. Phys. C Solid State Phys. 6, 1181–1203 (1973). [DOI] [PubMed] [Google Scholar]
  33. Beasley M. R., Mooij J. E. & Orlando T. P. Possibility of vortex-antivortex pair dissociation in two-dimensional superconductors. Phys. Rev. Lett. 42, 1165–1168 (1979). [Google Scholar]
  34. She J.-H. & Balatsky A. V. Berezinskii-Kosterlitz-Thouless transition to the superconducting state of heavy-fermion superlattices. Phys. Rev. Lett. 109, 077002 (2012). [DOI] [PubMed] [Google Scholar]
  35. Reyren N. et al. Anisotropy of the superconducting transport properties of the LaAlO3/SrTiO3 interface. Appl. Phys. Lett. 94, 112506 (2009). [Google Scholar]
  36. Chandrasekhar B. S. A note on the maximum critical field of high-field superconductors. Appl. Phys. Lett. 1, 7 (1962). [Google Scholar]
  37. Kim J. S. et al. Nonlinear Hall effect and multichannel conduction in LaTiO3/SrTiO3 superlattices. Phys. Rev. B 82, 201407 (2010). [Google Scholar]
  38. Caviglia A. D. et al. Tunable Rashba spin-orbit interaction at oxide interfaces. Phys. Rev. Lett. 104, 126803 (2010). [DOI] [PubMed] [Google Scholar]
  39. Ben Shalom M., Sachs M., Rakhmilevitch D., Palevski A. & Dagan Y. Tuning spin-orbit coupling and superconductivity at the SrTiO3/LaAlO3 interface: a magnetotransport study. Phys. Rev. Lett. 104, 126802 (2010). [DOI] [PubMed] [Google Scholar]
  40. Khalsa G., Lee B. & MacDonald A. H. Y. Theory of t2g electron-gas Rashba interactions. Phys. Rev. B 88, 041302(R) (2013). [Google Scholar]
  41. Shanavas K. V. & Satpathy S. Electric field tuning of the rashba effect in the polar perovskite structures. Phys. Rev. Lett. 112, 086802 (2014). [Google Scholar]
  42. Maekawa S. & Fukuyama H. Magnetoresistance in two-dimensional disordered systems: effects of Zeeman splitting and spin-orbit scattering. J. Phys. Soc. Jpn 50, 2516–2524 (1981). [Google Scholar]
  43. Fête A., Gariglio S., Caviglia A. D., Triscone J.-M. & Gabay M. Rashba induced magnetoconductance oscillations in the LaAlO3-SrTiO3 heterostructure. Phys. Rev. B 86, 201105(R) (2012). [Google Scholar]
  44. Reyren N. et al. Gate-controlled spin injection at LaAlO3/SrTiO3 interfaces. Phys. Rev. Lett. 108, 186802 (2012). [DOI] [PubMed] [Google Scholar]
  45. Santander-Syro A. F. et al. Giant spin splitting of the two-dimensional electron gas at the surface of SrTiO3. Nat. Mater. 13, 1085–1090 (2014). [DOI] [PubMed] [Google Scholar]
  46. Santander-Syro A. F. et al. Two-dimensional electron gas with universal subbands at the surface of SrTiO3. Nature 469, 189–193 (2011). [DOI] [PubMed] [Google Scholar]
  47. Son W.-J., Cho E., Lee B., Lee J. & Han S. Density and spatial distribution of charge carriers in the intrinsic n-type LaAlO3-SrTiO3 interface. Phys. Rev. B 79, 245411 (2009). [Google Scholar]
  48. Berner G. et al. Direct k-Space mapping of the electronic structure in an oxide-oxide interface. Phys. Rev. Lett. 110, 247601 (2013). [DOI] [PubMed] [Google Scholar]
  49. Salluzzo M. et al. Orbital reconstruction and the two-dimensional electron gas at the LaAlO3/SrTiO3 interface. Phys. Rev. Lett. 102, 166804 (2009). [DOI] [PubMed] [Google Scholar]
  50. Koster G., Boike L. K., Rijnders G. J. H. M., Blank D. H. A. & Rogalla H. Quasi-ideal strontium titanate crystal surfaces through formation of strontium hydroxide. Appl. Phys. Lett. 73, 2920–2922 (1998). [Google Scholar]
  51. Kawasaki M. et al. Atomic control of the SrTiO3 crystal surface. Science 266, 1540–1542 (1994). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-3 and Supplementary References

ncomms7028-s1.pdf (644.3KB, pdf)

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES