Avoided valence transition in a plutonium superconductor

Significance

One way to search for new superconductors is to find a magnetic metal and then suppress the magnetism using chemical doping or pressure. Heavy-fermion superconductors are the archetypal family of magnetic superconductors, but PuCoGa₅—the heavy fermion with the highest $T_c$ (18.5 K)—has no static magnetism. What other mechanism, then, is driving superconductivity in PuCoGa₅? We measured the elastic constants of PuCoGa₅ and found that the bulk modulus softens dramatically before $T_c$—evidence for fluctuations of the plutonium valence as opposed to magnetic fluctuations associated with the suppression of magnetic order. Valence fluctuations resolve the missing magnetism conundrum in PuCoGa₅ by providing an alternative mechanism for the high-temperature superconductivity.

Abstract

The d and f electrons in correlated metals are often neither fully localized around their host nuclei nor fully itinerant. This localized/itinerant duality underlies the correlated electronic states of the high-$T_c$ cuprate superconductors and the heavy-fermion intermetallics and is nowhere more apparent than in the $5f$ valence electrons of plutonium. Here, we report the full set of symmetry-resolved elastic moduli of PuCoGa₅—the highest $T_c$ superconductor of the heavy fermions ($T_c$ = 18.5 K)—and find that the bulk modulus softens anomalously over a wide range in temperature above $T_c$. The elastic symmetry channel in which this softening occurs is characteristic of a valence instability—therefore, we identify the elastic softening with fluctuations of the plutonium 5f mixed-valence state. These valence fluctuations disappear when the superconducting gap opens at $T_c$, suggesting that electrons near the Fermi surface play an essential role in the mixed-valence physics of this system and that PuCoGa₅ avoids a valence transition by entering the superconducting state. The lack of magnetism in PuCoGa₅ has made it difficult to reconcile with most other heavy-fermion superconductors, where superconductivity is generally believed to be mediated by magnetic fluctuations. Our observations suggest that valence fluctuations play a critical role in the unusually high $T_c$ of PuCoGa₅.

PuCoGa₅ enters the superconducting state below $T_c=18.5$ K (1)—an order of magnitude higher than all Ce- or U-based superconductors. This contrast raises the question of whether PuCoGa₅ simply benefits from a higher superconducting-pairing energy scale than its U- and Ce-based relatives or instead, whether PuCoGa₅ is host to a completely different pairing mechanism. In many lanthanide and actinide compounds, the f electrons are nearly degenerate with the conduction band. In addition, the outer f-shell states are close in energy and may support two (or more) nearly degenerate valence configurations (2). In some cases, this valence degeneracy becomes unstable, leading to valence fluctuations and ultimately, a transition to a different valence state as a function of temperature, pressure, and/or doping (3). X-ray and photoemission spectroscopy (4, 5), neutron form factor measurements (6), and theoretical calculations (7) all indicate that PuCoGa₅ is in an intermediate valence state, with the $5f^6$ (Pu²⁺), $5f^5$ (Pu³⁺), and $5f^4$ (Pu⁴⁺) orbitals all residing near the chemical potential and all partially occupied. PuCoGa₅ exhibits no localized magnetic moments (6), and like other plutonium systems, its 5f electrons cannot be treated as fully localized or fully itinerant (4). [Strong Curie–Weiss-like magnetic susceptibility was initially reported for PuCoGa⁵, consistent with a local moment. However, additional susceptibility measurements (including polarized neutron scattering) have not reproduced this result (6).]

In contrast, the analogous CeMIn₅ (M = Co, Rh, and Ir) series of superconductors has localized Ce 4f magnetic moments, with a tendency toward antiferromagnetic order (8). These systems reside close to an antiferromagnetic quantum critical point (9), where antiferromagnetic fluctuations are believed to mediate unconventional superconductivity. Because there is no evidence for PuCoGa₅ being in proximity to a magnetically ordered state (10), it is unlikely that magnetic fluctuations are the primary driver of its anomalously high $T_c$ of 18.5 K (11). Valence fluctuations—where the total number of f electrons per ion fluctuates with the conduction band or the configuration of a fixed number of f electrons fluctuates between two or more nearly degenerate f states (sometimes known as orbital fluctuations)—have been proposed as a possible alternative mechanism for superconductivity in several heavy-fermion systems (12–15). Here, we report that PuCoGa₅’s elastic moduli soften over a large temperature range above $T_c$. Analysis of the observed temperature dependence of the softening, the symmetry channel in which it occurs, and its interplay with the superconducting transition suggests that valence fluctuations are critical for superconductivity in this system.

Results

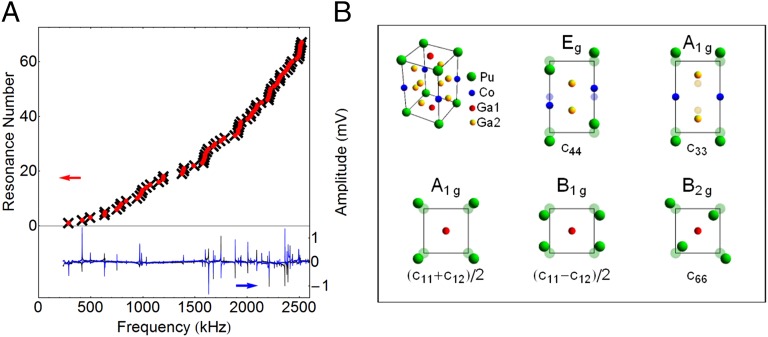
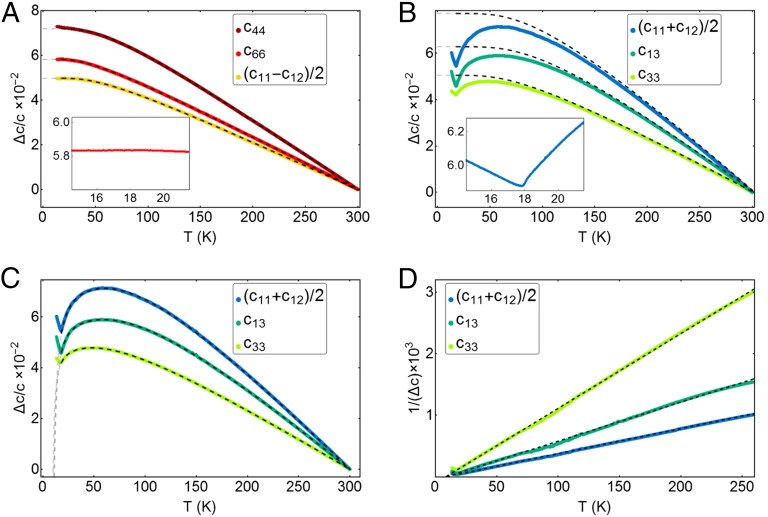
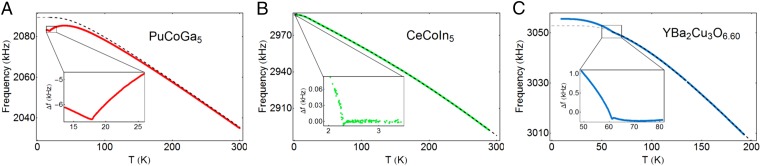
Elastic moduli measurements are a powerful tool for revealing valence instabilities and transitions (2, 16). Recent advances (17) in resonant ultrasound spectroscopy, further extended in this work (SI Text, section II), have allowed us to resolve all of the elastic moduli of PuCoGa₅ to low temperature in a single-temperature sweep. These advances provide a unique opportunity to explore the unusual valence of plutonium with a thermodynamic, symmetry-sensitive probe. Fig. 1A shows the first 65 resonances–the lowest-frequency vibrational modes—of a $2.208\times 2.240\times 0.641$-mm single crystal of PuCoGa₅ (SI Text, section I). [The $T_c$ of freshly grown PuCoGa⁵ is 18.5 K. Because the sample self-irradiates because of the decay of plutonium, this $T_c$ decreases at a rate of 0.2 K per month (1), resulting in our sample having a $T_c$ of 18.1 K. We checked the elastic moduli over the period of 1 mo and observed no qualitative changes in the moduli induced by radiation damage other than the decrease of $T_c$.] Each resonance frequency is uniquely determined by crystal geometry, density, and six elastic moduli—a consequence of the five irreducible strains in this tetragonal system (Fig. 1B); conversely, the redundant set of measured resonance frequencies uniquely determines the six elastic moduli. The temperature dependencies of the elastic moduli from room temperature to below the superconducting transition are shown in Fig. 2 (an example of the fit at $T=295\hspace{0.17em}\text{K}$ is shown in Fig. 1A; details of the data analysis are in SI Text, section II). There are three shear moduli associated with volume-preserving strains (transforming as $B_{1g}$, $B_{2g}$, and $E_g$ irreducible representations), which are shown in Fig. 2A, and three compressional moduli associated with two volume-changing strains (both transforming as the $A_{1g}$ representation, which we will refer to as scalar, because they preserve the lattice symmetry), which are shown in Fig. 2B. The measured scalar moduli behave very differently from the shear moduli: the shear moduli show no anomalies over the entire temperature range (including through $T_c$), and their temperature dependence is well-described by the standard Einstein oscillator model for an anharmonic lattice (18) (linear at high temperature and saturating at low temperature) (Fig. 2A). In contrast, the scalar moduli fall below the anharmonic background well above $T_c$, as shown in Fig. 2D [note that the bulk modulus (see Fig. 4A) is a particular combination of scalar moduli for hydrostatic strain]. All three scalar moduli show $\sim 1/\left(T-T_v\right)$ behavior, where $T_v\approx 9\hspace{0.17em}\text{K}$, as seen in Fig. 2C. This softening is truncated when superconductivity sets in at $T_c=18.1$ K before this nominal valence transition at $T_v\approx 9K$. Softening of the elastic constants before $T_c$ is not observed in either the related compound CeCoIn₅, where the Ce $4f$ electrons are localized, or the high-$T_c$ superconductor YBa₂Cu₃O_6.58 (see Fig. 3). Below $T_c$, however, the elastic moduli of PuCoGa₅ behave similarly to these other unconventional superconductors, suggesting that this anomalous behavior is confined only to temperatures above $T_c$ (Fig. 3).

Fig. 1.

Vibrational spectrum of PuCoGa₅ at room temperature. (A) Transmitted ultrasonic signal as a function of frequency (real and imaginary components in blue and black, respectively, on the right vertical axis) showing the first 65 resonances at room temperature. The position of each resonance is indicated by a red dot (left vertical axis): the elastic moduli are calculated precisely by fitting these resonance positions—a highly overdetermined problem with six moduli and 65 resonances. The calculated resonance positions (black crosses) not only have a small residual error but also, reproduce the correct structure of the data. (B) The PuCoGa₅ unit cell and the five irreducible representations of strain allowed by tetragonal symmetry and their associated elastic moduli. The sixth modulus ($c_{13}$) is the coupling coefficient between the two $A_{1g}$ strains. These six moduli at $T=295\hspace{0.17em}\text{K}$ are measured to be $\left(c_{11}+c_{12}\right)/2=119.8$, $c_{13}=64.5$, $c_{33}=176.1$, $\left(c_{11}-c_{12}\right)/2=73.1$, $c_{44}=60.8$, and $c_{66}=54.2$ (all values in gigapascals).

Fig. 2.

Temperature dependence of the elastic moduli of PuCoGa₅. (A) Shear moduli normalized to the room temperature values. The dashed black line represents a three-parameter fit to the standard temperature dependence from an anharmonic lattice: $c\left(T\right)=a-s/\left(e^{T_D/T}-1\right)$ (18). (B) Scalar moduli show softening across a broad temperature range and truncating at $T_c$. The dashed black line represents the anharmonic background, from which the data deviate strongly at low temperature. (C) The scalar moduli from B with a fit to the anharmonic background plus a $-a/\left(T_v-T\right)$ contribution, where $T_v=9\pm 1.0$ K. (D) The inverse of the difference between the scalar moduli and the anharmonic background; this residual is linear above $T_c$, and the intercept is $9\pm 1.5$ K.

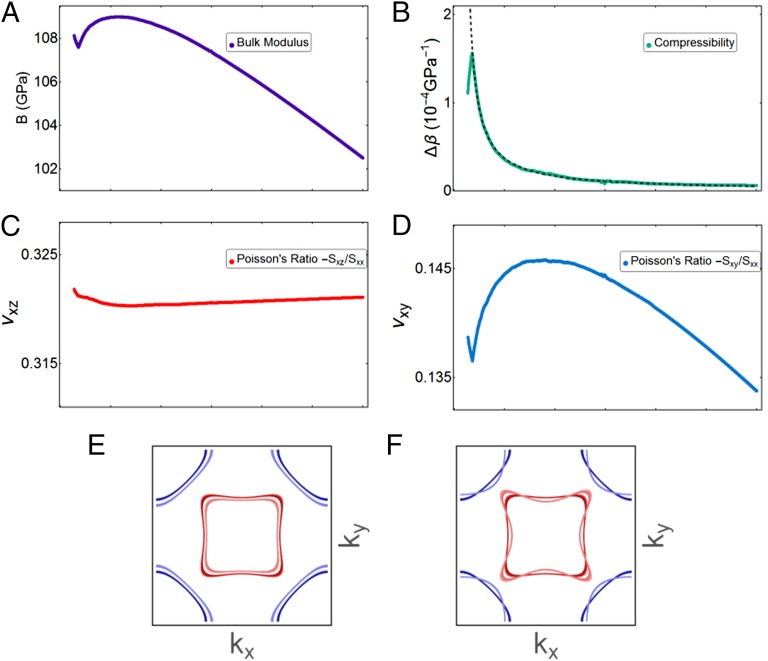
Fig. 4.

Valence fluctuations in PuCoGa₅. (A) The bulk modulus, (B) anomalous compressibility (inverse of the bulk modulus), (C) out-of-plane Poisson’s ratio (${\nu }_{xz}\equiv -S_{xz}/S_{xx}$, where $S_{ij}$ values are the elastic compliances from the inverted modulus tensor), and (D) in-plane Poisson’s ratio (${\nu }_{xy}\equiv -S_{xy}/S_{xx}$). The Poisson’s ratio ${\nu }_{xz}$ has a magnitude typical of most metals (29) and is only weakly temperature-dependent. The ratio ${\nu }_{xy}$, however, is strongly temperature-dependent and anomalously small, reminiscent of other mixed-valence systems (24). The anomalous contribution to the compressibility is shown in B along with a fit to $-a/\left(T_v-T\right)$, where $T_v=9\pm 1$ K. (E and F) Schematic Fermi surface of PuCoGa₅ (based on refs. 37 and 31) with hole pockets in red and electron pockets in blue. $A_{1g}$ symmetry Fermi surface distortions are shown in lighter shades, with fluctuations of the total carrier density in E and a distortion caused by hybridization fluctuations that preserves total carrier number in F.

Fig. 3.

Compressional resonances in three unconventional superconductors. Resonance modes dominated by the scalar moduli are shown for (A) PuCoGa₅, (B) CeCoIn₅, and (C) YBa₂Cu₃O_6.60. Although all three materials show a discontinuity at $T_c$ and all stiffen immediately below $T_c$, only PuCoGa₅ shows the dramatic softening above $T_c$.

Fig. 2D shows that the deviation from the anharmonic background extends over a broad temperature range for all three scalar moduli. Analogous to the Curie–Weiss susceptibility of a ferromagnet, $\chi \propto 1/\left(T-T_c\right)$—where an applied magnetic field $\overrightarrow{H}$ couples linearly to the magnetic order parameter $\overrightarrow{M}$ in the free energy (i.e., $\mathrm{\Delta }F=-\overrightarrow{M}\cdot \overrightarrow{H}$); the $\sim 1/\left(T-T_v\right)$ softening of the scalar moduli requires that the fluctuating order parameter η is linearly coupled to a strain of the same symmetry (i.e., η is nonmagnetic and scalar) (19, 20) (SI Text, section III). As in other mixed-valence systems that show elastic softening in the scalar channel, the elastic softening that we observe in PuCoGa₅ suggests valence physics (2, 3, 16). Valence fluctuations (a term that we will use to encompass both fluctuations in the local ionic electron number and fluctuations that preserve total occupation but change the distribution among the 5f states) can naturally lead to an anomalous temperature dependence of the scalar elastic moduli through coupling to volume (or derivatives thereof) (21, 22). This softening is perhaps more easily understood as a divergence in the compressibility (inverse of the bulk modulus), which we show with a fit in Fig. 4B. It is important to note that fluctuations in the (complex) superconducting order parameter-$\mathrm{\Psi }$ cannot be responsible for the scalar softening, because strain couples to superfluid density, not to the order parameter itself ($n_s\propto \mathrm{\Psi }\mathrm{\ast }\mathrm{\Psi }$; i.e., quadratic in order parameter). The same restriction also holds true for any order parameter modulated at finite wave vector [i.e., a charge density wave (CDW) or antiferromagnetism (19)]. Finally, we do not observe the onset of softening at the Kondo temperature in PuCoGa₅[$T_K\approx 250$ (23)], and the temperature dependence that we do observe is qualitatively different from what is observed in integral-valent Kondo systems [e.g., CeCu₆ and CeRu₂Si₂ (24)]. Thus, we attribute the softening to valence fluctuations. Similar behavior is observed in YbInCu₄, which also shows $\sim 1/\left(T-T_v\right)$ elastic softening over a broad temperature range before undergoing a valence transition (3).

Next, we consider the behavior of the elastic moduli across the superconducting transition. A sharp drop in the scalar moduli at $T_c$ (Fig. 2B, Inset) is of order $\mathrm{\Delta }c/c\approx 3\times {10}^{-4}$—within a factor of three of the estimate made from the specific heat jump and $\partial T_c/\partial P$ using Erhenfest relations (1, 25). On entering the superconducting state at 18.1 K, the softening in the $A_{1g}$ channel is truncated (Fig. 2B), indicating that the opening of the superconducting gap on the Fermi surface suppresses the valence fluctuations. Below the superconducting transition, the elastic modulus PuCoGa₅ stiffens at a rate similar to other unconventional superconductors that show no anomalous softening (Fig. 3). One can draw an analogy here with superfluid ³He, where the Cooper pairing is mediated by spin fluctuations and where these fluctuations are truncated on entering the superfluid state (26–28).

The softening of scalar elastic moduli in mixed-valence systems is often accompanied by an anomalously small and/or strongly temperature-dependent Poisson’s ratio (16) [e.g., YbInCu₄ (3)]. In a conventional material, compression along one axis produces a dilation strain along the perpendicular axes, and the ratio of the perpendicular strains is Poisson’s ratio. In a mixed-valence system, compression can force the nearly degenerate valence orbitals to adopt a new configuration (e.g., by increasing the hybridization of the f electrons with the conduction band). This degeneracy results in an anomalous elastic response to uniaxial strain and a small or even negative Poisson’s ratio. Fig. 4 C and D shows the temperature dependences of the two Poisson’s ratios: ${\nu }_{xy}$ describes the in-plane strain response, and ${\nu }_{xz}$ describes the out-of-plane strain response. The magnitude of ${\nu }_{xz}$ for PuCoGa₅ is typical of most metals (29) and nearly temperature-independent (Fig. 4C). The magnitude of ${\nu }_{xy}$, however, is anomalously small, and its temperature dependence mirrors that of the scalar moduli [also note that the softening in $c_{33}$ is much smaller than in $\left(c_{11}+c_{12}\right)/2$] (Fig. 2B). This anomalous anisotropic behavior of the Poisson’s ratios implies an anisotropic character to the valence fluctuations in PuCoGa₅.

Discussion

Valence fluctuations in PuCoGa₅ could manifest as fluctuations between 5f orbitals of different in-plane directional character [e.g., $f_{xyz}$ vs. $f_{z\left(x^2-y^2\right)}$], resulting in fluctuations of the hybridization with neighboring Ga atoms (Fig. 1B) while preserving the total number of f electrons per site (Fig. 4F). This mechanism was proposed to explain non-Fermi liquid behavior and superconductivity in some of the Ce-based heavy fermions (30). In PuCoGa₅, this possibility is supported by recent resonant X-ray emission spectroscopy measurements (5) on PuCoGa₅ and the related compound PuCoIn₅ [in which the 5f electrons are more localized and magnetic than in PuCoGa₅ (10)]. These measurements delineate an intermediate valence state for PuCoGa₅, where a dominant $5f^5$ configuration (Pu³⁺ valence, 62% weight) is degenerate with $5f^4$ (Pu⁴⁺, 29%) and $5f^6$ (Pu²⁺, 9%), resulting in an average valence $z\approx 3.2$. In PuCoIn₅, which has a 9% longer $\widehat{c}$ axis and 8% longer $\widehat{a}$ and $\widehat{b}$ axes than PuCoGa₅, the configurational weight among the 5f orbitals is distributed differently: 77% of $5f^5$, 21% of $5f^4$, and 2% of $5f^6$, with the same average valence of $z\approx 3.2$. If PuCoGa₅, with its smaller unit cell, is analogous to PuCoIn₅ under strain, these measurements suggest the dominant effect of scalar elastic strain is to change the distribution of weights among the 5f orbitals rather than change the average valence ($z\approx 3.2$). The hybridization between Pu and Ga in PuCoGa₅ and the resulting band structure are quasi-2D (31), and thus, transitions between different 5f-shell states that preserve total valence have the largest effect on the in-plane properties. This scenario would explain why ${\nu }_{xy}$ has a strong fluctuation signature, whereas ${\nu }_{xz}$ does not (Fig. 4). The observed truncation of fluctuations at $T_c$ also has a natural explanation: fluctuations of hybridization are intimately connected to the Fermi surface, which becomes gapped at $T_c$. Finally, these valence fluctuations may be responsible for the near-linear resistivity that is observed in PuCoGa₅ (1, 14, 30). We note that previous NMR measurements have reported the presence of spin fluctuations in PuCoGa₅ (32). Our measurements do not exclude this possibility, and they do not rule out their role in the superconductivity; rather, we suggest that valence fluctuations have a dominant role.

The low temperature of the avoided valence transition ($T_v\approx 9$ K) suggests the proximity of a valence quantum critical point in the pressure–temperature phase diagram of PuCoGa₅ that drives the superconductivity (12, 14, 30). A similar scenario has been proposed for CeCu₂Si₂, where one superconducting dome forms around an antiferromagnetic quantum critical point at low pressures and a second, higher-$T_c$ dome forms around a valence quantum critical point at higher pressures (13). For PuCoGa₅, we predict that $T_c$ will reach a maximum, where $T_v$ is tuned (with the correct tuning parameter) to the valence quantum critical point (30, 33). [Because the order parameter associated with a valence transition is scalar, a first-order transition is allowed by symmetry. However, the transition will look second order close to the critical end point of the transition. As long as this end point is low enough in temperature that thermal fluctuations do not dominate, critical fluctuations can still drive superconductivity (33). The low temperature of $T_v$ and the fact that we observe softening suggests that this scenario is the case for PuCoGa₅.] The $T_c$ of PuCoGa₅ can be tuned to $\approx 22$ K with $\approx 10$ GPa of hydrostatic pressure (25). Observing an increase in elastic softening toward maximum $T_c$, perhaps by puled-echo ultrasound under pressure, would further strengthen the case for valence fluctuation-mediated superconductivity in PuCoGa₅. It would also be interesting to explore the effects of uniaxial strain on the magnitude of elastic softening, $T_v$, and $T_c$. Making the crystal structure more or less tetragonal with uniaxial strain along the $\widehat{c}$ axis should tune the 5f degeneracy (34, 35) and thus, the valence fluctuations: uniaxial strain may be the tuning parameter required to reach the valence quantum critical point and may realize even higher $T_c$ values than hydrostatic pressure.

Materials and Methods

Large single crystals of PuCoGa₅ were grown by the self-flux method, which was described in the work by Sarrao et al. (1). A single crystal was polished to the dimensions of $2.208\times 2.240\times 0.641$ mm, with the tetragonal long axis being 2.208 mm. The drive transducer of the resonant ultrasound spectroscopy apparatus was driven well below its own first compressional resonance from 100 kHz to 2.5 MHz. The response voltage generated on the pickup transducer—maximum whenever the drive frequency coincides with a sample resonance—was measured with a custom-built heterodyne amplifier (36). Temperature control was provided by an He⁴ flow cryostat. The temperature was swept over 28 h from 295 to 13 K, sweeping at one-half the rate in the 50- to 13-K region. More details are in SI Text, sections I and II.