Abstract
The Prisoner's Dilemma (PD) is a widely employed metaphor for problems associated with the evolution of cooperative behavior. We have recently proposed an alternative approach to the PD, by exploring "spatial games" in which players--who are either pure cooperators, C, or pure defectors, D--interact with neighbors in some spatial array; in each generation, players add up the scores from all encounters, and in the next generation a given cell is retained by its previous owner or taken over by a neighbor, depending on who has the largest score. Over a wide range of the relevant parameters, we find that C and D persist together indefinitely (without any of the complex strategies that remember past encounters, and anticipate future ones, which characterize essentially all previous work on the iterated PD). Our earlier work, however, dealt with symmetric spatial lattices in two dimensions, deterministic winning and discrete time. We show here that the essential results remain valid in more realistic situations where the spatial distributions of cells are random in two or three dimensions, and where winning is partly probabilistic (rather than being determined by the largest local total). The essential results also remain valid (pace Huberman and Glance [Huberman, B. A. & Glance, N. S. (1993) Proc. Natl. Acad. Sci. USA 90, 7716-7718]) when interactions occur in continuous rather than discrete time.
Full text
PDF

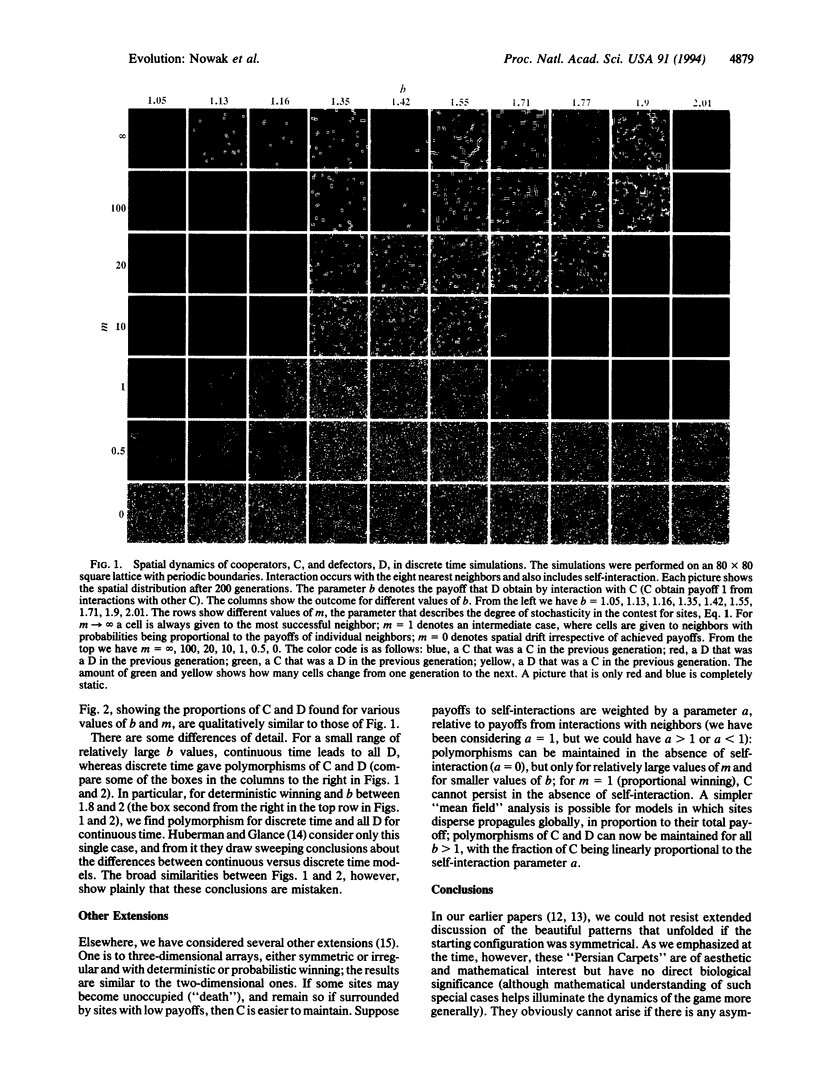
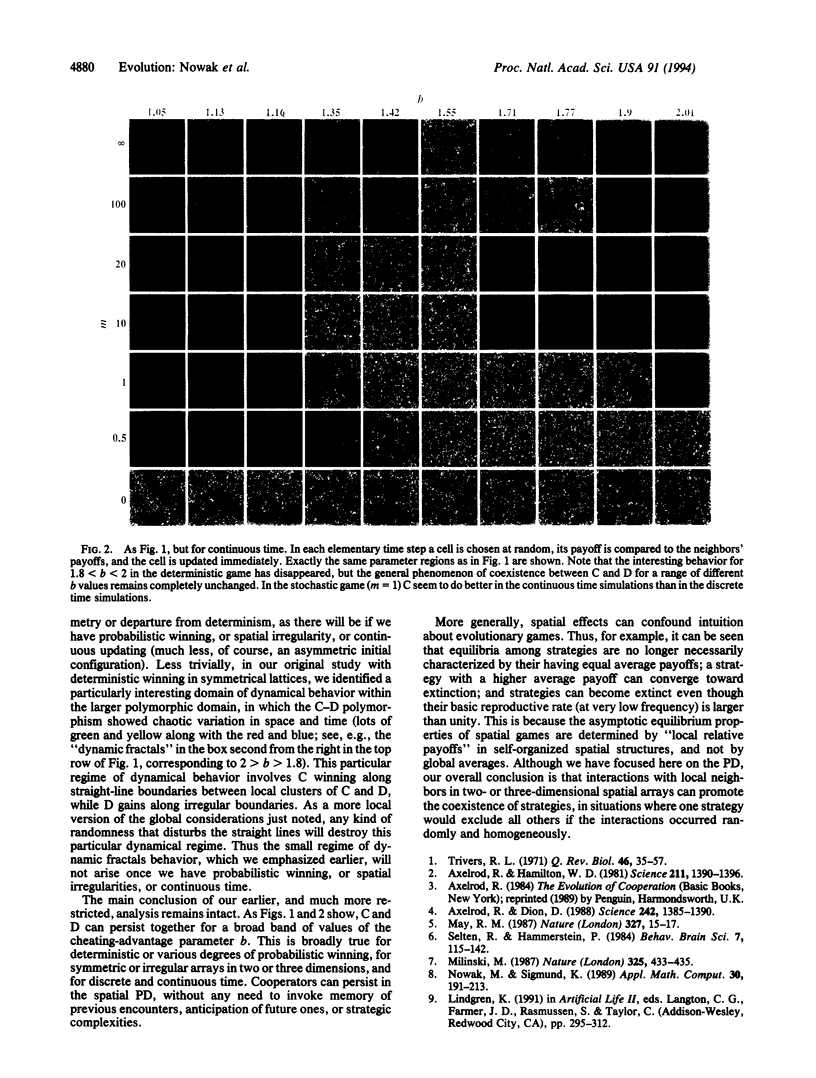

Images in this article
Selected References
These references are in PubMed. This may not be the complete list of references from this article.
- Axelrod R., Dion D. The further evolution of cooperation. Science. 1988 Dec 9;242(4884):1385–1390. doi: 10.1126/science.242.4884.1385. [DOI] [PubMed] [Google Scholar]
- Axelrod R., Hamilton W. D. The evolution of cooperation. Science. 1981 Mar 27;211(4489):1390–1396. doi: 10.1126/science.7466396. [DOI] [PubMed] [Google Scholar]
- Huberman B. A., Glance N. S. Evolutionary games and computer simulations. Proc Natl Acad Sci U S A. 1993 Aug 15;90(16):7716–7718. doi: 10.1073/pnas.90.16.7716. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Milinski M. TIT FOR TAT in sticklebacks and the evolution of cooperation. 1987 Jan 29-Feb 4Nature. 325(6103):433–435. doi: 10.1038/325433a0. [DOI] [PubMed] [Google Scholar]
- Nowak M., Sigmund K. A strategy of win-stay, lose-shift that outperforms tit-for-tat in the Prisoner's Dilemma game. Nature. 1993 Jul 1;364(6432):56–58. doi: 10.1038/364056a0. [DOI] [PubMed] [Google Scholar]