A three-dimensional, extended field of view ultrasound method for estimating large strain mechanical properties of the cervix during pregnancy

Abstract

Cervical shortening and cervical insufficiency contribute to a significant number of preterm births. However, the deformation mechanisms that control how the cervix changes its shape from long and closed to short and dilated are not clear. Investigation of the biomechanical problem is limited by 1) lack of thorough characterization of the three-dimensional anatomical changes associated with cervical deformation and 2) difficulty measuring cervical tissue properties in vivo. The objective of the present study was to explore the feasibility of using three-dimensional ultrasound and fundal pressure to obtain anatomically accurate numerical models of large-strain cervical deformation during pregnancy and enable non-invasive assessment of cervical tissue compliance. Healthy subjects (n=6) and one subject with acute cervical insufficiency in the midtrimester were studied. Extended field of view ultrasound images were obtained of the entire uterus and cervix. These images aided construction of anatomically accurate numerical models. Cervical loading was achieved with fundal pressure, which was quantified with a vaginal pressure catheter. In one subject, the anatomical response to fundal pressure was matched by a model-based simulation of the deformation response, thereby deriving the corresponding cervical mechanical properties and showing the feasibility of non-invasive assessment of compliance. The results of this pilot study demonstrate the feasibility of a biomechanical modeling framework for estimating cervical mechanical properties in vivo. An improved understanding of cervical biomechanical function will clarify the pathophysiology of cervical shortening.

INTRODUCTION

Preterm birth affects close to 13% of pregnancies in the United States,¹ contributes $26 billion to health care costs,² and accounts for more than 50% of neonatal deaths.³ Survivors of preterm birth often experience severe consequences, such as cerebral palsy, respiratory morbidity, mental retardation, blindness, deafness, cardiovascular disease and cancer.⁴ Two therapies are available, progesterone supplementation and cerclage (a stitch around the cervix), but these have not appreciably reduced the incidence of preterm birth.^1,5-7 This is likely because the approach to treatment, as well as research, has been simplistic while the pathways to preterm birth are complex.^8,9

In a significant number of cases, preterm birth is associated with preterm deformation of the cervix. The clinical presentation of cervical deformation is cervical shortening.^10-12 The cervix is a cylindrical anatomical structure and forms the lower part of the uterus. In normal pregnancy, the cervix remains closed until term to permit fetal growth and development. Preterm birth is associated with early, undesired shortening, often in the absence of uterine contractility.^10-12 Since cervical shortening is a strong risk factor for preterm birth, serial evaluation of cervical length using transvaginal ultrasound is recommended to identify women at increased risk.^13,14

Preterm cervical shortening could be caused, in part, by weakened mechanical properties of the cervical stroma. Cervical mechanical properties arise from its fibrous extracellular matrix,¹⁵ which undergoes extensive remodeling during pregnancy to prepare for childbirth.^16,17 Cervical remodeling is apparent on physical examination as softening and shortening. In previous in vitro work, we developed a stringent protocol for measuring the large-strain mechanical properties of cervical tissue in vitro.¹⁸ We showed that the large stress- strain response of the tissue was nonlinear and anisotropic and varied according to obstetric history.^18,19 Our in vitro work was extended by using numerical models from idealized anatomy and a computational framework to demonstrate cervical deformation associated with preterm birth.^20,21 However, this work was based on idealized conditions and did not relate to patient-specific data.

To establish a causal relationship between weakened mechanical properties and preterm cervical shortening, it is necessary to estimate tissue properties in individual patients. However, estimation of tissue properties in vivo presents significant technical hurdles. First, it is necessary to deform the cervix safely and reproducibly. Prior studies of in vivo tissue properties used biomechanical devices to deform the cervix^22,23 but biomechanical devices can be uncomfortable and inconvenient. Second, current biomechanical devices can only access the external os but cervical ripening initiates at the internal os (the junction between the cervix and uterus), where the extracellular matrix composition and properties are different.²⁴ Last, cervical tissue exhibits a nonlinear large-strain response¹⁹ but prior attempts to estimate tissue properties use a limited displacement range (1 – 3 mm).^23,25

Fundal pressure is a safe and common clinical maneuver used to elicit cervical changes during pregnancy.¹¹ Fundal pressure is most often applied between 18 – 26 weeks gestational age. At this gestational age, the uterine fundus (top of the uterus) is palpable near the umbilicus. To elicit cervical changes, the clinician applies pressure to the uterine fundus with an examining hand while monitoring cervical length with transvaginal ultrasound. In most patients, the cervix does not change in response to fundal pressure. In some women, the cervix shortens in real time (the “dynamic” cervix). Dynamic shortening is a known risk factor for preterm birth.¹¹

The goal of the present study was to explore the feasibility of using ultrasound and fundal pressure to obtain anatomically accurate numerical models of large-strain cervical deformation during pregnancy. To achieve this goal, extended field of view ultrasound images were used to obtain numerical models that encompassed the entire uterus and cervix. Fundal pressure was used for cervical loading and was quantified with a vaginal pressure catheter. We present preliminary data on estimation of cervical mechanical properties from one subject using this ultrasound-based method and a previously described inverse finite element framework developed for 3D ultrasound imaging.²⁶

METHODS

Subject Selection

A cross-sectional study was performed at a single tertiary care center. The study was approved by the Institutional Review Board. Subjects were invited to participate either 1) at their second trimester fetal anatomy survey or 2) after admission to Labor and Delivery because of acute cervical insufficiency. Exclusion criteria included symptomatic uterine contractions, higher order multiple gestation, vaginal bleeding and known or suspected placenta previa. An informed consent was obtained before each scan. Patient demographics, pregnancy history and delivery details were obtained by review of medical records.

Extended Field of View Ultrasound

The objective was to obtain an ultrasound image of the entire uterus and cervix appropriate for construction of numerical models (Fig. 1). To achieve this objective, it was necessary to register overlapping 3D volumes to obtain an adequate field of view. Image registration followed a three-step protocol (Fig. 1).

• 1) A series of six 3D ultrasound volumes were obtained using an iU22 Ultrasound System (Philips Medical Systems, Bothell, WA) with a 3D6-2 curved array transducer. The six volumes were saved on a DVD and transferred to a desktop computer for offline image processing.

• 2) The ultrasound volumes were converted into a multidimensional biomedical imaging format (AnalyzeImage 7.5, AnalyzeDirect, Overland Park, KS) using a previously published protocol.²⁷ It was necessary to convert the ultrasound volumes to the Analyze format to allow for manual alignment and image fusion (see below)

• 3) The volumes were manually aligned and fused using a 3D voxel registration module (Analyze 7.0, AnalyzeDirect). The most useful image feature for registration was the border between the amniotic fluid and uterine wall. Sharp contrast was seen between the amniotic fluid and uterine wall. Hence, this feature could be used to align adjacent volumes. At the completion of the protocol, the six volumes had been converted into a single volume with an extended field of view covering the entire uterus and cervix (Figs. 1, 2).

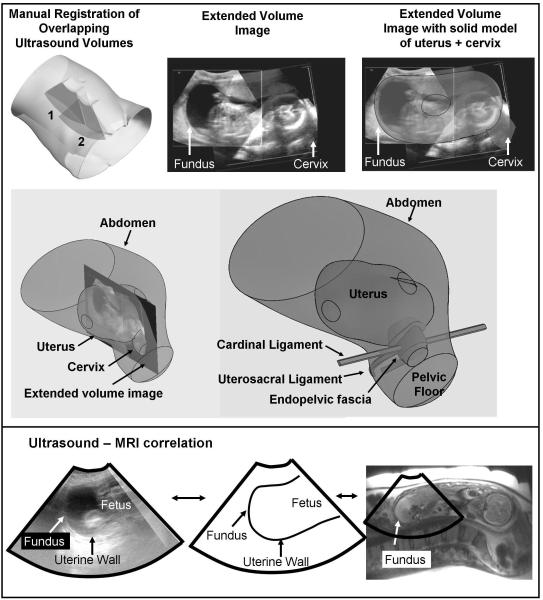
Fig. 1.

Solid model superimposed on an extended field of view ultrasound image of the uterus and cervix

Six ultrasound volumes were manually registered to create a single, extended volume image of the entire uterus and cervix (top). Extended volume images were used to guide development of solid models that captured the shape of the uterus and cervix (middle). Note that only the cervix and uterus were seen with ultrasound images. The pelvic support anatomy (endopelvic fascia, cardinal ligament, uterosacral ligament) were not seen with ultrasound. Previous experience with pelvic MRI and knowledge of anatomic relationships were used to contruct pelvic support anatomy. In one subject, MRI and ultrasound was performed on the same day showing correlation between the two imaging modalities (bottom)

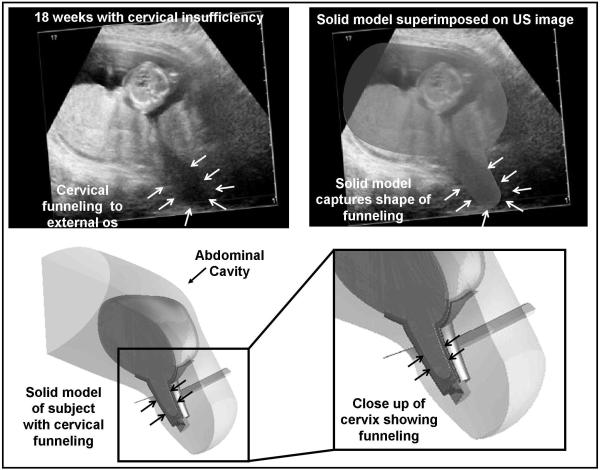
Fig. 2.

Solid model corresponding to the subject with cervical insufficiency

A subject with cervical insufficiency was studied prior to the placement of a physical-examination indicated cerclage. The ultrasound images show protrusion of the amniotic sac to the level of the external os (white arrows, top). A solid model of this anatomy was constructed, which shows the marked cervical deformation associated with cervical insufficiency (bottom). The solid models accurately captured the anatomy of interest. Of note, this subject delivered at 26 weeks gestation.

Numerical Model from Extended Field of View Ultrasound

A numerical model was obtained from the extended field of view ultrasound following a previously published protocol.^27,28 Briefly, 2D images were selected from the 3D volume and placed in the workspace of solid modeling software (Solidworks, Concord, MA). The anatomy of interest was traced on the 2D images and the tracings were combined into a solid model using software tools (Fig. 3). The solid models was exported in CAD format and imported into a commercial finite element program (ABAQUS, Providence, RI).

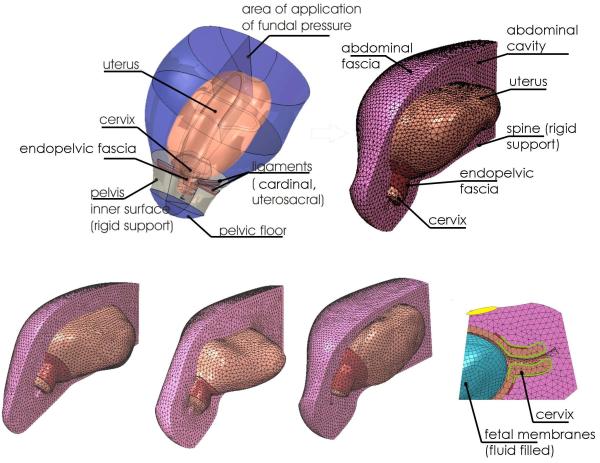
Fig.3.

Numerical models of the uterus and cervix from 5 healthy subjects

The patient-specific solid models comprise the uterus and cervix as well as the surrounding abdominal/pelvic region and the cervical support structures. The model is imported into a finite element package (ABAQUS) and discretized (meshed) with tetrahedral elements. The four models shown come from healthy subjects who delivered at term. The model at the bottom right shows the fluid filled anatomic cavity. This cavity makes contact with the inner surface of the uterine wall.

Vaginal Pressure Increase Associated with Fundal Pressure

To quantify the increase in vaginal pressure associated with fundal pressure, an Urodynamic system (Dorado KT, Laborie, Ontario, Canada) was used. A vaginal pressure catheter (#CAT875, Laborie) was placed anterior to the cervix and vaginal pressure was read continuously (Fig. 4).

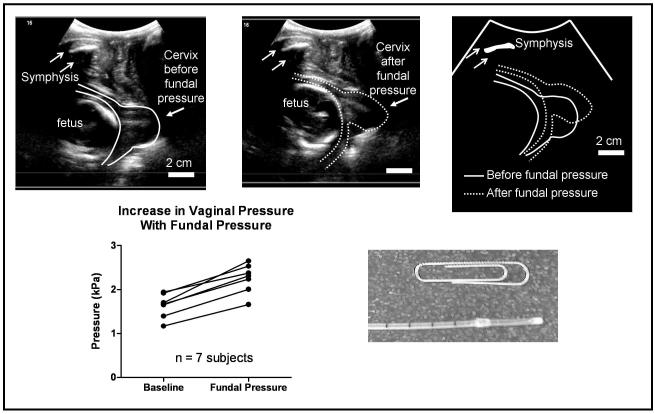
Fig 4.

Quantification of cervical changes and vaginal pressure increases associated with fundal pressure

The ultrasound images show the cervical position before and after fundal pressure. Absolute change in cervical position was measured because a fixed bony landmark was present (symphysis, white arrows). The cartoon at right shows superposition of the cervical images before and after fundal pressure. This cervical displacement was used to guide modeling efforts (Fig. 5). The increase in vaginal pressure (bottom, left) was measured with a vaginal catheter (bottom, right) and was also used to guide modeling efforts. Each data point represents the mean of three measurements.

Cervical Displacement Associated with Fundal Pressure

To measure cervical displacement associated with fundal pressure, it was necessary to use transperineal ultrasound (Fig. 4). The advantage of transperineal ultrasound was that the pelvic bone (symphysis pubis) was visualized in the same field of view as the cervix. The symphysis was used as a fixed reference to determine cervical displacement associated with fundal pressure.²⁹ Transperineal ultrasound did not interfere with vaginal pressure measurement.

Protocol

The following protocol was used for each subject. First, the transabdominal scans were performed, which were used to obtain the extended field of view ultrasound image. Second, the vaginal pressure catheter was placed. Third, fundal pressure was applied for 5 seconds and cervical displacement was measured with transperineal ultrasound. Vaginal pressure was measured before and after fundal pressure with the pressure catheter. Fundal pressure was repeated a total of three times. The mean of the three measurements of vaginal pressure was recorded. The time required for subject involvement was 30 minutes.

Magnetic Resonance Imaging

In 1 subject, MRI and ultrasound was performed on the same day to compare image features using these two modalities for corroboration. The MR scan was performed on a GE Signa HDxt 1.5T system with a single shot fast spin echo sequence (Fig. 1).

Inverse Finite Element Analysis

We have previously described an inverse finite-element modeling framework for estimation of soft tissue mechanical properties using full-field deformation data obtained from 3D ultrasound.²⁶ Briefly, the pelvic anatomy was simplified, as shown in Figure 3, and streamlined so as to capture the biomechanics of cervical deformation while minimizing model complexity. The identified structures include (Table 2, Figs. 1, 3): the uterus and cervix; the fluid-filled amniotic sac (the fetus is not explicitly modeled); the endopelvic fascia and ligaments supporting the cervix; the “abdominal region” (an homogenized representation of the abdominal organs surrounding the uterus); the “abdominal fascia”, an outer layer representing abdominal fascia/muscle/skin; the pelvic floor; and the pelvic bones and spine (modeled as rigid supports/constraints).

Table 2.

Anatomic structures and model properties

Anatomy	Property	Comment
Uterus	Simplified version of full constitutive model19, 20 μ0=10 kPa
Cervix	Simplified version of full constitutive model19, 20 μ0= 0.5 - 50 kPa	Final result for cervix tissue modulus = 6.7 kPa (see results)
Fetal membranes	Incompressible hyperelastic; Polynomial strain energy function (C10= 2 MPa, C30=300 kPa); Thickness 1 mm	Contact with inner uterine surface (see methods)
Amniotic sac (fluid)	Bulk modulus 2.2 GPa; Intrauterine pressure 1 kPa	Volumetric compliance of water
Endopelvic fascia	Elastic, transversely isotropic; Longitudinal modulus 100 kPa; Transverse modulus 10 kPa Longitudinal shear modulus 5 kPa; Bulk modulus 10 MPa	Thickness 1 cm
Cardinal ligaments	See endopelvic fascia
Uterosacral ligaments	See endopelvic fascia
Abdominal cavity	Linear elastic; Modulus 10 kPa; Poisson’s ratio 0.1 – 0.4	Final result abdominal Poisson’s ratio= 0.2 (see results)
Abdominal fascia	Linear, isotropic elastic; Modulus 100 kPa; Poisson’s ratio 0.4
Pelvic floor	Linear, isotropic elastic; Modulus 1.0 MPa; Poisson’s ratio 0.4; Thickness 1 cm
Pelvic bones	Rigid constraint at pelvis inner surface	Figure 3
Spine	Rigid constraint at posterior midline	Figure 3

The anatomy was modeled using both solid (continuum) and shell elements. The solid regions were modeled using continuum linear tetrahedron elements (3D, 4-node (C3D4), Abaqus) and the abdominal fascia, pelvic floor, and fetal membrane were modeled by general-purpose finite strain shell elements (S3, S4, Abaqus), with initial thicknesses of 1 cm for the fascia and pelvic floor and 1mm for the membrane. The separate regions of the model are endowed with suitable tissue properties. The amniotic sac was modeled as a fluid filled cavity with the volumetric compliance of water (i.e., a bulk modulus of 2.2 GPa), and the amniotic membranes were modeled as incompressible, hyperelastic with a polynomial strain energy function (C₁₀= 2 MPa, C₃₀=300 kPa).

A simplified hyperelastic constitutive model was employed for the stroma of the cervix and the uterine walls. The constitutive model is a simplified implementation of the full constitutive model for cervical stroma.^19,20 The bulk response of the tissue, combining the volumetric contribution of the collagen network and the osmotic contributions of glycosaminoglycans and proteoglycans in the ground substance, is modeled as linear elastic with a bulk modulus of 10 MPa for both cervical and uterine stroma. The equilibrium shear properties of the stroma are primarily controlled by the collagen network. The collagen network is represented by an 8-chain model with Langevin statistics whose nonlinear response is prescribed by two material parameters: a small–strain (or “initial”) modulus μ₀, which differs between the uterine and cervical stroma, and a locking stretch λ_L , fixed at λ_L=1.07 for both cervical and uterine stroma, which controls the stiffening of tissue response (modulus increase) at large strains.²⁰ A gradual transition (3 mm) in material properties is established over a narrow section above the internal cervical os, to switch from the properties of the uterine walls, with μ₀=10 kPa, to the properties of the cervix. The initial modulus of cervical tissue, typically in a range μ_0-CRVX=0.5–50 kPa, is the primary fitting parameter to be determined through inverse FE modeling by matching the model predictions to the observed cervical deformation pattern. The abdominal fascia and pelvic floor were modeled as a linear isotropic elastic materials with Young’s moduli of 100 kPa and 1 MPa, respectively, and Poisson’s ratio ν =0.4. The endopelvic fascia and ligaments were modeled as elastic, transversely isotropic, with longitudinal and transverse Young’s moduli of 100 kPa and 10 kPa, respectively, longitudinal shear moduli of 5 kPa, and isotropic volumetric response with a bulk modulus of 10 MPa. The abdominal region was modeled as linear elastic with a Young’s modulus of 10 kPa. The bulk compliance of the abdominal region, defined in terms a homogenized Poisson’s ratio (range ν_ABD =0.1–0.4), is the secondary fitting parameter to be determined through inverse FE modeling by matching the model predictions to the measured vaginal pressure increase associated with fundal pressure.

Loading conditions on the model include gravity (in the posterior direction as the patient is supine during the exam) and an intrauterine pressure of 1 kPa. When the intrauterine pressure is applied to the model, the amniotic sac expands and comes in contact with the uterus; membrane adhesion to the uterus (rough contact with small sliding) is thereby assumed for the contact conditions between the membranes and the uterine inner surface. The application of fundal pressure is simulated by displacing the corresponding area (Figure 3) at the top boundary of the model 1.5 cm posterior and 3 cm inferior. The model was rigidly fixed along the posterior midline (spinal column) and around the pelvis inner surface.

The inverse modeling procedure to fit the primary (μ_0-CRVX ) and secondary (ν_ABD ) model parameters relied on an iterative approach with manual search to minimize error (mismatch) in the displacement of 6 fiduciary markers (3 anterior and 3 posterior) placed along the sagittal midplane of cervix, at the internal os, midpoint, and external os. Bulk compliance of the abdominal organs (as measured by ν_ABD) was optimized to match the increase in pressure measured by the vaginal transducer when fundal pressure is applied.

RESULTS

Patient population

Table 1 shows the demographics of the study population. A total of 7 subjects were enrolled in the study. Six healthy subjects were recruited at the fetal anatomy survey. One subject with cervical insufficiency was recruited prior to the placement of a physical examination-indicated cerclage (“rescue” cerclage). This subject presented at 18 weeks with 2 cm of cervical dilation and fetal membranes prolapsed to the external os (Fig. 2). The subject with a cerclage entered spontaneous labor at 26 weeks gestation. The newborn weight was 1040 gm. The six healthy subjects delivered at term.

Table 1.

Subject demographics

Demographics	Healthy (n = 6)	Cerclage (n = 1)
Age (yr)	29 [27 - 33]	30
Body Mass Index (kg/m2)	33.3 ± 8.6	30.4
Ethnicity  White, not Hispanic  Hispanic	5 1	1
Primiparous (percent)	50 %	0 %
Gestational Age of Scan (weeks)	21 [19 – 24]	18
Gestational Age of Delivery (weeks)	40 [39 – 41]	26
Birthweight (gm)	3399 ± 666	1040

Data presented as mean ± standard deviation, median [range], or percent as indicated.

Solid Model from Extended Field of View Ultrasound

Figure 1 shows a solid model superimposed on an extended field of view ultrasound image of the uterus and cervix. By inspection, the geometry of the uterus and cervix is adequately represented by the shape and volume of the solid model. The extended field of view ultrasound image was used to define the uterus and cervix but not the supporting anatomy such as the endopelvic fascia, and cardinal/uterosacral ligaments. Although the fascia and ligaments are biomechanically relevant, they are difficult to visualize with 3D ultrasound. However, our previous experience with MRI-based datasets^20,28 was a valuable aid to refine the ultrasound-based model to include the endopelvic fascia, the cardinal and uterosacral ligaments and the pelvic floor.

Figure 2 shows a solid model corresponding to the subject with cervical insufficiency. The ultrasound image shows funneling of the amniotic membranes to the external os, which is captured by the solid model. Construction of the solid model from the ultrasound volumes is performed manually and it is a time-intensive task (approximately two days per subject).

Ultrasound – MRI correlation

Figure 1 (right) demonstrates the correlation between the ultrasound image and the MRI image of the same patient. The boundary of the amniotic fluid and uterine wall was used as the anatomical landmark for image segmentation.

Numerical Model from Solid Model

The computational domain was discretized (meshed) with tetrahedral elements as illustrated in Figure 3. Patient-specific computational models from four healthy subjects are shown. The time required to import each solid model into the FE framework and mesh the model was, approximately, 2 days per model. There was a significant improvement in efficiency gained with experience.

Vaginal Pressure and Anatomical Changes Associated with Fundal Pressure

The mean ± SD baseline vaginal pressure was 1.64 ± 0.27 kPa (Fig. 4). This value was similar to previously reported values for vaginal pressure in non-pregnant patients undergoing routine clinical cystometry.³⁰ The mean (95% confidence interval) rise in vaginal pressure associated with fundal pressure was 0.62 (0.47 – 0.77) kPa. Fundal pressure was also associated with significant cervical displacement (Fig. 4).

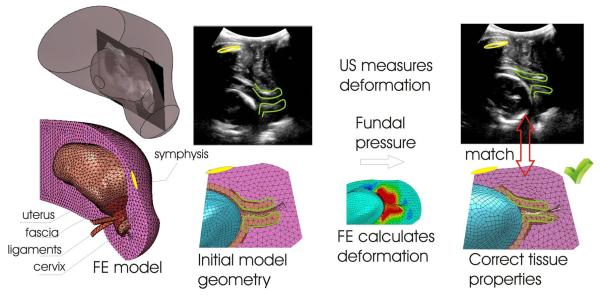
Estimation of Cervical Mechanical Properties During Pregnancy

Under the applied fundal pressure, the cervix descends in the pelvis and deforms against the supporting structures (fascia, ligaments, pelvic floor, abdominal organs), as illustrated in Fig. 4. The pattern of cervical deformation in relation to the surrounding organs provides and indirect measure of tissue compliance. A stiff cervix will tend to maintain its shape, while translating and rotating into the pelvis, transmitting higher loads to the surrounding organs which will deform to accommodate cervical displacement. In contrast, a soft cervix will not just translate and rotate but it will also change its shape and, in the most extreme conditions, funnel to accommodate the penetration of the amniotic sack in the cervical canal. The finite element model predictions of structural deformation will match the observed anatomical changes in cervical geometry due to fundal pressure only if the relative stiffness of the cervical tissue compared to the surrounding organs is properly captured. The measurement of vaginal pressure increase under fundal pressure provides an additional constraint against which the model must be calibrated, to ensure that the model can correctly estimate the magnitude of tissue properties rather than just their relative compliance. The iterative fitting procedure provided a value for the Poisson ratio of the abdominal region ν_ABD = 0.2 to yield a satisfactory match to the measured average rise in vaginal pressure of 0.6 kPa. Figure 5 illustrates the deformation-matching procedure. For the pilot example in the figure, a suitable agreement between model predictions and measured tissue deformation was found for a cervical tissue modulus μ_0-CRVX=6.7 kPa. The position and attitude of the symphysis provides a reliable landmark against which the ultrasound images and the deformed finite element model can be registered.

Fig. 5.

Model-based estimation of cervical mechanical properties

The mechanical properties of the cervix are systematically varied so that the model response matches the observed anatomical response. Only if correct tissue properties are used (right) will the model response match the anatomic response.

DISCUSSION

In this study, extended field of view ultrasound aided construction of anatomically accurate numerical models of the uterus and cervix. Fundal pressure was used for cervical loading, which was quantified with a vaginal pressure catheter. In one subject, the anatomical response to fundal pressure was matched by a model-based simulation of the deformation response, thereby providing an estimate of cervical mechanical properties. Results of this pilot study demonstrate the feasibility of the proposed biomechanical modeling framework to estimate cervical mechanical properties in vivo.

Obstetricians suspect weakened load bearing properties of the cervical stroma contribute to preterm birth. However, this hypothesis is difficult to prove. The lack of a method to measure cervical mechanical properties limits the opportunity for clinical investigation. Others have proposed biomechanical devices to estimate cervical mechanical properties in both pregnant^22,23 and non-pregnant women.^31-34 Also in development are quantitative ultrasound techniques to obtain objective information about cervical microstructure.^35,36 In addition, fundal pressure and transvaginal ultrasound has been used in a semi-quantitative manner to quantify the 2D cervical response to loading.³⁷ The present study is novel for 1) quantifying the response to fundal pressure with a pressure catheter and 2) using a 3D biomechanical modeling framework to estimate cervical mechanical properties during pregnancy.

Fundal pressure was associated with a significant rise in vaginal pressure. The rise in vaginal pressure was seen in all subjects, though some variability was seen. It is not surprising that variability was seen with this measurement. Fundal pressure was applied on the maternal abdomen. The pressure catheter was located in vaginal canal. Given distance between the pressure catheter and the applied load, a significant degree of variation in the measured rise of vaginal pressure was to be expected.

The most useful imaging feature for construction of anatomically accurate numerical models was the border between the amniotic fluid and uterine wall. Sharp contrast between sonolucent fluid and echogenic uterine wall allowed accurate reconstruction of uterine shape. The most challenging anatomical features were the cervix and surrounding endopelvic fascia. Endopelvic fascia is difficult to visualize with ultrasound. Prior experience with MRI datasets aided construction of the cardinal and uterosacral ligaments, which provided appropriate boundary conditions for the cervix in the biomechanical model.²⁸ Accurate numerical models of uterine and pelvic anatomy were essential to capture the large strain deformation response of the cervix during pregnancy.

The most significant limitation of the present study is the amount of time required to obtain anatomically accurate numerical models. No automatic segmentation tools were available for the anatomy of interest. Thus, manual segmentation was performed and several days were required to convert ultrasound data to a numerical model. Semi-automated algorithms to speed segmentation of important structures will be an important improvement before this technique is applied in a clinical setting. Obstetrical imaging lends itself to automated segmentation because the boundaries between the amniotic fluid and uterine wall are readily identifiable. However, image segmentation has been applied less in ultrasound than CT or MRI because ultrasound image segmentation is strongly influenced by the quality of the data. Ultrasound data is complicated by multiple artifacts such as speckle, unclear boundaries, signal attenuation, shadows, and dropout.³⁸ Although there are challenges associated with ultrasound image segmentation, ultrasound is more practical than other imaging modalities. In addition, recent advances in spatial/temporal resolution and 3D volume imaging have improved data quality, and thus the renewed interest in ultrasound image segmentation. Improved resolution of the displacement field in the cervix following fundal pressure may also provide sufficient signal to capture the effects of inhomogeneous tissue properties, allowing the implementation of more refined simulation models with axial and radial gradients in cervical compliance.

Patient-specific inverse FE modeling techniques are a powerful tool but require an extensive and costly modeling effort, which is arguably beyond the scope of routine prenatal care. Rather, patient-specific models and inverse FE methods can be employed to develop a simplified assessment framework for clinical practice. The development of an alternative, simplified protocol to estimate a “cervical tissue compliance index” based solely on US-measured dynamic changes and pelvic deformation patterns under fundal pressure is an ambitious goal for the proposed methodologies, with direct clinical relevance.

The clinical consequence of weakened cervical load bearing properties is cervical shortening and cervical insufficiency. Although shortening clearly precedes preterm birth in many patients,¹¹ less clear is whether shortening is a cause of preterm birth or a consequence of a different pathophysiology. There is an important need in the field to clarify this question so that interventions to improve cervical function can be appropriately developed. The long-term goal of the present research is to provide a mechanistic basis for how the cervix stays closed in normal pregnancy but shortens in preterm birth.

In summary, we report a technique to use extended field of view ultrasound to obtain anatomically accurate numerical models of the uterus and cervix during pregnancy. The models were used with an inverse finite element framework to obtain preliminary estimates of cervical mechanical properties in vivo. Our proposed testing modality, which can safely assess the mechanical response of the cervical tissue at the internal os, could be extremely useful to both research and clinical applications. A better understanding of cervical biomechanical function will clarify the pathophysiology of normal and abnormal cervical shortening. An improved understanding of deformation mechanisms will point to therapies that aim to improve cervical performance and thus prevent spontaneous preterm birth