Significance
Cells contain 30–40% dissolved protein and RNA by volume. In vivo protein binding and stability might differ significantly from in vitro measurements. Crowding by the excluded volume of these macromolecules has been studied extensively by experiment and theory. When the crowding effects of macromolecules, water, and ions are treated on an equal footing, the effect is opposite to that commonly believed. Large molecules are less effective at crowding than water and ions. There is also a surprisingly weak dependence on crowder size. Molecules of medium size have the same effect as much larger macromolecules like proteins and RNA. These results require a reassessment of observed high-concentration effects and of strategies to mimic in vivo conditions with in vitro experiments.
Keywords: macromolecular crowding, hard sphere fluids, exclusion effects, depletion effects
Abstract
The aqueous milieu inside cells contains as much as 30–40% dissolved protein and RNA by volume. This large concentration of macromolecules is expected to cause significant deviations from solution ideality. In vivo biochemical reaction rates and equilibria might differ significantly from those measured in the majority of in vitro experiments that are performed at much lower macromolecule concentrations. Consequently crowding, a nonspecific phenomenon believed to arise from the large excluded volume of these macromolecules, has been studied extensively by experimental and theoretical methods. However, the relevant theory has not been applied consistently. When the steric effects of macromolecular crowders and small molecules like water and ions are treated on an equal footing, the effect of the macromolecules is opposite to that commonly believed. Large molecules are less effective at crowding than water and ions. There is also a surprisingly weak dependence on crowder size. Molecules of medium size, ∼5 Å radius, have the same effect as much larger macromolecules like proteins and RNA. These results require a reassessment of observed high-concentration effects and of strategies to mimic in vivo conditions with in vitro experiments.
The milieu inside cells contains a large amount of solutes that include small ions, metabolites, and macromolecules. Typical protein/RNA concentrations range from 300 mg/mL to 400 mg/mL or about 30–40% by weight. These are 10-fold or more higher than the macromolecular concentrations usually encountered in in vitro measurements and could lead to significant nonideality in solution behavior. The possibility that in vivo biochemical reaction rates and equilibria might be quite different from measured values due to this nonideality has stimulated considerable research. Macromolecular crowding in particular has stimulated a large number of studies, which have been extensively reviewed (1–5). Crowding has been variously described as physical occupation of volume by the macromolecules, which is then unavailable to other molecules, as an excluded volume effect, as a nonspecific effect due to steric repulsion, and as eliminating positions at which the protein can be placed (2, 5, 6). The other contribution to nonideality is from interactions between various solution components, either attractive or repulsive—repulsive interactions distinct from the van der Waals core repulsion or steric factor underlying crowding. We know very little about the contribution of intersolute interactions to nonideality in vivo. Crowding has been more extensively studied because the steric or excluded volume of a macromolecule is a well-defined property, it is always present, and its qualitative effect on biochemical reactions seems obvious (2). However, as I demonstrate in this paper, the effect of crowding macromolecules on a biochemical reaction is not obvious, the relevant theory has not been consistently applied, and indeed the effect is opposite to that commonly believed.
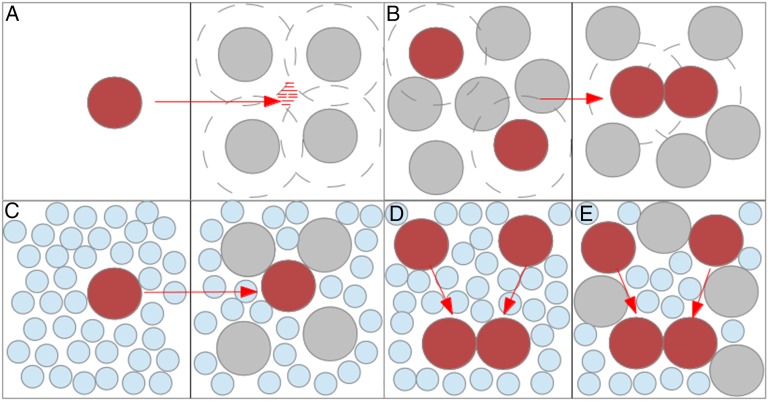
Analyses of the effect of crowding macromolecules on biochemical reactions often start with a schematic similar to that in Fig. 1A. Here a protein or other macromolecule is introduced into a solution containing some concentration of large crowding molecules. Avoidance of steric overlap between the crowders and the protein constrains the protein’s possible positions. Its center can explore only the inside of the small red shaded region. Therefore, the crowders, it is argued, make it harder to transfer the protein into the solution. Equivalent ways of expressing this are that the crowders increase the chemical potential of the protein, its effective concentration in the intermacromolecular volume is increased, or its activity is increased. Presumably, the excluded volume effect is greater the larger the crowders are and the higher their concentration is. In Fig. 1B, this qualitative picture is extended to a binding reaction: Applying the effective concentration argument leads almost inescapably to the conclusion that the crowders enhance binding. This all seems very reasonable, and more quantitative analyses of crowding then aim to measure or calculate exactly how much the protein’s configurational space is reduced by given size crowders at particular concentrations. In this argument the crowding effect of macromolecules is predicated on the idea that other molecules must avoid energetically costly steric overlaps, so they are constrained to a smaller volume of the solution. What is missing, however, is the solvent: principally water and small ions. These solvent molecules also cannot overlap each other, the protein, or the crowders. If the protein is constrained to the smaller volume between crowders, why is it not also constrained to the smaller volume between the waters? The volume between big crowding molecules is also filled with small crowding molecules! More than 20 y ago Berg argued that water must be included (7), but with rare exceptions (8, 9) his work was ignored. More realistic schematics of the chemical potential and dimerization reactions are shown in Fig. 1 C–E. The issue is not that crowders (protein, RNA, cosolutes like Ficoll, and PEG) exclude; everything excludes. It is that these crowders are significantly larger than water and ions. Now the pertinent questions are “Is it easier to insert a protein into a packed solvent of small molecules or a packed ‘solvent’ of small and large molecules?” and “Is it easier to form a protein complex by removing protein surface area from a well-packed solvent of small molecules or from a packed solvent of small and large molecules?” The theory of hard sphere (HS) fluid mixtures is tailor made to answer these questions because it treats in a rigorous way the thermodynamic properties of mixtures of different size particles than cannot overlap because of steric interactions. The rest of this paper applies the theory to crowding by high concentrations of macromolecules with some surprising conclusions. To focus on size effects, I treat all molecules as rigid. Flexible macromolecular crowders introduce interesting complications from polymer theory that are beyond the scope of this paper. This paper deals only with effects on equilibria, such as protein binding and protein stability. The effect of crowders on rates, through solution viscosity changes for example, is not considered.
Fig. 1.
Excluded volume effects. (A) Insertion of protein (in red) into a solution containing macromolecular crowders (in gray). Dashed circles indicate the zone around crowders that excludes the protein: Its center can access only the red hatched volume. (B) Dimerization in the presence of macromolecular crowders. The dashed circles indicate how the volume of the zone from which crowder centers are excluded is decreased upon binding. (C) Transfer of a protein from water (blue spheres) into an aqueous solution containing macromolecular crowders. (D and E) Binding in water vs. an aqueous solution of crowders.
Theory
Hard Sphere Fluid Mixtures.
The theory of HS fluid mixtures is well established and rigorous (10–14). Before applying it quantitatively to crowding, it is useful to describe qualitatively what it says about the processes depicted in Fig. 1 C–E. This introduces the physical content of the theory and perhaps shakes the automatic assumption that large molecules must be better crowders than small ones.
All molecules must avoid steric overlaps. In statistical mechanics terms, this avoidance lowers the solution entropy relative to an ideal gas at the same number density due to the reduction in the mutual configurational phase space available for all of the molecules. There is a concomitant increase in free energy and chemical potential. Simplifying somewhat, when we place a protein into solution, some fraction of the solvent molecules have their configurational entropy reduced by avoiding overlap with the protein and each other (Fig. 1C). From here on the term solvent refers to everything the protein may encounter: water, ions, cosolutes, and crowders. The change in entropy can be quantified by changes in the local density distributions of the solvent molecules caused by the solute, as described in detail below. These local density changes occur principally for solvent close to or in contact with the protein solute. Now in Fig. 1C, Left (no macromolecular crowders) a larger number of small molecules are affected by the presence of the protein, whereas in Fig. 1C, Right a lesser number of molecules (some large, some small) are affected, because one large crowder replaces many smaller solvent molecules. Note that the solution volume is increased by approximately the same amount in each case, by the protein’s partial molar volume. The net entropy change depends both on the magnitude of local density changes and the number of molecules affected. We must resist the intuition from mechanics that it is somehow harder to move a large molecule out of the way vs. a small one. In thermodynamics the entropy change from a given change in local density is the same for a small molecule and a large one. Of course different size molecules may experience different solute-related perturbations in their local density, but because more molecules are affected by the presence of the protein when we have just small solvent molecules, i.e., no crowders, it should hopefully no longer be obvious that there is a larger configurational entropy decrease upon adding a protein to a solution with large crowders. To proceed further we need the quantitative theory of HS fluids as applied to a mixture of different size molecules.
In the theory of liquids and solutions, a quantity of particular importance is the radial distribution function (rdf) gij(r), which describes the relative probability of finding molecules of types i and j at a distance r from each other. gij(r) is the local density of molecule i around j at distance r relative to the bulk density of i, ρi(r)/ρibulk (and vice versa). For hard sphere species i and j with diameters di and dj gij(r) is zero when r < di/2 + dj/2, which is the essence of the excluded volume effect. The value of the rdf at the point of contact, gij(r = di/2 + dj/2), is denoted here by g0ij. HS theory provides expressions for g0ij for every (i, j) in terms of the diameters di and mole fractions xi of all of the species and the packing fraction ξ (the volume fraction filled by the hard spheres) (12, 13). From this one can then determine the excess entropy of the fluid with respect to an ideal gas, Sex (12–14). Sex, which is negative in sign, accurately describes the reduction in entropy due to the mutual avoidance of overlaps between all of the component spheres. From the excess entropy it is straightforward to obtain an expression for the excess HS chemical potential μiex of solute i with respect to the ideal solute chemical potential term ln(ρi), where ρi is the number density or concentration of the solute. μiex is positive in sign and represents the extra work of introducing a spherical solute into a solvent represented as a mixture of different size hard spheres while avoiding any steric overlaps. For component i in a mixture of two hard sphere components 1 and 2, μiex can be written as a cubic polynomial of the solute’s diameter di (14, 15),
| [1] |
where k is Boltzmann's constant, and T is temperature. The coefficients in Eq. 1 are given by
| [2] |
| [3] |
| [4] |
| [5] |
The factors D1, D2, and D3 are the mole fraction-weighted averages of the solvent diameter, diameter squared, and diameter cubed, respectively. For a two-component solvent
| [6] |
and x1 + x2 = 1.
Although the expression for chemical potential given by Eqs. 1–6 looks rather complicated, there is a straightforward physical interpretation for each of the terms. The cubic and quadratic terms describe the dependence on solute volume and area, respectively. The linear term may be interpreted as the curvature of the solute, so collectively the linear, quadratic, and cubic terms account for the shape and size of the solute. All are positive and so contribute unfavorably to the free energy of introducing the protein solute into solution.
Two physically distinct factors enter into the expressions for the coefficients: (i) the solvent packing fraction ξ and (ii) the diameter averages, Di=1,2,3. These averages depend on the solvent composition, which is defined by the amounts and sizes of the two solvent components, namely water (x1 and d1) and crowder (x2 and d2). The constant term L0 is independent of solvent composition and is not discussed further. The other three coefficients L1, L2, and L3 do depend on solvent composition through Di=1,2,3. Attention is drawn to the factors of in their denominators. Now πD3/6 = Vav is the number-averaged solvent molecular volume. For pure water (x1 = 1) Vav would simply be the hard sphere volume of a water molecule. As increasing amounts of a large crowder are added, Vav increases because d2 >> d1. The number-averaged solvent diameter (D1) and diameter squared (D2) that appear in the numerators of the coefficients also increase. However, they increase less rapidly than D3 as they depend on lower powers of d2. So as large crowders are added, the volume, area, and curvature coefficients Li=1,2,3 all decrease.
The coefficient L3 is unique in that it alone depends on (d1 − d2), the difference in diameter between the two solvent components. This difference appears in the second term of Eq. 5 and reduces the contribution of the volume term to the excess chemical potential. Physically it arises because there are more ways to pack a mixture of small and large spheres together than the pure components separately.
Now consider protein folding or binding. The effect of crowders depends on how they change the difference in excess chemical potential between two states: unfolded vs. folded or free vs. bound, respectively. Partial molecule volume changes, although significant for these processes, are typically less than 1% of the total volume of the proteins themselves for folding (16, 17) and dimerization (18). Changes in solvent accessible area upon folding or binding, in contrast, are of the same order of magnitude as the total protein accessible areas, perhaps 30% or more (19). So the leading contribution from excluded volume effects will be controlled by the magnitude of the area coefficient L2, whatever the sign of the volume change (The area change upon folding or binding of course is negative). The curvature term can be thought of as a correction to the surface area term, but it becomes less important for larger protein solutes (SI Text). L2 quantifies the excluded volume contribution to a macromolecule’s surface free energy. It favors reduction in solvent exposed area and thus the folded state and the bound state. But large crowders reduce this driving force.
In summary, HS fluid theory states that the steric effect of large crowders reduces the excess chemical potential of a protein in solution relative to pure water. The idea that the small size of water contributes to a high surface free energy at the solute–water interface is not new. Indeed this is the basis for a physical explanation of the hydrophobic effect (20). Large crowders reduce the steric penalty for solvent exposed surface area of a protein, so they disfavor association and folding. Large crowders also act on the volume and curvature contributions in the same manner. The underlying reason is that the entropic cost of avoiding overlaps with a single large molecule is less than that of the many smaller solvent molecules it displaces. This is a nonspecific, purely excluded volume effect that arises when we treat the steric effects of large crowders and small molecules like water and ions on an equal footing.
Relationship to Earlier Applications of Hard Sphere Theory.
In the limit of x2 = 0 in Eq. 1, Di = d1i, L3 = (ξ + ξ2 + ξ3)/((1 − ξ)3d13) and we recover the standard equations for scaled particle theory (SPT) (10, 21) in which the solvent (e.g., pure water) is homogeneous with respect to particle size. This form of SPT has been used, for example, to study solvation of apolar solutes in water (21). The other limit, x2 = 1, Di = d2i, corresponds to pure crowder and has been applied to macromolecular crowding (22). However, as illustrated in Fig. 1A this omits the effect of water and the crucial fact that the solvent consists of molecules of different sizes. Berg, on the other hand, treated the solvent as a mixture of different size spheres representing water and crowders (7). The expression he used for excess chemical potential is mathematically identical to Eqs. 1–5 used here, with two distinctions. First, the coefficients were not explicitly factored into separate solvent packing and composition terms in the manner of Snider and Herrington (14). So the effects of crowder size and solvent packing were not separately analyzed, as they are here. Second, Berg applied the theory in a manner whereby the composition and solvent density covary in a complex way to balance what is known as the virtual HS pressure. This leads to unrealistically large mixing volume changes (SI Text).
SI Text
Crowding Effects on Binding.
To illustrate the physical effect of large crowders on binding I consider a monomer–dimer equilibrium. Rather than attempt to model the dimer through extension of HS theory to nonspherical objects, which involves considerable complexity for even very simple shapes, I apply a simple dimensional analysis. The coefficients L1, L2, and L3 of Eq. 1 (main text) are interpreted literally as multipliers of the radius of curvature, area, and volume of either monomer or dimer. Then the excluded volume contribution from the solvent to the free energy of binding is given by the difference in reactant and product excess chemical potentials,
| [S1] |
where the subscripts m and d refer to monomer and dimer, respectively. ΔAbind and ΔVbind are the change in solvent accessible area and partial molar volume of binding, respectively, and rm and rd are the effective or average radii of curvature of the monomer and dimer, respectively. The volume term in Eq. S1 is assumed to be negligible, for reasons given in the main text. So the change in free energy of binding arising from addition of a crowding molecule is
| [S2] |
The changes in curvature and area coefficient are written as explicit functions of the amount (x2) and size (d2) of crowder, to emphasize that Eq. S2 represents the effect of solvent composition relative to pure water, where ΔLi=1,2 = 0. To focus on crowder size and concentration effects I again assume that ξ is constant. Clearly ΔAbind is large and negative for proteins. ΔL2 is also negative (main text, Fig. 2 A and C) so the area term of Eq. S2 is positive and disfavors binding.
Fig. 2.
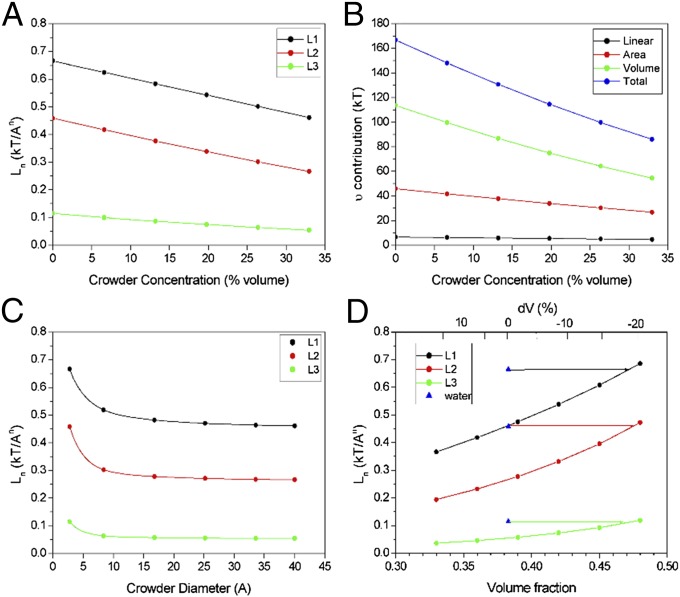
Linear/curvature (L1), area (L2), and volume (L3) excess chemical potential coefficients. (A) Dependence on the concentration of a 40-Å diameter crowder in a solvent with packing fraction 0.383. (B) Corresponding excess chemical potential contributions for a solute of 10 Å diameter. (C) Dependence on crowder size for a solvent with packing fraction 0.383 containing 33% crowder and 67% water by volume. (D) Effect of solvent packing fraction for a 40-Å diameter crowder at 33% concentration by volume. Blue triangles indicate coefficients for pure water at a packing fraction of 0.383 for reference (from A). Horizontal lines indicate the approximate increase in packing fraction required for the crowder solution to match pure water.
Consider now the relative size of the curvature term. First, from Fig. 2 A and C (main text) ΔL1/ΔL2 ∼ 1 Å. Second, it is reasonable to assume that the effective or average radius of curvature ri scales with the linear dimension of the protein. In fact, for a spherical solute ri = di/2. The area of course scales as the second power, so (rd − 2rm) ∼ O(d) whereas ΔAbind ∼ O(d2). Then the ratio of linear to area terms,
| [S3] |
scales inversely with solute diameter. For a protein of diameter 10 Å or larger, the curvature term is an order of magnitude smaller than the area term.
Finally, consider the sign of the curvature contribution. A linear dependence of radius of curvature on diameter is equivalent to a 1/3 power dependence on volume, so a dimer of twice the volume of one monomer would have about 21/3 greater average radius of curvature (rd is exactly 21/3rm for the completely spherical case). It is quite probable, then, that rd < 2rm in which case the curvature term in Eq. S2 is also positive and reinforces the area term. Otherwise it opposes the area term but is smaller in magnitude. This is the justification for the argument in the main text that the effect of crowders is primarily on the area, or surface free energy term, and that curvature effects, i.e., deviations from sphericity, are a second-order correction.
Relationship to Hard Sphere Mixture Treatment of Berg.
The equation for hard sphere fluid mixtures used by Berg is mathematically identical to Eqs. 1–5 in the main text (7). His coefficients, however, are undifferentiated with respect to solution packing, ξ, and solution composition (size and concentration of crowder) given by Di=1,2,3. Snider and Herrington’s insightful formulation separates these two components (14). In the main text this is used to separate the effects of crowder size and solution packing, revealing the fact that at a given packing fraction larger molecules are poorer crowders. A further difference in treatment is that Berg varies crowder concentration under conditions of equal hard sphere pressure. This requires that solvent density and crowder concentration covary in a complicated way [Berg’s appendix (7), equations A8–A10]. Because the virtual pressure exerted by hard spheres is orders of magnitude larger than the ambient pressure of 1 Atm, balancing it requires significant density changes. Using Berg’s equation relating solution packing and hard sphere pressure (ref. 7, equation A9), one finds that adding a 40-Å diameter crowder to water to obtain a 33% concentration by volume requires a packing fraction change of about 0.09. Using ΔVpack = 100(0.383/ξ − 1), this translates into mixing volume changes of the order of −20%, which seem unrealistically large. These large packing changes would oppose the effects of crowder size, and Berg also concludes that large molecules are weak crowders.
Fig. S1.
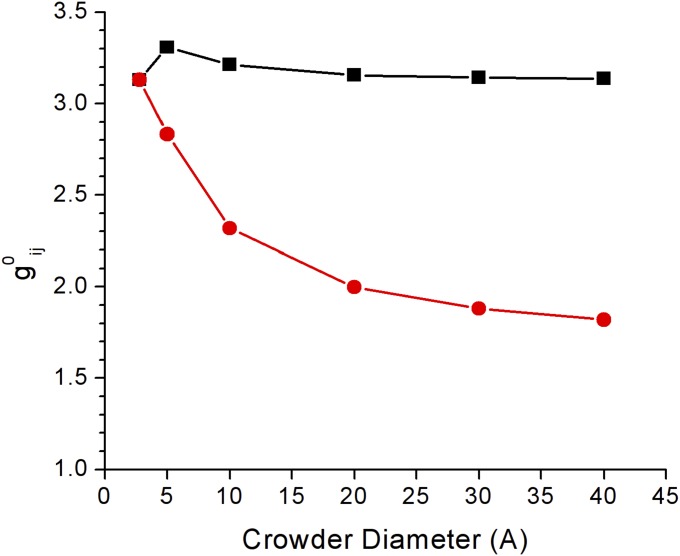
Local excess solvent molecule densities in contact with a 40-Å solute, relative to their concentration in bulk. g01j is for small solvent spheres of the diameter of water, d1 = 2.8 Å (red circles). g02j is for crowders ranging in diameter d2 from 2.8 Å to 40 Å (black squares). An equimolar mixture of water and crowder was used for the solvent to permit comparison of both absolute and relative densities at contact. Values were calculated using equations of Lebowitz (12).
Results
To illustrate the application of HS fluid mixture theory to crowding, Eqs. 1–5 were evaluated with parameters corresponding to typical crowding conditions in the experimental literature. For water d1 = 2.8 Å. Given the experimental density this corresponds to a packing fraction ξ = 0.383. To isolate the effect of crowder concentration and size, the same packing fraction is used for crowder solutions. The effect of different packing fractions is considered once solvent composition effects are examined.
Fig. 2A shows the effect of a 40-Å diameter crowding molecule on the chemical potential coefficients Li=1,2,3 for crowder concentrations from 0% to 33% by volume in water. For pure water the coefficients are all positive. The crowder decreases all three coefficients monotonically as its concentration is increased. Taking the magnitudes of Li=1,2,3 as a measure of crowding power, the large molecule is a poorer crowder than water. This is illustrated by the effect of crowder concentration on the net excess chemical potential of a solute of diameter 10 Å (Fig. 2B). The crowder decreases the excess chemical potential by about a factor of 2. Looking at the individual contributions, most come from the solute volume and area-dependent terms. The curvature term is smaller, indicating that corrections to the crowding effect for nonspherical solutes will be second order relative to the area term.
Next, the effect of crowder size was examined. Its diameter d2 was increased from the size of water, 2.8 Å, up to 40 Å. The volume concentration was kept fixed at fv = 33% by setting the crowder’s mole fraction to
| [7] |
The values of the curvature, area, and volume coefficients shown in Fig. 2C all decrease as the crowder gets larger: Thermodynamically speaking, crowding is reduced. The effect plateaus for a crowder/water diameter ratio of about 4, corresponding to a surprisingly modest crowder diameter of ∼10 Å. This is considerably smaller than globular proteins, which have diameters of 30 Å and up. The physical basis for the plateau is straightforward. As the crowder size increases, the mole fraction required to make up 33% of the volume of the solvent decreases, and these two effects balance. The solvent composition terms in Eqs. 3–5 are the mole fraction averaged solvent dimensions Di=1,2,3. Using Eqs. 6 and 7, one can show that these tend to the limit Di = d1i/(1 − fv) as d2 >> d1, becoming independent of crowder size.
In Fig. 2B, the 4:1 crowder/protein solute diameter ratio used corresponds approximately to the case investigated by Benton et al. (23), who studied the effect of the large crowder Ficoll 70 on a small 9,200 g/mol protein chymotrypsin inhibitor. Application to other crowder–solute size ratios used in the literature is straightforward using Eqs. 1–5. Because the solute volume, area, and curvature coefficients are all affected in the same way by crowder size, the direction of the net effect is the same whether the crowder is smaller than or larger than the protein solute. The conclusion from Fig. 2 A–C is that based purely on steric factors large molecules are less effective crowders than water or solvent components of similar size to water. Because water is pretty much the smallest solvent component available, this effectively rules out more effective crowders based on size.
Finally I consider the effect of changes in packing fraction of the solvent when large crowder molecules are added to it. Calculations were done for a constant solvent composition of 33% crowder and 67% water by volume. A crowder diameter of 40 Å was used, well into the plateau region of Fig. 2C, so crowder size is eliminated as a factor. Fig. 2D shows the curvature, area, and volume coefficients L1, L2, and L3 as the packing fraction is varied from 0.33 to 0.48. The triangles in blue are the coefficient values for pure water at ξ = 0.383 for reference. L1, L2, and L3 with crowder present are less than the pure water values over a wide range of packing fractions, meaning less crowding power. The horizontal lines indicate that the packing fraction would have to increase to ∼0.48 to reverse the size effect and make a macromolecule as good as water as a crowder. To be better than water would require a still larger increase. To put this in perspective, the change in solvent volume relative to pure water corresponding to a change in ξ is given by ΔV(%) = 100(0.383/ξ − 1). This is plotted on the upper abscissa scale in Fig. 2D. Changes greater than −20% are required. What packing fraction changes actually occur in vivo needs to be investigated.
Discussion
The contribution of HS fluid mixture theory to understanding crowding is twofold. First, it explicitly recognizes that all of the components of a solvent—water, ions, and macromolecules—will exclude the solute. Second, it accounts for the fact that each macromolecular crowder replaces many smaller solvent molecules. Thus, a smaller number of solvent molecules are perturbed by the presence of a given size solute, with a reduction in the excess chemical potential contribution from steric effects. The particular model used to quantify this is idealized (What model for steric effects is not?), treating the molecules as spherical and rigid. However, to first order the effect depends on relative numbers of perturbed solvent molecules, so the conclusions reached here are likely to be very general. Moreover, the terms depending on area and curvature are affected in the same way by crowders, suggesting the conclusions carry over to nonspherical solutes. This analysis also indicates that more attention to packing effects is needed. For example, Graziano has shown, using scaled particle theory, that one can explain the protein-stabilizing effect of the small molecule sucrose through packing changes (9).
The effects of excluded volume are framed here in terms of reduction in solvent entropy due to avoidance of steric overlap, but there is a close connection to other theories of solutions through the radial distribution function gij(r). The local excess/deficit of species i at a distance r from species j is proportional to gij(r) − 1, a factor that appears in theories of the Kirkwood–Buff type (24). Integrating ρibulk(gij(r) − 1) over the solvent volume gives the net excess/deficit of species i around species j, resulting in a positive or negative preferential interaction coefficient, Γ, respectively (25, 26). Positive Γ indicates a favorable interaction between the species, i.e., a net reduction in the chemical potential of j due to i (and of i due to j). In terms of Gibbs’ theory for adsorption isotherms, a positive excess lowers the surface free energy, whereas a depletion raises the free energy (27). The radial distribution function value at contact, g0ij, plays the same role in HS theories because the relevant interaction is a contact potential. At constant packing fraction, addition of larger molecules to the solvent reduces the chemical potential coefficients (Fig. 2 A–C, particularly the area term). Concomitantly, the local excess of larger molecules at the solute surface (g02j) is greater than that of the smaller ones (g01j) (SI Text). There is a preferential interaction with the solute of the larger spheres over the smaller spheres. In terms of Gibbs’ theory, the surface free energy is lowered. So addition of a larger crowder reduces the penalty for unbound/unfolded states of larger surface area. Noting that −kTln(gij(r)) = ω(r) defines a potential of mean force (pmf) between i and j (28), then g02j > g01j can also be interpreted as a more favorable (or more accurately, less unfavorable) interaction between the solute and a larger crowder. It should be emphasized that because there are no direct forces between pairs of particles from the hard sphere potential, the interactions described by g0ij, whatever terminology is used, are purely entropic, multibody effects.
Referring to the binding equilibrium in Fig. 1 D and E, the solvent-excluded volume effect favors the bound state with the buried surface area: It has a lower free energy. So the free energy must at some point change as the surfaces approach. In other words, there must be a force, which overall acts to drive the surfaces together and desolvate them. Indeed, the component of the solvent-induced force arising from cosolute or crowder exclusion is known as the depletion force (29, 30). Depletion forces were first described by Asakura and Oosawa (31), who pointed out that when the distance of closest approach of two solute surfaces was less than the size of any cosolute, that component was excluded or depleted from the intersolute region. This imparts a long-range force on the scale of the largest solvent component. In the Asakura–Oosawa (AO) model the force is given by the osmotic pressure of the excluded cosolute at its bulk concentration, effectively a mean-field treatment, where the water is treated as a continuum. The leading contribution to depletion forces, as the name suggests, is avoidance of energetically costly steric overlaps. Not surprisingly, the depletion effect is built into HS theories (32). In a recent review of the theory of depletion forces Trokhymchuk and Henderson note that in the AO model, the net free energy decrease going from fully separated surfaces to complete depletion at contact is given by ω(0)/kT = −3ξdi/2d2 (in the notation of this paper) (30). Thus, at constant packing fraction, a cosolute molecule of smaller diameter d2 produces a larger net attraction (albeit the depletion force sets in at shorter range). In a more accurate treatment of depletion forces, also based on HS theory, Trokhymchuk and Henderson (ref. 30, figure 4) find the same qualitative behavior: At constant packing fraction smaller cosolutes produce a larger net attractive energy at full contact, although it develops at shorter range. Thus, for crowder size effects on equilibria, both models for depletion forces agree with the conclusions of this work. Although kinetic aspects are not considered here, it should be noted that the range and profile of the depletion forces, whether there are barriers arising from the granularity of the water and cosolutes, become important for rates of binding or folding.
There are two difficulties with the standard crowding model depicted in Fig. 1 A and B. First, it predicts a general, nonspecific enhancement of every putative protein–protein association in the cell and a global enhancement in protein stability. As one review author puts it, excluded volume effects are like gravity, inescapable because all macromolecules exclude (2). It is thus difficult to explain lack of crowding effects or weaker than expected effects in specific cases. For example, 200 mg/mL of dextran increases the stability of protein L by less than 0.5 kcal/mol (33), which is almost below the resolution of stability measurements. ApoMB dimerization was enhanced by 200 mg/mL of RNaseA but not by the same concentration of human serum albumin (34). Other examples are not rare (35, 36) and perhaps are underreported in the literature as negative results often are. Of particular relevance is the recent study of Benton et al. in which careful control experiments compare the effect of large and small crowders at the same volume concentration and show little effect (23). This is exactly the behavior expected from the HS fluid mixture model results in Fig. 2C.
The second difficulty is that observed effects of osmolytes and crowders on protein binding and folding often have significant enthalpic contributions (23, 37–39). Excluded volume effects are entropic, whether treated by the mean-field AO, scaled particle, or HS mixture models. The implications of these enthalpic changes for the mechanism of osmolyte stabilization were pointed out by Politi and Harries (37): There must be other intermolecular forces acting in addition to steric crowding. Sukenik et al. categorized possible mechanisms based on the measured sign and relative magnitudes of enthalpic and entropic contributions (38). Senske et al. did a similar classification of possible mechanisms for protein stabilization based on observed changes in enthalpy of unfolding and melting temperature Tm (which depends also on entropy) (39). To assign possible mechanisms one must first determine the baseline effect: the sign and magnitude of the entropy change expected from purely excluded volume effects from all solvent components, water and crowders, as emphasized here. Interestingly, Politi and Harries found that the favorable entropy for protein folding is diminished upon adding cosolutes (37), which they point out is opposite to that predicted by the conventional steric crowding model. However, this is the direction expected from the HS fluid mixture theory used here. Replacement of multiple small water molecules by each larger cosolute reduces the net entropy change.
Looking to the future, to move beyond purely steric excluded volume effects and account for enthalpic as well as entropic effects of crowders and cosolutes, it will be necessary to augment the hard sphere fluid mixture model with the other, longer-range intermolecular forces. One successful approach is to treat these forces as a perturbation to the hard sphere potential (40, 41). Sapir and Harries have already shown that adding longer-range forces to the mean-field Asakura–Oosawa model for exclusion effects successfully accounts for enthalpic effects of cosolutes (29, 42). Adding the water/small ion exclusion effects to those of the crowders, as described here, should result in further progress.
In summary, it should be emphasized that I am not saying that high concentrations of macromolecules in the cell do not cause significant nonideality and hence potentially different behavior from in vitro experiments. There are many experimental studies that see effects at high concentrations. I am saying that purely excluded volume effects from macromolecules do not work in the manner and direction commonly assumed. The effect is arguably much less important than believed, as indicated by its observed absence in cases where it should be present and by the predicted weak dependence on macromolecular size. Finally, caution should be exercised in incorporating high macromolecular concentration conditions into existing in vitro experiments with the aim of making them more realistic in vivo models. Assuming excluded volume effects are much smaller than believed, there is a risk of introducing unanticipated and unrealistic intersolute interaction effects instead. Even apparently neutral polymer crowders like PEG, dextran, and Ficoll are known to make specific differential interactions with proteins and nucleic acids. These interactions are potent enough that they are widely used for affinity separation by aqueous two-phase polymer systems (43, 44).
Supplementary Material
Acknowledgments
I thank Gary Pielak for critical comments; Greg van Duyne, Kushol Gupta, and Brian Fuglestad for helpful discussions; and the E. R. Johnson Foundation for support.
Footnotes
The author declares no conflict of interest.
This article is a PNAS Direct Submission.
This article contains supporting information online at www.pnas.org/lookup/suppl/doi:10.1073/pnas.1505396112/-/DCSupplemental.
References
- 1.Minton AP. The influence of macromolecular crowding and macromolecular confinement on biochemical reactions in physiological media. J Biol Chem. 2001;276(14):10577–10580. doi: 10.1074/jbc.R100005200. [DOI] [PubMed] [Google Scholar]
- 2.Ellis RJ. Macromolecular crowding: Obvious but underappreciated. Trends Biochem Sci. 2001;26(10):597–604. doi: 10.1016/s0968-0004(01)01938-7. [DOI] [PubMed] [Google Scholar]
- 3.Ellis RJ. Macromolecular crowding: An important but neglected aspect of the intracellular environment. Curr Opin Struct Biol. 2001;11(1):114–119. doi: 10.1016/s0959-440x(00)00172-x. [DOI] [PubMed] [Google Scholar]
- 4.Ellis RJ, Minton AP. Protein aggregation in crowded environments. Biol Chem. 2006;387(5):485–497. doi: 10.1515/BC.2006.064. [DOI] [PubMed] [Google Scholar]
- 5.Zhou H-X, Rivas G, Minton AP. Macromolecular crowding and confinement: Biochemical, biophysical, and potential physiological consequences. Annu Rev Biophys. 2008;37(1):375–397. doi: 10.1146/annurev.biophys.37.032807.125817. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Zhai Y, Winter R. Effect of molecular crowding on the temperature-pressure stability diagram of ribonuclease A. ChemPhysChem. 2013;14(2):386–393. doi: 10.1002/cphc.201200767. [DOI] [PubMed] [Google Scholar]
- 7.Berg OG. The influence of macromolecular crowding on thermodynamic activity: Solubility and dimerization constants for spherical and dumbbell-shaped molecules in a hard-sphere mixture. Biopolymers. 1990;30(11–12):1027–1037. doi: 10.1002/bip.360301104. [DOI] [PubMed] [Google Scholar]
- 8.Davis-Searles PR, Saunders AJ, Erie DA, Winzor DJ, Pielak GJ. Interpreting the effects of small uncharged solutes on protein-folding equilibria. Annu Rev Biophys Biomol Struct. 2001;30(1):271–306. doi: 10.1146/annurev.biophys.30.1.271. [DOI] [PubMed] [Google Scholar]
- 9.Graziano G. How does sucrose stabilize the native state of globular proteins? Int J Biol Macromol. 2012;50(1):230–235. doi: 10.1016/j.ijbiomac.2011.10.025. [DOI] [PubMed] [Google Scholar]
- 10.Reiss H, Frisch HL, Helfand E. Statistical mechanics of rigid spheres. J Chem Phys. 1959;31:369–380. [Google Scholar]
- 11.Reiss H, Frisch HL, Helfand E, Lebowitz JL. Aspects of the statistical thermodynamics of real fluids. J Chem Phys. 1960;32:119–124. [Google Scholar]
- 12.Lebowitz JL. Exact solution of generalized Percus Yevick equation for a mixture of hard spheres. Phys Rev. 1964;133:A895–A899. [Google Scholar]
- 13.Mansoori GA, Carnahan NF, Starling KE, Leland TW. Equilibrium thermodynamic properties of the mixture of hard spheres. J Chem Phys. 1971;54:1523. [Google Scholar]
- 14.Snider NS, Herrington TM. Hard sphere model of binary liquid mixtures. J Chem Phys. 1967;47:2248–2255. [Google Scholar]
- 15.Neff RO, McQuarrie D. A statistical mechanical theory of solubility. J Phys Chem. 1973;77:413–418. [Google Scholar]
- 16.Brun L, Isom DG, Velu P, García-Moreno B, Royer CA. Hydration of the folding transition state ensemble of a protein. Biochemistry. 2006;45(11):3473–3480. doi: 10.1021/bi052638z. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Pappenberger G, Saudan C, Becker M, Merbach AE, Kiefhaber T. Denaturant-induced movement of the transition state of protein folding revealed by high-pressure stopped-flow measurements. Proc Natl Acad Sci USA. 2000;97(1):17–22. doi: 10.1073/pnas.97.1.17. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Marchal S, et al. Under pressure that splits a family in two. The case of lipocalin family. PLoS ONE. 2012;7(11):e50489. doi: 10.1371/journal.pone.0050489. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 19.Chothia C. The nature of the accessible and buried surfaces in proteins. J Mol Biol. 1976;105(1):1–12. doi: 10.1016/0022-2836(76)90191-1. [DOI] [PubMed] [Google Scholar]
- 20.Lee B. The physical origin of the low solubility of nonpolar solutes in water. Biopolymers. 1985;24(5):813–823. doi: 10.1002/bip.360240507. [DOI] [PubMed] [Google Scholar]
- 21.Pierotti RA. Aqueous solutions of nonpolar gases. J Phys Chem. 1965;69:281–289. [Google Scholar]
- 22.Minton AP. Excluded volume as a determinant of macromolecular structure and reactivity. Biopolymers. 1981;20(10):2093–2120. [Google Scholar]
- 23.Benton LA, Smith AE, Young GB, Pielak GJ. Unexpected effects of macromolecular crowding on protein stability. Biochemistry. 2012;51(49):9773–9775. doi: 10.1021/bi300909q. [DOI] [PubMed] [Google Scholar]
- 24.Kirkwood JG. Theory of Liquids. Gordon and Breach; New York: 1968. [Google Scholar]
- 25.Schellman JA. Selective binding and solvent denaturation. Biopolymers. 1987;26(4):549–559. doi: 10.1002/bip.360260408. [DOI] [PubMed] [Google Scholar]
- 26.Anderson CF, Record MT. Polyelectrolyte theories and their application to DNA. Annu Rev Phys Chem. 1982;33:191–222. [Google Scholar]
- 27.Adamson AW. Physical Chemistry of Surfaces. 3rd Ed John Wiley & Sons; New York: 1976. [Google Scholar]
- 28.Donald McQuarrie . Statistical Mechanics. Harper & Row; New York: 1976. [Google Scholar]
- 29.Sapir L, Harries D. Is the depletion force entropic? Molecular crowding beyond steric interactions. Curr Opin Colloid Interface Sci. 2015;20(1):3–10. [Google Scholar]
- 30.Trokhymchuk A, Henderson D. Depletion forces in bulk and in confined domains: From Asakura–Oosawa to recent statistical physics advances. Curr Opin Colloid Interface Sci. 2015;20(1):32–38. [Google Scholar]
- 31.Asakura S, Oosawa F. On interaction between two bodies immersed in a solution of macromolecules. J Chem Phys. 1954;22:1255–1256. [Google Scholar]
- 32.Rosenfeld Y. Phase separation of asymmetric binary hard-sphere fluids: Self-consistent density functional theory. Phys Rev Lett. 1994;72(24):3831–3834. doi: 10.1103/PhysRevLett.72.3831. [DOI] [PubMed] [Google Scholar]
- 33.Adén J, Wittung-Stafshede P. Folding of an unfolded protein by macromolecular crowding in vitro. Biochemistry. 2014;53(14):2271–2277. doi: 10.1021/bi500222g. [DOI] [PubMed] [Google Scholar]
- 34.Zorrilla S, Rivas G, Acuña AU, Lillo MP. Protein self-association in crowded protein solutions: A time-resolved fluorescence polarization study. Protein Sci. 2004;13(11):2960–2969. doi: 10.1110/ps.04809404. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Miklos AC, Li C, Sorrell CD, Lyon LA, Pielak GJ. An upper limit for macromolecular crowding effects. BMC Biophys. 2011;4:13. doi: 10.1186/2046-1682-4-13. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Schlesinger AP, Wang Y, Tadeo X, Millet O, Pielak GJ. Macromolecular crowding fails to fold a globular protein in cells. J Am Chem Soc. 2011;133(21):8082–8085. doi: 10.1021/ja201206t. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Politi R, Harries D. Enthalpically driven peptide stabilization by protective osmolytes. Chem Commun. 2010;46(35):6449–6451. doi: 10.1039/c0cc01763a. [DOI] [PubMed] [Google Scholar]
- 38.Sukenik S, Sapir L, Harries D. Balance of enthalpy and entropy in depletion forces. Curr Opin Colloid Interface Sci. 2013;18(6):495–501. [Google Scholar]
- 39.Senske M, et al. Protein stabilization by macromolecular crowding through enthalpy rather than entropy. J Am Chem Soc. 2014;136(25):9036–9041. doi: 10.1021/ja503205y. [DOI] [PubMed] [Google Scholar]
- 40.Wertheim MS. Fluids with highly directional attractive forces. II. Thermodynamic perturbation theory and integral equations. J Stat Phys. 1984;35(1–2):35–47. [Google Scholar]
- 41.Wertheim MS. Fluids with highly directional attractive forces. IV. Equilibrium polymerization. J Stat Phys. 1986;42(3–4):477–492. [Google Scholar]
- 42.Sapir L, Harries D. Origin of enthalpic depletion forces. J Phys Chem Lett. 2014;5(7):1061–1065. doi: 10.1021/jz5002715. [DOI] [PubMed] [Google Scholar]
- 43.Brooks DE, Sharp K, Fisher D. Theoretical aspects of partitioning. In: Walter H, Brooks DE, Fisher D, editors. Partitioning in Aqueous Two Phase Systems. Academic; New York: 1986. pp. 11–126. [Google Scholar]
- 44.Johansson G. Partitioning of proteins. In: Walter H, Brooks DE, Fisher D, editors. Partitioning in Aqueous Two Phase Systems. Academic; New York: 1986. pp. 161–219. [Google Scholar]