Abstract
A structural model of the in vivo cornea, which accounts for tissue swelling behaviour, for the three-dimensional organization of stromal fibres and for collagen-swelling interaction, is proposed. Modelled as a binary electrolyte gel in thermodynamic equilibrium, the stromal electrostatic free energy is based on the mean-field approximation. To account for active endothelial ionic transport in the in vivo cornea, which modulates osmotic pressure and hydration, stromal mobile ions are shown to satisfy a modified Boltzmann distribution. The elasticity of the stromal collagen network is modelled based on three-dimensional collagen orientation probability distributions for every point in the stroma obtained by synthesizing X-ray diffraction data for azimuthal angle distributions and second harmonic-generated image processing for inclination angle distributions. The model is implemented in a finite-element framework and employed to predict free and confined swelling of stroma in an ionic bath. For the in vivo cornea, the model is used to predict corneal swelling due to increasing intraocular pressure (IOP) and is adapted to model swelling in Fuchs' corneal dystrophy. The biomechanical response of the in vivo cornea to a typical LASIK surgery for myopia is analysed, including tissue fluid pressure and swelling responses. The model provides a new interpretation of the corneal active hydration control (pump-leak) mechanism based on osmotic pressure modulation. The results also illustrate the structural necessity of fibre inclination in stabilizing the corneal refractive surface with respect to changes in tissue hydration and IOP.
Keywords: cornea, hydration, collagen-swelling interaction, proteoglycan, osmotic pressure, pump-leak
1. Introduction
In the simplest terms, the cornea is a fibre-reinforced fluid membrane, which resists the intraocular pressure (IOP) applied on its internal boundary. Its tensile strength derives from the three-dimensional organization of collagen fibres and its bulk properties derive from the interfibrillar fluid pressure. The fluid pressure depends on both the IOP and the tissue osmotic pressure. The osmotic pressure causes the cornea to have a tendency to swell by imbibing water from the adjacent anterior chamber of the eye [1,2]. In the in vivo cornea, the osmotic pressure is modulated by active ionic transport processes as a means to control the level of tissue hydration, which is important for transparency and also affects the mechanical behaviour of the tissue (cf. bending of a swollen and non-swollen cornea). Ideally, the tissue fluid pressure, swelling effects (including active modulation processes) and the local interaction of collagen fibres with the swelling tissue, should be accounted for in any structural model based on first principles. Some important modelling concepts towards this end have already been introduced, as described below. However, no fully three-dimensional and comprehensive model has yet been presented. It is noteworthy that current finite-element-based models for structural analysis of the cornea treat the interfibrillar fluid as an incompressible or nearly incompressible elastic solid [3–6]. While this approach is convenient, it cannot describe swelling behaviour or (steady-state) bulk compressibility. The goal of this work is to present a mathematical description of the corneal stroma as an electrolyte gel, characterizing the fluid pressure, swelling behaviour and collagen-swelling interaction. We show that such a model can extend predictive accuracy in corneal biomechanics.
The cornea has five principal layers which, from anterior to posterior, are the epithelium, Bowman's, stroma, Descemet's and endothelium. Bowman's and Descemet's layers are basement membranes for the cellular epithelium and endothelium layers, respectively. The stroma occupies 90% of the cornea's thickness and like other soft, highly hydrated and charged collagenous tissues such as cartilage and intervertebral disc, is a polyelectrolyte gel consisting of a mixture of interacting fluid, solid and ionic phases. Water is the principal component of stroma which saturates the collagen solid phase, solvates the ionic phase and accounts for about 78% of the cornea by weight [7]. A small portion of the water is cellular or bound to the stromal collagen [8], but much of the water is free to flow within the stroma in response to gradients in fluid pressure. The solid phase of corneal stroma comprises a flexible collagen network, organized as fibrils within lamellae and associated proteoglycans (PGs). Stromal PGs have sulfated linear side chains of negatively charged disaccharide units called glycosaminoglycans (GAGs) that are covalently bound at one end to the PG core protein [9,10]. At normal pH, the stromal fixed charge is almost entirely due to GAG ionization [1]. The ionic phase includes dissolved salts, primarily Na+ and Cl−, and metabolites such as 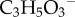 (lactate ion) and
(lactate ion) and 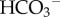 (bicarbonate ion).
(bicarbonate ion).
The stromal mobile ions interact electrostatically with the GAG fixed charges and form cloud-like distributions giving rise to osmotic pressure. The aqueous humour filling the anterior chamber produces the IOP, acts as an ionic bath for the stroma and provides a reservoir of water that is available for exchange with the stroma. Water transport across the permeable endothelial layer is driven by the osmotic pressure difference between the stroma and the aqueous humour. When the stromal ionic concentration exceeds the ionic concentration in the aqueous humour, a positive osmotic pressure difference exists and the stroma will swell by inflow of water from the anterior chamber until the ionic concentrations are equalized or the expansion is restrained by some agent. By contrast, the anterior stroma is sealed by the epithelial cellular layer, which is nearly impermeable to water and ions (although permeable to O2 and CO2) [11]. In the in vivo cornea, the endothelial layer supports active molecular mechanisms that produce an outward flux of ions from the stroma into the aqueous humour. Our analysis herein confirms that such active pumping reduces the stromal ionic concentrations and hence lowers the osmotic pressure [1]. The regulatory system and details of the molecular mechanisms responsible for active ion transport, which requires Na+, K+, ATPase and carbonic anhydrase activity to transport 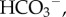 Cl− and possibly Na+, are not yet fully understood [12].
Cl− and possibly Na+, are not yet fully understood [12].
From a macroscopic perspective, the control of hydration has been explained by a ‘pump-leak’ theory [12–14]. In the pioneering work of [15], based on the phenomenological membrane transport theory of Kedem–Katchalsky (KK) [16], non-equilibrium fluid and ionic fluxes through the corneal stroma and endothelium were modelled and time-dependent swelling resulting from osmotic perturbations were predicted. This work, and the related study by Ruberti & Klyce [17], provides a theoretical confirmation that active endothelial ion transport produces an osmotic gradient across the endothelium which can modulate water flow and swelling. Li & Tighe [18] later proposed an extended model which considers multiple ionic species and Leung et al. [19] have further extended the theory to include metabolites (e.g. 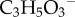 ) and metabolic reactions in a steady-state model. These models employ a one-dimensional representation of the cornea, do not consider stromal fixed charge or collagen interaction with swelling, and the swelling (i.e. osmotic) pressure is not derived, but assumed as an empirical function of tissue hydration based on measurements by Hedbys & Dohlman [20]. In the important extension by Bryant & McDonnell [21], a spherically symmetric (one-dimensional) steady-state model based on the triphasic theory of Lai et al. [22] was combined with KK theory for passive and active transport across the endothelium. This model describes ionic interaction with fixed charges, fluid interaction with a solid elastic phase and ideal Donnan osmotic pressure. While greatly simplifying the stromal elasticity, this model does capture the full range of interactions in an elegant triphasic framework. The above models are complex, and it is not surprising that they do not agree for all predictions, for example, on the sign of the stromal fluid pressure. In this work, we build on these foundational efforts but take an alternative modelling approach.
) and metabolic reactions in a steady-state model. These models employ a one-dimensional representation of the cornea, do not consider stromal fixed charge or collagen interaction with swelling, and the swelling (i.e. osmotic) pressure is not derived, but assumed as an empirical function of tissue hydration based on measurements by Hedbys & Dohlman [20]. In the important extension by Bryant & McDonnell [21], a spherically symmetric (one-dimensional) steady-state model based on the triphasic theory of Lai et al. [22] was combined with KK theory for passive and active transport across the endothelium. This model describes ionic interaction with fixed charges, fluid interaction with a solid elastic phase and ideal Donnan osmotic pressure. While greatly simplifying the stromal elasticity, this model does capture the full range of interactions in an elegant triphasic framework. The above models are complex, and it is not surprising that they do not agree for all predictions, for example, on the sign of the stromal fluid pressure. In this work, we build on these foundational efforts but take an alternative modelling approach.
In the proposed model, conditions of thermodynamic equilibrium are assumed to hold, rendering water and ionic fluxes time-independent. Such an approach has utility when the long-time, steady-state response of the cornea to disease processes or surgical alteration is desired. While modelling based on steady-state conditions does not allow the time course of swelling to be described, it does avoid some of the high complexity of non-equilibrium multiphasic theory and the analytical simplicity of the resulting theory leads to a practical and effective theory for finite-element-based general structural analysis of the living cornea.
Modelled as an electrolyte gel, the free energy is taken to be additively decomposed into various components which characterize the behaviour of the tissue under general deformations [23,24]. A key ingredient in this approach is the statement of electrostatic free energy. For the ex vivo cornea with no active ionic transport, we use the mean-field approximation of the Helmholtz free energy for a binary electrolyte which measures the energy of the GAG-based fixed charge, the osmotic energy of mobile ions in a Boltzmann distribution and the dielectric free energy [25]. To account for active endothelial ion transport in the in vivo cornea, we consider thermodynamic equilibrium expressed in terms of ionic and water electrochemical potentials and show that the stromal mobile ions satisfy a modified Boltzmann distribution that depends on the active ionic fluxes and endothelial ionic permeability. The theory easily takes into account the important volume exclusion effects associated with collagen and keratocyte populations [23] and the electrostatic free energy corresponding to the modified Boltzmann ionic distribution is readily found and used to predict the osmotic pressure in the in vivo cornea. Under certain assumptions on the electrostatic potential, we are able to find analytical approximations for the electrostatic free energy density and osmotic pressure for the in vivo cornea. The electrostatic free energy functional is convex in the volume dilation and provides the key variational ingredient for the finite-element formulation. The approach can be easily implemented within a standard finite-element framework using only the displacement field.
Turning to collagen-swelling interaction, the influence of collagen architecture on tissue swelling is readily demonstrated in the ex vivo cornea. When a sample of human corneal stroma is immersed in deionized water, the tissue undergoes extreme swelling to many times its original thickness by absorbing water from the surrounding bath. However, the observed swelling is far from uniform, reflecting the interaction of local swelling with the collagen network. The swelling is observed to occur primarily in the thickness direction and is most pronounced in the posterior region of the stroma [26–29]. An analogous situation also occurs in the in vivo swollen cornea [30]. This observed rigidity of the anterior cornea with respect to swelling under extreme hydration states has been ascribed to the specific architecture of the collagen fibres [29,31].
The stroma consists of 200–500 superposed sheet-like lamellae. Meek et al. [32] quantified the orientation of lamellae when viewed in the plane perpendicular to the optical axis using X-ray diffraction. That study, and numerous others since, described superior–inferior and nasal–temporal preferential collagen orientations in the central cornea and a circumferential orientation near the limbus. These data have been employed directly or indirectly in a number of continuum mechanics-based models to characterize the elastic anisotropy induced by this ‘in-plane’ description of collagen architecture, e.g. [3–6]. Abahussin et al. [33] have also performed X-ray diffraction studies on isolated third-thickness central stromal samples and showed that the percentage of lamellae exhibiting preferential orientation is greatest in the posterior stroma and reduces towards the anterior.
To investigate the inclination of lamellae as seen in corneal cross-sections, Morishige et al. [34] used second harmonic-generated (SHG) image stacks to reveal lamellae exiting Bowman's layer at steep angles (average 23° maximum 43°) and extending up to 120 µm into the stromal depth. It has long been thought that lamella inclination and insertion into Bowman's layer provides mechanical stabilization of the refractive surface [26–29]. High-resolution macroscopic imaging, which combines many SHG image stacks to produce entire limbus-to-limbus stromal three-dimensional cross-sections, has allowed some lamellae to be traced over millimetres, even with branching and led to the discovery of ‘bow string’ fibres that enter and exit Bowman's layer [35,36]. Recently, Winkler et al. [37] processed SHG images to quantify inclination angle distributions in the anterior stroma and found that lamellae inclination angles satisfy a Gaussian distribution with inclination angles being maximum at the anterior stroma and reducing linearly towards the posterior.
In this work, we have synthesized X-ray scattering intensity data and SHG image processing to describe the spatially varying, three-dimensional orientation of lamellae at every point in the human stroma, including through the depth. The raw data are still incomplete in some regions and extrapolation has been necessary, as described in §5. Nevertheless, the most significant features of collagen architecture are now most probably accounted for, allowing a comprehensive description of the elastic anisotropy of the stromal collagen network and regional collagen-swelling interaction.
The proposed model is implemented in a finite-element framework and employed to predict free and confined swelling of stroma in an ionic bath. For the in vivo cornea, the model is used to simulate corneal hydration changes which occur as a result of increasing IOP, such as occurs in glaucoma. Corneal swelling in Fuchs' corneal dystrophy is employed as an illustration of how the model can be adapted to describe certain pathological conditions. Whenever the cornea is altered by the surgical removal of tissue, the tissue fluid pressure will change and a swelling response can be anticipated. We analyse the biomechanical response of the in vivo cornea to a typical LASIK surgery for myopia and predict, for the first time, how the fluid pressure and tissue swelling will respond. As a validation of the model, the predicted biomechanical response of the cornea (based on elastic deformation and swelling) is compared to extensive clinical data.
2. Electrolyte gel in thermodynamic equilibrium
2.1. Electrostatic free energy
Consider a binary electrolyte gel, consisting of a mixture of solid, fluid and ionic phases, immersed in an ionic bath at constant electrostatic potential 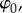 hydrostatic pressure p0, and ionic concentration
hydrostatic pressure p0, and ionic concentration 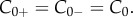 The stroma can be modelled as such a binary electrolyte of Na+ and Cl−. This is justified by the fact that the molar concentration of these ions accounts for about 85% of the total molarity of the aqueous humour [18,19], which acts as an ionic bath. Noting that all ionic species in the stroma have the same valence value of −1, we set the ionic concentration of Na+ and Cl− in the aqueous humour to
The stroma can be modelled as such a binary electrolyte of Na+ and Cl−. This is justified by the fact that the molar concentration of these ions accounts for about 85% of the total molarity of the aqueous humour [18,19], which acts as an ionic bath. Noting that all ionic species in the stroma have the same valence value of −1, we set the ionic concentration of Na+ and Cl− in the aqueous humour to  and thereby account for the total molarity of all solutes in the aqueous humour, which is approximately 300 mM [18,19,23].
and thereby account for the total molarity of all solutes in the aqueous humour, which is approximately 300 mM [18,19,23].
The reference state for the electrostatic free energy is taken to be the normo-hydrated cornea (i.e. the physiological state) which can be routinely measured. A unit cell reference configuration is denoted Ω0, with volume V0, and with a fixed charge concentration distribution modelled by the function 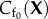 with
with 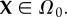 The motion maps Ω0 into the current configuration Ω with deformation gradient F, considered uniform over the unit cell by virtue of the small unit cell size. The volume of Ω is V = JV0 with
The motion maps Ω0 into the current configuration Ω with deformation gradient F, considered uniform over the unit cell by virtue of the small unit cell size. The volume of Ω is V = JV0 with  The fixed charge concentration distribution in the current configuration is modelled by the function Cf(x, J) with
The fixed charge concentration distribution in the current configuration is modelled by the function Cf(x, J) with 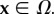 The fixed charge concentration functions
The fixed charge concentration functions 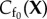 and Cf(x, J) are developed in §3.1.
and Cf(x, J) are developed in §3.1.
The reference configuration Ω0 will generally not be a stress-free configuration for the solid (collagen) phase1 and it is necessary to introduce a stress-free reference configuration for the solid phase, denoted  [3]. The deformation gradient F′ from
[3]. The deformation gradient F′ from  to the current configuration Ω may be multiplicatively decomposed such that
to the current configuration Ω may be multiplicatively decomposed such that  where F0 is the deformation gradient from
where F0 is the deformation gradient from  to Ω0. Algorithms for finding
to Ω0. Algorithms for finding 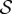 and F0 have been described, for example, by Pinsky et al. [3] and Gee et al. [38].
and F0 have been described, for example, by Pinsky et al. [3] and Gee et al. [38].
The Gibbs free energy density, measured per unit reference volume, of the electrolyte gel in Ω may be additively decomposed [24] into four components
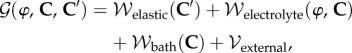 |
2.1 |
where 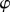 is the electrostatic potential and
is the electrostatic potential and 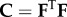 and
and  are right Cauchy–Green deformation tensors. The elastic strain energy density
are right Cauchy–Green deformation tensors. The elastic strain energy density  of the solid phase is associated with fibril stretching and matrix shearing and must be based on the three-dimensional collagen organization within the stroma. A detailed description of this term is deferred to §5.
of the solid phase is associated with fibril stretching and matrix shearing and must be based on the three-dimensional collagen organization within the stroma. A detailed description of this term is deferred to §5.
The mean-field approximation for the Helmholtz free energy density 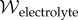 of a binary electrolyte in Ω is given by [25]
of a binary electrolyte in Ω is given by [25]
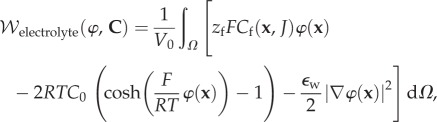 |
2.2 |
where Cf(x, J) is the fixed charge concentration in Ω and measured in moles per litre, zf = −1 is the fixed charge valence value, and R, T, F and 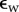 are the gas constant, temperature, Faraday constant and dielectric permittivity of the electrolyte solvent, respectively. The first term in the integrand measures the free energy of the fixed charge. The second term includes the excess mean concentration of the mobile ions at any point in the electrolyte compared to the bath and, after multiplication by RT, may be interpreted as the osmotic work of introducing excess ions into the neighbourhood of the fixed charges. The last term
are the gas constant, temperature, Faraday constant and dielectric permittivity of the electrolyte solvent, respectively. The first term in the integrand measures the free energy of the fixed charge. The second term includes the excess mean concentration of the mobile ions at any point in the electrolyte compared to the bath and, after multiplication by RT, may be interpreted as the osmotic work of introducing excess ions into the neighbourhood of the fixed charges. The last term 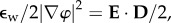 where
where 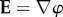 is the electric field and
is the electric field and 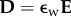 is the electric displacement, measures the dielectric free energy. Observe that
is the electric displacement, measures the dielectric free energy. Observe that 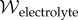 is defined to be the average electrostatic free energy density over the unit cell. The average free energy density of the bath is
is defined to be the average electrostatic free energy density over the unit cell. The average free energy density of the bath is
| 2.3 |
Finally, 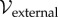 is the potential of the external loads, including body forces and boundary tractions. The list of terms appearing in the free energy expression (2.1) is not exhaustive; for example, the free energies of mixing and disassociation [39,40] can also be considered. However, osmotic energy is dominant in highly hydrated gels [2,39] and additional terms are neglected in the current theory.
is the potential of the external loads, including body forces and boundary tractions. The list of terms appearing in the free energy expression (2.1) is not exhaustive; for example, the free energies of mixing and disassociation [39,40] can also be considered. However, osmotic energy is dominant in highly hydrated gels [2,39] and additional terms are neglected in the current theory.
2.2. Thermodynamic equilibrium
The condition 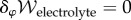 corresponds to electrostatic equilibrium and the Euler–Lagrange equation is the Poisson–Boltzmann equation for the electrostatic potential on Ω,
corresponds to electrostatic equilibrium and the Euler–Lagrange equation is the Poisson–Boltzmann equation for the electrostatic potential on Ω,
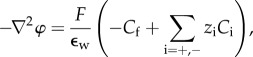 |
2.4 |
with ionic concentrations satisfying the Boltzmann distribution
| 2.5 |
The condition for mechanical equilibrium is found by setting 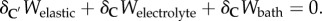 The variation of the elastic free energy
The variation of the elastic free energy  is taken with respect to C′ to incorporate the solid phase prestress, as discussed above. Noting that
is taken with respect to C′ to incorporate the solid phase prestress, as discussed above. Noting that 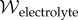 and
and  depend on C only through J, it follows that:
depend on C only through J, it follows that:
| 2.6 |
The Euler–Lagrange equation of this variational form on Ω is found to be
| 2.7 |
where the effective Cauchy stress σ is given in the standard manner by
| 2.8 |
in which 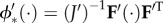 is the second-order push-forward operator from
is the second-order push-forward operator from  to Ω, with
to Ω, with  The unit cell osmotic pressure Pos in Ω is given by
The unit cell osmotic pressure Pos in Ω is given by
| 2.9 |
It is also seen that,
| 2.10 |
so that the electrolyte fluid pressure p is given by
| 2.11 |
2.3. Recovery of Donnan osmotic pressure
To illustrate the application of functional (2.2), consider the special case in which the reference fixed charge density 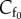 is uniform over the unit cell. The fixed charge density in the deformed configuration is then simply
is uniform over the unit cell. The fixed charge density in the deformed configuration is then simply
| 2.12 |
For this problem, the (Donnan) electrostatic potential, denoted  is uniform over the unit cell and be may be solved from (2.4) as
is uniform over the unit cell and be may be solved from (2.4) as
| 2.13 |
Observing that 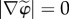 and introducing (2.13) into (2.2) and with use of (2.12), the Helmholtz electrostatic free energy density reduces to
and introducing (2.13) into (2.2) and with use of (2.12), the Helmholtz electrostatic free energy density reduces to
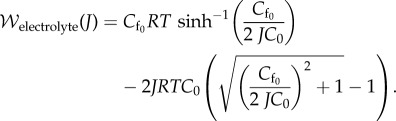 |
2.14 |
Employing the above equation in (2.9) yields
 |
2.15 |
which recovers the classical Donnan osmotic pressure [41,42].
3. Application to the human cornea
3.1. Fixed charge concentration
The predominant corneal GAGs are keratan sulfate, dermatan sulfate and chondroitin sulfate [9,10]. A detailed FACE2 [43] analysis reveals that only a portion of the available GAG monosaccharides is sulfated in the human cornea. It has been estimated [23] that the average ionization fraction (i.e. ratio of the charge per disaccharide unit to the number of groups that are ionizable) over all corneal GAGs is favg = 72.6%. If the unit cell within the normo-hydrated stroma contains Ng GAG chains with average contour length Lc and if b is the length of a monosaccharide unit, the total GAG fixed charge in the unit cell is
| 3.1 |
where e is the unit charge. The average fixed charge concentration, measured in mM units, is then
| 3.2 |
where 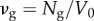 is the average GAG number density and F is the Faraday constant. Cavg has been measured indirectly [1,2], and the value used in this study is taken from [23] and given in table 1.
is the average GAG number density and F is the Faraday constant. Cavg has been measured indirectly [1,2], and the value used in this study is taken from [23] and given in table 1.
Table 1.
Parameters of the electrolyte and collagen organization models.
| parameter | value |
|---|---|
| ϕcol, volume fraction of the collagen fibrils (%) | 24.9a |
| ϕker, volume fraction of the keratocytes (%) | 11.2a |
| ϕcoat, volume fraction of the PG-dense coating (%) | 13.3a |
| Cavg, average charge density of the corneal stroma (mM) | 38.6b |
| λ, the charge partition parameter | 0.65c |
| Q+Q−, dimensionless parameter by the ionic pumping effect | 0.965d, 1.0e |
| μ, matrix shear modulus (kPa) | 5.0f |
| α, collagen fibril stiffness parameter (MPa) | 0.03f |
| β, collagen fibril stiffness parameter | 75.0f |
| p0, the hydrostatic pressure in bath solution (mmHg) | 0e, 15.0g |
| C0, bath concentration (mM) | 150, 0.00015h |
| T, temperature (K) | 298 |
bCalibrated value by Cheng & Pinsky [23].
cValue estimated by Cheng & Pinsky [23].
dValues calibrated for in vivo corneas (see §4).
eValue for ex vivo corneas.
fValues estimated by Petsche [44].
gValues for in vivo corneas.
hRepresentative of the ionic concentrations for aqueous humour and deionized water.
X-ray scattering studies under varying tissue hydration [45] suggest that some portion of stromal PGs form a charge-rich and water-binding PG-coating surrounding each collagen fibril. The radius of the coating has been measured to be rc = 18.25 nm and this radius is insensitive to hydration over a wide range. The existence of such a surface ultrastructure on the collagen fibrils is corroborated by image studies from [46,47]. In addition, a theoretical study on transparency by Twersky [48] proposed that collagen fibrils must be centred in a transparent coating and the coated fibrils occupy approximately 60% of the matrix volume, giving rc = 21.56 nm. Recent three-dimensional electron microscopy reconstructions of corneal collagen and GAG chains [9] also suggests that some GAG chains are in close association with the fibrils while the remaining GAG chains have random orientation in the interfibrillar fluid.
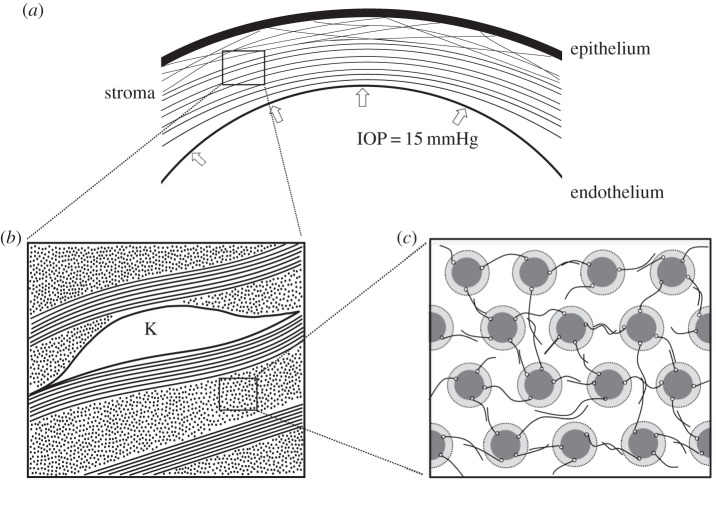
Given the regular arrangement of fibrils within a lamella, the nanoscale unit cell is selected to be of the form shown in figure 1c. It is a rectangular prism with cross-section as shown in figure 1c and with axial axis aligned with the collagen fibrils. Each fibril has a GAG-coating region. As described below, fixed charge concentrations in the unit cell will depend on various volume fractions, including the collagen and coating regions.
Figure 1.
(a) The cornea is a fibre-reinforced electrolyte gel that resists the IOP. The collagen lamellae mostly follow the corneal curvature except in the anterior region where the lamellae are interweaving and inclined relative to the corneal surface, and are seen to insert into Bowman's layer. (b) An illustration of the organization of the corneal stroma showing several lamellae and a keratocyte cell. Collagen fibrils within lamellae are found in parallel arrays following the fibril direction; keratocyte cells are found interspersed between adjacent lamellae. (c) Cross-section of an idealized unit cell representing the hexagonal collagen fibril lattice. The coating region around the central cylinder (representing the collagen fibril) and the irregular lines in between are illustrative of the coating GAGs and interfibrillar GAGs, respectively.
Let the unit cell current configuration Ω be partitioned into collagen fibril, coating and inter-coating domains (figure 1c) such that  and where
and where 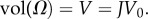 We seek expressions for the fixed charge density over the subregions of Ω as a function of dilation J. A parameter λ is used to partition the total unit cell GAG fixed charge Q into interfibrillar GAG fixed charge λQ and coating GAG fixed charge (1 − λ)Q. The value of λ = 0.65 has been estimated from swelling pressure measurements [23]. Note that because all GAG chains bind at one end to a collagen fibril, the interfibrillar GAG chains must pass through the GAG-coating region and their charges therefore combine in that region. The coating and interfibrillar GAGs are assumed to produce uniform charge concentration distributions over their respective domains.
We seek expressions for the fixed charge density over the subregions of Ω as a function of dilation J. A parameter λ is used to partition the total unit cell GAG fixed charge Q into interfibrillar GAG fixed charge λQ and coating GAG fixed charge (1 − λ)Q. The value of λ = 0.65 has been estimated from swelling pressure measurements [23]. Note that because all GAG chains bind at one end to a collagen fibril, the interfibrillar GAG chains must pass through the GAG-coating region and their charges therefore combine in that region. The coating and interfibrillar GAGs are assumed to produce uniform charge concentration distributions over their respective domains.
In determining the fixed charge concentrations as a function of macroscopic dilation J, account must be taken of keratocyte volumes which are assumed to exclude GAG fixed charge. Keratocytes are morphologically flattened fibroblasts that occur between lamellae [11]. They are considerably larger than the unit cell and their effect is therefore introduced in an average way (figure 1b). Stromal collagen and keratocyte volume fractions are denoted ϕcol and ϕker, respectively. Assuming that keratocyte cells dilate with the tissue dilation [49] and assuming collagen fibrils are non-swelling [1,50,51], the charge concentrations in the current unit cell configuration Ω are then given by Ccoat and Cinter in the coating and inter-coating regions, respectively, as
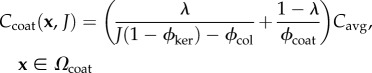 |
3.3 |
and
| 3.4 |
where ϕcoat is the volume fraction of the coating region. Note that the coating volume is independent of dilation J as required by the experimental observations of Fratzl & Daxer [45]. In (3.3) and (3.4), Cavg = Q/FV0 is the average charge concentration in the reference configuration Ω0 given by (3.2). It is easily verified that (3.3) and (3.4) conserve unit cell total fixed charge under any volume dilation J. Volume fraction values used in this study are provided in table 1.
Finally, assuming that keratocytes have the same mass density as water, the unit cell volume dilation J can be related to the stromal hydration Hw, defined as water weight per unit dry (collagen) weight, as follows:
| 3.5 |
where ρw = 1 g cm−3 and ρcol = 1.36 g cm−3 are the mass density of the water and dry collagen fibrils [51], respectively, and ϕr is the relative volume fraction of collagen molecules within the fibrils (which excludes the intrafibrillar bound water) [1,50]. The value of ϕr is estimated to be 0.75 such that the stromal hydration Hw is 3.2 at the normal condition J = 1 [1]. Therefore, equation (3.5) is rewritten as
| 3.6 |
The linearity is consistent with measurements [52,53]. It should also be noted that the estimated value fr = 0.75 is in very close agreement with the value 0.77 reported by Goodfellow et al. [50].
3.2. Active endothelial ion transport
The corneal endothelium is a 5 µm thin cellular monolayer located on the posterior surface of the cornea. It is permeable to water, metabolic species, including glucose and lactate ion, and other salt ions [11]. The endothelium regulates stromal hydration by providing active ion transport across the endothelium, from the stroma to the aqueous humour [1,12]. To allow sufficient generality, we model steady and independent anion and cation active transport and measure their effects (singularly or combined) on osmotic pressure by deriving a modified Boltzmann ion distribution for the stroma and the corresponding electrostatic free energy density for the in vivo cornea.
The endothelial layer is here modelled as an ideal membrane in which the action of the ion pumps is represented by independent active steady anion flux Ja− and cation flux Ja+, transporting ions out of the stroma and into the aqueous humour [21]. Because the endothelium is thin compared with the stroma, we will assume transport across the endothelium is one-dimensional. A coordinate z is introduced with z = z0 at the endothelium–aqueous interface and z = z* at the endothelium–stroma interface. At equilibrium, the net ionic fluxes J− and J+, which result from both active and passive transport, must vanish so that
| 3.7 |
where Li is the membrane permeability of ionic species i and Jai > 0 is the steady active ionic flux across the endothelium, from the stroma and into the aqueous humour. The ionic electrochemical potential μi is given by
| 3.8 |
where Mi(i = +,−) are the cation and anion atomic weights, respectively, 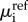 are the ionic chemical potentials at the reference state. Using (3.8) in (3.7) gives
are the ionic chemical potentials at the reference state. Using (3.8) in (3.7) gives
| 3.9 |
and adding both equations in (3.9) results in
| 3.10 |
Integrating (3.10) across the endothelium leads to
| 3.11 |
where 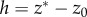 is the thickness of the endothelial layer,
is the thickness of the endothelial layer, 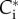 are the ionic concentrations at
are the ionic concentrations at 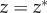 and C0 is the bath (i.e. aqueous humour) ionic concentration. Observe that the right-hand side of (3.11) is dimensionless as required. Defining,
and C0 is the bath (i.e. aqueous humour) ionic concentration. Observe that the right-hand side of (3.11) is dimensionless as required. Defining,
| 3.12 |
it follows that 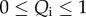 and from (3.11),
and from (3.11),
| 3.13 |
Finally, integrating (3.9) across the endothelium, and taking the electrostatic potential in the aqueous 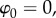 gives
gives
| 3.14 |
where 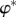 is the electrostatic potential at z = z*.
is the electrostatic potential at z = z*.
At thermodynamic equilibrium, the gradients of the ion electrochemical potentials vanish and 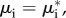 where μi is the electrochemical potential at any point in the stroma, and assuming that ionic concentrations are continuous across the endothelial–stroma interface, this condition implies that
where μi is the electrochemical potential at any point in the stroma, and assuming that ionic concentrations are continuous across the endothelial–stroma interface, this condition implies that
| 3.15 |
Introducing (3.14) into the above equation allows the stromal ionic concentrations Ci to be expressed as
| 3.16 |
which generalizes the Boltzmann distribution (2.5). From this result, it may be seen that at any point in the stroma, the ionic concentrations C+ and C− must satisfy the condition
| 3.17 |
3.3. Modified electrostatic free energy
Based on (3.16), a modified Poisson–Boltzmann equation for the electrostatic potential in the stroma is introduced as
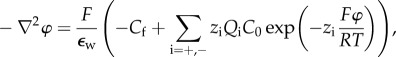 |
3.18 |
and the corresponding modified electrostatic free energy density is
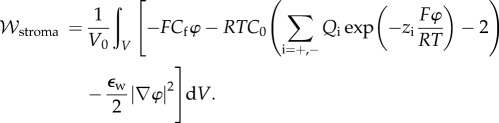 |
3.19 |
It may be verified that this functional is stationary at the solution of the modified Poisson–Boltzmann equation (3.18), i.e. 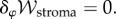 It may also be observed that if the active fluxes Ja− and Ja+ increase, the osmotic free energy, measured by the second term in the integrand, reduces.
It may also be observed that if the active fluxes Ja− and Ja+ increase, the osmotic free energy, measured by the second term in the integrand, reduces.
3.4. Analytical approximation for osmotic pressure
Recognizing that the fixed charge density Cf(x, J) is piecewise constant over the unit cell, and noting that the unit cell is large compared to the Debye length [23], we employ a piecewise constant Donnan potential 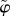 as an approximation for the exact electrostatic potential φ which is required to satisfy the Poisson–Boltzmann equation (3.18). By assumption
as an approximation for the exact electrostatic potential φ which is required to satisfy the Poisson–Boltzmann equation (3.18). By assumption 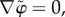 and equation (3.18) may be used to solve for the Donnan potential. Recalling that the fixed charge density
and equation (3.18) may be used to solve for the Donnan potential. Recalling that the fixed charge density 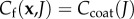 for
for 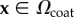 and
and 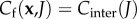 for
for 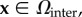 the Donnan potentials over Ωcoat and Ωinter are given by
the Donnan potentials over Ωcoat and Ωinter are given by
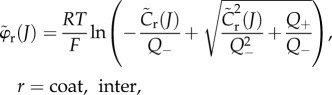 |
3.20 |
where the non-dimensional fixed charge concentrations 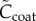 and
and 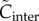 are defined by
are defined by
| 3.21 |
and where the fixed charge densities Ccoat(J) and Cinter(J) are given by (3.3) and (3.4), respectively.
Employing potentials (3.20), the Helmholtz free energy density (3.19) reduces to
 |
3.22 |
where 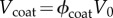 and
and 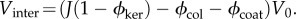 It can be shown that
It can be shown that 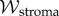 is convex in J. The unit cell osmotic pressure then follows from (2.9) as
is convex in J. The unit cell osmotic pressure then follows from (2.9) as 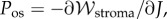 giving
giving
 |
3.23 |
in which a modified inverse hyperbolic sine function is introduced to simplify the expression
| 3.24 |
The osmotic compressibility, defined as 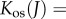
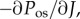 is the core function in the stroma electrolyte tensor
is the core function in the stroma electrolyte tensor  required for the finite-element tangent operator (see the electronic supplementary material for details) and is given by
required for the finite-element tangent operator (see the electronic supplementary material for details) and is given by
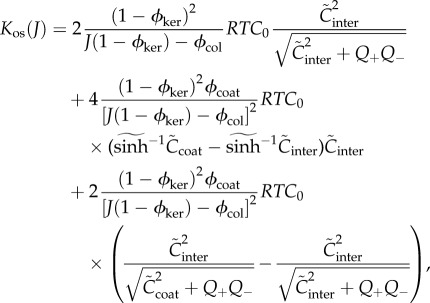 |
3.25 |
Equations (3.23)–(3.25) show that the osmotic pressure and compressibility are dictated by the combined ionic transport parameters Q+Q−. These parameters must be estimated indirectly since direct measurement is not currently feasible. This is considered in the following section. In the ex vivo cornea, no active transport occurs and Q+ = Q− = 1. This condition is employed in §6.1 to model in vitro free swelling and swelling pressure experiments on isolated stroma samples in ionic baths to provide evidence for the accuracy of the proposed model.
4. Model calibration from imbibition pressure
The presented model requires the determination of Q+Q−. From (3.12), it is found that
| 4.1 |
the above equation implies that any increase in permeability, described by Li, that is not balanced with a compensatory increase in ionic pump function, described by Jai, will result in stromal swelling and eventually clinical oedema [54]. The permeability and pumping rates have been estimated via transient swelling experiments [55,56], but these are not relevant for equilibrium conditions. Instead, we shall infer the value of Q+Q− from measurement of the imbibition pressure in rabbit.
Imbibition pressure is measured in the living cornea by inserting a saline-filled cannula into the stroma [57]. At equilibrium, a stable suction pressure is developed in the cannula and is referred to as the imbibition pressure Pimb. As the saline solution is in contact with the stroma in front of the cannula tip, Donnan equilibrium is reestablished locally [21] and equilibrium of water requires
| 4.2 |
where 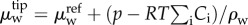 and
and 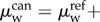
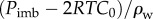 are the water electrochemical potentials of the stroma in front of the tip and of the saline solution in the cannula, respectively. The stromal fluid pressure is p and Pimb is the hydrostatic (imbibition) pressure measured in the cannula. Setting the local osmotic pressure difference
are the water electrochemical potentials of the stroma in front of the tip and of the saline solution in the cannula, respectively. The stromal fluid pressure is p and Pimb is the hydrostatic (imbibition) pressure measured in the cannula. Setting the local osmotic pressure difference 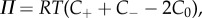 equation (4.2) implies
equation (4.2) implies
| 4.3 |
As noted by Bryant & McDonnell [21], П also corresponds to the ex vivo osmotic pressure because the canula and ionic bath have the same ionic concentration C0. In this case, equation (4.3) states that imbibition pressure is the difference between the stromal fluid pressure and the ex vivo osmotic pressure. However, direct experimental in vivo measurement of imbibition pressure in rabbit [57] indicated that the following relationship holds:
| 4.4 |
where is the IOP and is the independently measured swelling pressure in an ionic bath of concentration . As discussed in §1, for the stroma it can be safely assumed that swelling and osmotic pressure coincide and therefore equations (4.3) and (4.4) allow the conclusion that in rabbit the stromal fluid pressure is equal to the IOP, i.e. p = IOP at normal hydration. Furthermore, the measured Pimb was found to have insignificant variation throughout the cornea [57]. It is therefore reasonable to conclude that the fluid pressure is p = IOP everywhere in the normal in vivo rabbit cornea. Lacking any alternative, we shall take this condition to also hold in the human cornea.3
Recalling that equilibrium fluid pressure in the cornea is given by (2.11), and setting p0 = IOP, it follows that:
| 4.5 |
However, by the above argument based on imbibition pressure measurements, we must set p = IOP. In this case, equation (4.5) implies that in the normal in vivo cornea, 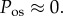
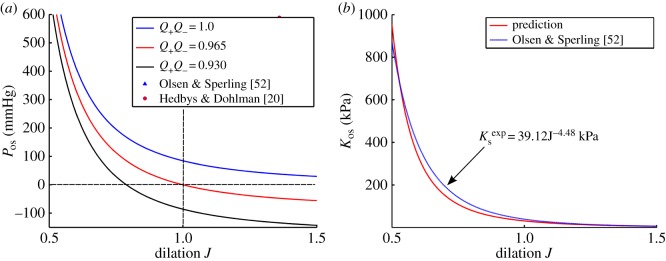
The condition 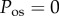 is used to estimate Q+Q− as follows. Figure 2 depicts the variation of Pos with Q+Q− at normal hydration, determined from (3.23). A nearly linear relationship between Pos and Q+Q− is found. The curve indicates that stromal osmotic pressure reduces monotonically with increasing active ionic flux values. A calibrated value of Q+Q− = 0.965 is found for the normo-hydrated in vivo cornea. As will be discussed in §6.2.1, the positive slope of figure 2 is a necessary requirement for hydration regulation.
is used to estimate Q+Q− as follows. Figure 2 depicts the variation of Pos with Q+Q− at normal hydration, determined from (3.23). A nearly linear relationship between Pos and Q+Q− is found. The curve indicates that stromal osmotic pressure reduces monotonically with increasing active ionic flux values. A calibrated value of Q+Q− = 0.965 is found for the normo-hydrated in vivo cornea. As will be discussed in §6.2.1, the positive slope of figure 2 is a necessary requirement for hydration regulation.
Figure 2.
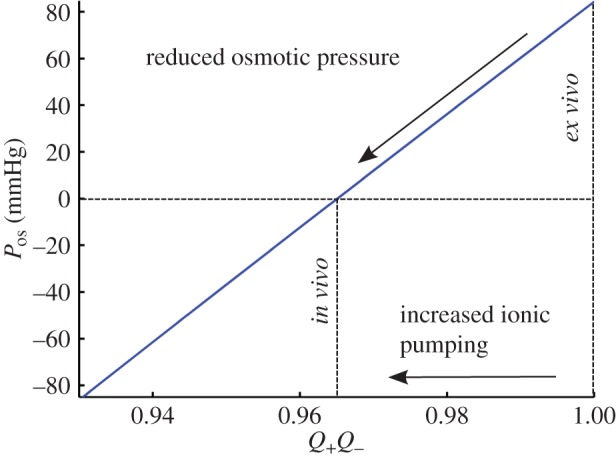
The in vivo osmotic pressure Pos at normal hydration (i.e. J = 1) as a function of the ionic transport parameter Q+Q−. The osmotic pressure Pos reduces monotonically with increasing active ionic flux values (decreasing Q+Q−). The calibrated value of Q+Q− is found to be 0.965 for the normo-hydrated in vivo cornea. (Online version in colour.)
5. Collagen organization and stromal elasticity
5.1. Angular averaging
In this section, we review the modelling of the stromal fibres which contribute to the elastic strain energy density  appearing in (2.1). It is assumed that
appearing in (2.1). It is assumed that  is additively decomposed into an anisotropic part describing the strain energy density of the three-dimensional collagen fibre network and an isotropic part that gives a simple phenomenological description of the small background shear stiffness of the extracellular matrix such that
is additively decomposed into an anisotropic part describing the strain energy density of the three-dimensional collagen fibre network and an isotropic part that gives a simple phenomenological description of the small background shear stiffness of the extracellular matrix such that
| 5.1 |
Recall from §2.1, that  describes the deformation measured from the stress-free configuration
describes the deformation measured from the stress-free configuration 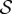 , and F′ is the deformation gradient from the stress-free configuration
, and F′ is the deformation gradient from the stress-free configuration 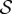 to the current configuration Ω. The invariant
to the current configuration Ω. The invariant 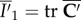 is used to describe an isotropic contribution based on the unimodular right Cauchy–Green deformation tensor
is used to describe an isotropic contribution based on the unimodular right Cauchy–Green deformation tensor 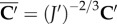 and
and 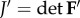 [58].
[58].
The invariant 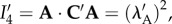 which measures the stretch along a fibre with direction A, is used to describe elastic anisotropy. It is important to note that
which measures the stretch along a fibre with direction A, is used to describe elastic anisotropy. It is important to note that 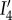 depends on the dilation through its dependence on C′, allowing the description of fibre stretching resulting from electrolyte swelling. The form of
depends on the dilation through its dependence on C′, allowing the description of fibre stretching resulting from electrolyte swelling. The form of 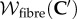 is based on the use of angular integration [3,59] such that
is based on the use of angular integration [3,59] such that
| 5.2 |
| 5.3 |
where ω is the unit sphere, ρ(X, A) is the distribution of fibre directions, 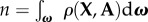 is the normalization and the fibre stretching energy
is the normalization and the fibre stretching energy  is described below.
is described below.
The function ρ(X, A) may be based on fibre orientation information obtained from X-ray diffraction and SHG imaging as described in [6]. A fibre direction A can be expressed in spherical coordinates with azimuthal angle 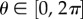 and inclination angle
and inclination angle  As the anterior and posterior surfaces have different curvatures, a local coordinate system is introduced that varies pointwise through the corneal thickness and matches both the anterior and posterior curvatures at those limits; details can be found in [6]. Every material point within the cornea has a unique angular distribution with no perfect symmetry over quadrants. We set
As the anterior and posterior surfaces have different curvatures, a local coordinate system is introduced that varies pointwise through the corneal thickness and matches both the anterior and posterior curvatures at those limits; details can be found in [6]. Every material point within the cornea has a unique angular distribution with no perfect symmetry over quadrants. We set 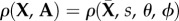 where
where 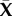 is a point on the cornea anterior surface,
is a point on the cornea anterior surface, 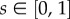 is the non-dimensional corneal depth measured from the anterior surface, and assume,
is the non-dimensional corneal depth measured from the anterior surface, and assume,
| 5.4 |
where 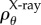 is based on X-ray diffraction data and
is based on X-ray diffraction data and 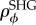 is based on SHG imaging. The current model generalizes the approach in [6] in two respects:
is based on SHG imaging. The current model generalizes the approach in [6] in two respects: 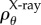 incorporates depth-dependence of preferred fibre directions, and
incorporates depth-dependence of preferred fibre directions, and 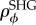 incorporates direct statistical measurement of inclination data. These are briefly summarized below.
incorporates direct statistical measurement of inclination data. These are briefly summarized below.
5.2. Azimuthal distribution 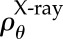
In [3], analytical distribution functions were introduced to represent data from X-ray diffraction experiments by Meek et al. [60]. Following [6], we have eliminated the need to introduce analytical distribution functions and directly employ the raw X-ray data. These data provide scattering intensities versus orientation on a discrete grid of points over the cornea's anterior surface. At any point in the cornea, the orientation distribution is obtained by interpolating the X-ray diffraction measurements taken at the nearest four grid points using bilinear Lagrange functions. This procedure ensures that any subtle variations in distributions found in the X-ray data are faithfully reproduced in the model. At any scan point 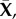 the X-ray scattering data
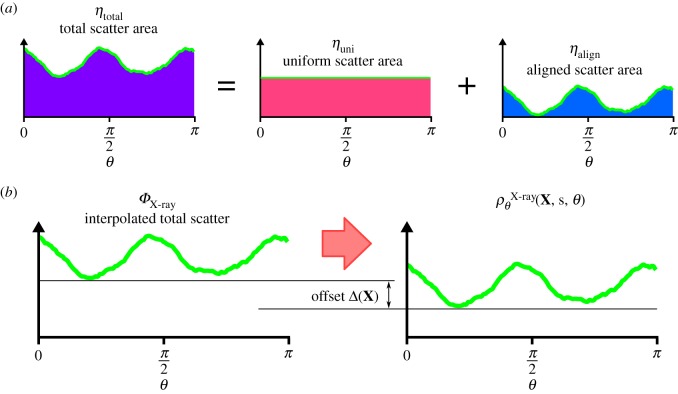
the X-ray scattering data 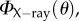 which corresponds to a measurement through the entire thickness, can be analysed in the following way. The total scatter ηtotal is the area under the curve,
which corresponds to a measurement through the entire thickness, can be analysed in the following way. The total scatter ηtotal is the area under the curve,
| 5.5 |
This can be additively decomposed into aligned and uniform parts 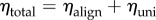 where
where  and
and  (figure 3a).
(figure 3a).
Figure 3.
(a) The X-ray scattering intensity can be additively decomposed into uniform and aligned parts. (b) The distribution interpolated from surrounding scan points is offset based on the depth within the stroma to increase alignment in the posterior.
The percentage of all aligned fibres 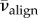 is then
is then
| 5.6 |
The depth-dependence of aligned fibres may be introduced based on the work of Abahussin et al. [33], who performed X-ray diffraction studies on isolated third-thickness stromal samples and characterized the variation of scattering intensity with depth at the corneal centre. They found the average percentage of total fibres exhibiting preferred azimuthal directions valign was 22%, 31% and 42% in the anterior, central and posterior thirds, respectively. However, depth-dependent alignment was not measured at the cornea's periphery where there is significantly more alignment. A linear best-fit of alignment as a function of depth for the data is 0.3s+1/6 with R2 = 0.997. Based on the slope of this function, a first approximation for the depth-dependence of aligned fibres is  For the central full-thickness X-ray scans in the current database of implemented X-ray diffraction data (four donor corneas, including a right and left cornea from the same donor), we find
For the central full-thickness X-ray scans in the current database of implemented X-ray diffraction data (four donor corneas, including a right and left cornea from the same donor), we find 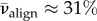 for all subjects, which corresponds well to the mid-thickness value measured by Abahussin et al. [33]. Thus, to evaluate δ we set
for all subjects, which corresponds well to the mid-thickness value measured by Abahussin et al. [33]. Thus, to evaluate δ we set 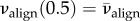 which gives
which gives 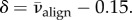 Then,
Then,
| 5.7 |
defines the fraction of aligned fibres based on in-plane position 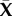 and depth s (figure 4).
and depth s (figure 4).
Figure 4.
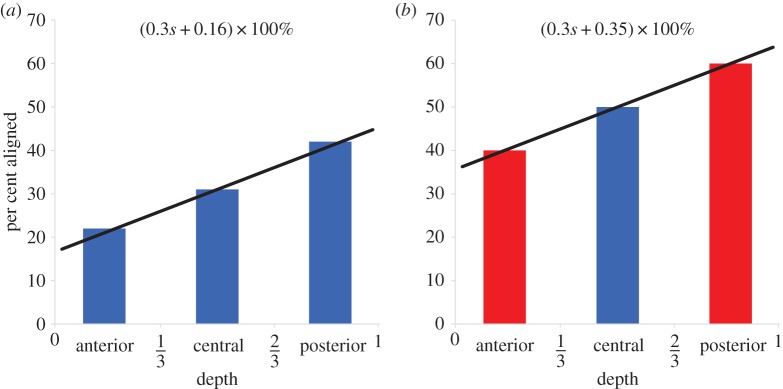
Data from [33] of alignment percentage for each third in the central cornea is fit with a linear curve (a). The depth-dependence of alignment is approximated from a full-thickness X-ray scan near the limbus by fitting with a line of the same slope (b). (Online version in colour.)
In order to generate a depth-dependent distribution, the full thickness distribution ηtotal is augmented by Δ(s) such that 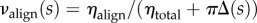 (figure 3b). Solving for Δ(s) gives
(figure 3b). Solving for Δ(s) gives
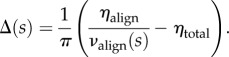 |
5.8 |
The final distribution will then be,
Incorporating the X-ray diffraction data in this manner describes the depth-dependent anisotropy resulting from the well-documented S–I and N–T preferred directions of lamellae in the vicinity of the corneal vertex as well as the circumferentially preferred orientations at the limbus [44].
5.3. Inclination distribution 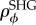
The presence of inclined fibres has been well-documented with macroscale SHG imaging [34–36]. However, because the angular distribution of inclined fibres was missing, an approximation was proposed by Petsche & Pinsky [6] based on inspection of the macroscale images and calibration based on the depth-dependence of shear properties. Recently, Winkler et al. [37] have processed SHG images to detect and quantify the spatial distribution of inclined lamellae and their distribution is employed in this study. Winkler et al. [37] analysed half cross-sectional image stacks to measure the inclination angle of every lamella in the anterior half of the stroma. Lamellae were binned based on depth, corneal quadrant and radial position. For each bin, a histogram of fibre inclination was fit to a Gaussian distribution for the full-width at half-maximum (FWHM), with the mean fixed at 0° from the plane tangent to the anterior surface (figure 5).
Figure 5.
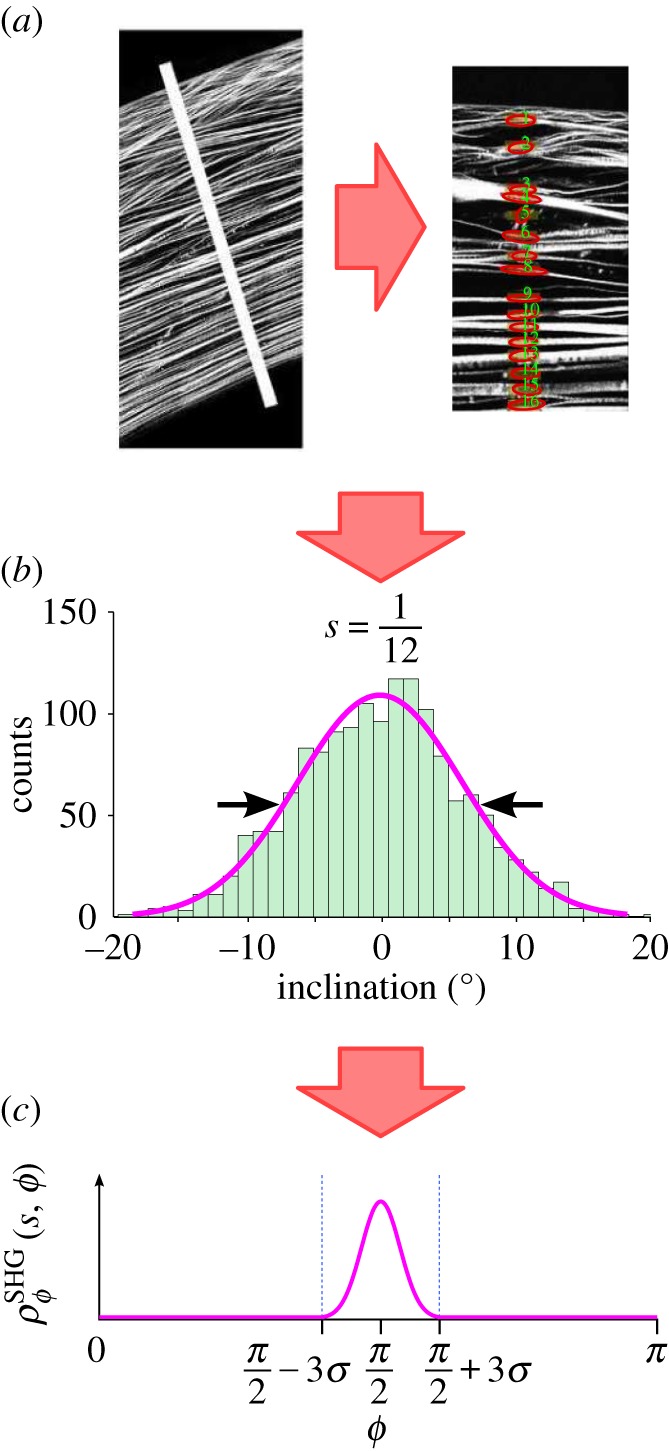
SHG images were processed by Winkler et al. [37] to obtain quantitative depth-dependent inclination distributions. Images were scanned for lamella direction by first isolating a narrow section of the image and rotating it so the anterior surface was horizontal. Then the image processing algorithm searched for fibres by scanning through the depth. Data were processed to discard artefacts and then plotted in a histogram for each depth. A Gaussian distribution was fit to the data to define the FWHM value for each depth and radial position. The FWHM value was then used to define the inclination distribution  (Online version in colour.)
(Online version in colour.)
They found no significant differences between quadrants and radial position but measured a significant variation with depth. On the anterior half of the cornea, where analysis was performed, a linear decrease of FWHM was found. In order to complete the data through full thickness, it is assumed that FWHM extrapolates linearly to zero at the posterior surface. A best-fit line that forces no inclined lamellae at s = 1 (R2 = 0.87) was used to find FWHM as a function of depth (figure 6).
Figure 6.
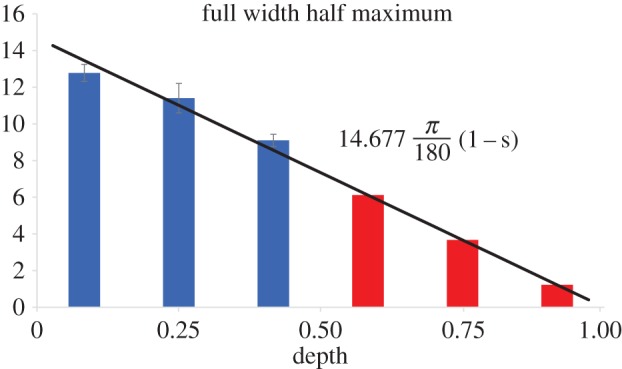
FWHM values were estimated in the posterior half by fitting a linear trend line that intersected 0° at the posterior surface. (Online version in colour.)
Using the one-to-one relationship between FWHM and the standard deviation σ this gives
| 5.9 |
The inclination distribution therefore takes the form of a Gaussian
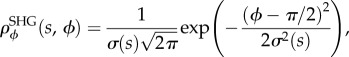 |
5.10 |
where the mean is π/2 because ϕ is the zenith angle [44].
5.4. Fibril and shear elasticity
The term  in (5.3) represents the strain energy density resulting from fibril stretching. In tension, collagenous tissues are characterized by a J-shaped stress–strain curve resulting from unkinking of collagen molecules at low strains followed by the stretching of stiffer covalent bonds at high strains. However, they exhibit negligible compressive stiffness due to buckling effects. To capture this behaviour, Markert et al. [61] introduced a strain energy function of the form
in (5.3) represents the strain energy density resulting from fibril stretching. In tension, collagenous tissues are characterized by a J-shaped stress–strain curve resulting from unkinking of collagen molecules at low strains followed by the stretching of stiffer covalent bonds at high strains. However, they exhibit negligible compressive stiffness due to buckling effects. To capture this behaviour, Markert et al. [61] introduced a strain energy function of the form
| 5.11 |
This model has been applied to the cornea by Studer et al. [5]. The model parameters α and β have been identified using corneal inflation experimental data and optimal values found to be α = 30 kPa and β = 75 [44]. These values satisfy the constraints on α and β given by Markert et al. [61] and render 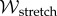 convex in
convex in 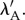
The matrix shear term is modelled as simply as possible and is assumed to be
| 5.12 |
where μ = 5 kPa is the matrix shear modulus which has been measured experimentally by Petsche et al. [62] and Sondergaard et al. [63].
Finally, the elasticity tensor 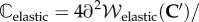
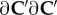 and electrolyte tensor
and electrolyte tensor 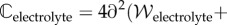
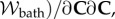 needed for the finite-element tangent operator, are described in the electronic supplementary material.
needed for the finite-element tangent operator, are described in the electronic supplementary material.
5.5. Boundary conditions
The in vivo cornea is loaded by the IOP at the endothelial–aqueous interface (figure 1a). The anterior stroma is sealed by the epithelium, which is a stratified squamous cellular membrane that is composed of four to six cellular layers and approximately 50 µm in thickness. In contrast to the endothelium, epithelial cells exhibit tight junctions and the epithelium is essentially impermeable to water and ions under normal conditions [11]. As the mechanical stiffness of both epithelium and endothelium is insignificant compared to the stroma [11,64], the mechanical roles of these bounding layers are reasonably ignored. Therefore, for modelling purposes, the IOP is assumed to act directly on the stroma and the posterior boundary condition is written as
| 5.13 |
where σ is the effective Cauchy stress, p is the stromal fluid pressure, p0 = IOP is the hydrostatic pressure in the aqueous humour and n is the normal unit vector. At the epithelium, no external pressure is applied and then
| 5.14 |
A full interpretation of the above boundary conditions is extremely important since it provides insight into specific corneal structural function, and in particular, the implied necessity of fibre inclination in the anterior (such that 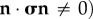 needed to balance the stromal fluid pressure at the anterior corneal surface. A detailed discussion is presented in §7.
needed to balance the stromal fluid pressure at the anterior corneal surface. A detailed discussion is presented in §7.
6. Results
6.1. Swelling in the ex vivo stroma
6.1.1. Confined and unconfined swelling pressure
Finite-element predictions for ex vivo swelling pressure were compared to experimental measurements on a sample of isolated corneal stroma immersed in a bath solution (figure 7a). Stromal swelling pressure is the mechanical pressure required to maintain a specified thickness of the tissue at a specified bath ionic concentration. Ex vivo conditions (no active ion transport) are modelled by setting the ionic transport function Q+Q− = 1 in equation (3.23). The stroma sample was modelled as a 7 mm diameter cylinder with an initial thickness of 0.5 mm and immersed in an ionic bath of concentration C0 = 150 mM [20,52]. The finite-element mesh employed 2500, 27-node (tri-quadratic) hexahedral elements. Fixed displacement boundary conditions were applied on the bottom surface and prescribed transverse displacements were applied to the top surface to model changes in sample thickness. The equilibrium swelling pressure at any sample thickness t is computed as the total reaction force on the top surface divided by the surface area. In order to model confined [20] and unconfined [52] experimental measurements, edge boundary conditions were taken as zero radial displacement or free, respectively (figure 7a). Predictions are compared with experimental measurements [20,52] in figure 7b. The results show good agreement for the full range of experimental measurements with t varying from 0.75 mm (swollen) to 0.2 mm (highly compressed), with the corresponding swelling pressure values varying over two orders of magnitude. Swelling pressure predictions for the confined and unconfined cases are nearly identical; this is expected because lateral expansion of the sample is restricted by the collagen fibrils and, as a consequence, stromal volume change in these tests is predicted to be associated with changes in sample thickness [1].
Figure 7.
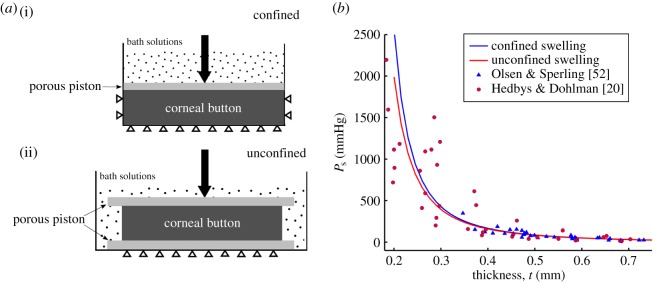
(a) Illustrations of confined (i) and unconfined (ii) swelling pressure experiments for ex vivo cornea, respectively [20,52]. In both experiments, a mechanical pressure needs to be applied in the transverse direction to maintain a specified thickness. (b) The predicted confined and unconfined swelling pressure Ps versus corneal thickness t and their comparison with experimental measurements [20,52] over thickness range  The swelling pressure data have been extracted from the original papers by free graphics software Plot Digitizer, and the hydration data H from [20] have been transformed to thickness t by the observed linear relation H = 7.09t − 0.44 [52,53]. (Online version in colour.)
The swelling pressure data have been extracted from the original papers by free graphics software Plot Digitizer, and the hydration data H from [20] have been transformed to thickness t by the observed linear relation H = 7.09t − 0.44 [52,53]. (Online version in colour.)
6.1.2. Free swelling of a stromal sample
The free swelling of the above described cylindrical sample of ex vivo stroma is modelled to investigate the role of the collagen organization in mediating the swelling response. Results for edge-confined free swelling of the stroma sample are shown in figure 8a (solid curve), which depicts the ratio of swollen thickness to original thickness (swelling ratio) versus bath ionic concentration C0. For dilute bath solutions, the model predicts that the stroma sample will swell to approximately four times its original thickness, which may be compared to experimental measurements in de-ionized water [28,29] in which human corneas were observed to swell to approximately three times their original thickness. For concentrated bath solutions, the abundance of ions results in ionic shielding of the fixed charges, reduction in osmotic pressure and minimal swelling. Both limiting states are captured by the swelling predictions.
Figure 8.
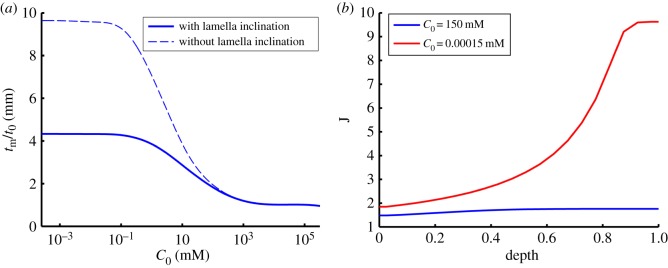
(a) The effect of lamella inclination on stromal swelling ratio during free swelling of the sample in a bath ionic concentration range of 10−3–105 mM. At each value of C0, the swelling ratio is calculated as the ratio of the swollen thickness tm to the original thickness t0 at which the swelling pressure is zero. (b) Predicted depth-dependent swelling at physiological bath concentration (C0 = 150 mM) and deionized water (C0 = 150 × 10−6 mM). (Online version in colour.)
It has been observed in free swelling experiments that the anterior third of the stroma remains virtually unswollen with most swelling taking place in the deeper stroma [28,29]. We used the model to investigate the depth-dependence of stromal swelling at two bath ionic concentrations C0 = 150 mM and 150 × 10−6 mM, corresponding to the physiological state and de-ionized water, respectively. The results are presented in figure 8b which depicts the profile of local volume dilation J across the depth of the stroma (J = 1 corresponding to the normo-hydrated state). At C0 = 150 mM, the local volume dilation varies from 1.5 in the anterior to 1.8 in the posterior. Massive swelling occurs when C0 = 150 × 10−6 mM and volume dilation varies from 1.8 in the anterior to 10.0 in the posterior. The highly non-uniform swelling across the corneal thickness agrees qualitatively with experimental observations [28,29]. Comparison of the two results in figure 8b indicates that the stromal anterior third thickness is predicted to maintain its thickness for any bath concentration, indicating the rigidity of this region with respect to extreme hydration changes.
A theoretical study was also undertaken to confirm the importance of lamellae inclination with respect to anterior stromal rigidity. The above study was repeated while constraining lamellae to have zero inclination. This is accomplished by replacing the Gaussian inclination distribution given by (5.10) with 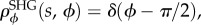 where δ is the Dirac delta. In this case, all lamellae throughout the corneal depth will have zero inclination. As shown in figure 8a (dashed curve), for dilute bath solutions the stroma sample now swells to almost 10 times its original thickness. In fact, the only mechanical constraint preventing infinite swelling is the work done by the matrix shear stiffness which bounds the swelling. As expected, the model still predicts little swelling when the bath ionic concentration is high.
where δ is the Dirac delta. In this case, all lamellae throughout the corneal depth will have zero inclination. As shown in figure 8a (dashed curve), for dilute bath solutions the stroma sample now swells to almost 10 times its original thickness. In fact, the only mechanical constraint preventing infinite swelling is the work done by the matrix shear stiffness which bounds the swelling. As expected, the model still predicts little swelling when the bath ionic concentration is high.
6.2. Swelling in the in vivo cornea
6.2.1. Effect of active endothelial ion transport
Recall that the active endothelial ionic transport term (equation (4.1)) satisfies 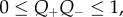 with Q+Q− = 1 corresponding to no active transport and Q+Q− = 0.965 corresponding to normal active transport in the in vivo cornea. Predicted values of osmotic pressure Pos (equation (3.23)) are plotted in figure 9a for these two values of the ionic transport function and also for Q+Q− = 0.930 which corresponds to hyper-active ionic transport. Osmotic pressure is reduced with reducing values of Q+Q−. For Q+Q− = 1, the osmotic pressure is positive for all dilation J and the tissue will tend to swell. For the normal in vivo cornea with Q+Q− = 0.965, it is seen that a reduction in dilation from the physiological condition (J = 1) will result in positive stromal osmotic pressure which will produce a tendency to swell. On the other hand, an increase in dilation will result in negative stromal osmotic pressure and produce a tendency to de-swell. Consider the curve for the hyper-active Q+Q− = 0.930. In this case, positive or negative deviations in dilation from J = 1 will always result in negative osmotic pressure and produce a tendency to de-swell. These conditions illustrate the hydration regulation mechanism of active endothelial ion transport. Increasing active transport shifts the osmotic pressure–dilation curve downwards in figure 9a. Predicted values of osmotic compressibility Kos (equation (3.25)) versus dilation J are shown in figure 9b for no active transport. Values closely match measurements reported in [52].
with Q+Q− = 1 corresponding to no active transport and Q+Q− = 0.965 corresponding to normal active transport in the in vivo cornea. Predicted values of osmotic pressure Pos (equation (3.23)) are plotted in figure 9a for these two values of the ionic transport function and also for Q+Q− = 0.930 which corresponds to hyper-active ionic transport. Osmotic pressure is reduced with reducing values of Q+Q−. For Q+Q− = 1, the osmotic pressure is positive for all dilation J and the tissue will tend to swell. For the normal in vivo cornea with Q+Q− = 0.965, it is seen that a reduction in dilation from the physiological condition (J = 1) will result in positive stromal osmotic pressure which will produce a tendency to swell. On the other hand, an increase in dilation will result in negative stromal osmotic pressure and produce a tendency to de-swell. Consider the curve for the hyper-active Q+Q− = 0.930. In this case, positive or negative deviations in dilation from J = 1 will always result in negative osmotic pressure and produce a tendency to de-swell. These conditions illustrate the hydration regulation mechanism of active endothelial ion transport. Increasing active transport shifts the osmotic pressure–dilation curve downwards in figure 9a. Predicted values of osmotic compressibility Kos (equation (3.25)) versus dilation J are shown in figure 9b for no active transport. Values closely match measurements reported in [52].
Figure 9.
(a) The computed osmotic pressure Pos versus volume dilation J for three representative values of Q+Q− and comparison with swelling pressure measurements [20,52]. (b) The predicted osmotic compressibility Kos versus volume dilation J and its comparison with experimental measurements [52]. The measured modulus is given by 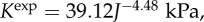 which is computed by the power law fit function for swelling pressure
which is computed by the power law fit function for swelling pressure 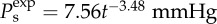 from [52]. (Online version in colour.)
from [52]. (Online version in colour.)
6.2.2. Swelling of a cornea with Fuchs' dystrophy
Fuchs' dystrophy is usually characterized by morphological changes in endothelial cells or by an accelerated loss of endothelial cells [11,54]. In this situation, the cornea will swell due to increasing endothelial permeability, decreasing active ion flux, or both mechanisms simultaneously. The effect of these pathological changes on stromal ionic concentrations is described by the ionic transport function Q+Q− (equation (4.1)). Because the absolute values of endothelial ionic permeability L+ and L− and active ionic fluxes Ja+ and Ja− have limited clinical significance, swelling effects due to relative changes in these parameters are considered. For simplicity and without loss of generality, we consider active anion transport only and set Q+ = 1. Let  and
and 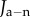 be the anion membrane permeability and active anion flux rate of the normal cornea, respectively, and consider pathological increases in ionic permeability such that
be the anion membrane permeability and active anion flux rate of the normal cornea, respectively, and consider pathological increases in ionic permeability such that  and reductions active flux such that
and reductions active flux such that 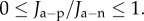 For any value of
For any value of  and
and 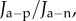 the value of
the value of 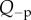 can be found through equation (4.1)
can be found through equation (4.1)
| 6.1 |
where 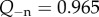 is the calibrated value for normal cornea as determined in §4.
is the calibrated value for normal cornea as determined in §4.
The cornea was modelled geometrically with a central thickness of 520 µm and the anterior and posterior surfaces defined as spherical surfaces with radii of 7.87 and 6.7 mm, respectively. Part of the sclera was also modelled with anterior and posterior surfaces defined with radii of 12.00 and 11.38 mm, respectively. Because the sclera swells much less than the cornea [8], its bulk behaviour was modelled as a (non-swelling) compressible neo-Hookean material, as described by Petsche & Pinsky [6], and the limbal corneal collagen fibre elasticity was extended to the scleral tissue. At the junction of the cornea and sclera, there is an abrupt interface of swelling and non-swelling tissue, which should be replaced by use of a gradual transition (although we have not done so). Homogeneous Dirichlet boundary conditions are applied to fix the periphery of the scleral section. The solution proceeds by first solving for the normal cornea4 with 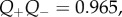 and then solving a sequence of problems in which
and then solving a sequence of problems in which 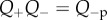 is systematically varied to simulate changes in endothelial permeability and active anionic flux.
is systematically varied to simulate changes in endothelial permeability and active anionic flux.
Figure 10a shows the predicted swollen central corneal thickness (CCT) for endothelial ionic permeability increased with 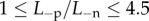 and with normal active ionic flux
and with normal active ionic flux 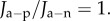 Figure 10b shows the predicted swollen CCT for active ionic flux rate reduced up to a factor of 0.2, so that
Figure 10b shows the predicted swollen CCT for active ionic flux rate reduced up to a factor of 0.2, so that 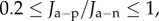 with normal anionic permeability
with normal anionic permeability  In both cases, the cornea swells. The predicted maximum swollen CCT of approximately 690 µm lies in the range of clinical observation (500–1000 m) measured for patients with Fuchs' dystrophy [30].
In both cases, the cornea swells. The predicted maximum swollen CCT of approximately 690 µm lies in the range of clinical observation (500–1000 m) measured for patients with Fuchs' dystrophy [30].
Figure 10.
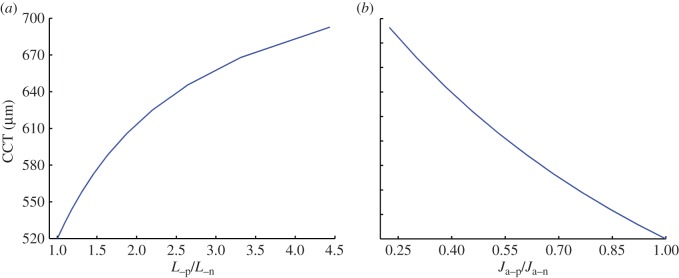
The predicted swollen CCT in Fuchs' dystrophy when (a) the endothelial ionic permeability is increased up to a factor of 4.5 (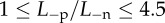 ) with normal active ionic flux (
) with normal active ionic flux (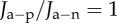 ) and (b) the active ionic flux rate is reduced up to a factor of 0.2 (
) and (b) the active ionic flux rate is reduced up to a factor of 0.2 (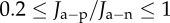 ) with normal anionic permeability (
) with normal anionic permeability (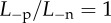 ). (Online version in colour.)
). (Online version in colour.)
The model predicts that at any value of the swollen CCT, the anterior surface deforms much less than the posterior surface, with swelling concentrated in the posterior region. This prediction agrees with the clinical observation of Brunette et al. [30] that the anterior surface of the cornea is nearly normal among patients with Fuchs' dystrophy, whereas the posterior surface shows significant change. Figure 11 provides a fringe plot of axial (vertical) displacements due to swelling resulting from a 78% reduction in active ion transport,  The results again suggest the stability of the anterior surface with respect to swelling resulting from the presence of inclined lamellae.
The results again suggest the stability of the anterior surface with respect to swelling resulting from the presence of inclined lamellae.
Figure 11.
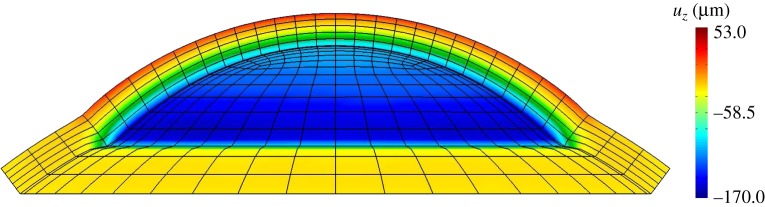
Fringe plot of the vertical displacement field uz at  for a cornea with Fuchs' dystrophy. The anterior surface of the cornea deforms much less than that of the posterior surface. It is noted that the sclera elements are distorted at the limbus. This artefact is due to the abrupt transition from swelling to non-swelling tissue, and may be removed by defining a transition zone between the cornea and sclera.
for a cornea with Fuchs' dystrophy. The anterior surface of the cornea deforms much less than that of the posterior surface. It is noted that the sclera elements are distorted at the limbus. This artefact is due to the abrupt transition from swelling to non-swelling tissue, and may be removed by defining a transition zone between the cornea and sclera.
6.2.3. Swelling due to changes in intraocular pressure
An example of corneal oedema with an intact and functional endothelium occurs in acute glaucoma. In this case, elevated IOP combined with normal stromal osmotic pressure can create an increase in corneal thickness [65]. Likewise, corneal thickening is reported in patients with ocular hypertension [66,67]. We emphasize that we are considering the equilibrium solution that is achieved after long-time exposure to increased IOP. It is very likely that the short-time non-equilibrium response of the cornea is that of elastic thinning. In fact, if we model an increase in IOP and do not allow the fluid pressure to equilibrate, the model does predict small corneal thinning. However, persistent elevated IOP produces an increased pressure gradient which drives more fluid across the endothelium, creating oedema of the stroma (and epithelium), as seen in acute angle-closure glaucoma [65,68]. This is undoubtedly a simplified view of a complex problem but it is supported by predictions from the current model.
There are two competing mechanisms with regard to corneal swelling. The increased (mechanical) IOP loading at the endothelial–aqueous humour interface acts to thin the cornea, but an increase in stromal fluid pressure produces a countering ‘inflation’ effect. At Bowman's layer, the stromal fluid pressure must be balanced by forces in the inclined lamellae, because there is no external pressure loading at that boundary which can balance the stromal fluid pressure (figure 12). As shown in figure 13 (solid curve), at 44.5 mmHg, the CCT is predicted to swell by approximately 15 µm, which is in agreement with clinical data [65] in which the CCT was measured to be 575 and 593 µm for a group of patients who had normal IOP (average 15.8 mmHg) in one eye and high IOP (average 44.5 mmHg) in the other eye, giving a thickness difference of 18 µm. In the simulation of increasing IOP, the active ionic pumping was maintained at a normal level throughout. It is important to note that other measurements, performed on eyes with retinal detachment or non-human species, or performed within a relatively short timescale, have concluded an opposite trend [69–71].
Figure 12.
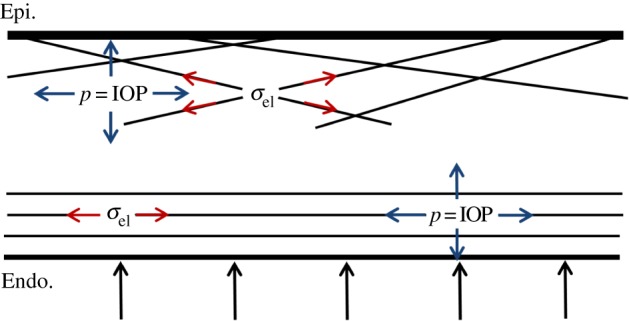
Illustration of the stress state in the normal living cornea. A nearly uniform fluid pressure exists in the stroma at the magnitude of the IOP. The in-plane lamellae are responsible for resisting such pressure in the lateral direction. In the transverse direction, the applied IOP at the posterior surface balances with the fluid pressure. At the anterior surface, the inclined lamellae insert into Bowman's layer and act as anchors resisting the stromal fluid pressure applied to the epithelium. (Online version in colour.)
Figure 13.
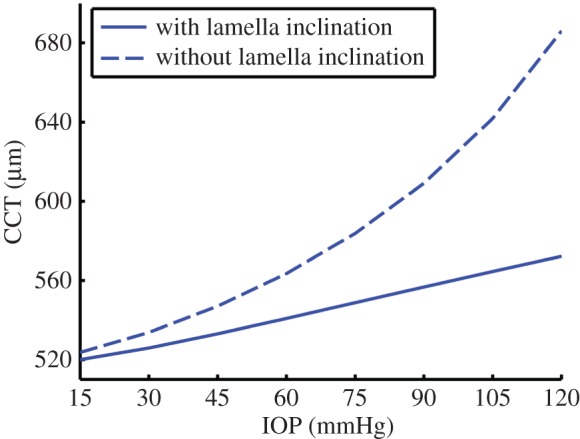
The predicted CCT response to increased IOP from 15 to 120 mmHg. The absence of inclined lamellae results in a much more swollen cornea. (Online version in colour.)
To illustrate the importance of lamella inclination in resisting the IOP, the cornea was reanalysed with lamella inclination constrained to be zero, as described in §6.1.2. In this case, the response shown in figure 13 (dashed curve) includes a significant further increase in swelling.
6.2.4. Biomechanical behaviour after LASIK surgery
LASIK (laser in-situ keratomileusis) is commonly used to correct refractive errors including myopia and hyperopia. An anterior circular hinged flap of about 150 µm thickness is first created using a femtosecond laser. The flap is then folded back to expose the stromal bed which is reshaped by excimer laser ablation. The procedure is completed by returning the flap to conform with the ablated profile. Although laser ablation creates a new central corneal curvature with the goal of achieving emmetropia, the biomechanical response of the residual (significantly thinned) stromal tissue is an important but challenging factor that should be considered [72]. In this study, we assume that active endothelium ionic pumping is functioning normally and use the proposed model to investigate the biomechanical response of the cornea, including stromal fluid pressure and local swelling, resulting from a typical and representative myopic correction.
The geometry shown in figure 14 is for the pre-operative cornea and defines the shape of the normo-hydrated cornea and surgical profile while the cornea is supporting an IOP of 15 mmHg. The ablation depth profile was determined using theory presented by Munnerlyn [73] for an ablation radius of curvature of 8.847 mm (corresponding to an intended correction of approx. −5 dioptres). The CCT is 520 µm, and the maximum ablation depth of 87 µm combines with the 150 µm flap to produce a residual central stromal thickness of 283 µm. With this ablation profile, the residual stromal thickness is 54.4% at the cornea centre and gradually increases to 71.2% at the periphery of the flap. The flap is returned after ablation (where negative osmotic pressure holds it in place by suction) but it is no longer mechanically integrated with the stroma and can be easily separated at any time after surgery [72]. LASIK therefore effectively removes that part of the stroma in which lamella inclination is most pronounced (the anterior third).
Figure 14.
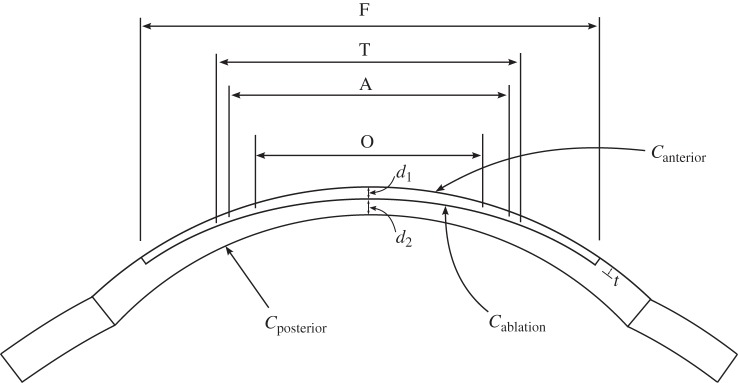
Illustration of the flap geometry and ablation profile in the LASIK surgery designed for an intended correction of approximately −5 dioptres. The anterior flap diameter F = 9 mm, transition zone diameter T = 6.16 mm, sculpted surface diameter A = 6 mm and optic zone diameter (for least-squares curvature extraction) O = 5 mm. The CCT is 520 µm, and the maximum ablation depth of 87 µm combines with the 150 µm flap thickness to produce a residual central stromal thickness of d2 = 283 µm.
Because the collagen fibril distribution is not symmetric about any axis, a full 360° model is required. The eye is supported in the eye socket by fatty tissue and stabilized by six muscles responsible for eye movement. However, it is not necessary to extend the sclera to the posterior pole; numerical experiments were conducted and it was determined that extending the scleral tissue for an arc length of 2 mm was sufficient, at which point the tissue can be ‘clamped’. The finite-element model consists of 7008 27-node hexahedral elements. The solution was obtained in two phases. In the presurgical phase, the normal cornea was loaded by an IOP of 15 mmHg and a global fixed-point algorithm was employed to obtain the collagen prestress. With this prestress, the normal cornea is in equilibrium under the IOP and is in the pre-operative geometry of figure 14. In the postsurgical phase, the elements corresponding to the flap and ablation region are removed from the mesh while maintaining the IOP and normal ionic pumping. The model is no longer in equilibrium and the new deformed state is obtained by Newton's method.
We first compare the predicted biomechanical response to clinical measurements. Ortiz et al. [74] made measurements of changes in corneal anterior curvature and correlated these to the surgical radius of curvature in the ablation profile. The case study analysed 85 eyes with myopia or myopic astigmatism and subdivided them into two groups: 44 with flaps created by microkeratome (‘mechanical’) and 41 with flaps created by femtosecond laser (‘laser’). The biomechanical response to LASIK was evaluated by means of the coefficient of curvature change defined by
where Rpost is the postsurgical corneal radius at one month and RS is the surgical radius imposed on the stromal bed by ablation. The postsurgical corneal radius was measured by topography over a central 5 mm diameter central zone. Likewise, the postsurgical corneal radius of the finite-element model used least-squares fitting over a 5 mm diameter zone.5 The mean value of C found by Ortiz et al. [74] is compared to the predicted value found by the present model in table 2. Ortiz et al. [74] also performed a statistical analysis for a linear fit function between Rpost and RS and found 
 for the ‘mechanical’ group and
for the ‘mechanical’ group and 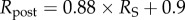
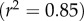 for the ‘laser’ group. Using the model value
for the ‘laser’ group. Using the model value 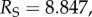 the fit functions for Rpost are compared to the predicted value in table 2. Finally, the spherical equivalent
the fit functions for Rpost are compared to the predicted value in table 2. Finally, the spherical equivalent 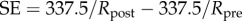 measures the power change in dioptres achieved by changing the presurgical radius Rpre to the postsurgical radius Rpost. Clinical values for SE with Rs = 8.847 are compared to the predicted value6 in table 2. It may be seen that the finite-element model predictions agree closely with the clinical data.
measures the power change in dioptres achieved by changing the presurgical radius Rpre to the postsurgical radius Rpost. Clinical values for SE with Rs = 8.847 are compared to the predicted value6 in table 2. It may be seen that the finite-element model predictions agree closely with the clinical data.
Table 2.
Comparisons of the curvature change coefficient C, postsurgical corneal radius Rpost and spherical equivalent SE calculated by FEM and measured by Ortiz et al. [74].
| C | Rpost (mm) | SE (1/m) | |
|---|---|---|---|
| Ortiz et al.—mech | −3.2% ± 1.9% | 8.516 | −3.638 |
| Ortiz et al.—laser | −1.6% ± 1.3% | 8.685 | −4.411 |
| FEM | −3.12% | 8.571 | −3.892 |
We analysed the fluid pressure and swelling response of the postsurgical cornea. The fluid pressure is given by 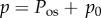 (equation (2.11)), where Pos is the osmotic pressure and p0 is the bath pressure (IOP) of 15 mmHg. Because most inclined lamellae have been removed by surgery, it may be anticipated that the fluid pressure must approach zero in the anterior region of the residual stroma. Since p0 is constant, this implies that the tissue will swell and the osmotic pressure will become negative (see also figure 9a). Fluid pressure fringes are shown in the cutaway half-mesh of figure 15a and local volume dilation fringes are shown in 15b. Figure 15a shows that the fluid pressure reduces to approximately half of its normal value in the ablation region.7 The local swelling shown in figure 15b is necessary to produce a negative osmotic pressure. It is concentrated in the upper region of the residual stroma, particularly where the ablation is deepest and lamella inclination is lost. It is observed that the swelling is modest, around 3.5% maximum and decreasing with depth. This is so because only small swelling is needed to produce negative osmotic pressure of the order of 15 mmHg (figure 9a). This will add some contribution to the biomechanical response in LASIK, but it is probably secondary to the elastic response. However, if IOP increases, then the swelling increases significantly, as shown in table 3.
(equation (2.11)), where Pos is the osmotic pressure and p0 is the bath pressure (IOP) of 15 mmHg. Because most inclined lamellae have been removed by surgery, it may be anticipated that the fluid pressure must approach zero in the anterior region of the residual stroma. Since p0 is constant, this implies that the tissue will swell and the osmotic pressure will become negative (see also figure 9a). Fluid pressure fringes are shown in the cutaway half-mesh of figure 15a and local volume dilation fringes are shown in 15b. Figure 15a shows that the fluid pressure reduces to approximately half of its normal value in the ablation region.7 The local swelling shown in figure 15b is necessary to produce a negative osmotic pressure. It is concentrated in the upper region of the residual stroma, particularly where the ablation is deepest and lamella inclination is lost. It is observed that the swelling is modest, around 3.5% maximum and decreasing with depth. This is so because only small swelling is needed to produce negative osmotic pressure of the order of 15 mmHg (figure 9a). This will add some contribution to the biomechanical response in LASIK, but it is probably secondary to the elastic response. However, if IOP increases, then the swelling increases significantly, as shown in table 3.
Figure 15.
Fringe plots of (a) the fluid pressure and (b) volume dilation of a postsurgical cornea after LASIK designed for an intended correction of −5 dioptres.
Table 3.
Predicted volume dilation of the anterior residual stroma after LASIK for three values of IOP.
| IOP (mmHg) | dilation J |
|---|---|
| 15 | 1.031 |
| 30 | 1.086 |
| 45 | 1.147 |
7. Discussion
The proposed model follows the general theory for hydrophilic and highly swollen polyelectrolyte gels introduced by Katchalsky & Michaeli [24] combined with the membrane transport theory of Kedem & Katchalsky [16]. The main work was the characterization of the stromal electrolyte and elastic free energies, including the modelling of modifications due to active endothelial ionic transport. The resulting model predicts the ex vivo (no active endothelial ionic transport) osmotic pressure and osmotic compressibility with good accuracy compared to experimental measurements (as described in §6.1.1) and provides some confidence in the general framework. Previous equilibrium modelling of corneal swelling by Bryant & McDonnell [21] was based on numerical solution of the spherically symmetric (one-dimensional) fluid and ionic continuity equations in a multiphasic theory [22,75]. The current energy-based approach is equivalent (see the electronic supplementary material) in that it implies satisfaction of the same governing equations but it offers some distinct advantages.
The energy approach provides an average (or homogenized) energy-equivalent value of osmotic pressure over a unit cell (see equation (2.9)). This is useful for consistently embedding nanoscale effects, for example, the distribution of GAG charge into collagen coating and non-coating regions, in a macroscopic theory. By contrast, the triphasic theory produces a pointwise varying expression for the osmotic pressure. The energy approach is also convenient for describing the deviation of the stromal electrolyte from ideal conditions, including electrolyte volume exclusion effects associated with the collagen fibrils and stromal cell (keratocyte) population. Also noteworthy is the fact that the energy formulation is a natural approach for finite-element approximation which can be based directly on the variational forms provided by the free energy terms. The proposed formulation only requires solving for the displacement field, which gives practical convenience in finite-element implementation. The model contains numerous physical constants and parameters (see reference values in table 1), but most are well characterized and all simulations presented used these reference values—no problem-dependent parameters appear in the theory.
Modelling the influence of active endothelial ionic fluxes on the biophysics of corneal tissue hydration in a fully three-dimensional context presents a challenge. In this approach, we have analytically integrated the phenomenological KK [16] membrane transport equations to describe the membrane osmotic gradient as a function of the active ionic fluxes and endothelial ionic permeability.8 This leads to a modified Boltzmann ionic distribution in the stroma and corresponding electrostatic free energy. The effects are contained in the introduced ionic transport parameters Q+ and Q− which correspond to cation and anion transport, respectively. Interestingly, for a binary electrolyte the influence of active ionic transport appears as a function of the combined parameter Q+Q−. The model suggests that active endothelial ionic transport reduces osmotic pressure and hence swelling pressure (these are essentially equivalent in the case of the highly hydrated cornea) and the pump-leak mechanism can be explained as active pumping producing a downward shift of the swelling pressure–hydration curve as discussed in §6.2.1 and illustrated in figure 9a.
Precise quantification of the fluid and osmotic pressures remains an open question for the human in vivo cornea, as direct measurements are not currently feasible. Conventional analysis [15,18,19] predicts a negative fluid pressure throughout the cornea. In this study, osmotic calibration based on imbibition pressure measurements for rabbit indicate positive fluid pressure at normal hydration, which is in agreement with [21]. The calibration suggests that stromal fluid pressure must be close to IOP and osmotic pressure must be close to zero. It is interesting to analyse how the forces acting at the corneal bounding surfaces are balanced under these conditions. The posterior stroma boundary condition, equation (5.13), may be rewritten in the direction normal to the corneal posterior surface as 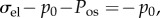 where σel is the collagen effective stress component and p0 denotes the IOP. As the posterior collagen fibres are parallel to the corneal surface, they can provide negligible force in the transverse direction and so
where σel is the collagen effective stress component and p0 denotes the IOP. As the posterior collagen fibres are parallel to the corneal surface, they can provide negligible force in the transverse direction and so 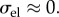 Thus, it may be concluded that the in vivo osmotic pressure Pos must be zero at the posterior stroma—in agreement with the calibration.
Thus, it may be concluded that the in vivo osmotic pressure Pos must be zero at the posterior stroma—in agreement with the calibration.
The situation is quite different at the epithelial layer. Here there is no external pressure loading to balance the stromal fluid pressure (figure 12). Inclined lamellae insert into Bowman's layer [35] where they must act to resist the stromal fluid pressure applied to the epithelium. The mechanical balance equation across the epithelium, equation (5.14), written in the normal direction is  Thus, the corneal anterior boundary could not be stably maintained without the contribution from inclined fibres and which leads to the following hypothetical question—if lamella inclination was absent, how would the boundary condition then be satisfied? This in essence is what occurs in LASIK where the inclined lamellae are largely removed by flap creation and ablation. In this case, the effective stress will become zero in the normal direction
Thus, the corneal anterior boundary could not be stably maintained without the contribution from inclined fibres and which leads to the following hypothetical question—if lamella inclination was absent, how would the boundary condition then be satisfied? This in essence is what occurs in LASIK where the inclined lamellae are largely removed by flap creation and ablation. In this case, the effective stress will become zero in the normal direction  and the in vivo cornea must swell until a negative osmotic pressure is generated so that
and the in vivo cornea must swell until a negative osmotic pressure is generated so that 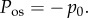 This is precisely what the model predicts in LASIK as described in §6.2.4 (although only approx. 3% swelling is needed to achieve
This is precisely what the model predicts in LASIK as described in §6.2.4 (although only approx. 3% swelling is needed to achieve 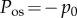 ).
).
We have calibrated this model for the normal living cornea by assuming that GAG fixed charge is not depth-dependent; this leads to a hydration state that is also uniform with depth. It has been observed by Castoro et al. [76] that the anterior stroma may be slightly less hydrated than the posterior. The water content of these two stromal layers is measured to be 75% and 79% for rabbit, respectively [77]. Furthermore, biochemical studies on bovine corneas have shown that posterior stroma has a higher ratio of keratan sulfate to dermatan sulfate [76]. For human corneas, the depth-dependence of GAG types is unknown. Given that the hydration gradient is small through the corneal depth (less than 5%), it seems justifiable to employ a uniform fixed charge distribution as a first approximation. However, this can be easily modified as new information becomes available. Likewise, flexible GAG chains, the linear macromolecular structures formed from disaccharide units, may contribute an entropic elastic energy [23]. Each GAG chain is covalently bound at one end to the PG core protein and so is anchored to a collagen fibril (and thereby providing fixed charges). It has been proposed [9,10] that two or more GAG chains can link to form antiparallel duplex structures bridging between neighbouring collagen fibrils and can transmit entropic-based forces deriving from thermal motions. Such contributions can be modelled through appropriate free energy expressions but are considered to be a second-order effect and neglected in this study.
The stromal electrolyte free energy (equation (3.22)) is convex in the dilation J and represents a rational basis for modelling the osmotic behaviour of the tissue. Indeed we have shown that the model is capable of simulating massive swelling and the associated hydration change in the case of stroma immersed in an ionic bath. The predictions also show that swelling interaction with the collagen network leads to the observed anisotropy of swelling (primarily through the thickness direction) and inhomogeneity (most swelling occurs in the posterior cornea) which strongly supports the idea that the anterior cornea exhibits rigidity with respect to changes in hydration [29,31]. Measurements for in vivo swelling are not precise, but model predictions appear to agree qualitatively in all presented cases, and quantitatively for the rather subtle problem of swelling under increasing IOP. The model has provided predictions for swelling under Fuchs' dystrophy and should also be adaptable to modelling other diseases of the cornea such as macular corneal dystrophy, where the GAG concentration is found to be abnormally low.
The model supports the necessity of lamellae inclination for stability of the corneal anterior (refractive) surface [29,31]. When lamella inclination is removed synthetically by adjusting the fibre distributions, the model predicts an enormous increase in swelling in a stroma sample immersed in de-ionized water (§6.1.2). Theoretically, such swelling would become infinite, but in this model is limited by the small matrix shear stiffness. The structural role of lamella inclination has been examined here for the first time and the results suggest that this aspect of the collagen architecture is extremely important for understanding swelling behaviour and how the stromal fluid pressure is equilibrated.
Finally, it is remarked that clinical swelling (oedema), which results from hydration changes in the pathological cornea, can occur under a wide variety of circumstances. For example, during hypoxia due to contact lens wear, lactate ion production increases and results in oedema [19,78]. The underlying mechanism has been identified as the accumulation of lactate acid due to increasing anaerobic respiration [78]. Extension of the model to include these multiple species and their interactions through metabolic reactions for aerobic and anaerobic respiration is currently under development.
Supplementary Material
Supplementary Material
Endnotes
For example, in application to the in vivo cornea, collagen fibres in Ω0 are prestressed by the action of the IOP.
Fluorophore-assisted carbohydrate electrophoresis.
If future studies produce a different conclusion regarding human stromal fluid pressure, including variation through depth, the value of Q+Q− can be re-calibrated without any change to the general theory.
This requires iterative solution for the configuration  and collagen prestress that equilibrates the IOP—see §2.
and collagen prestress that equilibrates the IOP—see §2.
The model analysed the biomechanical (elastic and swelling) response of the residual stroma. The flap was not analysed and the postsurgical anterior radius of the cornea was taken to be that of the postsurgical ablation profile radius, found by least-squares fitting, extended by a constant flap thickness of 150 µm.
The mean clinical and finite-element model values of Rpre were 7.8 and 7.87 mm, respectively—the value Rpre = 7.8 mm was used in calculating SE for consistency.
Because p0 is constant at 15 mmHg (0.002 MPa), we have Pos = p−0.002, and the fringe plot can be interpreted in terms of osmotic pressure.
Water permeability does not appear because at equilibrium water flux will be zero.
Authors' contributions
X.C. and P.M.P. designed the research and developed the mathematical modelling of the electrolyte; S.J.P. and P.M.P. developed the mathematical modelling of the stromal elasticity; X.C. and P.M.P. performed the numerical analysis and interpretation; all authors wrote the paper jointly.
Competing interests
We declare we have no competing interests.
Funding
This research was supported by the Stanford University Bio-X Interdisciplinary Initiatives Program (IIP). X.C. received a Stanford Graduate Fellowship (SGF) from Stanford University. S.J.P. received a Bio-X Graduate Student Fellowship from Stanford University.
References
- 1.Elliott GF, Hodson SA. 1998. Cornea, and the swelling of polyelectrolyte gels of biological interest. Rep. Prog. Phys. 61, 1325–1365. ( 10.1088/0034-4885/61/10/001) [DOI] [Google Scholar]
- 2.Hodson SA. 1971. Why the cornea swells. J. Theor. Biol. 33, 419–427. ( 10.1016/0022-5193(71)90090-7) [DOI] [PubMed] [Google Scholar]
- 3.Pinsky PM, Heide D, Chernyak D. 2005. Computational modeling of mechanical anisotropy in the cornea and sclera. J. Cataract. Refract. Surg. 31, 136–145. ( 10.1016/j.jcrs.2004.10.048) [DOI] [PubMed] [Google Scholar]
- 4.Pandolfi A, Holzapfel GA. 2008. Three-dimensional modeling and computational analysis of the human cornea considering distributed collagen fibril orientations. J. Biomech. Eng. 130, 061006 ( 10.1115/1.2982251) [DOI] [PubMed] [Google Scholar]
- 5.Studer H, Larrea X, Riedwyl H, Büchler P. 2010. Biomechanical model of human cornea based on stromal microstructure. J. Biomech. 43, 836–842. ( 10.1016/j.jbiomech.2009.11.021) [DOI] [PubMed] [Google Scholar]
- 6.Petsche SJ, Pinsky PM. 2013. The role of 3-D collagen organization in stromal elasticity: a model based on X-ray diffraction data and second harmonic-generated images. Biomech. Model. Mechanobiol. 12, 1101–1113. ( 10.1007/s10237-012-0466-8) [DOI] [PubMed] [Google Scholar]
- 7.Maurice DM. 1962. Clinical physiology of the cornea. Int. Ophthalmol. Clin. 2, 561–572. ( 10.1097/00004397-196210000-00002) [DOI] [PubMed] [Google Scholar]
- 8.Meek KM. 2008. The cornea and sclera. In Collagen: structure and mechanics (ed. Fratzl P.), pp. 359–396. Berlin, Germany: Springer. [Google Scholar]
- 9.Lewis PN, Pinali C, Young RD, Meek KM, Quantock AJ, Knupp C. 2010. Structural interactions between collagen and proteoglycans are elucidated by three-dimensional electron tomography of bovine cornea. Structure 18, 239–245. ( 10.1016/j.str.2009.11.013) [DOI] [PubMed] [Google Scholar]
- 10.Scott JE. 1988. Proteoglycan–fibrillar collagen interactions. Biochem. J. 252, 313–323. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Dawson DG, Ubels JL, Edelhauser HF. 2011. Cornea and sclera. In Adler's physiology of the eye (eds Levin LA et al.), pp. 71–130, 11th edn Amsterdam, The Netherlands: Elsevier. [Google Scholar]
- 12.Bonanno JA. 2012. Molecular mechanisms underlying the corneal endothelial pump. Exp. Eye. Res. 95, 2–7. ( 10.1016/j.exer.2011.06.004) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Maurice DM. 1972. The location of the fluid pump in the cornea. J. Physiol. 221, 43–54. ( 10.1113/jphysiol.1972.sp009737) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Fischbarg J, Maurice DM. 2004. An update on corneal hydration control. Exp. Eye. Res. 78, 537–541. ( 10.1016/j.exer.2003.09.010) [DOI] [PubMed] [Google Scholar]
- 15.Klyce SD, Russell SR. 1979. Numerical solution of coupled transport equations applied to corneal hydration dynamics. J. Physiol. 292, 107–134. ( 10.1113/jphysiol.1979.sp012841) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 16.Kedem O, Katchalsky A. 1958. Thermodynamic analysis of the permeability of biological membranes to non-electrolytes. Biochim. Biophys. Acta. 27, 229–246. ( 10.1016/0006-3002(58)90330-5) [DOI] [PubMed] [Google Scholar]
- 17.Ruberti JW, Klyce SD. 2003. NaCl osmotic perturbation can modulate hydration control in rabbit cornea. Exp. Eye. Res. 76, 349–359. ( 10.1016/S0014-4835(02)00301-9) [DOI] [PubMed] [Google Scholar]
- 18.Li LY, Tighe B. 2006. Numerical simulation of corneal transport processes. J. R. Soc. Interface 3, 303–310. ( 10.1098/rsif.2005.0085) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 19.Leung BK, Bonanno JA, Radke CJ. 2011. Oxygen-deficient metabolism and corneal edema following epithelial hypoxia in the rabbit. Prog. Retin. Eye. Res. 30, 471–492. ( 10.1016/j.preteyeres.2011.07.001) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 20.Hedbys BO, Dohlman CH. 1963. A new method for the determination of the swelling pressure of the corneal stroma in vitro. Exp. Eye. Res. 2, 122–129. ( 10.1016/S0014-4835(63)80003-2) [DOI] [PubMed] [Google Scholar]
- 21.Bryant MR, McDonnell PJ. 1998. A triphasic analysis of corneal swelling and hydration control. Trans. ASME 120, 370–381. ( 10.1115/1.2798004) [DOI] [PubMed] [Google Scholar]
- 22.Lai WM, Hou JS, Mow VC. 1991. A triphasic theory for swelling and deformation behaviors of articular cartilage. J. Biomech. Eng. 113, 245–258. ( 10.1115/1.2894880) [DOI] [PubMed] [Google Scholar]
- 23.Cheng X, Pinsky PM. 2013. Mechanisms of self-organization for the collagen fibril lattice in the human cornea. J. R. Soc. Interface 10, 20130512 ( 10.1098/rsif.2013.0512) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 24.Katchalsky A, Michaeli I. 1955. Polyelectrolyte gels in salt solutions. J. Polym. Sci. 15, 69–86. ( 10.1002/pol.1955.120157906) [DOI] [Google Scholar]
- 25.Sharp KA, Honig B. 1990. Calculating total electrostatic energies with the nonlinear Poisson–Boltzmann equation. J. Phys. Chem. 94, 7684–7692. ( 10.1021/j100382a068) [DOI] [Google Scholar]
- 26.Lee D, Wilson G. 1981. Non-uniform swelling properties of the corneal stroma. Curr. Eye. Res. 1, 457–461. ( 10.3109/02713688109019986) [DOI] [PubMed] [Google Scholar]
- 27.Kikkawa Y, Hirayama K. 1970. Uneven swelling of the corneal stroma. Investig. Ophthalmol. Vis. Sci. 9, 735–741. [PubMed] [Google Scholar]
- 28.Meek KM, Leonard DW, Connon CJ, Dennis S, Khan S. 2003. Transparency, swelling and scarring in the corneal stroma. Eye 17, 927–936. ( 10.1038/sj.eye.6700574) [DOI] [PubMed] [Google Scholar]
- 29.Muller LJ, Pels E, Vrensen GF. 2001. The specific architecture of the anterior stroma accounts for maintenance of corneal curvature. Br. J. Ophthalmol. 85, 437–443. ( 10.1136/bjo.85.4.437) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 30.Brunette I, Sherknies D, Terry MA, Chagnon M, Bourges JL, Meunier J. 2009. 3-D characterization of the corneal shape in Fuchs' dystrophy and pseudophakic keratopathy. Invest. Ophthalmol. Vis. Sci. 52, 206–214. ( 10.1167/iovs.09-4101) [DOI] [PubMed] [Google Scholar]
- 31.Bron AJ. 2001. The architecture of the corneal stroma. Br. J. Ophthalmol. 85, 379–383. ( 10.1136/bjo.85.4.379) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Meek KM, Blamires T, Elliott GF, Gyi TJ, Nave C. 1987. The organisation of collagen fibrils in the human corneal stroma: a synchrotron X-ray diffraction study. Curr. Eye. Res. 6, 841–846. ( 10.3109/02713688709034853) [DOI] [PubMed] [Google Scholar]
- 33.Abahussin M, Hayes S, Knox Cartwright NE, Kamma-Lorger CS, Khan Y, Marshall J, Meek KM. 2009. 3D collagen orientation study of the human cornea using X-ray diffraction and femtosecond laser technology. Invest. Ophthalmol. Vis. Sci. 50, 5159–5164. ( 10.1167/iovs.09-3669) [DOI] [PubMed] [Google Scholar]
- 34.Morishige N, Wahlert AJ, Kenney MC, Brown DJ, Kawamoto K, Chikama TI, Nishida T, Jester JV. 2007. Second-harmonic imaging microscopy of normal human and keratoconus cornea. Invest. Ophthalmol. Vis. Sci. 48, 1087–1094. ( 10.1167/iovs.06-1177) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 35.Winkler M, Chai D, Kriling S, Nien CJ, Brown DJ, Jester B, Juhasz T, Jester JV. 2011. Nonlinear optical macroscopic assessment of 3-D corneal collagen organization and axial biomechanics. Investig. Ophthalm. Vis. Sci. 52, 8818–8827. ( 10.1167/iovs.11-8070) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 36.Jester JV, Winkler M, Jester BE, Nien C, Chai D, Brown DJ. 2010. Evaluating corneal collagen organization using high-resolution non-linear optical macroscopy. Eye Contact Lens 36, 260–264. ( 10.1097/ICL.0b013e3181ee8992) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 37.Winkler M, Shoa G, Xie Y, Petsche SJ, Pinsky PM, Juhasz T, Brown DJ, Jester JV. 2013. Three-dimensional distribution of transverse collagen fibers in the anterior human corneal stroma. Investig. Ophthalm. Vis. Sci. 54, 7293–7301. ( 10.1167/iovs.13-13150) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Gee MW, Forster C, Wall WA. 2010. A computational strategy for prestressing patient-specific biomechanical problems under finite deformation. Int. J. Numer. Meth. Biomed. Engng. 26, 52–72. ( 10.1002/cnm.1236) [DOI] [Google Scholar]
- 39.Li Y, Suo Z, Vlassak JJ. 2014. A model of ideal elastomeric gels for polyelectrolyte gels. Soft. Matt. 10, 2582–2590. ( 10.1039/c3sm52751d) [DOI] [PubMed] [Google Scholar]
- 40.Hong W, Zhao X, Suo Z. 2010. Large deformation and electrochemistry of polyelectrolyte gels. J. Mech. Phys. Solids 58, 558–577. ( 10.1016/j.jmps.2010.01.005) [DOI] [Google Scholar]
- 41.Buschmann MD, Grodzinsky AJ. 1995. A molecular model of proteoglycan-associated electrostatic forces in cartilage mechanics. J. Biomech. Eng. 117, 179–192. ( 10.1115/1.2796000) [DOI] [PubMed] [Google Scholar]
- 42.Urban JPG, Maroudas A, Bayliss MT, Dillon J. 1979. Swelling pressures of proteoglycans at the concentrations found in cartilaginous tissues. Biorheology 16, 447–464. [DOI] [PubMed] [Google Scholar]
- 43.Plaas AH, West LA, Thonar EJ, Karcioglu ZA, Smith CJ, Klintworth GK, Hascall VC. 2001. Altered fine structures of corneal and skeletal keratan sulfate and chondroitin/dermatan sulfate in macular corneal dystrophy. J. Biol. Chem. 276, 39 788–39 796. ( 10.1074/jbc.M103227200) [DOI] [PubMed] [Google Scholar]
- 44.Petsche SJ. 2014. Multiscale mechanics and experimental investigation of the human cornea. PhD thesis, Stanford University, USA.
- 45.Fratzl P, Daxer A. 1993. Structural transformation of collagen fibrils in corneal stroma during drying. Biophys. J. 64, 1210–1214. ( 10.1016/S0006-3495(93)81487-5) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 46.Miyagawa A, Kobayashi M, Fujita Y, Hamdy O, Hirano K, Nakamura M, Miyake Y. 2001. Surface ultrastructure of collagen fibrils and their association with proteoglycans in human cornea and sclera by atomic force microscopy and energy-filtering transmission electron microscopy. Cornea 20, 651–656. ( 10.1097/00003226-200108000-00019) [DOI] [PubMed] [Google Scholar]
- 47.Muller LJ, Pels E, Schurmans LR, Vrensen GF. 2004. A new three-dimensional model of the organization of proteoglycans and collagen fibrils in the human corneal stroma. Exp. Eye. Res. 78, 493–501. ( 10.1016/S0014-4835(03)00206-9) [DOI] [PubMed] [Google Scholar]
- 48.Twersky V. 1975. Transparency of pair-correlated, random distributions of small scatterers, with applications to the cornea. J. Opt. Soc. Am. 65, 524–530. ( 10.1364/JOSA.65.000524) [DOI] [PubMed] [Google Scholar]
- 49.Huang Y, Meek KM. 1999. Swelling studies on the cornea and sclera: the effects of pH and ionic strength. Biophys. J. 77, 1655–1665. ( 10.1016/S0006-3495(99)77013-X) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Goodfellow JM, Elliot GF, Woolgar AE. 1978. X-ray diffraction studies of the corneal stroma. J. Mol. Biol. 119, 237–252. ( 10.1016/0022-2836(78)90436-9) [DOI] [PubMed] [Google Scholar]
- 51.Meek KM, Fullwood NJ, Cooke PH, Elliott GF, Maurice DM, Quantock AJ, Wall RS, Worthington CR. 1991. Synchrotron X-ray diffraction studies of the cornea, with implications for stromal hydration. Biophys. J. 60, 467–474. ( 10.1016/S0006-3495(91)82073-2) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 52.Olsen T, Sperling S. 1987. The swelling pressure of the human corneal stroma as determined by a new method. Exp. Eye. Res. 44, 481–490. ( 10.1016/S0014-4835(87)80159-8) [DOI] [PubMed] [Google Scholar]
- 53.Hedbys BO, Mishima S. 1966. The thickness–hydration relationship of cornea. Exp. Eye. Res. 5, 221–228. ( 10.1016/S0014-4835(66)80010-6) [DOI] [PubMed] [Google Scholar]
- 54.Elhalis H, Azizi B, Jurkunas U. 2010. Fuchs endothelial corneal dystrophy. Ocul. Surf. 8, 173–184. ( 10.1016/S1542-0124(12)70232-X) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 55.Bourne WM. 1998. Clinical estimation of corneal endothelial pump function. Trans. Am. Ophthalmol. Soc. 96, 229–242. [PMC free article] [PubMed] [Google Scholar]
- 56.Watsky MA, McDermott ML, Edelhauser HF. 1989. In vitro corneal endothelial permeability in rabbit and human: the effects of age, cataract surgery and diabetes. Exp. Eye. Res. 49, 751–767. ( 10.1016/S0014-4835(89)80036-3) [DOI] [PubMed] [Google Scholar]
- 57.Hedbys BO, Mishima S, Maurice DM. 1963. The imbibition pressure of the corneal stroma. Exp. Eye. Res. 2, 99–111. ( 10.1016/S0014-4835(63)80001-9) [DOI] [PubMed] [Google Scholar]
- 58.Hartmanna S, Neffb P. 2003. Polyconvexity of generalized polynomial-type hyperelastic strain energy functions for near-incompressibility. Int. J. Solids. Struct. 40, 2767–2791. ( 10.1016/S0020-7683(03)00086-6) [DOI] [Google Scholar]
- 59.Lanir Y. 1979. A structural theory for the homogeneous biaxial stress–strain relationships in flat collagenous tissues. J. Biomech. 12, 423–436. ( 10.1016/0021-9290(79)90027-7) [DOI] [PubMed] [Google Scholar]
- 60.Meek KM, Tuft SJ, Huang Y, Gill PS, Hayes S, Newton RH, Bron AJ. 2005. Changes in collagen orientation and distribution in keratoconus corneas. Invest. Ophthalmol. Vis. Sci. 46, 1948–1956. ( 10.1167/iovs.04-1253) [DOI] [PubMed] [Google Scholar]
- 61.Markert B, Ehlers W, Karajan N. 2005. A general polyconvex strain–energy function fiber-reinforced materials. Proc. Appl. Math. Mech. 5, 245–246. ( 10.1002/pamm.200510099) [DOI] [Google Scholar]
- 62.Petsche SJ, Chernyak D, Martiz J, Levenston ME, Pinsky PM. 2012. Depth-dependent transverse shear properties of the human corneal stroma. Investig. Ophthalmol. Vis. Sci. 53, 873–880. ( 10.1167/iovs.11-8611) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 63.Sondergaard AP, Ivarsen A, Hjortdal J. 2013. Corneal resistance to shear force after UVA-riboflavin cross-linking. Investig. Ophthalmol. Vis. Sci. 54, 5059–5069. ( 10.1167/iovs.12-10710) [DOI] [PubMed] [Google Scholar]
- 64.Elsheikh A, Alhasso D, Rama P. 2008. Assessment of the epithelium's contribution to corneal biomechanics. Exp. Eye. Res. 86, 445–451. ( 10.1016/j.exer.2007.12.002) [DOI] [PubMed] [Google Scholar]
- 65.Ytteborg J, Dohlman CH. 1965. Corneal edema and intraocular pressure II. Clinical results. Arch. Ophthalmol. 74, 477–484. ( 10.1001/archopht.1965.00970040479008) [DOI] [PubMed] [Google Scholar]
- 66.Herman DC, Hodge DO, Bourne WM. 2001. Increased corneal thickness in patients with ocular hypertension. Arch. Ophthalmol. 119, 334–336. ( 10.1001/archopht.119.3.334) [DOI] [PubMed] [Google Scholar]
- 67.Lee GA, Khaw PT, Ficker LA, Shah P. 2002. The corneal thickness and intraocular pressure story: where are we now? Clin. Exp. Ophthalmol. 30, 334–337. ( 10.1046/j.1442-9071.2002.00551.x) [DOI] [PubMed] [Google Scholar]
- 68.Waring GO, Bourne WM, Edelhauser HF, Kenyon KR. 1982. The corneal endothelium: normal and pathologic structure and function. Ophthalmology 89, 531–590. ( 10.1016/S0161-6420(82)34746-6) [DOI] [PubMed] [Google Scholar]
- 69.Ehlers N. 1966. The fibrillary texture and the hydration of the cornea. Acta. Ophthalmol. 44, 620–630. ( 10.1111/j.1755-3768.1966.tb08080.x) [DOI] [PubMed] [Google Scholar]
- 70.Ehlers N, Riise D. 1967. On corneal thickness and intraocular pressure. Acta. Ophthalmol. 45, 809–813. [DOI] [PubMed] [Google Scholar]
- 71.Johnson CS, Mian SI, Moroi S, Epstein D, Izatt J, Afshari NA. 2007. Role of corneal elasticity in damping of intraocular pressure. Invest. Ophthalmol. Vis. Sci. 48, 2540–2544. ( 10.1167/iovs.06-0719) [DOI] [PubMed] [Google Scholar]
- 72.Dupps WJ, Wilson SE. 2009. Biomechanics and wound healing in the cornea. Exp. Eye. Res. 83, 709–720. ( 10.1016/j.exer.2006.03.015) [DOI] [PMC free article] [PubMed] [Google Scholar]
- 73.Munnerlyn CR, Koons SJ, Marshall J. 1988. Photorefractive keratectomy: a technique for laser refractive surgery. J. Cataract. Refract. Surg. 14, 46–52. ( 10.1016/S0886-3350(88)80063-4) [DOI] [PubMed] [Google Scholar]
- 74.Ortiz D, Alio JL, Pinero D. 2008. Measurement of corneal curvature change after mechanical laser in situ keratomileusis flap creation and femtosecond laser flap creation. J. Cataract. Refract. Surg. 34, 238–242. ( 10.1016/j.jcrs.2007.09.023) [DOI] [PubMed] [Google Scholar]
- 75.Huyghe JM, Janssen JD. 1997. Quadriphasic theory of swelling incompressible porous media. Int. J. Eng. Sci. 35, 793–802. ( 10.1016/S0020-7225(96)00119-X) [DOI] [Google Scholar]
- 76.Castoro JA, Bettelhem AA, Berrelheim FA. 1988. Water gradients across bovine cornea. Investig. Ophthalmol. Vis. Sci. 29, 963–968. [PubMed] [Google Scholar]
- 77.Turss R, Friend J, Reim M, Dohlman CH. 1971. Glucose concentration and hydration of the corneal stroma. Ophthalmic. Res. 2, 253–260. ( 10.1159/000264570) [DOI] [Google Scholar]
- 78.Klyce SD. 1981. Stromal lactate accumulation can account for corneal oedema osmotically following epithelial hypoxia in the rabbit. J. Physiol. 321, 49–64. ( 10.1113/jphysiol.1981.sp013971) [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.