The good, the bad and the ugly of marine reserves for fishery yields

Abstract

Marine reserves (MRs) are used worldwide as a means of conserving biodiversity and protecting depleted populations. Despite major investments in MRs, their environmental and social benefits have proven difficult to demonstrate and are still debated. Clear expectations of the possible outcomes of MR establishment are needed to guide and strengthen empirical assessments. Previous models show that reserve establishment in overcapitalized, quota-based fisheries can reduce both catch and population abundance, thereby negating fisheries and even conservation benefits. By using a stage-structured, spatially explicit stochastic model, we show that catches under quota-based fisheries that include a network of MRs can exceed maximum sustainable yield (MSY) under conventional quota management if reserves provide protection to old, large spawners that disproportionally contribute to recruitment outside the reserves. Modelling results predict that the net fishery benefit of MRs is lost when gains in fecundity of old, large individuals are small, is highest in the case of sedentary adults with high larval dispersal, and decreases with adult mobility. We also show that environmental variability may mask fishery benefits of reserve implementation and that MRs may buffer against collapse when sustainable catch quotas are exceeded owing to stock overestimation or systematic overfishing.

1. Introduction

Recent evidence of widespread decline of marine populations and extensive loss of marine ecosystem functions and services critical to human well-being has emphasized the need for more effective approaches to marine conservation and resource management [1–3]. The use of marine reserves (MRs)—areas where extractive activities are prohibited—is a spatial approach to marine conservation and management aimed at protecting depleted populations, conserving and restoring whole ecosystems, and replenishing fisheries through larval and adult spillover [4–6]. However, outcomes of MR establishment have been highly variable, and an active debate has been going on for a couple of decades on what social and ecological impacts might be expected. Empirical evidence of the conservation benefits of MRs has rapidly accumulated over the past years [7–10], but reviews have highlighted limitations of the design and interpretation of empirical assessments [11,12]. Moreover, concerns about whether MRs can increase or even just maintain fisheries catches still remain [13–16]. Establishment of MRs reduces the extent of fishable areas, thereby potentially causing immediate economic losses and hardship to fishers. Thus, the establishment of MRs is often perceived as trading off fisheries yields and profits for conservation objectives. However, recovery of depleted populations within reserve boundaries and larval and/or adult spillover into adjacent areas may increase catches within a few years to decades from reserve establishment, thereby generating both conservation and fisheries benefits [6].

Increased export of larvae and recruits and increased catch per unit effort (CPUE), and in some cases, total catches outside boundaries have been documented for MRs and fisheries closures in coral reefs, temperate rocky reefs, continental shelf and estuarine environments [3,6–10,17–22]. These studies typically show fairly localized effects, with increased catches within hundred metres to a few kilometres from MR boundaries, and have variable results depending on whether increased CPUE around MRs compensate losses associated with closure of fishing grounds (but see reference [23]). However, networks of MRs were implemented only recently [24,25] and their fishery effects are still controversial [15,26,27]. The general lack of data on yield before and after MR establishment and in controlled and experimental settings makes it difficult to evaluate the fisheries benefits of MRs empirically. Consequently, this question has been examined primarily through modelling.

While most modelling analyses predict that well enforced MRs reduce chance of collapse of overexploited fisheries, theoretical work has produced a range of contrasting predictions about the expected fishery benefits of MR implementation [28]. Hastings & Botsford [29] showed that, for sedentary adults with a larval phase joining a common pool and redistributing along an infinite coastline, MRs produce yields equivalent to those from traditional fisheries management. Models including spatial structure of populations, variable larval dispersal and the combination of MRs with fisheries management have shown that MRs may increase and/or stabilize fisheries yields, particularly but not exclusively for overexploited fisheries [16,30–38]. Additional models that considered dispersal only in the juvenile and adult stages (i.e. non-larval) showed that the conservation benefits of MRs are maximized for species with low rates of movement, whereas fisheries yields are enhanced most for those with intermediate dispersal [39].

In contrast to these models, Hilborn et al. [14] found that, if fisheries are already managed by the establishment of a total allowable catch (TAC), the addition of MRs may result in a reduction of both catch and total stock abundance. These results are of concern, because fisheries regulation by setting TACs is very common throughout North America, Australia, New Zealand, Africa and Europe [40–43].

The widespread use of TAC regulation is mainly owing to its simplicity: once the total allowable quota is set, typically on an annual basis as a fraction of the yearly stock, a TAC system does not necessarily require further limitations, for instance, to fishery access (such as vessel size and number), but just needs, in its most basic form, close monitoring of commercial fisheries landings. When the yearly quota is reached, the fishery is closed until the following year when a stock assessment is performed again and a new quota set accordingly. Under this institutional framework, fishers compete with one another for a share of the same harvestable stock. As any fish left by a fisher is going to be caught by others, this inevitably leads to a ‘race-to-fish’ [44] before the fishery is closed when the quota is reached, a process that eventually increases the fishing effort to a level greater than that required for maximizing the yield or profit in a single-owner fishery. This condition is clearly inefficient from an economic standpoint but could still be effective for the conservation of targeted species as long as the TAC is correctly set at or below the maximum sustainable yield (MSY). However, Hilborn et al. [14] showed that the redistribution of fishing effort after MR implementation in a TAC-regulated fishery may lead to a drop not only of catch but also of stock abundance, at least when the harvestable quota is computed as a fraction of the whole stock, outside and inside MRs: with the overcapitalized fishing fleet moving out of MRs and squeezing into non-protected areas, the stock can be so overexploited, despite the TAC guideline, that the increase in population size within MRs cannot compensate for population drop in fishable areas, leading to a net loss of fishery yields.

A critical component that was missing in the original formulation of the Hilborn et al.’s [14] model is the effect of protection on age and size structure, particularly of older, larger and more fecund individuals. One of the most common consequences of fishing is a reduction in maximum and mean body size in exploited populations [45–47]. Conversely, shifts in age and size structure towards larger size classes and older individuals have been commonly documented in MRs across multiple fish and invertebrate species and in different ecosystem types [7,17,18,48–50]. For many fish and invertebrates, fecundity may increase more-than-linearly with body mass or more than the cube of somatic length. Consequently, older, larger individuals may contribute disproportionately to total reproductive output, producing quantities of gametes up to orders of magnitude greater than small reproductive individuals [51–55]. For example, 60-cm-long individuals of red snapper (Lutjanus campechanus) are estimated to produce as many eggs as over 200 40-cm-long individuals combined [54]. Similar relationships between size and fecundity exist for rockfish, abalone and other valuable fisheries species, with the difference between fecundity at size at sexual maturity and fecundity of large old spawners typically an order of magnitude [51–55].

Another relevant component missing in Hilborn et al. [14], as well as in the vast majority (but not all) of modelling studies of the MR impact of fishery yield is the effect of year-to-year environmental variability on fishery output: recruitment to fishery might exhibit wild year-to-year variations [56,57] that can mask differences in performance of alternative fishery management schemes even if monitoring programmes are conducted for many years. It is therefore crucial to account for environmental variability when assessing fishery benefits of MRs [58,59]. Finally, a further aspect that has been largely neglected in the theoretical analysis is whether the TAC should be computed only on the stock outside MRs or on the whole stock inside and outside MRs, and what the conservation and fishery consequences of this decision might be [60].

Theoretical predictions of the socio-economic and ecological consequences of reserve establishment are critically important because they set clear expectations that account for environmental and human circumstances, such as the life histories of species and the fishing pressure outside reserves, and can guide empirical assessments of the impacts of MRs. Thus, we argue that the first step towards resolving uncertainties about the impacts of MRs is to conduct thorough mechanistic explorations, through modelling, of the factors and conditions that may underlie the variable outcomes of reserve establishment. With this goal, we extended the spatially explicit population model used by Hilborn et al. [14] to include environmental stochasticity and population size structure, in particular the reproductive contribution of large spawners protected within MRs. Specifically, our overarching goal was to investigate whether the inclusion of MRs in a TAC-regulated, overcapitalized fishery can improve or decrease fishery yield. Specifically, we asked the following questions: (i) to what extent does the gain in fecundity of old, large individuals present in MRs affect fishery yield in a TAC-regulated fishery in comparison with MSY under conventional management (i.e. with no MRs)? (ii) How are detrimental or positive effects of MRs on populations and fishery yield influenced by combinations of MR configurations (e.g. fraction of protected habitat and number/size of MRs), dispersal distances in larval versus juvenile/adult stages, and ways to estimate the harvestable quota? (iii) How does year-to-year environmental variability affect our ability to detect differences in fishery performance between conventional management and a fishery scheme that includes MRs? We conclude by identifying general guidelines for the design and assessment of MR networks as conservation and fisheries management tools when MRs are combined with existing TAC fisheries regulations. Caveats and limitations of our modelling approach (such as the lack of inclusion of trophic interactions and habitat heterogeneity) are presented in §4.

2. Model structure

Our stochastic model is an extension of the deterministic one [14] for a population distributed across a linear array of S contiguous patches along a coastline. While Hilborn et al. [14] did not account for age/size structure, our model described the dynamics of a population structured in three stage/size classes (j = 1–3) with increasing fecundity. The equations describing population dynamics and management scenarios are reported in appendix S1 and S2 in the electronic supplementary material; here, we summarize the main features of the model. The life cycle is schematically described in electronic supplementary material, figure S1. The backbone of the spatially explicit model is represented by the equations describing the dynamics of the unfished population in the zero-dimensional (non-spatially explicit) case, namely:

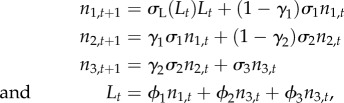where n_j is the number of individuals in each stage/size class, the first stage/size class n₁ includes pre-reproductive individuals (fecundity ϕ₁ = 0), whereas the third stage n₃ represents large spawners whose per capita fecundity (ϕ₃) was assumed to be larger than fecundity (ϕ₂) of average-size adults, σ_L(L) is density-dependent larval survival, i.e. a decreasing function of the reproductive output L, σ_j is the annual survival in size class j, γ_j is the fraction of individuals that move from size class j to the next one every year, and w_j is the mean per capita mass (or price) in stage j.

In the spatially explicit version of the model, the larvae produced in each patch dispersed to contiguous patches according to a Gaussian kernel calibrated by setting the range of dispersal of 90% of larvae produced in any given patch (electronic supplementary material, figure S1). The fraction σ₀(L) of larvae that successfully settled in a patch and recruited to stage 1 the following year was described by a density-dependent, Beverthon–Holt function, namely σ₀(L) = σ_0max/(1 + δL), with σ_0max the maximum survival at low larval density, δ a saturation constant proportional to the strength of the density dependency and L local larval density. Juveniles and adults could also move to adjacent patches according to a Gaussian kernel (see appendix S1 in the electronic supplementary material).

Catchability q of large spawners was assumed to be larger than that of average-size adults (q₃ > q₂) under the hypothesis that larger individuals are targeted more intensively because they are more valuable and/or that fishing gears (e.g. nets, traps) select for larger individuals. We also assumed that stage 1 fish were not exploited (q₁ = 0) either because they were below the minimum legal size or because they were not selected by the fishing gear. Further simulations with q₃ = q₂ to represent fishing mortality caused by non-selective fishing gear, and q₁ slightly larger than zero to represent accidental take of individuals below the minimal legal size provided similar results and did not change the conclusions of this work. Following Hilborn et al. [14], fishers were assumed to concentrate their harvesting effort where they expected higher catch, i.e. where the harvestable biomass was larger [61].

TAC was computed as a fraction 1 − exp(−qE) of the stock biomass in stage 2 and 3 (equation S9 in the electronic supplementary material), with fishing effort E set to E^MSY (unless stated otherwise), i.e. the fishing effort that provides MSY under conventional quota management (without MRs). The implementation of MRs displaced fishing effort outside the protected patches in the fishable ground. Yearly harvest could not exceed the annual TAC. To allow for the representation of the most extreme case, where there is fishery overcapitalization, total effort (TE) was assumed to be larger than that required for MSY under conventional management (i.e. TE > TE^MSY). To contrast our results with those by Hilborn et al. [14], TAC was computed on the whole stock inside and outside MRs. However, the choice of including also the stock protected in MRs, although legitimate [60], is also debatable from a conservation viewpoint. Consequently, we also simulated fishery performance when the TAC was computed only on the stock in the fishable ground (outside MRs) and compared the outcome of these two alternatives with fishery performance under conventional management.

(a). Model parametrization

The model was parametrized so as to represent the population dynamics of species characterized by decreasing mortality with increasing body size and a lifespan between 10 and 20 years, a typical range for many benthic invertebrates and reef fishes. The fertility in the first life stage, ϕ₁, was set to zero. Following empirical evidence of increasing fecundity with size (appendix S3 in the electronic supplementary material), we initially assumed that the large spawner fecundity (ϕ₃) was 10 times larger than fecundity of average-size adults (ϕ₂). This assumption was then relaxed in a sensitivity analysis, and MR performances were assessed also for smaller and larger values of ϕ₃.

Parameters σ_{0 min} and δ of the density-dependent survival were set so that the carrying capacity in each patch in the absence of fishing was normalized to 1000 individuals and the Goodyear compensation ratio (GCR)—the ratio of maximum larval survival at low density σ_0max to survival at unfished natural abundance—was set to 10 (see appendix S2 in the electronic supplementary material). Meta-analysis of stock recruitment data [62] indicates that GCR is in the range 3–50, with likely values for long-lived benthic species between 5 and 20, with larger values typical of species with faster dynamics. A sensitivity analysis was run to assess how model results are affected by assumptions on GCR value. For model parameters reported in table 1, 25% of the spawner biomass at the unfished equilibrium is in the third size class and contributes to about 40% of the reproductive output (pre-settlement larvae).

Table 1.

Default settings for demographic and fleet parameters. The coefficient of variation (cv) of model parameters is reported in parentheses where applicable. The coefficient of variation of the unfished population corresponding to these model parameters is reported in electronic supplementary material, table S1. Electronic supplementary material, figure S3 illustrates a realization of population dynamics over a 100-year period.

name		stage 1	stage 2	stage 3
(a) stage-dependent parameters
σj	annual survival in stage j	0.5 (cv = 20.7%)	0.7 (cv = 10.6%)	0.9 (cv = 3.1%)
γj	transition probability from stage j to stage j + 1	0.1	0.01	—
ϕj	per capita fecundity	0	104 (cv = 30%)	105 (cv = 30%)
rdj	range of dispersal of 90% of individuals in stage j	1 km 90% retention rate (cv = 30%)	1 km 90% retention rate (cv = 30%)	1 km 90% retention rate (cv = 30%)
wj	body-mass (prices) [arbitrary units]	0.2	1	5
qj	catchability (effort−1 y−1)	0	0.1 (cv = 30%)	0.15 (cv = 30%)
(b) other model parameters
σ0max	larval survival at low density	1.986 × 10−3 (cv = 30%)
δ	strength of density-dependence in larval settlement	3.817 × 10−6 (cv = 30%)
GCR	Goodyear compensation ratio	10
rdL	range of dispersal of 90% of larvae	between 5 and 100 km (cv = 30%)
φ	scaling parameter proportional to effort aggregation in the areas of highest biomass	2 (cv = 30%)
TAC	total allowable catch (eqn S9 in the electronic supplementary material)	= (1 − e−fMSY) × biomass in stage 2 and 3 (with a 30% cv in stock assessment)
S	number of contiguous patches of suitable habitat along the coastline (each patch 1 km long)	100

The coastline was divided into 100 patches of 1 km each. Initial density corresponded to that of a stock harvested at MSY. Stochastic simulations were run by drawing each year's values of model parameters from a beta truncated probability distribution function with mean corresponding to the value of model parameters reported in table 1, minimum and maximum value equal to ±50% of the mean value and coefficient of variation set to 30% for all model parameters (unless reported otherwise in table 1). The same sequence of year-to-year environmental variability in the demographic parameters was used to simulate population dynamics under conventional quota management and MR implementation. Additional stochastic simulations by using a bias-corrected lognormal distribution provided similar results. We thus reported here the results obtained by using a truncated beta distribution. Even though the transient dynamics after MR implementation may have relevant fishery impacts [45], in this study, we were specifically interested in contrasting the results of our stochastic simulations with the long-term equilibria of other published models [14,16,35,36]. As a consequence, we discarded transient dynamics after MR implementation and assessed fishery performances (i.e. mean annual catch and biomass) over a 10 year period replicated 500 times to test for differences in fishery output under alternative managements scenarios with and without MRs. Specifically

• (1) We computed the number of vessels TE^MSY that provided the MSY at equilibrium in the absence of MRs and the corresponding fishing mortality f^MSY = qE^MSY.

• (2) We then used f^MSY to compute the TAC_t at time t and simulated the dynamics of an overcapitalized fishery in the absence of MRs with TE = 3 TE^MSY.

• (3) We determined the effects of implementing MRs of different sizes—ranging from a single MR to a network of multiple MRs—with individual MRs ranging between 1 and 50 km wide and covering up to 50% of the coastline (table 2). We calculated TAC_t as the fraction f^MSY of the total stock biomass inside and outside MRs in the second and third size classes. Fishery performances under MR regime were reported in terms of relative increase (or decrease) in catch and stock biomass with respect to MSY under conventional quota management.

• (4) We repeated the analyses at point 3 above by computing TAC_t only on the stock outside MRs.

Table 2.

Optimal size and number of marine reserves as a function of larval dispersal in an overcapitalized TAC-regulated fishery in which the fishing effort is three times that required for MSY in a single-owner fishery. Here, a MR layout (i.e. the combination of number and size of MRs) is considered optimal when it provides the highest catch for a specific level of larval dispersal. The annual TAC is computed by setting fishing mortality at MSY (f = f^MSY = qE^MSY) under conventional quota management (i.e. without MRs) and the fishable stock is either assumed to be the total biomass in size classes 2 and 3 along the coastline (i.e. both outside and inside MRs) or, alternatively, only the biomass in the fishable ground (i.e. outside MRs). Effects of reserves on catch and stock biomass are reported as per cent increment (or decrement, if negative) with respect to those obtained at MSY under conventional quota management, i.e. in the absence of reserves. Statistical significance is computed as the fraction of times in which the mean catch (biomass) over a 10-year span under reserve implementation is greater than that under conventional management. Dispersal range and reserve size are measured in the same units as the coastline, which comprises 100 contiguous 1-km patches (i.e. 100 km). In (a), juveniles and adults are assumed to be sedentary (retention rate = 96% in stages 1–3), whereas, in (b), juveniles and adults are mobile (retention rate = 13% in stages 1–3). All other parameters are as reported in table 1.

range of dispersal of 90% of larvae	larval retention rate (%)	optimal size of protected area (total, %)	optimal no. reserves	mean size of each reserve	TAC computed on fish stock inside and outside reserves		TAC computed only on fish stock outside reserves
					Δbiomass (%)	Δcatch (%)	Δbiomass (%)	Δcatch (%)
(a) sedentary adults (adult retention rate = 96%)
5	26	40	40	1	16 ± 7.8**	8.9 ± 7.2† (n.s.)	53 ± 12**	−12 ± 7.2† (n.s.)
10	13	38	20	1.9	22 ± 10**	13 ± 9*	57 ± 14**	−11 ± 7.8† (n.s.)
20	7	36	10	3.6	28 ± 12**	17 ± 11**	62 ± 15**	−9.2 ± 8.5† (n.s.)
50	3	36	4	9	34 ± 11**	20 ± 11**	66 ± 15**	−10 ± 8.4*
100	1	36	2	18	29 ± 11**	21 ± 11**	59 ± 14**	−13 ± 7.3*
(b) mobile adults (adult retention rate = 13%)
5	26	40	24	4	0 ± 1.5 (n.s.)	0 ± 1.4 (n.s.)	42 ± 9.2**	−8 ± 6.22 (n.s.)
10	13	40	12	5	1.4 ± 3.1 (n.s.)	0 ± 2.9 (n.s.)	48 ± 11*	−10 ± 6.5† (n.s.)
20	7	41	6	6.6	3.9 ± 2.2*	3.4 ± 2.0*	44 ± 9**	−13 ± 5.5† (n.s.)
50	3	41	2	20	24 ± 10**	13 ± 9*	63 ± 15**	−15 ± 7.5*
100	1	40	1	40	39 ± 14**	23 ± 13**	74 ± 19**	−14 ± 8.9*

*0.01 ≤ p < 0.05; **p < 0.01; ^†(n.s.) 0.05 ≤ p < 0.10; (n.s.) p > 0.

Simulations of fishery performance with and without a MR network were run under alternative assumptions on mean larval dispersal distances, ranging between 5 and 100 km to represent a variety of reef fish and invertebrate species [63]. First, we considered species with fairly sedentary adults (90% retention rate in each patch) and then species with juvenile and adults that move. For each case, we investigated the effects of different spatial configurations of the protected area, that is, a single large MR versus a network of smaller MRs. We then simulated long-term fishery performances as a function of (i) large spawners' fecundity; (ii) GCR; and (iii) increasing fishing mortality with resect to f^MSY. Simulations were run in Mathcad 15 PTC™.

3. Results

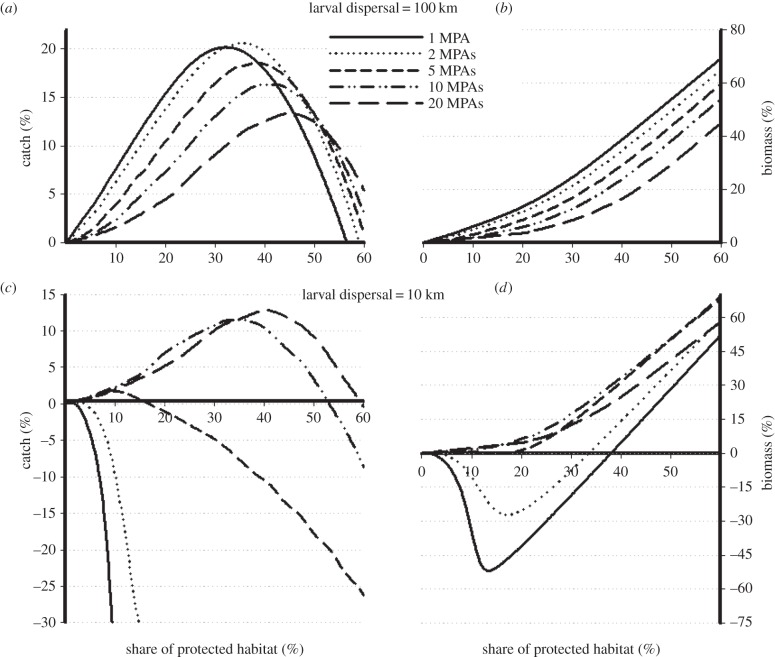
Simulations show that the implementation of an MR network in a TAC-regulated, overcapitalized fishery result in increased, equivalent, or decreased fishery yields and stock biomass depending upon assumptions on the spatial configuration of the MRs network, the extent of larval dispersal and adult movement, and whether the TAC was computed over the whole stock (inside and outside the MRs) or only in the fishable ground (see examples in electronic supplementary material, figures S3–S5). For species with long larval dispersal and sedentary adults, fishery yields with MRs exceed MSY under conventional management for a wide range of protection levels and regardless of the number and size of individual MRs (figure 1a), so long as the TAC is computed on the whole stock inside and outside the MRs (table 2). Catch peaks when slightly more than one third of the fishable ground is set aside from fishing in two or four reserves (figure 1a). Biomass increases slowly at first and then more rapidly with the level of protection, and is larger for fewer MRs (figure 1b).

Figure 1.

Effects of marine reserves on the performance of a TAC-regulated fishery, measured in terms of incremental benefit (or loss, when negative) in mean catch and stock biomass with respect to a conventional TAC-regulated fishery (i.e. with no MRs) at MSY. In (a,b), larval dispersal range is 100 km; in (c,d), larval dispersal is 10 km. The fishery is overcapitalized (TE = 3TE^MSY) and here TAC is computed on the whole stock inside and outside reserves. All other parameters are as in table 1.

For shorter larval dispersal (90% of larvae settle within 5 km from each side of their parental patch), the net fishery benefits are roughly half that in the case of high dispersal and peak only when a significant portion of the fishable ground (from 30% to 50%) is set aside in 10–20 MRs. In the case of only one or two large MRs, not only does the catch drop dramatically (figure 1c), but also overall biomass shows large decreases (−45%) with respect to conventional fishing (figure 1d) when the TAC is computed on the whole stock inside and outside MRs. The negative effect on stock biomass of a single wide reserve is removed either when protection is enforced through several small MRs (figure 1d) or when the TAC is computed only on the stock in the fishable ground (table 2a). In the former case, the fishery under MR regime could still provide catches exceeding or equivalent to MSY under conventional management. In the latter case, however, despite large conservation benefits, the catch is systematically lower than MSY under conventional management.

Adult movements always result in a reduction of fishery benefits of MR implementation, even though yields could still exceed MSY under conventional management (table 2b and electronic supplementary material, table S2b). Yet, the marginal benefit of including MRs in the fishery management scheme with respect to conventional management could be partially recovered by implementing fewer larger MRs (electronic supplementary material, table S2c).

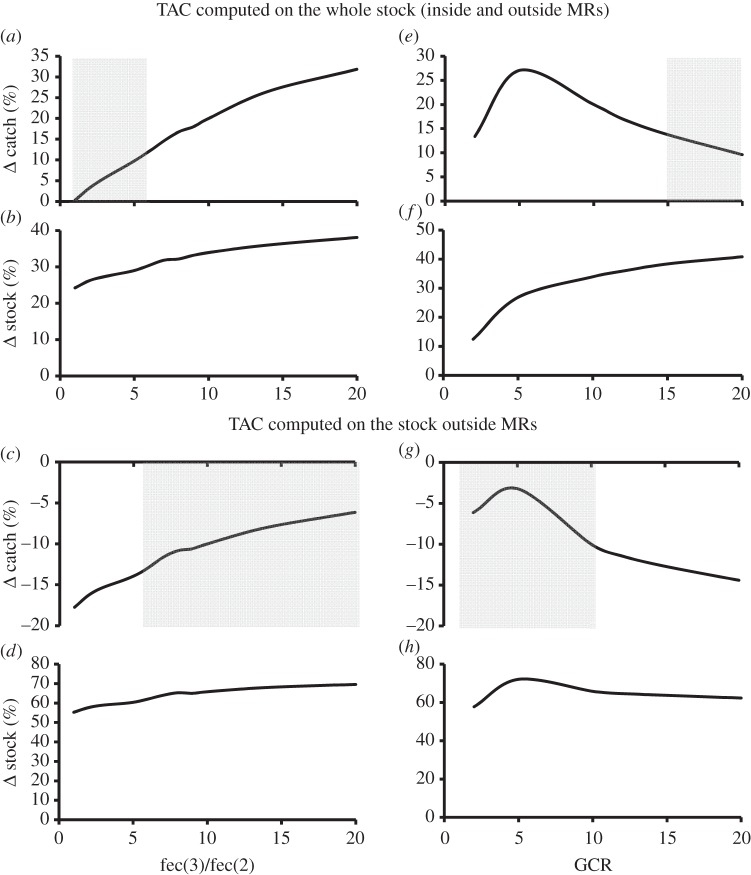
A sensitivity analysis showed that fishery performance is proportional to the fecundity of large spawners (figure 2a,c). For the level of year-to-year variability included in the stochastic simulations, differences in performances with respect to conventional management, although positive, are not significant when large spawner fecundity is up to five times greater than that of average-size adults (figure 2a). The marginal benefit of including MRs in a TAC-regulated fishery peaks for GCR equal to 6 (figure 2e,g) and is lower either for very low GCR (when the settlement probability is so low that spawners within MRs are unable to supplement recruitment outside MRs) and for medium-to-high GCR (when a few reproductive individuals outside MRs are still able to produce a high number of settlers in spite of population overexploitation, thus making large spawner contribution to recruitment less important).

Figure 2.

Effect of fecundity of large spawners (a–d) and Goodyear compensation ratio (e–h) on fishery performance of a TAC-regulated fishery including MRs, relative to a conventional TAC-regulated fishery (without MRs) at MSY. Large spawner fecundity is normalized with respect to the fecundity of average-size adults. Shaded areas represent values of fecundity or GCR where the differences between fishery performance with MRs and under conventional management are not significant (p ≥ 0.05) at the level of environmental variability simulated in the model. All the other parameters as in table 1, with larval dispersal range equal to 50 km, adult dispersal 1 km, and 36% of the fishing ground set aside for protection in four MRs of 9 km each.

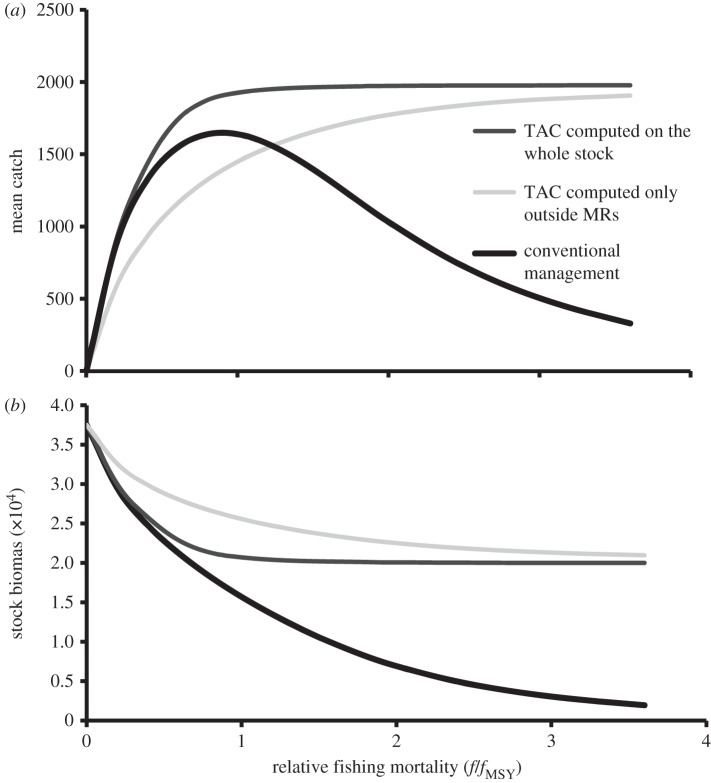
Unbiased errors in stock assessment have no effect on a fishery including MRs compared with conventional management (electronic supplementary material, table S2d). On the contrary, a systematic tendency to overestimate the stock and/or setting quotas larger than estimated by using f^MSY boosts the benefits of including MRs in the fishery's management with respect to conventional management (electronic supplementary material, table S2). Figure 3 shows that high fishery yield and stock biomass can be maintained in fishery schemes including MRs even when fishing mortality largely exceeds that at MSY, whereas catch and stock drop in a conventional TAC-regulated fishery under high fishing pressure. The probability that the population declines below 20%, or collapses below 10% of the unfished carrying capacity over a 10-year period, increases with increasing fishing pressure under conventional management but it is minimally affected by fishing pressure when the management scheme includes MRs (electronic supplementary material, figure S6). The coefficients of variation of both catch and biomass are substantially lower with the implementation of MRs than under conventional management (electronic supplementary material, table S1). Further simulations show that the general trends reported in figures 1–3 are not affected by alternative assumptions regarding the magnitude of year-to-year environmental variability.

Figure 3.

Mean harvest and biomass as a function of fishing mortality (scaled with respect to fishing mortality at MSY) for alternative management regimes. Continuous black line: conventional management (with no MRs); dark grey line: fishery management scheme, including a network of MRs with TAC computed on the whole stock inside and outside MRs; light grey line: fishery management scheme including a network of MRs with TAC computed only on the stock outside MRs. All the other parameters as in table 1, with larval dispersal range equal to 50 km, adult dispersal 1 km, and 36% of the fishing ground set aside for protection in four MRs of 9 km each.

4. Discussion

Our analysis showed that the ultimate effect of including MR network in a TAC-regulated, overcapitalized fishery can be positive, neutral or negative with respect to fishery performance in a conventional quota system, depending upon the specific set of assumptions used to simulate the system and, in particular, by a combination of life-history traits, levels of larval dispersal, adult movements, the extent of fishing ground set aside in reserves, the spatial layout of the MR network and whether the TAC is estimated on the whole stock or only on the stock outside MRs. This context-dependent variability of outcomes makes empirical documentation of reserve effects challenging. However, model simulations provide testable predictions about when positive ecological and social (e.g. fisheries) impacts of MRs might be expected, and provide some guidance on the power of empirical assessments that might be required under different environmental variability regimes.

Conclusions that MRs invariably lead to a drop in fishery yields and loss of jobs because of the reduction of fishable ground or, conversely, to fishery and conservation benefits, thanks to larval and adult spillover are not supported by the results of our analysis. We showed that a well-designed network of MRs can increase yields above MSY under conventional quota-based management if (i) protection fosters the recovery of old, large and fecund spawners that contribute disproportionally to recruitment with respect to average-size adults; (ii) pelagic larval duration is long enough to allow for dispersal over significant distances (relative to the size of reserves); (iii) 30–40% of the suitable fishing ground is protected in MRs whose individual size is proportional to larval dispersal; and (iv) the annual TAC is computed on the whole stock, inside and outside the MRs. If one or more of these criteria are not met, the inclusion of MRs can still provide catches that are comparable to MSY under conventional management, but not greater.

In agreement with other theoretical analyses [31,36,64,65], adult movement reduces the effectiveness of MRs by increasing fishing mortality. Yet, in contrast with qualitative and modelling conclusions [15,66], we found that, when the TAC is computed on the whole stock, MRs can still provide conservation benefits compared with conventional management even when adults move across reserve boundaries (table 2b and electronic supplementary material, table S2b). Moreover, even though we did not simulate the effect of protecting nursery areas in this work, other studies [33,36,65] have shown that highly mobile species can still benefit from MRs if these include nursery areas, with maximum benefits if MRs include both a spawning and nursery area.

Our analysis showed that, in very specific circumstances (limited larval dispersal and protection of large fractions of the fishing ground in just one or two reserves), MRs can lead not only to a drop in fishery yields, but also in the whole fish stock compared with a conventional TAC-regulated fishery at MSY, a fact that is generally not recognized by a majority of theoretical papers on the effects of MRs on fishery performance—with the notable exception of Hilborn et al. [14]. This paradoxical outcome—MRs are expected to protect target stocks, not to further drive down their decline—occurs because large spawners located in the middle of wide MRs are unable to supplement recruitment to the fishing ground owing to limited larval dispersal. At the same time, the fishery, squeezed into a smaller fishing ground, depletes the stock in an attempt to reach the quota, and the low adult densities left in the fishable ground are unable to provide an effective contribution to recruitment. Ultimately, stock depletion in the fishable ground is so extreme that it cannot be compensated for by the increased abundance of large spawners within the MRs and this results in a decline of the overall stock below the level achieved by conventional TAC-regulated fisheries at MSY (i.e. with no MRs).

The negative effect on biomass of MRs in a TAC-regulated fishery can be reversed by either dividing the protected area into several smaller MRs or by computing the TAC on the stock outside MRs (or both). In the first case, spawners within small MRs can still supplement recruitment outside the reserves and thus contribute to maintaining yields, even though in this case fishery outputs may barely exceed MSY under conventional management. In the second case, computing the TAC only on the stock in the fishable ground leads to a remarkable increase in biomass with respect to conventional quota management, but at the cost of significant loss in fishery yields with respect to conventional management. Therefore, in the case of species with limited larval dispersal, the decision of whether the annual TAC should be computed on the whole stock or only on the portion outside MRs should be determined on the basis of the relative importance of fishery versus conservation goals and the opportunity of trading off one with the other, in addition to a number of other considerations, including social goals and circumstances, food security and economic drivers that are beyond the scope of this study.

For species with high larval dispersal, there exists the possibility of achieving a win–win solution in achieving both conservation and fisheries goals as long as the set of conditions listed above are met. Among these conditions, one that is intrinsic to the biology of the target species—and thus cannot be changed or manipulated through management actions, as in the case of MR spatial configuration—is the incremental gain in reproductive output of old, large spawners with respect to average-size adult fish. If the increment in fecundity with size is small, spawners within MRs are unable to produce the surplus of larvae required to supplement recruitment to the overharvested stock outside MRs. Our results are in agreement with empirical studies that suggested that the large individuals protected in MRs may contribute disproportionately to reproductive output and export propagules to the fishing ground [22,48,50,54,67].

Therefore, the key mechanism for the increase in fishery yield above MSY under conventional management is given by a combination of the disproportionate gain in fecundity of large spawners with intermediate levels of density-dependent recruitment. In fact, thanks to the saturating shape of density-dependent settlement function (eqn S4 in the electronic supplementary material), recruitment within reserves remains high in spite of larval dispersal from MRs to adjacent areas, as high local production of larvae supported by old, large, high fecund spawners within reserves can sustain population density at levels close to the undisturbed (non-fished) carrying capacity. At the same time, larval export from MRs to fishing grounds provides a substantial contribution to local recruitment in areas subject to harvesting where the population is severely depleted and its reproductive capacity impaired. This might explain why, in contrast to our more general predictions, Le Quesne & Codling [16] found—by using an age-structured, spatially explicit model of the North Sea cod, Gadus morhua—that no network of MRs can provide yields larger than MSY under conventional management. In their model, fecundity grew approximately linearly with body mass but somatic growth was limited by the use of a Von Bertalanffy (VB) growth model that did not account for plasticity in body size, i.e. by the possibility that at any given age some fish may be larger than the mean body size at that age. Therefore, the increase in fecundity of older individuals within MRs was curbed in their model by the VB asymptotic length. This might explain why they found that, unless the stock is overfished, management schemes including MRs have generally lower performances than conventional management, in agreement with our results that the net fishery benefit of MRs is lost when gains in fecundity of old, large individuals are small.

An important outcome of our analysis is that year-to-year background environmental variability may mask the effects of MRs. Therefore, although catch projections developed with deterministic models may support the hypothesis of a net fishery benefit deriving from the implementation of a suitable network of MRs with respect to conventional management [36,37] or to a net loss with respect to a conventional TAC-regulated fishery at MSY [16], in practice, it is difficult to detect significant changes in fishery yields, if not through a highly replicated BACI experimental design [68]. It is important to note that maximizing yield is often not the only goal of implementing MRs. In agreement with previous models [31,36,69], our results suggest that MRs—and more broadly, spatial management that includes also no-take areas—can reduce the risk of fishery collapse, thereby providing some insurance against the tendency to bargain for high quotas and systematically overharvest the stock [42,43].

Our model was kept deliberately simple and, as such, it is not exempt from limitations. In particular, our theoretical analysis assumed that MRs are evenly spaced through a homogeneous fishing ground. Therefore, we did not account for heterogeneities in habitat quality, which might affect recruitment abundance and distribution [38], or for other ocean uses that might constrain the placement of MRs [70]. In assessing fishery performance, we did not account for transient dynamics [45] or the trade-off between short-term costs and long-term benefits [71], the cost of fuel as a function of distance of fishing grounds from harbours [58], the cascading and often nonlinear effects of trophic and non-trophic species interactions [72], the consequences of advective larval transport driven by local oceanographic conditions [73], the role of larval behaviour in determining dispersal distance [74] and the effect of protecting nursery or spawning areas of mobile species [33,36,65]. In addition, model parameters and their variance were not tuned to time-series data through formal calibration, and thus we assessed their relevance through a simple sensitivity analysis. Our modelling exercise also neglected other biological traits that might enhance the reproductive output of large spawners, such as increased size, survivorship and growth rate of larvae produced by large spawners [75]. Therefore, the results of our analysis should be considered with these caveats in mind and by no means taken as prescriptive in terms of specific size and placement on MR networks.

Despite the simplicity of our modelling approach, and in agreement with other theoretical studies [34–36,71,76], our analysis suggests that networks of MRs can be a robust strategy for the conservation and supplementary management of TAC-regulated, overcapitalized fisheries allowing maintenance of (and in some cases even enhancement of) fish stock and fishery yields with respect to MSY under conventional management. Importantly, models link the variable outcomes of MRs to specific life histories of species and management settings, providing a framework for designing and conducting empirical assessments of the impacts of reserves on exploited populations and fisheries.

Authors' contributions

G.A.D.L. and F.M. conceived the problem and designed the study. G.A.D.L. developed the computer program and ran the simulations. G.A.D.L. and F.M. wrote the manuscript.

Competing interests

We declare we have no competing interests.

Funding

G.A.D.L. was partially supported by MIUR-PRIN2008 grant (award no. 2008E7KBAE), by EU grant ‘Towards COast to COast NETworks of marine protected areas' (CoCoNet) and by the US NSF-OA Program (award no. OCE-1416934). F.M. was supported through the US NSF-CNH Program (award no. DEB-1212124).