Exploration of metastability and hidden phases in correlated electron crystals visualized by femtosecond optical doping and electron crystallography

Using femtosecond photodoping and crystallography to explore metastable and hidden quantum phases in tantalum disulfide.

Abstract

Characterizing and understanding the emergence of multiple macroscopically ordered electronic phases through subtle tuning of temperature, pressure, and chemical doping has been a long-standing central issue for complex materials research. We report the first comprehensive studies of optical doping–induced emergence of stable phases and metastable hidden phases visualized in situ by femtosecond electron crystallography. The electronic phase transitions are triggered by femtosecond infrared pulses, and a temperature–optical density phase diagram is constructed and substantiated with the dynamics of metastable states, highlighting the cooperation and competition through which the macroscopic quantum orders emerge. These results elucidate key pathways of femtosecond electronic switching phenomena and provide an important new avenue to comprehensively investigate optical doping–induced transition states and phase diagrams of complex materials with wide-ranging applications.

INTRODUCTION

Phase transitions are among the most fascinating properties of many-particle systems, and they exhibit common features across very different scales ranging from exotic forms of nucleus (1) to structured water (2) and galactic evolution (3). Whereas classical phase change phenomena have been well classified, understanding the mechanisms of the phase transitions in quantum many-body systems is an emerging field. Layered transition metal oxide and chalcogenide compounds can exhibit multiple quantum phases, including Mott insulator, superconductor, and spin or charge density wave (CDW) states that competitively emerge with subtle physical tunings, including applying heat and doping (4). When multiple electronic and structural orders are entangled, giant responses in the electronic and lattice degrees of freedom may occur, as occurs in the resistivity change by several orders of magnitude in doping (or temperature)–induced phase switching in manganite, magnetite, and vanadium oxide (5). Identifying the origins for the competitive or cooperative emergence of various functional states and their tuning by doping, temperature, strain, or electrostatic and magnetic fields is of vital importance for elucidating the basic physics and leveraging their enormous technological potential. 1T-TaS₂ is viewed as a prototype material for investigating the emergence of quantum orders in correlated electron systems (6) because, despite its relative compositional simplicity, it exhibits an assortment of intriguing electronic phases (as reproduced in Fig. 1A) including various textured CDW orders (7), as well as a recently reported superconducting (SC) phase under chemical doping (8, 9) and pressure (6, 10). 1T-TaS₂ is also the only transition metal dichalcogenide (TMD) material to develop the Mott-insulating (MI) ground state, which coexists with the CDW state (6, 11), as occurs in the phase diagrams of several heavy fermion and high-temperature superconductor compounds.

Fig. 1. CDW phase transitions in 1T-TaS₂ induced by thermodynamic (temperature, chemical doping, or applying pressure) and femtosecond optical doping.

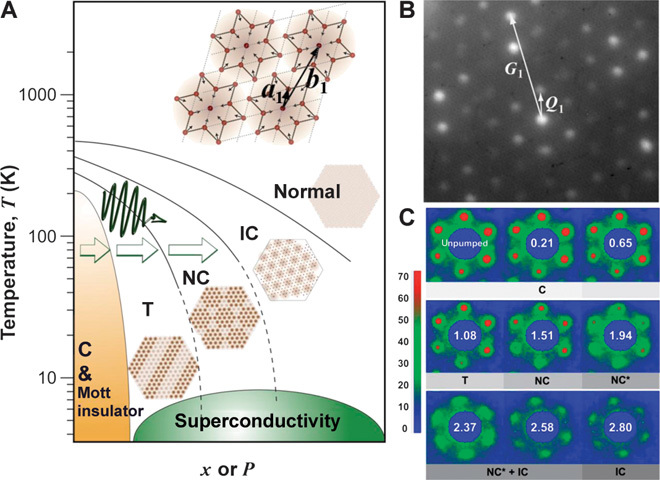
(A) Generic phase diagram of 1T-TaS₂ under various physical domains (temperature, doping x, or pressure P) reconstructed based on (6, 8–10). The CDW phase evolution can be characterized by the changes in the hexagonal CDW diffraction peaks at reciprocal vector Q (upper right corner). Upper right shows the SD 13-atom cluster representing the unit cell of C-CDW in real space. The lattice distortion within each star is coupled with a strong charge density redistribution. The angle between the CDW vector b_i and lattice vector a_i is 13.9°. (B) Scale-up view of the ultrafast electron diffraction pattern, showing the hexagonal diffraction patterns of C-CDW (Q) surrounding the lattice Bragg peaks (G). (C) Photoinduced phase transition from C-CDW (unpumped) all the way to IC-CDW. The number denotes the absorbed photon density n_λ in the unit of nm⁻³. Among the phase transitions, the intensity of CDW reduces approximately by half, and Q rotates 13.9° from C-CDW to IC-CDW.

The exact nature of the emergence of the various CDW textured states and their connection to unique electronic properties such as a pseudogap (12, 13), polaronic conductivity (14), and superconductivity, are open questions in current condensed matter physics research (6, 8, 10, 14–16). The angle-resolved photoelectron spectroscopy (ARPES) on pristine (12, 13) and doped TaS₂ (8) clearly identified coexistence of a Mott-Hubbard gap and a nesting-driven CDW gap in different pockets within the Brillouin zone (Γ and M-K, respectively). The gap of ~0.4 eV (17) at Γ in the low-temperature state (C phase) was explained in terms of Mott physics because such a gap was found to collapse after the insulator-metal transition (IMT) upon heating, whereas the gap at M-K persists until melting of CDW at higher temperatures (8). Theoretical approaches to reproduce the energy gap used a single-band Hubbard model with an intrasite Coulomb repulsion larger than the bandwidth, producing an MI state (18–21). However, this picture has recently been questioned by Darancet et al. (22), who used density functional theory (GGA+U) to demonstrate that the C phase with periodic 1c vertical stacking is a one-dimensional (1D) metal (gapless in the vertical direction) with a bandwidth of 0.45 eV and a renormalized single-site Coulomb repulsion of 0.18 eV. This drastic renormalization of the Coulomb repulsion parameter is due to the delocalized nature of the Wannier orbital over the reconstructed CDW unit cell [the so-called Star-of-David (SD)], which gives rise to a metallic band at the Fermi energy. This band is extremely sensitive to different vertical stackings of the SD motif as seen in the calculations by Ritschel et al. (23) and Portmann (24). Introducing stacking faults or disorder causes the bandwidth of the metallic band to narrow, and it is possible that Mott physics along with disorder has to be invoked to understand the low-temperature and low-energy experiments. Furthermore, because of the limitation of GGA and GGA+U in describing the low-energy physics of strongly interacting systems, the origin of the photoemission gap (whether a Mott gap or a Peierls stacking gap or some mixture as derived from the interplay between electron-electron and electron-phonon interactions) is still uncertain.

Although there have been no direct time-resolved structural investigations that detail the structural pathways and dynamics, the steady-state results suggest that pattern formation above the IMT is controlled by the kinetics of domain walls, which increase in size (25, 26), resulting in smaller C domains. The correlated gradual changes in the conductance and CDW wave vector (Q) upon warming indeed support the phase segregation view that metallicity arises primarily within the domain wall region, whereas locally, the C domain remains insulating. Meanwhile, the most recent crystallographic study on pressure-induced CDW transitions indicates that the domain walls in the textured near-commensurate (NC) phase are only a few atoms thick (10), suggesting strong phase coherence between the domains as also pointed out by the ARPES study on doped TaS₂ (8). These new results lead to an open debate on whether transport in the NC-CDW is mediated predominantly through interdomain discommensurate regions (6) or nonexclusively via a mid-gap shallow band at Γ created by deconstructing the Mott state (8, 10). The latest experiments on pressure-driven or chemically doped TaS₂ demonstrate that the physics of formation of various textured CDW phases above the IMT is more involved and raises the possibility that different CDW structures may develop through heating, or modifying the interaction energy, such as through doping or applying pressure. Notably, a hidden metallic state (H state) of TaS₂ was discovered recently by shining a single intense femtosecond laser pulse on its insulating ground state (27).

Complex quantum phases emerge because of strong coupling and competition between electronic, lattice, spin, orbital, and other degrees of freedom. At equilibrium, it is difficult to disentangle the competing interactions and entropic effects; however, ultrafast pump-probe experiments often temporally isolate the various degrees of freedom while also revealing hidden states that have not been accessible using conventional experiments (27–30). This report introduces high-fidelity approaches that combine femtosecond electron crystallography and controlled femtosecond optical excitation to reveal the ultrafast structural dynamics of complex quantum phases in response to photonic tuning of interaction energy as an alternative route to chemical doping or applying pressure (19, 28, 31), but without uncontrolled effects due to strain or disorder. We have performed the first comprehensive study of photoinduced phase transitions (PIPT) in 1T-TaS₂ that lead to a succession of metastable phases. The different nature of photoinduced phases is articulated on the basis of controlled mid-infrared pulses for the differentiation of thermal and interaction-driven processes. We also identify a hidden state and the rugged energy landscape that defines more distinctly the various textured CDW phases in the interaction-driven transitions in contrast to their counterparts under thermodynamic conditions. A unique ultrafast two-step switching process is found, which we argue is driven by photodoping-induced changes of the energy landscape. Ultrafast switching phenomena driven by femtosecond photodoping provide a unique approach to understanding the complex energy landscape of electronic crystals and enable new opportunities for stable, controllable, ultrafast switching.

In strongly correlated systems, the electrons often have the tendency to self-organize into an ordered insulating state at low temperatures, which, in the case of 1T-TaS₂, is intertwined with formation of a commensurate CDW (C-CDW). The periodic density wave order is composed of the SD 13-atom building block (see inset of Fig. 1A), where, driven by the polaronic effect, the 6 Ta atoms in each of the two rings move inward to the central 13th atom, where the valence charge density is the highest. Above T_C^(T) ~223 K, the commensuration energy is reduced, giving rise to spatial inhomogeneities—first into a stripe phase where the CDW reconstructs into a triclinic incommensurate ordering (T-CDW) (32, 33) and then into an NC phase with hexagonally ordered domains (NC-CDW) above T_C^(NC) ~280 K (25). In these textured phases, scanning tunneling microscopy (25, 34) determined that the domain walls are discommensurate regions that completely dissolve above T_C^(IC) ~350 K, where a new domain-free state arises with incommensurate CDW order (IC-CDW). Far-field probes, such as x-ray and electron diffraction, can clearly determine the various CDW phases through their respective wave vector (Q) displaying distinctively different orientation angle Φ with respect to lattice G (see Fig. 1B, Φ in C-CDW and IC-CDW is 13.9° and 0°, respectively). The transitions are generally strongly first order, exhibiting strong hysteresis (see Table 1). In particular, the T phase (32) is hysteretic and occurs only upon heating, and has distinctive anomalies at ~280 K in resistivity, thermal expansion (35), and specific heat (36) from the NC phase.

Table 1. The different CDW phase in 1T-TaS₂ and their manifestation in terms of the CDW orientation angle Φ (the angle between the CDW unit cell and that of the unreconstructed atomic lattice) and CDW amplitude.

The values of transition temperature and angle associated with different CDW phases under the thermodynamic conditions (cooling and warming) are taken from (7, 35). The values of the transition optical dose and the normalized CDW intensity Î_CDW are extracted from the metastable phases obtained at +20 ps in the optically induced phase transitions characterized by femtosecond electron crystallography.

Thermally driven
Phases	T on cooling (K)	T on warming (K)	Φ on cooling (°)	Φ on warming (°)
C-CDW	<183	<223	13.9	13.9
T-CDW	–	223 < T < 280	–	13.0 – 12.3
NC-CDW	183 < T < 347	280 < T < 357	10.9 – 12.3	12.3 – 11.5
IC-CDW	347 < T < 543	357 < T < 543	0	0
Normal	>543	>543	–	–

Optical doping–driven (TB = 150 K)
Phases	Optical density, nλ (nm-3)	Φ (°)	Normalized intensity, ÎCDW
C-CDW	<0.65	13.9	1
T-CDW	0.65 < nλ < 1.38	13.7	0.91
NC-CDW	1.38 < nλ < 1.78	12.9	0.78
NC*-CDW	1.78 <nλ < 2.38	11.7	0.60
IC-CDW	>2.38	0	0.45

We elucidate the stable and transitory many-body states of 1T-TaS₂ induced by femtosecond optical doping using two different laser wavelengths of λ = 800 and 2500 nm, with photon energies E_λ of 1.55 and 0.5 eV, respectively. The initial electronic state in the experiments is deep inside the insulating regime from 25 to 150 K. Given that the band is strongly gapped (0.3 to 0.4 eV) (8) over the entire Brillouin zone in the C phase, the two pump photons deliver distinctly different excess energy Δ = E_λ − E_g to drive the initial electronic temperature T_e. Meanwhile, under the same absorbed pump fluence, the long wavelength photons are significantly more efficient in generating charge carriers within the gapped bands, which can effectively modify the electronic state nearly spontaneously, given the strongly interacting nature of this system. A pioneering ultrafast electron diffraction study initiated in the NC phase (37) provided evidence of strong cooperativity between the quenching of the CDW order and the electronic order in an optically driven transition, but did not observe the formation of the IC phase. Here, we comprehensively investigate phase transitions into all of the CDW phases, elucidating both the temperature-driven dynamics typical of the high-energy pump pulse near T_C and the nonthermal behavior evidenced by the mid-infrared pump pulse at low temperatures. The temperature–optical doping phase behavior is characterized by tuning the electron temperature T_e, optical doping x, and crystal base temperature T_B for both pulse energies.

We prepared thin flakes of TaS₂ (30 to 50 nm) from a 1T-type bulk single crystal by the Scotch tape method widely used in exfoliating 2D materials (see the Supplementary Materials for details). The samples were then transferred onto a transmission electron microscopy (TEM) grid as free standing to avoid strain on the crystals. The sample grid is firmly clamped onto a variable temperature cryostage docked inside an ultrahigh vacuum chamber for ultrafast electron diffraction investigation (38). Our pump laser was spectrally tuned using an optical parametric amplifier, delivering 50-fs mid- to near-infrared pulses, which were set to 400-μm full width at half maximum (FWHM) so the sample flake could be illuminated homogeneously. The femtosecond electron beam was microcollimated to probe a single flake and produced very high quality single-crystal diffraction patterns (see Fig. 1B). The ability to sample a large reciprocal space all at once by high-energy electrons (30 keV) provides a unique opportunity to study the interplay between lattice and electronic ordering through respective Bragg and satellite features (39). As clearly demonstrated in Fig. 1C, the photoexcitation resulted in a succession of angular and amplitude suppressions, driving the C phase in well-defined steps into the IC phase (corresponding values of Φ and normalized intensity Î_CDW are listed in Table 1). Here, the CDW-induced lattice modulation amplitude A, a function of the scattering intensity at Q [Α ∝ I_CDW(Q)^1/2] (39), is particularly well characterized, because the negative time data (t < 0) provide an internal reference frame for judging the pumped state (t > 0) within iterative pump-probe cycles, strongly alleviating the instabilities encountered over steady-state heating or cooling.

RESULTS

We first studied the laser pump fluence (F)–dependent conversion of the C phase state at T_B = 150 K into various textured phases. We found that at times greater than 20 ps after the initial pump pulse, the sample had reached a quasi-equilibrium state, so we chose the time delays of −10 and +20 ps as the reference states before and after the laser pump. The laser pump repetition rate (≤1 kHz) corresponds to a much longer time scale. We monitored the relative change of the CDW peak intensity [I_CDW(+20 ps) − I_CDW(−10 ps)]/I_CDW(−10 ps) as well as rotation ΔΦ, and used them to map out the fluence-dependent phase behavior. First, we describe the responses to the 800-nm pump laser pulse. The transitions follow a succession of jumps in both peak intensity and rotation angle (Table 1 and Fig. 2) as shown at F = 1.8, 3.6, and ~6 mJ/cm². They are driven by near-infrared (800 nm) photons corresponding to absorbed energy density ΔH = 1.1, 2.1, and ~3.5 eV/nm³ (see the Supplementary Materials for details). This succession of changes mimics the thermodynamic transformations upon warming, in which clear first-order transitions can only be identified between C to T and NC to IC, whereas optically induced transition visibly shows discrete jumps in all cases, assuming that the optically driven pathway follows similar topological orders. The threshold (1.8 mJ/cm²) for leaving the C phase is close to the threshold for melting the Mott state identified by the latest ultrafast photoemission study using the near-infrared pulses (40). Below this threshold, the quenching (partial) of the gap is reversed within 680 fs (19), suggesting the existence of a structure bottleneck in entering the metallic phase. At this threshold, the electronic temperature reaches 3000 K initially (40), and the energy transferred to the lattice is similar to heating the lattice to above T_C, suggesting that the behavior may be described using thermodynamic reasoning.

Fig. 2. Phase transitions driven by heating and femtosecond optical pumping using near-infrared (800 nm) and mid-infrared (2500 nm) photons.

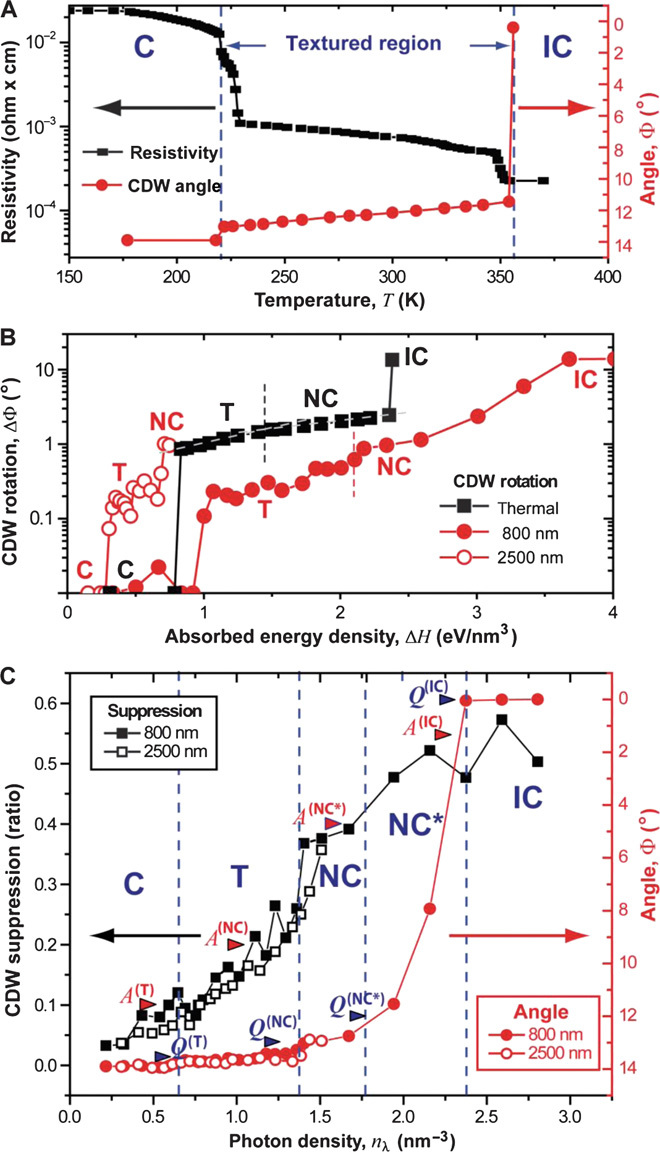
(A) Transitions of 1T-TaS₂ upon heating, showing complementary changes in the resistivity and the CDW orientation angle φ extracted on the basis of electron diffraction (35). (B) Comparison between the thermal and optically induced changes of ϕ over absorbed energy density (see the Supplementary Materials for details). The temperature of 1T-TaS₂ is at 150 K initially. (C) Optically induced evolution of CDW states characterized by CDW suppression (in ratio, based on unperturbed CDW intensity) and CDW angle Φ at various absorbed photon density for two different pumps: 800 and 2500 nm.

However, for the mid-infrared (2500 nm) pump, we find that the optically driven phase transitions in 1T-TaS₂ are nonthermal. To demonstrate this, we study the fluence-dependent phase change behavior for the mid-infrared pulse and compare it to the phase behavior described above for the 800-nm pump. To make a quantitative comparison, we convert fluence-dependent maps at the two wavelengths into absorbed energy density maps (see Fig. 2B) which clearly show that the critical energy densities for inducing various quasi-equilibrium phases are very different for the two laser wavelengths. The black curve is the reference curve of equilibrium measurement reconstructed from Fig. 2A in log scale, where the emergence of NC from T can be seen from the slope change (35). For comparison, the horizontal axis is converted into absorbed energy (ΔH) on the basis of integration of specific heat (see the Supplementary Materials). This result shows that the energy density required for phase transitions with 800-nm photons is slightly higher than the thermodynamic value, whereas that with 2500-nm photons is much lower.

To understand the effect of photodoping, we convert the absorbed enthalpy (ΔH) into photoinduced carrier density n_λ (n_λ = ΔH/E_λ, assuming that each absorbed photon generates an electron and a hole). With n_λ as the x axis, the two curves for the 800- and 2500-nm pump pulses now agree very well (see Fig. 2C), showing that to induce the same state with different infrared photon pumps, it is necessary to induce the same density of charge carriers rather than to provide the same enthalpy change. This strongly suggests that the system is driven by charge carrier doping, rather than through thermal pathways. Moreover, photodoping may generate similar effects as those produced via chemical doping or applying pressure. The upper valence band is the reconstructed Ta-5d orbital with the charge density located around the center of the SD and is localized, whereas the lower conduction band consists of strongly hybridized orbitals between the nearby Ta atoms. Pumping electrons from the localized upper valence band into the conduction band directly weakens the SD reconstruction, and a spontaneous charge separation occurs. This transient charge redistribution is expected to affect the electron-electron and electron-phonon couplings near E_F, leading to a strong interaction energy shift, mimicking the effects of doping or applying pressure (14, 19, 27). The stepwise transition and metastability of photoinitiated phases shown here demonstrate that whereas the typical electron-hole recombination is on the picosecond time scale at a low doping, once a critical density is reached, the reconstructed free energy surface becomes dominant and can lock in the new charge density with a stability persisting beyond the first 100 ps (27).

We compare the critical optical doping level to the carrier density induced by applying electrostatic fields or chemical doping in other TMD materials, which commonly develop CDW states at or close to half-filling (41). A large capacitance sustained by an ionic liquid electrical double layer (EDL) was used to deliver a 2D carrier density n_2D up to 1.5 × 10¹⁴ cm⁻² by electrostatic doping to vary the band filling in MoS₂ (42), and a field doping–induced SC dome was established with a peak at n_2D = 1.5 × 10¹⁴ cm⁻². Optical doping in 1T-TaS₂ can easily reach this level, and the n_2D calculated from the critical doping to induce the NC phase is ~1 × 10¹⁴ cm⁻², matching the critical values found in MoS₂. The corresponding doping level is x ~0.1, which is often sufficient to induce new phases by chemical doping. This is amazing because so far it has not been possible to gate-dope 1T-TaS₂ with EDL because of its high intrinsic carrier concentrations, and so the optical doping method established here offers an alternative route to access higher doping concentrations than chemical doping and to explore various doping-induced novel quantum many-body states without substantially deforming the lattice.

To further examine the interaction-driven nature of photoinduced phase transitions, we conducted the same fluence-dependent experiment at 25 K using 800-nm laser pulses where the excess energy is insufficient to heat the lattice to 150 K. The results presented in Fig. 3A show a very similar trajectory to that of the 150 K Φ-n_λ diagram (Fig. 2C), affirming that the major results are insensitive to the base temperature; however, the low-temperature fluence-dependent curve indicates an additional step between NC and IC, suggesting the emergence of a new metastable phase. Given that its CDW angle still falls inside the conventional broad NC range, we designate this hidden phase NC* to differentiate it from the NC state that has a smaller angle.

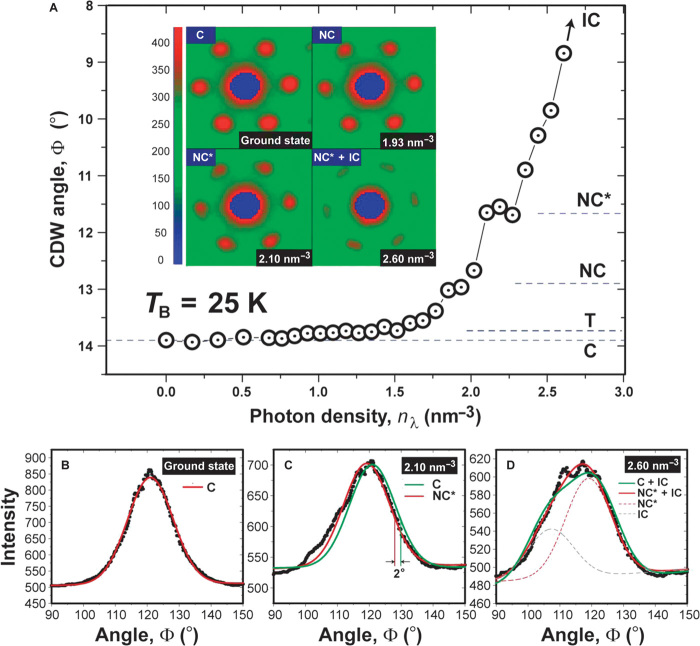
Fig. 3. Evolution of CDW phases in 1T-TaS₂ induced by mid-infrared pulses at 25 K.

(A) Optically induced evolution of CDW states characterized by CDW suppression (in ratio, based on unperturbed CDW intensity) and CDW angle Φ at various absorbed photon density at a base temperature T_B of 25 K using the 800-nm pump photons. The inset shows the diffraction images of the unpumped (ground state) and excited CDW at the quasi-equilibrium time, +20 ps. The central Bragg peak is masked out to highlight the changes in CDW intensity. (B to D) Angular profiles of the CDW peak at selected pump optical density n_λ. At n_λ = 2.6 nm⁻³, the CDW is in a mixed state between NC* and IC. The fit shows the individual components of the IC (dashed gray) and NC* (dashed red) to compose the best fit of the mixed state (solid red), whereas the green curve represents an attempted fit by using the C and IC as the constituent states.

To validate that NC* is indeed a metastable phase, we examine the angular distribution of the CDW reflections at three different stages based on the optical dose (see the bottom panels in Fig. 3). At n_λ < 0.9 nm⁻³, the system remains in the C phase (121°), which is our reference state. At n_λ = 2.1 nm⁻³, the system evolves into the NC* phase (119°), characterized by a ~2° rotation, and a very small percentage of IC is seen in the left shoulder at 107.1° (ΔΦ = −13.9°). At n_λ = 2.6 nm⁻³, the system evolves into a mixed state, with NC* and IC coexisting. Given that the mixed state maintains the memory of NC*, rather than C or NC, the phase coexistence must be driven by the competition between NC* and IC, indicating that NC* is indeed a metastable state. The fact that NC* is less pronounced at a higher temperature (150 K) and is largely absent in a temperature-driven transformation (Fig. 2A) suggests that it is unstable at a high ambient temperature.

We further surveyed the photodoping-induced phases at various temperatures from which we construct a temperature–carrier density phase diagram for 1T-TaS₂ as depicted in Fig. 4A. From this figure, it is evident that the optical doping–driven phase diagram shows similarities to the ones constructed on the basis of chemical doping (or pressure) depicted in Fig. 1A. At low temperatures, the critical density (or pressure) is insensitive to the temperature changes of the sample, and the phase transitions may be characterized as interaction-driven where new dominant phases emerge from the change in the interaction free energy rather than lattice entropy. Conversely, in the regime with low doping (or pressure), the phase transition is mostly temperature-driven, where the critical lines are inclined toward the temperature axis. On the basis of this contrasting trend, it is to be expected that the critical fluence in an optically induced transition is analogous to that of a thermally driven transition when the experiment is conducted using the 800-nm pump close to the T_C of the undoped TaS₂. This generic quasi-thermal behavior identified in transitions close to T_C addresses a long-standing puzzle in the ultrafast studies of 1T-TaS₂ (19, 40) and other photoinduced phase transitions, such as vanadium dioxide and magnetite, conducted near T_C, revealing a thermal-like threshold in absorbed optical energy density (30, 43).

Fig. 4. Temperature–optical density phase diagram and phase transition pathways.

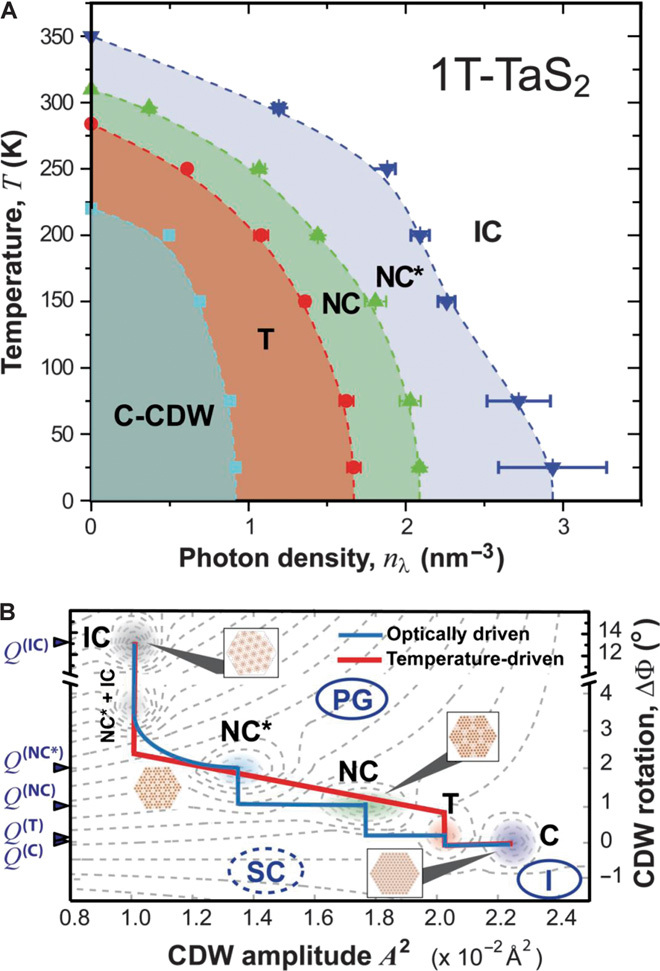
(A) Temperature–photon density phase diagram of 1T-TaS₂. (B) Cartoon depiction of the transition pathways between the commensurate state (C) and the incommensurate state (IC) under optically driven and temperature-­driven transformations over the free energy contour, also for the intermediate state triclinic (T) and near-commensurate states (NC and NC*) based on the critical amplitude A² and the CDW angle change ΔΦ. A is scaled to 0.15 Å at C-CDW state based on (7). The underlying electronic states in different regions of CDW are highlighted in circles.

We construct an optical doping–induced transition pathway from the C-CDW to IC-CDW according to the discrete jumps at the various phase boundaries (Φ_C, A_C) identified in the quasi-equilibrium phase diagram (Fig. 2C). Figure 4B shows a (schematic) comparison between the optically driven and the temperature-driven pathways, where the different discrete nature of the transitions from one phase to the other is indicative of the fundamentally different physics underlying these two transition pathways. In a thermodynamic transition, the competition and transition between two phases are governed by the complex free energy (either Helmholtz F = U − TS or Gibbs G = H − TS) surface, where entropy (S) plays an important and sometimes dominant role. In contrast, in the nonthermal optical doping pathway, the free energy basins are defined predominantly by the interaction energy (U or H) with very little entropic contribution. It is therefore to be expected that the doping-induced NC-to-IC transformation is different from a thermally driven one. For example, it is possible that two phases (1 and 2) may have interaction enthalpies such that H₁ < H₂, whereas for T > T_C, the free energy G₂ < G₁. In this case, in a nonthermal phase transition, the system will go from an initial phase to phase 1, whereas under thermal conditions, the transition will be to phase 2. This can explain the two rather markedly different behaviors proposed to describe the NC-to-IC transition, namely, coherently driven and discommensuration-driven, which were developed on the basis of experimental setups probing different pathways (8, 10, 25, 26). In the discommensuration-driven thermal transition from the NC to the IC phase, the size of the discommensurate regions (domain walls) increases at the cost of commensurate regions that vanish in the IC phase. In contrast, in a coherently driven transition, photodoping may drive commensurate regions to an incommensurate state in a cooperative manner. More details of distinct nonthermal behaviors of the interaction-driven pathway can be seen in the ultrafast phase transitions as discussed below.

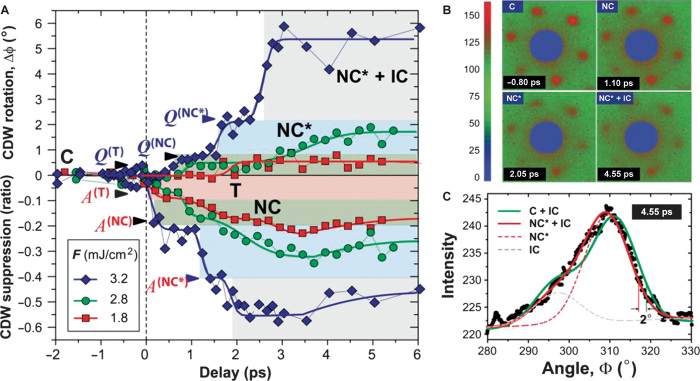
A remarkable capability of ultrafast crystallography data is elucidation of nonequilibrium transition state pathways as depicted in Fig. 5A. The transformations are induced using 2500-nm photons and T_B = 150 K, in a region predominantly influenced by doping effects. We drive the system with three selected photon densities, targeting the “final states” of T, NC, and NC*. This near-gap mid-infrared pump ensures a small excess energy to leave a small thermal imprint on the dynamics after reaching the quasi-equilibrium state. The results in the upper panel of Fig. 5A show the evolution in Φ, and the lower panel depicts the corresponding changes in A (through the I_CDW). These transient features are highly organized and exhibit surprisingly sharp jumps in temporal steps as short as 200 fs (2σ in error function fit, resolution limited). The plateau regions following the transitions represent the transient metastable phases, which can be identified with the Φ_C and A_C as those established on the basis of the quasi-equilibrium states by comparing −10 and +20 ps (see Table 1). However, the dynamical responses from the two CDW parameters (A and Φ) to reach the new metastable state are not synchronized. Quite persistently, the change in A leads the change of Φ, implying that the local CDW must first deconstruct (reduction in CDW amplitude), whereas the new phase often swiftly reemerges at a different periodic order after an incubation period, as manifested in the sudden change of Φ after the suppression. This unique phenomenon is difficult to reconcile using thermodynamic reasoning. Whereas dynamical CDW features do display broadening during the NC*-to-IC transition period (Fig. 5B), signaling a mixed state, its angular profile can be well described by the superposition of two discrete states with no broadening in their respective angular profile (as described in Fig. 5C), which is reached within 1 ps, in contrast to percolation-driven thermal switching. This transient phase coexistence may be due to decoherence in the responses from different domains at a longer time, because, given a sufficient driving dose and starting already at the NC phase, the transition into the IC phase can proceed rapidly without experiencing extensive phase coexistence, as shown in fig. S2 (C to E). It is important to note that the major transformations described here occur within the typical electron-lattice coupling time of the system (several picoseconds), so the evolution may be considered to be within a closed system with essentially no change in temperature. Furthermore, all the transformations cease to evolve after 4 ps, which we assign as the minimum time scale for carrier recombination (27).

Fig. 5. Femtosecond electron crystallography signatures of nonequilibrium CDW phase transitions induced by selected mid-infrared optical doping.

(A) Dynamics of CDW state transformations inspected via the rotation of CDW wave vector Q away from C-CDW and the suppression of I_CDW(t) [in ratio based on the I_CDW (−10 ps)]. The solid lines are drawn on the basis of fitting the staircase rises using a Gauss error function. (B) Diffraction images at selected time scales depicting the transient state of CDW under F = 3.2 mJ/cm². The central Bragg peak is masked out to highlight the intensity changes of the CDW satellites, which are typically two orders of magnitude weaker. (C) Angular profile of a selected CDW satellite to show the presence of initial (NC*) and final (IC) state population in a mixed state at 4.55 ps. The transient angular profile cannot be properly fitted by using the C as the initial state, suggesting that NC* is a stable doorway state leading to the transition.

DISCUSSION

The phenomenology of the observed phase conversions manifests the key features of the so-called PIPTs (44), or photoinduced domino effect (45), including threshold-like behavior (29) (Figs. 2C and 3A), high efficiency in photogeneration yield (29, 44) (Fig. 2B), and dynamical and nonlinear nature reflected in the incubation period (Fig. 5A) which is inversely proportional to the pump intensity in a two-state conversion (45, 46). Theoretical understanding of these phenomena has been based on a long-range coupling term that is exclusively controlled by photoexcitation, rendering a highly nonlinear interconversion rate for describing the bistable switching with the above-mentioned major features (45). Nonetheless, to capture the first-order–like sharp transitions in many PIPT systems, for example, manganites (29, 47) and organometallic spin-crossover complexes (45, 46), spatial inhomogeneities must be introduced for engineering a competitive first-order process (44, 45). However, given the typical NC domain size of 73 Å (25) and the ultrafast time scale of the transitions, the stairwise successions discovered in the ultrafast 1T-TaS₂ phase evolution cannot be explained on the basis of a percolative picture. An alternative route that is interaction-driven while preserving the nonlinear conversion and competitive first-order transition feature must be provided.

To intuitively understand the unique features of 1T-TaS₂ ultrafast phase evolution, a microscopic picture involving strong response to femtosecond optical doping and respecting the geometrical constraints is constructed here. First, mid-infrared femtosecond optical excitation coherently relaxes the SD motif of the CDW state, readily leading to transient suppression of the CDW amplitude. However, our data show that this excitation does not have a global and lasting impact until a critical dose is reached, after which a new metastable phase can arise. Therefore, the more profound PIPT effects come from the transformation of the interaction potential driven by photodoping, allowing the system to access the energy landscape of the new phase. The nonequilibrium dynamics results indicate that the initial adjustment of charge density leads to the long-range correlation, enabling the system to swiftly move toward the metastable state, whereas the periodic atomic lattice distortion (Q) remains unchanged. However, the reconstruction required for the new CDW state, as indicated by the rotation of Q, cannot proceed in a downhill fashion because of lattice constraint. Proper domain walls must be introduced to reconcile the electronic and elastic energy now competing in the precursor stage. An adjustment period to reach a certain degree of synchronization must occur between different domains to form a new global state spontaneously. Although this can explain the key ultrafast features of the observed CDW dynamics, the exact nature of the interaction(s) that controls the global energy landscape is still in question. Judging from the time scale of initial response, the redefinition of the interaction energy landscape cannot be a direct result of altering the stacking sequences. The driving force at a shorter time scale likely comes from correlation or polaronic coupling, which is directly sensitive to the femtosecond optical doping. However, it is possible that competing with the soft mode response for the rotation is the need to alter the stacking to balance both electronic and elastic energy scales for the phase reconstruction as observed in the different stacking orders (or disorders) associated with the NC and C phases under steady-state conditions (32, 48, 49). At present, quantitative ultrafast models and computational methods to test these ideas remain an extreme challenge.

It is interesting to compare the recently uncovered H state (27) and the NC* state (also a hidden phase) observed here. The key processes, such as the existence of a threshold pump fluence (~1 mJ/cm²), a critical pulse length dependence (~4 ps), and the requirement for low crystal temperature (<70 K) to access the hidden states, indicate that the production of the H state adheres to similar principles of PIPT. Indeed, the necessary conditions for generating the H state from an insulating ground state are markedly analogous to those required in the optical doping–induced insulator-metal switching. Nonetheless, the H state is likely a different state than NC* because NC* is stable at 150 K and all our processes are reversible. Given that the H state is stable at an arbitrarily long time before applying a long heating pulse, it is likely a hidden state supercooled at a minimum energy basin below 70 K. Such stabilization may be engineered in a film supported by a substrate because of the presence of strain. For example, we found that in thin TaS₂ films that exhibit surface ripples or in very thick films, the transitions between different phases are deferred to longer time scales and show partial trapping when an intense femtosecond laser pulse is applied. Further studies are required to investigate this in detail; however, these results do highlight the ruggedness of the free energy landscape of 1T-TaS₂ because of the combined effects of doping, strain, and/or disorder, which may in turn be used to engineer or sustain new functional states.

We expect that the studies presented here will have significant ramifications in several areas of research. First, the presented methodology opens up a new avenue to survey the complex energy landscape and provides a new perspective on doping-induced phase diagrams, avoiding the difficulty of electrostatic gating or confounding effects because of defects and/or disorder resulting when doping by intercalation or substitution. Second, the speed and degree of photodoping substantially exceed that achievable with conventional methods, creating opportunities to generate new phases (27, 29). Third, observation of robust nonthermal switching at mesoscales and at ultrafast time scales provides a platform for new applications of correlated crystals for designing high-speed low-energy consumption nanophotonics and electronics devices.

MATERIALS AND METHODS

Single crystals of the 1T form of TaS₂ were grown by the chemical vapor transport technique (see the Supplementary Materials for details). We prepared thin flakes of TaS₂ from a 1T-type bulk single crystal in mass by the Scotch tape method. The thin samples were removed from the Scotch tape by submerging the tape in acetone. Acetone solution containing samples was then dropped onto standard microscope grids with a pipette. The suitable samples (30 to 50 nm in thickness) were prescreened from the contrast in an optical microscope, and then the thicknesses were determined by performing zero-loss electron energy loss spectroscopy thickness mapping using a TEM. It is central for this experiment to use stress-free, thin, and homogeneously exfoliated samples to ensure unhindered macroscopic reconstruction of domains during optically driven phase transitions. We performed ultrafast electron crystallography experiments in an ultrahigh vacuum chamber equipped with a custom-made cryogenic sample goniometer, with temperature control that ranges from 20 K to above room temperature. Temperature was measured with 0.01 K precision at the back of the sample holder, which was in solid thermal contact with the sample grid, which was clamped into a copper counter sink on the sample holder. To reach high sensitivity, we used a microcollimated electron beam (typically ≤30 μm wide), which is adjusted to balance between temporal resolution and sensitivity—to map the phase diagram, ~10,000 electrons per pulse were used to gain efficiency, whereas to study the dynamics, ~500 electrons per pulse were delivered to reach ~300-fs resolution (FWHM) at 30 keV (38). The pump pulse was generated by an optical parametric amplifier driven by an 800-nm amplified laser system (Ti:Sapphire Regenerative Amplifier, Spectra-Physics), and could be tuned from 200 to 3000 nm in wavelength.

The CDW dynamics were analyzed on the basis of the angular intensity profile extracted by performing the line scan along the circular arc of the CDW satellites at a radius |Q| (see the Supplementary Materials for details). The I_CDW values presented in Figs. 2 and 5 are the integrated intensity following a second-order polynomial background subtraction. The CDW angle Φ, as defined in Fig. 1, is determined using a single Gaussian function to fit the angular profile. During a transition from one stable phase to another, Φ represents a weighted average from the populations within the two distinctive phases. Alternatively, to closely examine the coexistence state, a two-Gaussian model is used to fit the bifurcated CDW angular profiles (see Figs. 3D and 5C), where each Gaussian is fixed at the angular position of the respective initial and final state. The σ of the Gaussian is fixed at the value of the ground state, justified by the instrument-limited peak width (depending on the collimation of the electron beam). The integrated intensity drops by more than one half from C to IC, and given that the mixed state between NC* and IC has bifurcated peaks, the signal strength for the Gaussian profile fitting can drop by a factor ranging from two to five times, leading to very different uncertainties depending on the state. Between 7 and 15 CDW angular profiles were typically combined to yield a statistically sound analysis presented here.

SUPPLEMENTARY MATERIALS

Supplementary material for this article is available at http://advances.sciencemag.org/cgi/content/full/1/5/e1400173/DC1

Text

Fig. S1. Optical image of a 45-nm-thick exfoliated single-crystal 1T-TaS₂ sample supported on a standard 1000-mesh gold TEM grid.

Fig. S2. Steady-state and dynamical diffraction patterns of 1T-TaS₂ in the NC-CDW state taken at room temperature.

Fig. S3. The selected CDW patterns under different optical excitation fluences for constructing the optical doping–induced phase diagram obtained at T_B = 150 K using the 800-nm femtosecond photons for optical doping.

Fig. S4. The determination of CDW phase boundaries based on the presence of a step or a slope change.

References (50–54)