Skip to main content
PMC home page
Scientific Reports logoLink to Scientific Reports
. 2015 Dec 17;5:18342. doi: 10.1038/srep18342

A Revisit to High Thermoelectric Performance of Single-layer MoS2

Zelin Jin 1,2,*, Quanwen Liao 3,*, Haisheng Fang 1,2,b, Zhichun Liu 3,c, Wei Liu 3, Zhidong Ding 2, Tengfei Luo 4, Nuo Yang 1,2,a
PMCID: PMC4683674  PMID: 26677953

Abstract

Both electron and phonon transport properties of single layer MoS2 (SLMoS2) are studied. Based on first-principles calculations, the electrical conductivity of SLMoS2 is calculated by Boltzmann equations. The thermal conductivity of SLMoS2 is calculated to be as high as 116.8 Wm−1K−1 by equilibrium molecular dynamics simulations. The predicted value of ZT is as high as 0.11 at 500 K. As the thermal conductivity could be reduced largely by phonon engineering, there should be a high possibility to enhance ZT in the SLMoS2-based materials.

Thermoelectric materials are essential for converting waste heat to electricity and solid-state cooling, which have attracted much attention recently1,2,3,4,5,6,7,8,9,10. The dimensionless figure of merit (ZT) is utilized to evaluate the efficiency of the thermoelectric conversion, defined as: 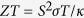Inline graphic, where S is the Seebeck coefficient, σ is the electrical conductivity, T is the absolute temperature, and κ is the total thermal conductivity. The κ is composed of electrons’ contribution (κe) and phonons’ contribution (κp). The ZT value for most commercial materials are around one, which is far below the critical value of three that is comparable with the traditional energy conversion in efficiency3. In the past two decades, nano-materials and nano-structured materials are expected to have excellent energy conversion efficiency due to the higher power factor (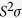Inline graphic)11,12 and lower κp13,14,15, which are also known as the electron-crystal and phonon-glass.

The graphene, as the first two dimensional material, has extraordinary electronic property as well as super high thermal conductivity16. However, the pristine graphene, a semi-metal, has zero band gap and very small S17. Different from graphene, single layer MoS2 (SLMoS2) is a semiconductor and has a direct band-gap18, which enables its wide applications in electronic and optical devices, such as field effect transistor19.

Recently, some works have studied the electronic and phononic properties of SLMoS2. Eugene et al. have calculated the electronic structure of SLMoS2 which is compared with that of bulk MoS220, and revealed the transition mechanism from the direct band gap of SLMoS2 to the indirect band gap of bulk MoS2. Emilio et al. have shown that, after applying compressive or tensile bi-axial strain, the electronic structure of SLMoS2 transitions from semiconductor to metal21. Li et al. calculated the intrinsic electrical transport and electron-phonon interaction properties of SLMoS222. Moreover, the thermoelectric potential of SLMoS2 has been explored and a maximum ZT, at room temperature, is obtained as 0.5 by Huang et al.23 using the ballistic model. The scatterings of electrons are not considered in their ballistic model, which should have led to an over-estimation of ZT. Fu et al. studied SLMoS2 ribbons and calculated the ZT value to be up to 3.424. Besides theoretical predictions, Wu et al. has experimentally reported a value of S as 30 mV/K for SLMoS225, which indicates an appealing potential for thermoelectric applications.

Besides electron properties, some works focused on the phonon properties of SLMoS2. The SLMoS2 nanoribbon has a low thermal conductivity due to the size effect. Jiang et al. claimed that κp of SLMoS2 nanoribbon was around 5 Wm−1K−1 at room temperature by molecular dynamics (MD) simulations26. Zhang et al. reported three results for SLMoS2 nanoribbons which were 1.35 Wm−1K−1 by equilibrium molecular dynamics (EMD)27, 23.2 Wm−1K−1 by non-equilibrium Green’s function28, and 26.2 Wm−1K−1 by Boltzmann transport equation29. However, there are also reports on the thermal conductivities for MoS2 with higher values. Li et al. predicts the κ as 83 Wm−1K−1 from ab initio calculations30. With high-quality sample, the κ of suspended few layers MoS2 has been measured as 52 Wm−1K−1 31 and 35 Wm−1K−1 32. Liu et al. claimed that the basal-plane thermal conductivity of single crystal MoS2 would be 85–110 Wm−1K−1 33. There is not an agreement on the κ of SLMoS2, and it needs more works on this issue.

In this paper, both electron and phonon transport properties of SLMoS2 are studied (the structure as shown in Fig. 1). Based on the electronic band structure from first-principles calculations, the electrical conductivity of SLMoS2 is calculated by Boltzmann equations. Both the electronic structure and phonon dispersion relation are calculated. Together with κp calculated from classical EMD simulations, the thermoelectric properties are obtained. The results show that SLMoS2 is a promising material for thermoelectric engineering.

Figure 1. The structure of single layer MoS2.

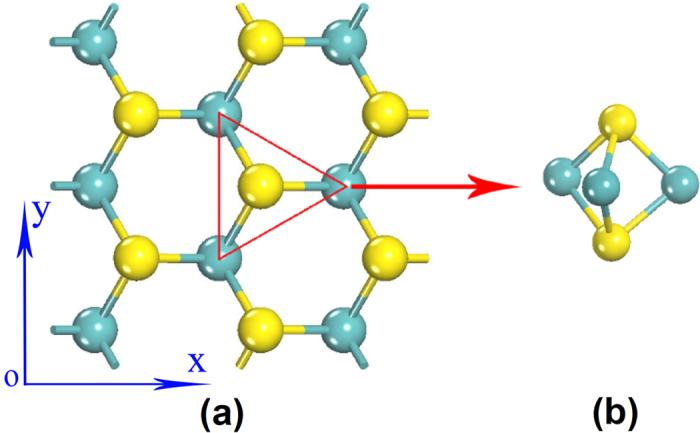
Figure 1

Open in a new tab

(a) The top view, a hexagonal lattice structure. (b) The side view of the inset triangle. Each sulfur atom has three molybdenum atoms as its first nearest neighbor atom. Each molybdenum atom has six sulfur atoms as its first nearest neighbor atom.

Results and Discussions

The electronic band structure of SLMoS2 along the high-symmetry points in Brillouin zone is shown in Fig. 2(a). At the K point, there is a direct band gap as 1.86 eV which agrees well with previous calculations (1.69 ~ 1.98 eV)20,21,22,23,34,35. Another characteristic in the SLMoS2 band structure is that there is a Q valley along the Γ-K path. The Q valley yields a larger effective mass than the K valley, which leads to strong electron-phonon interactions in MoS2 at this point22. The large effective mass of carriers and multi-valleys band structure are favorable for a high ZT36. As shown in the density of state (DOS) electrons (Fig. 2), there are sharp gradients at the edges of both conduction and valence band and several peaks near band edges, due to the quantum size effects in the 2D structure, which may enhance ZT as the prediction of Mahan and Sofo12.

Figure 2.

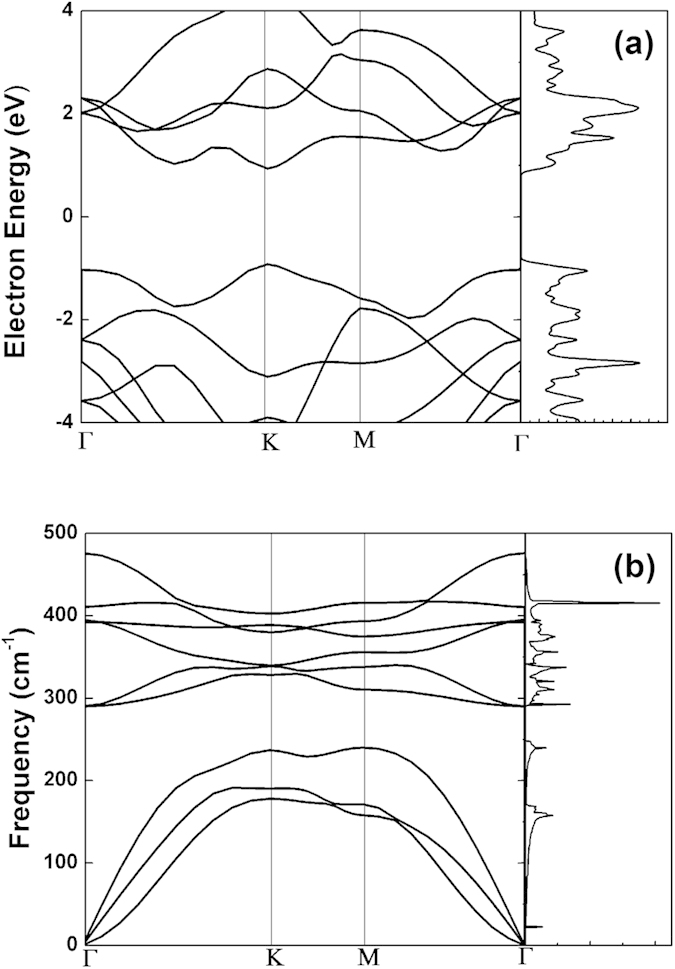
Figure 2

Open in a new tab

(a) Electron band structure and density of state along the symmetry line. The Fermi energy is set in the middle of the gap. (b) The phonon dispersion for SLMoS2 and the phonon density of state in the whole Brillouin zone.

We made a full calculation of the thermoelectric properties of SLMoS2 at 300 K, 400 K and 500 K. As shown in Fig. 3(a,b), κe and σ increase as the increasing of carrier concentration (ne). When the Fermi level is in the band gap, ne and σ is much smaller. As the Fermi level moves up into the conduction band, ne and σ increases quickly (more details shown in Fig. S4 and S6 in supporting information). Shown in Fig. 3(a), the Seebeck coefficient has a large value and decreases with the increase of ne. The Fermi level for ZT peak locates around the first DOS peak, and this is consistent with the prediction that a delta DOS would result in an optimum ZT12. It leads to a power factor as high as several hundreds of μWcm−1K−2 (shown in Fig. 3(c)), which is compared with those of high ZT thermoelectric materials, such as BiTe37 and PbTe38.

Figure 3. The thermoelectric transport properties of n-type SLMoS2 at 300K, 400K and 500K.

Figure 3

Open in a new tab

(a) The electrical conductivity and Seebeck coefficient; (b) The electrical thermal conductivity; (c) The power factor; (d) The figure of merit. The thermoelectric transport properties of p-type is shown in supporting information.

The phonon dispersion relation of SLMoS2 is also calculated and shown in Fig. 2(b). In the vicinity of Γ point, the out-of-plane transverse acoustic branch (ZA) has a quadratic relation, both the transversal acoustic branch (TA) and longitudinal acoustic branch (LA) have linear relations. The group velocities at Γ point along Γ-M direction are around 667.5 m/s (TA) and 1080.2 m/s (LA), which are much smaller than the group velocities in graphene39, as 3743 m/s (TA) and 5953 m/s (LA).

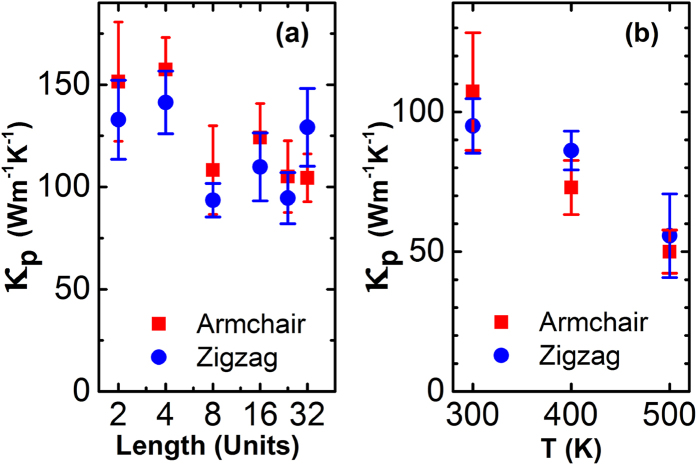
For semiconductors, the thermal conductivity is mainly contributed by phonons (κp). We calculated κp by EMD and show in Fig. 4. The κp of SLMoS2 exhibits a size dependence on the simulation cell and reaches a converged value when the simulation cell is larger than 8 × 8 × 1 units3 (8.66 × 7.50 × 0.616 nm3) (Fig. 4(a)). A weak anisotropy is observed in thermal conductivities along armchair and zigzag direction. The average value of κp along armchair and zigzag directions is 116.8 Wm−1K−1 for simulation cell as large as 32 × 32 × 1 units3 (34.7 × 30.0 × 0.616 nm3) at 300K. In Fig. 4(b), the κp of SLMoS2 decreases with the increasing temperature (79.6 Wm−1K−1 and 52.9 Wm−1K−1 at 400 K and 500 K, respectively), because there are more three phonon Umklapp scatterings for high temperature. A lower κp is good for enhancing thermoelectric properties.

Figure 4.

Figure 4

Open in a new tab

(a) The dependence of thermal conductivity (κp) of SLMoS2 upon the size of simulation cell. The size of simulation cell size equals the length (L) times a supercell (1.083 × 0.938 × 0.616 nm3). (b) The thermal conductivity of SLMoS2 for three different temperature as 300K, 400K and 500K, respectively.

Comparing with previous results (details in Table 1), we obtained a maximum value of κp of SLMoS2. Some of these works focused on the SLMoS2 nanoribbons26,27,29 which have very low thermal conductivities, because the phonon confinement effect in nanostructures40,41. Using the same empirical potential in MD simulations, our results for SLMoS2 is around 20 times larger than that of nanoribbon with 34.6 × 30 × 0.61 nm3 in size. Besides, due to the interlayer coupling by van Der Waals forces42, the multilayer structures31,33 should be lower than the single layer27,28,30,32 in thermal conductivity. Due to the absence of impurities, defects and interlayer scatterings in MD simulations, the κp of SLMoS2 is a little higher than the measurements of bulk multilayer SLMoS233, 85 ~ 110 Wm−1K−1. Our value is comparable to the result predicted from ab initio calculation30 , where stated that the lower bound of κp as 83 Wm−1K−1 at 300 K in the considering of phonon scatters and the simplification in calculation of BTE model. Another advantage for our results is that both the nonequilibrium Green’s function calculation28 and the Boltzmann transport equation29 adopt artificial relaxation time approximations for phonon-phonon Umklapp scatterings, which is not required in the MD simulations.

Table 1. The comparison of thermoelectric properties for different MoS2 structures, including single layer (SL), few layers (FL), single layer ribbon (SLR), and bulk MoS2.

Struct.& Ref. Method T(K) Carrier type σ(Scm−1) S(μVK−1) κe κph ZT
(Wm−1K−1)
SL DFT + BTE + MD 300 n 14625 −110 8.94 116.8 0.04
p 16957 72.9 11.39 0.02
500 n 11714 −161 9.69 52.9 0.26
p 8853 150 8.40 0.16
SL23 DFT + Ballistic model 300 n 54 −202 0.021 0.243 0.25
p 108 215 0.040 0.244 0.53
SLR24,27 DFT + BTE + MD 300 n 7770 −204 2.89 1.02 2.5
p 14300 223 5.20 3.4
SL CVD25 Experiment 300 – – ≤30000 – – –
SL FET59 Experiment 300 – – 400–100000 – – –
Bulk60 Experiment 90–873 – – 500–700 – – –
SL27 EMD 300 – – – – 1.35 –
SL30 DFT + BTE – – – – >83 –
SL28 DFT + NEGF – – – – 23.2 –
SLR29 DFT + BTE – – – – 26.2 –
SLR26 NEMD – – – – 5 –
FL31 Experiment – – – – 52 –
SL32 Experiment – – – – 35.4 –
Bulk33 Experiment – – – – 85– 110 –
Open in a new tab

Generally, there are two types of commonly used MD simulation methods, EMD and non-equilibrium MD (NEMD). The EMD is better than NEMD in predicting a bulk structure by applying the periodic boundary condition, because NEMD need impose artificial heat bath and use the extrapolating method. However, NEMD gets the advantage of EMD in predicting a structure with a finite size. There is a EMD report which showed a low value of κp of SLMoS2 as 1.35 Wm−1K−127 The potential functions used in Ref. [27] are determined by tight-binding quantum chemistry calculations and used to reproduce the crystal structure and Raman spectrum. The empirical potential function is important to obtain a reliable value of thermal conductivity. Differently, the Stillinger-Weber potential26 ,used in this work, can reproduce a better phonon dispersion relations, which will describe the heat transfer properties with a better reliability (details in supporting information).

With the above calculations of electron and phonon properties, ZT profiles can be obtained and are shown in Fig. 3(d). There is a parabolic tendency for ZT in the whole carrier concentration range. The optimized ZT values are 0.04, 0.07, and 0.11 for 300 K, 400 K and 500 K, respectively. These values get bigger as temperature increases because of the improved power factors and the reduced thermal conductivity. These optimized ZT values correspond to the situations where the Fermi level moves up to the first peak in the conduction band.

As shown in Table 1, we list some recently results on thermoelectric properties of different SLMoS2 structures. The value of ZT is in the same order of Ref. [23] and one order smaller than that of SLMoS2 ribbon. Our results of κp is higher than others. As shown in Fig. 4(a), our results of κp overcome the size confinement effect and corresponds to an infinite SLMoS2 sheet. Moreover, different from NEMD, it does not need the assumption of linear relationship between 1/κp and 1/L.

Compared to nanoporous silicon analyzed by Lee43, we get the similar ZT trend and magnitude of these transport values. As shown in Fig. 3(c), the power factor of SLMoS2 is larger than that of nanoporous silicon. The large power factor of SLMoS2 comes from a larger intrinsic σ and a comparable S. It indicates that the SLMoS2 has comparable electron properties as the optimized nanoporous silicon. However, due to the high κe and κp, the SLMoS2 has a modest ZT value. It is also worth noting that the ZT value here is smaller than the prediction from ballistic models by Huang et al. where neglects the phonon scatterings23.

Although the predicted ZT value of SLMoS2 is not over one, SLMoS2-based materials may be a good candidate for thermoelectric application. Our results show that SLMoS2 has a much higher thermal conductivity (~116 Wm−1K−1, at 300 K) than other thermoelectric materials (on the order of 1 Wm−1K−1)6,44. The higher thermal conductivity makes a bigger room for thermal conductivity reduction by phonon engineering. There are some conventional ways to reduce thermal conductivity by phonon engineering, such as isotope doping45, nanoporous structure14,43,46,47, nanoribbons48, or folding49 etc. The mechanism is to introduce more phonon scatterings which can shorten phonon mean free paths. For example, bulk silicon has a ZT value as low as 0.003. Then, with phonon engineering, Si-based nanomaterials, such as Si nanowires45,50, nanoporous Si14,43,46,47, and nanostructured Si51, may reach a two orders larger ZT. Another inspiration example is the graphene. The high pristine thermal conductivity of graphene can be reduced largely by phonon engineering48,49,52 which make a ZT as high as 352

The values of ZT and power factor of SLMoS2 are much higher than those of silicon and graphene. With a reduced thermal conductivity and kept electron transport properties, the values of ZT of SLMoS2-based materials may be larger than one. Generally, a side-effect of phonon engineering is the reduction of power factor. However, the side-effect is not obvious because the mean free paths of electrons are around two orders smaller than that of phonons, such as what is shown in the recent thermoelectric results on Si phononic crystals47

Conclusion

The thermoelectric properties of SLMoS2 are explored using theoretical calculations. The electronic structure and phonon dispersion relation are calculated using DFT calculations. Combined with molecule dynamics simulations and Boltzmann equations, thermoelectric properties are predicted as a function of carrier concentration at room temperature. With the lattice thermal conductivity as 116.8 Wm−1K−1, 79.6 Wm−1K−1, and 52.9 Wm−1K−1, the optimized ZT of SLMoS2 is found to be of 0.04, 0.07 and 0.11 at 300 K, 400 K and 500 K, respectively. As SLMoS2 has a higher ZT than other pristine structure, like silicon and graphene, there will be a big room to enhance ZT in SLMoS2-based materials by the developing phonon-engineering.

Methods

To calculate electronic properties, the first-principles calculation is implemented by QUANTUM ESPRESSO in the frame of density functional theory (DFT)53. The local density approximation (LDA) is used in the exchange-correlation approximation while the semi-core valence for molybdenum is considered with the Goedecker-Hartwigsen-Hutter-Tetter method54. The wave-functions in electronic calculation are cut off at 160 Ry, and the irreducible Brillouin zone is sampled with a 16 × 16 × 1 Monkhorst-Pack grid.

The hexagon primitive cell is used to structure relaxation and property prediction in DFT calculation. Structure relaxation for SLMoS2 yields lattice constant of about 3.13 Å, consistent with previous predictions of 3.12–3.16 Å20,21,22. For the consistency of property evaluation, the thickness of SLMoS2 is assumed to be 6.16 Å – the same as that of the single-sheet in bulk MoS255. The calculations on both electrons and phonons are based on this optimized structure.

In the calculations of transport coefficients, a k-point mesh as 28 × 28 × 1 (denser enough to obtain converged results) is used over the irreducible Brillouin zone. With the assumption of constant relaxation time, the transport coefficient for electrons can be calculated using BoltzTrap56 which solves Boltzmann transport equation (more details in supporting information).

The thermal conductivity of SLMoS2, κp, is calculated by EMD with the Green-Kubo approach57. All the simulations are carried out utilizing the LAMMPS software package58. The Stillinger-Weber potential with parameters fitted by Jiang et al.26 is adopted in our simulations. The SLMoS2 film is constructed by periodic arrangement of supercell illustrated in Fig. 1, and the sizes of 1 × 1 × 1 units3 supercell corresponds to 1.083 × 0.938 × 0.616 nm3. To study the finite size effect on thermal conductivities, we calculated the simulation cells with the volumes from 2 × 2 × 1 to 32 × 32 × 1 units3 at room temperature (more details in supporting information).

Additional Information

How to cite this article: Jin, Z. et al. A Revisit to High Thermoelectric Performance of Single-layer MoS2. Sci. Rep. 5, 18342; doi: 10.1038/srep18342 (2015).

Supplementary Material

Supplementary Information
srep18342-s1.pdf (574.5KB, pdf)

Acknowledgments

This project was supported in part by the grants from the National Natural Science Foundation of China: 51476068 (H.F.), 51576076(N.Y.), and 51376069 (Z.L.) and the Major State Basic Research Development Program of China 2013CB228302 (Z.L.). The authors are grateful to Lina Yang and Jing-Tao Lű for useful discussions. The authors thank the National Supercomputing Center in Tianjin (NSCC-TJ) for providing help in computations.

Footnotes

Author Contributions Z.J. and Z.D. carried out the first-principles calculations. Q.L. carried out the MD calculations. N.Y., Z.J., Q.L. and Z.D. analyzed the calculation data. H.F., Z.L., W.L. and T.L. discussed and commented on the manuscript. Z.J., Q.L., Z.D. and N.Y. edited the manuscript. N.Y., H.F. and Z.L. supervised the research.

References

  1. Chen B. J., Sun X. W. & Xu C. Fabrication of zinc oxide nanostructures on gold-coated silicon substrate by thermal chemical reactions vapor transport deposition in air. Ceram Int 30, 1725–1729, 10.1016/j.ceramint.2003.12.140 (2004). [DOI] [Google Scholar]
  2. Vineis C. J., Shakouri A., Majumdar A. & Kanatzidis M. G. Nanostructured Thermoelectrics: Big Efficiency Gains from Small Features. Adv. Mat. 22, 3970–3980, 10.1002/adma.201000839 (2010). [DOI] [PubMed] [Google Scholar]
  3. Shakouri A. Recent Developments in Semiconductor Thermoelectric Physics and Materials. Annu Rev Mater Res 41, 399–431, 10.1146/annurev-matsci-062910-100445 (2011). [DOI] [Google Scholar]
  4. Vaqueiro P. & Powell A. V. Recent developments in nanostructured materials for high-performance thermoelectrics. J. Mater. Chem. 20, 9577–9584, 10.1039/c0jm01193b (2010). [DOI] [Google Scholar]
  5. Sootsman J. R., Chung D. Y. & Kanatzidis M. G. New and Old Concepts in Thermoelectric Materials. Angew. Chem.-Int. Edit. 48, 8616–8639, 10.1002/anie.200900598 (2009). [DOI] [PubMed] [Google Scholar]
  6. Minnich A. J., Dresselhaus M. S., Ren Z. F. & Chen G. Bulk nanostructured thermoelectric materials: current research and future prospects. Energy Environ. Sci. 2, 466–479, 10.1039/b822664b (2009). [DOI] [Google Scholar]
  7. Snyder G. J. & Toberer E. S. Complex thermoelectric materials. Nat. Mater. 7, 105–114, 10.1038/nmat2090 (2008). [DOI] [PubMed] [Google Scholar]
  8. Dresselhaus M. S. et al. New Directions for Low‐Dimensional Thermoelectric Materials. Adv. Mat. 19, 1043–1053, 10.1002/adma.200600527 (2007). [DOI] [Google Scholar]
  9. Nolas G. S., Poon J. & Kanatzidis M. Recent developments in bulk thermoelectric materials. Mrs Bulletin 31, 199–205, 10.1557/Mrs2006.45 (2006). [DOI] [Google Scholar]
  10. Martín-González M., Caballero-Calero O. & Díaz-Chao P. Nanoengineering thermoelectrics for 21st century: Energy harvesting and other trends in the field. Renew. Sust. Energ. Rev. 24, 288–305, 10.1016/j.rser.2013.03.008 (2013). [DOI] [Google Scholar]
  11. Hicks L. D. & Dresselhaus M. S. Effect of quantum-well structures on the thermoelectric figure of merit. Phys. Rev. B 47, 12727–12731, 10.1103/PhysRevB.47.12727 (1993). [DOI] [PubMed] [Google Scholar]
  12. Mahan G. D. & Sofo J. O. The best thermoelectric. Proceedings of the National Academy of Sciences 93, 7436–7439, 10.1073/pnas.93.15.7436 (1996). [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Jiang J. W., Yang N., Wang B. S. & Rabczuk T. Modulation of thermal conductivity in kinked silicon nanowires: phonon interchanging and pinching effects. Nano Lett. 13, 1670–1674, 10.1021/nl400127q (2013). [DOI] [PubMed] [Google Scholar]
  14. Yang L., Yang N. & Li B. Extreme low thermal conductivity in nanoscale 3D Si phononic crystal with spherical pores. Nano Lett. 14, 1734–1738, 10.1021/nl403750s (2014). [DOI] [PubMed] [Google Scholar]
  15. Poudel B. et al. High-thermoelectric performance of nanostructured bismuth antimony telluride bulk alloys. Science 320, 634–638, 10.1126/science.1156446 (2008). [DOI] [PubMed] [Google Scholar]
  16. Balandin A. A. Thermal properties of graphene and nanostructured carbon materials. Nat Mater 10, 569–581, 10.1038/nmat3064 (2011). [DOI] [PubMed] [Google Scholar]
  17. Zuev Y. M., Chang W. & Kim P. Thermoelectric and magnetothermoelectric transport measurements of graphene. Phys Rev Lett 102, 096807, 10.1103/PhysRevLett.102.096807 (2009). [DOI] [PubMed] [Google Scholar]
  18. Mak K. F., Lee C., Hone J., Shan J. & Heinz T. F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 105, 136805, 10.1103/PhysRevLett.105.136805 (2010). [DOI] [PubMed] [Google Scholar]
  19. Radisavljevic B., Radenovic A., Brivio J., Giacometti V. & Kis A. Single-layer MoS2 transistors. Nat Nanotechnol 6, 147–150, 10.1038/nnano.2010.279 (2011). [DOI] [PubMed] [Google Scholar]
  20. Kadantsev E. S. & Hawrylak P. Electronic structure of a single MoS2 monolayer. Solid State Commun. 152, 909–913, 10.1016/j.ssc.2012.02.005 (2012). [DOI] [Google Scholar]
  21. Scalise E., Houssa M., Pourtois G., Afanas’ev V. V. & Stesmans A. Strain-induced semiconductor to metal transition in the two-dimensional honeycomb structure of MoS2. Nano Res 5, 43–48, 10.1007/s12274-011-0183-0 (2012). [DOI] [Google Scholar]
  22. Li X. D. et al. Intrinsic electrical transport properties of monolayer silicene and MoS2 from first principles. Phys. Rev. B 87, 115418, 10.1103/PhysRevB.87.115418 (2013). [DOI] [Google Scholar]
  23. Huang W., Da H. X. & Liang G. C. Thermoelectric performance of MX2 (M = Mo,W; X = S, Se) monolayers. J. Appl. Phys. 113, 104304, 10.1063/1.4794363 (2013). [DOI] [Google Scholar]
  24. Fan D. D. et al. MoS2 nanoribbons as promising thermoelectric materials. Appl. Phys. Lett. 105, 133113, 10.1063/1.4897349 (2014). [DOI] [Google Scholar]
  25. Wu J. et al. Large thermoelectricity via variable range hopping in chemical vapor deposition grown single-layer MoS2. Nano Lett. 14, 2730–2734, 10.1021/nl500666m (2014). [DOI] [PubMed] [Google Scholar]
  26. Jiang J. W., Park H. S. & Rabczuk T. Molecular dynamics simulations of single-layer molybdenum disulphide (MoS2): Stillinger-Weber parametrization, mechanical properties, and thermal conductivity. J. Appl. Phys. 114, 064307, 10.1063/1.4818414 (2013). [DOI] [Google Scholar]
  27. Liu X. J., Zhang G., Pei Q. X. & Zhang Y. W. Phonon thermal conductivity of monolayer MoS2 sheet and nanoribbons. Appl. Phys. Lett. 103, 133113, 10.1063/1.4823509 (2013). [DOI] [Google Scholar]
  28. Cai Y., Lan J., Zhang G. & Zhang Y.-W. Lattice vibrational modes and phonon thermal conductivity of monolayer MoS2. Phys. Rev. B 89, 035438, 10.1103/PhysRevB.89.035438 (2014). [DOI] [Google Scholar]
  29. Wei X. et al. Phonon thermal conductivity of monolayer MoS2: A comparison with single layer graphene. Appl. Phys. Lett. 105, 103902, 10.1063/1.4895344 (2014). [DOI] [Google Scholar]
  30. Li W., Carrete J. & Mingo N. Thermal conductivity and phonon linewidths of monolayer MoS2 from first principles. Appl. Phys. Lett. 103, 253103, 10.1063/1.4850995 (2013). [DOI] [Google Scholar]
  31. Sahoo S., Gaur A. P. S., Ahmadi M., Guinel M. J. F. & Katiyar R. S. Temperature-Dependent Raman Studies and Thermal Conductivity of Few-Layer MoS2. J. Phys. Chem. C 117, 9042–9047, 10.1021/jp402509w (2013). [DOI] [Google Scholar]
  32. Yan R. et al. Thermal conductivity of monolayer molybdenum disulfide obtained from temperature-dependent Raman spectroscopy. ACS Nano 8, 986–993, 10.1021/nn405826k (2014). [DOI] [PubMed] [Google Scholar]
  33. Liu J., Choi G. M. & Cahill D. G. Measurement of the anisotropic thermal conductivity of molybdenum disulfide by the time-resolved magneto-optic Kerr effect. J. Appl. Phys. 116, 233107, 10.1063/1.4904513 (2014). [DOI] [Google Scholar]
  34. Molina-Sánchez A. & Wirtz L. Phonons in single-layer and few-layer MoS2and WS2. Phys. Rev. B 84, 155413, 10.1103/PhysRevB.84.155413 (2011). [DOI] [Google Scholar]
  35. Kaasbjerg K., Thygesen K. S. & Jacobsen K. W. Phonon-limited mobility in n-type single-layer MoS2 from first principles. Phys. Rev. B 85, 115317, 10.1103/PhysRevB.85.115317 (2012). [DOI] [Google Scholar]
  36. Pei Y. et al. Convergence of electronic bands for high performance bulk thermoelectrics. Nature 473, 66–69, 10.1038/nature09996 (2011). [DOI] [PubMed] [Google Scholar]
  37. Poudel B. et al. High-thermoelectric performance of nanostructured bismuth antimony telluride bulk alloys. Science 320, 634–638, 10.1126/science.1156446 (2008). [DOI] [PubMed] [Google Scholar]
  38. Biswas K. et al. High-performance bulk thermoelectrics with all-scale hierarchical architectures. Nature 489, 414–418, 10.1038/nature11439 (2012). [DOI] [PubMed] [Google Scholar]
  39. Ong Z.-Y. & Pop E. Effect of substrate modes on thermal transport in supported graphene. Phys. Rev. B 84, 075471, 10.1103/PhysRevB.84.075471 (2011). [DOI] [Google Scholar]
  40. Yang N., Zhang G. & Li B. Violation of Fourier’s law and anomalous heat diffusion in silicon nanowires. Nano Today 5, 85–90, 10.1016/j.nantod.2010.02.002 (2010). [DOI] [Google Scholar]
  41. Volz S. G. & Chen G. Molecular dynamics simulation of thermal conductivity of silicon nanowires. Appl. Phys. Lett. 75, 2056, 10.1063/1.124914 (1999). [DOI] [Google Scholar]
  42. Chen J., Zhang G. & Li B. Substrate coupling suppresses size dependence of thermal conductivity in supported graphene. Nanoscale 5, 532–536, 10.1039/c2nr32949b (2013). [DOI] [PubMed] [Google Scholar]
  43. Lee J.-H., Galli G. A. & Grossman J. C. Nanoporous Si as an efficient thermoelectric material. Nano Lett. 8, 3750–3754, 10.1021/nl802045f (2008). [DOI] [PubMed] [Google Scholar]
  44. He J., Kanatzidis M. G. & Dravid V. P. High performance bulk thermoelectrics via a panoscopic approach. Materials Today 16, 166–176, 10.1016/j.mattod.2013.05.004 (2013). [DOI] [Google Scholar]
  45. Yang N., Gang Z. & Li B. Ultralow Thermal Conductivity of Isotope-Doped Silicon Nanowires. Nano Lett. 8, 276–280, 10.1021/nl0725998 (2007). [DOI] [PubMed] [Google Scholar]
  46. Tang J. Y. et al. Holey Silicon as an Efficient Thermoelectric Material. Nano Lett. 10, 4279–4283, 10.1021/Nl102931z (2010). [DOI] [PubMed] [Google Scholar]
  47. Yang L., Yang N. & Li B. Thermoelectric Properties of Nanoscale three dimensional Si Phononic Crystal. arXiv preprint arXiv:1410.8193 (2014).
  48. Liang L., Cruz-Silva E., Girao E. C. & Meunier V. Enhanced thermoelectric figure of merit in assembled graphene nanoribbons. Phys. Rev. B 86, 115438, 10.1103/PhysRevB.86.115438 (2012). [DOI] [Google Scholar]
  49. Yang N., Ni X., Jiang J.-W. & Li B. How does folding modulate thermal conductivity of graphene? Appl. Phys. Lett. 100, 093107, 10.1063/1.3690871 (2012). [DOI] [Google Scholar]
  50. Vo T. T., Williamson A. J., Lordi V. & Galli G. Atomistic design of thermoelectric properties of silicon nanowires. Nano Lett. 8, 1111–1114, 10.1021/nl073231d (2008). [DOI] [PubMed] [Google Scholar]
  51. Bux S. K. et al. Nanostructured Bulk Silicon as an Effective Thermoelectric Material. Adv. Funct. Mater. 19, 2445–2452, 10.1002/adfm.200900250 (2009). [DOI] [Google Scholar]
  52. Chang P. H., Bahramy M. S., Nagaosa N. & Nikolic B. K. Giant Thermoelectric Effect in Graphene-Based Topological Insulators with Heavy Adatoms and Nanopores. Nano Lett. 14, 3779–3784, Doi 10.1021/Nl500755m (2014). [DOI] [PubMed] [Google Scholar]
  53. Giannozzi P. et al. Quantum Espresso: a modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Mat. 21, 395502, 10.1088/0953-8984/21/39/395502 (2009). [DOI] [PubMed] [Google Scholar]
  54. Hartwigsen C., Goedecker S. & Hutter J. Relativistic separable dual-space Gaussian pseudopotentials from H to Rn. Phys. Rev. B 58, 3641–3662, 10.1103/PhysRevB.58.3641 (1998). [DOI] [PubMed] [Google Scholar]
  55. Villars, P. et al. SpringerMaterials; sm_lbs_978-3-540-32682-3_19 (Springer-Verlag GmbH, Heidelberg, 2006), http://materials.springer.com/lb/docs/sm_lbs_978-3-540-32682-3_19 10.1007/10920503_19. [DOI]
  56. Madsen G. K. H. & Singh D. J. BoltzTraP. A code for calculating band-structure dependent quantities. Comput Phys Commu 175, 67–71, 10.1016/j.cpc.2006.03.007 (2006). [DOI] [Google Scholar]
  57. Hoover W. G. et al. Lennard-Jones triple-point bulk and shear viscosities. Green-Kubo theory, Hamiltonian mechanics, and nonequilibrium molecular dynamics. Phys. Rev. A 22, 1690–1697, 10.1103/PhysRevA.22.1690 (1980). [DOI] [Google Scholar]
  58. Plimpton S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 117, 1–19, 10.1006/jcph.1995.1039 (1995). [DOI] [Google Scholar]
  59. Buscema M. et al. Large and tunable photothermoelectric effect in single-layer MoS2. Nano Lett. 13, 358–363, 10.1021/nl303321g (2013). [DOI] [PubMed] [Google Scholar]
  60. Mansfield R. & Salam S. Electrical properties of molybdenite. Proceedings of the Physical Society. Section B 66, 377, 10.1088/0370-1301/66/5/305 (1953). [DOI] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information
srep18342-s1.pdf (574.5KB, pdf)

Articles from Scientific Reports are provided here courtesy of Nature Publishing Group

RESOURCES