Equations of the End: Teaching Mathematical Modeling Using the Zombie Apocalypse

Abstract

Mathematical models of infectious diseases are a valuable tool in understanding the mechanisms and patterns of disease transmission. It is, however, a difficult subject to teach, requiring both mathematical expertise and extensive subject-matter knowledge of a variety of disease systems. In this article, we explore several uses of zombie epidemics to make mathematical modeling and infectious disease epidemiology more accessible to public health professionals, students, and the general public. We further introduce a web-based simulation, White Zed (http://cartwrig.ht/apps/whitezed/), that can be deployed in classrooms to allow students to explore models before implementing them. In our experience, zombie epidemics are familiar, approachable, flexible, and an ideal way to introduce basic concepts of infectious disease epidemiology.

INTRODUCTION

Mathematical modeling is an invaluable tool in the study of infectious diseases, with a long history of providing insights into patterns of infectious disease transmission such as herd immunity and periodic epidemics. These theoretical representations allow researchers to explore questions that would be empirically infeasible for financial, practical, or ethical reasons. These models have found utility in public health and policy spanning pandemic preparedness (9), vaccine development and deployment (17), and intervention evaluation (15). Models can also provide advice to decision makers during emerging epidemics, such as the 2014 West African Ebola epidemic (4, 20).

Two chief challenges exist in teaching mathematical modeling to students in biology or public health. The first is teaching the concepts of mathematical modeling using approachable mathematical techniques so that students may develop an intuition for the subject. This allows them to interact with models as they encounter them in the literature and to gain a solid foundation before moving on to advanced topics. The second, equally important, challenge is the use of a working example disease that allows students to explore the design, implementation, and analysis of mathematical models without needing to acquire expertise on a daunting range of pathogens.

Here, we discuss the incorporation of zombie epidemics into three different introductory programs by the authors: a one-day workshop during a conference (led by EL), a full-semester undergraduate course (taught by RC), and a public outreach event (directed by KC).

A BRIEF HISTORY OF ZOMBIES

The classic zombie is thought to have originated in Haiti as a legend associated with voodoo culture. The first feature-length zombie film was White Zombie released in 1932 (10), which told the story of a woman visiting Haiti and becoming transformed into a zombie by a voodoo practitioner. The modern zombie first appeared in George Romero’s 1968 movie, Night of the Living Dead (21). Rather than the possessed zombie of Haitian influence, this new type of zombie was a reanimated corpse intent on eating human flesh. This type of ambling, “slow” zombie, brought back to life by radiation or other means, typified popular culture for approximately 30 years. With the 2002 release of 28 Days Later (2), the zombie genre was given new life with the invention of the “fast” zombie. Unlike the Romero zombies, these were not truly dead but rather “infected” with a virus that quickly turns victims into fast-moving, violent creatures. Zombieism as an infectious disease has shown up repeatedly since, and is prominently featured in The Walking Dead graphic novel and television series (25, 12).

The recent surge of popularity has generated a variety of zombie (un)natural histories consistent with the diversity studied in infectious disease biology; they can be caused by viruses (The Walking Dead, World War Z (3), Resident Evil (1)), bacteria (Deck Z (18)), prions (Zombieland (8)), or fungi (The Last of Us (24)). The fungal scenarios are based on the real-life Cordyceps genus of endo-parasitic fungi, which infects insects and dramatically changes their behavior as it replaces host tissue (11). In zombie fiction, the most common mode of pathogen transmission is via a bite, but zombieism can also be transmitted via air (The Infection (6)), bodily fluids (28 Days Later (2)), water (The Crazies (7)), or even vectors (Neuropathology of Zombies (5)); and incubation periods can range from practically nonexistent (28 Days Later (2)) to indefinite (The Walking Dead (12)).

WHY MODEL ZOMBIES?

The popularity of zombie fiction makes it an obvious topic for the introduction of epidemiological models into the classroom. First, no prior knowledge of a biological system is necessary, as students can become experts over a night of movies, and may find discussions of zombie outbreaks more approachable. One of the authors (TCS) has examined the epidemiology of zombies in a similar manner as a way to facilitate discussions about preparing for emerging pathogens to a wide, non-scientific audience (22).

Second, due to the diversity of (un)natural zombie histories in popular culture, instructors have the flexibility to cover different models and processes in epidemiology without deviating from the zombie mythos. Instructors can implement density-dependent transmission, frequency-dependent transmission (16), environmental transmission (26), latency periods (14), asymptomatic carriers, etc. Beyond that, zombie stories involve a number of interventions, such as quarantines, culling, and social distancing, the effects of which can be explored in advanced models.

This flexibility allows for a wide range of classroom discussions about modeling infectious diseases without requiring a change in disease context every time a new concept in modeling is introduced. This is an essential feature for the teaching of modeling, as it reduces the number of variables being changed and allows students to see changes in the zombie model as entirely the result of the alteration in model structure and not switching systems. It also promotes a back-and-forth on how to model pathogens; rather than accepting that a particular disease has a canonical model form, zombie outbreaks lend themselves well to new hypothetical scenarios, from new interventions to the fundamental question of whether the zombies are the slow, shambling creatures of Romero’s works, or the swift hunters of many modern films. In our experience, students are much more willing to use their imaginations when modeling zombie epidemics than for real-life diseases, where they are often focused on learning the “true” model. Epidemiology educators may use zombie epidemics as a good “starting point” to engage in conversation with the public about experiences, real-life diseases, conversations about “good health practices” (e.g., hand washing or staying home from work or school when sick). Although the scenarios are fictional in their focus, students often encounter many of the same questions asked when modeling real-world infectious diseases: Are there interventions we have not thought of? Are our assumptions about the natural history of the disease correct? Does being ill change host behavior? Encouraging a natural exploration of these questions helps support a more critical design of models from the outset and a more contemplative review of models encountered in the literature.

ONE-DAY WORKSHOP FOR PUBLIC HEALTH PROFESSIONALS

A Gentle Introduction to Mathematical Modeling: Real-life Lessons from the Living Dead is a one-day workshop, most recently presented at the American Public Health Association’s Annual Conference in 2014. Its audience was public health professionals, most of whom had encountered mathematical models before but had little or no experience with their design or analysis.

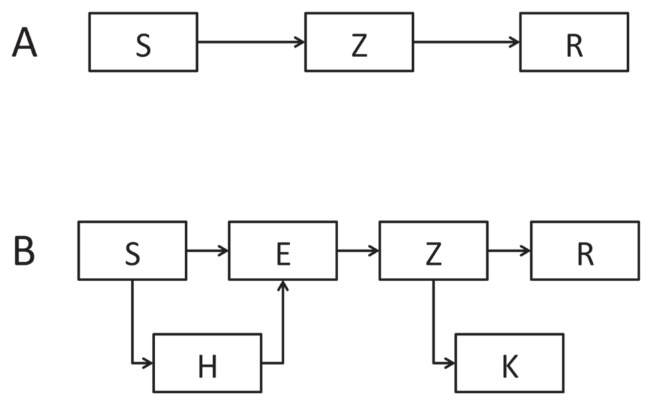
The workshop was structured in a hybrid format, with introductory lectures followed by guided, hands-on practice with coding and implementation. Each lecture featured one or several video clips illustrating the concept being discussed as it applied to modeling, e.g., a discussion between the characters in Dawn of the Dead (23) about the time it takes between someone being bitten and becoming dangerous (incubation time) or the Zombieland narrator’s discussion of the “rules” that govern his behavior when introducing agent-based models (8). Because the participants were largely non-modelers from other public health professions, an emphasis was placed on the critical evaluation of models in the literature: what assumptions they make, when these assumptions are tenable, where parameter estimates come from, etc. The course built up from a simple “SZR” model, where the population is divided up into susceptible (S), zombie (Z), or removed (R) classes, to a much more complex model involving survivors finding shelter, fighting back against zombies, etc. (Fig. 1).

FIGURE 1.

Schematic representation of two models used during one-day modeling workshop. Panel A depicts a simple “SZR” model, wherein the population is divided into three compartments: Susceptible (S), Zombies (Z), and Removed (R). Panel B depicts a more complex model, adding compartments for individuals who have been infected but not yet turned into zombies (E), susceptible individuals who have found shelter (H), and zombies who were killed during interactions with susceptible individuals (K).

The hands-on portion of the workshop continued with the same emphasis on critical evaluation, while the students were given a chance to implement two models on their own: the basic zombie epidemic model introduced during the lecture and a worked example model from the literature (13). The code for every compartmental model used during the workshop, as well as the hands-on section, was made available in both Python and R at the workshop’s GitHub repository (https://github.com/elofgren/zombies) for students to refer to later. Both this workshop and the course discussed below primarily used difference-equations models, which allow for many of the same insights as more advanced differential-equation models, but require no more than a high school level understanding of algebra.

Further discussion focused on many of the problems encountered while implementing models, such as balancing a desire for realism with computational and mathematical tractability, or the sensitivity of results to unexpected parameters, to try to give students an understanding of when they should seek the assistance of a modeling specialist. This was meant to demystify what that specialist does so as to combat the “black box” mentality that sometimes exists about mathematical modeling, where models are given only a cursory evaluation by subject-matter experts because of the presence of equations and their results accepted without critique.

A SEMESTER-LONG COURSE

RC taught Biology of Infectious Diseases at Rice University in fall 2011, which included a semester-long group modeling project involving zombies. The class (approximately 100 students) was divided into 20 groups. Each group was given one of five zombie scenarios:

1. Island Zombies: the outbreak occurs initially on an island, which has a sister island. Migration is possible between them.

2. Leper Zombies: the zombie outbreak co-occurs with an outbreak of leprosy. Zombies infected with leprosy decay at a fast rate.

3. Redhead Zombies: only certain people (genetic redheads) become zombies when they are infected. Everyone else dies.

4. Vector Zombies: zombieism is transmitted by an insect vector (frequency-dependent transmission).

5. Reservoir Zombies: zombieism is transmitted by contact with an animal reservoir (density-dependent transmission).

The groups developed their model throughout the semester, writing two drafts and a final paper. Students were encouraged to embellish as they saw fit. For the first, five-page draft, the groups had to outline their scenario and define a suitable difference-equation model for the transmission of zombieism. They had to explicitly define their assumptions, variables, and parameters, and write such that someone who was not taking the class could understand what they were doing. For the second, eight-page draft, the groups updated their models to include natural birth and death of survivors and hunting of zombies. They extended their existing work by analyzing their models to determine possible equilibrium values and the conditions under which zombieism increases or decreases. This analysis consisted of analytical solutions and simulations conducted in Excel. A spreadsheet program like Excel makes it possible for students with no programming experience to implement recursion and visualize the results.

For the final, 15-page paper, the groups extended their drafts by fitting their models to datasets provided by RC, estimating the model parameters and predicting the future of their scenarios. The datasets were designed to only partially match scenarios to make students think about why their “real” data and model did not agree. The students fit their models using the Excel simulations they constructed in part two; Excel’s Solver or Poptools (downloadable from www.poptools.org) were used by groups to optimize the fit of their model to the data.

Groups also presented their findings to the class throughout the semester. One group from each scenario presented their results when draft 1 and draft 2 were due, and the remaining groups presented when the final paper was due. All papers were shared on the internal, class website, and students were encouraged revise their projects based on ideas found in the work of other groups, both in their scenario and in other scenarios. As part of the final exam, students were tested on what they learned about each of the five scenarios.

PUBLIC HEALTH EDUCATION AND OUTREACH

The zombie scenario is an engaging and fun way of teaching immunity, vaccination, and basic epidemiology not only to public health professionals or college students, but also to younger students at science-focused outreach events. Chemical transference or paper-and-pencil are standard school activities used to simulate the spread of disease.

The Biocomplexity Institute of Virginia Tech has created a viral transmission simulation activity known as Virus Tracker (https://virustracker.vbi.vt.edu) that includes sophisticated visualization and analysis of the epidemic’s spread. Virus Tracker simulates the spread of a virus and the critical role of vaccination in combating a disease outbreak. It involves a game host who controls various aspects of the outbreak, maintains a record of the players’ states, collects statistics on the players’ states, and provides visualizations of the outbreak’s progress. Infected players can transmit the dreaded (but imaginary) Zombie Plague Virus to their contacts. Game play is deepened by the opportunity for players to vaccinate others. The virus mutates under control of the game’s host, so that regular re-vaccination is needed to maintain immunity. Players answer questions related to public health correctly to obtain a vaccine they can distribute and are awarded points both for vaccinating and for infecting other players.

A variety of events utilizing Virus Tracker have been extremely well received including the 2014 National Boy Scout Jamboree, which involved 29,000 infected players.

The most current version of Virus Tracker is a game that can be played with address labels and/or an App (available on Google Play and the Apple App store). The dual approach in play is meant to allow any age group, with any level of technological sophistication or logistical support, to be accommodated. Young children and adults without smart phones are the key users for the label-based game.

This dual approach allowed, for example, a pair of siblings, one with a smart phone and another without, to compete with each other to infect or vaccinate participants, generating excitement about the game and, as a result, the epidemiological concepts behind it.

A version of this game, Virus Tracker-in-a-Box (http://vtib.vbi.vt.edu), has also been developed for use in middle and high school classrooms. In this version, players are given a bar coded address label to represent their infection with a virus. The first person to be infected (patient zero) can choose how many wristbands to give away. Those receiving the next round of bands can also choose how many others to infect and so on until all bands are accounted for. Each game kit includes a scanner so that the barcodes can be scanned and linked together in a database to show a visualization of the resulting transmission tree, as well as other statistics about the epidemic. Each participant can find his or her place in the tree, and trace the transmissions backwards to find patient zero. The game kit itself is free and includes the needed software, scanners, barcodes, instruction manual, and suggested curriculum materials.

SOFTWARE RESOURCES

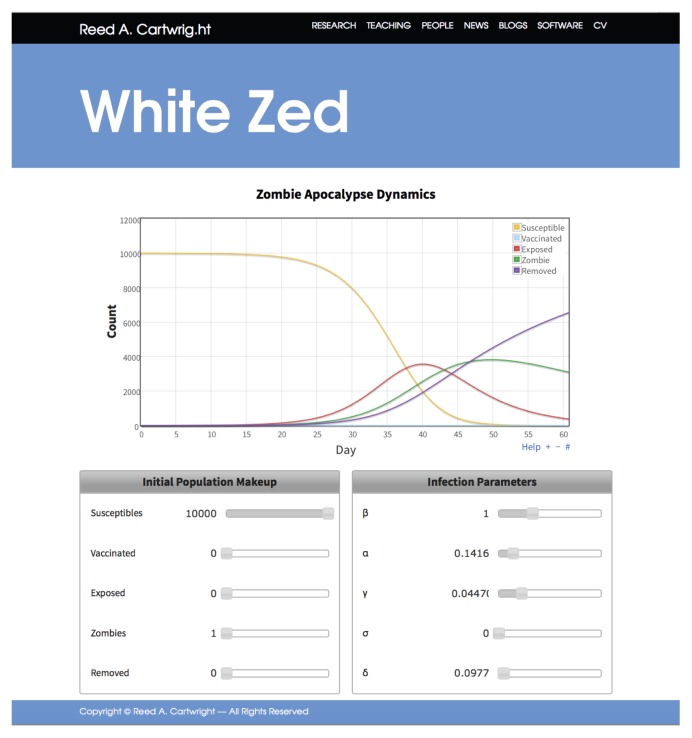
In order to facilitate the translation of our experiences using zombie epidemics to teach infectious diseases, we have developed White Zed, a web-based application available at http://cartwrig.ht/apps/whitezed/ (Fig. 2). White Zed is designed to allow for interactive exploration of mathematical models of disease, and is a starting point for introducing zombie epidemics into class work. At its core, White Zed contains a modified SVEIR differential-equation model, integrated using the Runge-Kutta method (19). Students use sliders to adjust disease parameters and the makeup of the population at time 0. The resulting epidemic is visualized by a graph that plots the number of individuals in each category versus time. This graph updates every tenth of a second so students can see the effect of their parameter changes in real time. All of this is achieved by performing the calculations in the student’s web browser; there is no backend, and our server only hosts the static files needed to define White Zed.

FIGURE 2.

Screenshot of the White Zed simulation website. The graph displays population dynamics of a zombie apocalypse. The left control panel allows users to specify the population makeup at the beginning of the simulation, while the right control panel allows users to specify the infection parameters of the disease.

We refer to White Zed’s model as “SVEZR” to reflect the involvement of zombieism. This model was chosen to allow instructors the flexibility to start with simple models and expose students to more complicated dynamics by enabling additional features. The SVEZR model contains five classes of individuals that interact in a prescribed way. Individual survivors (S) come into contact with infectious zombies (Z) and become exposed (E) to the disease at rate β. Exposed individuals become zombies with incubation rate α, and zombies survive for a period of time before succumbing with rate γ to decay, accidents, etc. and being permanently removed (R) from the population. This model also contains vaccinated individuals (V), who do not become zombies even if they are exposed; the vaccination rate is ζ. In addition to this typical epidemiological model, SVEZR allows S, V, and E individuals to destroy zombies during interactions at rate δ.

Several common epidemiological models can be modeled within White Zed by setting only specific parameters to non-zero. For example, if β and γ are non-zero, White Zed simulates an SZR analog of the classic “Susceptible-Infected-Removed” model. Making α non-zero introduces a latency period (the SEZR model). These models and more like them are fully described in Appendix 1. A sample student worksheet appropriate for a standalone lecture introducing mathematical modeling is included in Appendix 2.

CONCLUSION

Models of infectious disease play an important role in our understanding of how pathogens spread through populations. Important concepts like herd immunity and critical vaccination thresholds, the role of social structure in the spread of disease, and the potential effectiveness of many public health interventions can be easily demonstrated using mathematical and computational models. Treating mathematical models as more than simply a black box from which results emerge is essential for critical engagement with the infectious disease literature. As such, it is important that students of biology, epidemiology, and public health be conversant in their use, interpretation, and limitations, whether or not they intend to use models in their own work.

We have described the use of zombie epidemics to introduce fundamental concepts in infectious disease modeling and epidemiology in three different settings with students at very different levels. In each setting, we sought to use a familiar cultural reference point in order to help students engage with the concepts behind infectious disease modeling without the barrier of extensive disease-specific expertise. This approach is not intended to be an endpoint in students’ education about epidemiology, but rather, it brings the teaching of infectious disease modeling into line with how biology, epidemiology, and public health are often taught, with subject-matter expertise being acquired after, or alongside, general methodological sophistication, rather than acting as an impediment to it.

SUPPLEMENTAL MATERIALS

Appendix 1: Model descriptions

Appendix 2: Example worksheet