Abstract
Two-dimensional layered transition metal dichalcogenides (TMDCs) show great potential for optoelectronic devices due to their electronic and optical properties. A metal-semiconductor interface, as epitaxial graphene - molybdenum disulfide (MoS2), is of great interest from the standpoint of fundamental science, as it constitutes an outstanding platform to investigate the interlayer interaction in van der Waals heterostructures. Here, we study large area MoS2-graphene-heterostructures formed by direct transfer of chemical-vapor deposited MoS2 layer onto epitaxial graphene/SiC. We show that via a direct transfer, which minimizes interface contamination, we can obtain high quality and homogeneous van der Waals heterostructures. Angle-resolved photoemission spectroscopy (ARPES) measurements combined with Density Functional Theory (DFT) calculations show that the transition from indirect to direct bandgap in monolayer MoS2 is maintained in these heterostructures due to the weak van der Waals interaction with epitaxial graphene. A downshift of the Raman 2D band of the graphene, an up shift of the A1g peak of MoS2 and a significant photoluminescence quenching are observed for both monolayer and bilayer MoS2 as a result of charge transfer from MoS2 to epitaxial graphene under illumination. Our work provides a possible route to modify the thin film TDMCs photoluminescence properties via substrate engineering for future device design.
The study of graphene, a two dimensional (2D) atomic crystal formed of carbon atoms arranged in a honeycomb structure, is one of the hottest topics in material science due to its unique capabilities1,2. The importance of graphene not only lies in its properties but also on the fact that it opened the way and promoted the synthesis of many other 2D materials3. The last 10 years of research on graphene have led to many methods for synthesizing, transferring, manipulating and characterizing the properties of this 2D material, which can be applied to all layered van der Waals (vdW) materials. As one has full control of the 2D crystals, one can also create a stack of these crystals in a completely new heterostructures. Since the portfolio of the original 2D crystals is very rich4,5,6, a new world of materials is accessible. Combining different 2D layers with complementary characteristics can lead to new vdW heterostructures with tunable properties leading to an outstanding range of possible applications7,8. Among these systems, the combination of a transition metal dichalcogenide (TMDCs) such as MoS2 and graphene, forming an heterostructure is very interesting, since it combines the excellent optical properties of MoS2 and the high mobility and transparency of graphene9,10,11,12.
One well-established method to produce high quality wafer-scale monolayer graphene is the epitaxial approach based on the graphitization of the Si face of SiC13,14. A considerable advantage of this technique lies in the fact that the wide band gap semiconductor SiC wafers can be employed as a substrate, so that no additional transfer step is required to conduct electrical or optical measurements. To our knowledge, until now there are few works discussing the possibility of growing TMDCs materials (MoS2 or WS2) on epitaxial graphene/SiC15,16,17,18. In particular a direct growth approach by Chemical Vapor Deposition (CVD)15,16,18,19,20,21 or metal-organic chemical vapour deposition (MOCVD)17 is generally used. Even if the authors obtained high quality interfaces between TMDCs film and graphene, the direct growth method suffers of the fact that the obtained TMDC grain size is small (ranging from hundreds of nm to few microns). However the possibility to obtain large area TMDCs/graphene heterostructures is important for a large variety of in situ characterization techniques, and is also a basic requirement for realistic applications. Recently Han et al. developed a novel seeded CVD patterned growth method to obtain highly crystalline MoS2 flakes on oxidized silicon substrate22. MoS2 grown by this approach has high crystallinity, with large flakes (between 20–100 μm) and electrical and optical properties comparable to exfoliated material. These CVD-grown flakes are suitable for transfer onto epitaxial graphene/SiC substrate in order to obtain large area of MoS2/graphene heterostructures. Using a transfer process (see results and discussion and Fig. 1(a)) we have obtained monolayer and bilayer MoS2/graphene heterostructures, allowing the study of interlayer interaction between TDMCs materials and graphene on a large scale, using several complementary techniques. Angle resolved photoemission spectroscopy (ARPES) measurements were used to study the electronic structure of the MoS2/epitaxial graphene heterostructure. Due to a weak interlayer coupling the electronic structure of graphene and MoS2 are well retained in their respective layers. However the band alignment in the MoS2/graphene heterostructure allows under illumination a charge transfer process from MoS2 to graphene. This is revealed by a downshift of the graphene 2D Raman bands, an upshift of the A1g Raman band of MoS2, and a strong quenching of photoluminescence (PL) of MoS2/graphene heterostructure. Complementary we performed photocurrent measurements to elucidate how the presence of the semiconductor affects the photoconductive properties of graphene. In that respect, this work may open a new way in graphene optoelectronics by modulating the graphene photoelectric response through 2D materials interfacing.
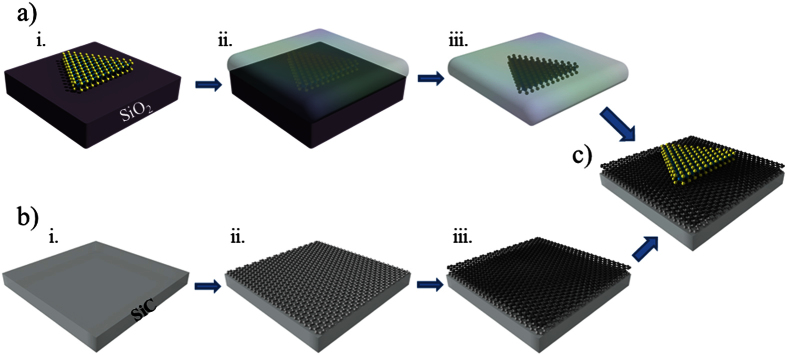
Figure 1.
Schematic transfer process of MoS2 on epitaxial grapheme: (a) i. Growth of MoS2 on Si/SiO2. ii. Coating of PMMA on the top of the sample iii. KOH etching of SiO2 substrate. (b) i. 4H-SiC substrate. ii. Interface layer. iii. Monolayer epitaxial Graphene. (c) Monolayer MoS2 on epitaxial grapheme.
Results and Discussions
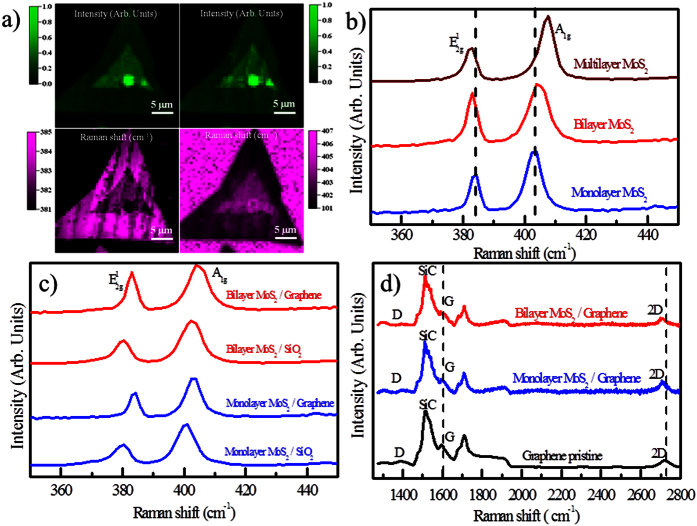
TMDCs/graphene heterostructures were made from MoS2 flakes grown by chemical vapor deposition on oxidized silicon substrates that were then transferred onto epitaxial graphene grown on SiC(0001) (Fig. 1(a)). The graphene underlayer used in this study was obtained by annealing 4H-SiC(0001) (see methods) (Fig. 1(b)i–iii). The CVD growth procedure of MoS2 on SiO2 results in characteristic single-crystal domains shaped as well-defined equilateral triangles22 (Figure S1). The single- crystal flakes with mono, bi and multilayer thicknesses were identified by their optical contrast and characteristic triangular shape, and further confirmed by micro-Raman, and micro photoluminescence (micro-PL) measurements. For the transfer step, we spin-coated PMMA onto the MoS2 flakes and peeled them off from the SiO2 substrate by wet etching in KOH solution (Fig. 1(a)ii,iii). Afterward, we transferred the PMMA/MoS2 layer onto the graphene/SiC substrate (Fig. 1(c)). We finally removed the PMMA using acetone. Due to the high density of MoS2 flakes on the Si/SiO2 substrate (50% of the total area of the sample), we were able to obtain several flakes with various stacking order and orientation in a single transfer step (Fig. 2(a)). The MoS2 domains transferred onto the graphene retain their triangular shapes with lateral sizes of ~20 to ~100 μm. To further clean the surface and interface of the MoS2/graphene heterostructure, we annealed the samples at T = 300 °C for 30 mn in UHV (base pressure below P ~ 10−10 mbar). For the following experiments, the monolayer, bilayer and multilayer coverage was estimated from optical analysis to be around 83%, 15% and 2% respectively.
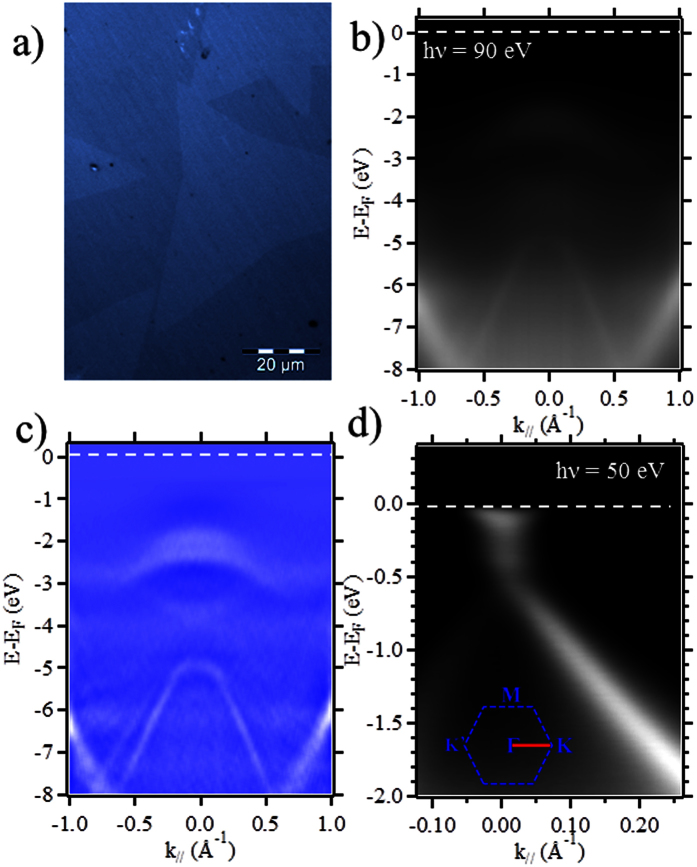
Figure 2.
ARPES measurement for monolayer and bilayer MoS2/graphene heterostructures: (a) typical optical image of the MoS2 transferred on epitaxial graphene layer, (b) ARPES measurements of MoS2/graphene measured at hν = 90 eV along to the K′ΓK direction of graphene Brillouin zone, and (c) Second-derivative spectra of (b) to enhance the visibility of the bands. (d) ARPES spectrum around the K point of graphene of the MoS2/graphene heterostructure at hv = 50 eV. The white dotted lines in panels (b–d) indicate the Fermi level position.
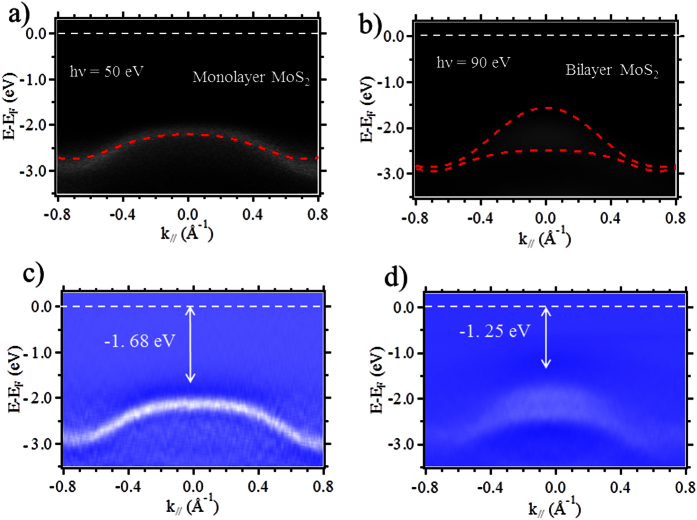
In order to better understand the electronic properties of the MoS2/graphene/SiC heterostructure, we measured its band structure by angle-resolved photoemission spectroscopy (ARPES) at the Cassiopée beamline of Synchrotron Soleil. The small x-ray spot size (50 × 50 μm2) allowed the measurement of the band structure of a single flake (monolayer or bilayer) forming the MoS2/graphene heterostructure. The photoelectron intensity is presented in Fig. 2(b) as a function of energy and k-momentum, along the K′–Γ–K direction of the first graphene Brillouin zone. The second-derivative spectrum in Fig. 2(c) is provided to enhance the visibility of the band structure. The zero of the binding energy (i.e., the Fermi level) was determined by fitting the leading edge of the graphene layer at the same photon energies and under the same experimental conditions. Beside the typical linear dispersion of the π bands of graphene, a new set of bands is visible at the Γ point of the Brillouin zone independently of the orientation angle between the flake and the graphene underlayer, which is the signature of MoS2 valence band. A close inspection of the K point of graphene Brillouin zone is shown in Fig. 2(d). This spectrum is obtained orienting the sample along the Γ-K direction of the graphene Brillouin zone. In this case the mismatch angle between MoS2 flake and graphene underlayer is critical. In order to obtain a perfect alignment of the Brillouin zone of the two materials we need a mismatch angle of zero degree. As we can see in Fig. 2(d), along the Γ-K direction, we only see the graphene signature. The two spin split bands expected at the K(K′) point23,24 of MoS2 are not visible indicating a non-zero mismatch angle for this flake. However we clearly see the graphene band structure, in particular the π bands of graphene preserve their linearity characteristic of a massless Dirac fermions signature, indicating a high structural quality of the MoS2/graphene heterostructure. Moreover, similar to pristine monolayer graphene, the Dirac point (ED) is located at 0.3 eV below the Fermi level (FL). From a linear fit, using the relation E = ħvFk, we obtain the value of the Fermi velocity vF ~ 1.1 × 106 m/s, which matches the expected value for monolayer graphene on SiC. As the linear dispersion and Fermi velocity of the pristine graphene is preserved in the MoS2/graphene heterostructure, we can infer that the MoS2 transfer does not affect the electronic structure of monolayer graphene in the heterostructure formation. In addition, this feature is expected theoretically since in van der Waals heterostructures, the superposition of each layer electronic structure constitutes a good approximation of the electronic structure of the multilayer. Differently from previous work of Diaz et al. no signature of interlayer hybridization25 is present on the π-band of graphene. This is probably due to the mismatch angle between the MoS2 flake and the graphene underlayer. However identifying the dependence of this effect on the mismatch angle would clearly require a further work and go much beyond the main objective of this paper. Figure 3(a,b) show a direct comparison of the calculated band structures (see method) and the corresponding ARPES spectra of the monolayer and bilayer MoS2 on epitaxial graphene, along the K-Γ-K direction in the hexagonal Brillouin zone, the respective second derivative are shown in Fig. 3(c,d). Comparisons with our Density Functional Theory (DFT) calculations clearly show that the monolayer, bilayer-dependent band structure evolution shows excellent agreement with theoretical calculations. Monolayer MoS2 presents only one band at the Γ point (maximum at Binding Energy (BE) ~ −1.68 eV ± 0.05 eV), and this structure evolves into two branches in the case of bilayer MoS2 (maximum at BE ~ −1.25 eV ± 0.05 eV). This evolution is representative of the splitting of the bands due to the weak van der Waals interaction between the two MoS2 layers. The relative position of the top of the valence bands at the Γ point in the bilayer film is closer to the Fermi level than the one obtained from the monolayer (Figure S2). This indicates that MoS2 undergoes a crossover from an indirect to a direct bandgap in monolayer9,23,26, as predicted theoretically (Figure S3). Indeed, we can observe from Figure S3 (a) to (c) the evolution of the band structure of MoS2 from monolayer to bi- and trilayer, calculated in DFT. Even though the trilayer MoS2 has not been considered in details here experimentally, we show the corresponding DFT result to exhibit the evolution of the band structure when considering multilayer MoS2. In the bi- and trilayer systems, the top of the valence band is located at the Γ point, yielding an indirect band gap with the bottom of the conduction band between the K (K′) and Γ points. However, when considering the monolayer band structure, the top of the valence band becomes very flat near the Γ point, leading to a direct gap at the K (K′) point. The evolution of the valence band at the Γ point provides a straightforward method to identify the thickness of ultrathin MoS2 films, and also proves the high quality of MoS2 transferred on epitaxial graphene. The epitaxial graphene underlayer does not affect the MoS2 band structure, as expected for a van der Waals heterostructure. However, we do not exclude the presence of the universal buckled form of 2D crystal in our MoS2 layer3.
Figure 3.
Electronic structure of monolayer and bilayer MoS2/graphene heterostructures: (a,b) ARPES spectra and calculated band structures (red dotted lines) of monolayer and bilayer MoS2 thin films at hν = 50 and 90 eV respectively. (c,d) Second-derivative spectra of (a,b), respectively, to enhance the visibility of the bands. White dotted lines indicate the Fermi Level position. The arrows indicate the distance of the top of the valence band at the Γ point to the Fermi level.
To further investigate the electronic properties of mono and bilayer MoS2/graphene heterostructure, micro photoluminescence (micro-PL) and micro-Raman measurements are performed at room temperature (see method).
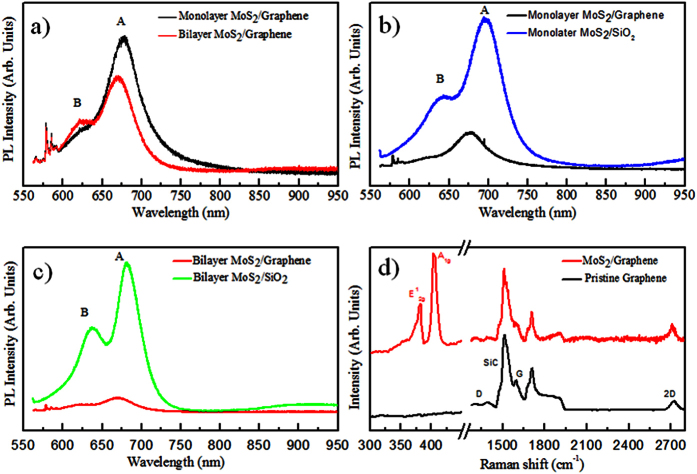
The PL spectra present the two characteristic excitonic peaks A and B27,28,29,30,31 originating from the transition at the K-point of the Brillouin zone (Fig. 4(a)). The spectra also reflect the band structure change from indirect band gap semiconductor of 2ML MoS2 to direct gap semiconductor in monolayer MoS2. The PL signal is strongly enhanced when a direct band gap is present. The same behavior was also observed for the as grown MoS2 on SiO2 (Figure S4). From the PL spectrum we can extrapolate the band gap value, which corresponds to 1.83 eV in agreement with our DFT calculations and previous experimental works32,33,34,35,36.
Figure 4.
Photoluminescence and Raman spectra for monolayer and bilayer MoS2/graphene heterostructures: (a) Photoluminescence spectra of MoS2/graphene heterostructures sample for monolayer (black data) and bilayer (red data) MoS2, (b,c) Photoluminescence spectra of MoS2/graphene heterostructures and MoS2/SiO2 sample for monolayer (MoS2/graphene black data and MoS2/SiO2 blue data) and bilayer respectively (MoS2/graphene red data and MoS2/SiO2 green data), (d) Graphene wavenumber region of the Raman spectra of pristine graphene (black data) and graphene capped with MoS2 (red data).
In the case of vertical vdW heterostructures, possible PL intensity variations could arise from the interference effects due to different optical constants and thickness of the different layers forming the heterostructure37. Buscema et al. defined in their work37 a substrate-dependent enhancement factor Γ−1 which allows a normalization procedure of the spectra taking into account the effect of optical interferences37, for the ML MoS2 on different substrate. Following their results we can see that Γ−1 ~ 1 in the case of SiO2 substrate. In the case of very thick FLG (15 nm), Γ−1 is between 8–14, and presents a huge decrease as the thickness of graphene layer is reduced (~2 in the case of 5 nm FLG). In our case, we have only one graphene layer, meaning that as for SiO2 we can suppose Γ−1 ~ 1. Therefore, in both cases we can neglect the effect of optical interferences, and directly compare the PL raw data of MoS2/SiO2 and MoS2/graphene heterostructures as shown in Fig. 4(b,c). In MoS2/SiO2 heterostructure strain can exist due to the different thermal expansion coefficients of MoS2 and the SiO2 substrate during the MoS2 flakes growing38. The transfer process on the graphene substrate due to the weak van der Waal forces at the interface releases the lattice strain39. This effect is reflected on the PL spectrum as a blue-shift of the A peak in the MoS2/graphene heterostructure38,39,40. Moreover, the PL signal in the case of graphene underlayer is strongly quenched (about 60–70% with respect to the SiO2 substrate). This phenomenon was explored also as a function of the laser power. In Figure S5 the integrated PL intensity as a function of excitation power is shown. As expected in this range of powers (between 0.5 mW and 25 mW) the MoS2 PL intensity evolves linearly with increasing laser excitation41,42 for both substrate SiO2 and graphene. But in the case of graphene underlayer the PL signal is quenched for each laser power.
This phenomenon is the signature of electron transfer from MoS2 to the graphene which hinders the recombination of electron−hole pairs created by the photoexcitation32,43. This electron transfer is not attributed to a strong coupling between MoS2 and graphene (since we consider a weak van der Waals interaction for this structure), but rather to a standard hopping from an electron in MoS2 conduction band to an unoccupied state at the same energy in graphene43,44.
Figure 4(d) shows typical Raman spectra of the
MoS2/graphene heterostructure and pristine graphene layer45 in the wavenumber range of
300–2800 cm−1. Besides the
typical second-order Raman bands that originate from the SiC substrate, the three
main structures typical of graphene are present on the pristine graphene and
MoS2/graphene spectra: i) the D band (defect induced mode), ii) the G
band (in-plane vibration mode) and iii) the 2D band (two-phonon mode)46. In the case of MoS2/graphene heterostructure within the wavenumber
range between 350–450 cm−1 two
new peaks are present. These two characteristic features correspond to the in-plane
vibration (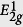 ) and out of plane (A1g) of Mo
and S atoms in the MoS2 film34,47. The intensity and
Raman shift (Δ) maps of the A1g and
) and out of plane (A1g) of Mo
and S atoms in the MoS2 film34,47. The intensity and
Raman shift (Δ) maps of the A1g and 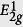 are shown in Fig. 5(a). The intensity and the Raman
shift Δ increase with the number of MoS2 layers. The average
Raman spectra obtained from each layer are shown in Fig. 5(b).
The obtained values of Δ are
~19 cm−1,
~21 cm−1 and
24 cm−1 corresponding to monolayer,
bilayer and multilayer (~three layers) MoS2,
respectively34,47. To further examine the role of the
interaction with the substrate, we compare the MoS2 on graphene and on
SiO2 substrates (Fig. 5(c)). After the transfer
we observed that the A1g and
are shown in Fig. 5(a). The intensity and the Raman
shift Δ increase with the number of MoS2 layers. The average
Raman spectra obtained from each layer are shown in Fig. 5(b).
The obtained values of Δ are
~19 cm−1,
~21 cm−1 and
24 cm−1 corresponding to monolayer,
bilayer and multilayer (~three layers) MoS2,
respectively34,47. To further examine the role of the
interaction with the substrate, we compare the MoS2 on graphene and on
SiO2 substrates (Fig. 5(c)). After the transfer
we observed that the A1g and 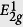 of the 1ML and
2ML MoS2 on graphene upshifted by about 4 and
3 cm−1 and 3 and
2 cm−1, respectively, meanwhile the line
widths of these peaks, calculated by the full widths at half maximum (fwhm)
result of a Lorentzian fitting, are narrower than those on SiO2
(~3 cm−1 smaller for 1 ML and
~2 cm−1 for the 2 ML for both
peaks). As in the case of the PL spectra, the upshift of the in plane Raman mode
(
of the 1ML and
2ML MoS2 on graphene upshifted by about 4 and
3 cm−1 and 3 and
2 cm−1, respectively, meanwhile the line
widths of these peaks, calculated by the full widths at half maximum (fwhm)
result of a Lorentzian fitting, are narrower than those on SiO2
(~3 cm−1 smaller for 1 ML and
~2 cm−1 for the 2 ML for both
peaks). As in the case of the PL spectra, the upshift of the in plane Raman mode
(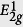 ) is the result of tensile strain release
after the MoS2 transfer on the graphene underlayer38,39,40,48. The A1g mode shows a weaker strain
dependence than the
) is the result of tensile strain release
after the MoS2 transfer on the graphene underlayer38,39,40,48. The A1g mode shows a weaker strain
dependence than the 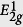 . Consequently, its large upshift
upon the transfer can be explained as the result of additional effects: i) the
establishment of a van der Waals interaction between MoS2 and
graphene47, and ii) a decrease in the electron concentration of
the MoS249. This latest, as shown by the micro-PL
measurements is due to a charge transfer under illumination from MoS2 to
graphene. As illustrated by Zhou et al., the important upshift of the
A1g is also a signature of the high quality of interface between
MoS2 and graphene48. Moreover, when MoS2 is
transferred from SiO2 to graphene the reduced substrate surface roughness
and impurities as well as the similar lattice structure are responsible for the
narrowing of the MoS2 Raman features50.
. Consequently, its large upshift
upon the transfer can be explained as the result of additional effects: i) the
establishment of a van der Waals interaction between MoS2 and
graphene47, and ii) a decrease in the electron concentration of
the MoS249. This latest, as shown by the micro-PL
measurements is due to a charge transfer under illumination from MoS2 to
graphene. As illustrated by Zhou et al., the important upshift of the
A1g is also a signature of the high quality of interface between
MoS2 and graphene48. Moreover, when MoS2 is
transferred from SiO2 to graphene the reduced substrate surface roughness
and impurities as well as the similar lattice structure are responsible for the
narrowing of the MoS2 Raman features50.
Figure 5.
Raman results for monolayer and bilayer MoS2/graphene
heterostructures: (a) Raman maps images of the peaks position and the
peaks intensity of the 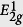 and A1g
modes of MoS2 on epitaxial graphene, (b) Raman spectra of
monolayer, bilayer and multilayer MoS2 on graphene, (c)
Comparison of the Raman spectra of monolayer and bilayer MoS2 on
epitaxial graphene and on SiO2. (d) Raman spectra of
pristine graphene and MoS2/graphene heterostructures sample.
and A1g
modes of MoS2 on epitaxial graphene, (b) Raman spectra of
monolayer, bilayer and multilayer MoS2 on graphene, (c)
Comparison of the Raman spectra of monolayer and bilayer MoS2 on
epitaxial graphene and on SiO2. (d) Raman spectra of
pristine graphene and MoS2/graphene heterostructures sample.
A detailed analysis of the Raman spectra of the monolayer and bilayer
MoS2/graphene heterostructure and pristine graphene layer in the
wavenumber range of
1300–2800 cm−1 are shown in
Fig. 5(d). As explained before the presence of graphene is
indicated by three main structures: i) the D band, ii) the G band and iii) the 2D
band. The D peak is small (~1% of the G peak intensity), indicating the
high quality of pristine graphene. The intensity of this peak did not increase after
transfer, suggesting that the MoS2 transfer process did not induce
defects in the graphene substrate. The Raman spectra of the graphene below
MoS2 domains showed clear differences from that of the free-graphene
areas. First, we observe a broad background which increases with higher wavenumbers.
This background comes from the PL of MoS2, which confirms the presence of
both graphene and MoS2 in the measured area. Second, the intensity of the
2D band was reduced by MoS2. Third, both the G and the 2D bands are
shifted. In pristine graphene the 2D band is located at
2722 cm−1 and it is shifted at 2710, and
2708 cm−1 for monolayer and bilayer
MoS2, respectively. There are two factors that can influence the
Raman 2D band position: charge transfer45,51,52, and strain53,54,55. In our Raman measurements, the spectra were taken at room
temperature and the laser power was low (~5 mW) to avoid the
influence of laser heating. Thus, the observed 2D band downshift does not originate
in differences of temperature, which can induce different strain in graphene and
MoS2. It is known that depending on the introduced carriers, the 2D
band position shifts differently56, with up- and downshifts
corresponding to hole and electron doping, respectively51. Then in
MoS2/graphene heterostructure this downshift indicates an increase in
the electron concentration in graphene under illumination (n-type doping)53. This phenomenon is in agreement with the up-shift of the Raman
feature of the MoS2, and confirms that the photoelectrons generated by
the Raman laser are transferred from the MoS2 to graphene. At the same
time the G peak presents an upshift. If we focus our attention on monolayer
MoS2/graphene we have an upshift of
~3 cm−1 ± 1cm−1.
From this shift we can obtain a quantitative analysis of the level of electron
doping under illumination53,57,58,59. In fact the G peak frequency
blue-shifts linearly with the Fermi level position as 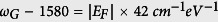 .
From this expression, we estimate the Fermi level position with respect to the Dirac
point for pristine graphene and 1ML MoS2/graphene as
.
From this expression, we estimate the Fermi level position with respect to the Dirac
point for pristine graphene and 1ML MoS2/graphene as 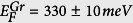 and
and 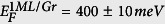 , respectively. Then the
upshift of the G mode of ~3 cm−1
implies a change in the graphene Fermi level of ~70 meV.
From these values we can calculate the variation in the n-doping of graphene using
the relation
, respectively. Then the
upshift of the G mode of ~3 cm−1
implies a change in the graphene Fermi level of ~70 meV.
From these values we can calculate the variation in the n-doping of graphene using
the relation 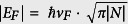 , and the value of the Fermi velocity
obtained before by the linear fit of the Dirac cone in Fig.
2(d)
(vF = 1.1 × 106 m/s),
we obtained an electron density for pristine graphene
|N| ~ 7 × 1012 cm−2
which increases to
|N| ~ 1013 cm−2
for 1ML MoS2/graphene under illumination.
, and the value of the Fermi velocity
obtained before by the linear fit of the Dirac cone in Fig.
2(d)
(vF = 1.1 × 106 m/s),
we obtained an electron density for pristine graphene
|N| ~ 7 × 1012 cm−2
which increases to
|N| ~ 1013 cm−2
for 1ML MoS2/graphene under illumination.
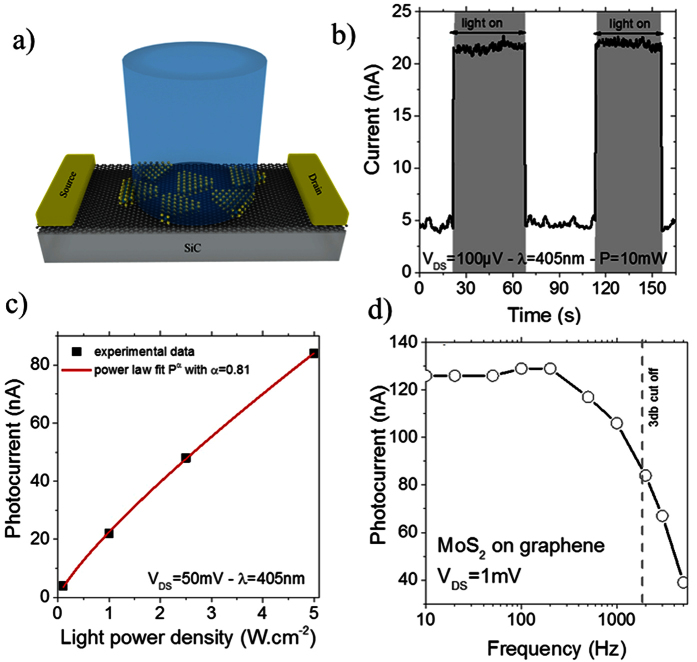
Phototransport properties of the sample are investigated in a planar geometry (i.e. the electrodes are connected to MoS2 decorated graphene) at room temperature while illuminating the sample with light energy (λ = 405 nm (hν ≈ 3 eV)) above the MoS2 band gap (Fig. 6(a)). We prepared a lateral device by standard optical lithography. We used dry etching to define a graphene mesa and titanium/gold contacts (20/200 nm). Compared to pristine graphene the photoresponse of the vdW heterostructure is significantly enhanced and a clear modulation of the current is observed under illumination, see Fig. 6(b). The generated photocurrent presents almost no dependence with the applied bias (Figure S6). Similar behavior has already been observed and attributed to thermoelectric effect60 and the later result from the inhomogeneous distribution of the MoS2 flake at the scale of the light source spot. On the other hand, if the size of the device is reduced down to a single MoS2 flake, the phototransport is very different since we observe a more usual photoconductive behavior, where the slope of the I-V curve chnage under illumination (Figure S7).
Figure 6.
(a) Scheme of the device based on epitaxial graphene MoS2 flakes, (b) Current as a function of time under constant applied electric field while the light is turned on and off (VDS = 0.1 mV − λ = 405 nm), (c) photocurrent as a function of the light intensity under constant applied electric field (VDS = 50 mV − λ = 405 nm), (d) frequency dependence of the photocurrent under constant applied electric field and light intensity.
Since the spot diameter of the laser is 1 mm2, and the density of the MoS2 fakes is about 30% of the sample we can estimate the responsivity to be around 6 μA.W−1. This limited value is the result of the limited absorption of the semiconductor (MoS2) because of its thickness and limited coverage. The photocurrent generation in TMDC heterostructure generally suffers from two main limitations from an applied point of view, which are their slow response time35 and their strong dependence of the photoresponse with the light intensity61,62. In the following we investigate these two properties. We measured the light intensity dependence of the response and found an almost linear dependence of the current with the photon flux (Φ) see Fig. 6(c). More precisely the power dependence of the current (I) can be fitted using a power low44, IαΦ0.8. Such dependence is weaker than for large gain system like graphene–PbS quantum dot hybrid system63, which allows using MoS2 decorated graphene system over a larger range of light flux. While modulating the incident laser intensity thanks to a signal generator we can extract the frequency dependence of the graphene-MoS2 system64. The 3dB cut off frequency is measured to be 2 kHz, see Fig. 6(d).
In summary, we have studied the electronic properties of the wet transfer of large area MoS2 on epitaxial graphene layer. From the PL, ARPES and micro-Raman data presented in Figs 1, 2, 3, it is clear that the MoS2/graphene heterostructure presents a good long-range order at large scale. Our ARPES measurements on the heterostructure showed that graphene and MoS2 largely retained their original electronic structure indicating weak van der Waals interactions between the two crystals. The PL quenching in the MoS2-graphene heterostructure and the upshift of the A1g Raman mode of MoS2 and the downshift of the 2D Raman mode of graphene confirm the charge transfer between the MoS2 and graphene layer. Our work suggests that the optical properties of MoS2 are strongly affected by the underlayer graphene. The fact that an interaction is visible between MoS2 and graphene is a clear signature of the quality of the wet transfer process and of the absence of interfacial contaminations. Moreover, this charge transfer can be influenced by the underlayer graphene doping, varying the band alignment in the heterostructure, which should be considered in device design and fabrication. Furthermore, efficient photoresponse was observed on large 2D MoS2/epitaxial graphene devices, opening a new way in graphene optoelectronics.
Methods
Growth of graphene/SiC(0001)
Monolayer graphene studied in this paper is produced via a two-step process beginning with a starting substrate of 4H-SiC(0001)65. Prior to graphitization, the substrate is hydrogen etched (100% H2) at 1550 °C to produce well-ordered atomic terraces of SiC. Subsequently, the SiC sample is heated to 1000 °C at a pressure of about 10−5 mbar and then further heated to 1550 °C in an Ar atmosphere. This graphitization process results in the growth of an electrically active graphene layer on top of the buffer layer, covalently bound to the substrate66. The sample was cooled down to room temperature and transferred ex-situ to perform different measurements.
Growth of MoS2/SiO2/Si(001)
MoS2/SiO2 samples were grown via CVD in a 1″ quartz tube furnace. Microliter droplets of saturated ammonia heptamolybdate solution were dried onto the corners of a Si/SiO2 growth substrate that had previously been coated with a layer of sodium cholate (1% solution spin coated 4000 rpm for 60 sec). Sodium cholate is a known growth promoter, acting to increase diffusion of the molybdenum source by increasing the surface adhesive energy relative to the adatom cohesive energy22. The growth substrate was placed in the center of the furnace and heated to 800 °C. A 25 mg sulfur pellet was placed on a piece of silicon and positioned upstream in the furnace such that its temperature was approximately 150 °C. Carrier gas (500 sccm N2) was used to bring sulfur vapor into the furnace for a 30 min growth period. The sample was then rapidly cooled by cracking open the furnace and sliding it downstream with respect to the quartz tube.
Characterization of MoS2/graphene heterostructure
The PL measurements were carried out using a confocal commercial Renishaw micro-Raman microscope with a 100× objective and a Si detector (detection range up to ~2.2 eV). The Raman spectra measurements were performed on the same microscope using a 532 nm laser in an ambient environment at room temperature. To ensure the reproducibility of the data, we followed a careful alignment and optimization protocol. In addition, the excitation laser was focused onto the samples with spot diameter of ~1 μm and incident power of ~5 mW. The integration time was optimized to obtain a satisfactory signal-to-noise ratio. We obtained Raman spatial maps by raster scanning with 0.3 μm step size using a precision 2D mapping stage.
The ARPES measurements were conducted at the CASSIOPEE beamline of Synchrotron SOLEIL (Saint-Aubin, France). We used linearly polarized photons of 50 eV and 90 eV and a hemispherical electron analyzer with vertical slits to allow band mapping. The total angle and energy resolutions were 0.25° and 16 meV. The mean diameter of the incident photon beam was smaller than 50 μm. All ARPES experiments were done at room temperature.
For electrical measurements the samples are electrically characterized in air at room temperature. Temporal and power dependence of the current are obtained while biasing the sample and measuring the current with a Keithley 2634B as sourcemeter. Illumination is ensured by a 405 nm blue laser diode with tunable light intensity. The frequency dependence of the photocurrent is measured while the sample is biased using a Keithley 2634B. The output signal is amplified in a Keithley 427 current amplifier and the signal acquired on HP oscilloscope.
DFT calculations
First-principles calculations have been performed using a very efficient DFT localized orbital molecular dynamic technique (FIREBALL)67,68,69,70. Basis sets of sp3d5 for S and Mo were used with cutoff radii (in atomic units) s = 4.3, p = 4.7, d = 5.5 (S) and s = 5.0, p = 5.6, d = 4.8 (Mo). In this study we have considered standard unit cells of 3, 6 and 9 atoms to describe respectively a mono-bi- and trilayer of MoS2. Each configuration has been relaxed using a sample of 32 k-points in the Brillouin zone. In case of multilayer MoS2, we have considered the most stable AB stacking, and the equilibrium distance has been determined using the LCAO-S2 + vdW formalism71,72. Finally, a set of 300 special k points along the K′–Γ–K path has been used for the band structure calculations. The corresponding band structures as well as extended atomic representations of the multilayers MoS2 are provided in Fig. S3(a–f) in the Supplementary Informations.
Additional Information
How to cite this article: Pierucci, D. et al. Large area molybdenum disulphide - epitaxial graphene vertical Van der Waals heterostructures. Sci. Rep. 6, 26656; doi: 10.1038/srep26656 (2016).
Supplementary Material
Acknowledgments
This work was supported by the ANR H2DH grants. This work is supported by a public grant overseen by the French National Research Agency (ANR) as part of the “Investissements d’Avenir” program (reference: ANR-10-LABX-0035, Labex NanoSaclay). C.H.N. and A.T.C.J. acknowledge support from the National Science Foundation EFRI-2DARE program, grant number ENG- 1542879.
Footnotes
The authors declare no competing financial interests.
Author Contributions D.P., H.H. and H.S. grows the graphene sample and carried the Raman spectroscopy, C.H.N., A.B. and A.T.C.J. grow the MoS2 on SiO2. J.R., F.B., P.F. and A.O. conducted the measurements ARPES. E.L. and H.H. conducted the Phototransport measurement. Y.J.D. carried the DFT calculations. All the authors participated in analyzed the data and writing the paper.
References
- Geim A. K. Graphene: status and prospects. Science 324, 1530–4 (2009). [DOI] [PubMed] [Google Scholar]
- Geim A. K. & Novoselov K. S. The rise of graphene. Nat. Mater. 6, 183–91 (2007). [DOI] [PubMed] [Google Scholar]
- O’Hare A., Kusmartsev F. V & Kugel K. I. A Stable “Flat” Form of Two-Dimensional Crystals: Could Graphene, Silicene, Germanene Be Minigap Semiconductors? Nano Lett. 12, 1045–1052 (2012). [DOI] [PubMed] [Google Scholar]
- Geim A. K. & Grigorieva I. V. Van der Waals heterostructures. Nature 499, 419–25 (2013). [DOI] [PubMed] [Google Scholar]
- Butler S. Z. et al. Progress, Challenges, and Opportunities in Two-Dimensional Materials Beyond Graphene. ACS Nano 7, 2898–2926 (2013). [DOI] [PubMed] [Google Scholar]
- Novoselov K. S. Nobel Lecture: Graphene: Materials in the Flatland. Rev. Mod. Phys. 83, 837–849 (2011). [DOI] [PubMed] [Google Scholar]
- Novoselov K. S. et al. A roadmap for graphene. Nature 490, 192–200 (2012). [DOI] [PubMed] [Google Scholar]
- Novoselov K. S. & Castro Neto A. H. Two-dimensional crystals-based heterostructures: materials with tailored properties. Phys. Scr. T146, 014006 (2012). [Google Scholar]
- Wang Q. H., Kalantar-Zadeh K., Kis A., Coleman J. N. & Strano M. S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 7, 699–712 (2012). [DOI] [PubMed] [Google Scholar]
- Britnell L. et al. Strong Light-Matter Interactions in Heterostructures of Atomically Thin Films. Science 340, 1311–1314 (2013). [DOI] [PubMed] [Google Scholar]
- Roy K. et al. Graphene–MoS2 hybrid structures for multifunctional photoresponsive memory devices. Nat. Nanotechnol. 8, 826–830 (2013). [DOI] [PubMed] [Google Scholar]
- Zhang W. et al. Ultrahigh-Gain Photodetectors Based on Atomically Thin Graphene-MoS2 Heterostructures. Sci. Rep. 4, 1–8 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pallecchi E. et al. High Electron Mobility in Epitaxial Graphene on 4H-SiC(0001) via post-growth annealing under hydrogen. Sci. Rep. 4, 4558 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pierucci D. et al. Self-organized metal-semiconductor epitaxial graphene layer on off-axis 4H-SiC(0001). Nano Res. 8, 1026–1037 (2015). [Google Scholar]
- Lin Y.-C. et al. Direct Synthesis of van der Waals Solids. ACS Nano 8, 3715–3723 (2014). [DOI] [PubMed] [Google Scholar]
- Lin Y. et al. Atomically Thin Heterostructures Based on Single-Layer Tungsten Diselenide and Graphene. 1–6 (2014). [DOI] [PubMed]
- Eichfeld S. M. et al. Highly Scalable, Atomically Thin WSe2 Grown via Metal-Organic Chemical Vapor Deposition. ACS Nano 9, 2080–2087 (2015). [DOI] [PubMed] [Google Scholar]
- Miwa J. A. et al. Van der Waals Epitaxy of Two-Dimensional MoS2-Graphene Heterostructures in Ultrahigh Vacuum. ACS Nano 9, 6502–6510 (2015). [DOI] [PubMed] [Google Scholar]
- Liu X. et al. Rotationally Commensurate Growth of MoS2 on Epitaxial Graphene. ACS Nano, 10, 1, 1067–1075, (2016). [DOI] [PubMed] [Google Scholar]
- Shi Y. et al. Van der Waals Epitaxy of MoS2 Layers Using Graphene as Growth Templates. Nano Lett. 12, 2784–2791 (2012). [DOI] [PubMed] [Google Scholar]
- Ago H. et al. Controlled van der Waals Epitaxy of Monolayer MoS2 Triangular Domains on Graphene. ACS Appl. Mater. Interfaces 7, 5265–5273 (2015). [DOI] [PubMed] [Google Scholar]
- Han G. H. et al. Seeded growth of highly crystalline molybdenum disulphide monolayers at controlled locations. Nat. Commun. 6, 6128 (2015). [DOI] [PubMed] [Google Scholar]
- Jin W. et al. Direct Measurement of the Thickness-Dependent Electronic Band Structure of MoS2 Using Angle-Resolved Photoemission Spectroscopy. Phys. Rev. Lett. 111, 106801 (2013). [DOI] [PubMed] [Google Scholar]
- Brumme T., Calandra M. & Mauri F. First-principles theory of field-effect doping in transition-metal dichalcogenides: Structural properties, electronic structure, Hall coefficient, and electrical conductivity. Phys. Rev. B 91, 155436 (2015). [Google Scholar]
- Diaz H. C. et al. Direct observation of interlayer hybridization and Dirac relativistic carriers in graphene/MoS2 van der Waals heterostructures. Nano Lett. 15, 1135–40 (2015). [DOI] [PubMed] [Google Scholar]
- Kuc A., Zibouche N. & Heine T. Influence of quantum confinement on the electronic structure of the transition metal sulfide TS2. Phys. Rev. B 83, 245213 (2011). [Google Scholar]
- Splendiani A. et al. Emerging photoluminescence in monolayer MoS2. Nano Lett. 10, 1271–5 (2010). [DOI] [PubMed] [Google Scholar]
- Eda G. et al. Photoluminescence from Chemically Exfoliated MoS2. Nano Lett. 11, 5111–5116 (2011). [DOI] [PubMed] [Google Scholar]
- Mak K. F., Lee C., Hone J., Shan J. & Heinz T. F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 105, 136805 (2010). [DOI] [PubMed] [Google Scholar]
- Fang H. et al. Strong interlayer coupling in van der Waals heterostructures built from single-layer chalcogenides. Proc. Natl. Acad. Sci. USA 111, 6198–202 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Zhang X. et al. Vertical heterostructures of layered metal chalcogenides by van der Waals epitaxy. Nano Lett. 14, 3047–54 (2014). [DOI] [PubMed] [Google Scholar]
- Bhanu U., Islam M. R., Tetard L. & Khondaker S. I. Photoluminescence quenching in gold - MoS2 hybrid nanoflakes. Sci. Rep. 4, 5575 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lagarde D. et al. Carrier and Polarization Dynamics in Monolayer MoS2. Phys. Rev. Lett. 112, 047401 (2014). [DOI] [PubMed] [Google Scholar]
- Li H. et al. From Bulk to Monolayer MoS2: Evolution of Raman Scattering. Adv. Funct. Mater. 22, 1385–1390 (2012). [Google Scholar]
- Yin Z. et al. Single-Layer MoS2 Phototransistors. ACS Nano 6, 74–80 (2012). [DOI] [PubMed] [Google Scholar]
- Deng Y. et al. Black Phosphorus -Monolayer MoS2 van der Waals Heterojunction p-n Diode. ACS Nano 8, 8292–8299 (2014). [DOI] [PubMed] [Google Scholar]
- Buscema M., Steele G. A., van der Zant H. S. J. & Castellanos-Gomez A. The effect of the substrate on the Raman and photoluminescence emission of single-layer MoS2. Nano Res. 7, 561–571 (2015). [Google Scholar]
- Wang S., Wang X., Warner J. H., All Chemical Vapor Deposition Growth of MoS2:h-BN Vertical van der Waals Heterostructures. ACS Nano 9, 5246 (2015). [DOI] [PubMed] [Google Scholar]
- Liu K. et al. Elastic Properties of Chemical-Vapor-Deposited Monolayer MoS2, WS2, and Their Bilayer Heterostructures. Nano Lett. 14, 5097–5103 (2014). [DOI] [PubMed] [Google Scholar]
- Conley H. J. et al. Bandgap Engineering of Strained Monolayer and Bilayer MoS2. Nano Lett. 13, 3626–3630 (2013). [DOI] [PubMed] [Google Scholar]
- Ko P. J. et al. Laser Power Dependent Optical Properties of Mono- and Few-Layer MoS2. J. Nanosci. Nanotechnol. 15, 6843–6846 (2015). [DOI] [PubMed] [Google Scholar]
- Korn T., Heydrich S., Hirmer M., Schmutzler J. & Schüller C. Low-temperature photocarrier dynamics in monolayer MoS2. Appl. Phys. Lett. 99, 102109 (2011). [Google Scholar]
- Mose L. S. Large-Area Single-Layer MoSe2 and Its van der Waals Heterostructures. ACS Nano 8, 6655–6662 (2014). [DOI] [PubMed] [Google Scholar]
- Chen Z., Biscaras J. & Shukla A. A high performance graphene/few-layer InSe photo-detector. Nanoscale 7, 5981–6 (2015). [DOI] [PubMed] [Google Scholar]
- Trabelsi A. B. G. et al. Charged nano-domes and bubbles in epitaxial graphene. Nanotechnology 25, 165704 (2014). [DOI] [PubMed] [Google Scholar]
- Ni Z. et al. Raman spectroscopy of epitaxial graphene on a SiC substrate. Phys. Rev. B 77, 115416 (2008). [Google Scholar]
- Lee C. et al. Anomalous Lattice Vibrations of Single-and Few-Layer MoS2. ACS Nano 4, 2695–2700 (2010). [DOI] [PubMed] [Google Scholar]
- Zhou K. et al. Raman Modes of MoS2 Used as Fingerprint of van der Waals Interactions. ACS Nano 9914–9924 (2014). [DOI] [PubMed] [Google Scholar]
- Chakraborty B. et al. Symmetry-dependent phonon renormalization in monolayer MoS2 transistor. Phys. Rev. B 85, 161403 (2012). [Google Scholar]
- Li L. et al. Raman shift and electrical properties of MoS2 bilayer on boron nitride substrate. Nanotechnology 26, 295702 (2015). [DOI] [PubMed] [Google Scholar]
- Bkakri R. et al. Effects of the graphene content on the conversion efficiency of P3HT:Graphene based organic solar cells. J. Phys. Chem. Solids 85, 206–211 (2015). [Google Scholar]
- Bkakri R., Kusmartseva O. E., Kusmartsev F. V., Song M. & Bouazizi A. Degree of phase separation effects on the charge transfer properties of P3HT:Graphene nanocomposites. J. Lumin. 161, 264–270 (2015). [Google Scholar]
- Das A. et al. Monitoring dopants by Raman scattering in an electrochemically top-gated graphene transistor. Nat. Nanotechnol. 3, 210–5 (2008). [DOI] [PubMed] [Google Scholar]
- Ferrari A. C. & Basko D. M. Raman spectroscopy as a versatile tool for studying the properties of graphene. Nat. Nanotechnol. 8, 235–46 (2013). [DOI] [PubMed] [Google Scholar]
- Federspiel F. et al. Distance dependence of the energy transfer rate from a single semiconductor nanostructure to graphene. Nano Lett. 15, 1252–8 (2015). [DOI] [PubMed] [Google Scholar]
- Froehlicher G. & Berciaud S. Raman spectroscopy of electrochemically gated graphene transistors: Geometrical capacitance, electron-phonon, electron-electron, and electron-defect scattering. Phys. Rev. B 91, 205413 (2015). [Google Scholar]
- Yan J., Zhang Y., Kim P. & Pinczuk A. Electric Field Effect Tuning of Electron-Phonon Coupling in Graphene. Phys. Rev. Lett. 98, 166802 (2007). [DOI] [PubMed] [Google Scholar]
- Chen C.-F. et al. Controlling inelastic light scattering quantum pathways in graphene. Nature 471, 617–20 (2011). [DOI] [PubMed] [Google Scholar]
- Jnawali G. et al. Observation of Ground- and Excited-State Charge Transfer at the C 60/Graphene Interface. ACS Nano 9, 7175–7185 (2015). [DOI] [PubMed] [Google Scholar]
- Buscema M. et al. Photocurrent generation with two-dimensional van der Waals semiconductors. Chem. Soc. Rev. 44, 3691–718 (2015). [DOI] [PubMed] [Google Scholar]
- Lopez-Sanchez O., Lembke D., Kayci M., Radenovic A. & Kis A. Ultrasensitive photodetectors based on monolayer MoS2. Nat. Nanotechnol. 8, 497–501 (2013). [DOI] [PubMed] [Google Scholar]
- Boscher N. D., Carmalt C. J., Palgrave R. G., Gil-Tomas J. J. & Parkin I. P. Atmospheric Pressure CVD of Molybdenum Diselenide Films on Glass. Chem. Vap. Depos. 12, 692–698 (2006). [Google Scholar]
- Sun Z. et al. Infrared photodetectors based on CVD-grown graphene and PbS quantum dots with ultrahigh responsivity. Adv. Mater. 24, 5878–83 (2012). [DOI] [PubMed] [Google Scholar]
- Yung K. C., Wu W. M., Pierpoint M. P. & Kusmartsev F. V. Introduction to graphene electronics – a new era of digital transistors and devices. Contemp. Phys. 54, 233–251 (2013). [Google Scholar]
- Pallecchi E. et al. Observation of the quantum Hall effect in epitaxial graphene on SiC(0001) with oxygen adsorption. Appl. Phys. Lett. 100, 253109 (2012). [Google Scholar]
- Lalmi B. et al. Flower-shaped domains and wrinkles in trilayer epitaxial graphene on silicon carbide. Sci. Rep. 4, 4066 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lewis J. et al. Further developments in the local-orbital density-functional-theory tight-binding method. Phys. Rev. B 64, 195103 (2001). [Google Scholar]
- Lewis J. P. et al. Advances and applications in the FIREBALLab initio tight-binding molecular-dynamics formalism. Phys. Status Solidi 248, 9, 1989–2007, (2011). [Google Scholar]
- Jelínek P., Wang H., Lewis J., Sankey O. & Ortega J. Multicenter approach to the exchange-correlation interactions in ab initio tight-binding methods. Phys. Rev. B 71, 235101 (2005). [Google Scholar]
- Sankey O. F. & Niklewski D. J. Ab initio multicenter tight-binding model for molecular-dynamics simulations and other applications in covalent systems. Phys. Rev. B 40, 3979–3995 (1989). [DOI] [PubMed] [Google Scholar]
- Dappe Y., Ortega J. & Flores F. Intermolecular interaction in density functional theory: Application to carbon nanotubes and fullerenes. Phys. Rev. B 79, 165409 (2009). [Google Scholar]
- Švec M. et al. van der Waals interactions mediating the cohesion of fullerenes on graphene. Phys. Rev. B 86, 121407 (2012). [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.