The Pale Orange Dot: The Spectrum and Habitability of Hazy Archean Earth

Abstract

Recognizing whether a planet can support life is a primary goal of future exoplanet spectral characterization missions, but past research on habitability assessment has largely ignored the vastly different conditions that have existed in our planet's long habitable history. This study presents simulations of a habitable yet dramatically different phase of Earth's history, when the atmosphere contained a Titan-like, organic-rich haze. Prior work has claimed a haze-rich Archean Earth (3.8–2.5 billion years ago) would be frozen due to the haze's cooling effects. However, no previous studies have self-consistently taken into account climate, photochemistry, and fractal hazes. Here, we demonstrate using coupled climate-photochemical-microphysical simulations that hazes can cool the planet's surface by about 20 K, but habitable conditions with liquid surface water could be maintained with a relatively thick haze layer (τ ∼ 5 at 200 nm) even with the fainter young Sun. We find that optically thicker hazes are self-limiting due to their self-shielding properties, preventing catastrophic cooling of the planet. Hazes may even enhance planetary habitability through UV shielding, reducing surface UV flux by about 97% compared to a haze-free planet and potentially allowing survival of land-based organisms 2.7–2.6 billion years ago. The broad UV absorption signature produced by this haze may be visible across interstellar distances, allowing characterization of similar hazy exoplanets. The haze in Archean Earth's atmosphere was strongly dependent on biologically produced methane, and we propose that hydrocarbon haze may be a novel type of spectral biosignature on planets with substantial levels of CO₂. Hazy Archean Earth is the most alien world for which we have geochemical constraints on environmental conditions, providing a useful analogue for similar habitable, anoxic exoplanets. Key Words: Haze—Archean Earth—Exoplanets—Spectra—Biosignatures—Planetary habitability. Astrobiology 16, 873–899.

1. Introduction

Early in Earth's history, an anoxic atmosphere could have supported the formation of an organic haze (Pavlov et al., 2001a; Trainer et al., 2004, 2006; DeWitt et al., 2009; Hasenkopf et al., 2010; Zerkle et al., 2012; Kurzweil et al., 2013; Claire et al., 2014; Izon et al., 2015) that strongly interacted with visible and UV radiation, cooling the planet's climate (Pavlov et al., 2001b; Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008; Wolf and Toon, 2010; Hasenkopf et al., 2011). This hydrocarbon haze, generated by methane (CH₄) photolysis, would have formed when the ratio of CH₄ to carbon dioxide (CO₂) in the atmosphere exceeded about 0.1 (Trainer et al., 2006).

Unlike the hazes that may exist around exoplanets with thick hydrogen-dominated atmospheres (Sing et al., 2011; Knutson et al., 2014; Kreidberg et al., 2014), the Archean (3.8–2.5 billion years ago) haze was likely biologically mediated via CH₄ produced from methanogenesis, one of the earliest metabolisms (Woese and Fox, 1977; Ueno et al., 2006). In addition, several abiotic processes including serpentinization (the hydration of ultramafic rocks, mainly olivine and pyroxenes) can form methane (Kelley et al., 2005; Etiope and Sherwood Lollar, 2013; Guzmán-Marmolejo et al., 2013), but the biotic flux of methane to the Archean atmosphere was likely much higher than the abiotic flux (Kharecha et al., 2005), as it is on Earth today. While the climatic effects of this haze have been studied (e.g., Pavlov et al., 2001b), impacts of haze on Archean Earth's habitability have not been previously investigated using tightly coupled climate-photochemical models. This coupling is critical to consider because of potential feedbacks between the impact of temperature on haze formation and the effects of haze on the atmosphere's temperature structure. Additionally, although we anticipate planetary diversity in the exoplanet population, existing spectral studies are largely focused on the observables of modern-day Earth (e.g., Sagan et al., 1993; Woolf et al., 2002; Robinson et al., 2011, 2014a). Those spectral studies that consider Archean Earth and anoxic planets have not examined hazes (Meadows, 2006; Kaltenegger et al., 2007; Domagal-Goldman et al., 2011). As we will show, hydrocarbon haze has profound spectral impacts for both reflected light and transit transmission spectra.

1.1. Evidence for an Archean haze

Geochemical data suggest 3–5 distinct intervals of organic haze during the later Archean (Zerkle et al., 2012; Izon et al., 2015), supporting theoretical studies on the causes and consequences of photochemical haze formation in the atmosphere (Pavlov et al., 2001a, 2001b; Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008; Kurzweil et al., 2013; Claire et al., 2014) as well as experimental data (Trainer et al., 2004, 2006; DeWitt et al., 2009; Hasenkopf et al., 2010, 2011) and theory on their potential radiative effects (Wolf and Toon, 2010). The geochemical evidence, described below, implies Neoarchean hazy intervals (Zerkle et al., 2012; Izon et al., 2015) lasting for less than 1 million years. The constraint on the duration of these intervals is based on the lower limit of shale sedimentation rates. In addition, the modeling work of Domagal-Goldman et al. (2008) suggests a longer Mesoarchean to Neoarchean hazy period between 3.2 and 2.7 Ga.

Here, we present an overview of the evidence for the Archean haze. The line of evidence most often invoked comes from analyses and modeling of sulfur isotope fractionation data from Earth's rock record. Several studies have proposed links between haze and the mass-independent sulfur isotope fractionation signal (S-MIF) (Farquhar et al., 2000) preserved in the geological record before the Great Oxygenation Event (GOE) at about 2.5 Ga (Domagal-Goldman et al., 2008; Zerkle et al., 2012; Kurzweil et al., 2013; Claire et al., 2014; Izon et al., 2015). We present a brief review of this evidence here, beginning with an overview of sulfur mass-independent fractionation, on which much of the evidence for an Archean haze is based.

Sulfur has four stable isotopes: ³²S, ³³S, ³⁴S, and ³⁶S. Isotope fractionations are reported in parts per thousand (‰) using delta notation (δ) such that

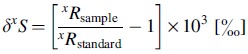Here, ^xR_sample represents isotope ratios of the given minor to major isotope (for sulfur, ^xR means ^xS/³²S with x = 33, 34, 36) of sampled material. ^xR_standard represents isotope ratios of a standard reference material.

Reactions following classical equilibrium or kinetic behaviors produce isotope fractionation that depend only on the mass differences of the isotopes such that the δ³³S composition of a material is approximately half the δ³⁴S amount, and the δ³⁶S composition is roughly twice the δ³⁴S amount. For elements with more than two stable isotopes, mass-dependent fractionation (MDF) quantifies this expected three-isotope relationship, and samples following MDF will have δ³³S ∼ 0.515 × δ³⁴S and δ³⁶S ∼ 1.89 × δ³⁶S.

Mass-independent fractionation (MIF) occurs when samples deviate from this expected three-isotope behavior and is quantified with “capital delta” notation where Δ³³S = δ³³S − 0.515 × δ³⁴S and Δ³⁶S = δ³⁶S − 1.89 × δ³⁶S. MIF in naturally occurring samples is very unusual and is generally diagnostic of quantum chemistry such as can occur in certain atmospheric reactions. While the precise mechanism (or mechanisms) that produces S-MIF is unknown, photolysis of sulfur gases in an anoxic atmosphere is the only known mechanism that produces large-magnitude Δ³³S and Δ³⁶S seen in the rock record (Farquhar et al., 2001, 2007).

The S-MIF signal is variable throughout the Archean, and it vanishes completely once O₂ builds up to non-negligible levels in the atmosphere after the GOE at 2.5 Ga. Its recurrence at both ends of the Archean eon implies that, within 0.8 billion years of Earth's formation, a common mechanism for S-MIF production was already established in the atmosphere (Thomassot et al., 2015). After the GOE, O₂ and the ozone (O₃) derived from O₂ photochemical reactions block the UV photons necessary to photolyze sulfur gases and produce S-MIF. Also, S₈ is the most important species to rain out S-MIF from the atmosphere; because a more reducing atmosphere enhances the ability of S₈ to polymerize, S-MIF is more easily preserved under reducing conditions (Zahnle et al., 2006). After the GOE, all the sulfur in the atmosphere would have been oxidized into a single exit channel, eliminating any fractionation created in the atmosphere (Pavlov and Kasting, 2002). Thus, S-MIF is generally regarded as robust evidence for an anoxic Archean atmosphere.

Δ³³S typically correlates with enrichments in δ³⁴S and with depletions in δ³⁶S, and variations in magnitude and sign of these isotopic signals in Earth's geological record hint that strong constraints on Archean atmospheric chemistry will be possible when the precise MIF formation mechanisms are identified (Claire et al., 2014). Δ³⁶S/Δ³³S in Archean sedimentary rocks is generally around −1, but stratigraphic variations in this slope have been observed in the geological record and interpreted as evidence of changes to the S-MIF production mechanism resulting from changes in atmospheric composition (Zerkle et al., 2012; Kurzweil et al., 2013; Izon et al., 2015), suggesting the influence of haze.

Domagal-Goldman et al. (2008) and Haqq-Misra et al. (2008) studied potential links between S-MIF, hazes, and Archean glaciation. At ∼2.9 Ga, there is geological evidence suggesting a glaciation event (Young et al., 1998) may have occurred during the same period when the S-MIF Δ³³S signal dips to lower values. An upper atmosphere haze that decreased tropospheric SO₂ photolysis by blocking UV photons and cooled the planet could explain both the glaciation and the decrease in S-MIF. In this conceptual model, the end of the cold period typified by low Δ³³S may be due to a decrease in the atmospheric CH₄/CO₂ ratio, which would have cleared any haze present in the atmosphere. If true, this change in atmospheric composition and radiative scattering would have enabled UV photons to penetrate deeper into the atmosphere, interacting with sulfurous gases and affecting their isotopic signatures (Claire et al., 2014). Earth's record of sedimentary sulfates does show a significant change in their minor sulfate isotope behavior between 2.73 and 2.71 Ga (Kurzweil et al., 2013; Izon et al., 2015) that may in fact reflect this change, although predictive models of sulfur isotope fractionation are not yet able to reproduce these trends seen in the rock record (Claire et al., 2014).

Zerkle et al. (2012) discussed the discovery of geochemical evidence consistent with the Archean haze hypothesis. The authors analyzed sediments aged 2.65–2.5 Ga collected from the Ghaap Group in South Africa and showed that variations of Δ³⁶S/Δ³³S associated with changes in atmospheric chemistry were contemporaneous with highly negative excursions of δ¹³C_org values. Negative values of δ¹³C_org below −37‰ are typically interpreted as evidence for methanogenesis (biological methane production) followed by subsequent incorporation into sediments by methanotrophy (methane consumption), which imparts a strongly negative δ¹³C_org because organisms preferentially uptake the ¹²C (Urey and Greiff, 1935; Schopf, 1983; Schidlowski, 2001; Eigenbrode and Freeman, 2006). The contemporaneous excursions of the sulfur and C_org isotopes suggest a close linkage between S-MIF signals and biogenic methane. The links between S-MIF signals and biogenic methane production have been recently expanded over multiple cores and locations, suggesting multiple changes in atmospheric chemistry during the Neoarchean (Izon et al., 2015). Changes observed in the slope of Δ³⁶S/Δ³³S vary between −1.5 and −0.9 and are interpreted to reflect changes in the S-MIF source reactions driven by varying atmospheric haze thicknesses.

Kurzweil et al. (2013) noted that an increase in magnitude of S-MIF signals after 2.73 Ga (Thomazo et al., 2009) occurred during a prolonged negative shift in δ¹³C_org, suggesting enhanced biological methane activity at this time. Similar to Zerkle et al. (2012), they discuss a change in the slope of Δ³⁶S/Δ³³S from −1.5 to −0.9 at 2.71 Ga and interpret this to be caused by a decrease in the CH₄/CO₂ ratio at 2.71 Ga, possibly indicating an organic haze was present for some period of time prior to 2.71 Ga and cleared afterward. In this interpretation, haze-free and reducing atmospheric conditions dominated after 2.71 Ga, with haze reappearing in brief intervals of time as suggested by the Zerkle et al. (2012) and Izon et al. (2015) measurements.

Given the apparent occurrence of haze in the Archean, we investigated the impact of this haze on the climate, spectral appearance, and surface UV flux by simulating the hazy Archean environment with boundary conditions consistent with recent geochemical constraints. Unlike previous studies of the Archean climate under a haze, we use realistic fractal (rather than spherical) particles, which have different spectral properties and climatic effects. Our study also represents the first time temperature feedbacks have been investigated in relation to haze production in Archean Earth's atmosphere. Previous studies (Pavlov et al., 2001b; Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008) involving climate modeling have included the haze's impact on temperature but not corresponding temperature feedbacks on haze formation. Temperature feedbacks have significant impacts on the resultant hazes: as we discuss below, hazes produce stratospheric temperature inversions, and warmer atmospheres produce larger haze particles, so hazes generated by chemistry models without temperature feedbacks may not produce realistic results.

2. Models and Methods

To simulate the hazy Archean environment with boundary conditions consistent with recent geochemical constraints, we used a coupled 1-D photochemical-climate model we call Atmos and a 1-D radiative transfer model, SMART (Spectral Mapping Atmospheric Radiative Transfer).

2.1. Coupled photochemical-climate model

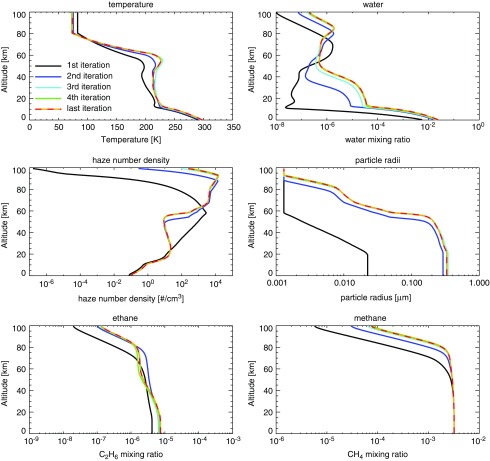
Our coupled photochemical-climate model, Atmos, is used to simulate Archean Earth's photochemistry and climate. To use Atmos, the photochemical model (which includes particle microphysics) is run first to generate an initial atmospheric state based on user-specified boundary conditions [gas mixing ratios or fluxes, the solar constant at 2.7 Ga (Claire et al., 2012), the stellar spectral type, total atmospheric pressure, the initial temperature-pressure profile]. Then, the output files from the photochemical model for altitude, pressure, gas mixing ratios, haze particle sizes, and haze number densities are passed into the climate model. The climate model uses the photochemical model's solution as its initial conditions and runs until it reaches a converged state. It then feeds updated temperature and water vapor profiles back into the photochemical model. The models iterate back and forth in this manner until convergence is reached. An example of Atmos finding convergence can be seen in Fig. 1.

FIG. 1.

Shown is an example of the Atmos model convergence process. This atmosphere, which has CH₄/CO₂ = 0.17 and pCO₂ = 0.02 (total pressure 1 bar) goes through five coupling iterations. The initial temperature profile it uses was stored from a previous similar atmosphere. Here we show the temperature, water, haze number density, haze particle radii, C₂H₆ profile, and CH₄ profile for each iteration of the coupled model.

2.1.1. Photochemical model

The photochemical portion of the code is based on the 1-D photochemical code developed originally by Kasting et al. (1979), but the version we use here was significantly modernized and updated by Zahnle et al. (2006) and uses the haze formation scheme described by Pavlov et al. (2001b). It was modified by E. Wolf to include fractal hydrocarbon hazes following the methods presented by Wolf and Toon (2010) and was first used to study fractal hazes on Archean Earth by Zerkle et al. (2012). Note that the version of the model used here can simulate atmospheres ranging from extremely anoxic (pO₂ = 10⁻¹⁴) to modern-day O₂ levels (Zahnle et al., 2006). Subsequent studies using this model or other versions of it to study fractal haze formation include those of Harman et al. (2013), Kurzweil et al. (2013), and Claire et al. (2014), with the latter two of these studies also derived from the same Zahnle et al. (2006) model branch used here. This model also has a long heritage of being used to study photochemistry in nonhazy atmospheres (e.g., Kasting and Donahue, 1980; Pavlov and Kasting, 2002; Ono et al., 2003; Segura et al., 2003, 2005, 2007, 2010; Zahnle et al., 2006; Grenfell et al., 2007; Catling et al., 2010; Domagal-Goldman et al., 2011, 2014; Rugheimer et al., 2013, 2015; Harman et al., 2015; Schwieterman et al., 2016).

The photochemical model parameters are as follows. Our model atmosphere is divided into 200 plane-parallel layers from the surface to 100 km, with a layer spacing of 0.5 km. We show a list of chemical reactions in our Supplementary Table S1 (Supplementary Data are available online at www.liebertonline.com/ast). Our Archean scheme includes 76 chemical species, 11 of which are short-lived (Supplementary Table S2). Short-lived species are considered in photochemical equilibrium (i.e., their atmospheric transport is neglected) and are not part of the Jacobian solved self-consistently at each time step. The mixing ratio of each species is found by solving flux and mass continuity equations in each layer simultaneously using a reverse-Euler method, providing exact solutions at steady state. Vertical transport by molecular and eddy diffusion is included, and boundary conditions that drive the model can be set for each species at the surface and the top of the atmosphere. A δ-2-stream method is used for radiative transfer (Toon et al., 1989). Fixed isoprofiles are assumed for CO₂ and N₂ in the atmospheres considered here.

Similarly to the work of Zerkle et al. (2012), we set a fixed mixing ratio of CH₄ at the surface; the model then calculates the surface flux necessary to maintain this mixing ratio. Since haze formation scales with the CH₄/CO₂ ratio, we find this is the most straightforward way to explore haze thicknesses in our atmospheres. Note that when we discuss CH₄/CO₂ values in this study, these refer to the ratio at the planetary surface because CH₄ does not follow an isoprofile.

Aerosol formation follows the method used in Kasting et al. (1989) and described and updated in Pavlov et al. (2001b). Immediate precursors to haze particles are formed through the reactions C₂H + C₂H₂ → C₄H₂ + H and C₂H + CH₂CCH₂ → C₅H₄ + H. Since the full chemical scheme that leads to aerosol formation is not well understood despite both laboratory and theoretical studies (e.g., Hallquist et al., 2009; Hicks et al., 2015), it is assumed that C₄H₂ and C₅H₄ condense directly to haze particles (called HCAER and HCAER2 in Supplementary Table S1). In a real atmosphere, the molecules would be larger before aerosols condense, and back-reactions should occur, so this model may overestimate the rate of aerosol formation. Pavlov et al. (2001b) suggested that if the real aerosol formation rate was slower, the atmosphere would compensate by increasing the CH₄/CO₂ ratio, which would increase the polymerization rate. Further discussion of haze formation pathways and caveats of the approach we use here can be found in Section 4.4. The model's particles form initially with a radius of 0.001 μm. Each layer of the atmosphere has a monomodal size distribution calculated by comparing the coagulation lifetime to the particle removal lifetime via diffusion into another layer or by sedimentation. The aerosols can grow when the coagulation lifetime is longer than the lifetime for removal in a layer.

The maximum radius of a spherical haze particle (i.e., a haze “monomer”) is set to 0.05 μm, the same nominal value used by Wolf and Toon (2010) and similar to the size of the monomers of Titan's fractal haze aggregates (Rannou et al., 1997; Tomasko et al., 2008). Particles larger than this size are treated as fractal agglomerates of n_mon spherical monomers of radius R_mon that clump into a larger aggregate with an effective geometric radius R_f given by the relation

Here, α represents a dimensionless constant of order unity, and D_f is the “fractal dimension,” which can take on values between 1 and 3. D_f = 3 represents a spherical (nonfractal or classical Mie) particle, while D_f = 1 represents a string of linearly chained monomers. Titan's fractal aggregates are thought to have a fractal dimension of about 2 on average for the aerosol population (Rannou et al., 1997; Larson et al., 2015). Note that the “effective geometric radius” we refer to above is used only to conceptualize the size of a fractal particle and does not indicate that we use Mie scattering for our fractal particles; with the exception of sub-monomer-sized particles (R < 0.05 μm) which remain spherical and thus Mie, we use the mean field approximation for fractal scattering physics for all particles (Botet et al., 1997). The model's fractal production methods are discussed by Zerkle et al. (2012) (including their supplementary online information), where they were first implemented. Additional information about fractal particles and their geometry can be found in the works of, for example, Köylü et al. (1995) and Brasil et al. (1999). The mean field approximation we use for fractal scattering has been validated against scattering by silica fractal aggregates (Botet et al., 1997) and Titan's hazes (Rannou et al., 1997; Larson et al., 2015).

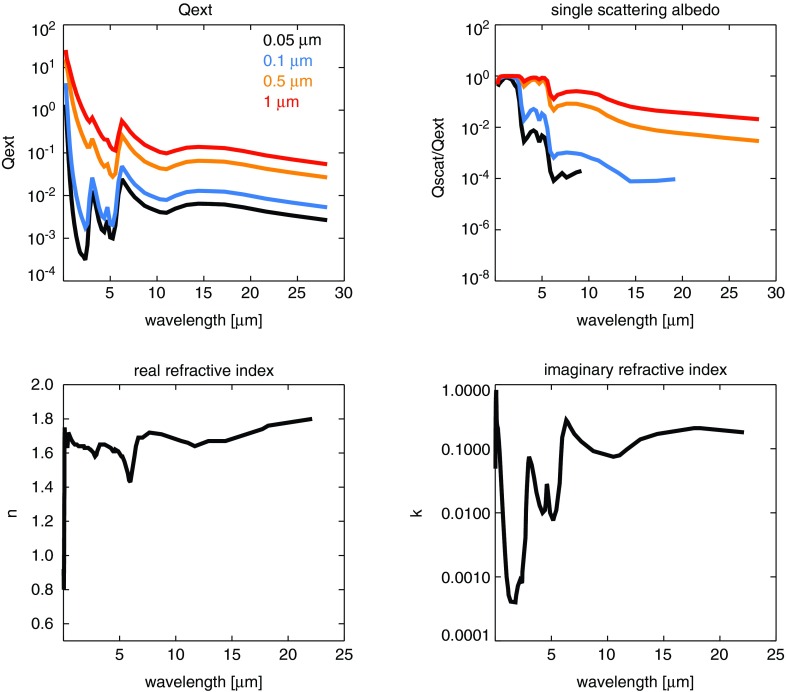
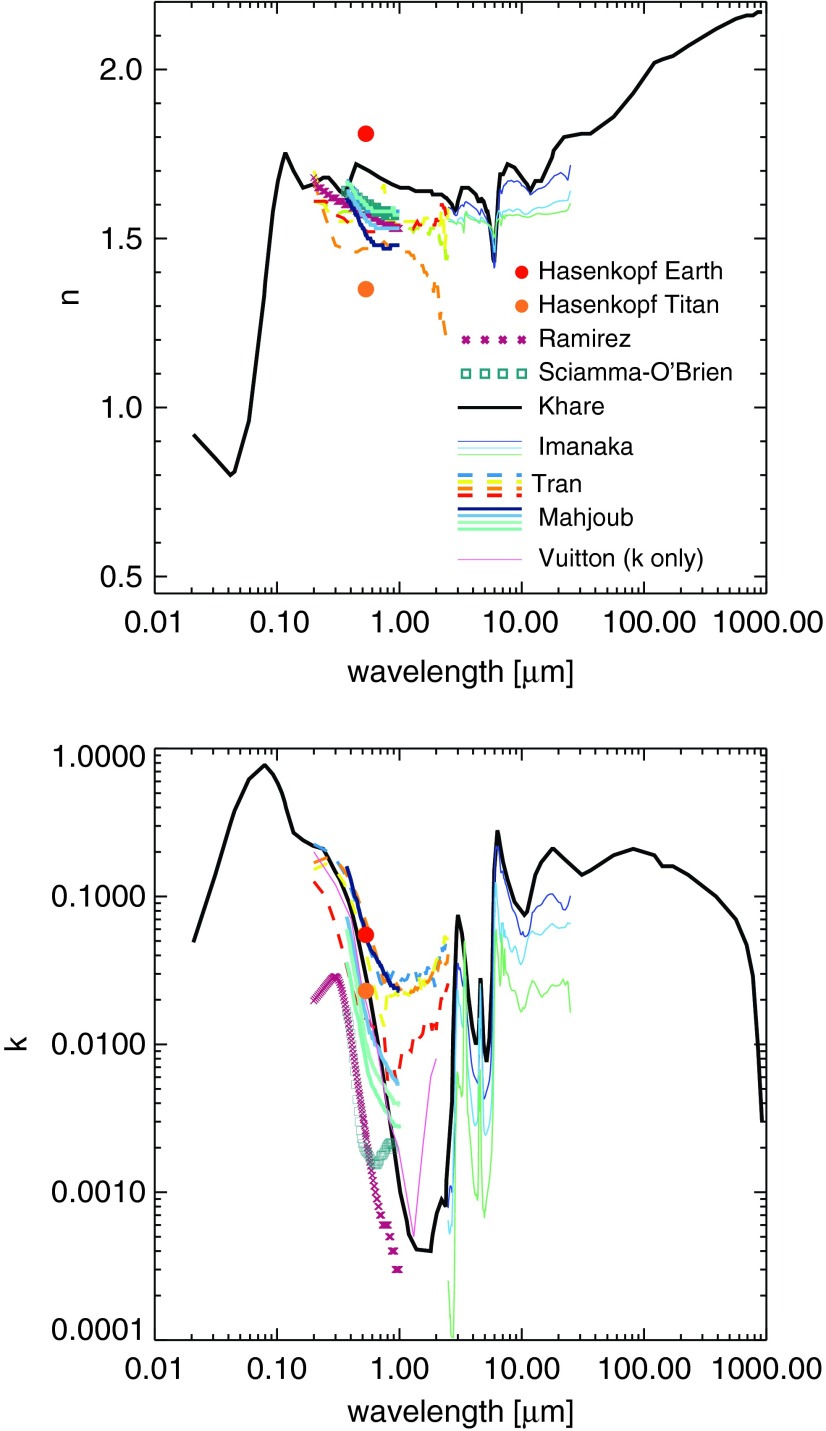
As in the work of Wolf and Toon (2010), the fractal dimension of our particles varies from 1.5 to 2.4 for aggregate particles, and larger aggregates have a larger fractal dimension to account for folding as the particles coagulate. In general, compared to spherical particles, fractal particles produce more extinction in the ultraviolet (UV) but less in the visible and near infrared (NIR). In addition, fractals tend to be more forward scattering in the visible and NIR and more isotropically scattering in the UV compared to equal-mass spherical particles. Their weakened visible extinction and enhanced forward scattering compared to spherical particles means they produce less cooling since they scatter less incident sunlight back to space (see Fig. 3 in Wolf and Toon, 2010). Figure 2 shows the extinction efficiency (Q_ext) and single-scattering albedo of different fractal particle sizes together with the haze optical constants we adopt in this study (Khare et al., 1984a). A discussion of our choice of optical constants and comparison to others in the literature can be found in Section 4.5.

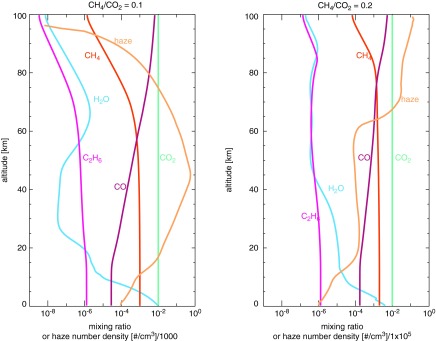
FIG. 3.

The gas profiles for H₂O, CH₄, CO, CO₂, and C₂H₆ for planets with pCO₂ = 0.01 bar for CH₄/CO₂ = 0.1 (on the left) and CH₄/CO₂ = 0.2 (on the right). Also shown are the profiles for the haze particle number density (in pale orange). The CH₄/CO₂ = 0.1 haze profile is divided by 1000, and the CH₄/CO₂ = 0.2 haze profile is divided by 1 × 10⁵ in order to plot it on the same axis as the gases. The profiles in the right panel show larger amounts of CH₄, H₂O, and C₂H₆ above 60 km in altitude and illustrate how haze-induced shielding can prevent photolysis of these gases. The sharp decrease in haze particle number density between 60 and 70 km in the right panel shows where fractal coagulation occurs. The atmosphere above the fractal coagulation region is populated by spherical submonomer particles.

FIG. 2.

The top panels present the extinction efficiency (Qext) and single-scattering albedo ( = Qscat/Qext) of four sizes of fractal hydrocarbon particles used in this study and in Wolf and Toon (2010). The spherical monomers comprising these particles are 0.05 μm in radius. The radii on the plot correspond to the radii of equivalent-mass spherical particles, and the fractal dimensions of these particles, from smallest to largest, are 3 (spherical), 1.51, 2.28, and 2.40. The number of monomers in these particles are 1, 8, 1000, and 8000. These particles tend to scatter and absorb light more efficiently at shorter wavelengths, and larger particles have flatter wavelength dependence for the scattering efficiency. Refractive indices, shown in the bottom panels, are presented from information in Khare et al. (1984a).

In the version of the photochemical model used here, we corrected an error relating to the calculation of the number of C₅H₄ molecules composing HCAER2 haze particles. Previously, the model calculated the number of molecules per HCAER2 particle inappropriately using the mass of C₄H₂ instead of C₅H₄. In addition, we added more particle sizes to the model's scattering grid, increasing the number from 34 particle sizes to 51, and we added options to use different monomer sizes and optical constants than the ones used here for our nominal haze study; how variation of these parameters impacts haze formation is a subject of future work. Gas mixing ratios at the surface can be more finely tuned than in previous versions of the model from the addition of a significant figure to the species boundary conditions input file.

The photochemical model is considered converged when redox is conserved and a re-run of the model using last run's output as initial conditions occurs quickly (i.e., <50 time steps).

2.1.2. Climate model

Our climate model was originally developed by Kasting and Ackerman (1986). The model we use here has evolved considerably since its first incarnation, and versions of it have been applied in subsequent studies on varied topics such as the habitable zones for several stellar spectral types (Kopparapu et al., 2013), the climate of early Mars (Ramirez et al., 2013), the atmospheres of Earth-like planets around various stellar types (Segura et al., 2003, 2005, 2010; Rugheimer et al., 2013), clouds in exoplanet atmospheres (Kitzmann et al., 2010, 2011a), and the climate of early Earth (Haqq-Misra et al., 2008). The version we use here is based directly on that used by Kopparapu et al. (2013). It uses a correlated-k method to compute absorption by spectrally active gases (O₃, CO₂, H₂O, O₂, CH₄, and C₂H₆). This model has CO₂ and H₂O correlated-k coefficients updated as described by Kopparapu et al. (2013). Our older CH₄ coefficients may overestimate the surface temperature by ≲5 K at the CH₄ mixing ratios used here (Byrne and Goldblatt, 2015). However, as we discuss in Section 4.2, our model underpredicts the Archean temperature by about 2–5 K compared to 3-D climate models with more complete physics describing the planetary system, so these two effects may cancel each other out. The aforementioned gas profiles are passed to the climate model from the photochemical model when running in coupled mode. The net absorbed solar radiation in each layer of the atmosphere is computed using a δ-2-stream multiple scattering algorithm (Toon et al., 1989) spanning from λ = 0.2 to 4.5 μm in 38 spectral intervals. For net outgoing IR radiation, we use a separate set of correlated-k coefficients for each gas in 55 spectral intervals spanning wave numbers of 0–15,000 cm⁻¹.

We have made several modifications to the climate model used here. The model previously incorporated the spectral effects of spherical hydrocarbon particles, and it has been updated in our study to include fractal hydrocarbon scattering efficiencies using the mean field approximation of Botet et al. (1997) discussed previously. We have also updated the model so that haze profiles can be passed to it from an input file or by the photochemical code; in previous versions of the climate model, haze distributions were hard-coded and had to be edited manually. We corrected a discrepancy in the spacing between atmospheric layers in the routine that outputs coupling files for the photochemical model: our photochemical model layer spacing is 0.5 km, but a layer spacing of 1 km had been hard-coded. Coupling subroutines have been improved to be able to accept information about atmospheric pressure, stellar parameters, and haze parameters as input from the photochemical model. We also added options to turn ethane opacity and 1-D ice-albedo feedbacks (described in Section 4.1.1) on or off.

We have been unable to run the climate model to convergence using the same top-of-atmosphere pressure used for the photochemical model: the photochemical model extends to 100 km, but we have only been able to successfully run the climate model up to about 80 km for our 1 bar atmospheres. Thus, when temperature and water profiles are passed from the climate model to the photochemical model, they become isoprofiles above the top of the climate grid based on the highest-altitude temperature from the climate grid calculations. At these altitudes the atmosphere is thin, and the particles are very small; both of these effects lead to this portion of the atmosphere having little impact on radiative transfer and climate. We performed a sensitivity test of how the temperature at these altitudes affects the resultant haze distribution in the photochemical model, and the sizes of the largest haze particles produced by an atmosphere that becomes an 80 K isotherm above 80 km versus a 150 K isotherm differ by less than 5%. In the climate model, shifting the particles in Fig. 1 above 80 km down to lower altitudes alters the surface temperature by <0.5 K.

The climate model is considered converged when the change in temperature between time steps and change in flux out the top of the atmosphere are sufficiently small (typically on the order of 1 × 10⁻⁵).

2.2. The SMART model

To generate synthetic spectra for the atmospheres we produce with Atmos, we feed outputs from the Atmos model (the temperature-pressure profile, gas mixing ratio profiles, and the haze particle profile), into the SMART code, a 1-D line-by-line fully multiple scattering radiative transfer model (Meadows and Crisp, 1996; Crisp, 1997). SMART has been validated against observations of multiple solar system planets (Robinson et al., 2011; Arney et al., 2014). The Line-by-Line Absorption Coefficients (LBLABC) code, a companion to SMART, creates line-by-line absorption files for input gas mixing ratios and temperature-pressure profiles using HITRAN 2012 line lists (Rothman et al., 2013). SMART can also incorporate aerosols: as input, it requires “cloud files” with altitude-dependent opacities as well as the particle asymmetry parameter and the extinction, scattering, and absorption efficiencies (Q_ext, Q_scat, and Q_abs). For spherical particles (our small monomers), we use the code “Miescat,” to calculate these efficiencies using the indices of refraction measured by Khare et al. (1984a). For fractal hydrocarbon particles, we use scattering inputs from the Wolf and Toon (2010) photochemical study generated with the fractal mean field approximation (Botet et al., 1997). Spherical particles use a full Mie phase function, while fractal particles employ a Henyey-Greenstein phase function (Henyey and Greenstein, 1941). To generate transit transmission spectra, we use the SMART-T model (Misra et al., 2014a, 2014b). This version of SMART uses the same inputs as the standard code but simulates the longer path lengths and refraction effects associated with transit transmission observations.

To create SMART cloud files from Atmos haze outputs, we have written a script that bins the haze particles generated by the photochemical model into specified radii (also called particle “modes”) while preserving the total mass of each atmospheric layer. The particle mode sizes we use span from 0.001 to 2 μm; larger particles do not exist in our atmospheres due to rainout. Spherical modes are R = 0.001, 0.005, 0.01, and 0.05 μm. Fractal modes are R = 0.06–2 μm with four modes between 0.06 and 0.1, 10 equally spaced modes between 0.1 and 1 μm, and 2 μm. In total, this represents 19 particle modes.

In each layer of the SMART cloud files, we include a mixture of two particle modes; the mass density contributed by the two modes is selected based on the distance in log space of the Atmos particle radius to each neighboring SMART size bin. For example, if Atmos produces a particle of radius 0.33 μm in a layer, the corresponding layer in SMART will include 0.3 and 0.4 μm particles each comprising 50% of the layer's mass. This binning is necessary because the photochemical model generates many dozens of finely differentiated haze particle radii, but SMART model run time with this many particle sizes is infeasible.

Once we have binned the Atmos particle radii to our SMART size grid, we must compute the total optical depth from each particle mode at a reference wavelength in each atmospheric layer. We arbitrarily select 1 μm as our reference wavelength. Optical depth in a layer, τ, from particles of a given radius, R, depends on the number density of particles per particle size, n(R), the thickness of the atmospheric layer, z, and the wavelength-dependent extinction efficiency, Q_ext:

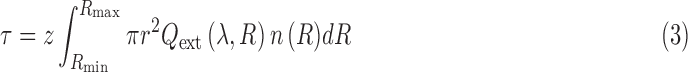For fractal particles (R > 0.05 μm), the cross-sectional area and the corresponding extinction efficiencies are computed relative to the radius of an equal-mass spherical particle, following the conventions of mean-field approximation (Botet et al., 1997). Spherical particles in SMART are binned according to log-normal size distributions using the radii mentioned previously and a mode standard deviation of 1.5, which is realistic for an aerosol distribution (Tolfo, 1977). For fractal particles, we use a monodisperse distribution, the same size distribution used to compute our inputs from the previous Wolf and Toon (2010) fractal haze study and the same distribution used in the Atmos model.

2.3. Model inputs

In the photochemical model, we set a haze monomer density of 0.64 g/cm³, which is consistent with the laboratory results of Trainer et al. (2006) for early Earth. This density is used in the model to calculate the masses of haze particles and is updated from the value of 1 g/cm³ used by previous studies employing our photochemical model. Hörst and Tolbert (2013) measured a similar effective particle density, 0.65 g/cm³, for a 0.1% CH₄ haze experiment using a UV lamp. One-tenth percent CH₄ is consistent with the atmospheres we simulate, although the Hörst and Tolbert hazes were Titan analog simulants lacking the CO₂ present in the Trainer et al. experiments. We apply a Manabe/Wetherald relative humidity model for the troposphere (Manabe and Wetherald, 1967) with a surface relative humidity of 0.8 in both the climate and photochemistry models. This humidity parameterization is further described by Pavlov et al. (2000). Our Archean simulations use the solar constant at 2.7 Ga (0.81 = S/S₀, where S₀ is the modern solar constant and S is the solar constant at 2.7 Ga) modified by a wavelength-dependent solar evolution correction (Claire et al., 2012). We chose this time because it corresponds to the age of the constraints on CO₂ used by our study (Driese et al., 2011). We set the mixing ratio of O₂ at the surface to 1.0 × 10⁻⁸, consistent with the Zerkle et al. (2012) study. These conditions reflect the time period after the evolution of oxygenic photosynthesis but prior to Earth's GOE in which substantial biogenic fluxes of both oxygen and methane would have vented into a predominantly reducing atmosphere (Claire et al., 2014). Unless otherwise specified, the surface albedo used by the climate model is 0.32. This includes the effect of clouds, which is standard in this 1-D treatment (Kopparapu et al., 2013) and is the albedo that reproduces the average temperature of present-day Earth (288 K) with modern atmospheric conditions. Of course, the true cloud distribution on Archean Earth is unknown, and clouds may have had important climatic effects on our early planet (Goldblatt and Zahnle, 2011). The solar zenith angles (SZAs) used in the climate and photochemical models were chosen to best represent globally averaged behavior of the physics in each specific model, which Segura et al. (2003) found as SZA = 45° in the photochemical model and SZA = 60° in the climate model. These zenith angles are both tuned to reproduce modern-day Earth's average chemical profiles and climate, respectively.

For our SMART spectral simulations, our nominal spectra assume an ocean surface albedo (McLinden et al., 1997). In cases where an icy surface is used, we use an albedo from the USGS Digital Spectral Library (Clark et al., 2007). Our solar spectrum was modeled by Chance and Kurucz (2010) and was scaled by the solar evolution model (Claire et al., 2012) mentioned previously. The SZA is set at 60° for the reflection spectra, which approximates a planetary disk average near quadrature (planet half illuminated to the observer).

3. Results

In this section, we first describe the climate results from Atmos. Following this, we quantify the strength of a hazy UV shield for surface organisms, and we show and describe the spectral consequences of this haze in reflected light and transit transmission spectroscopy.

Recent paleosol measurements have constrained the CO₂ partial pressure (pCO₂) in the Archean at 2.7 Ga to be between 0.0036 and 0.018 bar [10–50 × the present atmospheric level (PAL)] (Driese et al., 2011), while recent estimates of Archean surface pressure (P_surf) are consistent with values as low as 0.5 bar (Som et al., 2012; Marty et al., 2013). We simulated four types of atmospheres that span these constraints to examine a range of conditions: pCO₂ = 0.01 and P_surf = 1 bar of total pressure (Case A), pCO₂ = 0.018 and P_surf = 1 bar (Case B), pCO₂ = 0.01 and P_surf = 0.5 bar (Case C), and lastly, pCO₂ = 0.0036 and P_surf = 0.5 bar (Case D). These are summarized in Table 1. The haze thickness scales with the CH₄ abundance relative to CO₂, so we investigated a range of CH₄ levels for each of these atmospheres. In the sections below, we refer to these Case A–D planets. Figure 3 shows an example of the atmospheric profiles for several gases in atmospheres with two different CH₄/CO₂ ratios (0.1 and 0.2), plus the haze number density profiles scaled to fit on the same x axis. The insignificant haze present in the CH₄/CO₂ = 0.1 atmosphere is spectrally indistinguishable from an atmosphere with no haze. The larger amounts of CH₄, C₂H₆, and H₂O at higher altitudes in the CH₄/CO₂ = 0.2 atmosphere illustrate how the haze can shield these gases from photolysis.

Table 1.

Atmosphere Parameters for Cases A–D

	Case A	Case B	Case C	Case D
pCO2 (bar)	0.01	0.018	0.01	0.0036
Psurf (bar)	1	1	0.5	0.5

Our results presented here required about 60 Atmos model runs. In total, we ran about twice this number for model debugging and testing. Each coupled Atmos run can take between 3 and 15 h depending on how many coupling iterations are required. Note that the run time for the climate model scales nonlinearly with the number of radiatively active gases: a model run that takes less than 20 min without CH₄ or C₂H₆ will require well over an hour with both of these gases turned on. All the results presented here, except as noted in Section 4, were generated with both CH₄ and C₂H₆.

Note that in the context of the results presented here, a “thick” haze refers to the haze at a CH₄/CO₂ ratio ∼0.2.

3.1. Hazy climates

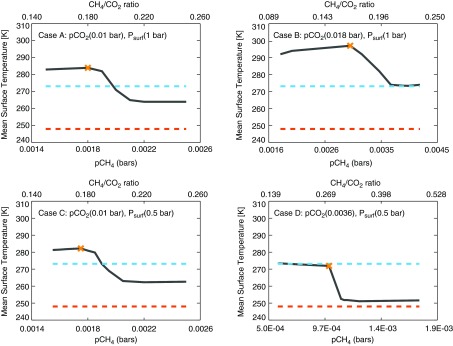
We find that hazy Archean climates were cold but most likely habitable (Fig. 4). Previous 1-D climate modeling efforts assumed that planets with globally averaged surface temperatures (T_GAT, which is equivalent to our 1-D surface temperature, T_surf) below 273 K will experience runaway glaciation (e.g., Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008). However, more recent 3-D studies have shown that Archean Earth can maintain an open ocean fraction of >50% for T_GAT ≥ 260 K and an equatorial open ocean belt for T_GAT ≥ 248 K (Wolf and Toon, 2013; Charnay et al., 2013). Furthermore, Abbot et al. (2011) argued that ocean open belts can remain climatologically stable, even if the ice latitude is reduced to 5–15°. Since a planet with any nonzero fraction of open ocean is habitable, we regard these updated globally averaged temperatures—all of which are significantly below freezing—to be more realistic habitability thresholds than 273 K. We adopt T_GAT ≥ 248 K as our habitability threshold here.

FIG. 4.

Mean surface temperatures as a function of CH₄ for Archean Cases A–D. The dashed blue line shows the freezing point of water (273 K), and the dashed orange line marks our lower threshold of habitability (248 K) for an equatorial ocean belt (Charnay et al., 2013). The X in each panel indicates the initiation of haze-induced cooling.

Figure 4 shows that when haze reaches a threshold thickness, further increases in CH₄ result in rapid increases in haze thickness and a corresponding steep falloff in surface temperature. However, at higher CH₄/CO₂ ratios, the haze thickness (and the surface temperature) stabilizes because UV self-shielding inhibits methane photolysis, shutting down haze formation. Thus, we find there is a maximum haze optical thickness—and a minimum temperature from haze-induced cooling—for each atmosphere. Interestingly, this negative feedback haze self-shielding appears to prevent catastrophic cooling. Note that even using the conventional habitability threshold of 273 K, Cases A–C have a hazy solution space where T_surf > 273 K, and Case B stabilizes at T_surf = 274 K with its thickest haze. By using the updated habitability threshold of T_surf > 248 K, all our cases even with thick hazes are habitable. Table 2 summarizes these results and includes a sensitivity test of the ice-albedo effect, described below.

Table 2.

Temperature Results for Cases A–D

	CH4/CO2 to initiate haze formation	Maximum Tsurfwithout haze (K)	Stabilized Tsurfwith haze (K)	Tsurfwith ice-albedo feedback (K)
Case A	0.18	284	263	257
Case B	0.15	299	274	271
Case C	0.19	282	262	257
Case D	0.28	273	251	241

Although the cold climates we have simulated are “habitable” in the sense that they have open ocean, a cold climate with extended ice caps (T_surf < 273 K) from a thick haze may be consistent with a reported glaciation event at 2.9 Ga (Young et al., 1998) as a previous study has suggested (Domagal-Goldman et al., 2008). Later purported hazy periods around 2.7 Ga (Kurzweil et al., 2013; Izon et al., 2015) and between 2.65 and 2.5 Ga (Zerkle et al., 2012) are not associated with glaciations and may be consistent with the thinner-haze solution space of Cases A and C or even the thickest haze solution space of the warmer Case B.

3.1.1. Ice-albedo feedback

To test how ice-albedo feedbacks can affect our retrieved temperatures, we tested the influence of these feedbacks on the minimum temperatures reached by our four cases by parameterizing our model's 1-D surface albedo (A) by the relation (based on the results of Charnay et al., 2013) to include the albedo effect of clouds and ice as a function of the globally averaged temperature:

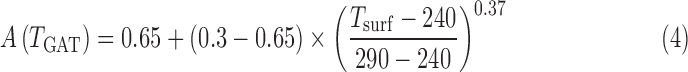As stated above, the surface albedo used by our nominal model is 0.32. The surface albedos for the Case A, B, C, and D minimum temperatures with this ice-albedo parameterization are 0.39, 0.35, 0.39, and 0.45. The climate model was run to convergence starting with the solution for the minimum stabilized temperature for each case (i.e., when the haze becomes self-shielding and reaches maximal thickness) as a test of the sensitivity of our minimum temperatures to ice-albedo feedbacks. The temperatures of planets A, B, C, and D with ice-albedo feedbacks are 257, 271, 257, and 241 K, a decrease of 3–10 K compared with simulations with the nominal albedo. The Bond albedos produced in these cases including haze are 0.26, 0.24, 0.26, and 0.29.

These ice-albedo temperatures may be underestimates because once haze forms, deposition of dark hydrocarbons onto ice-covered areas will lower the albedo of the ice. This decreased ice albedo may then melt the ice, reverting parts of the surface back to ocean water. Because haze absorbs strongly at blue wavelengths, the radiation that reaches the surface under a haze would have a higher proportion of longer, redder wavelengths compared to shorter, bluer wavelengths. While ice is very reflective at visible wavelengths, it becomes more absorbing at wavelengths > 0.7 μm, changing the true ice-albedo parameterization. Because of this, planets orbiting stars emitting a high proportion of radiation at NIR wavelengths are harder to freeze (Shields et al., 2013). Additionally, stratospheric and mesospheric circulation patterns on Earth presently impact high-altitude aerosol distributions by transporting particles preferentially to the poles (Bardeen et al., 2008). In this case, the climatic impact of haze could be reduced with warmer surface temperatures at the equator. On the other hand, hazes can also act as cloud condensation nuclei, enhancing cloud formation (Hasenkopf et al., 2011). This might lead to cooling of the planet or even warming depending on cloud particle size and the altitude—and therefore temperature—of the cloud layer (Goldblatt and Zahnle, 2011). A complete treatment of the impact of ice-albedo feedback, haze deposition, haze circulation, and cloud feedbacks is left to future General Circulation Model (GCM) studies better equipped to deal with these inherently 3-D issues.

3.1.2. Temperature feedbacks on haze production

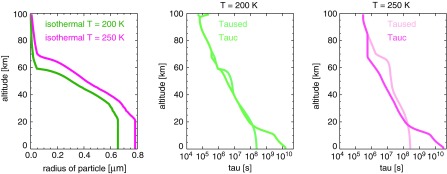
As the haze gets optically thicker, absorption of UV photons produces an atmospheric temperature inversion (Fig. 5) similar to that produced by ozone in the modern atmosphere. We find there is a relationship between the size of the haze particles generated and the temperature of the atmosphere. To isolate the effect, we tested haze production by the photochemical model using two completely isothermal temperature profiles of 200 and 250 K with all other parameters held constant (Fig. 6). The largest particles produced by the 250 K atmosphere have a geometric radius of 0.8 μm compared to 0.65 μm radius particles for the 200 K atmosphere. In the photochemical model, when the coagulation timescale (τ_coag) is shorter than the timescale for removal in an atmospheric layer, the particles can grow. As temperature increases, τ_coag decreases since particles moving faster collide more frequently (Tolfo, 1977). In the hotter atmosphere, τ_coag is smaller than τ_sed through most of the atmospheric column.

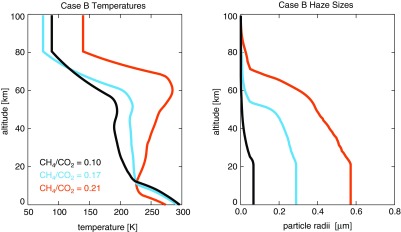
FIG. 5.

The left panel presents the temperature profiles of three CH₄/CO₂ ratios for the Case B planet. Note the strengthening temperature inversion as the CH₄ content of the atmosphere increases. The right panel shows the size of haze particles produced in these three atmospheres, showing the dependence of haze particle size on temperature. From least to most CH₄ (and thinnest to thickest haze), the particles reach a maximum radius of 0.067, 0.28, and 0.57 μm. Note that the temperature profiles become isothermal at the top of the climate model grid when transferred to the larger photochemical model grid.

FIG. 6.

The haze particle sizes for two completely isothermal atmospheres together with the coagulation and sedimentation timescales for these atmospheres.

3.2. UV shielding

The impact of these hazes on the biosphere goes beyond temperature reduction: their fractal nature makes them strong absorbers at short wavelengths and therefore a potential shield against damaging UV radiation for the anoxic Archean (Wolf and Toon, 2010), which would have received significantly more UV at the surface than the planet today (Rugheimer et al., 2015). DNA damage is most acute in the UVC (λ < 0.28 μm) wavelength range (Pierson et al., 1992; Dillon and Castenholz, 1999), but in the modern atmosphere, UVC is fully blocked by O₂ and ozone. For the haze-free Case B atmosphere (CH₄/CO₂ = 0.1), our models calculate the flux of UVC at the surface as about 0.93 W/m² for a SZA of 60° and 2.62 W/m² for SZA = 0°. Both of these values are sufficient for sterilization (Pierson et al., 1992). In contrast, the surface UVC flux under a haze for Case B (CH₄/CO₂ = 0.21) would have been about 0.03 W/m² for SZA = 60° and 0.22 W/m² for SZA = 0°. We compare these values to the tolerances of Chloroflexus aurantiacus (Pierson et al., 1992), a deep-branching, mat-forming anoxygenic phototroph with UV resistance that has been studied as an analogue for Archean phototrophs. Our SZA = 60° flux, 0.03 W/m², is low enough to allow growth of Chloroflexus aurantiacus over the length of a day in the late Archean [about 18–19 h for a day-night cycle; Denis et al. (2002)]. Our SZA = 0° flux, 0.22 W/m², is naturally worse but does not cause immediate sterilization of Chloroflexus aurantiacus, allowing modest growth for roughly 10 h. In a real atmosphere, the UV flux will change with SZA, but it will not exceed the SZA = 0° flux. At latitudes where the SZA is never 0°, UV survival prospects are better, although these higher latitudes may be icy for our cold planets. Under an Archean haze, it is possible that organisms similar to Chloroflexus aurantiacus with robust UV protection mechanisms could have lived at or near the planet's surface. We summarize the UV protection of several types of atmospheres, including ones with water clouds that can confer additional UV protection, in Table 3. This table only includes Case B, but the other cases produce similar results for UV shielding because they have similar optical thicknesses.

Table 3.

The UV Fluxes at the Planetary Surface for Several Overlying Atmospheres

				Case B CH4/CO2 = 0.1			Case B CH4/CO2 = 0.17			Case B CH4/CO2 = 0.2
	Modern Earth	Proterozoic 1% PAL O2	Proterozoic 0.1% PAL O2	H	H + C	H + S	H	H + C	H + S	H	H + C	H + S
SZA = 0°
UVA	70.5	59.1	59.3	55.5	50.84	38.1	48.8	44.3	33.2	22.8	20.2	15.0
UVB	2.49	6.18	10.6	10.2	9.32	7.26	8.11	7.38	5.76	2.19	1.96	1.52
UVC	∼0	0.00764	2.03	2.62	2.41	1.95	1.87	1.71	1.38	0.216	0.196	0.158
SZA = 60°
UVA	28.9	24.4	24.5	23.0	18.42	13.2	17.7	14.4	10.4	4.93	4.14	3.00
UVB	0.446	1.77	3.90	3.82	3.29	2.51	2.51	2.18	1.67	0.337	0.29	0.22
UVC	∼0	7.29 × 10−4	0.565	0.932	0.841	0.673	0.512	0.471	0.376	0.0318	0.0290	0.0252

All values quoted have units of W/m². The solar constant for geological times has been scaled according to Claire et al. (2012) at 2.5 Ga for the Proterozoic and 2.7 Ga for the Archean. All calculations have been performed assuming that the Sun is either directly overhead (SZA = 0°) or at a SZA of 60°. There are three Archean UV fluxes per UV band and CH₄/CO₂ ratio: they refer to haze only (labeled “H”), haze plus cirrus cloud (labeled “H + C”), and haze plus stratocumulus cloud (labeled “H + S”). The Modern Earth and Proterozoic atmospheres are cloud- and haze-free. UVA spans λ = 0.315–0.400 μm. UVB spans λ = 0.280–0.315 μm. UVC is λ < 0.280 μm.

Possibly, an Archean haze aided the survival of life at or near the surface of our early planet. There is evidence that Archean stromatolitic communities lived in inter- and supratidal zones (Allwood et al., 2006; Noffke and Awramik, 2013) experiencing frequent, sometimes extended, exposure to the surface environment, and it has been suggested that microbial mats existed on land as early as 2.7–2.6 Ga (Watanabe et al., 2000). Interestingly, this interval overlaps with periods when haze has been proposed for the Archean atmosphere (Zerkle et al., 2012; Kurzweil et al., 2013; Izon et al., 2015).

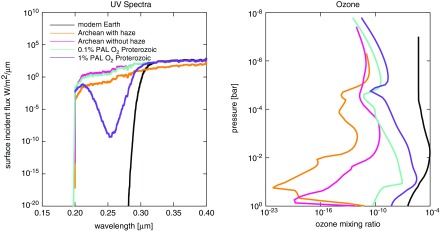
It has widely been assumed that Proterozoic Earth's surface received less UV than the Archean due to the rise of oxygen (O₂) and ozone (e.g., Rugheimer et al., 2015), but a recent study of chromium isotopes suggests that the Proterozoic O₂ mixing ratio was, at most, 0.1% PAL (Planavsky et al., 2014). We tested the strength of an ozone UV shield generated by our photochemical model under these low-oxygen conditions against the strength of our hazy UV shield. For the Proterozoic atmospheres, we tested ozone generation at 0.1% and 1% PAL O₂ levels (Fig. 7) with pCO₂ fixed at 0.01 bar and pCH₄ at 0.0003 bar. Total pressure is set to 1 bar at the surface. According to these assumptions, Proterozoic Earth with 0.1% PAL O₂ would have received 0.57 W/m² of UVC at the surface, so in this case, the Archean hazy UV shield was stronger. Note also that haze is a better shield against UVA (λ = 0.315–0.400 μm) and UVB (λ = 0.280–0.315 μm) than ozone or O₂.

FIG. 7.

Shown are surface UV spectra (left) and ozone column abundances (right) for Archean, Proterozoic, and modern Earth atmospheres. A modest amount of O₂ in the Proterozoic (1% PAL) produces a stronger UV shield than the Archean haze, but the haze shown here cuts out more UVA (320–400 nm) and UVB (280–320 nm) radiation than ozone in all situations. The haze can produce a stronger UV shield compared to the low O₂ atmosphere (0.1% PAL) proposed recently by Planavsky et al. (2014) for our atmospheric assumptions.

3.3. Spectra

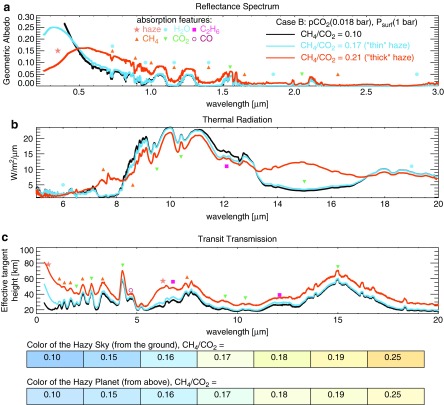
The strong interaction of haze with radiation means hazes can impact the exoplanet spectra that future space-based telescopes will attempt to detect. In Fig. 8, we show reflectance, thermal emission, and transit transmission spectra for our nominal Case B with an ocean surface; the other cases produce similar spectra as discussed below. Our predicted spectra of hazy Archean Earth show diagnostic absorption features from H₂O, CO₂, CH₄, C₂H₆, CO, and from the haze itself. These features are labeled in Fig. 8, and another way to show where these gases and the haze absorb is presented in Figs. 9 and 10 for reflectance and transit transmission spectra, respectively. Figures 9 and 10 were produced by systematically removing each gas or the haze; in places where a given species absorbs, the original spectrum differs from those with the absorbers removed. To consider the spectral effect of haze without contamination from other atmospheric aerosols, the spectra in this section do not include water clouds, even though cloud albedo is implicit in the parameterization of the Atmos model's surface albedo. This makes the albedos of the planets whose spectra are shown in this section darker than those in the Atmos parameterization. However, since clouds have a major impact on the planet's spectral appearance and albedo (e.g., Kitzmann et al., 2011b), we show spectra with water clouds included in Section 3.3.1. The best way to treat the climatic and spectral impact of both clouds and haze would be in a fully coupled 3-D climate-photochemical model that fully considers radiative and photochemical effects of cloud and haze particles, but this is outside the scope of this work. To our knowledge, such a 3-D model does not yet exist, but its development would be useful for the comprehensive treatment of this problem.

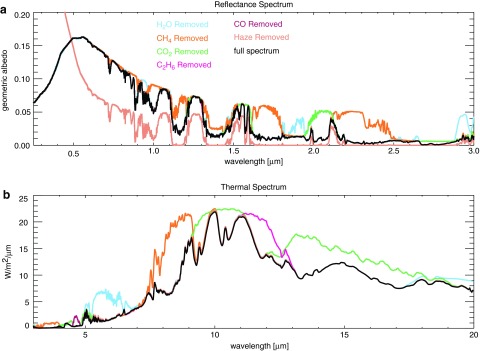
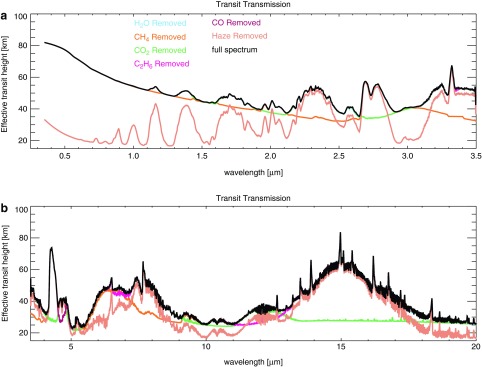
FIG. 8.

Shown here are spectra for Case B. Haze and gas absorption features are labeled with the symbols indicated. (a) At short wavelengths in direct imaging, haze absorption decreases the planet's brightness; scattering brightens the planet at longer wavelengths. (b) Thermal emission from the hot stratosphere of the thickest haze planet (CH₄/CO₂ = 0.21) fills in absorption bands near 8 and 16 μm. (c) The y axis shows the effective transit height above the planet's surface that light is able to penetrate, and absorption features are inverted compared to (a) and (b) due to an increase in the effective planet radius during transit resulting from an increase in absorption at these wavelengths. The bottom section shows the approximate color of the hazy sky and planet. Sky colors are computed using the diffuse radiation spectrum at the ground. “Effective tangent height” refers to the minimum altitude above the planet's surface that light is able to penetrate on transit transmission paths.

FIG. 9.

A reflectance spectrum for a hazy Case B planet in the visible and NIR (a) and mid-IR (b) is presented with gases and the hydrocarbon haze removed to show where each spectral component interacts with radiation. The full spectrum is shown in black. Places where the black spectrum deviates from the colored spectra indicate where each gas or haze absorbs. For example, the green line shows a spectrum where CO₂ is omitted, and a strong CO₂ feature is present near 15 μm in (B) as shown by the deviation of the green spectrum from the black spectrum. At some wavelengths, gas and haze absorptions are complex to detangle because multiple species are absorbing: in these cases, the key on Fig. 8 will indicate which gases are the dominant absorbers in a region.

FIG. 10.

A transit transmission spectrum for a hazy Case B planet in the visible and NIR (a) and mid-IR (b) is presented with gases and the hydrocarbon haze removed to show where each spectral component interacts with radiation. The full spectrum is shown in black. Places where the black spectrum deviates from the colored spectra indicate where each gas or haze absorbs. For example, the orange line in (A) indicates CH₄ absorption features near 1.15, 1.4, 1.7, 2.3, and 3.3 μm.

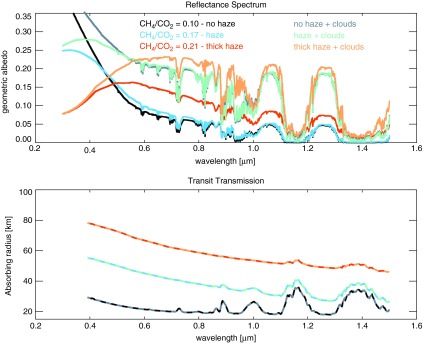
In reflected light (Fig. 8a), the broad UV absorption feature reddens the color of the planet by masking the short-wavelength reflectivity due to Rayleigh scattering. See the bottom section of Fig. 8 for the estimated color of the planet to the eye. The planet colors were calculated using the “Spectral Color Spreadsheet” from brucelindbloom.com with the same method used by Charnay et al. (2015) for GJ 1214b. A spectrum can be input to the calculator, which then outputs RGB values. While these colors should be understood as approximations, we tested the colors produced for the modern Earth sky and Titan as a check, and the results appeared reasonable. Colors and photometric bands have been considered as indicators of Earth-like worlds (Traub, 2003; Crow et al., 2011; Krissansen-Totton et al., 2016), but hazy Archean Earth suggests that not all Earth-like planets will be pale blue dots. Because methane-producing metabolisms evolved early and Earth's atmosphere was anoxic for about a billion years after the origin of life, pale orange dots may proliferate in the Galaxy if other habitable worlds evolve on similar paths to Earth.

Several spectral features are apparent in Fig. 8. The haze-mediated stratospheric thermal inversion is clearly seen in thermal emission near 8 and 16 μm (Fig. 8b). Similar to the Titan transmission spectrum derived from Cassini solar occultation measurements (Robinson et al., 2014b), our simulated hazy transit transmission spectra (Fig. 8c) are sloped in the visible and NIR. Gas absorption features in the visible and NIR are muted by the presence of a haze in transit transmission, but mid-IR absorption features are less affected because the haze is relatively transparent at longer wavelengths. In Earth-like clear-sky atmospheres, the minimum atmospheric altitude transit observations are able to probe will typically be limited by refraction (García Muñoz et al., 2012; Bétrémieux and Kaltenegger, 2014; Misra et al., 2014b), but in hazy atmospheres, haze controls the minimum effective tangent height, especially at shorter wavelengths where it controls the transit transmission spectral slope. Absorption from the haze itself can be seen as the “bump” in the “thick” haze (CH₄/CO₂ = 0.21) transit transmission spectrum at 6 μm, a wavelength region accessible with the James Webb Space Telescope (Wright et al., 2004). There is also a very weak haze feature near 3 μm in transit transmission that can be most easily seen in Fig. 10. These features can also be seen in the peaks of the haze imaginary refractive index (Fig. 2).

Note the presence of a C₂H₆ absorption feature near 12 μm. This C₂H₆ forms from photochemistry involving CH₄, and its buildup in our spectra is not inconsistent with the results of Domagal-Goldman et al. (2011), which showed much greater C₂H₆ accumulation on planets orbiting low-mass stars compared to worlds orbiting the Sun. However, the CH₄ levels in the Domagal-Goldman et al. solar simulations were an order of magnitude lower than the ones shown here. C₂H₆ is a greenhouse gas, and its ability to warm in a CH₄- and haze-rich atmosphere has been discussed previously (Haqq-Misra et al., 2008).

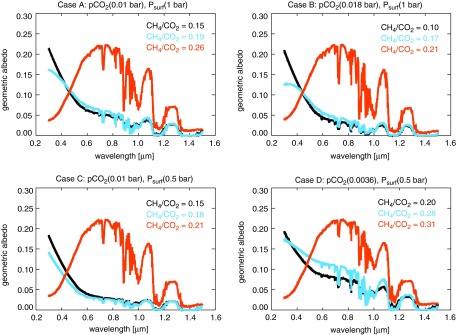
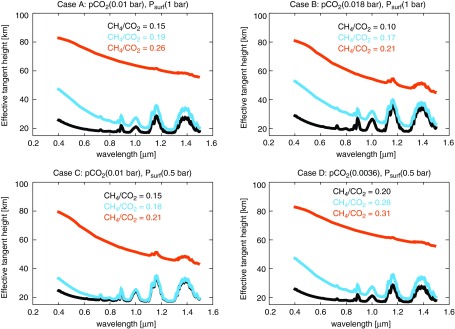
Figures 8–10 showed spectra for our Case B planet, but Figs. 11 and 12 show representative reflected light and transit transmission spectra for all our Cases A–D in the visible and NIR. The reflectance spectra in Figs. 8 and 9 assumed a pure ocean surface albedo to isolate the spectral consequence of atmospheric haze from other spectral changes, but the spectra shown in Fig. 11 are constructed from a weighted average of ocean and ice surfaces according to the ice line latitudes reported by Wolf and Toon (2013) for Archean atmospheres with CO₂ and CH₄. The hazy planets in Fig. 11 are more reflective than the spectra shown in Fig. 8 due to this ice coverage. Figure 12 shows how thick hazes strongly mute the strength of gaseous absorption features in transit transmission at shorter wavelengths where these hazes are more optically thick.

FIG. 11.

Example reflectance spectra, intended as analogues for exoplanets like Archean Earth, for all the types of planets investigated in this study are presented here. Fractional ice coverage is included in these spectra using a weighted average of icy and liquid water surfaces as described in the text.

FIG. 12.

Transit transmission spectra in the visible and NIR for Cases A–D are presented here. For thicker hazes, absorption features shortward of approximately 1 μm vanish. These relatively featureless spectra result because high-altitude hazes are effective at obscuring the lower atmosphere with the long path lengths taken by light in transit spectroscopy measurements.

3.3.1. Water clouds

The goal of the nominal haze spectra we have presented is to show the spectral impact of organic haze independent of any other atmospheric aerosols. However, it is interesting and important to also consider how water clouds affect our hazy spectra. To test the impact of clouds in addition to haze on the spectra of Earth-like planets, we added water clouds to the Case B atmospheres shown in Fig. 8. Because these are 1-D spectra, we incorporate clouds with a weighted average of cloudy and pure haze spectra where we assume 50% of the planet is covered by haze only, 25% is covered by cirrus clouds (at 10 km altitude) and haze, and 25% by stratocumulus clouds (at 1 km altitude) and haze (Robinson et al., 2011). The resulting spectra are presented in Fig. 13. In contrast to hydrocarbon haze particles, which are more transparent in the NIR compared to shorter wavelengths, water vapor clouds have an approximately gray opacity from the visible into the NIR. Thus, at longer wavelengths, cloudy worlds are brighter than their haze-only counterparts. Table 4 shows the total integrated brightness of the reflectance spectra for the worlds with clouds divided by their cloud-free counterparts between 0.4 and 1 μm and between 1 and 2 μm to quantify the spectral impact of clouds.

FIG. 13.

Here we show the impact of water clouds on our Case B spectra with no haze, a thin haze, and a thick haze. The spectra with cloud and haze are shown in the pale colored lines. The dashed lines over our transit transmission spectra indicate that the spectra with and without water clouds are the same.

Table 4.

The Relative Brightness of Spectra with and without Water Clouds

CH4/CO2 =	0.4–1 μm With clouds/No clouds	1–2 μm With clouds/No clouds
0.10	2.34	4.80
0.17	2.12	4.24
0.21	1.56	2.24

The disproportionate increase in brightness from clouds at longer wavelength compared to shorter wavelengths means that the peak of the reflectance spectrum also shifts toward longer wavelengths for the worlds with clouds: for CH₄/CO₂ = 0.17, the reflectance spectrum peak shifts from 0.31 to 0.38 μm, and for CH₄/CO₂ = 0.21, it shifts from 0.56 to 0.68 μm. Adding clouds also raises the spectral continuum level, making absorption features appear deeper. This enhanced reflectivity also potentially increases the detectability of water vapor in reflected light spectra, as more reflected flux from the planet reduces noise on the continuum, enhancing the detectability of absorption features that deviate from that continuum. A detailed discussion of the impact of water clouds on the spectra of Earth-like planets for different cloud altitudes and fractional cloud coverages can be found in the work of Kitzmann et al. (2011b).

In transit transmission, water clouds have no spectral impact because they form in the atmosphere at a level below the maximum tangent height set by refraction. The tropopause on Earth is at roughly 10 km, and refraction prohibits transmission of path lengths below about 20 km even for our clear-sky worlds. As water vapor is at very low abundance in Earth's stratosphere, it would be difficult, in general, to see it in transmission observations that can only probe down to stratospheric altitudes. Abundant stratospheric water vapor would imply that the planet is in the midst of a moist or runaway greenhouse state and thus is not conventionally habitable.

4. Discussion

The hazes investigated here have a major spectral impact at short wavelengths due to their strong blue and UV absorption. It has been suggested that the Rayleigh scattering slope could be used to constrain atmospheric pressure on exoplanets (Benneke and Seager, 2012), but this would not be possible on planets with hydrocarbon hazes due to these strong short-wavelength absorption effects. In reflected light, the haze's broadband UV absorption feature, observed together with methane bands, would strongly imply the existence of hydrocarbon haze in an atmosphere. In the IR, the diagnostic haze absorption feature at 6 μm (and the weaker one at 3 μm) in transit transmission would allow chemical identification of hydrocarbon haze. Even absent the detection of these specific features in transit transmission, the presence of CH₄ bands together with the haze UV-visible-NIR spectral slope would strongly imply the presence of this haze.

4.1. Haze and biology

Our study shows how an Archean haze would have profoundly impacted our planet's environment, habitability, and spectrum. It is important to note that geochemical evidence suggests hazy conditions were not present throughout the entire Archean, and its periodic collapse may have put stress on the biosphere if organisms migrated to the surface or near-surface and adapted to lower UV levels created by the haze. On the other hand, if organisms remained protected by some other UV shield such as minerals, layers of overlying microbial mat, or water (Cockell, 1998), changes in UV radiation levels should not affect them as strongly, so the colder conditions created by the haze might have been the larger source of stress on organisms. These stressors might have driven evolutionary adaptations as life responded to its changing environment. Note that photosynthetic organisms would not likely have been photon limited by the lower light levels under the haze: the lower light limit for red algae is 6 × 10¹⁵ photons/m²/s (Littler et al., 1986). Under our Case B CH₄/CO₂ = 0.21 haze, total PAR at the surface is 7.1 × 10²⁰ photons/m²/s, orders of magnitude above this extreme.

Laboratory experiments on organic haze formation have shown that haze-formation chemistry can involve the formation of important prebiotic molecules such as amino acids and nucleotide bases (Khare et al., 1986; McDonald et al., 1994; DeWitt et al., 2009; Hörst et al., 2012; Trainer, 2013)—see also our discussion of haze formation pathways in Section 4.4. Although the hazy periods we invoke here occurred hundreds of millions of years after the origin of life on Earth, there may be earlier hazy epochs not yet discovered in the geological record [see Kasting (2005) for a discussion of earlier atmospheric methane], and hazy Titan has been regarded as a type of prebiotic chemical laboratory (Khare et al., 1984b; Clarke and Ferris, 1997).

While we know that abiotic hydrocarbon hazes are possible (e.g., on extremely cold worlds like Titan with reducing atmospheres), on a planet like Archean Earth, the presence of hydrocarbon haze may require a higher level of methane production than is possible from abiotic sources alone. The maximum abiotic methane production rate from serpentinization, its primary nonbiological source, has been estimated as 6.8 × 10⁸ and 1.3 × 10⁹ molecules/cm²/s for rocky planets of 1 and 5 Earth masses, respectively (Guzmán-Marmolejo et al., 2013), although there has been earlier speculation of higher abiotic production rates (Kasting, 2005; Shaw, 2008), especially if ancient seafloor spreading rates were faster or the amount of iron-rich ancient seafloor rock was greater. Based on their calculations, Guzmán-Marmolejo et al. (2013) suggested that an atmospheric CH₄ concentration greater than 10 ppmv is suggestive of life. At the range of pCO₂ allowed by Driese et al. (2011), we find that the CH₄ flux needed to initiate haze formation ranges between about 1 × 10¹¹ and 3 × 10¹¹ molecules/cm²/s, broadly consistent with estimates for the biological Archean methane flux after the origin of oxygenic photosynthesis (Kharecha et al., 2005; Claire et al., 2014). The higher of the plausible rocky planet abiotic CH₄ fluxes from Guzmán-Marmolejo et al., 1.3 × 10⁹ molecules/cm²/s, will not form a haze in our model even at a pCO₂ level 4 orders of magnitude smaller than the lower limit allowed by Driese et al. (2011), and such a world would be completely frozen given the Archean solar constant. Remote identification of a hydrocarbon haze with a concurrent measurement of CO₂ around a planet that absorbs an Earth-like amount of radiation could therefore imply a surface methane flux consistent with biological production. The strength and width of the hydrocarbon haze absorption feature below about 0.5 μm implies it would be easier to detect than methane itself given sufficient instrumental sensitivity to this range, so the occurrence of haze in the habitable zone may be a way to flag interesting planets for careful follow-up study that would search for other indicators of life and quantify the concentration of CH₄ and other gases.

4.2. Comparison with other climate studies

To test the robustness of the mean surface temperatures calculated by our computationally efficient 1-D climate model, we compared our temperature result for a haze-free Case A atmosphere with pCO₂ = 0.01 and pCH₄ = 0.002 (but no ethane) and a solar constant for 2.5 billion years ago to the Laboratoire de Météorologie Dynamique (LMD) GCM run with the same inputs. We adopt the same average albedo produced by the LMD model in this simulation, setting A_surf = 0.33 for our planet (as before, this albedo includes the effect of clouds). For an ocean-covered planet with no haze, the LMD model produces a mean surface temperature of 287 K (Charnay et al., 2013). This is comparable to, but 5 K warmer than, our global average 1-D result of 282 K. The Charnay et al. results for the same atmospheric properties but with an equatorial supercontinent result in the same overall planetary albedo (0.33) but a lower mean temperature of 285 K, which is closer to our result. We achieve the closest match to the Charnay et al. results for a modern continental land mass arrangement: in the GCM, this yields an average albedo of 0.34 and a temperature of 283.7 K, close to our result of 281.1 K for this configuration.

We also tested our model results against the Community Atmosphere Model (CAM) GCM nominal Archean atmosphere reported by Wolf and Toon (2013). For this planet, the solar constant is 80% modern, pCO₂ = 0.06 bar, there is no CH₄, no haze, and the planet has an average albedo of 0.317. For this world, the CAM produces a global average surface temperature of 287.9 K. Our model produces 285.3 K for this configuration, a difference of 2.6 K.

The GCMs we compare to can include a variety of effects our 1-D model cannot, including atmospheric circulation, precipitation, cloud formation, and cloud scattering and absorption. Our comparison with these 3-D models suggests the temperatures we present in this work are reasonable but may be underestimates by about 3–5 K. One reason that our 1-D results may be colder than the GCM results is that while we have incorporated identical planetary albedos (with clouds), we are still missing the long-wave radiative forcing from clouds, which would have a warming effect.

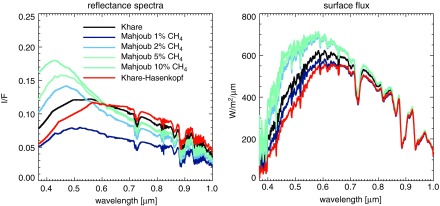
Haqq-Misra et al. (2008) similarly studied the climate of Archean Earth with hydrocarbon hazes and high amounts of CO₂, CH₄, and C₂H₆ with an earlier incarnation of the 1-D models we use here. The haze-free surface temperatures we generate are broadly consistent with the Haqq-Misra et al. nonhazy results with C₂H₆. Haqq-Misra et al. show that a planet with pCO₂ = 0.01 and CH₄/CO₂ = 0.1 has a surface temperature of about 282 K, which is close to our 283.4 K for a comparable atmosphere. Similar to our study, the Haqq-Misra et al. study found it was difficult to maintain surface temperatures above the freezing point of water with spherical haze particles. However, as we have argued, a mean surface temperature of the freezing point of water is not a useful threshold for global habitability (Charnay et al., 2013; Shields et al., 2013; Wolf and Toon, 2013; Kunze et al., 2014), so some of the Haqq-Misra et al. spherical haze results may actually be “habitable.” In general, we are able to achieve warmer hazy solutions in our study because, as previously discussed, fractal hydrocarbon hazes produce less extinction of visible wavelengths compared to equal-mass spherical haze particles. For example, for a planet with 1 bar of pressure and 2% CO₂, the Haqq-Misra et al. spherical haze drops the planet's temperature to below 260 K. The same planet with a fractal haze in our study remains above 273 K (without considering ice-albedo effects not examined by the Haqq-Misra et al. study) after haze self-shielding levels off the temperature. Our results suggest that fractal hazes do indeed produce less antigreenhouse cooling than spherical particles. However, since our nonhazy comparison atmosphere was about 1.4 K warmer than the comparable Haqq-Misra atmosphere, a small component of the warmer temperatures we see here may also result in part from updates to our climate model made by Kopparapu et al. (2013).

4.3. Potential for NH₃ greenhouse gas shielding

The optical thickness of the haze impacts its ability to shield molecules from photodissociation. Once the UV opacity of the haze exceeds approximately unity, the surface flux of CH₄ necessary to maintain a given atmospheric methane mixing ratio drops due to haze-induced CH₄ shielding. At higher haze thicknesses, the opacity of the haze levels off because this self-shielding inhibits the methane photolysis needed to initiate haze formation. Wolf and Toon (2010) commented on the possibility of a fractal hydrocarbon haze shielding ammonia (NH₃) from photolysis, allowing this greenhouse gas to build up in the Archean atmosphere. Following Sagan and Chyba (1997), Wolf and Toon calculated a NH₃ atmospheric lifetime of 7 × 10⁷ years for a solar incident flux at a 45° angle assuming τ ∼ 11 at 200 nm. Following Wolf and Toon, we find our maximum haze thickness levels off at τ ∼ 5 at 200 nm, which results in a significantly shorter NH₃ lifetime of 1 × 10⁴ years, although we did not include NH₃ in our photochemical scheme. Our future work will include NH₃ in the photochemical and climate model to study, in a self-consistent atmosphere, how much of this gas can exist in a hazy atmosphere and what its climatic effect could be.

4.4. Haze formation pathways

Following the mechanism proposed for the formation of Titan's hydrocarbon haze (Allen et al., 1980; Yung et al., 1984), every model of hydrocarbon haze formation in early Earth's atmosphere—including ours—has assumed that aerosol formation will occur through the formation of acetylene (C₂H₂) and its further polymerization to higher polyacetylene chains (Pavlov et al., 2001b; Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008; Zerkle et al., 2012; Kurzweil et al., 2013; Claire et al., 2014). The two reaction pathways described in Section 2.1.1 provide an initial picture of the process, but haze formation is likely considerably more complex and is still not well understood. Unlike early Earth, we now have access to direct observations of the chemical processes ongoing in Titan's atmosphere. In situ measurements by several instruments on board Cassini have found direct evidence for long hydrocarbons and nitriles chains, benzene (C₆H₆) and toluene (C₆H₅CH₃), and indirect evidence for polycyclic aromatic hydrocarbons (PAHs) and nitrogen-containing polycyclic aromatic hydrocarbons (PANHs), indicating that these compounds might play a role in the formation of Titan's hazes (Waite et al., 2007; López-Puertas et al., 2013).

Moreover, early Earth's atmosphere was likely not as reducing as Titan's. The chemical pathways for haze formation, including the C₂H₂ polymerization pathways, may therefore be inappropriate. Early Earth's atmosphere would have contained negligible O₂ but significant amounts of CO₂ (e.g., Kasting, 1993; Driese et al., 2011), whereas Titan's atmosphere is extremely reducing (de Kok et al., 2007). Even in Titan's highly reducing atmosphere, it was suggested that CO may contribute to oxygen incorporation in the organic aerosols (Hörst et al., 2012). This oxygen incorporation is expected to be much more important to aerosol chemistry in early Earth's far less reducing atmosphere. By using far-UV radiation (115–400 nm), organic aerosol production from a CH₄/CO₂/N₂ mixture was shown to exceed that from a pure CH₄/N₂ mixture (Trainer et al., 2006), and organic aerosol formation was experimentally observed to occur down to C/O ratios as low as 0.1 (Trainer et al., 2006; DeWitt et al., 2009). From the chemical analysis of primary condensed-phase products of photochemistry, it is clear that the composition of the aerosol analogues formed in early Earth-like atmospheres with C/O < 1 differs greatly from the aerosol analogues formed in Titan-like atmospheres where C/O >> 1. Instead of limiting the formation of organic molecules as initially predicted, the O-atoms released from CO₂ photolysis are incorporated into the molecular structure of the organic aerosols. Mass spectrometry of aerosol analogues formed with C/O = 0.1 indicates the formation of carbonyl and carboxyl groups rather than aromatic cycles and long-aliphatic chains, and even suggests the formation of organic acids such as succinic acid (C₄H₆O₄) (DeWitt et al., 2009).

Finally, haze formation chemistry gets considerably more complex when one considers the coexistence not only of O-heteroatoms but also of N-heteroatoms in aerosol organics. Nitrogen incorporation was recently observed in the aerosols generated by far-UV photolysis of CH₄/CO₂/N₂ gas mixtures (Trainer, 2013) and in CH₄/N₂ mixtures (Sebree et al., 2015). These results bring to light a significant but still unknown mechanism regarding the activation of nitrogen and its inclusion in oxygenated organics, thus providing a new and quantifiable source for these two elements into the early Earth aerosols. Studies have shown the formation of HCN, CH₃CN, and other nitrile gas species are formed using the same type of UV source in a CH₄/N₂ gas mixture, thus corroborating the indirect nitrogen photochemistry (Trainer et al., 2012; Yoon et al., 2014). These results suggest that N₂ chemical activation could be due to its reaction with the methylidyne (CH) radical formed from CH₄ photolysis, to form two radical intermediates, diazomethyl HCNN and its isomer HNCN, which might then react to form HCN and other products.

The formation of aerosols in early Earth's atmosphere is thus tightly intertwined with the formation of organic molecules containing more than a few C/H/N/O atoms. These compositional differences should change the properties of the aerosol material sufficiently to be able to distinguish a hazy early Earth from a modern-day Titan (Hasenkopf et al., 2010). For instance, organic molecules with oxygen-containing functional groups (alcohols, carbonyls) tend to have stronger absorbances at longer UV wavelengths as compared to similar hydrocarbon molecules (Workman, 2000). The NIR absorption bands of the Archean aerosol analogues would also shift in response to the inclusion of the types of oxygen and nitrogen heteroatom functionalities that have been indicated in the compositional studies.

4.5. Optical constants

The implications of compositional differences of Archean hazes versus titanian hazes for the topics presented in this study and for prebiotic chemistry underscore the need for measurements of Archean Earth analog optical constants as well as a better understanding of the haze formation chemical pathways. Unfortunately, only one study, that of Hasenkopf et al. (2010), has measured an Archean Earth haze refractive index (as opposed to a titanian haze), and this was only done at a single wavelength (532 nm).

In our study, we have used the hydrocarbon refractive indices from the work of Khare et al. (1984a) to allow us to draw comparisons with previous works involving our suite of models (Pavlov et al., 2001b; Domagal-Goldman et al., 2008; Haqq-Misra et al., 2008; Zerkle et al., 2012; Kurzweil et al., 2013; Claire et al., 2014), as well as the Wolf and Toon (2010) study, which all used the Khare optical constants. An additional advantage of the Khare refractive indices is that they span an extremely wide wavelength range, ranging from 0.02 to 920 μm, so only one set of optical constants is needed to cover all the wavelengths relevant to photochemistry, climate, and spectra.

However, more recent measurements of hydrocarbon refractive indices over more restricted wavelength ranges indicate disagreement with the Khare measurements (Ramirez et al., 2002; Tran et al., 2003; Vuitton et al., 2009; Hasenkopf et al., 2010; Imanaka et al., 2012; Mahjoub et al., 2012; Sciamma-O'Brien et al., 2012), although these measurements themselves show considerable variation among each other (Fig. 14). Differences in the composition of Archean hazes compared to Titan's (and thus differences in their optical constants) are expected as discussed in Section 4.4. Again, note the single measurement by Hasenkopf et al. (2010) for an Archean analog haze; of all the optical constants plotted in Fig. 14, the Khare indices actually produce the closest (although still too low) match to the Hasenkopf Archean real refractive index (n) near 532 nm and produce a reasonable match to the Hasenkopf Archean imaginary refractive index (k), agreeing to within approximately 40% near 532 nm.

FIG. 14.

This shows the diversity of optical constants measured by several studies. The studies the figure key refers to are as follows: Hasenkopf et al., 2010; Ramirez et al., 2002; Sciamma-O'Brien et al., 2012; Khare et al., 1984a; Imanaka et al., 2012; Tran et al., 2003; Mahjoub et al., 2012; Vuitton et al., 2009. Note in particular the single point measured under Archean Earth-like laboratory conditions by Hasenkopf et al. (2010).

As an example and test of the impact different refractive indices have on our spectra, we examined the sensitivity of our nominal spectra to varied refractive indices measured by Mahjoub et al. (2012). The Mahjoub study tested the impact of methane concentration in the gas phase on the resultant hydrocarbon optical properties with gas mixtures containing 1%, 2%, 5%, and 10% CH₄ in CH₄-N₂ mixtures. Note the 1% CH₄ Mahjoub imaginary refractive index agrees to within 5% of the Hasenkopf Archean measurement near 532 nm. Mahjoub et al. found that refractive indices have a strong dependency on the CH₄ concentration over 0.37–1 μm: results indicate that the imaginary index of refraction (k) decreases with increasing CH₄ concentration, and the real index of refraction (n) increases with CH₄ for the compositions tested. We generated the spectra shown in Fig. 15 by producing new fractal input files using the Mahjoub optical constants. These files were then used to replace the Khare files in our SMART inputs for the nominal CH₄/CO₂ = 0.21 Case B spectrum. In addition, we generated a spectrum to test the Hasenkopf Archean haze measurement by applying a scaling factor to the Khare optical constants to match the Hasenkopf n and k values at 532 nm. This spectrum is called “Khare-Hasenkopf” in the Fig. 15 caption. Of course this does not account for differences expected in the spectral shape of Archean haze analogues across the UV-visible-IR relative to the titanian haze analogues.

FIG. 15.

A comparison of reflectance spectra and surface flux spectra using Khare et al. (1984a) and Mahjoub et al. (2012) optical constants, plus a spectrum generated by shifting the Khare constants to match the Archean haze refractive indices measured by Hasenkopf et al. (2010) at 532 nm (called “Khare-Hasenkopf”).

Besides affecting the top-of-atmosphere spectrum, these different optical constants alter how much radiation can reach the surface under a haze. We find that, for the Mahjoub 1%, 2%, 5%, and 10% CH₄ optical constants, 0.92, 1.11, 1.13, and 1.16 times the nominal (Khare) total integrated 0.37–1 μm flux reaches the surface of the planet. For the Khare-Hasenkopf spectrum, which shifts both the real and imaginary refractive indices to larger values, this drops to 0.89 times the nominal flux. The Mahjoub constants do not extend shortward of 0.37 μm, but we should anticipate variation at these shorter wavelengths as well.

The variation in surface-incident flux shows us that we should expect differences in the hazy Archean climates we calculate depending on the optical constants used. We tested how the Hasenkopf Archean measurement might impact the climate for a pCO₂ = 0.01 and CH₄/CO₂ = 0.2 atmosphere. The nominal Khare constants produce a surface temperature of 272 K for this atmosphere. The “Khare-Hasenkopf” optical constants yield a cooler temperature of 267 K, which is expected because these optical constants produce a haze with more efficient scattering and absorption. This difference in temperature is smaller than the difference of using spherical versus fractal particles: our comparison to the Haqq-Misra et al. (2008) study in Section 4.2 shows that particle shape can result in temperature differences >10 K. A full treatment of the impact of varied optical constants using the coupled photochemical-climate model to generate new self-consistent atmospheres and climates is outside the scope of our present study.

Updated haze optical constants generated under Archean Earth-like laboratory conditions (rather than Titan-like conditions) to produce plausible Archean analog haze compositions would be of immense value to future studies of organic hazes in Earth-like atmospheres, including exoplanets, and would allow updates of the results presented in this study. Due to the properties of fractal hazes, these particles are relatively transparent at wavelengths longer than approximately the visible range, so measurements of refractive indices at visible wavelengths in particular would allow us to improve our estimates of the climatic impacts of this haze. In addition, better constraints on Archean UV refractive indices would allow us to better quantify how good a UV shield these hazes actually are.

5. Conclusions

We have shown that a hazy Archean Earth consistent with geochemical constraints on CO₂ concentration and geological constraints on surface pressure could have had habitable surface temperatures. Although the fractal hazes simulated here cool the planet by up to ∼20 K, these fractal particles produce significantly less cooling than a haze of equivalent-mass spherical particles. The climatic effects of this haze could have been part of feedbacks between biological CH₄ production, atmospheric chemistry, and surface UV radiation. Haze can cut down the surface-incident UVC radiation on Archean Earth from ∼0.9 W/m² to ∼0.03 W/m² for a SZA of 60°, and may have allowed survival of otherwise unshielded life at the surface of our Archean planet. The presence of similar hydrocarbon haze on an exoplanet could be observed, as demonstrated by strong features present in synthetic spectra of these worlds. For habitable exoplanets similar to Archean Earth, hydrocarbon haze may be strongly biologically mediated and serve as a novel non-gaseous biosignature with a strong spectral signature. Discovering habitable exoplanets dissimilar to modern Earth will increase the diversity of known habitable environments. Leveraging our understanding of Earth's history provides us with a variety of analogues with which we can expand our expectations for the “Earth-like” planets beyond our solar system; future observations of such worlds can provide us with a window into the evolution of terrestrial worlds like our home.

Abbreviations List

CAM

Community Atmosphere Model

GCM

General Circulation Model

GOE

Great Oxygenation Event

LMD

Laboratoire de Météorologie Dynamique

MDF

mass-dependent fractionation

MIF

mass-independent fractionation

NIR

near infrared

PAL

present atmospheric level

SMART

Spectral Mapping Atmospheric Radiative Transfer

SZA

solar zenith angle

Acknowledgments

This work was performed as part of the NASA Astrobiology Institute's Virtual Planetary Laboratory, supported by the National Aeronautics and Space Administration through the NASA Astrobiology Institute under solicitation NNH12ZDA002C and Cooperative Agreement Number NNA13AA93A. G. Arney was supported in part by the NASA Astrobiology Institute Early Career Collaboration Award. E.T. Wolf acknowledges NASA Planetary Atmospheres Program award NNH13ZDA001N-PATM and NASA Exobiology Program award NNX10AR17G for financial support. B. Charnay acknowledges support from an appointment to the NASA Postdoctoral Program, administered by Universities Space Research Association. E. Hébrard was supported by an appointment to the NASA Postdoctoral Program at NASA Goddard Space Flight Center, administered by Universities Space Research Association through a contract with NASA. Simulations were facilitated through the use of the Hyak supercomputer system at the University of Washington eScience Institute. We are grateful to C. McKay and three other anonymous reviewers whose comments substantially improved the quality of our manuscript. We thank R. Buick, D. Crisp, N. Kiang, and M. Parenteau for conversations and advice. Spectra shown in this work will be archived at the Virtual Planetary Laboratory online spectral database.

Author Disclosure Statement

No competing financial interests exist.